The entropic penalty for associative reactions and their physical treatment during routine computations

Abstract

A systematic study of the entropic penalty for associative reactions is presented. It is shown that computed solution-phase Gibbs free energies typically overestimate entropic contributions. This entropic penalty for associative reactions in solution, i.e., if the number of particles decreases along the reaction coordinate (sum of stoichiometric numbers 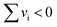), originates from the insufficient treatment of entropic effects by implicit solvent models. We propose an additive correction scheme to Gibbs free energies that is suitable for routine applications by non-expert users. This correction is based on Garza's formalism for the solution-phase entropy [A. J. Garza, J. Chem. Theory Comput., 2019, 15, 3204.] that is physically sound and embedded into an efficient black-box type algorithm. To critically evaluate the entropic penalty and its proposed treatment, we compiled an experimental benchmark set of 31 Δ_rG and 22 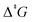in 15 different solvents. Using a representative best-practice computational protocol (at wave function theory (WFT) based DLPNO-CCSD(T) and density functional theory (DFT) based revDSD-PBEP86-D4 level with an implicit solvent model), we determined a sizeable entropic penalty ranging from 2–11 kcal mol⁻¹. Using the correction scheme presented herein, the entropic penalty is corrected to the chemical accuracy of ≤1 kcal mol⁻¹ (WFT and DFT). The same applies to 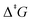at the WFT level. Barriers at the DFT level are overestimated by 2 kcal mol⁻¹ (classic) and underestimated by 2 kcal mol⁻¹ (corrected). This effect is attributed to the finding that barriers computed at the DFT level are systematically 2–3 kcal mol⁻¹ lower than barriers obtained with WFT.