Percolation threshold and electrical conductivity of conductive polymer composites filled with curved fibers in two-dimensional space

Abstract

Quantifying the influence of fiber curvature on the percolation behavior of flexible conductive fiber and further on the electrical conductivity of conductive polymer composites (CPCs) is crucial for the design of CPCs. This study considers CPCs as a random packing of soft curved discorectangles (CDCRs) in a polymer matrix. The geometry of CDCR is developed, and an inter-CDCR contact detection algorithm is used to generate a random packing structure of CDCRs. The effects of aspect ratio α and bending central angles θ of CDCR on the percolation threshold ϕ_c of the overlapped CDCR system in a two-dimensional plane are then investigated using the finite-size scaling method. The result reveals that ϕ_c decreases monotonically as α grows and increases monotonically as θ rises. A shape-independent power law formula, denoted as ϕ_c = 2.2015 A^−0.8172_dex is developed to quantify the relationship between the A_dex and ϕ_c. A comparison of our numerical simulations, published data, and predictions verifies the reliability and universality of the fitting model. Subsequently, a resistor network searching algorithm (RNSA) is proposed to construct the random resistor network model (RRNM). A power law model, denoted as 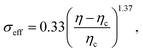is developed to evaluate the effects of the normalized reduced density (η − η_c)/η_c on the effective conductivity σ_eff of CPC. Comparing our predictions with data from the literature and our simulation verifies the reliability of our RNSA and the fitting model. This paper's methodology and findings may provide a theoretical hint for the CPC's design.