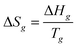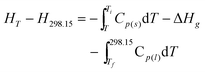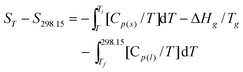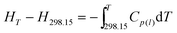Low-temperature heat capacities of 1-alkyl-3-methylimidazolium bis(oxalato)borate ionic liquids and the influence of anion structural characteristics on thermodynamic properties†
Received
7th September 2010
, Accepted 8th October 2010
First published on 23rd November 2010
Abstract
Two chelated orthoborate ionic liquids (ILs), 1-butyl-3-methylimidazolium bis(oxalato)borate ([Bmim][BOB]) and 1-hexyl-3-methylimidazolium bis(oxalato)borate ([Hmim][BOB]), were prepared and characterized. Their thermodynamic properties were studied using adiabatic calorimetry and differential scanning calorimetry (DSC). The thermodynamic properties of the two ILs were evaluated and compared with each other, and then with those of other [Bmim] type ILs. The results clearly indicate that for a given cation (or anion) and at a certain temperature, the more atoms in the anion (or cation), the higher the heat capacity; the higher glass-transition temperatures of [BOB] type ILs than others are mainly caused by the higher symmetry of the orthoborate anion structure. It is suggested that a high content of strong electronegative atoms and Cn or Cnv (n = 1,2,3,…,∞) point group symmetry in the anion are favorable for the design and synthesis of room temperature ILs with a wide liquid range.
1. Introduction
Ionic liquids (ILs) are very promising replacements for traditional volatile organic solvents because of their unique physicochemical properties, such as low vapor pressure, non-combustibility and good solubility.1,2 Another attractive advantage of ILs is that they are designable.3,4 The cation and anion of ILs can be varied and combined in nearly infinite ways which lead to a quite large number of different compounds with unique desirable properties. Correspondingly, the properties of ILs including viscosity, solubility and melting point etc. could be adjusted. Therefore, understanding the relationship between the basic physical properties and the molecular structure characteristics of ILs is one of key issues for ILs' design, evaluation and successful use.
Although much effort has been made on the synthesis and application of ILs in various areas such as catalysis, electrochemistry and separation sciences, the research work on their physicochemical properties, especially thermodynamic properties is still scanty.5Imidazolium based ILs as one of the most popular classes has been widely investigated, and for some of them, the thermodynamic properties are available.6–12 In 2000, A. B. McEwen et al. investigated the thermal properties of several imidazolium based ILs by using DSC and TGA/SDTA.10 After that, J. F. Brennecke et al. reported the densities, melting temperatures, glass-transition temperatures, decomposition temperatures and heat capacities for a series of 13 imidazolium based ILs.11 It is found that the properties of imidazolium based ILs follow quite reasonable trends which can help to design and synthesize more useful and task-specific ILs. Our research group has paid much attention to the fundamental thermodynamic investigation of ILs, and the heat capacities, standard enthalpies of formation and thermodynamic functions of some ILs have been investigated.13–16 Recently, the ILs based on various chelated orthoborate anions attracted much attention because of their potential electrochemistry and enantiomeric recognition application.17,18 Moreover, they are interesting solvents for large-molecule dissolution and a good model system for a fundamental liquid-state study.19 Very recently, we have also successfully achieved a new extra-large microporous borophosphate through the use of chelated orthoborate ionic liquids as a special boron source.20
As a continuation of the research work in our group on the thermodynamic properties of ILs, we prepared and characterized two chelated orthoborate ionic liquids, 1-butyl-3-methylimidazolium bis(oxalato)borate ([Bmim][BOB], CAS No. 566135-35-1) and 1-hexyl-3-methylimidazolium bis(oxalato)borate ([Hmim][BOB]). Their thermodynamic properties were studied by using adiabatic calorimetry and differential scanning calorimetry (DSC). The phase change behavior and thermodynamic properties were evaluated and compared with other imidazolium based ILs.
2. Experimental section
2.1 Materials
All reagents were purchased from commercial suppliers. 1-Methylimidazole (Industrial grade, Linhai Kaile chemical factory, China), 1-bromobutane (AR grade, Sinopharm Chemical Regent Co., China) and 1-bromohexane (AR grade, Sinopharm Chemical Regent Co., China) were distilled before use. Lithium bis(oxalato)borate (LiBOB) (Premium Battery grade, Chemetall GmbH, Germany), acetonitrile (AR grade, Tianjin Fengchuan Chemical Reagent Co., China) and dichloromethane (AR grade, Beijing Chemical Reagent Co., China) with purities ≥99.5% were used in the synthesis process without further purification.
2.2 Preparation and characterization of [Bmim][BOB] and [Hmim][BOB] ILs
1-Methylimidazole (1 mol) was reacted with an excess of 1-bromobutane or 1-bromohexane (1.1 mol) in a round-bottomed flask under a nitrogen atmosphere at 70 °C for two days to prepare [Bmim][Br] or [Hmim][Br]. The product [Bmim][Br] was purified by recrystallization using acetonitrile as a solvent several times, and [Hmim]Br was purified by passing an active carbon and Al2O3 packed column. For preparation of chelated orthoborate ILs, an equal molar amount of [Bmim][Br] or [Hmim][Br] and LiBOB were used to conduct to an anion metathesis procedure as described elsewhere.19 The insolubility of lithium bromide in dichloromethane is sufficient enough to purify the orthoborate ILs by solvent extraction. After filtering of the LiBr, the dichloromethane phase containing the final product was extracted by water several times until the test for trace of bromide using an AgNO3 solution showed no more cloudiness. Dichloromethane was removed under reduced pressure by using a rotary evaporator, and the product was finally dried under high vacuum at 70 °C for 48 h. The reaction scheme was shown in Scheme 1.
![Preparation scheme of [Bmim]/[Hmim][BOB] ILs.](/image/article/2011/CP/c0cp01744b/c0cp01744b-s1.gif) |
| | Scheme 1 Preparation scheme of [Bmim]/[Hmim][BOB] ILs. | |
The bromide and orthoborate products were characterized by a Bruker DRX 400 NMR spectrometer, and the 1H, 13C and 11B NMR spectra of orthoborate ILs were presented in Fig. S1–S6 in the ESI.† Their corresponding chemical shifts were as following:
[Bmim][BOB]: NMR in DMSO-d6: 1H (400 MHz) δ = 9.102 (s, 1H), 7.761 (s, 1H), 7.697 (s, 1H), 4.192–4.156 (q, 2H, ΔJ = 7.2 Hz), 3.867 (s, 3H), 1.816–1.742 (m, 2H, ΔJ = 7.2 Hz), 1.320–1.227 (m, 2H, ΔJ = 7.2 Hz), 0.926–0.889 ppm (t, 3H, ΔJ = 7.2 Hz). 13C (100.6 MHz) δ = 158.228, 136.538, 123.645, 122.280, 48.556, 35.778, 31.385, 18.802, 13.255 ppm. 11B (128.3 MHz) δ =7.591 ppm, with H3BO3 as external.
[Hmim][BOB]: NMR in DMSO-d6: 1H (400 MHz) δ = 9.101 (s, 1H), 7.725 (s, 1H), 7.664 (s, 1H), 4.213–4.176 (t, 2H, ΔJ = 7.4 Hz), 3.907 (s, 3H), 1.831–1.798 (m, 2H, ΔJ = 6.8 Hz), 1.266 (s, 3H), 0.831 ppm (s, 3H). 13C (100.6 MHz) δ = 158.386, 136.605, 123.619, 122.241, 49.015, 35.806, 30.599, 29.467, 25.242, 21.900, 13.666 ppm. 11B (128.3 MHz) δ = 7.608 ppm, with H3BO3 as external.
Heat capacity measurements were carried out in a high-precision automated adiabatic calorimeter described in detail elsewhere.21–23 To verify the reliability of the adiabatic calorimeter, the molar heat capacities for Standard Reference Material 720, Synthetic Sapphire (α-Al2O3) were measured. The deviations of our experimental results from the recommended values by NIST24 were within ±0.1% in the temperature range of 80–400 K.
The DSC measurements were carried out using a Netzsch differential scanning calorimeter (model: DSC 204) under N2 with a flow rate of 25 ml min−1. The samples were first cooled with a rate of 10 K min−1 from 300 K to 173 K, and then heated with the same rate from 173 K to 290 K.
3. Results and discussion
3.1 Low-temperature molar heat capacity
The experimental molar heat capacities of two ILs measured using an adiabatic calorimeter over the experimental temperature range (from 82 to 398 K) are listed in Table 1 and shown in Fig. 1. As seen in Fig. 1, glass transition behavior was obviously observed from 230 to 244 K for [Bmim][BOB], and from 230 to 241 K for [Hmim][BOB]. No other thermal anomaly or phase transition took place in the solid phases and liquid phases for both of them.
![Experimental molar heat capacity Cp,m of [Bmim][BOB] and [Hmim][BOB] as a function of temperature.](/image/article/2011/CP/c0cp01744b/c0cp01744b-f1.gif) |
| | Fig. 1 Experimental molar heat capacity Cp,m of [Bmim][BOB] and [Hmim][BOB] as a function of temperature. | |
Table 1 Experimental molar heat capacities of [Bmim][BOB] and [Hmim][BOB]
|
T/K |
C
p,m/J K−1 mol−1 |
T/K |
C
p,m/J K−1 mol−1 |
T/K |
C
p,m/J K−1 mol−1 |
|
[Bmim][BOB]
|
| 82.260 |
162.68 |
187.494 |
291.06 |
294.720 |
551.30 |
| 84.253 |
164.80 |
189.427 |
293.99 |
296.704 |
548.95 |
| 86.175 |
167.39 |
191.350 |
296.21 |
298.689 |
549.07 |
| 88.114 |
169.92 |
193.303 |
298.74 |
300.668 |
550.11 |
| 90.038 |
172.86 |
195.291 |
300.98 |
302.649 |
550.60 |
| 91.957 |
175.54 |
197.267 |
303.12 |
304.624 |
550.99 |
| 93.888 |
178.05 |
199.233 |
305.52 |
306.599 |
551.46 |
| 95.810 |
180.61 |
201.627 |
309.09 |
308.573 |
552.38 |
| 97.724 |
182.92 |
204.037 |
312.02 |
310.543 |
552.45 |
| 99.653 |
185.50 |
206.020 |
315.00 |
312.509 |
553.28 |
| 102.331 |
189.45 |
208.000 |
317.55 |
314.473 |
555.34 |
| 104.994 |
193.24 |
209.971 |
320.03 |
316.435 |
556.40 |
| 106.920 |
195.26 |
211.938 |
321.68 |
318.394 |
557.61 |
| 108.859 |
197.53 |
213.896 |
323.98 |
320.352 |
557.15 |
| 110.775 |
199.69 |
215.852 |
326.20 |
322.303 |
558.16 |
| 112.702 |
202.09 |
217.801 |
329.42 |
324.256 |
559.28 |
| 114.642 |
204.87 |
219.806 |
332.92 |
326.204 |
560.50 |
| 116.562 |
207.20 |
221.863 |
335.96 |
328.152 |
560.98 |
| 118.493 |
209.88 |
223.919 |
339.45 |
330.096 |
561.78 |
| 120.441 |
211.92 |
225.971 |
342.57 |
332.092 |
563.39 |
| 122.365 |
214.00 |
228.020 |
345.96 |
334.140 |
564.45 |
| 124.304 |
216.71 |
230.056 |
350.39 |
336.185 |
564.63 |
| 126.259 |
218.92 |
232.074 |
361.82 |
338.224 |
566.77 |
| 128.193 |
220.92 |
234.141 |
361.55 |
340.266 |
568.03 |
| 130.108 |
223.20 |
236.241 |
370.61 |
342.302 |
568.32 |
| 132.039 |
225.31 |
238.241 |
394.03 |
344.335 |
569.25 |
| 133.982 |
227.87 |
240.030 |
473.56 |
346.365 |
570.84 |
| 135.911 |
230.25 |
242.083 |
525.22 |
348.392 |
571.96 |
| 137.852 |
232.95 |
244.303 |
528.69 |
350.415 |
572.99 |
| 139.814 |
235.14 |
246.290 |
528.83 |
352.437 |
573.08 |
| 141.756 |
235.90 |
248.334 |
528.83 |
354.455 |
573.61 |
| 143.679 |
238.11 |
250.372 |
529.19 |
356.471 |
574.41 |
| 145.590 |
241.90 |
252.415 |
528.85 |
358.482 |
576.20 |
| 147.521 |
244.43 |
254.450 |
529.21 |
360.492 |
578.27 |
| 149.463 |
246.62 |
256.486 |
530.58 |
362.499 |
579.53 |
| 151.952 |
248.86 |
258.520 |
531.80 |
364.503 |
579.76 |
| 154.404 |
251.85 |
260.553 |
531.94 |
366.499 |
580.19 |
| 156.317 |
254.53 |
262.579 |
533.59 |
368.490 |
582.08 |
| 158.267 |
256.13 |
264.608 |
534.57 |
370.480 |
582.13 |
| 160.198 |
258.45 |
266.632 |
534.39 |
372.463 |
583.83 |
| 162.118 |
261.49 |
268.653 |
536.90 |
374.456 |
585.44 |
| 164.072 |
264.24 |
270.677 |
537.26 |
376.445 |
582.92 |
| 166.057 |
266.60 |
272.694 |
537.44 |
378.428 |
585.20 |
| 168.031 |
267.94 |
274.709 |
538.78 |
380.417 |
587.00 |
| 169.988 |
270.01 |
276.721 |
539.56 |
382.395 |
587.98 |
| 171.937 |
272.91 |
278.733 |
540.89 |
384.374 |
589.30 |
| 173.874 |
275.19 |
280.744 |
542.47 |
386.346 |
588.94 |
| 175.798 |
276.91 |
282.758 |
542.58 |
388.317 |
590.79 |
| 177.710 |
279.16 |
284.767 |
542.56 |
390.289 |
592.57 |
| 179.656 |
281.05 |
286.744 |
556.25 |
392.256 |
593.07 |
| 181.633 |
283.48 |
288.759 |
544.97 |
394.216 |
591.44 |
| 183.597 |
286.34 |
290.758 |
549.07 |
396.203 |
596.40 |
| 185.552 |
288.38 |
292.740 |
551.92 |
398.239 |
598.12 |
|
[Hmim][BOB]
|
| 83.600 |
182.21 |
189.062 |
322.00 |
295.453 |
599.83 |
| 85.596 |
183.90 |
191.025 |
324.67 |
297.481 |
597.88 |
| 87.516 |
186.71 |
192.971 |
326.98 |
299.507 |
598.99 |
| 89.457 |
189.85 |
194.912 |
327.94 |
301.535 |
596.30 |
| 91.381 |
193.51 |
196.837 |
330.20 |
303.549 |
600.05 |
| 93.300 |
196.77 |
198.798 |
335.70 |
305.575 |
602.38 |
| 95.230 |
199.44 |
200.798 |
338.30 |
307.594 |
602.99 |
| 97.152 |
202.40 |
203.219 |
340.82 |
309.613 |
601.75 |
| 99.065 |
205.16 |
205.598 |
344.23 |
311.626 |
602.02 |
| 101.761 |
208.48 |
207.552 |
346.98 |
313.636 |
604.40 |
| 104.455 |
212.11 |
209.557 |
350.94 |
315.649 |
604.33 |
| 106.367 |
214.75 |
211.562 |
352.93 |
317.655 |
603.88 |
| 108.290 |
217.82 |
213.555 |
354.70 |
319.658 |
606.29 |
| 110.230 |
219.95 |
215.545 |
358.27 |
321.610 |
640.72 |
| 112.140 |
223.45 |
217.532 |
362.00 |
323.726 |
617.23 |
| 114.069 |
227.71 |
219.513 |
365.99 |
325.774 |
609.87 |
| 116.013 |
229.97 |
221.547 |
370.10 |
327.761 |
612.50 |
| 117.933 |
231.26 |
223.636 |
373.98 |
329.752 |
616.75 |
| 119.867 |
233.90 |
225.718 |
380.16 |
331.740 |
616.17 |
| 121.815 |
236.46 |
227.796 |
385.72 |
333.722 |
616.98 |
| 123.744 |
238.84 |
229.856 |
391.45 |
335.705 |
618.71 |
| 125.651 |
241.78 |
231.870 |
409.86 |
337.684 |
619.09 |
| 127.577 |
243.64 |
233.889 |
420.76 |
339.662 |
619.27 |
| 129.516 |
246.01 |
235.815 |
468.47 |
341.634 |
620.48 |
| 131.439 |
248.76 |
237.524 |
551.66 |
343.604 |
622.85 |
| 133.381 |
250.67 |
239.332 |
575.78 |
345.576 |
622.52 |
| 135.333 |
253.60 |
241.650 |
583.30 |
347.540 |
622.03 |
| 137.275 |
256.53 |
243.967 |
580.69 |
349.501 |
625.75 |
| 139.197 |
258.79 |
245.941 |
578.80 |
351.463 |
625.38 |
| 141.135 |
261.75 |
247.914 |
580.61 |
353.411 |
627.88 |
| 143.092 |
264.39 |
249.889 |
580.96 |
355.416 |
631.33 |
| 145.034 |
265.58 |
251.862 |
580.26 |
357.462 |
629.85 |
| 146.958 |
267.04 |
253.834 |
580.79 |
359.497 |
633.48 |
| 148.867 |
269.40 |
255.802 |
582.26 |
361.528 |
634.99 |
| 150.796 |
271.85 |
257.774 |
582.42 |
363.537 |
635.85 |
| 153.301 |
275.44 |
259.743 |
578.44 |
365.564 |
635.68 |
| 155.779 |
277.28 |
261.707 |
578.20 |
367.598 |
638.71 |
| 157.704 |
280.92 |
263.669 |
582.16 |
369.627 |
637.66 |
| 159.672 |
284.18 |
265.633 |
583.67 |
371.643 |
638.92 |
| 161.624 |
286.08 |
267.595 |
582.63 |
373.654 |
643.64 |
| 163.562 |
288.93 |
269.549 |
583.95 |
375.664 |
642.97 |
| 165.490 |
291.82 |
271.508 |
582.90 |
377.621 |
641.54 |
| 167.453 |
292.54 |
273.457 |
583.71 |
379.623 |
644.09 |
| 169.446 |
294.62 |
275.407 |
585.61 |
381.640 |
645.41 |
| 171.429 |
296.99 |
277.352 |
588.66 |
383.614 |
645.21 |
| 173.395 |
300.83 |
279.359 |
587.14 |
385.578 |
647.82 |
| 175.356 |
303.92 |
281.352 |
587.85 |
387.530 |
650.01 |
| 177.305 |
306.21 |
283.350 |
589.18 |
389.526 |
648.42 |
| 179.241 |
308.79 |
285.339 |
592.75 |
391.513 |
647.81 |
| 181.168 |
310.79 |
287.330 |
594.64 |
393.448 |
650.54 |
| 183.125 |
314.07 |
289.371 |
594.05 |
395.446 |
655.72 |
| 185.118 |
316.44 |
291.402 |
595.56 |
397.436 |
656.71 |
| 187.098 |
318.06 |
293.426 |
600.80 |
|
|
In order to fit the heat capacity data well to a polynomial equation, the temperature T was replaced by the reduced temperature X which is defined as:
| | | X = [T − 0.5(Tmax + Tmin)]/[0.5(Tmax − Tmin)] | (1) |
where
T is the thermodynamic temperature,
Tmax and
Tmin are the maximum and the minimum of the temperatures in the experimental temperature range. Then, −1 ≤
X ≤ 1.
The values of the experimental heat capacities can be fitted to the following polynomial equations with the least square method:
For [Bmim][BOB], before the glass transition (82–230 K),
| | | Cp,m/(J K−1 mol−1) = 253.937 + 87.233X − 0.752X2 + 1.271X3 + 2.181X4 + 5.415X5 | (2) |
where the reduced temperature
X = (
T − 156)/74. The above equation is valid from 82 to 230 K. The correlation coefficient of the fitted curve is
R2 = 0.99992, the standard deviation calculated from fitted data is ±0.2%.
After the glass transition (244–398 K),
| | | Cp,m/(J K−1 mol−1) = 557.853 + 33.803X + 16.311X2 + 3.391X3 − 38.341X4 − 0.604X5 + 27.596X6 − 2.181X7 | (3) |
where the reduced temperature
X = (
T − 321)/77. The above equation is valid from 244 to 398 K. The correlation coefficient of the fitted curve is
R2 = 0.99927, the standard deviation calculated from fitted data is ±0.1%.
For [Hmim][BOB], before the glass transition (83–230 K),
| | | Cp,m/(J K−1 mol−1) = 279.346 + 89.957X + 11.183X2 + 18.811X3 − 32.367X4 − 26.454X5 + 28.079X6 + 23.536X7 | (4) |
where reduced temperature
X = (
T − 156.5)/73.5. The above equation is valid from 83 to 230 K. The correlation coefficient of the fitted curve is
R2 = 0.99986, the standard deviation calculated from fitted data is ±0.3%.
After the glass transition (241–398 K),
| | | Cp,m/(J K−1 mol−1) = 607.245 + 38.353X + 9.484X2 + 37.313X3 − 8.442X4 − 83.323X5 + 9.964X6 + 44.552X7 | (5) |
where reduced temperature
X = (
T − 319.5)/78.5. The above equation is valid from 241 to 398 K. The correlation coefficient of the fitted curve is
R2 = 0.99772, the standard deviation calculated from fitted data is ±0.2%.
3.2 Thermodynamic parameters of glass transition
It can be seen from Fig. 1 that the heat capacity jumps, corresponding to the glass transition of the two ILs, took place in the range from 230 to 244 K for [Bmim][BOB], and from 230 to 241 K for [Hmim][BOB]. The glass-transition temperatures, 237.18 and 235.75 K, respectively, were taken from the average temperature of the starting and end points of the heat capacity jumps. The molar enthalpies, ΔHg, and entropies, ΔSg, of their glass transition were determined by the following equations:25| |  | (6) |
| | 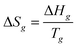 | (7) |
where Q is the total energy introduced into the calorimeter during the course of glass transition; Ti is a temperature point slightly lower than the temperature of the starting glass transition, and Tf is the temperature a little higher than the temperature of the ending glass transition; Tg is the glass-transition temperature; Hcell is the average heat capacity of the empty calorimeter; Cp(s) is the heat capacity of the sample in solid region at temperature (Ti + Tg)/2; Cp(l) is the heat capacity of the sample in liquid region at temperature (Tg + Tf)/2; n is the mole number of the sample. The results of the calculation were ΔHg = 0.989 kJ mol−1 and ΔSg = 4.17 J K−1 mol−1 for [Bmim][BOB], and ΔHg = 1.841 kJ mol−1 and ΔSg = 7.809 J K−1 mol−1 for [Hmim][BOB].
3.3 Thermodynamic functions
The thermodynamic functions (HT − H298.15) and (ST − S298.15) of the two ILs relative to the reference temperature 298.15 K were calculated in the experimental temperature range with an interval of 5 K, by using the polynomial equations of heat capacity and thermodynamic relationships as follows:
For [Bmim][BOB], before the glass transition,
| | 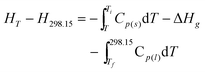 | (8) |
| | 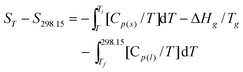 | (9) |
after the glass transition,
| | 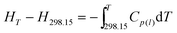 | (10) |
| |  | (11) |
where
Ti is the temperature at which the solid–liquid phase transition started;
Tf is the temperature at which the solid–liquid phase transition ended;
Tg is the temperature of glass transition; Δ
Hg is the molar enthalpy of glass transition.
For [Hmim][BOB], the calculation of thermodynamic functions is the same as those of [Bmim][BOB]. The thermodynamic functions (HT − H298.15) and (ST − S298.15) of the two ILs are listed in Table 2.
Table 2 Thermodynamic functions of [Bmim][BOB] and [Hmim][BOB]
|
T/K |
C
p,m/J K−1 mol−1 |
H
T
− H298.15/kJ mol−1 |
ST − S298.15/J K−1mol−1 |
C
p,m/J K−1 mol−1 |
H
T
− H298.15/kJ mol−1 |
ST − S298.15/J K−1mol−1 |
| |
[Bmim][BOB]
|
[Hmim][BOB]
|
| 80 |
158.42 |
−67.89 |
−357.39 |
175.50 |
−76.65 |
−406.50 |
| 85 |
165.87 |
−67.07 |
−347.55 |
183.55 |
−75.75 |
−395.59 |
| 90 |
172.96 |
−66.23 |
−337.87 |
191.25 |
−74.81 |
−384.88 |
| 95 |
179.75 |
−65.35 |
−328.34 |
198.75 |
−73.84 |
−374.35 |
| 100 |
186.31 |
−64.43 |
−318.95 |
206.12 |
−72.83 |
−363.97 |
| 105 |
192.69 |
−63.48 |
−309.71 |
213.37 |
−71.78 |
−353.74 |
| 110 |
198.94 |
−62.50 |
−300.60 |
220.49 |
−70.69 |
−343.64 |
| 115 |
205.08 |
−61.49 |
−291.62 |
227.44 |
−69.57 |
−333.68 |
| 120 |
211.15 |
−60.45 |
−282.76 |
234.20 |
−68.42 |
−323.85 |
| 125 |
217.17 |
−59.38 |
−274.02 |
240.77 |
−67.23 |
−314.15 |
| 130 |
223.14 |
−58.28 |
−265.38 |
247.14 |
−66.01 |
−304.59 |
| 135 |
229.10 |
−57.15 |
−256.85 |
253.35 |
−64.76 |
−295.15 |
| 140 |
235.03 |
−55.99 |
−248.41 |
259.44 |
−63.48 |
−285.83 |
| 145 |
240.95 |
−54.80 |
−240.06 |
265.46 |
−62.16 |
−276.63 |
| 150 |
246.86 |
−53.58 |
−231.79 |
271.46 |
−60.82 |
−267.53 |
| 155 |
252.76 |
−52.33 |
−223.60 |
277.51 |
−59.45 |
−258.53 |
| 160 |
258.65 |
−51.05 |
−215.49 |
283.66 |
−58.05 |
−249.62 |
| 165 |
264.54 |
−49.75 |
−207.44 |
289.92 |
−56.61 |
−240.79 |
| 170 |
270.43 |
−48.41 |
−199.45 |
296.32 |
−55.15 |
−232.04 |
| 175 |
276.32 |
−47.04 |
−191.53 |
302.85 |
−53.65 |
−223.35 |
| 180 |
282.24 |
−45.64 |
−183.66 |
309.48 |
−52.12 |
−214.72 |
| 185 |
288.19 |
−44.22 |
−175.85 |
316.17 |
−50.55 |
−206.15 |
| 190 |
294.19 |
−42.76 |
−168.08 |
322.88 |
−48.96 |
−197.63 |
| 195 |
300.28 |
−41.28 |
−160.36 |
329.59 |
−47.33 |
−189.16 |
| 200 |
306.48 |
−39.76 |
−152.68 |
336.32 |
−45.66 |
−180.74 |
| 205 |
312.85 |
−38.21 |
−145.03 |
343.13 |
−43.96 |
−172.35 |
| 210 |
319.43 |
−36.63 |
−137.41 |
350.24 |
−42.23 |
−164.00 |
| 215 |
326.28 |
−35.02 |
−129.82 |
357.98 |
−40.46 |
−155.67 |
| 220 |
333.48 |
−33.37 |
−122.24 |
366.91 |
−38.65 |
−147.34 |
| 225 |
341.12 |
−31.68 |
−114.66 |
377.88 |
−36.79 |
−138.97 |
| 230 |
Glass transition |
Glass transition |
| 235 |
|
|
|
|
|
|
| 240 |
|
|
|
|
|
|
| 245 |
528.80 |
−28.58 |
−101.38 |
581.18 |
−31.13 |
−114.94 |
| 250 |
528.78 |
−25.93 |
−91.12 |
580.77 |
−28.23 |
−103.21 |
| 255 |
530.00 |
−23.29 |
−81.03 |
580.60 |
−25.32 |
−91.73 |
| 260 |
531.96 |
−20.63 |
−71.11 |
580.92 |
−22.42 |
−80.46 |
| 265 |
534.31 |
−17.97 |
−61.33 |
581.83 |
−19.51 |
−69.38 |
| 270 |
536.78 |
−15.29 |
−51.71 |
583.31 |
−16.60 |
−58.48 |
| 275 |
539.23 |
−12.60 |
−42.22 |
585.25 |
−13.68 |
−47.75 |
| 280 |
541.57 |
−9.90 |
−32.87 |
587.54 |
−10.75 |
−37.18 |
| 285 |
543.75 |
−7.18 |
−23.65 |
590.04 |
−7.80 |
−26.76 |
| 290 |
545.79 |
−4.46 |
−14.56 |
592.63 |
−4.85 |
−16.47 |
| 295 |
547.71 |
−1.73 |
−5.59 |
595.23 |
−1.88 |
−6.33 |
| 298.15 |
548.89 |
0.00 |
0.00 |
596.84 |
0.00 |
0.00 |
| 300 |
549.58 |
1.02 |
3.26 |
597.78 |
1.11 |
3.69 |
| 305 |
551.43 |
3.77 |
12.01 |
600.26 |
4.10 |
13.59 |
| 310 |
553.33 |
6.53 |
20.64 |
602.68 |
7.11 |
23.36 |
| 315 |
555.31 |
9.30 |
29.17 |
605.07 |
10.13 |
33.02 |
| 320 |
557.42 |
12.08 |
37.60 |
607.49 |
13.16 |
42.57 |
| 325 |
559.65 |
14.88 |
45.93 |
609.99 |
16.20 |
52.01 |
| 330 |
562.02 |
17.68 |
54.17 |
612.63 |
19.26 |
61.34 |
| 335 |
564.52 |
20.50 |
62.32 |
615.44 |
22.33 |
70.58 |
| 340 |
567.10 |
23.33 |
70.38 |
618.44 |
25.41 |
79.73 |
| 345 |
569.74 |
26.17 |
78.35 |
621.62 |
28.51 |
88.79 |
| 350 |
572.38 |
29.02 |
86.24 |
624.93 |
31.63 |
97.76 |
| 355 |
574.98 |
31.89 |
94.06 |
628.31 |
34.76 |
106.64 |
| 360 |
577.50 |
34.77 |
101.80 |
631.66 |
37.91 |
115.45 |
| 365 |
579.92 |
37.67 |
109.46 |
634.88 |
41.08 |
124.18 |
| 370 |
582.23 |
40.57 |
117.05 |
637.89 |
44.26 |
132.83 |
| 375 |
584.47 |
43.49 |
124.57 |
640.64 |
47.46 |
141.41 |
| 380 |
586.73 |
46.42 |
132.03 |
643.17 |
50.67 |
149.91 |
| 385 |
589.13 |
49.36 |
139.42 |
645.64 |
53.89 |
158.34 |
| 390 |
591.89 |
52.31 |
146.75 |
648.42 |
57.12 |
166.69 |
| 395 |
595.30 |
55.28 |
154.02 |
652.11 |
60.37 |
174.98 |
| 400 |
599.77 |
58.26 |
161.23 |
657.68 |
63.65 |
183.20 |
3.4 Thermal property studies by DSC
The DSC curves of [Bmim][BOB] and [Hmim][BOB] are shown in Fig. 2. It should be noted that the scan began from 300 K cooling down to 173 K, followed by heating from 173 to 290 K. For [Bmim][BOB], one exothermic peak due to the freezing was observed at 196.3 K during the cooling process, and one endothermic peak due to glass transition appeared at 240.8 K when heating. The DSC curve of [Hmim][BOB] was similar to that of [Bmim][BOB], but the positions of exothermic and endothermic peaks moved to 207.4 and 238.0 K, respectively. Their glass-transition temperatures determined from DSC measurements are 238.8 and 235.4 K which are in agreement with the values observed from adiabatic calorimetry. The 238.8 K of Tg for [Bmim][BOB] is 5 K lower than the Tg value reported by Angell et al.19 This may be due to their faster heating rate (20 K min−1) used in the DSC measurements.
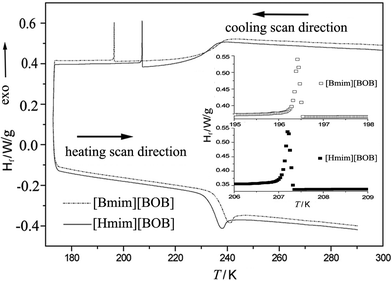 |
| | Fig. 2
DSC curves measured under high purity nitrogen. Hf is the heat flow. The inset shows the detailed exothermic peaks of the two ILs. | |
In general, three types of thermal behavior are observed for the imidazolium based ionic liquids.11 The first type has a distinct freezing point on cooling and a melting point on heating. The second type shows only glass transition with neither melting nor freezing points. The third one is characterized by cold crystallization. Upon heating, the compound passes from the glass to a sub-cooled liquid phase, and then cold crystallization occurs, followed by melting transition. In our cases, the thermal behavior of [Bmim][BOB] and [Hmim][BOB] is similar to the second type except for the additional appearance of freezing points during cooling. Cold crystallization occurs after the glass phase formation during cooling which is accompanied by exothermic behavior, as seen in the inset of Fig. 2. The molar enthalpy for the crystallization of the two ILs calculated through integration of the area of exothermic peaks is 37.7 J mol−1 and 57.1 J mol−1, respectively. No corresponding endothermic peak was observed during the heating process which might be due to the heat energy required for this phase transition being much smaller than that offered by the DSC equipment.
3.5 Estimation and comparison of thermodynamic properties for imidazolium based ionic liquids
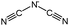
Although the data reported in literature for heat capacity values of ILs are rather scarce, some heat capacities at specific temperature for imidazolium based ILs are available, which are listed in Table 3. Analyzing the data in Table 3 indicates that at a certain temperature, the more atoms in the anion, the higher the molar heat capacity of the ILs.11 The molar heat capacity of [Hmim][BOB] is higher than that of [Bmim][BOB] at the same temperature due to the additional two –CH2groups in its cation. The higher molar heat capacity of [Bmim][dca] contrasting to [Bmim][BF4] at the same temperature is because that the [dca] anion has chemical triple bonds which can store more energy.
| Ionic liquid |
Molecular weight |
Cp(298 K)/J mol−1 K−1 |
C
p(323 K)/J mol−1 K−1 |
T
g
/K |
Anion structures |
Point group |
|
The data of other [Bmim] type ILs are taken from ref. 11 except that the Tg value of 243.8 K for [Bmim][BOB] from ref. 19 The data obtained in our work is presented in bold.
|
|
[Bmim][Cl]
|
174.6709 |
322.7 |
333.7 |
204 |
Cl
|
|
|
[Bmim][dca]
|
205.2598 |
364.6 |
370.0 |
183 |
|
C
2v
|
|
[Bmim][Br]
|
219.1222 |
316.7 |
323.6 |
223 |
Br
− |
|
|
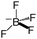
[Bmim][BF4]
|
226.0228 |
351.5 |
358 |
188 |
|
T
d
|
|
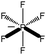
[Bmim][PF6]
|
284.1824 |
397.6 |
405.1 |
197 |
|
O
h
|
|
[Bmim][BOB]
|
326.0672 |
549.068 (at 298.69 K) |
559.276 (at 324.26 K) |
238.8
|
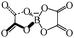
|
D
2d
|
| |
|
|
|
243.8 |
|
[Hmim][BOB]
|
354.1203 |
597.881 (at 297.48 K) |
610.325 (at 323.73 K) |
235.4
|
|
[Bmim][Tf2N]
|
419.3644 |
536.3 |
543.9 |
187 |
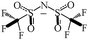
|
C
2v
|
|
[Bmim][methide]
|
550.4380 |
782.8 |
802.4 |
208 |

|
C
3v
|
The glass-transition temperatures of some [Bmim] based ILs are listed in Table 3. No simple correlation between glass-transition temperatures and the molar volume of anions can be found though it is well known that the glass-transition temperature increases with increasing of ion size.26 Another generally accepted viewpoint is that the decrease in ion symmetry and increase in the number of atoms with strong electronegativity in anion will help obtain desirable ILs with a wide liquid temperature range.27,28 Therefore, the structural symmetry of the anion or cation has a significant impact on the physicochemical properties of ILs. Checking the symmetry of anions in Table 3 finds that [dca], [Tf2N] and [methide] anions have the symmetry of C2v, C2v and C3v point group, respectively, which are the only three anions with dipole moments among those compounds listed in Table 3.29,30 The strong electronegative atoms, F, S or O, in these ILs can better disperse the negative charges of anions, which may contribute to the lower melting point or glass-transition temperature. For a given cation, when the anions have comparable capacities of negative charge delocalization, the glass-transition temperature increases with increasing of anion size. Based on this assumption, higher Tg of [BOB] type ILs can be easily explained. Although the content of O atoms in [BOB] anion is high which may help to delocalize the negative charge, its D2d symmetry without dipole moment counteracts this effect, which leads to a higher Tg. In terms of the above analysis, we suggest to design and synthesize the ILs with anions containing strong electro negative atoms, and with the symmetry of Cn or Cnv (n = 1,2,3,…,∞) point groups (only compounds with the above mentioned structural symmetries have dipole moments.29) so that the ILs have widened liquid ranges.
Conclusions
1-Butyl-3-methylimidazolium bis(oxalato)borate ([Bmim][BOB]) and 1-hexyl-3-methylimidazolium bis(oxalato)borate ([Hmim][BOB]) were prepared and studied by using adiabatic calorimetry and differential scanning calorimetry. Their thermodynamic properties and phase change behavior were compared with other series of [Bmim] based ionic liquids. The results indicate that their heat capacities increase with increasing of the atom number of the anion. The glass-transition temperature of ILs is normally affected by the ion size and the charge dispersion degree. The high content of strong electro negative atoms and Cn or Cnv (n = 1,2,3,…,∞) point group symmetry in the anion are recommended for the design room temperature ILs with a wide liquid temperature range.
Acknowledgements
We are thankful for financial support from the National Natural Science Foundation of China under grant NSFC No. 20901076 and 21073189, the Scientific Research Foundation for Returned Scholars from the Ministry of Education of China, and the Natural Science Foundation of Liaoning Province, China under grant No.20092174. A new ionic liquid database, recently launched by our research group, aims to collect comprehensive and up-to-date information on ionic liquids. The database—Delph-IL—is searchable by compounds or properties, and classifies data sets using quality indicators (Novionic, delph-IL, www.delphil.net, 2010).
References
- R. P. Swatloski, S. K. Spear, J. D. Holbrey and R. D. Rogers, J. Am. Chem. Soc., 2002, 124, 4974–4975 CrossRef CAS.
- T. Welton, Chem. Rev., 1999, 99, 2071–2083 CrossRef CAS.
- A. C. Cole, J. L. Jensen, I. Ntai, K. L. T. Tran, K. J. Weaver, D. C. Forbes and J. H. Davis, J. Am. Chem. Soc., 2002, 124, 5962–5963 CrossRef CAS.
- J. H. Davis, Chem. Lett., 2004, 33, 1072–1077 CrossRef.
- S. J. Zhang, N. Sun, X. Z. He, X. M. Lu and X. P. Zhang, J. Phys. Chem. Ref. Data, 2006, 35, 1475–1517 CrossRef CAS.
- Y. A. Sanmamed, P. Navia, D. Gonzalez-Salgado, J. Troncoso and L. Romani, J. Chem. Eng. Data, 2010, 55, 600–604 CrossRef CAS.
- R. Souda, J. Phys. Chem. B, 2008, 112, 15349–15354 CrossRef CAS.
- A. Muhammad, M. I. A. Mutalib, C. D. Wilfred, T. Murugesan and A. Shafeeq, J. Chem. Thermodyn., 2008, 40, 1433–1438 CrossRef CAS.
- H. Tokuda, K. Hayamizu, K. Ishii, M. Susan and M. Watanabe, J. Phys. Chem. B, 2005, 109, 6103–6110 CrossRef CAS.
- H. L. Ngo, K. LeCompte, L. Hargens and A. B. McEwen, Thermochim. Acta, 2000, 357–358, 97–102 CrossRef CAS.
- C. P. Fredlake, J. M. Crosthwaite, D. G. Hert, S. Aki and J. F. Brennecke, J. Chem. Eng. Data, 2004, 49, 954–964 CrossRef CAS.
- Y. Shimizu, Y. Ohte, Y. Yamamura, K. Saito and T. Atake, J. Phys. Chem. B, 2006, 110, 13970–13975 CrossRef CAS.
- Z. H. Zhang, W. Guan, J. Z. Yang, Z. C. Tan and L. X. Sun, Acta Physico-Chim. Sinica, 2004, 20, 1469–1471 Search PubMed.
- Z. H. Zhang, Z. C. Tan, Y. S. Li and L. X. Sun, J. Therm. Anal. Calorim., 2006, 85, 551–557 CrossRef CAS.
- Z. H. Zhang, Z. C. Tan, L. X. Sun, J. Z. Yang, X. C. Lv and Q. Shi, Thermochim. Acta, 2006, 447, 141–146 CrossRef CAS.
- B. Tong, Q. S. Liu, Z. C. Tan and U. Welz-Biermann, J. Phys. Chem. A, 2010, 114, 3782–3787 CrossRef CAS.
- H. Bruglachner, S. Jordan, M. Schmidt, W. Geissler, A. Schwake, J. Barthel, B. E. Conway and H. J. Cores, J. New Mater. Electrochem. Syst., 2006, 9, 209–220 CAS.
- S. F. Yu, S. Lindeman and C. D. Tran, J. Org. Chem., 2008, 73, 2576–2591 CrossRef CAS.
- W. Xu, L. M. Wang, R. A. Nieman and C. A. Angell, J. Phys. Chem. B, 2003, 107, 11749–11756 CrossRef CAS.
- M. Yang, F. F. Xu, Q. S. Liu, P. F. Yan, X. M. Liu, C. Wang and U. Welz-Biermann, Dalton Trans., 2010, 39, 10571–10573 RSC.
- Z. C. Tan, G. Y. Sun, Y. Sun, A. X. Yin, W. B. Wang, J. C. Ye and L. X. Zhou, J. Therm. Anal., 1995, 45, 59–67 CrossRef CAS.
- Z. C. Tan, L. X. Sun, S. H. Meng, L. Li, F. Xu, P. Yu, B. P. Liu and J. B. Zhang, J. Chem. Thermodyn., 2002, 34, 1417–1429 CrossRef CAS.
- Z. C. Tan, Q. Shi, B. P. Liu and H. T. Zhang, J. Therm. Anal. Calorim., 2008, 92, 367–374 CrossRef CAS.
- D. G. Archer, J. Phys. Chem. Ref. Data, 1993, 22, 1441–1453 CrossRef CAS.
- Z. C. Tan, B. Xue, S. W. Lu, S. H. Meng, X. H. Yuan and Y. J. Song, J. Therm. Anal. Calorim., 2000, 63, 297–308 CrossRef.
- W. Xu, E. I. Cooper and C. A. Angell, J. Phys. Chem. B, 2003, 107, 6170–6178 CrossRef CAS.
- Z. B. Zhou, H. Matsumoto and K. Tatsumi, Chem.–Eur. J., 2005, 11, 752–766 CrossRef CAS.
-
P. Wasserschaid, in Ionic Liquids in Synthesis, second ed., Wiley–VCH, Weinheim, Germany, second edn, 2007 Search PubMed.
-
G. D. Zhou and L. Y. Duan, in The Basis of Structural Chemistry, Peking University Publisher, China, forth edn, 2008 Search PubMed.
-
K. V. Reddy, in Symmetry and Spectroscopy of Molecules, New Age Science, Second edn, 2010 Search PubMed.
|
| This journal is © the Owner Societies 2011 |
Click here to see how this site uses Cookies. View our privacy policy here. ![Preparation scheme of [Bmim]/[Hmim][BOB] ILs.](/image/article/2011/CP/c0cp01744b/c0cp01744b-s1.gif)
![Experimental molar heat capacity Cp,m of [Bmim][BOB] and [Hmim][BOB] as a function of temperature.](/image/article/2011/CP/c0cp01744b/c0cp01744b-f1.gif)