Structural and thermodynamic similarities of phases in the Li–Tt (Tt = Si, Ge) systems: redetermination of the lithium-rich side of the Li–Ge phase diagram and crystal structures of Li₁₇Si_4.0−xGe_x for x = 2.3, 3.1, 3.5, and 4 as well as Li_4.1Ge

Abstract

A reinvestigation of the lithium-rich section of the Li–Ge phase diagram reveals the existence of two new phases, Li₁₇Ge₄ and Li_4.10Ge (Li_16.38Ge₄). Their structures are determined by X-ray diffraction experiments of large single crystals obtained from equilibrated melts with compositions Li₉₅Ge₅ and Li₈₅Ge₁₅. Excess melt is subsequently removed through isothermal centrifugation at 400 °C and 530 °C, respectively. Li₁₇Ge₄ crystallizes in the space group F4̄3m (a = 18.8521(3) Å, V = 6700.1(2) ų, Z = 20, T = 298 K) and Li_4.10Ge (Li_16.38Ge₄) in Cmcm (a = 4.5511(2) Å, b = 22.0862(7) Å, c = 13.2751(4) Å, V = 1334.37(8) ų, Z = 16, T = 123 K). Both phases are isotypic with their Si counterparts and are further representative of the Li₁₇Pb₄ and Li_4.11Si structure types. Additionally, the solid solutions Li₁₇Si_4−xGe_x follows Vegard's law. A comparison of the GeLi_n coordination polyhedra shows that isolated Ge atoms are 13- and 14-coordinated in Li₁₇Ge₄, whereas in Li_16.38Ge₄ the Ge atoms possess coordination numbers 12 and 13. Regarding the thermodynamic stability, Li_16.38Ge₄ is assigned a high-temperature phase existing between ∼400 °C and 627 °C, whereas Li₁₇Ge₄ decomposes peritectically at 520–522 °C. Additionally, the decomposition of Li_16.38Ge₄ below ∼400 °C was found to be very sluggish. These findings are manifested by differential scanning calorimetry, long-term annealing experiments and the results from melt equilibration experiments. Interestingly, the thermodynamic properties of the lithium-rich tetrelides Li₁₇Tt₄ and Li_4.1Tt (Li_16.4Tt₄) are very similar (Tt = Si, Ge). Besides Li₁₅Tt₄, Li₁₄Tt₆, Li₁₂Tt₇, and LiTt, the title compounds are further examples of isotypic tetrelides in the systems Li–Tt.

---

Introduction

In the last decade, the demand for high capacity lithium-ion batteries (LIBs) decisively influenced numerous fields of research; in particular, the chemistry of group 14 elements (tetrel = Tt) plays an important role in the development of more efficient anode materials. Since Si theoretically offers a specific capacity of 3579 mA h g⁻¹ (based on the formation of Li₁₅Si₄) and thus massively exceeds the capacity of the commonly used graphite anode (372 mA h g⁻¹, LiC₆),^1,2 research on Li–Si materials has been in focus for many years. As the high capacity of Si is associated with several problems such as a large volume expansion of up to 300% upon lithiation accompanied by contact loss of electrodes and poor cycle life, a large number of these studies target these issues.³ Further, Li–Si phases predominantly occur amorphously during charging and discharging, and only at low discharge voltages, crystalline Li₁₅Si₄ is observed.^1,2,4,5 However, the processes in working LIBs can be nicely monitored by in situ/ex situ NMR investigations.^6,7 Moreover, a fundamental understanding of thermodynamic properties and an unambiguous structural characterization of Li–Si phases are of considerable importance. Just recently, we reported on detailed investigations on lithium-rich silicides, including the metastable phase Li₁₅Si₄,⁸ Li₁₇Si₄⁹ and the high-temperature phase Li_4.11Si (Li_16.42Si₄).¹⁰ Further examples are given in ref. 11–17.

The heavier tetrel element Ge has received less attention regarding its use as an anode material due to its low natural abundance connected with a lower theoretical specific capacity compared to silicon (1564 mA h g⁻¹vs. 4056 mA h g⁻¹, based on the formation of Li_16.95Ge₄¹⁸ and Li₁₇Si₄,⁹ respectively). However, the diffusivity of lithium in Ge is approximately 400 times larger than in Si at room temperature,^19,20 which intrinsically puts germanium to the foreground. Since some Si analogues are not known we put emphasis on the structural variety of lithium germanides and their thermodynamic relation.

Li–Ge phases were first postulated in the 1950s and 1960s (Li₃Ge, Li₄Ge, LiGe),^21–23 fueling numerous investigations in this field later on. Before 2001, the ascertained Li–Ge representatives included Li₂₁Ge₅²⁴ (formerly described as Li₂₀Ge₅^25,26 and Li₂₂Ge₅^27,28), Li₁₅Ge₄,^28,29 Li₁₃Ge₄³⁰ (formerly reported as Li₇Ge₂³¹), Li₁₄Ge₆,^25,26 Li₉Ge₄,³² Li₁₂Ge₇,^25,26,33 LiGe (space group I4₁/a, ambient pressure),^22,34 LiGe (space group I4₁/amd, high pressure),³⁵ and Li₇Ge₁₂.^26,36 A previously described phase Li₁₁Ge₆³⁷ could not be reproduced in the course of the redetermination of the Li–Ge phase diagram by Grüttner,²⁵ and due to the “striking similarity” to Li₈MgSi₆,³⁸ it might have been ternary Li₈MgGe₆. Until then, solely Li₂₁Tt₅, Li₁₄Tt₆, Li₁₂Tt₇, and LiTt (LiSi³⁹ is obtainable from high-pressure synthesis) were known for both Si and Ge. We note that Li₁₃Si₄ and Li₁₃Ge₄ are not isotypic and crystallize with their own structure types. In the following years new Li–Tt phases were found and others were revised, e.g. a hexagonal high-pressure form of LiGe (space group P6₃/mmc),⁴⁰ metastable Li₁₅Si₄^1,2 being isotypic with the congruently melting phase Li₁₅Ge₄²⁵ (Li₁₅Tt₄), Li_∼17Ge₄ (Li_21+3/16Si₅ = Li_16.95Ge₄)¹⁸ revised from Li₂₁Ge₅, and the revision of the Li₇Ge₁₂ structure.⁴¹ The synthesis of solid solutions Li₁₅Si_4−xGe_x by mechanical ball-milling was also reported.⁴² An experimental determination of the Li–Ge phase diagram involving most of the aforementioned phases is given by Grüttner in his dissertation.²⁵

We recently reported on the single crystal structures and thermodynamic properties of Li₁₇Si₄,⁹ the high-temperature phase Li_4.11Si (Li_16.42Si₄),¹⁰ and metastable Li₁₅Si₄.⁸ The Li–Si phase diagram was revised accordingly. Consequently, we extended our studies on the Li–Ge system. Herein, we present the single crystal X-ray structure determination of Li₁₇Ge₄ and Li_4.10Ge which crystallize isotypically with their Si counterparts. Earlier structure reports on Li_∼17Ge₄ (Li_21+3/16Si₅ = Li_16.95Ge₄)¹⁸ involving partially occupied Li sites could not be confirmed. Solid solutions of the isotypic phases Li₁₇Si₄ and Li₁₇Ge₄ follow Vegard's law.⁴³ Due to clarity and a better comparability, the phase Li_4.10Ge is referred to as Li_16.38Ge₄. Furthermore, the lithium-rich section of the Li–Ge phase diagram (Li concentrations >79 at%) is reinvestigated by differential scanning calorimetry and long-term annealing experiments, manifesting Li_16.38Ge₄ as a high-temperature phase which possesses a very sluggish decomposition behavior below ∼400 °C. Li₁₇Ge₄ is peritectically formed at 520–522 °C from cooling an according melt.

Results and discussion

Single crystal X-ray structure determination of Li₁₇Ge₄ and Li_16.38Ge₄

Large single crystals of Li₁₇Ge₄ and Li_16.38Ge₄ were grown in Li–Ge melts Li₉₅Ge₅ and Li₈₅Ge₁₅ at 400 °C and 530 °C, respectively. For Li_16.38Ge₄, bar-shaped crystals with a size of up to 0.6 × 0.25 × 0.25 cm³ could be obtained. A representative specimen is depicted in Fig. 1. Generally, crystals of Li₁₇Ge₄ grew much smaller in a block-like shape with diameters of 0.1 × 0.1 × 0.1 cm³. Those crystals allowed acquisition of high quality single crystal X-ray diffraction data.

Fig. 1 Example of a bar-shaped single crystal of Li_16.38Ge₄ obtained from a melt Li₈₅Ge₁₅ at 530 °C.

The phase Li₁₇Ge₄ crystallizes in the space group F4̄3m with a = 18.8521(3) Å (V = 6700.1(2) ų) and Z = 20. The asymmetric unit consists of 13 Li and four Ge atoms each being located on a special position (Table 1). As already reported for Li₁₇Si₄, Li₁₇Sn₄, and Li₁₇Pb₄,^9,18,44 the Wyckoff position 4a with symmetry 4̄3m was found to be fully occupied. We note that the refinement of occupancy factors did not indicate significantly large deviations from full occupancy and thus they were regarded as being fully occupied. Accordingly, the cubic unit cell of Li₁₇Ge₄ contains 340 Li and 80 Ge atoms (cF420). Further, atomic displacement parameters were refined anisotropically with meaningful results for all atoms, revealing excellent reliability factors of R₁ = 0.022 and wR₂ = 0.038 (all data) for the final model (Table 6). The structure of Li₁₇Ge₄ is isotypic with Li₁₇Si₄, Li₁₇Sn₄, and Li₁₇Pb₄.^9,18,44

Table 1 Fractional atomic coordinates and isotropic equivalent atomic displacement parameters for Li₁₇Ge₄ (F4̄3m, Z = 20, T = 298 K, estimated standard deviations in parentheses)

Atom	Wyckoff position	x	y	z	U eq/Å^2·10^3
Ge1	16e	0.159545(8)	x	x	13.03(4)
Ge2	16e	0.916568(8)	x	x	11.37(4)
Ge3	24f	0.32102(1)	0	0	13.27(5)
Ge4	24g	0.57015(1)	1/4	1/4	13.06(4)
Li1	16e	0.0734(2)	x	x	31(1)
Li2	16e	0.3031(2)	x	x	21.6(8)
Li3	16e	0.4175(2)	x	x	22.3(9)
Li4	16e	0.5575(2)	x	x	23(1)
Li5	16e	0.6877(2)	x	x	28(1)
Li6	16e	0.8314(2)	x	x	27(1)
Li7	24f	0.1677(3)	0	0	25(1)
Li8	24g	0.0743(3)	1/4	1/4	23.1(9)
Li9	48h	0.0913(2)	x	0.2624(2)	32.0(9)
Li10	48h	0.0896(2)	x	0.7612(2)	31.1(8)
Li11	48h	0.1547(1)	x	0.5205(2)	32.5(8)
Li12	48h	0.1637(1)	x	0.0027(2)	22.5(8)
Li13	4a	0	0	0	17(2)

---

Li_16.38(2)Ge₄ crystallizes in the space group Cmcm with a = 4.5511(2) Å, b = 22.0862(7) Å, c = 13.2751(4) Å (V = 1334.37(8) ų) and Z = 16 referring to Li_4.096(4)Ge as one formula unit. The unit cell contains 10 Li and three Ge atom positions (Table 2) where Li4 and Li5 are disordered. A careful analysis of difference Fourier maps after assigning all Ge and nine Li atom positions (Li1–Li3 and Li5–Li10) revealed occupational disorder along the crystallographic a-axis like that reported for Li_16.42Si₄.¹⁰ As can be seen in Fig. 2a and 2b, the difference Fourier maps showing the strand-like residual electron density with peak-maxima at Wyckoff positions 4c and 8g are almost identical for Li_16.42(1)Si₄ and Li_16.38(2)Ge₄. Applying the disorder model as reported for Li_16.42(1)Si₄,¹⁰ we subsequently obtained a very similar occupancy ratio of 0.616(8)/0.384(8) for Li4A on 4c (1/2, y, 1/4) and Li4B on 8g (x, y, 1/4), respectively, compared with 0.575(3)/0.425(3) found in Li_16.42(1)Si₄. Analogously to Li_16.42(1)Si₄,¹⁰ a split position for Li5 on 8f (0, x, y) was introduced. The split fractions converged to 0.75(4) for Li5A and 0.25(4) for Li5B (0.848(7)/0.152(7) in Li_16.42(1)Si₄;¹⁰ refinement details and the geometric relevance of the atom split are given in the ESI†). In case of atoms being uninvolved in disorder, site occupancy factors were refined to values close to full occupancy and therefore those positions were constrained to full occupancy. Thus, the unit cell contains 65.54(7) Li atoms as a consequence of the disorder (65.70(3) in Li_16.42(1)Si₄¹⁰) and 16 Ge atoms, resulting in a crystallographic density of 2.011 g cm⁻³. The structure was finally solved with reliability factors of R₁ = 0.023 and wR₂ = 0.027 for all data (Table 6). Li_16.38(2)Ge₄ crystallizes isotypically with Li_16.42(1)Si₄.¹⁰

Fig. 2 Difference Fourier map (F_o − F_c) shown for the layer defined by Tt1, Tt2 and Li2 in (a) Li_16.42Si₄¹⁰ and (b) Li_16.38Ge₄ (parallel to the ab-plane, calculated from single crystal data at 100 K and 123 K for Li_16.42Si₄ (contour lines ±0.6 e Å⁻³) and Li_16.38Ge₄ (contour lines ±0.5 e Å⁻³), respectively; cell edges are shown in blue).

Table 2 Fractional atomic coordinates and isotropic equivalent atomic displacement parameters for Li_4.096(4)Ge (Cmcm, Z = 16, T = 100 K, estimated standard deviations in parentheses)

Atom	Wyckoff position	x	y	z	s.o.f.	U eq./Å^2·10^3
Ge1	4c	0	0.256372(6)	1/4	1	7.83(3)
Ge2	4c	1/2	0.454314(5)	1/4	1	6.35(3)
Ge3	8f	1/2	0.105220(4)	0.067609(6)	1	6.83(2)
Li1	4c	1/2	0.0333(1)	1/4	1	14.4(4)
Li2	4c	1/2	0.3297(1)	1/4	1	13.5(4)
Li3	4c	0	0.3922(1)	1/4	1	17.5(5)
Li4A	4c	1/2	0.1476(2)	1/4	0.616(8)	52(3)
Li4B	8g	0.210(1)	0.1385(2)	1/4	0.384(8)	21(2)
Li5A	8f	0	0.1741(3)	0.0842(7)	0.75(4)	14(1)
Li5B	8f	0	0.164(1)	0.123(4)	0.25(4)	24(6)
Li6	8f	0	0.04650(7)	0.1225(1)	1	13.9(3)
Li7	8f	0	0.31636(9)	0.0788(1)	1	16.5(3)
Li8	8f	0	0.47089(9)	0.0908(1)	1	18.7(3)
Li9	8f	1/2	0.23155(8)	0.1356(1)	1	19.1(3)
Li10	8f	1/2	0.40425(8)	0.0639(1)	1	14.5(3)

---

Structure description of Li₁₇Ge₄ and Li_16.38Ge₄

In our previous work we reported on the structures of Li₁₇Si₄ and Li_16.42Si₄ in detail.^9,10 Both phases were comparatively highlighted on the basis of SiLi_n coordination polyhedra and the disorder in Li_16.42Si₄ was illustrated with various structure models. Hence, we analogously elaborate on the structures of the isotypic phases Li₁₇Ge₄ and Li_16.38Ge₄ herein.

The structure of Li₁₇Ge₄ is closely related to the previously reported phase Li₂₁Ge₅²⁴ (Li_16.8Ge₄) only differing in the occupation of one fourfold special position. In detail, their common space group F4̄3m possesses four positions with site symmetry 4̄3m (4a–d). Whereas Wyckoff positions 4a–d were claimed to be void in Li₂₁Ge₅, we found a fully occupied 4a site in Li₁₇Ge₄ with short but reasonable next nearest neighbor distances of 2.397(7) Å for Li1–Li13 (cf. Fig. 3 and Table 4). We note that since Li₂₂Si₅^27,45 (its composition corresponds to a full occupancy of sites 4a–d) was revised to Li₂₁Si₅,⁴⁶ heavier analogues such as Li₂₂Ge₅ were supposed to crystallize with the Li₂₁Si₅ structure type as well (Li₂₁Ge₅).²⁴ We have already shown by computational methods that the fully relaxed structures of Li₁₇Si₄ and Li₂₁Si₅ decisively differ regarding the coordination environment around the 4a site.⁹ If this position is unoccupied, the first coordination shell, which is a (Li1)₄ tetrahedron (cf. Fig. 3), is markedly contracted, which was not observed in experimental data of Li₂₁Si₅ (Table S5 in the ESI†). Therefore it could be concluded that Li might have been overseen in the previous structure refinement (most likely a partial occupancy). Turning to Li₁₇Ge₄, the positional parameters are almost identical with Li₁₇Si₄ (Table S6 in the ESI†) and therefore an equal conclusion is reasonable.

Fig. 3 Coordination environment of Li13 on the Wyckoff position 4a (Ge = black; Li = white; thermal ellipsoids at 70% probability, single crystal data at room temperature). The 1st, 2nd, 3rd, and 4th coordination shell is formed by a (Li1)₄ and (Ge2)₄ tetrahedron as well as a (Li7)₆ octahedron and a (Li12)₁₂ cuboctahedron.

In 2001, Goward et al.¹⁸ have already reported on the revision of Li₂₂M₅ to Li₁₇M₄ (M = Ge, Sn, Pb). However, their model for “Li₁₇Ge₄” involved partially occupied Li sites (Table 3), namely Li1A on 16e (3/4 occ.), Li1B on 16e (1/4 occ.), and Li13 on 4a (3/4 occ.) resulting in the composition Li_16.95Ge₄ (note that for a better comparability the fractional atomic coordinates and labels were adapted to Li₁₇Ge₄). In the case of a void 4a site, the surrounding (Li1A)₄ tetrahedron (comparable with Li1 in Fig. 3) is slightly contracted to (Li1B)₄ whose vertices are markedly closer to the 4a center (2.52 Å vs. 1.91 Å).¹⁸ Interestingly, this scenario is in close agreement with the computationally relaxed structure of Li₂₁Si₅⁹ (Table S7 in the ESI†) where all fourfold positions are void. Consequently, a partial occupancy of the 4a position as reported for Li_16.95Ge₄ and hence the existence of a small homogeneity region Li_17−xTt₄ (0 < x < 0.2) are indeed meaningful. More recently, Lacroix-Orio et al.⁴⁷ presented a Zn-doped derivative of the Li₁₇Ge₄ compound. They could not find any evidence for partially occupied Li positions and instead found small concentrations of Zn being incorporated at the 4a position (Li_17−εZn_εGe₄, ε = 0.005(1)).

Table 3 Comparison of fractional atomic coordinates for Li₁₇Ge₄, Li_17−εZn_εGe₄, and Li_16.95Ge₄ (space group F4̄3 m (for all phases), Z = 20, estimated standard deviations in parentheses). Listed are x values for the special positions 16e (x, x, x), 24f (x, 0, 0), 24g (x, 1/4, 1/4) and x, z pairs for 48h (x, x, z)

At.	Wyck. pos.	Li17Ge4	Li17−εZnεGe4^47	Li16.95Ge4^18	At.	Wyck. pos.	Li17Ge4	Li17−εZnεGe4^47	Li16.95Ge4^18
Ge1	16e	0.15955(1)	0.15958(2)	0.15952(5)	Li8	24g	0.0743(3)	0.0740(6)	0.075(1)
Ge2	16e	0.91657(1)	0.91667(3)	0.91684(5)	Li9	48h	0.0913(2)	0.0906(3)	0.0907(7)
Ge3	24f	0.32102(1)	0.32118(4)	0.32112(7)			0.2624(2)	0.2631(4)	0.2660(8)
Ge4	24g	0.57015(1)	0.57020(4)	0.56965(7)	Li10	48h	0.0896(2)	0.0904(4)	0.0914(7)
Li1A	16e	0.0734(2)	0.0747(6)	0.0775			0.7612(2)	0.7613(4)	0.7597(9)
Li1B	16e	—	—	0.0587	Li11	48h	0.1547(1)	0.1554(3)	0.1540(5)
Li2	16e	0.3031(2)	0.3033(3)	0.3032(6)			0.5205(2)	0.5216(4)	0.5216(9)
Li3	16e	0.4175(2)	0.4179(5)	0.4169(9)	Li12	48h	0.1637(1)	0.1632(3)	0.1625(5)
Li4	16e	0.5575(2)	0.5584(4)	0.5579(6)			0.0027(2)	0.0025(5)	0.005(1)
Li5	16e	0.6877(2)	0.6864(4)	0.6876(7)	Li13	4a	0	0	0
Li6	16e	0.8314(2)	0.8331(4)	0.8329(7)	Zn1	4a	—	0	—
Li7	24f	0.1677(3)	0.1678(6)	0.170(1)

---

Concluding, the fractional atomic coordinates of all Li₁₇Ge₄ related phases listed in Table 3 are very similar and the scenario of an unoccupied 4a position in Li_16.95Ge₄ fits the calculated data of the corresponding Li₂₁Si₅ structure very well. This strengthens the existence of a homogeneity region Li_17−xTt₄ (0 < x < 0.2) which is deduced from the flexibility of the (Li1)₄(Tt₂)₄ tetrahedral star around the Wyckoff position 4a.⁹ Accordingly, the herein reported phase Li₁₇Ge₄ is regarded as the lithium-richest representative in the Li–Ge system.

The structure of Li₁₇Ge₄ is closely related to a 6 × 6 × 6 superstructure of the body centered cubic (bcc) structure.⁴⁶ As already shown by von Schnering and Nesper,⁴⁶ it can be easily interpreted by 26 atom clusters (M₂₆ with M = Tt, Li) centered at the special positions 4a–d whereas Ge₄ tetrahedra and Ge₆ octahedra (note that Ge atoms are isolated by distances larger than 4.44 Å) are situated around 4a, 4c and 4b, 4d, respectively, corresponding to the arrangement of Na and Tl atoms in the NaTl structure.⁴⁶

Yet another possible way of looking at the structure of Li₁₇Ge₄ is the comparison of GeLi_n coordination polyhedra and their arrangement in the unit cell. This has proven to be a neat method for the comparison of lithium-rich Li–Si phases solely bearing isolated Si atoms in their structures (Li₁₇Tt₄, Li_16.4Tt₄ and Li₁₅Tt₄).¹⁰ As shown in Fig. 4a, Ge1, Ge3 and Ge4 are 13-coordinated by Li atoms, whereas Ge2 attains a coordination number (CN) of 14. Corresponding to the first coordination shell of Ge atoms, Li–Ge distances range from 2.659(4) Å to 3.053(3) Å (Table 4). The second Li shell is clearly separated with distances larger than 4.2564(3) Å. Interestingly, the polyhedra around Wyckoff positions 4a, 4c and 4b, 4d either form supratetrahedra ([Ge1Li₁₃]₄ and [Ge2Li₁₄]₄ denoted as (1) and (2)) or supraoctahedra ([Ge3Li₁₃]₆ and [Ge4Li₁₃]₆ denoted as (3) and (4)), respectively (Fig. 4b). Accordingly, the structure of Li₁₇Ge₄ can be considered as two interpenetrating ccp lattices of (2) and (3), i.e. the NaCl structure, where tetrahedral voids are filled with (1) and (4).

Fig. 4 (a) GeLi_n coordination polyhedra (CN = coordination number) and (b) their relative arrangement in the cubic unit cell of Li₁₇Ge₄ (Ge = black; Li = white; thermal ellipsoids at 70% probability, single crystal data at room temperature). Supratetrahedra and supraoctahedra are formed from Ge1Li₁₃, Ge2Li₁₄ and Ge3Li₁₃, Ge4Li₁₃ coordination polyhedra (denoted as 1, 2, 3 and 4).

Table 4 Selected interatomic distances in Li₁₇Ge₄ (F4̄3m, Z = 20, T = 298 K, estimated standard deviations in parentheses)

Atom pair			d(Å)	Atom pair			d(Å)
Ge1	Li9	×3	2.659(4)	Ge3	Li10	×2	2.849(4)
	Li1		2.813(7)		Li3	×2	2.855(2)
	Li2	×3	2.884(1)		Li7		2.890(5)
	Li8	×3	2.898(3)		Li11	×4	2.977(2)
	Li12	×3	2.959(4)	Ge4	Li12	×2	2.680(3)
Ge2	Li12	×3	2.686(3)		Li11	×2	2.709(3)
	Li13		2.7244(3)		Li8		2.723(5)
	Li7	×3	2.734(3)		Li5	×2	2.769(4)
	Li6		2.780(6)		Li6	×2	2.856(2)
	Li10	×3	2.933(4)		Li10	×4	3.053(3)
	Li1	×3	2.969(4)	Li13	Li1	×4	2.397(7)
Ge3	Li9	×2	2.673(4)		Ge2	×4	2.7244(3)
	Li4	×2	2.760(3)		Li7	×6	3.162(5)

---

Besides Li₁₇Ge₄ and Li₁₅Ge₄, Li_16.38Ge₄ is yet another representative exclusively bearing isolated Ge atoms. It is isotypic with Li_16.42Si₄¹⁰ which possesses a peculiar structure involving occupational and positional disorder along the crystallographic a-axes (cf. Fig. 2). A convenient way of illustrating the disordered structure of Li_16.38Ge₄ is the assumption of a simplified ordered model which has already been applied for the description and band structure calculations of Li_16.42Si₄.¹⁰ In detail, the atom positions Li4A, Li4B and Li5A (cf. Table 2) affected by disorder corresponding to the Wyckoff positions 4c (0.616(8) occ.), 8g (0.384(8) occ.) and 8f (0.75(4) occ.), respectively, are either regarded as half (Li4A, Li4B) or fully occupied (Li5A) resulting in the composition Li_16.5Ge₄. An accordingly ordered model with fully occupied atom positions and crystallographically independent sites for Li4B′ and Li4B′′ (translate to Li4B in Cmcm) can be achieved by symmetry reduction and a cell enlargement (space group P2₁/m, a = 9.1016(4) Å, b = 13.2751(4) Å, c = 11.2744(4) Å, β = 101.643(1)°; for crystallographic details, see ref. 10). The GeLi_n polyhedra occurring in the respective model “Li_16.5Ge₄” are depicted in Fig. 5a.

Fig. 5 (a) GeLi_n coordination polyhedra occurring in an idealized model for Li_16.38Ge₄ with a composition of Li_16.5Ge₄ (space group P2₁/m, fully occupied and crystallographically independent atom positions Li4B′, Li4B′′ (both correspond to Li4B in Li_16.38Ge₄), Li4A, and Li5A); (b) stacking of GeLi_n polyhedra by sharing opposite faces resulting in parallel rods which run along the crystallographic a-axis (Ge = black, Li = white, thermal ellipsoids at 90% probability, single crystal data at 100 K). The polyhedra stacking in the structure model “Li_16.5Ge₄” is indicated by numbers X which correspond to the coordination polyhedra of atom GeX in (a).

Similar to Li₁₇Ge₄, the first shell of Li atoms surrounding each Ge atom is clearly separated from the second one with distances between 2.596(2) Å to 3.309(3) Å and distances above 4.103(4) Å (Table 5). Whereas Ge1 and Ge2 are permanently 13-coordinated (Ge1Li₁₃/Ge1′Li₁₃ and Ge2Li₁₃ denoted as (1)/(1′) and (2)), Ge3 attains coordination numbers of either 12 or 13 (Ge3Li₁₂ and Ge3′Li₁₃ denoted as (3) and (3′)). The different coordination of Ge3 is attributed to the varying occupation of atom positions Li4A, Li4B′ and Li4B′′. The arrangement of GeLi_n polyhedra in the unit cell of Li_16.38Ge₄ is achieved by stacking them into strands which proceed along the crystallographic a-axis (Fig. 5b). For the ordered model “Li_16.5Ge₄”, the stacking sequence is indicated by numbers corresponding to various GeLi_n polyhedra highlighted in Fig. 5a.

Table 5 Selected interatomic distances in Li_16.38Ge₄ (Cmcm, Z = 16, T = 123 K, estimated standard deviations in parentheses)

Atom pair			d(Å)	Atom pair			d(Å)
Ge1	Li7	×2	2.631(2)	Ge2	Li8	×4	3.127(1)
	Li5B	×2	2.64(2)	Ge3	Li4A		2.596(2)
	Li4B	×2	2.772(4)		Li7		2.603(2)
	Li9	×4	2.790(1)		Li8		2.693(2)
	Li2	×2	2.792(1)		Li6	×2	2.7188(9)
	Li5A	×2	2.854(8)		Li5B	×2	2.723(8)
	Li3		3.001(2)		Li5A	×2	2.747(3)
	Li4A	×2	3.309(3)		Li4B	×2	2.854(3)
Ge2	Li6	×2	2.647(2)		Li10	×2	2.875(1)
	Li3	×2	2.657(1)		Li1		2.896(1)
	Li10	×2	2.707(2)		Li9		2.932(2)
	Li2		2.753(2)		Li8		2.983(2)
	Li1	×2	2.867(2)

---

Comparing the TtLi_n coordination polyhedra of all lithium-rich Li–Tt phases, which exclusively comprise isolated Tt atoms in their structures, the coordination numbers consistently increase from CN = 12 (Li₁₅Tt₄), over CN = 12–13 (Li_16.4Tt₄) to CN = 13–14 (Li₁₇Ge₄).

Solid solutions Li₁₇Si_4−xGe_x [x = 2.30(2), 3.08(4), 3.53(3)]

Li₁₇Si₄ and Li₁₇Ge₄ are isotypic phases that form solid solutions. Single crystalline samples of Li₁₇Si_4−xGe_x were obtained from melt equilibration experiments and analyzed by single crystal and powder X-ray diffraction as well as energy dispersive X-ray spectroscopy. The Si–Ge ratios for Li₁₇Si_4−xGe_x samples obtained from single crystal X-ray diffraction data were x = 2.30(2), 3.08(4), and 3.53(3). We note that these values deviate from the initial ones x = 1.0, 2.0, and 3.0 corresponding to the employed melts “Li₉₀Si_7.5Ge_2.5”, “Li₉₀Si₅Ge₅” and “Li₉₀Si_2.5Ge_7.5” as the Si amount is reduced due to a partial reaction of Si with the stainless steel ampules; thus the Si–Ge ratios in the products are shifted toward higher Ge contents. As can be seen in Fig. 6, the cell axes and volumes (determined from PXRD patterns of the respective samples by Rietveld refinement, Fig. S2†) linearly increase with increasing Ge concentrations in Li₁₇Si_4−xGe_x revealing a perfect behavior obeying Vegard's law.⁴³ Additionally, results from EDX measurements agree well with the crystallographically determined Si–Ge ratios (impurities originating from the stainless steel ampule were not detected).

Fig. 6 Trend of cell volumes vs. Ge contents in Li₁₇Si_4−xGe_x (x = 0, 2.30(2), 3.08(4), 3.53(3), 4). Cell parameters were determined from respective PXRD patterns by Rietveld refinement (Fig. S2), and Ge contents were obtained from either single crystal X-ray diffraction data or EDX (error bars for a, V, and x_{single crystal} (in at%) are smaller than data point icons).

Analyzing the distribution of Si and Ge on atom positions Tt1–4, the Si–Ge ratios are very similar for Tt1, Tt3, and Tt4, whereas Tt2 on the Wyckoff position 16e (x, x, x) features a slight preference for Si (Table S1–4†). Comparing the coordination environment of Tt2 and Tt1, Tt3, Tt4, the former is 14- and the latter are 13-coordinated by Li atoms with similar Ge–Li distances (2.68 Å–2.95 Å vs. 2.65–3.04 Å). The phenomenon that different crystallographic sites are substituted differently is also known as the “coloring problem”.⁴⁸ In some cases the site preferences cannot be deduced on the basis of simple chemical reasoning (e.g. differences in electronegativities) and quantum chemical calculations may give a reasonable explanation.⁴⁹ Here, the focus is set on experimental work and we note that small differences in the distribution of Si and Ge on atom positions Tt2 and Tt1, Tt3, Tt4 were traced.

Thermodynamic stability of Li₁₇Ge₄ and Li_16.38Ge₄

The Li–Ge phase diagram was determined by Federov & Molochka²³ in 1966 and later revised by Grüttner²⁵ in his dissertation. However, a current compilation of the Li–Ge system⁵⁰ did not include Grüttner's results and, hence, significant information is missing. Therefore and due to the recent improvements of the Li–Si phase diagram (>76 at% Li),^8–10 a detailed redetermination of the respective portion of the Li–Ge system is essential.

The lithium-rich section of the Li–Ge phase diagram (>79 at% Li) was studied by DSC investigations of samples with systematically different compositions Li₁₇Ge₄, “Li_16.5Ge₄” and “Li₁₆Ge₄”. Accordingly, PXRD patterns correspond to pure-phase Li₁₇Ge₄, a mixture of Li₁₇Ge₄ and Li_16.38Ge₄, and a mixture of Li_16.38Ge₄ and Li₁₅Ge₄, respectively (Fig. 7).

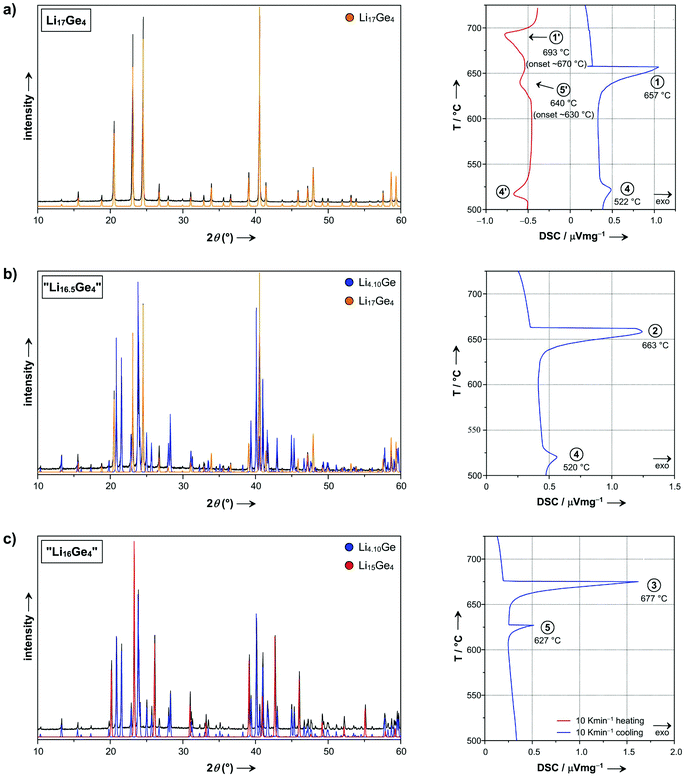Fig. 7 PXRD patterns and corresponding DSC thermograms of bulk samples (a) Li₁₇Ge₄, (b) Li_16.5Ge₄, and (c) Li₁₆Ge₄ (PXRD patterns: experimental = black, Li₁₇Ge₄ (calc.) = yellow, Li_4.10Ge (calc.) = blue, Li₁₅Ge₄ (calc.) = red; DSC thermograms: heating and cooling traces are shown in red and blue, respectively, a signal assignment is given in the Li–Ge phase diagram in Fig. 8b).

The thermograms of these samples are depicted in Fig. 7. The first thermal events in these cooling traces (signal 1–3) are assigned to the crossing of the liquidus boundary. Analogously to the Li–Si system,¹⁰ signals (4) and (5) at 520–522 °C and 627 °C are attributed to the peritectic formation temperatures of Li₁₇Ge₄ (481–486 °C for Li₁₇Si₄) and Li_16.38Ge₄ (618 °C for Li_16.42Si₄), respectively. This is additionally strengthened by our results from melt equilibration experiments (see above) where crystals of Li_16.38Ge₄ are only afforded above temperatures of 520 °C. Note that signal (5) corresponding to the peritectic formation of Li_16.38Ge₄ from melt and Li₁₅Ge₄ is superimposed by signals (1) and (2) in the cooling traces of Li₁₇Ge₄ and “Li_16.5Ge₄” (Fig. 7), respectively, but clearly visible for the “Li₁₆Ge₄” sample. Instead, it can be recognized at around 630 °C in the respective heating trace, exemplarily shown for Li₁₇Ge₄ (effect 5′). Furthermore, long term annealing experiments of “Li₁₆Ge₄” samples (Fig. S1 in the ESI†) established Li_16.38Ge₄ as a high-temperature phase being stable above ∼400 °C until 627 °C, just like Li_16.42Si₄ existing between 470 and 618 °C.¹⁰ We note that the decomposition behavior of Li_16.38Ge₄ is very sluggish and even harder to trace than in the case of its Si counterpart.

Finally, the recent results from DSC investigations are compiled in an updated Li–Ge phase diagram (Fig. 8b) which was revised from Grüttner's previously determined one shown in Fig. 8a. A comparison with the Li–Si phase diagram (Fig. 8c) reveals similarities to the Li–Ge system. An interesting difference is the stability of Li₁₅Tt₄. Whereas Li₁₅Si₄ is metastable and decomposes above ∼170 °C,⁸ Li₁₅Ge₄ is thermodynamically stable and melts congruently at 720 °C.²⁵ Furthermore, uncertainties regarding the isotherms at 610 °C and 618 °C in the Li–Si phase diagram¹⁰ could not be found for the Li–Ge system.

Fig. 8 (a) Excerpt of the Li–Ge phase diagram as reported from Grüttner²⁵ (the composition of the eutectic between Li₁₅Ge₄ and Li₁₃Ge₄ was not determined), (b) its revision based on DSC investigations and annealing experiments of “Li₁₆Ge₄” samples reported herein, and (c) the most recent Li–Si phase diagram for Li concentrations >76%.¹⁰

Conclusion

The germanides Li₁₇Ge₄ and Li_16.38Ge₄ were established as further representatives of the Li–Ge system. The latter is assigned a high-temperature phase which exists between ∼400 and 627 °C, the former, the lithium-richest Li–Ge phase, decomposes peritectically at 521 °C into melt and Li_16.38Ge₄. Li_16.38Ge₄ can be retained at room temperature if according melts are cooled to the respective temperature region and subsequently quenched. Both germanides are isotypic to the silicides Li₁₇Si₄ and Li_16.42Si₄ extending the family of isotypic lithium tetrelides to Li₁₇Tt₄, Li_16.4Tt₄, Li₁₅Tt₄, Li₁₄Tt₆, Li₁₂Tt₇, and LiTt (Tt = Si, Ge). The previously reported Li_16.95Ge₄ can be regarded as representative of a homogeneity region Li_17−xTt₄ (0 < x < 0.2) with Li₁₇Ge₄ and Li_16.8Ge₄ (Li₂₁Ge₅) as border phases only differing in the occupation of the Wyckoff position 4a. Moreover, the validity of Vegard's law for the solid solutions Li₁₇Si_4−xGe_x was confirmed and small differences in the distribution of Si and Ge to the four crystallographically independent atom positions were observed. Regarding the thermodynamic properties, the regions of stability for Li₁₇Tt₄ and Li_16.42Tt₄ are very similar. Interesting are also the thermodynamic and structural differences of lithium silicides and germanides. For instance, whereas Li₁₅Si₄ is a metastable phase, Li₁₅Ge₄ melts congruently at 720 °C. Further, the phases Li₉Ge₄ and Li₇Ge₁₂ are not known in the Li–Si system. In particular, the synthesis of a hypothetical phase Li₇Si₁₂ would be an intriguing field of research since it may serve as a precursor for a new allotrope of Si, just as was reported for Li₇Ge₁₂ and its mild oxidation to allo-Ge.^36,51

Experimental section

Synthesis

Starting materials were Li rods (99%, Rockwood-Lithium), Si powder (99.999%, Alfa Aesar) and Ge pieces (99.999%, Chempur). All steps of synthesis and sample preparation were carried out in a glove box (MBraun, Ar atmosphere, H₂O and O₂ levels <0.1 ppm). Ta and stainless steel ampules were thoroughly cleaned, heated to 1000 °C (Ta) or 800 °C (stainless steel) under dynamic vacuum (p < 1 × 10⁻³ mbar) for at least 2 h and transferred to the glove box. An all-glass Schlenk line supplied with Ar, which is dried over P₂O₅, molecular sieve and heated titanium sponge (T = 750 °C), was used for heating and handling under inert conditions.

Large single crystals of Li₁₇Ge₄ and Li_4.10Ge (Li_16.38Ge₄) (cf. Fig. 1) were obtained from equilibrating melts with compositions “Li₉₅Ge₅” and “Li₈₅Ge₁₅” at temperatures of 400 and 530 °C in Ta ampules (slow cooling from 700 °C at a rate of 5 K h⁻¹ followed by 48 hours dwelling at specified temperatures) and subsequent isothermal melt-centrifugation. Details of this procedure have already been described in ref. 9 and 52. Crystals of Li₁₇Si_4−xGe_x [x = 0, 2.30(2), 3.08(4), 3.53(3)] were grown analogously in stainless steel ampules from melts with compositions “Li₉₀Si₁₀”, “Li₉₀Si_7.5Ge_2.5”, “Li₉₀Si₅Ge₅” and “Li₉₀Si_2.5Ge_7.5” equilibrated at 450 °C.

Furthermore, elemental mixtures with compositions Li₁₇Ge₄, “Li_16.5Ge₄” and “Li₁₆Ge₄” with a total amount of 2.5 g each were loaded into Ta ampules which were sealed by arc welding inside the glove box. For achieving targeted compositions with sufficient precision, a batch size of 2.5 g was deemed appropriate to keep weighing errors minimal.^9,10 Subsequently, ampules were sealed in silica jackets under vacuum and annealed in a muffle furnace. The temperature was raised to 750 °C at a rate of 10 K min⁻¹ and held for 0.5 h followed by cooling to 500–550 °C at a rate of 10 K min⁻¹. After a dwell time of one hour, ampules were quenched in water and transferred back to the glove box. Obtained products were ground in agate mortars and characterized by powder X-ray diffraction (cf. Fig. 7).

Differential scanning calorimetry (DSC)

Differential scanning calorimetry was carried out with a Netzsch DSC 404 Pegasus apparatus. Cylindrical Nb crucibles (L = 15.0 mm, OD = 6.5 mm, ID = 5.0 mm) were thoroughly cleaned, heated to 1000 °C under dynamic vacuum (p < 1 × 10⁻³ mbar) for 2 h and transferred to an Ar-filled glove box. Crucibles were loaded with 30–50 mg of the sample (Li₁₇Ge₄, “Li_16.5Ge₄” and “Li₁₆Ge₄”). Subsequently, the open end was roughly closed by crimping and then sealed by arc-welding inside the glove box under cooling. A sealed Nb crucible without the sample served as a reference. For all measurements an Ar-flow of 60–70 mL min⁻¹ and a heating/cooling rate of 10 K min⁻¹ were used. Samples were recovered after the measurement inside an Ar-filled glove box. Data were handled with the program Proteus Thermal Analysis.⁵³

Annealing experiments

In order to further investigate the thermodynamic stability of Li_4.10Ge (Li_16.38Ge₄), batches of 100–150 mg of “Li₁₆Ge₄” bulk material were sealed in Ta ampules and annealed in a muffle furnace at 200, 400 and 510 °C (10 K min⁻¹ heating rate) for three days. Thereafter, ampules were quenched in water and transferred inside an Ar-filled glove box. Products were ground in agate mortars and subsequently characterized by powder X-ray diffraction (Fig. S1†).

Single crystal X-ray diffraction and structure determination

Crystals of Li₁₇Ge₄ and Li_4.096(4)Ge (Li_16.38(2)Ge₄) were handled in an Ar-filled glove box, selected under a microscope and sealed inside glass capillaries. For the best specimen, intensity data were collected at room temperature (Li₁₇Ge₄) and 123 K (Li_16.38(2)Ge₄) on a Bruker X-ray diffractometer equipped with a CCD detector (Apex II, κ-CCD), a fine-focused sealed tube with MoK_α radiation (λ = 0.71073 Å) and a graphite monochromator using the Bruker Apex2 software.⁵⁴ Integration, data reduction and absorption correction were done using SAINT and SADABS.^55,56 The space groups F4̄3m (Li₁₇Ge₄) and Cmcm (Li_16.38(2)Ge₄) were assigned on the basis of the systematic absence and the statistical analysis of the intensity distributions. For Li₁₇Ge₄, Friedel pairs were not merged since the assigned space group is non-centrosymmetric. The Flack⁵⁷ parameter was determined as 0.50(3). The structures were solved by direct methods (Shelxs-97⁵⁸) and refined with full-matrix least squares on F² (Shelxl-97⁵⁹). Difference Fourier maps F_o − F_c were calculated with Jana2006.⁶⁰ All refinement results are compiled in Table 6.

Table 6 Crystallographic data and structure refinement for Li₁₇Ge₄ and Li_4.096(4)Ge

a R 1 = ∑||Fo|−|Fc||/∑|Fo|. b wR2 = [∑w(Fo^2 − Fc^2)^2/∑w(Fo^2)^2]^1/2.
Empirical formula	Li17Ge4	Li4.096(4)Ge
T/K	298(2)	123(2)
Formula weight/g·mol^−1	408.34	101.01
Crystal size/mm^3	0.40 × 0.40 × 0.35	0.37 × 0.24 × 0.23
Crystal color	Metallic silver	Metallic silver
Crystal shape	Block	Bar
Space group	F4̄3m	Cmcm
Structure type	Li17Pb4	Li4.11Si
Unit cell dimension/Å	a = 18.8521(3)	a = 4.5511(2)
		b = 22.0862(7)
		c = 13.2751(4)
V/Å^3	6700.1(2)	1334.37(8)
Z	20	16
ρ (calc.)/g cm^−3	2.024	2.011
μ/mm^−1	8.832	8.860
F (000)	3580	709
θ Range/°	1.87–45.26	1.84–40.25
Index range hkl	−37 ≤ h ≤ +23	−6 ≤ h ≤ +8
	−37 ≤ k ≤ +30	−32 ≤ k ≤ +40
	−37 ≤ l ≤ +37	−24 ≤ l ≤ +19
Reflections collected	67 542	15 241
Independent reflections	2757 (Rint = 0.047)	2369 (Rint = 0.023)
Reflections with I > 2σ(I)	2547 (Rσ = 0.017)	1920 (Rσ = 0.017)
Data/restraints/parameter	2757/0/68	2369/0/82
Absorption correction	Multi-scan	Multi-scan
Goodness-of-fit on F^2	1.106	1.042
Final R indices [I > 2σ(I)]^a^,^b	R 1 = 0.018	R 1 = 0.015
	wR2 = 0.037	wR2 = 0.026
R indices (all data)^a^,^b	R 1 = 0.022	R 1 = 0.023
	wR2 = 0.038	wR2 = 0.027
Extinction coefficient	1.59(9) × 10^−4	1.52(7) × 10^−3
Flack parameter	0.50(3)	—
Largest diff. peak and hole/e Å^−3	0.46 and −0.69	0.69 and −0.52

---

Details of the single crystal X-ray structure determination, refinement data, fractional atomic coordinates and isotropic equivalent atomic displacement parameters for Li₁₇Si_4−xGe_x [(x = 2.30(2), 3.08(4), 3.53(3)] are given in Table S1–4 in the ESI.† Further data may be obtained from Fachinformationszentrum Karlsruhe, D-76344 Eggenstein-Leopoldshafen, Germany (fax: (+49)7247-808-666; e-mail: crysdata@fiz-karlsruhe.de) on quoting the depository numbers CSD-427231 (Li_4.10Ge (Li_16.38Ge₄)), CSD-427232 (Li₁₇Ge₄), CSD-427233 (Li₁₇Si_0.47Ge_3.53), CSD-427234 (Li₁₇Si_0.92Ge_3.08), and CSD-427235 (Li₁₇Si_1.70Ge_2.30).

Powder X-ray diffraction (PXRD)

PXRD patterns were recorded on a Stoe Stadi P diffractometer (Ge(111) monochromator for CuK_α1 radiation, λ = 1.54056 Å) equipped with a Dectris Mythen DCS 1 K solid state detector. Investigated samples were (i) single crystals of Li₁₇Si_4−xGe_x (x = 0, 2.30(2), 3.08(4), 3.53(3), 4), (ii) bulk samples of Li₁₇Ge₄, “Li_16.5Ge₄” and “Li₁₆Ge₄”, and (iii) samples of “Li₁₆Ge₄” annealed at different temperatures. These were thoroughly ground in agate mortars, sealed inside 0.3 mm glass capillaries and measured in a 2θ-range of 5–90° (PSD steps: 0.06–1.00°; time per step: 20–40 s).

Energy dispersive X-ray spectroscopy (EDX)

A Jeol-JSM 7500F scanning electron microscope equipped with an Oxford X-Max EDX analyzer with Mn as an internal standard was used for determining the Si–Ge ratios in Li₁₇Si_4−xGe_x [x = 2.30(2), 3.08(4), 3.53(3)]. Samples were handled inside an Ar-filled glove box and fixed on a graphite platelet which was mounted on an aluminum stub.

Acknowledgements

M.Z. thanks the Fonds der Chemischen Industrie for his fellowship, the authors thank K. Rodewald for EDX measurements.

References

1. M. N. Obrovac and L. Christensen, Electrochem. Solid-State Lett., 2004, 7, A93–A96.
2. T. D. Hatchard and J. R. Dahn, J. Electrochem. Soc., 2004, 151, A838–A842.
3. W. J. Zhang, J. Power Sources, 2011, 196, 13–24.
4. P. Limthongkul, Y. I. Jang, N. J. Dudney and Y. M. Chiang, Acta Mater., 2003, 51, 1103–1113.
5. P. Limthongkul, Y. I. Jang, N. J. Dudney and Y. M. Chiang, J. Power Sources, 2003, 119–121, 604–609.
6. B. Key, M. Morcrette, J. M. Tarascon and C. P. Grey, J. Am. Chem. Soc., 2011, 133, 503–512.
7. B. Key, R. Bhattacharyya, M. Morcrette, V. Seznec, J. M. Tarascon and C. P. Grey, J. Am. Chem. Soc., 2009, 131, 9239–9249.
8. M. Zeilinger, V. Baran, L. van Wüllen, U. Häussermann and T. F. Fässler, Chem. Mater., 2013, 25, 4113–4121.
9. M. Zeilinger, D. Benson, U. Häussermann and T. F. Fässler, Chem. Mater., 2013, 25, 1960–1967.
10. M. Zeilinger, I. M. Kurylyshyn, U. Häussermann and T. F. Fässler, Chem. Mater., 2013, 25, 4623–4632.
11. M. Zeilinger and T. F. Fässler, Acta Crystallogr., Sect. E: Struct. Rep. Online, 2013, 69, i81–i82.
12. D. Thomas, M. Abdel-Hafiez, T. Gruber, R. Hüttl, J. Seidel, A. U. B. Wolter, B. Büchner, J. Kortus and F. Mertens, J. Chem. Thermodyn., 2013, 64, 205–225.
13. P. Wang, A. Kozlov, D. Thomas, F. Mertens and R. Schmid-Fetzer, Intermetallics, 2013, 42, 137–145.
14. A. Debski, W. Gasior and A. Goral, Intermetallics, 2012, 26, 157–161.
15. S. Dupke, T. Langer, R. Pöttgen, M. Winter, S. Passerini and H. Eckert, Phys. Chem. Chem. Phys., 2012, 14, 6496–6508.
16. S. Dupke, T. Langer, R. Pöttgen, M. Winter and H. Eckert, Solid State Nucl. Magn. Reson., 2012, 42, 17–25.
17. A. Kuhn, P. Sreeraj, R. Pöttgen, H. D. Wiemhöfer, M. Wilkening and P. Heitjans, J. Am. Chem. Soc., 2011, 133, 11018–11021.
18. G. R. Goward, N. J. Taylor, D. C. S. Souza and L. F. Nazar, J. Alloys Compd., 2001, 329, 82–91.
19. C. S. Fuller and J. C. Severiens, Phys. Rev., 1954, 96, 21–24.
20. J. Graetz, C. C. Ahn, R. Yazami and B. Fultz, J. Electrochem. Soc., 2004, 151, A698–A702.
21. E. M. Pell, J. Phys. Chem. Solids, 1957, 3, 74–76.
22. G. I. Oleksiv, Probl. Rozvitku Prirodn. i Tochn. Nauk, Sb., 1964, 76–77.
23. P. I. Federov and V. A. Molochka, Izv. Akad. Nauk. SSSR, Neorg. Mater., 1966, 2, 1870–1871.
24. R. Nesper, Prog. Solid State Chem., 1990, 20, 1–45.
25. A. Grüttner, PhD thesis, University of Stuttgart, 1982.
26. A. Grüttner, R. Nesper and H. G. von Schnering, Acta Crystallogr., Sect. A: Cryst. Phys., Diffr., Theor. Gen. Cryst., 1981, 37, C161.
27. E. I. Gladyshevskii, G. I. Oleksiv and P. I. Kripyakevich, Kristallografiya, 1964, 9, 338–341.
28. Q. Johnson, G. S. Smith and D. Wood, Acta Crystallogr., 1965, 18, 131–132.
29. E. I. Gladyshevskii and P. I. Kripyakevich, Kristallografiya, 1960, 5, 574–576.
30. R. Nesper, Habilitation, University of Stuttgart, 1988.
31. V. Hopf, W. Müller and H. Schäfer, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1972, 27, 1157–1160.
32. V. Hopf, H. Schäfer and A. Weiss, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1970, 25, 653.
33. H. G. von Schnering, R. Nesper, J. Curda and K. F. Tebbe, Angew. Chem., 1980, 92, 1070.
34. E. Menges, V. Hopf, H. Schäfer and A. Weiss, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1969, 21, 1351–1352 . According to ref. 25, the structures of LiGe reported in ref. 22 and 34 are identical (only two different cell settings).
35. J. Evers, G. Oehlinger, G. Sextl and H. O. Becker, Angew. Chem., Int. Ed. Engl., 1987, 26, 76–78.
36. A. Grüttner, R. Nesper and H. G. von Schnering, Angew. Chem., Int. Ed. Engl., 1982, 21, 912–913.
37. U. Frank and W. Müller, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1975, 30, 313–315.
38. R. Nesper, J. Curda and H. G. von Schnering, J. Solid State Chem., 1986, 62, 199–206.
39. J. Evers, G. Oehlinger and G. Sextl, Angew. Chem., Int. Ed. Engl., 1993, 32, 1442–1444.
40. J. Evers and G. Oehlinger, Angew. Chem., Int. Ed., 2001, 40, 1050–1053.
41. F. Kiefer and T. F. Fässler, Solid State Sci., 2011, 13, 636–640.
42. Y. Hashimoto, N. Machida and T. Shigematsu, Solid State Ionics, 2004, 175, 177–180.
43. L. Vegard, Z. Phys., 1921, 5, 17–26.
44. C. Lupu, J. G. Mao, J. W. Rabalais, A. M. Guloy and J. W. Richardson, Inorg. Chem., 2003, 42, 3765–3771.
45. H. Axel, H. Schäfer and A. Weiss, Z. Naturforsch., B: Anorg. Chem. Org. Chem., 1966, 21, 115–117.
46. R. Nesper and H. G. von Schnering, J. Solid State Chem., 1987, 70, 48–57.
47. L. Lacroix-Orio, M. Tillard and C. Belin, J. Alloys Compd., 2008, 465, 47–50.
48. G. J. Miller, Eur. J. Inorg. Chem., 1998, 523–536.
49. N. Kazem, W. Xie, S. Ohno, A. Zevalkink, G. J. Miller, G. J. Snyder and S. M. Kauzlarich, Chem. Mater., 2014, 26, 1393–1403.
50. J. Sangster and A. D. Pelton, J. Phase Equilib., 1997, 18, 289–294 and references therein.
51. F. Kiefer, A. J. Karttunen, M. Döblinger and T. F. Fässler, Chem. Mater., 2011, 23, 4578–4586.
52. K. Puhakainen, M. Boström, T. L. Groy and U. Häussermann, J. Solid State Chem., 2010, 183, 2528–2533.
53. Netzsch Proteus Thermal Analysis, Version 4.8.2, Netzsch-Gerätebau GmbH, Selb, Germany, 2006.
54. APEX suite of crystallographic software, APEX 2 Version 2008.4, Bruker AXS Inc., Madison, WI, USA, 2008.
55. SAINT, Version 7.56a, Bruker AXS Inc., Madison, WI, USA, 2008.
56. SADABS, Version 2008/1, Bruker AXS Inc., Madison, WI, USA, 2008.
57. H. D. Flack, Acta Crystallogr., Sect. A: Fundam. Crystallogr., 1983, 39, 876.
58. G. M. Sheldrick, Shelxs-97 – Program for the Determination of Crystal Structures, University of Göttingen, Göttingen, Germany, 1997.
59. G. M. Sheldrick, Shelxl-97 – Program for Crystal Structure Refinement, University of Göttingen, Göttingen, Germany, 1997.
60. V. Petricek, M. Dusek and L. Palatinus, Jana 2006: The Crystallographic Computing System, Version 03/15/2013, Institute of Physics, Praha, Czech Rupublic, 2006.

---

Footnote

† Electronic supplementary information (ESI) available: Crystallographic data, refinement results, fractional atomic coordinates, and isotropic equivalent atomic displacement parameters for Li₁₇Si_4−xGe_x [x = 2.30(2), 3.08(4), 3.53(3)] (Table S1–4), comparison of experimental and computational relaxed fractional atomic coordinates for both Li₂₁Si₅ and Li₁₇Si₄ (Table S5), experimental fractional atomic coordinates for Li₁₇Si₄, Li₁₇Ge₄, Li_17−εZn_εGe₄, and Li_16.95Ge₄ (Table S6), comparison of experimental fractional atomic coordinates for Li_16.95Ge₄ and computational relaxed ones for Li₂₁Si₅ (Table S7), PXRD patterns of “Li₁₆Ge₄” samples annealed at various temperatures (Fig. S1), Rietveld refinement results for Li₁₇Si_4−xGe_x (x = 0, 2.30(2), 3.08(4), 3.53(3), 4) (Fig. S2). See DOI: 10.1039/c4dt00743c

---