Effect of lanthanum (La3+) doping on the structural and electrical properties of double perovskite Sr2NiMoO6
Abstract
To study the effect of La3+ doping on structural and electrical properties, a few compositions of the system Sr2−xLaxNiMoO6−δ (x = 0.02, 0.04, 0.06, 0.08, and 0.10) were synthesized via the citrate–nitrate route. Thermally activated electrical conductivity was observed in this system. The reduction of Mo6+ to Mo5+ was confirmed not only in the thermal analysis but also in the detailed XPS investigation of the samples. The structural behavior was probed by phase formation of the compositions confirmed in X-ray diffraction Rietveld analysis. The SEM images of the compositions indicated that a grain size of the order of a few micrometers was observed in this system. The highest conductivity was obtained for the composition with x = 0.04. The electrical behaviour of the system was explained in terms of the lattice strain, the impure phase present in the system and also in terms of the enhanced charge density 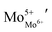 . The XPS and XRD analysis support the variation of conductivity behaviour with composition.
. The XPS and XRD analysis support the variation of conductivity behaviour with composition.


 Please wait while we load your content...
Please wait while we load your content...