Photosensitive microgels containing azobenzene surfactants of different charges†
Selina
Schimka
a,
Nino
Lomadze
a,
Maren
Rabe
a,
Alexey
Kopyshev
a,
Maren
Lehmann
b,
Regine
von Klitzing
b,
Artem M.
Rumyantsev
c,
Elena Yu.
Kramarenko
c and
Svetlana
Santer
*a
aInstitute of Physics and Astronomy, University of Potsdam, 14476 Potsdam, Germany. E-mail: santer@uni-potsdam.de
bInstitute of Chemistry, Technical University Berlin, 10623 Berlin, Germany
cFaculty of Physics, Lomonosov Moscow State University, Moscow, 119991, Russia
First published on 20th September 2016
Abstract
We report on light sensitive microgel particles that can change their volume reversibly in response to illumination with light of different wavelengths. To make the anionic microgels photosensitive we add surfactants with a positively charged polyamine head group and an azobenzene containing tail. Upon illumination, azobenzene undergoes a reversible photo-isomerization reaction from a trans- to a cis-state accompanied by a change in the hydrophobicity of the surfactant. Depending on the isomerization state, the surfactant molecules are either accommodated within the microgel (trans-state) resulting in its shrinkage or desorbed back into water (cis-isomer) letting the microgel swell. We have studied three surfactants differing in the number of amino groups, so that the number of charges of the surfactant head varies between 1 and 3. We have found experimentally and theoretically that the surfactant concentration needed for microgel compaction increases with decreasing number of charges of the head group. Utilization of polyamine azobenzene containing surfactants for the light triggered remote control of the microgel size opens up a possibility for applications of light responsive microgels as drug carriers in biology and medicine.
Introduction
Stimuli-sensitive microgel particles consisting of cross-linked polymers have received increasing attention due to their ability to encapsulate and/or release compounds upon controlled external triggers. This is related to the ability of microgels to undergo a volume transition in response to changes in pH, temperature, chemical composition of the solvent as well as application of light or static magnetic and electric fields.1–12 These properties make microgels a promising material class for applications in many scientific and industrial fields such as sensor design,13,14 optics, colloidal crystals,15 bio-medicine, or fabrication of pharmaceuticals, cosmetics, and food products.16–21In order to make microgels responsive to stimuli outlined above one typically modifies the microgels with functional groups or nanoparticles. For instance, one can incorporate semiconductor-, metal- or magnetic nanoparticles within the microgel, which in turn becomes responsive to optical or magnetic fields.22–26 Microgels made of, for instance, PNIPAM, PEO, PVCL or weak polyelectrolytes augmented with acidic or basic functional groups exhibit temperature or pH sensitivity.27–30
One of the most convenient external triggers for the remote control of microgel size is light. In order to make microgels photo-responsive one may exploit the photo-thermal effect. Here, a thermo-responsive microgel is modified with nanoparticles converting optical energy into heat in order to induce phase transition accompanied by a pronounced change in volume.31 Another possibility is to attach photosensitive groups such as spiropyran or azobenzene covalently to the microgel and make use of the light induced changes in polarity of the photosensitive groups, thus controlling the swelling behavior of the gel.32,33
Recently it was proposed to use azobenzene containing surfactants for rendering microgel particles photo-sensitive.34–37 The advantage of this approach is that any charged microgel particle can be made photo-responsive by immersing it into a solution with oppositely charged photosensitive surfactants. When azobenzene is incorporated into the hydrophobic tail of a surfactant molecule, the hydrophobicity of the whole surfactant can be switched by illumination with UV or blue light, between a more hydrophobic (trans-) and a rather hydrophilic (cis-) isomer.38 The physical mechanism of light responsive behavior of microgel particles can be described as follows.39 Microgel particles (for instance, negatively charged) are in a swollen state when they are dissolved in water at room temperature. Upon adding cationic surfactants, one can first achieve compaction because the surfactant diffuses into the particle, and replaces the counter ions at the charged groups of the gel. The counterions are subsequently expelled from the particle. The resulting drop in osmotic pressure induced by the micellization of surfactants within the gel in combination with screening of electrostatic interactions finally causes microgel particles to collapse and reduce their hydrodynamic radius significantly. Upon UV irradiation the surfactant molecules photo-isomerize to their cis-conformation and are thus rendered sufficiently hydrophilic to become non-aggregated and leave the particle, causing re-entering of counterions and re-swelling of the particle back to its initial size. This process can be reverted by stimulating fast back-relaxation to the trans isomer upon exposure to blue light. During this process, there is a fast transport of considerable amounts of surfactant molecules into and out of the particle.34,35,38
Here we propose to use azobenzene containing polyamine surfactants in order to realize light driven control of microgel particles. The microgel is based on N-isopropylacrylamide and co-monomer allyl acetic acid (NIPAM-AAA). The presence of two of these groups introduces thermo-responsive and pH-sensitive behavior of microgels.29,40–42 We work at neutral pH at which the microgels are found to be negatively charged. Their charge is distributed within and on the surface of the particles. Surfactants with polyamines as a head group are cationic and bear a valence depending on the number of amine groups and pH of the surroundings. In our study we utilize three molecules with 2, 3 and 4 amino groups in the surfactant head, which then acquires 1.5+, 2+ and 3+ charges at neutral pH, respectively. Synthetic polyamines are similar to the well-known natural compacting agent spermidine and have been used, e.g., in DNA compaction for transfection applications.41,43 Modified with azobenzene they have also recently been applied to achieve reversible compaction/decompaction of DNA molecules.44 Here we show experimentally and theoretically that negatively charged microgels can be shrunken by photosensitive polyamine surfactants as well. The critical concentration needed for a microgel to deswell increases with decreasing number of charges at the head group. The light-triggered remote control of the microgel size is achieved for all studied surfactants by periodic illumination with light of two different wavelengths: UV and blue. It is shown that upon irradiation it is possible to change the volume of the microgels by up to a factor more than 4 within only a few seconds. These unique properties of photosensitive microgels might be very attractive for biological applications, where the microgel can serve, for instance, as a container, which being in its compacted state, can deliver a substance and release it during subsequent decompaction triggered by irradiation with the appropriate wavelength.
Materials and methods
Synthesis of photosensitive azobenzene containing polyamines
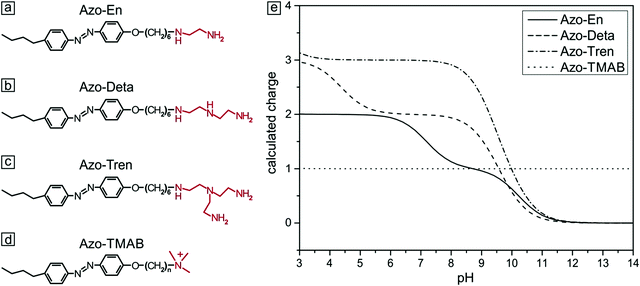
Cationic azo-polyamines were synthesized according to the published procedure in ref. 44. First, azocoupling of 4-butylaniline with phenol and a subsequent reaction with 1,6-dibromohexane were carried out, followed by quaternization with trimethylamine (Fig. 1d) or by the reaction with either ethylenediamine (Fig. 1a), diethylenetriamine (Fig. 1b) or tris(2-aminoethyl)amine (Fig. 1c). The resulting azobenzene containing polyamines differ in the number of amine groups in the head (2 to 4, Fig. 1).45 The polyamines were dissolved in water (Milli-Q) and kept in the dark for several days to ensure complete relaxation to the trans-state. The dependence of the amine charge on pH was calculated (ESI,† Fig. S1) from the Henderson–Hasselbach equation and local amine-pKa values were determined using Marvin Sketch.46 All experiments were performed at pH = 7, fixing the charge of Azo-En to 1.5, of Azo-Deta to 2.0 and of Azo-Tren to 3.0.Synthesis of the microgel
The microgel particles were synthesized as described elsewhere.36 In short, N-isopropylacrylamide (NIPAM, Aldrich, 97%), 5 mol% (with respect to the mass of NIPAM) of N,N′-methylenebisacrylamide (BIS, Aldrich, 99,5%), and 50 mol% (with respect to the mass of NIPAM) of the co-monomer allyl acetic acid (AAA, Aldrich, 97%) were dissolved in 100 mL Milli-Q water, heated to 70 °C under a constant nitrogen stream. Polymerization was initiated by the addition of 1 mg KPS. After synthesis the microgel was dialyzed for 14 days with Milli-Q water to remove oligomers and excess educts, and freeze-dried.Photoisomerization behavior of polyamines
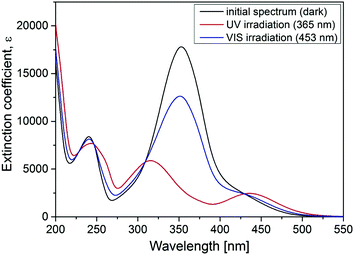
The photoisomerization behavior of the polyamines was found to be similar to a previously described cationic surfactant (azobenzene-containing trimethylammonium (TMAB), Fig. 1). This was expected since they share the same azobenzene containing tail.47–49 The trans isomer of all compounds discussed in this work has a characteristic absorption band (π–π* transition) with a maximum at 351 nm. The spectrum of the cis isomer is characterized by two absorption bands with maxima at 313 nm (π–π* transition) and at 437 nm (n–π* transition).Sample preparation
The microgel and the azobenzene containing surfactants were dissolved in de-ionized water (Milli-Q) to obtain initial solutions with concentrations of 2 × 10−5 M and 2 × 10−4 M, respectively. Both polyamines and microgel solutions were diluted prior to mixing to obtain a certain value of the molar ratio, MR, determined by the ratio between the total concentration of polyamine [Polyamine] and carboxylic groups [AAA]: MR = [Polyamine]/[AAA]. The concentrations of polyamine surfactants were kept far below their CMC, which is 0.11 mM, 0.14 mM, 0.18 mM for Azo-En, Azo-Deta, Azo-Tren, respectively. The CMC was determined using the Wilhelmy plate method (Kibron-Tensiometer, Kibron MicroTroughXL) as described in the ESI,† Fig. S4. The ionic strength was kept constant at 5 mM NaCl. All preparations and measurements in solution were carried out at 20 °C and under yellow light to avoid unwanted isomerization of the photosensitive substances.Preparation of samples for SEM measurements
SEM samples were prepared by pipetting microgel/polyamine solution on a silicon wafer followed by drying under ambient conditions.Methods
UV-Vis spectra were recorded using a Cary 5000 UV-Vis-NIR spectrophotometer (Varian Inc.) in quartz cuvettes with 10 mm length of the optical pathway.The dynamic light scattering (DLS) characterization of the microgel-surfactant complexes was performed in micro UV-Cuvettes using a Zetasizer Nano ZS (Malvern Instruments Ltd) at a scattering angle of 173°.
Electron micrographs were acquired using a transmission electron microscope, type JEM-1011 (JEOL Ltd, Japan) and a SEM device (Ultra Plus, Zeiss, Germany).
The lamps (VL-4.L, Vilber Lourmat, 365 nm, I = 1.2 mW cm−2; LED Spot Luxeon Royal Blue, P453E-PR09, 453 nm, 7 mW cm−2, Conrad Electronic) were used for the irradiation of the samples, at a duration of typically 10 min to guarantee the steady state behavior of the surfactant molecules.
An inverted optical microscope (Olympus IX71) equipped with two lasers (Samba 532 nm, Cobolt, Sweden; and 355 nm, Genesis CX, Coherent Inc., USA) is used to acquire images at a speed of 1 frame per second.
Theoretical considerations
Following ref. 39, the microgel is assumed to consist of ν flexible subchains each containing N monomer units. Some fraction f of the monomer units is ionic, so that both the total number of charges on the network and the number of microgel counterions equal νNf. Microgel swelling is described by the dimensionless swelling ratio α = R/R0, which is the ratio between the equilibrium microgel radius R, and the microgel radius R0 in the reference state. The reference state implies Gaussian coil conformations of the network subchains such that R0 = aN1/2ν1/3, a being a monomer unit size. The microgel concentration in solution is defined by the average distance between the centers of adjacent particles 2Rout = 2R0/γ with a dimensionless parameter γ ≪ 1 for diluted solutions.If δ is the charge of the surfactant ion and all other charged species in the system (microgel ionized groups, microgel and surfactant counterions) carry unit charges, the total number of surfactant molecules in the solution is ZfNν/δ; δ can assume fractional values in the case of pH-dependent surfactants. As in ref. 39, we use a two-zone model to account for the redistribution of ions between the microgel interior and the outer solution, resulting in the uncompensated microgel charge of Q/e = νNf(1 − β + sZ − tZ), where e is the elementary charge while s, β and t denote the fractions of surfactant molecules, network counterions and surfactant counterions, respectively, that are kept within the microgel. Thus, the charge binding ratio equals Zs.
We restrict our considerations to the case of relatively low surfactant concentration, not exceeding the CMC in the outer solution. Nevertheless, under these conditions micelle formation inside the microgel is still possible due to a reduction of the CMC within the oppositely charged polyelectrolyte network, as compared to a pure surfactant solution.39,50,51 This effect can most likely be attributed to the neutralization of an excess micelle charge by the oppositely charged microgel subchains so that surfactant counterions, neutralizing the micellar charge in the outer solution, are now able to leave the micelle vicinity gaining extra translational entropy.39
The free energy of the solution per microgel is given by:
| Ftot = Fel + Fel–st + Fnctr + Fsctr + Fint + Fstr + Fagg | (1) |
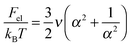 | (2) |
 | (3) |
The terms Fnctr and Fsctr are responsible for the translational entropy of the microgel and the surfactant counterions, which are regarded as ideal gases both inside and outside the microgel:
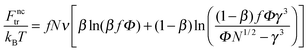 | (4) |
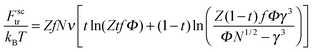 | (5) |
The free energy of van der Waals' interactions is written in the form of a virial expansion
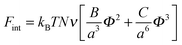 | (6) |
Finally, the last two terms are the surfactant contributions to the free energy. Since surfactant molecules are able to aggregate within the microgel, the last term corresponding to the aggregation energy
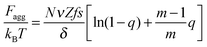 | (7) |
 | (8) |
 | (9) |
Results and discussion
To study the influence of photosensitive surfactants on the microgel compaction we synthesized a set of azobenzene containing molecules with different numbers of amine groups (Fig. 1a–c). The valence of charges on the head group depends on the pH. At pH 7 for instance, Azo-En, Azo-Deta, Azo-Tren have 1.5+, 2+ and 3+ charges per molecule (Fig. 1e). In this paper, for comparison we also discuss the cationic surfactant (azobenzene-containing trimethylammonium bromide) (Azo-TMAB) (Fig. 1d), the interaction of which with the microgels was published elsewhere.35 This surfactant carries 1 positive charge per molecule.All the four surfactants discussed here have similar UV-Vis absorption spectra (Fig. 2 shows the spectrum of Azo-Deta). The absorption spectra depend on the pH value (see ESI†, Fig. S1). For the present study, however, the pH was fixed at a value of 7. The trans isomer of all discussed compounds has a maximum adsorption at 351 nm, while the cis isomer is characterized by two absorption bands at 313 nm and at 437 nm (Fig. 2). The irradiation of the cis-isomers with blue light does not completely return the surfactant into its initial dark state (Fig. 2, blue curve). It was found that after irradiation with λ = 453 nm for 10 minutes ca. 66% of all the molecules are converted to the trans-state,35 while after irradiation with UV-light only 12% of the trans-isomer was detected following the absorption at 376 nm. The lifetime of the cis isomer in water is ∼72 hours; therefore, the spectra do not change considerably after the irradiation is turned off (see ESI,† Fig. S2). The transition from trans- to cis- and back to the trans-state triggered by irradiation with UV (λ = 365 nm) and blue (λ = 453 nm) light, respectively, takes place within only a few seconds.
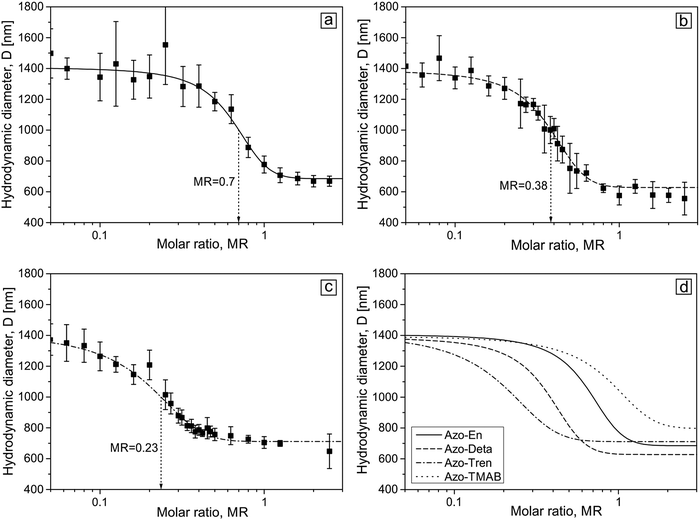
The complexes between the microgel particles and polyamine surfactants were prepared by mixing a water solution of both substances at a certain molar ratio. For this, the microgel concentration was kept constant (two concentrations were used in this study, their values expressed in concentrations of [AAA] units in the solution are 1 × 10−5 M and 5 × 10−6 M), while the surfactant concentration was varied between 5 × 10−7 M and 2 × 10−4 M. Fig. 3 shows the dependence of the hydrodynamic diameter of the microgel on the molar ratio between a total concentration of the polyamine surfactant [Polyamine] and the carboxylic groups [AAA] of the microgel defined as MR = [Polyamine]/[AAA].
When dissolved in water the microgel is in a swollen state with a hydrodynamic diameter of (1.4 ± 0.2) μm (Fig. 3). At a low surfactant concentration, the microgel is still in its swollen state up to some critical concentration at which contraction commences. For all the four surfactants the character of the size change is similar: with increasing surfactant concentration the size of the microgel decreases. Depending on the number of charges on the head group the contraction starts at varying molar ratio MR. A small amount of surfactant is needed to initiate the shrinkage of the microgel in the presence of molecules carrying 3 positive charges (Azo-Tren, Fig. 3c and d), while larger amounts of surfactant are required for 1.5 (Azo-En) and 2 (Azo-Deta) positive charges in the head group to induce the onset of microgel contraction. A further increase in the concentration results in a gradual decrease of the particle size down to (700 ± 100) nm at which the saturation of the microgel contraction occurs (Fig. 3). Also the onset of the saturation value depends on the valence of the surfactant head group: more surfactant is needed at smaller valences. The results for the Azo-TMAB surfactant interacting with the microgel are shown in Fig. 3d for comparison. A Boltzmann fit is applied to analyze the microgel size change with the inflection points of 0.23, 0.38, 0.70 and 1.10 for Azo-Tren, Azo-Deta, Azo-En and Azo-TMAB, respectively (Fig. 3d). These results indicate that with increasing number of charges on the surfactant head group smaller concentrations are required in order to induce the shrinkage of the microgel. The microgel-surfactant complexes are colloidal stable in the concentration range presented here.
Similar behavior is observed for smaller microgel concentration, c = 5 × 10−6 M (see ESI,† Fig. S3). For the case of smaller concentrations, the inflection point for all surfactants shifts to a larger molar ratio. The fact that in more concentrated solutions microgel collapse occurs at lower values of MR is fully supported by the theoretical calculations.39 An explanation is a higher entropy loss of surfactant molecules under their accumulation within the microgel at lower microgel concentrations (i.e. lower values of γ).39
To examine the impact of the ionic surfactant charge on the swelling behavior of the microgel, we modify the theoretical approach developed by us earlier for monovalent surfactants.39 A short description of the theory is presented in the Materials and methods section.
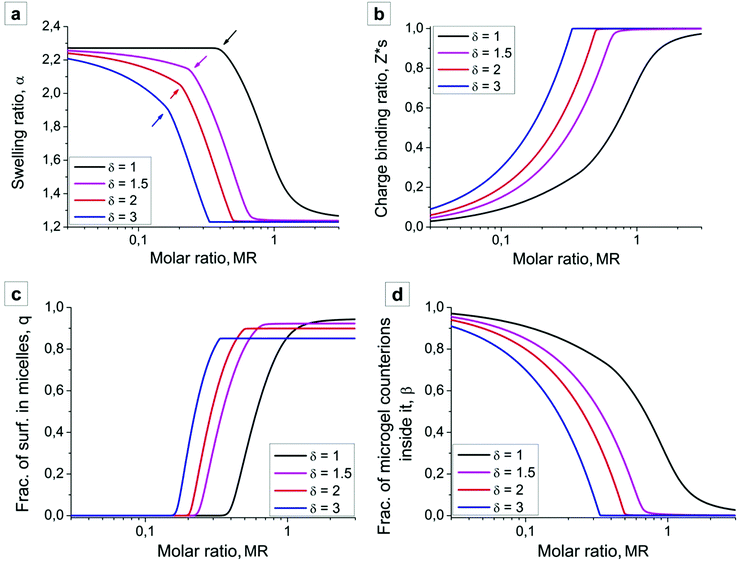
In the absence of surfactant molecules microgel swells due to the osmotic pressure generated by the counterions, and the swelling ratio is given by 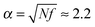 (see Fig. 4a, low values of the MR). Surfactant addition causes microgel contraction, and each swelling curve of the microgel (Fig. 4a) can be divided into three regions, depending on the fractions of the aggregated surfactant within the microgel (Fig. 4c). In the first region there is no micelle formation within the microgel, q = 0, and its contraction takes place only at δ > 1. In the course of ion exchange δ-valent surfactant ions substitute δ “native” counterions of the network (Fig. 4d) with their release to the outer solution, and thus some gain in translational entropy. Consequently, the amount of mobile ions within the microgel decreases, exerting osmotic pressure drops and microgel volume decays. The higher the value of δ, the steeper the microgel volume diminution. Microgel volume remains unchanged at δ = 1 because of (i) the constant total number of mobile ions (both “native” network counterions plus surfactant ions) within the network and (ii) low surfactant concentration outside the microgel. Indeed, the concentration of ions outside the microgel is manifold (approx. 1/Zγ3 times) lower than that inside it, so that the Donnan salting out effect is negligible.51
(see Fig. 4a, low values of the MR). Surfactant addition causes microgel contraction, and each swelling curve of the microgel (Fig. 4a) can be divided into three regions, depending on the fractions of the aggregated surfactant within the microgel (Fig. 4c). In the first region there is no micelle formation within the microgel, q = 0, and its contraction takes place only at δ > 1. In the course of ion exchange δ-valent surfactant ions substitute δ “native” counterions of the network (Fig. 4d) with their release to the outer solution, and thus some gain in translational entropy. Consequently, the amount of mobile ions within the microgel decreases, exerting osmotic pressure drops and microgel volume decays. The higher the value of δ, the steeper the microgel volume diminution. Microgel volume remains unchanged at δ = 1 because of (i) the constant total number of mobile ions (both “native” network counterions plus surfactant ions) within the network and (ii) low surfactant concentration outside the microgel. Indeed, the concentration of ions outside the microgel is manifold (approx. 1/Zγ3 times) lower than that inside it, so that the Donnan salting out effect is negligible.51
In the second region, i.e. at intermediate values of MR, an intense micelle formation within the microgel sets in, Fig. 4a. Arrows in Fig. 4a point at the sharp bend in swelling curves corresponding to the border between the first and the second regimes. Since micelles containing m ≫ 1 surfactants almost do not create osmotic pressure, microgel volume in this region rapidly goes down until all network counterions are substituted by surfactants. Microgel volume reaches values close to that of a neutral counterpart.
In the third region further surfactant addition hardly influences both the microgel dimensions and the internal structure; swelling ratio α (Fig. 4a) and the fraction of aggregated surfactants q (Fig. 4c) remain unchanged.
The results of our theoretical analysis coincide quite well with experimental observations. First, increasing surfactant charge leads to a shift of the swelling curves and microgel contraction at lower values of the surfactant molar ratio. Second, the shapes of the experimental and theoretical swelling curves (Fig. 3 and 4a) qualitatively coincide: in the first region microgel volume is almost unchanged in the solution of the univalent Azo-TMAB surfactant (δ = 1), while microgel contraction in the presence of Azo-Deta and Azo-Tren surfactants takes place even at their low concentrations, Z < 0.2. Finally, the theory containing the only adjusting parameter ΔF reproduces quantitatively not only the range of microgel contraction but also its amplitude: in the collapsed state the microgel radius is twice lower than that in the swollen state.
Assumption on the equal values of ΔF, i.e. equal CMCs of all surfactants, was adopted in order to reveal the effect of the surfactant charge rather than surfactant hydrophobicity. In fact, the CMC in water increases from 0.1 mM for Azo-TMAB to 0.18 mM for Azo-Tren as measured experimentally using the Wilhelmy plate (see ESI,† Fig. S4). However, it should be primarily ascribed to the repulsion of charged head groups of the surfactant in the water, while in the microgel interior it is largely neutralized by oppositely charged network subchains, and the identical hydrophobic moiety of all surfactants makes our presumption on the equal values ΔF rather justified.
Light driven remote control of the microgel size
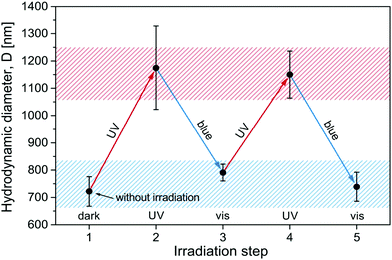
Since the surfactant molecules are photosensitive, the contraction of the microgel can be altered by illumination. To realize the light-induced reversible swelling/shrinkage process a sample in the compaction range with MR = 0.60 (Azo-Deta) was exposed periodically to irradiation with UV and blue light. The light-induced changes in microgel hydrodynamic diameter are shown in Fig. 5. One observes a completely reversible contraction/swelling transition of the microgel triggered by light. The change in the diameter of a microgel particle between ∼700 nm and 1200 nm corresponds to more than a four-fold change in volume.We should mention that the hydrodynamic diameter of the light induced decompacted state (1200 nm) is smaller than that of the swollen particles without surfactants (1400 nm). This is related to the fact that surfactants act as the salt suppressing gel swelling in accordance with the Donnan equilibrium and not all of them undergo photo-isomerization to the cis-state. In a previous publication35 we have found that under illumination with UV light ca. 12% of the surfactants are in the trans-state. At an overall molar ratio, MR = 0.60, the specific molar ratio of the trans-isomers is MRUVeff = 0.07 (MReff = 0.60 × 12%). This value of MR corresponds to the range of a gradual decrease in the microgel size (Fig. 3b).
Using the same argument we estimate that under irradiation with blue light the effective concentration of trans-isomers is MRblueeff = 0.42 (MRblueeff = MRUVeff + MRcis 66%, where MRcis = 0.60 − 0.07 = 0.53), corresponding to the range of incomplete contraction where the microgel diameter is ca. 800 nm.
A similar behavior was found for all surfactants studied here (see ESI,† Fig. S5). Only in the case of Azo-Tren with three charges the light induced swelling of the microgels is smaller than for other surfactants (ca. 1000 nm, Fig. S5c, ESI†). This phenomenon is discussed below.
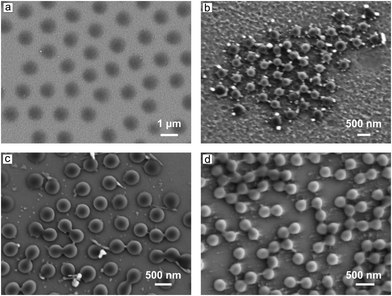
Since the change in the size of the micorgels is significant, one can easily observe it microscopically. Fig. 6 shows the SEM micrographs of the microgels (Azo-Deta complexes at different Z values and under irradiation conditions). At MR = 0.1, the microgels are in a swollen state (see for comparison Fig. 3b) with a diameter of D = 1200 nm (Fig. 6a). The diameter was measured as the average distance between the microgel particle centers. With increasing MR the diameter decreases down to a value of ca. 330 nm (Fig. 6b, MR = 2). Under irradiation with UV light, the particles swell again and the diameter increases to 440 nm (Fig. 6c shown for MR = 1), while upon irradiation with blue light, the diameter drops down to 300 nm (Fig. 6d).
The microgel particles were adsorbed on a silicon wafer from solutions of certain values of MR after irradiation with either UV or blue light and dried prior to measurement. Therefore, one cannot directly compare the diameter of the particles acquired from SEM micrographs and DLS measurements, however, the qualitative trend is similar in both cases, i.e. the diameter decreases for a larger value of MR, and increases upon UV irradiation.
The swelling/shrinkage process can directly be observed with optical microscopy (Fig. 7).
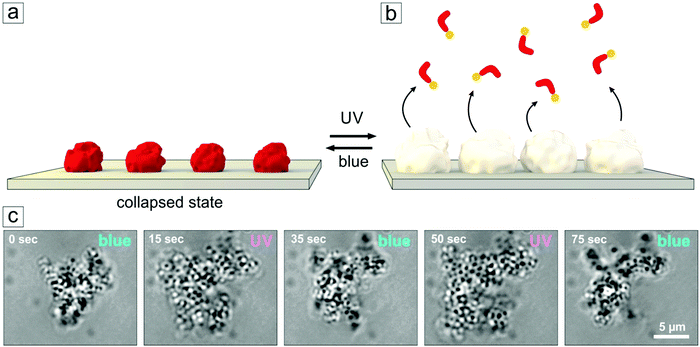 | ||
| Fig. 7 (a) Schematic view of the adsorbed microgel particles loaded with photosensitive surfactants. (b) Upon UV irradiation, the surfactants leave the microgel, particles assume a swollen state. (c) Snapshots of the swelling/deswelling process (video recording available in the ESI,† Fig. S7). Here a cluster of microgel particles is attached to a glass surface and shows a significant and fast change in volume in response to alternating exposure to UV and blue irradiation. | ||
Tracking the diameter change of the single microgel particles using an optical microscope is a difficult task, since the particles are small, mobile and transparent. But one can see the change in the size and shape of physisorbed aggregates consisting of lumps of microgel particles (Fig. 7). Here we make use of aggregate formation that occurs in aged solutions. Those containing photosensitive surfactants (shown for Azo-TMAB, at MR = 3) were irradiated periodically with UV and blue light within the setup of the optical microscope. Significant and reversible changes in the size of the aggregate could then be detected while changing the illumination conditions (Fig. 7).
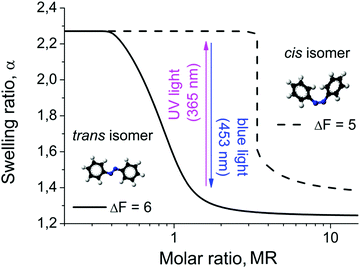
The light driven compaction/decompaction process within the microgels loaded with photosensitive surfactants can also be described within the theoretical model discussed above. The surfactant hydrophobicity is included through the free energy gain upon micelle formation ΔF. In Fig. 8 we plot the swelling curves calculated for two different values of ΔF. With increasing ΔF, i.e. with increasing hydrophobicity of the surfactant tail, the surfactant concentration required for microgel shrinkage decreases (Fig. 8). This is related to the fact that the CMC reduces with the increase of surfactant hydrophobicity. Since our model of microgel shrinkage is based on the drop of osmotic pressure during the formation of micelles, the reduction of the CMC with increasing hydrophobicity results in a shift of the transition region between the swollen and collapsed state toward smaller surfactant concentrations. The hydrophobicity of the surfactant can be altered by irradiation, i.e. in the trans-state the surfactant is more hydrophobic than in the cis-state. According to ref. 52–55, the ratio between the CMCs of cis- and trans-isomers isomers of the same azobenzene-containing surfactants varies over a broad range from ca. 2 to 20 depending on the surfactant structure and solution temperature. Since the CMC inside the microgel is estimated as exp(−ΔF − 1),38 the difference between free energy gains under micellization for trans- and cis-isomers is 0.7 ≤ (ΔFtrans − ΔFcis) ≤ 3. Thus, at the same absolute value of the surfactant concentration there is a range of Z at which the microgel is swollen in the presence of cis-isomers, and shrunken when loaded with trans- (Fig. 8).
In other words, under irradiation with UV light the micelles consisting of trans-isomers are disturbed, since trans- goes to the cis-state. The more hydrophilic cis-isomers leave the microgel, resulting in an uptake of counterions and thus the swelling of the particle. Upon irradiation with blue light, the cis-isomers photo-isomerise to the trans-conformation, thus diffusing back into the microgel and aggregating into micelles therein. At a certain concentration collapse of the microgel occurs. The process can be reversed by changing the irradiation wavelength. The change of the microgel size upon consequent UV and blue light irradiation decreases with increasing charge of the surfactant head group (see Fig. S5 and S6, ESI†). This tendency is especially pronounced for the case of the Azo-Tren surfactant. A probable reason for this behavior is the following. As we discussed above, multivalent ions induce the contraction of the microgel at smaller surfactant concentrations and the extent of the gel contraction is higher because with multivalent ions the drop in osmotic pressure within microgels becomes considerable even without surfactant micellization. The higher the value of the surfactant charge δ, the more counterions are released into the solution, and thus the gel adopts more shrunken conformations. As a result, the CMC within the gel is reached earlier (at a smaller molar ratio MR). Thus, if we choose (MR) in the region where the microgel with a single-charge surfactant is close to the transition between swollen and shrunken states (see Fig. S6a, ESI†), a small change in the surfactant hydrophobicity can induce a considerable change in the swelling ratio resulting from the reversible formation and destruction of micelles within the gel. At the same (MR) the microgel with a triple-charge surfactant is already far from the transition and switching between trans- and cis-isomers could only slightly change the amount of aggregated surfactants, thus, the variations in the gel volume upon irradiation are smaller. Moreover, the gel volume after blue light irradiation hardly depends on the surfactant charge (see Fig. S5 and S6, ESI†) because micelles comprising trans-form surfactants scarcely create exerting osmotic pressure within the gel, so that the amplitude of volume changes is governed by the gel volume after UV irradiation when cis-isomers are in the non-aggregated state.
Conclusions
We studied the interaction between anionic microgel particles and azobenzene-containing cationic surfactants. All surfactants considered in this work share the same type of hydrophobic tail, the incorporated photosensitive group and differ only in the head group, which can acquire average charges of 1+, 1.5+, 2+ and 3+. The presence of the azobenzene group in the hydrophobic tail allows for photo-induced switching of the hydrophobicity of the surfactant. It was shown that upon increasing the surfactant concentration the microgel undergoes a transition from a swollen to a collapsed state. The larger the number of charges at the head group, the smaller the surfactant concentration required to trigger the transition. This observation has been supported/explained theoretically. Two consequent stages of microgel shrinking, corresponding to the absence and presence of micelle formation within the microgel, were shown up. Indeed, at a low surfactant concentration surfactant absorption by the microgel appears as a result of an ion exchange between counterions initially trapped within the microgel and surfactant ions. At that, the microgel volume remains unchanged in the solution of a univalent surfactant while goes down in the event of multivalent ions due to moderate osmotic pressure diminution. Increasing the surfactant concentration results in the formation of micelles within the microgel at which most of the counterions are expelled from the interior and the microgel charges are largely compensated by micelles. At this stage the osmotic pressure drops significantly, and the microgel undergoes a transition to a collapsed state in the solutions of both univalent and multivalent surfactants. The concentration, at which this two-stage transition occurs, decreases with increasing number of charges in the head group since more counterions can be replaced by one surfactant molecule at once.During irradiation, the hydrophobicity of the surfactant tail is switched between a hydrophilic state (UV irradiation) and hydrophobic one (blue irradiation). The hydrophilic surfactant allows the microgel and the particles to swell, while the interaction of trans-isomers with the microgel results in its contraction. This process is completely reversible and can be carried out many times.
The light triggered reversible contraction/swelling behavior of the microgel induces a microscopic osmotic loading of the particles with surrounding solution, an effect which might be utilized for many applications such as artificial muscles, adaptive micro-fluidic devices and drug delivery systems.
Acknowledgements
This research is supported by the International Max Planck Research School on Multiscale Bio-Systems (IMPRS), Potsdam, German-Russian Interdisciplinary Science Center (G-RISC), Germany and Russian Foundation for Basic Research. M. L. and R. v. K. acknowledge the German Research Council (DFG) for financial support (KL 1165/12).References
- S. Nayak and L. A. Lyon, Angew. Chem., Int. Ed., 2005, 44, 7686–7708 CrossRef CAS PubMed.
- M. Das, H. Zhang and E. Kumacheva, Annu. Rev. Mater. Res., 2006, 36, 117–142 CrossRef CAS.
- L. A. Wells and H. Sheardown, Eur. J. Pharm. Biopharm., 2007, 65, 329–335 CrossRef CAS PubMed.
- H. Dou and M. Jiang, Polym. Int., 2007, 56, 1206–1212 CrossRef CAS.
- D. Klinger and K. Landfester, Soft Matter, 2011, 7, 1426–1440 RSC.
- Y. Wang, J. Nie, B. Chang, Y. Sun and W. Yang, Biomacromolecules, 2013, 14, 3034–3046 CrossRef CAS PubMed.
- W. Richtering and A. Pich, Soft Matter, 2012, 8, 11423 RSC.
- J. B. Thorne, G. J. Vine and M. J. Snowden, Colloid Polym. Sci., 2011, 289, 625–646 CAS.
- A. Pich and W. Richtering, Adv. Polym. Sci., 2011, 234, 1–37 CrossRef.
- for instance review: M. Motornov, Y. Roiter, I. Tokarev and S. Minko, Prog. Polym. Sci., 2010, 35, 174–211 CrossRef CAS.
- K. Gawlitza, C. Wu, R. Georgieva, D. Wang, M. B. Ansorge-Schumacher and R. von Klitzing, Phys. Chem. Chem. Phys., 2012, 14, 9594–9600 RSC.
- C. Scherzinger, O. Holderer, D. Richter and W. Richtering, Phys. Chem. Chem. Phys., 2012, 14, 2762–2768 RSC.
- I. Tokarev, I. Tokareva, V. Gopishetty, E. Katz and S. Minko, Adv. Mater., 2010, 22, 1412–1416 CrossRef CAS PubMed.
- M. Karg, I. Pastoriza-Santos, J. Pérez-Juste, T. Hellweg and L. M. Liz-Marzán, Small, 2007, 3, 1222–1229 CrossRef CAS PubMed.
- J. D. Debord and L. A. Lyon, J. Phys. Chem. B, 2000, 104, 6327–6331 CrossRef CAS.
- M. Hamidi, A. Azadi and P. Rafiei, Adv. Drug Delivery Rev., 2008, 60, 1638–1649 CrossRef CAS PubMed.
- N. A. Peppas and W. Leobandung, J. Biomater. Sci., Polym. Ed., 2004, 15, 125–144 CrossRef CAS PubMed.
- A. M. Kloxin, A. M. Kasko, C. N. Salinas and K. S. Anseth, Science, 2009, 324, 59–63 CrossRef CAS PubMed.
- T. Dvir, M. R. Banghart, B. P. Timko, R. Langer and D. S. Kohane, Nano Lett., 2010, 10, 250–254 CrossRef CAS PubMed.
- M. A. Moses, H. Brem and R. Langer, Cancer Cell, 2003, 4, 337–341 CrossRef CAS PubMed.
- D. A. LaVan, D. M. Lynn and R. Langer, Nat. Rev. Drug Discovery, 2002, 1, 77–84 CrossRef CAS PubMed.
- M. Das, S. Mardyani, W. C. W. Chan and E. Kumacheva, Adv. Mater., 2006, 18, 80–83 CrossRef CAS.
- B. Brugger and W. Richtering, Adv. Mater., 2007, 19, 2973–2978 CrossRef CAS.
- M. Karg, Y. Lu, E. Carbó-Argibay, I. Pastoriza-Santos, J. Pérez-Juste, L. M. Liz-Marzán and T. Hellweg, Langmuir, 2009, 25, 3163–3167 CrossRef CAS PubMed.
- A. O. Govorov and H. H. Richardson, Nano Today, 2007, 2, 30–38 CrossRef.
- N. S. Satarkar, D. Biswal and J. Z. Hilt, Soft Matter, 2010, 6, 2364 RSC.
- A. Z. Pich and H.-J. P. Adler, Polym. Int., 2007, 56, 291–307 CrossRef CAS.
- I. Berndt, J. S. Pedersen and W. Richtering, Angew. Chem., Int. Ed., 2006, 45, 1737–1741 CrossRef CAS PubMed.
- M. Karg, I. Pastoriza-Santos, B. Rodriguez-González, R. von Klitzing, S. Wellert and T. Hellweg, Langmuir, 2008, 24, 6300–6306 CrossRef CAS PubMed.
- Y. Hertle and T. Hellweg, J. Mater. Chem. B, 2013, 1, 5874 RSC.
- I. Gorelikov, L. M. Field and E. Kumacheva, J. Am. Chem. Soc., 2004, 126, 15938–15939 CrossRef CAS PubMed.
- A. Garcia, M. Marquez, T. Cai, R. Rosario, Z. Hu, D. Gust, M. Hayes, S. A. Vail and C.-D. Park, Langmuir, 2007, 23, 224–229 CrossRef CAS PubMed.
- L. Zhu, C. Zhao, J. Zhang and D. Gong, RSC Adv., 2015, 5, 84263–84268 RSC.
- M. Richter, Y. Zakrevskyy, M. Eisele, N. Lomadze, S. Santer and R. v. Klitzing, Polymer, 2014, 55, 6513–6518 CrossRef CAS.
- Y. Zakrevskyy, M. Richter, S. Zakrevska, N. Lomadze, R. von Klitzing and S. Santer, Adv. Funct. Mater., 2012, 22, 5000–5009 CrossRef CAS.
- K. Fan, M. Bradley and B. Vincent, J. Colloid Interface Sci., 2012, 368, 287–291 CrossRef CAS PubMed.
- M. Bradley, B. Vincent, N. Warren, J. Eastoe and A. Vesperinas, Langmuir, 2006, 22, 101–105 CrossRef CAS PubMed.
- Y. Zakrevskyy, J. Roxlau, G. Brezesinski, N. Lomadze and S. Santer, J. Chem. Phys., 2014, 140, 044906 CrossRef PubMed.
- A. M. Rumyantsev, S. Santer and E. Y. Kramarenko, Macromolecules, 2014, 47, 5388–5399 CrossRef CAS.
- R. v. Klitzing, J. Mater. Chem., 2010, 20, 3502–3507 RSC.
- A. Burmistrova, M. Richter, C. Uzum and R. v. Klitzing, Colloid Polym. Sci., 2011, 289, 613–624 CAS.
- A. Burmistrova, M. Richter, M. Eisele, C. Üzüm and R. von Klitzing, Polymers, 2011, 3, 1575–1590 CrossRef CAS.
- V. Vijayanathan, T. Thomas and T. J. Thomas, Biochemistry, 2002, 41, 14085–14094 CrossRef CAS PubMed.
- A. Venancio-Marques, A. Bergen, C. Rossi-Gendron, S. Rudiuk and D. Baigl, ACS Nano, 2014, 8, 3654–3663 CrossRef CAS PubMed.
- A. Kopyshev, C. J. Galvin, J. Genzer, N. Lomadze and S. Santer, Polymer, 2016, 98, 421–428 CrossRef CAS.
- Marvin (MarvinSketch) was used for drawing, displaying and characterizing chemical structures, substructures and reactions, Marvin 6.1.3, 2013, ChemAxon (http://www.chemaxon.com).
- Y. Zakrevskyy, E. Titov, N. Lomadze and S. Santer, J. Chem. Phys., 2014, 141, 164904 CrossRef PubMed.
- Y. Zakrevskyy, A. Kopyshev, N. Lomadze, E. Morozova, L. Lysyakova, N. Kasyanenko and S. Santer, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2011, 84, 021909 CrossRef PubMed.
- Y. Zakrevskyy, P. Cywinski, M. Cywinska, J. Paasche, N. Lomadze, O. Reich, H.-G. Löhmannsröben and S. Santer, J. Chem. Phys., 2014, 140, 044907 CrossRef PubMed.
- A. R. Khokhlov, E. Y. Kramarenko, E. E. Makhaeva and S. G. Starodubtzev, Die Makromol. Chemie, Theory Simulations, 1992, 1, 105–118 CrossRef CAS.
- A. R. Khokhlov, E. Y. Kramarenko, E. E. Makhaeva and S. G. Starodubtsev, Macromolecules, 1992, 25, 4779–4783 CrossRef CAS.
- L. Yang, N. Takisawa, T. Hayashita and K. Shirahama, J. Phys. Chem., 1995, 99, 8799–8803 CrossRef CAS.
- H. Kang, B. Lee, J. Yoon and M. Yoon, J. Colloid Interface Sci., 2000, 231, 255–264 CrossRef CAS PubMed.
- T. Shang, K. A. Smith and T. A. Hatton, Langmuir, 2003, 19, 10764–10773 CrossRef CAS.
- B. A. Cicciarelli, J. A. Elia, T. A. Hatton and K. A. Smith, Langmuir, 2007, 23, 8323–8330 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c6cp04555c |
| This journal is © the Owner Societies 2017 |