Ti₃BN monolayer: the MXene-like material predicted by first-principles calculations

Abstract

The discovery of graphene and other two-dimensional (2D) materials has set the foundation for exploring and designing novel single layered sheets. The family of 2D materials encompasses a wide selection of compositions including almost all the elements of the periodic table and they have the potential to play a fundamental role in the future of electronics, composite materials and energy technology. Therefore, searching for new 2D materials is a big challenge in materials science. In this work, we theoretically designed a monolayer of Ti₃BN following the strategy of “atomic transmutation”. The Ti₃BN monolayer can be considered as three Ti-atomic layers being interleaved with one N-atomic layer and one B-atomic layer, in the sequence of Ti₁–N–Ti₂–B–Ti₃. The moderate cohesive energy, positive phonon frequencies and high melting point are the best guarantees for good stability of Ti₃BN. Based on a global minimum structures search using the particle-swarm optimization (PSO) method, Ti₃BN is the lowest energy structure in 2D space, which holds great promise for the realization of layered Ti₃BN in experiment. Based on density functional theory (DFT) calculations, Ti₃BN is intrinsically metallic and its electronic properties can be modulated by varying the surface groups, such as OH or F-termination. If realized in experiment, it may find applications in many aspects.

---

1. Introduction

Since graphene was realized experimentally in 2004,¹ 2D materials have attracted significant interest and a large variety of freestanding monolayer solids have been successfully fabricated, such as hexagonal boron nitride (h-BN),² metal chalcogenides (MoS₂, WS₂),^3,4 silicene⁵ and so on. Due to their unique electronic, chemical, and mechanical properties, those 2D materials have found wide applications in supercapacitors,⁶ solar cells,⁷ lithium ion batteries⁸ and water splitting application⁹ and other areas of energy and environment.

Recently, a new family of graphene-like 2D materials termed as MXenes were successfully synthesized by selectively extracting the “A” element from the layered MAX phases (A is an A-group element, mostly Al or Si) in the aqueous HF.¹⁰ MAX phases are a large (>60 members) family of layered ternary early transition-metal carbides, nitrides, and carbonitrides with P6₃/mmc symmetry.¹¹ To date, several MXenes have been synthesized successfully, including Ti₃C₂,¹² Ti₂C,¹³ Ta₄C₃,¹⁴ V₂C,¹⁵ TiNbC,¹⁶ Nb₂C¹⁷ and Mo₂C.¹⁸

With the increasing interest in MXenes, a mass of experimental and theoretical efforts related to their synthesis, structures, properties and potential applications have been made experimentally and theoretically.^19,20 Among the as-synthesized MXene phases, the most studied MXene is Ti₃C₂, prepared by immersing Ti₃AlC₂ in HF solutions at room temperature.¹² Ti₃C₂ was predicted theoretically to be good electrical conductors and its electrical conductivities can be tuned by different surface terminations.²¹ What's more, Ti₃C₂ have been proved to be very promising as anode materials for Li-ion batteries and as hydrogen storage media.^22,23

Motivated by the Ti₃C₂ monolayer, which is composed of three Ti-atomic layers being interleaved with two C-atomic layers, herein we designed a new 2D material of Ti₃BN by performing density functional theory (DFT) calculations following the strategy of “atomic transmutation”, which means substituting certain types of elements with their neighboring elements in the periodic table but the total number of valence electrons is kept unchanged.^24,25 A major breakthrough has been made in finding and designing novel materials. For example, if one were to substitute the oxide ions in ZnO with N and F, one would ultimately obtain Zn₂NF whose conduction and valence band edges are more favorable for water splitting.²⁶ When two C atoms in graphene were transmutated with one B atom and one N atom, h-BN with wide band gap and new functionalities will be obtained.²⁷ Therefore Ti₃BN monolayer can be thought of as obtained by substituting the two C-atomic layers of Ti₃C₂ monolayer with one nitrogen-atomic layer and one boron-atomic layer, respectively, in consideration of that nitrogen and boron are two nearest-neighbors of carbon in the periodic table and Ti₃BN is isoelectronic to Ti₃C₂.

2. Computational method

DFT calculations were performed within the Perdew–Burke–Ernzerhof (PBE) generalized gradient approximation²⁸ and the projected augmented wave (PAW) method^29,30 as implemented in the Vienna ab initio simulation package (VASP). To reach convergence criteria for both energy and forces, a special k-point sampling with a k-point separation of 0.04 Å⁻¹ is applied for the Brillouin-zone integration and the cutoff energy for the plane wave basis set is 408 eV. The ground state geometries of the 2D Ti₃BN are obtained with all the atomic positions relaxed until their residual forces are less than 0.01 eV Å⁻¹. In building the monolayer models, a vacuum thickness of 15 Å is adopted to avoid the interactions between adjacent layers. The phonon dispersion curves of Ti₃BN monolayer was calculated using the density functional perturbation theory (DFPT)^31,32 as implemented in the PHONOPY program interfaced with VASP. To obtain the accurate electronic properties of Ti₃BN monolayer, hybrid functional calculations using Hetd–Scuseria–Ernzerhof (HSE06) functional³³ have been employed.

The thermal stability of Ti₃BN monolayer was assessed by first-principles molecular dynamics (MD) calculations using the PAW pseudo-potential and PBE functional as implemented in VASP.³⁴ For each temperature, a preheating for 1 ps was applied for the initial geometry structure. And the MD calculations were in NVT ensemble, lasting for 10 ps with a time step of 1.0 fs. To control the temperature, Nosé–Hoover method was applied.³⁵

The global minimum structure for Ti₃BN monolayer was searched by particle-swarm optimization (PSO) method within the evolution algorithm which was implemented in CALYPSO code.^36–38 The population size was set as 30, and the number of generation was maintained at 25. Unit cells containing 5, 10, and 15 atoms were considered. The structure relaxations during the PSO searching were carried by using PBE functional as implemented in VASP.

3. Results and discussion

3.1 Structural properties and stability of Ti₃BN monolayer

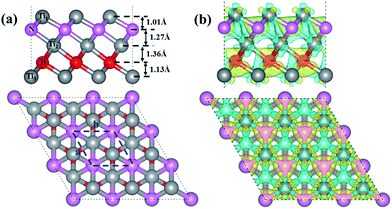
The optimized structure of our designed Ti₃BN monolayer is shown in Fig. 1(a). Similar with Ti₃C₂ monolayer, Ti₃BN monolayer is crystallized in the space group P3m1 (no. 156) and each unit cell contains five atoms, all in different atomic planes with the sequence of Ti₁–N–Ti₂–B–Ti₃. To avoid the interactions between the Ti₃BN monolayer and its periodic images among the normal direction, a vacuum layer of 15 Å is used and the calculated lattice constants for the unit cell are a = b = 3.095 Å. In Ti₃BN monolayer, the length of Ti₁–N and N–Ti₂ bonds (2.054 and 2.195 Å) are shorter than those of corresponding Ti–C bonds (2.064 and 2.212 Å)^19,20 in Ti₃C₂ monolayer, while the Ti₂–B and B–Ti₃ bond lengths are 2.249 and 2.117 Å, respectively, longer than those of corresponding Ti–C bonds in Ti₃C₂ monolayer. The thickness of Ti₃BN monolayer is 4.78 Å with the atomic layer distances of 1.01, 1.27, 1.36 and 1.13 Å, respectively.

Fig. 1 (a) Side (upper) and top (lower) views of the optimized structure of monolayer Ti₃BN. A 3 × 3 × 1 supercell is used in the projection, and the blue dash lines denote a unit cell where a, b represents the lattice constants. Gray, pink and red balls represent Ti, N and B atoms, respectively. (b) Side (upper) and top (lower) views of the isosurface of the deformation charge density of monolayer Ti₃BN with the isovalue of 0.008 Å⁻³. The blue (yellow) smooth shadings denote electron depletion (accumulation).

To elucidate the chemical bonding and stabilization mechanism of Ti₃BN monolayer, the deformation electronic density³⁹ has been calculated. As shown in Fig. 1(b), there is remarkable electron transfer from Ti atoms to N atoms and from Ti atoms to B atoms, indicating the electronically stabilization in the Ti₃BN monolayer. Bader charge analysis shows that the net charges on N and B atom are −1.65 e and −1.57 e and those on Ti₁, Ti₂, and Ti₃ atom are 0.97 e, 1.34 e and 0.91 e, respectively. The electron localization function³⁹ of Ti₃BN monolayer is also calculated to highlight the electron distribution. As seen from the isosurfaces of electron localization functions presented in Fig. S1,† the electrons are complete delocalized around the Ti atoms, and widely distributed in the N and B frameworks, which also suggests the electron transfer from Ti atoms to N and B atoms.

Although Ti₃BN monolayer possesses similar structure properties with Ti₃C₂ monolayer, the question whether Ti₃BN monolayer is as stable as Ti₃C₂ or not need to be answered. The cohesive energy of Ti₃BN monolayer is a useful argument for evaluating its stability, defined as E_coh = (xE_Ti + yE_N + zE_B − E_Ti3BN)/(x + y + z), where E_Ti, E_N, E_B and E_Ti3BN are the total energies of a single Ti atom, a single N atom, a single B atom and Ti₃BN monolayer, x, y and z are the number of Ti, N, and B atoms in the supercell, respectively. Based on our DFT calculations, the cohesive energy of Ti₃BN monolayer is 7.46 eV per atom, which is a little smaller than that of graphene (7.95 eV per atom)⁴⁰ and higher than that of Ti₃C₂ (about 7.00 eV).¹³ The relatively large cohesive energy of Ti₃BN monolayer indicates that Ti₃BN monolayer is a stable phase with strong chemical bonds. What's more, the small cohesive energy difference between Ti₃BN monolayer and Ti₃BN bulk (7.46 eV per atom vs. 7.82 eV per atom) means that it’s favorable to obtain Ti₃BN monolayer from its bulk phase. The elastic constants of Ti₃BN monolayer were then calculated to be C₁₁ = C₂₂ = 202.11 N m⁻¹, C₁₂ = C₂₁ = 59.24 N m⁻¹, which satisfy the mechanical stability criteria, indicating that the Ti₃BN monolayer is also mechanically stable.

The kinetic stability of Ti₃BN monolayer has been further confirmed by its phonon dispersion along the high-symmetry directions in the first Brillouin zone. As shown in Fig. 2(a), there is no appreciable imaginary frequency in the phonon dispersion curves, implying the good kinetic stability of Ti₃BN monolayer. The highest frequency of Ti₃BN monolayer is 653.60 cm⁻¹, higher than that of the widely studied MoS₂ monolayer (473 cm⁻¹)⁴⁰ and silicene (580 cm⁻¹).⁴⁰ The high frequency suggests that the related bonds in Ti₃BN monolayer are strong.

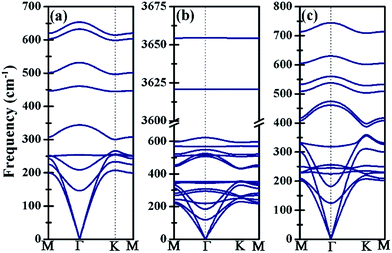Fig. 2 Phonon dispersion curves of (a) bare Ti₃BN monolayer; (b) Ti₃BN(OH)₂-IV monolayer; (c) Ti₃BNF₂-I monolayer.

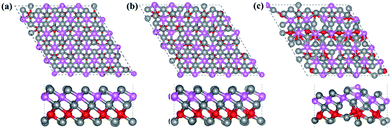
Finally, the thermal stability of Ti₃BN monolayer was investigated by first-principles molecular dynamics (MD) calculations. A 5 × 5 supercell containing 125 atoms was used here and three individual MD calculations for Ti₃BN monolayer at temperatures of 500 K, 800 K, and 1000 K were performed. Fig. 3 presents the snapshots of Ti₃BN monolayer at the end of 10 ps MD calculations. These snapshots show that Ti₃BN monolayer can maintain its structural integrity throughout a 10 ps dynamical calculation up to 800 K, however will be disrupted at the temperature of 1000 K. Those results reveal that the Ti₃BN monolayer has good thermal stability and the melting point of Ti₃BN monolayer is between 800 K and 1000 K.

Fig. 3 Top (upper) and side (lower) views of snapshots of the Ti₃BN monolayer equilibrium structures at the end of 10 ps MD simulations: (a) 500 K, (b) 800 K, and (c) 1000 K. Gray, pink and red balls represent Ti, N and B atoms, respectively.

3.2 Global minimum search for Ti₃BN monolayer in 2D space

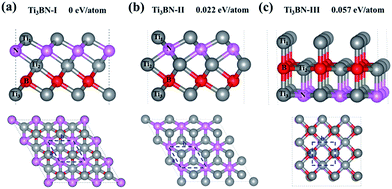
Although Ti₃BN monolayer has good stability based on the above results, the doubt about that the Ti₃BN monolayer is a local minimum or a global minimum needs to be solved. It's well known that the global minimum structure is more likely to be realized experimentally. Therefore, we carried out a global search for the lowest energy structure of Ti₃BN monolayer in the 2D space by adopting the first-principles based particle-swarm optimization method. After 25 generations, three low-energy structures for 2D Ti₃BN were obtained, labelled as Ti₃BN-I, Ti₃BN-II, and Ti₃BN-III in the order of increasing energy.

As shown in Fig. 4, in which the relative energy per atom is presented, the global minimum structure is Ti₃BN-I, which is just the above discussed Ti₃BN monolayer. Interestingly, Ti₃BN-II is also crystallized in the space group P3m1 (no. 156). The geometric construction, thickness, atomic layer distances of Ti₃BN-II is similar with that of Ti₃BN-I, while the biggest difference between them is the N-atomic layer just located above the B-atomic layer and the Ti₁-atomic layer above the Ti₃-atomic layer in Ti₃BN-II monolayer. The length of Ti₁–N, N–Ti₂, Ti₂–B, and B–Ti₃ bond in Ti₃BN-II monolayer is 2.043 Å, 2.202 Å, 2.254 Å, and 2.116 Å, respectively. Considering structure Ti₃BN-III, it is 0.057 eV per atom higher in energy than Ti₃BN-I. This high energy might be due to the more nonbonding electrons of Ti₁ atoms.

Fig. 4 Side (upper) and top (lower) views of low-energy 2D structures of Ti₃BN obtained from the PSO calculations. For Comparison, the relative energy per atom is given. The blue dash lines denote a unit cell where a, b represents the lattice constants. Gray, pink and red balls represent Ti, N and B atoms, respectively.

3.3 Electronic properties of Ti₃BN monolayer

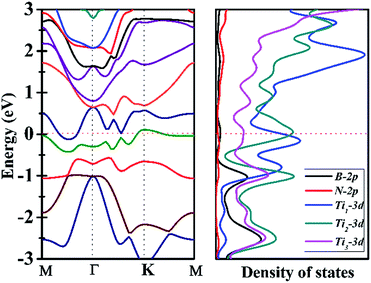
Since Ti₃BN-I monolayer holds great potential to be realized in experiment, does it have intriguing properties and promising applications? To figure out this issue, we have studied the electrical properties of the Ti₃BN-I monolayer using the hybrid density functional calculations. Fig. 5 illustrates the band structure and partial density of state (PDOS) of Ti₃BN-I monolayer. Obviously, a conduction band and a valence band across through the Fermi level, indicating that Ti₃BN monolayer has metallic properties. The PDOS analysis shows that mainly the Ti-3d states, especially the 3d states of Ti atoms from surface atomic layer, contribute to the high density of electron states around the Fermi level. Note that the high density of states near the Fermi level means available carriers which are beneficial to the high electric conductivity of the Ti₃BN monolayer.

Fig. 5 Band structure (left) and partial density of states (PDOS) of Ti₃BN-I monolayer obtained from hybrid functional calculations. For reference, the Fermi level is set at 0 eV. Ti₁ and Ti₃ represent the Ti atoms from the surface Ti-atomic layer and Ti₂ denotes the Ti atoms from inner Ti-atomic layer.

3.4 Surface modification of Ti₃BN monolayer by OH and F termination

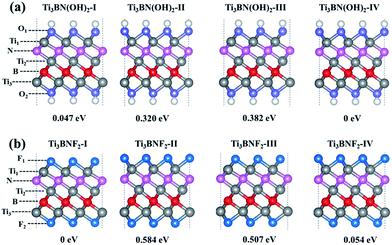
Moreover, the high DOS at the Fermi level mainly originated from Ti₁ and Ti₃ atoms indicates the high activity of surface Ti atoms, suggesting the feasibility in the surface modification and even in composite materials for Ti₃BN monolayer. For example, our theoretical studies have shown that the electronic properties of Ti₃BN can be tuned by surface functional groups (–OH, –F). Herein, four possible geometry structures of Ti₃BN monolayer with hydroxylated, and fluorinated surfaces are considered, as presented in Fig. 6. The top views of Ti₃BNF₂-I and Ti₃BNF₂-II configurations are depicted in Fig. S2.† In configuration I, all the –OH or –F groups are located above the hollow sites between the three neighboring C/B atoms or point directly toward the Ti₂ atoms on both sides of Ti₃BN monolayer. In configuration II, all the –OH or –F groups are oriented above the topmost sites of C and B atoms on the two sides of Ti₃BN monolayer, respectively. Afterwards, we can view the asymmetric arrangement of configuration III and IV as the combination of configuration I and II.

Fig. 6 Side views of optimized geometries of the hydroxylated and fluorinated Ti₃BN monolayer: (a) Ti₃BN(OH)₂; (b) Ti₃BNF₂. Gray, pink and red balls represent Ti, N and B atoms, respectively. The H, O, and F atoms are indicated by white, purple, and cyan balls.

The structural stability of different Ti₃BN(OH)₂ and Ti₃BNF₂ configurations can be estimated by comparing their relative total energies. For Ti₃BN(OH)₂, configuration IV is energetically most favorable. Ti₃BN(OH)₂-IV is energetically lower than Ti₃BN(OH)₂-I, Ti₃BN(OH)₂-II and Ti ₃BN(OH)₂-III by 0.047, 0.320, and 0.382 eV per unit cell, respectively. While for Ti₃BNF₂, configuration I is energetically most favorable, with its energy lower than that of Ti₃BNF₂-II, Ti₃BNF₂-III and Ti₃BNF₂-IV by 0.584, 0.507, and 0.054 eV per unit cell, respectively. Phonon dispersions of Ti₃BN(OH)₂-IV and Ti₃BNF₂-I has been further calculated to investigate their kinetic stability, as shown in Fig. 2(b) and (c). As expected, there are no imaginary frequencies in the phonon dispersion curves. The phonons at about 3654.56 cm⁻¹ and 3620.86 cm⁻¹ for Ti₃BN(OH)₂-IV should be dominated by the OH groups, and the phonons at about 744.73 cm⁻¹ for Ti₃BNF₂-I should be due to the F groups. These high-frequency phonons indicate the strong bond nature of the related bonds (Ti–O and Ti–F).

In comparison with bare Ti₃BN, the OH or F-terminated Ti₃BN monolayer have smaller lattice constants. With terminal groups, the bond lengths of N–Ti₂ and Ti₂–B shrink, while the bonds between the Ti₁–N and B–Ti₃ are elongated except the Ti₁–N bonds of Ti₃BNF₂-I and Ti₃BNF₂-III. Those results imply that the surface groups strongly interact with the original Ti₃BN block, in accordance with the corresponding phonon dispersions. For clearance, the calculated lattice constants and bond lengths are presented in Table S1.†

Though the Ti₃BN monolayer is metallic, its hydroxylated or fluorinated derivatives may be narrow-gap semiconductors or metals, depending on the geometrical arrangements of surface F and OH groups. Seen from Fig. 7(a), for Ti₃BN with surface OH groups, the most favorable Ti₃BN(OH)₂-IV has a semiconducting character, with a direct band gap of 0.09 eV. Ti₃BN(OH)₂-II also shows the electronic properties of direct semiconductor, and the band gap is 0.16 eV. However, Ti₃BN(OH)₂-I and Ti₃BN(OH)₂-III is metallic. For Ti₃BN with F-termination (Fig. 7(b)), the most stable configuration of Ti₃BNF₂-I and the metastable configuration of Ti₃BNF₂-III are metals. On the contrary, the band structures of Ti₃BNF₂-II and Ti₃BNF₂-IV demonstrate their indirect semiconducting characters, with the band gap of 0.07 eV and 0.37 eV, respectively. Those theoretical results prove that the electronic structure of Ti₃BN monolayer can be modulated by varying the surface functional groups.

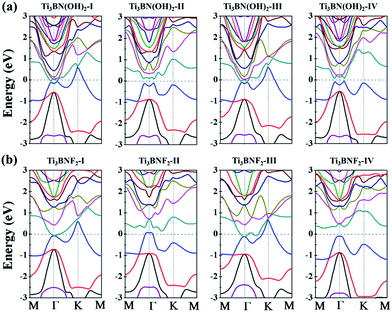Fig. 7 Band structures of the hydroxylated and fluorinated Ti₃BN monolayer obtained from hybrid functional calculations: (a) Ti₃BN(OH)₂; (b) Ti₃BNF₂.

4. Conclusions

In summary, we designed a new inorganic 2D material of Ti₃BN monolayer following the strategy of “atomic transmutation” by performing DFT calculations. In Ti₃BN monolayer, the Ti–B and Ti–N bonds are strong and the geometry structure is similar with the reported MXenes. The high cohesive energy, elastic constants, and absence of imaginary phonon frequencies prove that the Ti₃BN monolayer possess dynamic and mechanical stability. Particularly, MD simulation results verify that Ti₃BN monolayer can maintain its structural stability up to at least 800 K. The PSO method revealed that Ti₃BN monolayer is the global minimum structure in 2D space, suggesting the possibility to realize Ti₃BN monolayer in experiment. What's more, the electronic properties of Ti₃BN monolayer can be modified by varying the surface functional groups and their geometrical configurations. Therefore, if Ti₃BN is realized, it may find wide applications, such as in electrics, composite materials, Li-ion batteries and so on.

Acknowledgements

This work was supported by NSFC, China (21225524, 21527806, 21622509 and 21475122), Department of Science and Techniques of Jilin Province (20150201001GX and 20150203002YY), Jilin Province Development and Reform Commission (2016C014), Science and Technology Bureau of Changchun (15SS05).

References

1. K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, Y. Zhang, S. V. Dubonos, I. V. Grigorieva and A. A. Firsov, Science, 2004, 306, 666–669.
2. L. H. Li and Y. Chen, Adv. Funct. Mater., 2016, 26, 2594–2608.
3. L. Dominik and K. Andras, ACS Nano, 2012, 6, 10070–10075.
4. B. Liu, W. J. Zhao, Z. J. Ding, I. Verzhbitskiy, L. J. Li, J. P. Lu, J. Y. Chen, G. Eda and K. P. Loh, Adv. Mater., 2016, 28, 6457–6464.
5. T. Li, C. Eugenio, C. Daniele, G. Carlo, F. Marco, D. Madan, M. Alessandro and A. Deji, Nat. Nanotechnol., 2015, 10, 227–231.
6. X. Y. Zhang, S. H. Sun, X. J. Sun, Y. R. Zhao, L. Chen, Y. Yang, W. Lu and D. B. Li, Light: Sci. Appl., 2016, 5, e16130.
7. W. Wei, K. Sun and Y. H. Hu, J. Mater. Chem. A, 2016, 4, 12398–12401.
8. C. X. Peng, B. D. Chen, Y. Qin, S. H. Yang, C. Z. Li, Y. H. Zuo, S. Y. Liu and J. H. Yang, ACS Nano, 2012, 6, 1074–1081.
9. H. L. Zhuang and R. G. Hennig, Chem. Mater., 2013, 25, 3232–3238.
10. S. Kajiyama, L. Szabova, K. Sodeyama, H. Iinuma, R. Morita, K. Gotoh, Y. Tateyama, M. Okubo and A. Yamada, ACS Nano, 2016, 10, 3334–3341.
11. M. W. Barsoum, Prog. Solid State Chem., 2000, 28, 201–281.
12. M. Naguib, M. Kurtoglu, V. Presser, J. Lu, J. Niu, M. Heon, L. Hultman, Y. Gogotsi and M. W. Barsoum, Adv. Mater., 2011, 23, 4248–4253.
13. T. S. Zhao, S. H. Zhang, Y. Guo and Q. Wang, Nanoscale, 2016, 8, 233–242.
14. M. Naguib, O. Mashtalir, J. Carle, V. Presser, J. Lu, L. Hultman, Y. Gogotsi and M. W. Barsoum, ACS Nano, 2012, 6, 1322–1331.
15. M. Naguib, J. Halim, J. Lu, K. M. Cook, L. Hultman, Y. Gogotsi and M. W. Barsoum, J. Am. Chem. Soc., 2013, 135, 15966–15969.
16. O. Mashtalir, M. Naguib, V. N. Mochalin, Y. Dall'Agnese, M. Heon, M. W. Barsoum and Y. Gogotsi, Nat. Commun., 2013, 4, 1716–1723.
17. J. P. Hu, B. Xu, C. Y. Ouyang, Y. Zhang and S. Y. A. Yang, RSC Adv., 2016, 6, 27467–27474.
18. C. Xu, L. Wang, Z. Liu, L. Chen, J. K. Guo, N. Kang, X.-L. Ma, H.-M. Cheng and W. Ren, Nat. Mater., 2015, 14, 1135–1141.
19. S. K. Kim, Y.-J. Zhang, H. Bergstrom, R. Michalsky and A. Peterson, ACS Catal., 2016, 6, 2003–2013.
20. Q. Tang, Z. Zhou and P. Shen, J. Am. Chem. Soc., 2012, 134, 16909–16916.
21. S. Lai, J. Jeon, S. K. Jang, J. Xu, Y. J. Choi, J.-H. Park, E. Hwang and S. Lee, Nanoscale, 2015, 7, 19390–19396.
22. D. Er, J. Li, M. Naguib, Y. Gogotsi and V. B. Shenoy, ACS Appl. Mater. Interfaces, 2014, 6, 11173–11179.
23. Q. K. Hu, D. D. Sun, Q. H. Wu, H. Y. Wang, L. B. Wang, B. Z. Liu, A. G. Zhou and J. L. He, J. Phys. Chem. A, 2013, 117, 14253–14260.
24. J.-H. Yang, Y. Zhang, W. J. Yin, X. G. Gong, B. I. Yakobson and S.-H. Wei, Nano Lett., 2016, 16, 1110–1117.
25. C. Zhang and Q. Sun, J. Phys. Chem. Lett., 2016, 7, 2664–2670.
26. Y. Kubota, K. Watanabe, O. Tsuda and T. Taniguchi, Science, 2007, 317, 932–934.
27. S. R. Lingampalli, K. Manjunath, S. Shenoy, U. V. Waghmare and C. N. R. Rao, J. Am. Chem. Soc., 2016, 138, 8228–8234.
28. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
29. J. Furthmüller, J. Hafner and G. Kresse, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 15606.
30. G. Kresse and D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758.
31. B. Stefano, S. De Gironcoli and A. Dal Corso, Rev. Mod. Phys., 2001, 73, 515.
32. A. Togo and I. Tanaka, Scr. Mater., 2015, 108, 1.
33. J. Heyd, G. E. Scuseria and M. Ernzerhof, J. Chem. Phys., 2003, 118, 8207.
34. M. C. Payne, M. P. Teter, D. C. Allan, T. A. Arias and J. D. Joannopoulos, Rev. Mod. Phys., 1992, 64, 1045.
35. G. J. Martyna, M. L. Klein and M. E. Tuckerman, J. Chem. Phys., 1992, 97, 2635.
36. Y. C. Wang, J. Lv, L. Zhu and Y. M. Ma, Comput. Phys. Commun., 2012, 183, 2063–2070.
37. X. Y. Luo, J. H. Yang, H. Y. Liu, X. J. Wu, Y. C. Wang, Y. M. Ma, S.-H. Wei, X. G. Gong and H. J. Xiang, J. Am. Chem. Soc., 2011, 133, 16285–16290.
38. Y. C. Wang, M. S. Miao, J. Lv, L. Zhu, K. T. Yin, H. Y. Liu and Y. M. Ma, J. Chem. Phys., 2012, 137, 224108.
39. H. J. Zhang, Y. F. Li, J. H. Hou, K. X. Tu and Z. F. Chen, J. Am. Chem. Soc., 2016, 138, 5644–5651.
40. Y. F. Li, Y. L. Liao and Z. F. Chen, Angew. Chem., Int. Ed., 2014, 53, 7248–7252.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c7ra00483d

---