Characterization of blue-excited yellow phosphor (Y,Ca)_6+x/3Si₁₁(N,O)₂₁:Ce by the bond valence sum model

Abstract

The new bright yellow phosphor (Y,Ca)_6+x/3Si₁₁(N,O)₂₁:Ce (CYSON) providing broad emission excited by blue LED is the product of extensive substitution (about 70% of Y) of Y³⁺ → Ca²⁺ and N³⁻ → O²⁻ in the Y₆Si₁₁ON₂₀:Ce parent host, which exhibits a weak emission excited by near UV-excitation only. Such a considerable difference is caused by particular distribution of substituting ions through the crystal lattice sites. For the first time for the title host, these intricate effects have been thoroughly studied in the present paper. We analyzed distribution of cations and their anion surrounding for each of the three Y sites. In addition, the local charge balance was also considered in detail by calculating the Brown's bond valence sum (BVS). The results suggest that the number of oxygen ligands is likely to be 0 at the Y₁ and Y₂ sites, 2–3 at the Ca₁ and Ca₂ sites, 0–1 at the Y₃ site, and 3–4 at the Ca₃ site (the subscript enumerates the inequivalent sites in the CYSON lattice). These data indicate that the Ca ions are likely to be coordinated by a greater number of the oxygen ions than the Y ions, which leads to the conclusion that the Y–N bond should be extensively substituted by the Ca–O bond. Therefore, agglomeration of an increased number of the oxygen ions far away from the Ca²⁺ ions is suppressed. It is suggested that as the bond lengths of the cation–anion pairs in CYSON are much larger than the sum, 2.28 Å, of the ionic radii of the Y³⁺ and O²⁻ ions (which yields a smaller BVS and a small local charge balance), the substituted Ca²⁺–O²⁻ pair contributes to the stabilization of electric charge distribution. This is the first detailed study of the structural properties of this new phosphor that allowed to identify most probable coordination around each and every cation site in this complicated structure with many inequivalent crystallographic positions.

---

1 Introduction

For about a couple of decades, white phosphor-converted light-emitting diodes (LEDs) have been important devices because they provide an efficient conversion of electric energy to visible light. In addition, they are environmentally friendly, since no toxic Hg is used in their production compared to conventional incandescent and fluorescent lamps.¹ One of the main goals on the phosphor markets is the improved luminous efficacy of novel phosphors for various applications. The phosphors excited by the light of 450 nm-peak of efficient blue LED require a much longer wavelength of excitation than the conventional 254 nm wavelength of the excitation light. The nitridosilicates activated by Eu²⁺ or Ce³⁺ (ref. 2–10) ions are likely to have a strong crystal field splitting (CFS) and a low position of the Eu²⁺ or Ce³⁺ 5d states centroid due to coordination by the N³⁻ ions forming highly covalent chemical bonds. This leads to the enhanced nephelauxetic effect, which in turn decreases the energy difference between the ground 4f and the lowest 5d states of the lanthanide ions. In this case, the longest wavelength of excitation, equivalent to the energy difference between the 4f state and the lowest 5d state, increases.

Seto et al. found the new blue-excited yellow phosphor, (Y,Ca)_6+x/3Si₁₁(N,O)₂₁:Ce,¹¹ (here and thereafter called CYSON) which has two prominent distinguished features. Firstly, extensive substitution (about 70% of Y) of Y³⁺ → Ca²⁺ and N³⁻ → O²⁻ in Y₆Si₁₁ON₂₀:Ce results in (i) much better crystallinity and (ii) brighter emission under a usual condition of synthesizing nitridosilicate phosphor. The prepared Y₆Si₁₁ON₂₀:Ce itself is not a blue-excited phosphor but a near UV-excited phosphor, with much lower crystallinity and weaker emission. The crystal structure of CYSON is shown in Fig. 1. There are three Y³⁺ sites that can be partially substituted by the Ca²⁺ or Ce³⁺ ions and nine sites for the O²⁻, N³⁻ anions, as shown in Fig. 2.

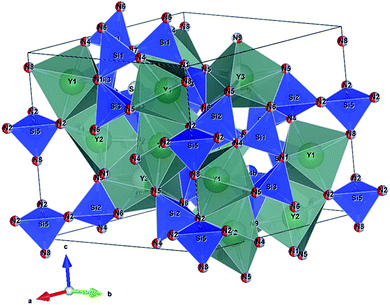Fig. 1 Crystal structure of (Y,Ca)_6+x/3Si₁₁(N,O)₂₁:Ce. Y₁, Y₂, and Y₃ indicates three sites of Y or Ca substituted for Y. N₁–N₉ indicates nine sites of nitrogen or oxygen.

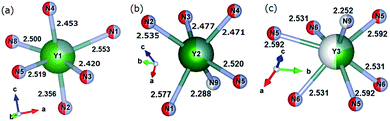Fig. 2 Coordination of Y₁ site (a), Y₂ site (b), and Y₃ site (c), respectively. Occupancy of Y, Ca, or Ce at each Y_n site is expressed by blue, green, or yellow colours, respectively.

In the present paper we set our goal to investigate whether such extensive and simultaneous substitution provides stable structure from the point of view of the electric charge balance at each cation site. For this purpose, we calculated the Brown's bond valence sum (BVS)¹² for each and every cation site, with and without taking into account effect of the crystal lattice relaxation upon the substitution. The analysis of the obtained results revealed certain peculiar features of coordination of various cations and allowed to understand how such extended cation substitution is realized in the CYSON crystal lattice.

2 Procedure of calculation

The Brown's equation has a wider area of application than the original Pauling's equation on the bond valence sum so far. According to Brown, the bond valence of cation i coordinating anion j can be calculated from the following eqn (1).^12–14where R_ij is the bond valence parameter of a cation i coordinating by an anion j, d_ij is the bond length between the cation i and anion j, and B is a constant value (the most reliable value for this parameter is 0.37 Å). The bond valence sum can be obtained for each atomic distance, cation species, and species of anion ligands.

We used the values of all d_ij, R_ij, and B from the reference to the CYSON structure,¹¹ and Brown's list of the bond balance parameter.^13,14 The R_ij value depends on the cation and anion species coordinating a particular cation.

CYSON's space group is P3₁c (no. 159) and its typical composition is Y_6+(x/3)-yCa_ySi₁₁N_20+x−yO_1−x+y:Ce (x = 1.2, y = 4.5, Ce molar ratio = 0.06). Each cation's occupancy in CYSON is shown in Table 1,¹¹ whereas the atomic distances (all in Å) in CYSON are shown in Table 2.¹¹ It is seen that the Y³⁺, Ca²⁺, or Ce³⁺ ions can occupy all three cationic sites. Taken together with anion substitution, this makes an analysis of the structural properties of this phosphor to be a non-trivial problem.

Table 1 Occupancies of Y, Ca, and Ce at each site determined by Rietveld refinement¹¹

	Ln1 site	Ln2 site	Ln3 site
Y	0.2171	0.3318	0.1978
Ca	0.7764	0.6645	0.3036
Ce	0.0066	0.0038	0.0243

---

Table 2 Atomic distances determined by Rietveld refinement¹¹

Ln1 site:	Ln1–(O/N)1	2.553(3)	Si1 site: Si1–(O/N)1	1.693(5)
	Ln1–(O/N)2	2.356(4)	Si1–(O/N)4	1.695(3)
	Ln1–(O/N)3	2.420(3)	Si1–(O/N)6	1.798(5)
	Ln1–(O/N)4	2.453(4)	Si1–(O/N)7	1.781(2)
	Ln1–(O/N)5	2.519(1)	Si2 site: Si2–(O/N)2	1.665(3)
	Ln1–(O/N)8	2.500(3)	Si2–(O/N)4	1.757(5)
Ln2 site:	Ln2–(O/N)1	2.577(3)	Si2–(O/N)5	1.678(5)
	Ln2–(O/N)2	2.535(3)	Si2–(O/N)6	1.816(4)
	Ln2–(O/N)3	2.477(4)	Si3 site: Si3–(O/N)1	1.754(5)
	Ln2–(O/N)4	2.471(2)	Si3–(O/N)3	1.722(3)
	Ln2–(O/N)5	2.520(4)	Si3–(O/N)5	1.823(3)
	Ln2–(O/N)9	2.288(2)	Si3–(O/N)6	1.690(5)
Ln3 site:	Ln3–(O/N)5	2.592(1)	Si4a site: Si4a–(O/N)3 × 3	1.684(6)
	Ln3–(O/N)5	2.592(1)	Si4b–(O/N)9	1.916(2)
	Ln3–(O/N)5	2.592(1)	Si4b site: Si4b–(O/N)3 × 3	1.665(3)
	Ln3–(O/N)6	2.531(4)	Si4b–(O/N)7	2.218(11)
	Ln3–(O/N)6	2.531(4)	Si5 site: Si5–(O/N)2 × 3	1.797(3)
	Ln3–(O/N)6	2.531(4)	Si5–(O/N)8	1.648(8)
	Ln3–(O/N)9	2.252(7)

---

The bond valence parameters R_ij used in the present calculation are 2.17 (R_ij for the Y–N pair), 2.14 (Ca–N), 2.019 (Y–O), and 1.967 (Ca–O).^13,14 Strictly speaking, due to the partial occupancies of the crystal lattice sites in CYSON it is not known how many N³⁻ or O²⁻ anions coordinate each of the Y³⁺, Ca²⁺, or Ce³⁺ ions, respectively. To eliminate any ambiguity in this important issue and understand better the reasons for the improved crystallinity and enhanced luminescence of the substituted phosphor, we considered all possible cases of the N³⁻ or O²⁻ configurations coordinating every Y³⁺ or Ca²⁺ cation at each Ln_q site. As Ln₁, Ln₂, and Ln₃ sites receive 6, 6, and 7 anion ligands, respectively (Fig. 2), there are 2⁶, 2⁶, and 2⁷ possible configurations of nitrogen and/or oxygen ligands coordinating the Ln₁, Ln₂, and Ln₃ sites, respectively.

As an example, Table 3 shows just a few anion arrangements out of all 64 cases of the nitrogen or oxygen coordination around the Y³⁺ or Ca²⁺ cations at the Ln₁ site. Table 4 shows the typical results of the BVS calculations for the case no. 9 from Table 3 (one of all 64 cases), including the difference between the BVS and cation's valence, which is called the value of charge imbalance here. All other cases can be calculated in a similar way and are not shown here for the sake of brevity. We present all the calculated results in the next section in the form of diagrams.

Table 3 Parts of all 64 cases that nitrogen or oxygen coordinates each Y or Ca of Ln₁ site

Cation–(anion)site no.	Species of anion ligand (the above: case number)
	1	2	3	4	5	6	7	8	9	…	61	62	63	64
Y–(O/N)1	N	O	N	N	N	N	N	O	O	…	O	O	N	O
Y–(O/N)2	N	N	O	N	N	N	N	O	N	…	O	N	O	O
Y–(O/N)3	N	N	N	O	N	N	N	N	O	…	N	O	O	O
Y–(O/N)4	N	N	N	N	O	N	N	N	N	…	O	O	O	O
Y–(O/N)5	N	N	N	N	N	O	N	N	N	…	O	O	O	O
Y–(O/N)8	N	N	N	N	N	N	O	N	N	…	O	O	O	O
Ca–(O/N)1	N	O	N	N	N	N	N	O	O	…	O	O	N	O
Ca–(O/N)2	N	N	O	N	N	N	N	O	N	…	O	N	O	O
Ca–(O/N)3	N	N	N	O	N	N	N	N	O	…	N	O	O	O
Ca–(O/N)4	N	N	N	N	O	N	N	N	N	…	O	O	O	O
Ca–(O/N)5	N	N	N	N	N	O	N	N	N	…	O	O	O	O
Ca–(O/N)8	N	N	N	N	N	N	O	N	N	…	O	O	O	O
Number of oxygen in 6 ligands	0	1	1	1	1	1	1	1	1	…	5	5	5	6

---

Table 4 Example of the BVS and the difference between BVS and cation's valence (charge imbalance) calculated in case no. 9 (one of all 64 cases of N/O coordination)

	R	dij (Å)	BV		R	dij (Å)	BV
Y1–O1	2.019	2.553	0.236	Ca1–O1	1.967	2.553	0.223
Y1–N2	2.17	2.356	0.604	Ca1–N2	2.14	2.356	0.514
Y1–O3	2.019	2.42	0.338	Ca1–O3	1.967	2.42	0.319
Y1–N4	2.17	2.453	0.465	Ca1–N4	2.14	2.453	0.396
Y1–N5	2.17	2.519	0.389	Ca1–N5	2.14	2.519	0.331
Y1–N8	2.17	2.5	0.410	Ca1–N8	2.14	2.5	0.349
BVS			2.442				2.131
Charge imbalance			0.558 (=3 − 2.442)				0.131 (=2.131 − 2)

---

3 Results and discussion

Fig. 3(a)–(c) show all the values of the calculated charge imbalance at the Ln₁, Ln₂, and Ln₃ sites, respectively. The smallest value of charge imbalance is obtained in the case of all nitrogen ligands, if the Y³⁺ ions occupy the Ln₁, Ln₂, and Ln₃ sites, whereas when the Ca²⁺ ions occupy the same sites, the smallest value is obtained in the case of 3 oxygen and 3 nitrogen ligands for the Ln₁ and Ln₂ sites, and in the case of 4 oxygen and 3 nitrogen ligands for the Ln₃ site. To understand the conditions that minimize the charge imbalance all the sets of the O²⁻ and N³⁻ ligands providing the smallest three values of charge imbalance are shown in Table 5. The minimal values of charge imbalance are relatively high, 0.2688, 0.3016, and 0.1120 for the Y₁, Y₂, and Y₃ sites, while the same values are much smaller, 0.0037, 0.0049, and 0.0014 for the Ca₁, Ca₂, and Ca₃ sites.

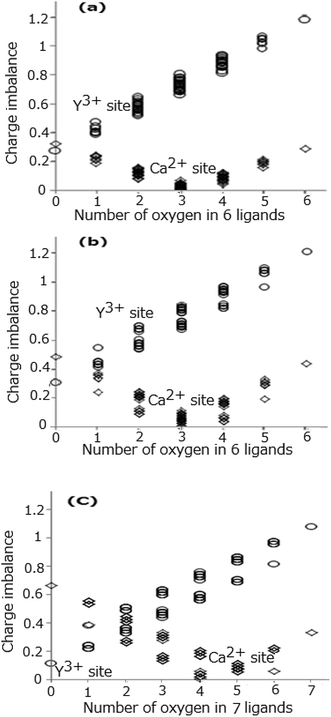Fig. 3 Difference (charge imbalance) between BVS and cation's valence at Ln₁ site (a), Ln₂ site (b), and Ln₃ site (c), respectively.

Table 5 The sets of O²⁻ and N³⁻ ligands providing the smallest values of charge imbalance

Sets of Y^3+/Ca^2+–O^2−/N^3−	Values of charge imbalance
Y1–[N1, N2, N3, N4, N5, N8]	0.2688
Y1–[O1, N2, N3, N4, N5, N8]	0.3877
Y1–[N1, N2, N3, N4, O5, N8]	0.3992
Ca1–[O1, O2, N3, O4, N5, N8]	0.0037
Ca1–[N1, O2, N3, O4, O5, N8]	0.0040
Ca1–[O1, O2, O3, N4, N5, N8]	0.0060
Y2–[N1, N2, N3, N4, N5, N9]	0.3016
Y2–[O1, N2, N3, N4, N5, N9]	0.4131
Y2–[N1, O2, N3, N4, N5, N9]	0.4265
Ca2–[O1, O2, N3, N4, N5, O9]	0.0049
Ca2–[O1, N2, N3, N4, O5, O9]	0.0101
Ca2–[N1, O2, N3, N4, O5, O9]	0.0239
Y3–[N5, N5, N5, N6, N6, N6, N9]	0.1120
Y3–[N5, N5, O5, N6, N6, N6, N9]	0.2191
Y3–[N5, O5, N5, N6, N6, N6, N9]	0.2191
Ca3–[N5, N5, N5, O6, O6, O6, O9]	0.0014
Ca3–[N5, N5, O5, N6, O6, O6, O9]	0.0182
Ca3–[N5, O5, N5, N6, O6, O6, O9]	0.0182

---

We may take another consideration that the atomic distance determined by the Rietveld analysis in Table 2 (ref. 11) may be an average of the corresponding distances for the Y³⁺-anion, Ca²⁺-anion, and Ce³⁺-anion. Then the actual distances for these three cation–anion sets may be slightly deviating from the average distance. For simply clarifying the behaviour of the substitution, Y³⁺ → Ca²⁺ and N³⁻ → O²⁻, the Ce³⁺-anion pair is neglected due to a tiny contribution of the Ce ions (because of low concentration) to the average distance. To account for a difference between the interionic distances, we use the following approach. For the quantitative estimation of the 5d-level energies of the Ce³⁺-activated phosphors Dorenbos¹⁵ assumed that the phosphor's anions relax radially inward or outward by half the difference ΔR between the ionic radius of the cation A and the ionic radius of the cation B for which the cation A substitutes. As the next step, we take the Dorenbos assumption, where the difference in distance between the Y³⁺-anion and Ca²⁺-anion pairs in the CYSON crystal should be 0.5ΔR = 0.5 × 0.10 Å because the corresponding Shannon radii are 0.90 Å (Y³⁺, coordination number (CN):6), 1.00 Å (Ca²⁺, CN:6), 0.96 Å (Y³⁺, CN:7), and 1.06 Å (Ca²⁺, CN:7). We take the distance in the Y³⁺-anion pair as (−0.025 Å + the distance of Ln_x-anion in Table 2) and the distance in the Ca²⁺-anion pair as (0.025 Å + the distance of Ln_x-anion in Table 2), where the 0.05 Å value (half of the difference in distance between Y³⁺-anion and Ca²⁺-anion) can be obtained from a relation [0.025 Å − (−0.025 Å)]. The BVS and the value of charge imbalance are calculated from these values of reasonably re-estimated in such way cation–anion distances. Fig. 4(a)–(c) show all the values of charge imbalance at the Ln₁, Ln₂, and Ln₃ sites originated from the modified bond distance, respectively. The sets of the O²⁻ and N³⁻ ligands providing the smallest three values of charge imbalance are shown in Table 6. The smallest value of charge imbalance significantly decreases from 0.2688 to 0.0779 (Y₁ site), from 0.3016 to 0.1131 (Y₂ site), and from 0.1120 to 0.0247 (Y₃ site) when the two Y³⁺-anion and Ca²⁺-anion distances at the same site are modified taking into account differences of the ionic radii in the corresponding pairs. The coordination number of oxygen yielding the smallest value of charge imbalance is slightly changed from 3 to 2 (Ca₁ and Ca₂ sites), from 4 to 3 (Ca₃ site), from 0 to 1 (Y₃ site).

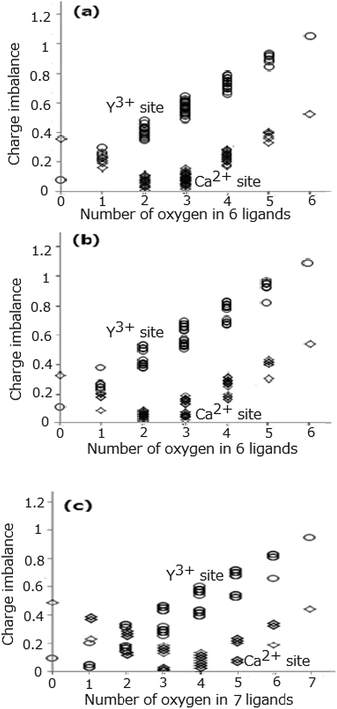Fig. 4 Difference (charge imbalance) between the BVS and cation's valence at the Ln₁ site (a), Ln₂ site (b), and Ln₃ site (c), respectively.

Table 6 The sets of O²⁻ and N³⁻ ligands providing the smallest three values of charge imbalance

Sets of Y^3+/Ca^2+–O^2−/N^3−	Values of charge imbalance
Y1–[N1, N2, N3, N4, N5, N8]	0.0779
Y1–[O1, N2, N3, N4, N5, N8]	0.2051
Y1–[N1, N2, N3, N4, O5, N8]	0.2174
Ca1–[N1, O2, O3, N4, N5, N8]	0.0042
Ca1–[N1, O2, N3, O4, N5, N8]	0.0098
Ca1–[O1, N2, N3, N4, O5, O8]	0.0174
Y2–[N1, N2, N3, N4, N5, N9]	0.1131
Y2–[O1, N2, N3, N4, N5, N9]	0.2322
Y2–[N1, O2, N3, N4, N5, N9]	0.2466
Ca2–[O1, N2, N3, N4, N5, O9]	0.0153
Ca2–[O1, O2, N3, N4, O5, N9]	0.0262
Ca2–[N1, O2, N3, N4, N5, O9]	0.0281
Y3–[N5, N5, O5, N6, N6, N6, N9]	0.0247
Y3–[N5, O5, N5, N6, N6, N6, N9]	0.0247
Y3–[O5, N5, N5, N6, N6, N6, N9]	0.0247
Ca3–[N5, N5, O5, N6, N6, O6, O9]	0.0076
Ca3–[N5, O5, O5, N6, N6, O6, O9]	0.0076
Ca3–[O5, N5, O5, N6, N6, O6, O9]	0.0076

---

On total consideration including both cases of the same distance (Table 2) and the different distances, the number of the oxygen ligands seems to be 0–1 at the Y sites and 2–4 at the Ca sites, which suggests that the Ca ions are likely to be coordinated by more oxygen ions than the Y ions. The result coincides with the rough but essential Pauling's second rule¹⁶ where O²⁻ should be substituted for the N³⁻ site near the Ca²⁺ ions rather than near Y³⁺ ions because it can minimize the local volume where the sum of electric charge, −1, caused by the Y³⁺ → Ca²⁺ substitution, is localized. The ratio O/(O + N) is likely to be 0% at Y³⁺, 33–50% at Ca²⁺ at the Ln₁ and Ln₂ sites, 0–14% at Y³⁺, 43–57% at Ca²⁺ at the Ln₃ site. Then, on the consideration of occupancies of (Y + tiny amount of Ce) and Ca in Table 1, the average ratio O/(O + N) is likely to be 26–39% at Ln₁ site, 22–33% at Ln₂ site, and 25–39% at Ln₃ site, respectively. Total ratio of O/(O + N) in CYSON is (1–1.2 + 4.5)/21 = 21%, which is roughly at the same level with the above ratios in all three Ln sites.

Table 2 and Fig. 1 shows that there are six four-fold coordinated sites for the Si⁴⁺ ions (Si₁, Si₂, Si₃, Si_4a, Si_4b, Si₅). We also calculated the BVS and the values of charge imbalance for these Si sites, which are shown in Table 7. The minimum value of charge imbalance is obtained for the Si–(O, N, N, N) composition in almost every Si site in CYSON. It means that the ratio O/(O + N) at Si sites, ∼25%, providing the minimum instability value, is almost at the same level as total ratio O/(O + N) of CYSON, 21%, again. From the above consistent results, it is considered that one of the reason for successful extensive substitution of Y–N → Ca–O is an existence of the structure with the bond lengths much greater than the sum, 2.28 Å, of radii of Y³⁺ (CN:6) and O²⁻ ions yielding a smaller BVS, which leads to an increased oxygen coordination and the stabilization of charge distribution near the Ca²⁺ ions.

Table 7 A set of anion ligands providing the minimum value of charge imbalance at each Si⁴⁺ site

A set of Si^4+ and O/N ligands	Value of charge imbalance
Si1–[O7, N1, N4, N6]	0.0331
Si2–[O2, N1, N4, N5]	0.0914
Si3–[O5, N1, N3, N6]	0.0036
Si4a–[O3, N3, N3, N9]	0.0418
Si4b–[O3, N3, N3, N7]	0.1536
Si5–[O2, N2, N2, N8]	0.1287

---

It is notable that the new type of calculation by use of Brown's BVS in this work shed light on the phenomenon, the substitution of O²⁻ for the N³⁻ site coordinating Ca²⁺ ions rather than Y³⁺ ions, because the phenomenon is consistent with the principle of ceramics that cations and anions are arranged so that local region forming electric charge as a sum might be minimized.

4 Conclusions

The unique extensive substitution (about 70% of Y) of Y³⁺ → Ca²⁺ and N³⁻ → O²⁻ in the blue-excited yellow phosphor (Y,Ca)_6+x/3Si₁₁(N,O)₂₁:Ce was investigated by calculating the Brown's bond valence sums at each three Y/Ca/Ce cation sites, including all inequivalent positions. Due to the partial occupancy of the cation and anion sites in the “parent” CYSON structure, the coordination of anions at each cationic site was not known up to now. The obtained results for the first time allow to determine the number of anions of different kinds at each site. In particular, the number of the oxygen ligands is likely to be 0 at Y₁ and Y₂ sites and 2–3 at Ca₁ and Ca₂ sites, 0–1 at Y₃ site, and 3–4 at Ca₃ site. It indicates that the oxygen ions prefer to form coordination around the Ca ions rather than around the Y ions, which leads to a further conclusion that the Y–N bond should be extensively substituted by the Ca–O bond instead of agglomeration of the oxygen ions far apart from the Ca²⁺ ions. On the basis of the performed analysis, it became possible to find a chemically-based strong explanation for the successful extensive substitution of the Y–N pairs by the Ca–O pairs. It can be realized on account of formation of a stable structure having the bond lengths much greater than the simple sum (equal to 2.28 Å) of the ionic radii of the six-fold coordinated Y³⁺ and O²⁻ ions. Such substitution yields smaller BVS values and is accompanied by increased oxygen coordination and the stabilization of charge distribution near the Ca²⁺ ions. The performed analysis can be efficiently applied to other crystals with partial cation/anion occupancy or solid solutions; it helps to understand the local coordination around various sites in crystal lattice. The method acquires a special importance for the doped phosphor materials, since in this case the nearest coordination of an impurity ion determines the crystal field strength, point symmetry and, as such, the overall pattern of the crystal field splitting of impurity ions energy levels, and, finally, spectroscopic properties of such doped material.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

We are thankful to Samsung R&D Research Institute for the published details of CYSON. M.G. Brik thanks the supports from the Recruitment Program of High-end Foreign Experts (Grant No. GDW20145200225), the Programme for the Foreign Experts offered by Chongqing University of Posts and Telecommunications, Ministry of Education and Research of Estonia, Project PUT430, and European Regional Development Fund (TK141).

Notes and references

1. S. Nakamura and G. Fasol, The blue laser diode: GaN Based Light Emitters and Lasers, Springer, Berlin, 1997.
2. K. Uheda, N. Hirosaki, H. Yamamoto, H. Yamane, Y. Yamamoto, W. Inami and K. Tsuda, The 206^th Annual Meeting of the Electrochemical Society (Abstract No. 2073), Honolulu, Oct 3 2004.
3. N. Kijima, T. Seto and N. Hirosaki, ECS Trans., 2009, 25, 247–252.
4. H. A. Hoppe, H. Lutz, P. Morys, W. Schnick and A. Seilmeier, J. Phys. Chem. Solids, 2000, 61, 2001–2006.
5. C. Hecht, F. Stadler, P. J. Schmidt, J. S. Gunne, V. Baumann and W. Schnick, Chem. Mater., 2009, 21, 1595–1601.
6. Y. Q. Li, A. C. A. Delising, G. de With and H. T. Hintzen, Chem. Mater., 2005, 17, 3242–3248.
7. R.-J. Xie, M. Mitomo, K. Uheda, F.-F. Xu and Y. Akimune, J. Am. Ceram. Soc., 2002, 85, 1229–1234.
8. N. Hirosaki, R.-J. Xie, K. Kimoto, T. Sekiguchi, Y. Yamamoto, T. Suehiro and M. Mitomo, Appl. Phys. Lett., 2005, 86, 211905.
9. Y. Liu, X. Zhang, Z. Hao, X. Wang and J. Zhang, J. Mater. Chem., 2011, 21, 6354–6358.
10. Y. Liu, J. Zhang, C. Zhang, J. Xu, G. Liu, J. Jiang and H. Jiang, Adv. Opt. Mater., 2015, 3, 1096–1101.
11. T. Seto and T. Izawa, ECS J. Solid State Sci. Technol., 2015, 4, R83–R88.
12. I. D. Brown, Structure and Bonding in Crystals, Academic Press, New York, 1981, vol. 2, pp. 1–30.
13. I. D. Brown and D. Altermatt, Acta Crystallogr., 1985, 41, 244–247.
14. N. E. Brese and M. O'Keeffe, Acta Crystallogr., 1991, 47, 192–197.
15. P. Dorenbos, Phys. Rev. B: Condens. Matter Mater. Phys., 2000, 62, 15640–15649.
16. P. E. D. Morgan, J. Mater. Sci., 1986, 21, 4305–4309.

---

Footnote

† T. Seto is currently at Advanced Technologies Research Institute, Denka Corporation, while he has done this work at the above address with M. G. Brik.

---