 Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Intriguing uniform elongation and accelerated radial shrinkage in an amorphous SiOx nanowire as purely induced by uniform electron beam irradiation
Jiangbin Su†
 ab and
Xianfang Zhu
ab and
Xianfang Zhu *ac
*ac
aChina-Australia Joint Laboratory for Functional Nanomaterials & Physics Department, Xiamen University, Xiamen 361005, People's Republic of China. E-mail: zhux@xmu.edu.cn
bExperiment Center of Electronic Science and Technology, School of Mathematics and Physics, Changzhou University, Changzhou 213164, People's Republic of China
cInstitute of Biomimetics and Soft Matter, Xiamen University, Xiamen 361005, People's Republic of China
First published on 27th September 2017
Abstract
At room temperature, an intriguing athermal uniform elongation and accelerated radial shrinkage in a straight and uniform amorphous SiOx nanowire (a-SiOx NW) as purely induced by uniform electron beam (e-beam) irradiation (without any external tensile pulling) was in situ observed and investigated in transmission electron microscope. According to a new model for NW taking into account the NW nanocurvature over its surface as well as the beam-induced athermal activation, a kinetic relationship between the shrinking radius and the irradiation time was established. The fitting results demonstrated that a curvature-dependent surface energy at the nanoscale (so-called nanocurvature effect) much higher than that predicted from the existing theories was exerted on the elongation and shrinkage of the NW. At the same time, the so-induced plastic flow of massive atoms and surface diffusion of atoms presented as well a further direct experimental evidence for our predicted soft mode and instability of atomic vibration as induced under energetic beam irradiation in amorphous materials. The study has important implications for the nanoprocessing or nanostability of future NW-based structures or devices. More importantly, it further demonstrates that the nanocurvature effect and the beam-induced atomic vibration soft mode and instability effect, which have been normally neglected or inadequately taken into account in the current literature, are universal concepts and applicable to explanation of energetic beam-induced nanoinstability or nanoprocessing of low dimensional nanostructure in general.
1. Introduction
Electron beam (e-beam) irradiation-induced structure change and processing in nanowires (NWs) have been studied extensively1–10 due to their intensive research interest and numerous promising applications in nanotechnology and nanodevices. Among these, the study on elongation of NWs7–10 in an in situ transmission electron microscope (TEM) is especially interesting and attractive. This is because such a study makes great sense not only to the fundamental understanding of NW deformation but also to widening of practical application of NW, which otherwise would be limited by the difficulties in controllable fabrication of NW with a desired shape such as length and diameter. Up till now, Zheng et al.,7 Han et al.8 and Zheng et al.9 have reported the low-intensity (non-focused) e-beam-assisted tensile pulling-induced elongation of crystalline Li2O and silicon NWs and amorphous silica NW respectively, in which both the elongation mechanisms and the stretching installations were complicated. Furthermore, Zhu et al.10 have irradiated amorphous SiOx (a-SiOx) NW by a focused e-beam, where a local S-type prolonging and necking (a non uniform elongation and deformation) of a-SiOx NW was realized. Even so, the elongation and necking kinetics were still complicated due to the non-uniform distributions of both positive and negative nanocurvatures as well as of the beam current density over the necked segment with the possible refilling of atoms into the necked segment.10 We expect to track the kinetics of uniform axial elongation and radial shrinkage of NW in which we would become able to correlate accurately our modeling of NW nanocurvature effect11 via a uniform irradiation with a beam spot size large enough over the entire NW. Such a NW must be straight and uniform in diameter all the way in the axis direction with a surface smooth enough to avoid the effect of any detectable non-uniformity of the nanocurvature on the sidewall of the NW. Moreover, such a NW must be thin enough from the very beginning to ensure a shrinkage to a few nanometers in diameter where within a feasible irradiation duration, during which the beam current can be kept high enough without any appreciable fluctuation. Actually, it has been proven rather difficult to obtain such a beam or such a NW.10 To our best knowledge, up till now, there has no report found on such a uniform elongation and radial shrinkage of NW as purely induced by a uniform irradiation of e-beam. Hence, the nanocurvature effect11 and the beam-induced atomic vibration soft mode and instability (or beam-induced athermal activation) effect11 underlying the as-induced uniform elongation and radial shrinkage of NWs remain unclear.Based on the above consideration, in this paper we particularly study the uniform elongation and radial shrinkage of a-SiOx NWs as purely induced by a uniform irradiation of non-focused e-beam in an in situ TEM. It was observed that the straight and uniform a-SiOx NW presented a tensile pulling-free uniform plastic elongation and an accelerated uniform radial shrinkage at the nanoscale intriguingly. According to our new model for NW taking into account the nanocurvature of the wire, the kinetic relationship between the shrinking wire radius and the irradiation time was established. The fitting results showed a much higher, curvature-dependent wire surface energy at the nanoscale than that predicted from the existing theories on the shrinkage (that is, a pronounced nanocurvature effect on the elongation and shrinkage). Furthermore, the arresting plastic flow of massive atoms and surface diffusion of atoms at room temperature demonstrate a direct experimental evidence for our predicted athermal soft mode and instability of atomic vibration as induced under non-focused, uniform e-beam irradiation in condensed matter.
2. Experimental
The a-SiOx NWs with the desired diameter were fabricated by our improved chemical vapor deposition technique where x is determined to be 2.3 (ref. 12). Such fabricated NWs can be straight and uniform in diameter all the way in the axis direction with a surface smooth enough to avoid the effect of any non-uniformity of the nanocurvature on the sidewall of wire. The as-fabricated NWs were well-dispersed in ethanol and then deposited onto the holey carbon film of microscopy grid for TEM studies. The as-prepared TEM specimens were irradiated at room temperature and the structure change and evolution of SiOx NWs was examined using our developed in situ TEM observation technique via a field-emission Tecnai F-30 TEM operating at a high tension of 300 kV. The irradiation was always targeted on individual straight NW segments with uniform diameter and clean and smooth surface which were protruding into the open space of the holes in the carbon film of microscopy grid. During the experiment, we always tilted the sample to make sure that axis of the wire is normal to the e-beam if there was any deviation of the wire from this position. In doing so, we ensured that there is no reduction in the projected or measured length of the wire which may be caused by any upward or downward tilting of the wire. Also, during the experiment, the beam current density at the specimen was kept uniform without any appreciable fluctuation over an area (about 600 nm in diameter) much larger than the zone or NW segment observed (about 200 nm in length). In doing so, with the accelerating voltage at 300 kV, the penetration depth of the electrons much exceeded the sample thickness or the NW diameter and the beam energy deposition rate would be uniform over all the materials in the sample.10 During the observation or taking a picture, the beam was spread to an around 100 times weaker intensity so that the corresponding irradiation effect can be minimized to a negligible degree and at the same time the image contrast can also be improved. Also note that during the electron irradiation, the beam was determined to heat the irradiated NW by no more than a few degrees10,13,14 due to its extremely large ratio of surface to volume and the dominant irradiation effect should be athermal. Therefore, it could be considered that the irradiated NW essentially remained at room temperature throughout the irradiation duration. In the experiments, the wire diameter was taken as an average value of several wire diameters which are measured at different representative positions across the wire from each micrograph; while the length and volume of the wire segment were measured and calculated between two red mark dots in Fig. 1 along the wire axis. The two red dots were carefully marked at the locations of two selected feature points at the two ends of wire and by further checking the relative positions of their surrounding feature points on the wire surface (see the arrows in Fig. 1). To observe the details of the two feature points, during marking we always enlarged TEM images to achieve a sufficient resolution. In doing so, the two feature points in each micrograph are observable due to the little but increasing coarseness on the wire surface. For a further confirmation, we performed the length measurement for many times by selecting other feature points or other NWs and similar results of the length change were observed.3. Results and discussions
The sequential TEM micrographs in Fig. 1 show the typical structure change and evolution of an individual a-SiOx NW segment during the uniform e-beam irradiation at room temperature. Prior to the irradiation, as shown in Fig. 1(a), the a-SiOx NW segment within the two red mark dots has a well-defined straight, smooth, and clean cylinder shape with an initial length of 148 nm with its two ends lying on the supporting carbon film. With the irradiation turned on, as demonstrated in Fig. 1(a–k) and 2, a continuous extension in the segment length was occurring intriguingly. Furthermore, the segment almost kept its straight and smooth cylinder shape until the marked wire segment being extended to a length about 192 nm before breakage as shown in Fig. 1(k). Without any external assistance of tensile pulling as used in ref. 7–9, such a uniform axial elongation from 148 nm to 192 nm within 3540 s irradiation with a net length extension of 44 nm in the segment demonstrates an average extension rate of 1.24 × 10−2 nm s−1 and an increment up to 30% in the length. Such a uniform elongation of the NW segment is also expected to be accompanied with a uniform radial shrinkage. Indeed, as experimentally demonstrated in Fig. 1(a–k), a notable uniform shrinkage in the wire diameter was observed from 19 nm to 8 nm, until finally leading to the wire breakage after irradiated to 3600 s (see Fig. 1(l)). Fig. 3 further shows the plot of radius of the NW segment in Fig. 1 against the irradiation time. It was observed that the shrinkage started to become intriguingly faster and faster when the radius reduced down to about 7 nm. In the existing literature, such an accelerated uniform radial shrinkage at the nanoscale under uniform e-beam irradiation has not been observed in NW, which should be greatly different from the cases of single-walled carbon nanotube (SWCNT)15 or nanocavity in Si11 due to the unique amorphous NW structure. Several similar uniform irradiations on other NW segments by lowering the beam current density to different levels down to 1 A cm−2 were repeated. It was observed that the essential features of the structure change and evolution were the same as shown in Fig. 1 although the elongation and radial shrinkage were both slower and less-proceeded when the wires were irradiated at a lower current density (for more about the beam current effect on the athermal diffusion and the athermal evaporation, see the later-on analysis and modeling). We also note that there is still a little coarseness on the NW segment surface after a long period of irradiation, as shown in Fig. 1. This is probably due to the minor non-uniformity or fluctuation either in local beam current density or in local wire structure or in both during the irradiation, which seems to be inevitable at a prolonged, later-on irradiation stage.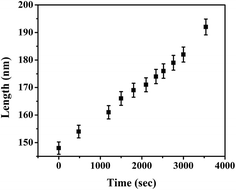 | ||
| Fig. 2 The change of length of the NW with the irradiation time as measured from Fig. 1. The length is measured as the length of the irradiated wire segment between the two red feature dots as shown in Fig. 1 to trace the change of wire length. | ||
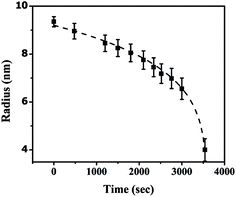 | ||
| Fig. 3 The uniform shrinkage of the SiOx NW radius (r) with the irradiation time (t) as observed in Fig. 1. The dashed line is the fitting result according to the eqn (8) with a value of the constant b at about 3.5. | ||
The interaction between energetic e-beam and nanostructures including low dimensional nanostructures (LDNs) has been conventionally attributed to knock-on mechanism14 or even e-beam heating effect.1,16,17 However, our previous experiments demonstrated that the knock-on mechanism and the related simulations neither are fully consistent with, nor can offer a full explanation for, the experimentally observed nanostructure change and evolution (i.e., nanoprocessing or nanostability), especially the above tensile pulling-free uniform elongation and the accelerated radial shrinkage at the nanoscale during the irradiation. This is because the existing theories such as the knock-on mechanism and their related simulations were at the first place built based on consideration of the nature of equilibrium, symmetry, periodicity, and linearity of bulk crystalline structure or its approximation, whereas the energetic beam (including electron, ion and photon beams)-induced nanoprocessing or nanostability of nanostructure is intrinsically of non-equilibrium, amorphous, and non-linear nature. Moreover, the atomic movements at the scale of atomic bond length are difficult to be observed with the current TEM techniques, and thus a direct comparison of the simulated atomic movements with the experimental results is impossible. On the other hand, as mentioned in the experimental part, due to the extremely high ratio of surface to volume of NW at the nanoscale, the e-beam irradiation is determined to heat the specimen by no more than a few degrees10,13,14 and the dominant irradiation effect should be athermal. In the experiment, we also observed that even after the above elongation and shrinkage processed for a time, they would stop immediately once the irradiation was suspended. This further demonstrates that the processes are predominately driven by an irradiation-induced, instant, athermal activation rather than a beam heating-induced, slow, thermal activation. In fact, our previous work on the energetic beam irradiation-induced nanoinstability or nanoprocessing of LDNs, such as nanocavities in Si,11 carbon nanotubes15,18,19 and amorphous SiOx nanowires6,10 has proven that our proposed novel nanocurvature effect11 and energetic beam-induced atomic vibration soft mode and instability effect11 are universal concepts and applicable in the prediction or explanation of all the above energetic beam irradiation-induced nanophenomena. In the following, we attempt to reveal the above two effects on the uniform elongation and the accelerated uniform radial shrinkage at the nanoscale of a-SiOx NW under uniform e-beam irradiation.
For the nanocurvature effect on a NW, we can suppose that, similar to the particle case,11 when the radius r of a NW approaches its atomic bond length d, a positive nanocurvature on the highly curved wire surface will become appreciable. Such a positive nanocurvature would cause an additional tensile stress on the electron cloud structure of surface atoms which would lead to a dramatic increase in surface energy of the NW as schematically shown in Fig. 4(a). This dramatically increased surface energy would lower the energy barrier for the surface atoms to migrate or escape thus causing an intrinsic nanoinstability in the NW. Especially, it would give rise to a strong tendency of self-contraction of the nanocurved wire surface and thus provide a thermodynamic driving force for the wire to shrink in the radius direction and a corresponding massive atom plastic flow as self-extruded towards two ends of the wire.
Although the positive nanocurvature on a NW can lower the energy barrier of surface atoms and thus cause the nanoinstability thermodynamically, a further assistance from the external excitation such as energetic beam irradiation is still needed to soften the wire and kinetically activate the shrinkage or contraction and the plastic flow. In the case of energetic e-beam irradiation in TEM, we can assume that beam energy deposition rate6 of the incident energetic beam can be so fast that there is no enough time for the deposited energy to transfer to thermal vibration energy of atoms within a single period of atomic vibration. In this way, the mode of atom thermal vibration would be softened or the vibration of atoms would lose stability.11 The induced soft mode or instability of the atomic vibration can further suppress the energy barrier for the atoms to migrate or escape and finally make the structure changes kinetically possible.
The above processes can be schematically illustrated in Fig. 4(b). When the NW segment is subjected to a uniform irradiation of e-beam, the mode of the atom thermal vibration is softened or the vibration of atoms loses stability within the whole irradiated NW segment. As a result, the energy barriers of atoms in the NW segment were suppressed, and the atoms became unstable and easy to diffuse or even flow towards two ends of the wire or evaporate into the vacuum under driving of the effect of self-extruding or self-contracting of the nanocurved wire surface. Meanwhile, since the nanocurvature distributed over the wire surface is also uniform along the wire axis, the as-induced athermal diffusion and athermal evaporation (we can also simply call them as diffusion and evaporation for short) of atoms (including athermal plastic flow of bulk massive atoms) would proceed uniformly along the wire axis. In this way, as demonstrated in Fig. 1 and further illustrated in Fig. 4(b), a uniform axial elongation and a uniform radial shrinkage would occur in the a-SiOx NW during the irradiation of a uniform e-beam. Thus, the novel plastic flow of massive atoms and surface diffusion of atoms offer a direct experimental evidence for our predicted athermal soft mode and instability of atomic vibration as induced by uniform e-beam irradiation in condensed matter. In particular, with the increase of irradiation time, the NW became thinner and demonstrated an intriguing accelerated radial shrinkage at the nanoscale as shown in Fig. 3. This can be attributed to the nanocurvature effect of the NW during the NW radius is thinning down to the nanoscale.
It is expected that only diffusion of atoms (including plastic flow of massive atoms) leads to the axial elongation of NW whereas both evaporation and diffusion of atoms result in the radial shrinkage of NW. Also, in ref. 6, it has been reported that the relative contribution of the atom diffusion or the atom evaporation to the structural changes of NW is fully adjustable or tunable by the irradiation parameters which determine the beam energy deposition rate such as accelerating voltage and beam current density, etc. as shown in eqn (1) in ref. 6. For example, for a specific current density of 5.66 A cm−2 in the present case, after a similar calculation via eqn (1) and (2) in ref. 20, we can obtain a value of the evaporated volume larger than that of the diffused volume. It thus indicates that at this current density or energy deposition rate the evaporation of atoms rather than the diffusion of atoms dominates the radial shrinkage of wire. At the same time, we can further lower down the beam energy deposition rate with a reducing in the beam current density from 5.66 to 1 A cm−2 or in the accelerating voltage from 300 to 200 kV so that we can achieve a diffusion-dominated nanoprocess. Although a slower radial shrinkage and a slower elongation would occur at a lower beam energy deposition rate, in the end, we can easily achieve a uniform elongation and accelerated radial shrinkage in amorphous SiOx nanowire fully caused by athermal atom diffusion or athermal plastic flow without any athermal atom evaporation or ablation with an irradiation at an enough lower beam energy deposition rate.
To reveal the kinetics of the accelerated radial shrinkage during the uniform elongation, we can consider an irradiated NW segment of any fixed length of L, as illustrated in Fig. 4 and correlated the thermodynamic driving force for the radius shrinkage with the surface energy (capillary force) of the wire through Laplace law
| F = (2πrL)σ/r, | (1) |
| σ = σ0 + α/rb, | (2) |
| σ ≈ α/rb. | (3) |
From kinetic consideration, we can further assume that rate of the mass transportation (decreasing number of atoms within the irradiated NW segment of the fixed length of L) during the elongation and the shrinkage is proportional to the driving force21 as shown in eqn (1). Note that the decreased number of the atom includes both the number of atoms diffused (or flowed) out of and that evaporated out of the irradiated NW segment of the fixed length of L. In this way, we can get
| −d(πr2Lρ)/dt ∝ F, | (4) |
| −d(πr2Lρ)/dt = KF. | (5) |
In eqn (4) or (5), ρ is the volume density of atoms which is taken as a constant value here, and K is the thermodynamic reaction constant which is defined as K = K0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(−ΔG*/RT), where K0 is a constant, R is the gas constant, T is the temperature, and ΔG* is the activation energy for the structure change.15 Combining eqn (1), (3), and (5), we then have
exp(−ΔG*/RT), where K0 is a constant, R is the gas constant, T is the temperature, and ΔG* is the activation energy for the structure change.15 Combining eqn (1), (3), and (5), we then have
| rb+1dr = −K′dt, | (6) |
| rt=0 = R0, rt=t = r(t). | (7) |
By integrating the eqn (6) and employing the conditions given in eqn (7), r takes the form as given below
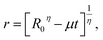 | (8) |
Based on the above considerations, we made a nonlinear fitting of the observed radius evolution of the NW as shown in Fig. 3 to the eqn (8). The dashed line in Fig. 3 shows a well-fitted result of the experimental data which gives a fitted value of the constant b at 3.5. This indicates that the additional curvature effect at the nanoscale on the surface energy of the NW is proportional to 1/r3.5 instead of 1/r2 as predicted from the existing theories.15 That is, the fitting results demonstrate a much faster increase of the surface energy with the curvature at the nanoscale than that predicted from the existing theories (called nanocurvature effect as defined previously). The reason for such a discrepancy is because the existing, related calculations have to rely on some approximations based on equilibrium, symmetric, periodic, and linear nature of a bulk crystalline structure, which may greatly underestimate real curvature effect of LDNs at the nanoscale. In fact, when the radius r of wire reduces down to a value comparable with the atomic bond length, the surface energy increases dramatically in the form of α/r3.5, which could cause a strong tendency of self-compression on the nanocurved wire surface thermodynamically. In this way, under the external activation of the uniform e-beam irradiation, the dramatically increased surface energy can athermally drive the near-surface atom diffusion or even plastic flow of massive atoms towards the two ends of wire as well as the near-surface atom evaporation into the vacuum. As a result, the a-SiOx NW shows a tensile pulling-free plastic elongation and an accelerated radial shrinkage at the nanoscale under the uniform e-beam irradiation, as demonstrated in Fig. 1–3.
In our previous work, we have obtained the constant b at a fitted value of 6 for the observed SWCNT radial shrinkage15 only via beam-induced athermal evaporation of atoms which is larger than that in the present case of a-SiOx NW at a fitted value of 3.5. This can be well accounted for from the great differences of structure configurations or nanocurvature effects between the SWCNT and the a-SiOx NW. For the SWCNT, it can be regarded as a hollow tube structure rolled up from an sp2 monoatomic layer of covalent bond with an outer surface and an inner surface. As a result, both a strong tensile stress by a positive curvature on the outer surface of the tube wall and a strong compressive stress by a negative curvature on the inner surface11 would lead to the dramatic increase in the surface energy of SWCNT. However, for the a-SiOx NW, it is an amorphous solid cylinder structure of Si–O–Si bridge bond with only an outer wire surface where only the tensile stress by a positive curvature could cause the increase in the surface energy. It thus indicates a less-notable nanocurvature effect and a lower surface energy exerting on the a-SiOx NW, which supports the conclusion of the constant b for the a-SiOx NW is smaller than that for the SWCNT.
4. Conclusions
In summary, the structure change and evolution of a-SiOx NW under uniform e-beam irradiation were in situ investigated in TEM. It was observed that the straight and uniform a-SiOx NW intriguingly manifests itself by a tensile pulling-free uniform plastic elongation and an accelerated uniform radial shrinkage at the nanoscale. According to our new model for NW taking into account the NW curvature at the nanoscale, the kinetic relationship between the shrinking radius and the irradiation time was established. The fitting results showed a much higher curvature-dependent surface energy at the nanoscale than that predicted from the existing theories on the shrinkage. Furthermore, the plastic flow of massive atoms and surface diffusion of atoms indicate a direct experimental evidence for our predicted athermal soft mode and instability of atomic vibration as induced under uniform e-beam irradiation in condensed matter. In doing so, the study further demonstrates that the novel nanocurvature effect and beam-induced atomic vibration soft mode and instability effect, which have been normally neglected or inadequately taken into account in the current literature, are able to explain the beam-induced NW nanoinstability or nanoprocessing. Thus, the new concepts could offer a mechanism different from the classical knock-on mechanism and e-beam heating effect and thus would well reveal the fundamentals of the beam-induced nanostability or nanoprocessing of NW of non-equilibrium, amorphous, and non-linear nature.Conflicts of interest
There are no conflicts to declare.Acknowledgements
We acknowledge the support in the TEM observation from Qiming Hong at Xiamen University. This work was supported by the NSFC project under grant no. 11574255, the Science and Technology Plan (Cooperation) Key Project from Fujian Province Science and Technology Department under grant no. 2014I0016.References
- S. Xu, M. Tian and J. Wang, et al., Nanometer-scale modification and welding of silicon and metallic nanowires with a high-intensity electron beam, Small, 2005, 1, 1221–1229 CrossRef CAS PubMed.
- M. Remeika and A. Bezryadin, Sub-10 nanometer fabrication: molecular templating, electron-beam sculpting and crystallization of metallic nanowires, Nanotechnology, 2005, 16, 1172–1176 CrossRef CAS.
- E. F. Pecora, A. Irrera and S. Boninelli, et al., Nanoscale amorphization, bending and recrystallization in silicon nanowires, Appl. Phys. A, 2011, 102, 13–19 CrossRef CAS.
- S. Dai, J. Zhao and L. Xie, et al., Electron-beam-induced elastic-plastic transition in Si nanowires, Nano Lett., 2012, 12, 2379–2385 CrossRef CAS PubMed.
- S. Dai, M. He and J. Zhu, E-beam-induced in situ structural transformation in one-dimensional nanomaterials, Sci. Bull., 2015, 60, 71–75 CrossRef CAS.
- J. Su and X. Zhu, Atom diffusion and evaporation of free-ended amorphous SiOx nanowires: nanocurvature effect and beam-induced athermal activation effect, Nanoscale Res. Lett., 2016, 11, 514 CrossRef PubMed.
- H. Zheng, Y. Liu and S. X. Mao, et al., Beam-assisted large elongation of in situ formed Li2O nanowires, Sci. Rep., 2012, 2, 1–4 Search PubMed.
- X. Han, K. Zheng and Y. Zhang, et al., Low-temperature in situ large-strain plasticity of silicon nanowires, Adv. Mater., 2007, 19, 2112–2118 CrossRef CAS.
- K. Zheng, C. Wang and Y. Q. Cheng, et al., Electron-beam-assisted superplastic shaping of nanoscale amorphous silica, Nat. Commun., 2010, 1, 24 Search PubMed.
- X. Zhu, J. Su and Y. Wu, et al., Intriguing surface-extruded plastic flow of SiOx amorphous nanowire as athermally induced by electron beam irradiation, Nanoscale, 2014, 6, 1499–1507 RSC.
- X. Zhu and Z. Wang, Nanoinstabilities as revealed by shrinkage of nanocavities in silicon during irradiation, Int. J. Nanotechnol., 2006, 3, 492–516 CrossRef CAS.
- S. Huang, Y. Wu and X. Zhu, et al., VLS growth of SiOx nanowires with a stepwise nonuniformity in diameter, J. Appl. Phys., 2011, 109, 084328 CrossRef.
- J. Zhang, L. You and H. Ye, et al., Fabrication of ultrafine nanostructures with single-nanometer precision in a high-resolution transmission electron microscope, Nanotechnology, 2007, 18, 155303 CrossRef.
- F. Banhart, Irradiation effects in carbon nanostructures, Rep. Prog. Phys., 1999, 62, 1181–1221 CrossRef CAS.
- X. Zhu, L. Li and S. Huang, et al., Nanostructural instability of single-walled carbon nanotubes during electron beam induced shrinkage, Carbon, 2011, 49, 3120–3124 CrossRef CAS.
- D. Ugarte, Curling and closure of graphitic networks under electron-beam irradiation, Nature, 1992, 359, 707–709 CrossRef CAS PubMed.
- C. H. Kiang, W. A. Goddard and R. Beyers, et al., Structural modification of single-layer carbon nanotubes with an electron beam, J. Phys. Chem., 1996, 100, 3749–3752 CrossRef CAS.
- X. Zhu, L. Li and J. Su, et al., Beam-induced nonuniform shrinkage of single-walled carbon nanotube and passivation effect of metal nanoparticle, J. Phys. Chem. C, 2015, 119, 6239–6245 CAS.
- X. Zhu, H. Gong and L. Yang, et al., Non uniform shrinkages of double-walled carbon nanotube as induced by electron beam irradiation, Appl. Phys. Lett., 2014, 105, 093103 CrossRef.
- J. Su and X. Zhu, Passivation effect of gold nanoparticles on uniform beam-induced structural changes of amorphous SiOx nanowire, J. Phys. Chem. C, 2017, 121, 15977–15983 CAS.
- X. Zhu, Shrinkage of nanocavities in silicon during electron beam irradiation, J. Appl. Phys., 2006, 100, 034304 CrossRef.
Footnote |
| † Both authors contributed equally to this work and should be considered as co-first authors. |
| This journal is © The Royal Society of Chemistry 2017 |


