Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
The photophysics of naphthalene dimers controlled by sulfur bridge oxidation†
Clàudia
Climent
a,
Mario
Barbatti
 b,
Michael O.
Wolf
b,
Michael O.
Wolf
 c,
Christopher J.
Bardeen
d and
David
Casanova
c,
Christopher J.
Bardeen
d and
David
Casanova
 *ef
*ef
aDepartament de Ciència de Materials i Química Física, Institut de Química Teòrica i Computacional (IQTCUB), Universitat de Barcelona, Martí i Franquès 1-11, Barcelona 08028, Spain
bAix Marseille Univ, CNRS, ICR, Marseille, France
cDepartment of Chemistry, University of British Columbia, 2036 Main Mall, Vancouver, BC V6T 1Z1, Canada
dDepartment of Chemistry, University of California Riverside, 501 Big Springs Road, Riverside, California 92521, USA
eKimika Facultatea, Euskal Herriko Unibertsitatea (UPV/EHU), Donostia International Physics Center, Paseo Manuel de Lardizabal, 4, Donostia 20018, Spain. E-mail: david.casanova@ehu.eus
fIKERBASQUE, Basque Foundation for Science, Bilbao 48013, Spain
First published on 24th April 2017
Abstract
In this study we investigate in detail the photophysics of naphthalene dimers covalently linked by a sulfur atom. We explore and rationalize how the oxidation state of the sulfur-bridging atom directly influences the photoluminescence of the dimer by enhancing or depriving its radiative and non-radiative relaxation pathways. In particular, we discuss how oxidation controls the amount of electronic transfer between the naphthalene moieties and the participation of the SOn bridge in the low-lying electronic transitions. We identify the sulfur electron lone-pairs as crucial actors in the non-radiative decay of the excited sulfide and sulfoxide dimers, which are predicted to proceed via a conical intersection (CI). Concretely, two types of CI have been identified for these dimers, which are associated with the photo-induced pyramidal inversion and reverse fragmentation mechanisms found in aryl sulfoxide dimers. The obtained results and conclusions are general enough to be extrapolated to other sulfur-bridged conjugated dimers, therefore proportionating novel strategies in the design of strongly photoluminescent organic molecules with controlled charge transfer.
1. Introduction
Organic photovoltaics (OPVs) represent a very promising alternative in the conversion of solar energy to electricity. Although OPVs have the potential to provide electricity at a lower cost than the first and second generations of solar technology, the current record efficiencies (∼13%)1 are still far too low to compete with the performance of silicon panels2 and other non-fossil energy sources. Organic solar cells present numerous advantages, i.e. an abundance of materials, well-developed organic chemistry for their synthesis, available chemical strategies to tune their properties and they can be produced as thin, flexible and light modules that can be easily manufactured at room temperature. However, in order for OPV technology to compete with other energy sources some fundamental obstacles need to be overcome. In particular, OPV technologies exhibit short device lifetimes and low dielectric constants, resulting in low energy conversion efficiency. One of the fundamental issues at the microscopic level is the generation of separated charges from the optical exciton. High exciton binding energies result in energy losses at the cell heterojunction that induce rather low open-circuit voltages (VOC). A promising and sophisticated strategy to increase VOC in OPVs is the use of symmetric molecular electron acceptors, such as covalent dimers of organic chromophores (bichromophores) that are able to undergo symmetry-breaking charge transfer (SBCT).3–5 In SBCT, the initial excitation generated by photoabsorption relaxes to an intramolecular charge transfer state that breaks the molecular symmetry. Then, electron CT at the donor/acceptor interface leads to an oxidized donor and a reduced acceptor separated by a neutral chromophore, preventing fast charge recombination. Organic bichromophores have also been proposed as highly emissive molecules to use in organic light emitting devices (OLEDs)6,7 as an alternative to large aromatic molecules. In addition to strong photoluminescence (PL), optimal molecular systems to be used in OLEDs must allow intermolecular CT.8The range of applicability of bichromophores is expected to be related to the nature of the bridged monomers and their interactions. The electronic coupling between the conjugated moieties depends on the geometry and electronic structure of the covalent linker, and understanding the parameters that ultimately control and determine such interactions becomes critical for the design of molecular systems with the desired characteristics. Recently, it was shown that the covalent linkage between conjugated chromophores via a sulfur bridge has a large impact on the fluorescence efficiencies of the parent chromophores, with a large increase in the PL yield observed upon the oxidation of the bridging sulfur atom, indicating a clear strategy in the search of strong molecular emitters.9 This trend was later scrutinized in the case of terthiophene dimers.10 The study concluded that rapid intersystem crossing (ISC) to the triplet state manifold is the main deactivation process limiting the fluorescence quantum yield, as observed in pristine terthiophene.11,12 The ISC efficiency is reduced in the presence of intramolecular charge transfer (CT), which can be tuned by the oxidation state of the bridging sulfur group. The electron lone-pairs on the sulfur atom screen the electronic interactions between the two chromophores, decreasing the CT and allowing efficient ISC for the sulfide and sulfoxide dimers, resulting in a larger PL efficiency for the sulfone bridge with no electron lone-pairs on S.
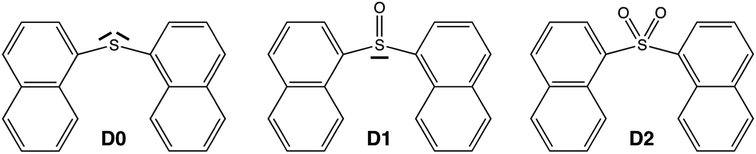
Despite the much less efficient ISC expected for naphthalene due to the molecular planarity and lack of sulfur atoms, the PL in SOn bridged naphthalene dimers exhibits the same trend as that found in the terthiophene analogues. Hence, in order to understand the origin of such behavior and to further explore the validity of the lone-pair screening concept, the present study features the photophysical properties of naphthalene covalent dimers linked through an SOn bridge, where n = 0, 1 and 2. We label these three molecules as D0 (sulfide), D1 (sulfoxide) and D2 (sulfone) throughout the paper (Scheme 1).
 | ||
| Scheme 1 Molecular representation of the Dn dimers. The sulfur electron lone pairs are explicitly indicated. | ||
Our study is organized as follows. First, we discuss the relative stability of the structural conformers of the Dn dimers and their potential interconversion paths. Then, we explore the nature of the low-lying singlet states of the low-energy conformers and the structural and electronic properties of the local minima on the excited state energy surface. Finally, we discuss the availability of non-radiative decay pathways from the lowest excited singlet to the ground state.
2. Computational details
Electronic structure calculations for the ground and excited states were performed within the framework of density functional theory (DFT)13,14 and its time-dependent version (TDDFT),15,16 respectively. To take into account the weak interactions and important electronic redistribution between the naphthalene moieties and the SOn bridge upon photoexcitation, the ωB97X-D functional17 was used together with the 6-31+G(d) basis set.18–20 Investigations on the dependence of the energy functional and basis set can be found as ESI (Tables S1 and S2†). Dichloromethane (DCM) solvent effects were taken into account with the polarizable continuum model using the integral equation formalism variant (IEFPCM).21,22 Critical points on the ground state potential energy surface (PES) were optimized with no restrictions and characterized within the harmonic approximation. The simulated emission spectra were calculated by convolution of the Gaussian functions (half-bandwidth of 2500 cm−1) centered at the computed vertical emission energies of all excited state minima and were averaged according to the ground or excited state Boltzmann populations (based on the relative electronic energies). Computation of the diabatic states was performed by means of the Edmiston–Ruedenberg localization scheme.23 Energy crossing points and derivative couplings between S0/S1 were computed at the spin-flip DFT (SF-DFT) approximation24 with the BHHLYP functional.25,26All calculations were performed with the Gaussian09 package, revisions B01 and D01,27 and the Q-Chem program.28
3. Results and discussion
3.1. Thermal conformers
The rotation around the S-naphthalene bonds in the Dn dimers results in different structural conformers, which are local minima on the ground state PES (Fig. 1 and S1†).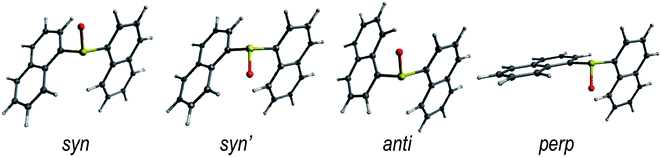 | ||
| Fig. 1 Lowest energy conformers for the ground state of the D1 dimer in DCM solution. The low energy conformers of D0 and D2 are shown in Fig. S1.† | ||
The energetically lowest forms of D0 and D1 in solution correspond to the syn- and anti-arrangements of the naphthalene units with syn being slightly lower in energy (Table 1). As a result, it is reasonable to expect both conformers to be present in the solution as indicated by their Boltzmann fractions. On the other hand, D2 shows a clear preference for the anti conformer, which is expected to be the main form found in DCM solution.
| Dimer | Conf. | ΔE | Pop. | q (S) | q (On) | q (Naph2) |
|---|---|---|---|---|---|---|
| D0 | syn | 0.0 | 74 | 0.00 | — | 0.00 |
| anti | 0.7 | 23 | 0.20 | — | −0.20 | |
| anti′ | 1.8 | 3 | −0.04 | — | 0.04 | |
| D1 | syn | 0.0 | 69 | 0.68 | −0.69 | 0.01 |
| syn′ | 3.5 | <1 | 0.48 | −0.69 | 0.21 | |
| anti | 0.5 | 30 | 0.68 | −0.71 | 0.03 | |
| perp | 2.8 | <1 | 0.54 | −0.69 | 0.14 | |
| D2 | syn | 2.5 | 1 | 0.18 | −0.97 | 0.79 |
| anti | 0.0 | 94 | 0.27 | −0.95 | 0.68 | |
| perp | 1.8 | 5 | 0.92 | −0.99 | 0.08 |
It is worth noting that while D0 only shows minor net charges in any of the fragments, i.e. the two naphthalenes and sulfur bridge in its anti conformer, the polarity of the S–O bond in the sulfoxide and sulfone bridges induces an electronic distribution towards the more electronegative O atoms (Table 1). Charge polarization in D1 basically affects the SO linker, with the S and O atoms carrying positive and negative charges, respectively. The presence of the two O atoms in D2 is able to pull considerable electron density from the two naphthalene units in the syn- and anti-conformers, resulting in a positive net charge on each chromophore. The different behavior observed for the perp form is related to the orthogonal orientation between naphthalene units, which results in asymmetrically charged chromophores.
In addition to identifying and characterizing the most stable conformations of the naphthalene dimers, it is also important to quantify the barriers for their interconversion. The structural transformation between the low energy conformers of each dimer can be achieved by the torsion of one naphthalene moiety with respect to the other one. The ground state energy profiles of these mechanisms are shown in Fig. S2–S4.† The computed barriers for the molecular torsion between the low-lying conformers are in the range of 2–6 kcal mol−1.
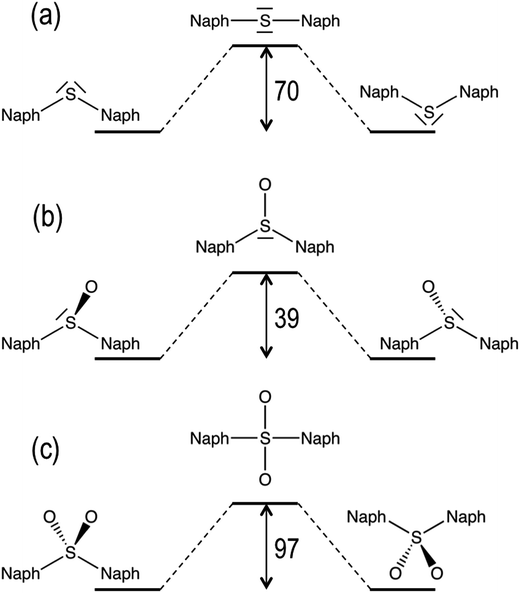
In addition to molecular torsion, the conversion between the conformers of D1 can be achieved by pyramidal inversion of the S atom (Fig. 2). The transition states for the inversion of the syn- and anti-conformers exhibit a planar geometry around the sulfur atom with a naph-S-naph angle close to 120°, i.e. corresponding to a trigonal planar geometry, and much larger than the ground state angle (close to the tetrahedral angle). The computed inversion barriers for the syn → syn′ and anti → anti conformational pathways are 38.4 and 38.6 kcal mol−1, respectively, in quantitative agreement with the computational estimations of the pyramidalization barrier for H2SO, DMSO29 and related sulfoxide heterodimers.30 Hence, thermal pyramidal inversion of the sulfoxide dimer is expected to be very slow, as previously observed for the racemization of aryl sulfoxides.31,32 Analogous to the pyramidal inversion observed in D1, the syn- and anti-conformers of the D2 dimer may interconvert by a tetrahedral inversion through the planarization of the sulfone center through a square planar transition state. Our calculations indicate that this geometry is very high in energy (97 kcal mol−1 with respect to the ground state anti-conformer), and hence thermal interconversion of the sulfone-bridged naphthalene dimer via planarization can be completely disregarded. In spite of the lack of oxygen atoms in the D0 bridge, it can also experience a similar inversion of the molecular structure by increasing the naph-S-naph angle at the bridge from the tetrahedral value (105°) in the syn and anti-ground state minima to a linear C–S–C disposition. Again, the computational estimation of the energy barrier for the structural inversion of D0 is too high (70 kcal mol−1) to be thermally available, which is in very good agreement with the linearization energy estimated for H2S.33
3.2. Photoabsorption
The computed vertical excitation energies from the ground state to the lowest excited singlet state of the Dn dimers are rather close to each other regardless of the oxidation state of the sulfur bridge and lie within the range of 4.2–4.4 eV, in fairly good agreement with the experimental absorption maxima measured in DCM solution (Table 2). Moreover, the transition energies and oscillator strengths show small variations between the different conformers.The main contribution to the lowest electronic transition from the most stable conformations (syn and anti) of the naphthalene dimers corresponds to a single electron promotion from the two highest occupied molecular orbitals (HOMO and HOMO−1) to the two lowest unoccupied molecular orbitals (LUMO and LUMO+1). The frontier orbitals are mostly delocalized over the two naphthalene moieties with some contribution from the SOn bridge, mainly for the HOMOs of the D0 and D1 molecules (Fig. 3).
 | ||
| Fig. 3 HOMOs (bottom) and LUMOs (top) of the syn-D0 (left), syn-D1 (middle) and anti-D2 (right) dimers computed in DCM solution. | ||
Despite the similarities between excitation energies and oscillation strengths of the S0 → S1 transition in the D0, D1 and D2 dimers, detailed electronic structure analysis brings to light the significant differences in the nature of the transition upon oxidation of the bridge (Table 2). Decomposition of the electronic transition in terms of diabatic states corresponding to the local excitations (LE) on the naphthalene moieties, charge transfer excitations between the aryl fragments (CT) and electronic transition from the bridge to the naphthalene chromophores (CTB) highlight the important differences in the nature of the vertical excitation upon oxidation of the sulfur atom linker (Table 2). In general, the main contribution corresponds to the π → π* excitations on both naphthalene moieties, particularly for the sulfone case. This contribution accounts for ∼90% of the transition in D1 and has proportionally a much lower role in the excitation of the D0 conformers. This decrease in the naphthalene-centered excitations is related to the larger involvement of the SOn orbitals in the transition, corresponding to the electron lone pairs on the sulfur (D0 and D1) and oxygen (D1) atoms, i.e. n(S) and n(SO) respectively. The presence of electron lone-pairs in the sulfide and sulfoxide linkers allows for sizeable CTB contributions, related to the different electron density distributions found in the HOMOs (Fig. 3). CTB contributions are already rather important in D1 (15% in the lowest syn conformer) and become the main contribution in syn-D0 (49%). On the other hand, the lack of available lone-pairs forbids the bridge-to-naphthalene electronic transitions in the S1 state of D2. For most of the cases, charge transfer between the two naphthalene fragments (CT) plays a minor role in the lowest excitation of the Dn dimers. It is worth noticing that the LE/CT electronic coupling increases with the number of oxygen atoms in the bridge. This trend can be attributed to the electronic screening by the sulfur electron lone-pairs, which limits the interactions between the two sets of π-electrons, as recently discussed in sulfur-bridged terthiophene dimers.10 On the other hand, the LE/CTB couplings are much larger in the sulfide than in the sulfoxide bridge in accordance with the amount of CTB in the excitation, which can be rationalized as a result of the presence of one additional electron lone-pair in the former.
3.3. Fluorescence emission
Thorough computational searches of the local minima on the lowest excited state PES of the naphthalene dimers identified a variety of states susceptible to decay back to the ground state via fluorescence emission (Table 3). The Dn dimers exhibit different structurally relaxed states corresponding to the stabilization of the π → π* excitations either localized on one naphthalene unit (L) or delocalized over both conjugated chromophores (D) or to the optimization of CT excitations from the SOn bridge (for n = 0 and 1) to the π* naphthalene empty orbitals (CTB). The lack of sulfur lone-pairs prohibits the stabilization of bridge CTB states on the S1 PES of D2, in line with the decomposition of the lowest excitation at the Franck–Condon (FC) geometry (Table 2).| Dimer | Conf. | Character | ΔEa (em) | f | ΔEb (Stokes) | ΔE(rel) |
|---|---|---|---|---|---|---|
| a The experimental emission maxima were obtained at 3.37 eV for the three dimers, while the measured Stokes shifts were 0.74, 0.82 and 0.79 eV for D0, D1 and D2, respectively.9 b The computed Stokes shift with respect to the vertical absorption of the most stable ground state conformer. | ||||||
| D0 | syn | π → π* (E) | 3.12 | 0.110 | 1.12 | 6.7 |
| syn | n(S), π → π* (L) | 3.58 | 0.316 | 0.66 | 1.9 | |
| anti | n(S), π → π* (L) | 3.39 | 0.215 | 0.85 | 2.6 | |
| syn | n(S), π → π* (D) | 3.51 | 0.325 | 0.73 | 2.1 | |
| anti | n(S), π → π* (D) | 3.43 | 0.322 | 0.81 | 0.0 | |
| D1 | syn | π → π* (E) | 2.95 | 0.078 | 1.45 | 0.0 |
| syn′ | π → π* (E) | 2.88 | 0.070 | 1.52 | 1.1 | |
| anti | n(SO), π → π* (L) | 3.65 | 0.191 | 0.76 | 3.7 | |
| perp | n(SO), π → π* (L) | 3.42 | 0.238 | 0.99 | 4.3 | |
| perp′ | n(SO), π → π* (L) | 3.23 | 0.167 | 1.18 | 3.6 | |
| syn | n(SO) → π* (D) | 3.06 | 0.004 | 1.35 | 2.9 | |
| anti | n(SO) → π* (D) | 3.02 | 0.019 | 1.39 | 2.8 | |
| D2 | syn | π → π* (E) | 2.92 | 0.077 | 1.50 | 0.0 |
| anti | π → π* (L) | 3.69 | 0.217 | 0.74 | 3.7 | |
| perp | π → π* (L) | 3.78 | 0.262 | 0.65 | 6.4 | |
The syn-Dn dimers hold excited state minima with excimeric nature and molecular geometries with the two naphthalene units close to the coplanar eclipsed relative orientation (Fig. S5†). These states present the largest Stokes shift for each dimer and are built as naphthalene π → π* excitations delocalized over the two chromophores without any involvement of the sulfur bridge. In addition, the large weights of the CT excitations (50% of the transition) for these states unequivocally identify them as naphthalene excimers (Fig. S6†). It is worth noting that while in D0 the excimer is the energetically highest optimized excited state conformer, it is the most stable state in D1 and D2, with larger interstate gaps in the latter. Moreover, the adiabatic energy gap with respect to the ground state syn conformer decreases as follows: D0 > D1 > D2, indicating a stronger excimer stabilization for the higher oxidation states of the bridge. The LE/CT couplings for the three syn excimers were computed at 626 (D0), 643 (D1) and 707 (D2) meV, considerably larger than the values obtained for the FC structures and with a trend in accordance with the electron lone-pairs screening of the electronic interactions.
The excited states with the highest oscillator strengths for the naphthalene dimers correspond to the π → π* excitations localized on one naphthalene or to the mixing between the π → π* and n(SOn) → π* (D0 and D1) excitations. The computed vertical emission energies and Stokes shifts for these states are in very good agreement with the experimental measurements. The D1 dimer also exhibits syn and anti low-lying states with virtually pure CTB character and small oscillator strengths. The emission energies for the n(SOn), π → π* (L) states are in very good agreement with the photoluminescence frequencies and intensities computed using the model systems with only one naphthalene unit (Table S3†), confirming the localized nature of the transition.
The excited state PES along the molecular torsion between the two naphthalene moieties of Dn dimers exhibit similar energy profiles to the ground state PES, with energy barriers for the conversion between the different conformers within the range of 2–7 kcal mol−1 (Fig. S2–S4†). The energy profiles of S0 and S1 PES around the ground state local minima are rather parallel, suggesting that depending on the experimental excitation conditions, two limiting situations might arise: (i) the initial excitation does not modify the conformer population and the emitting states are entirely controlled by the ground state equilibria or (b) the final emitting states are dictated by the relative stabilities between the minima in the S1 PES (excited state equilibria). The latter situation would be closer to the case with excitation energies high enough to surpass the torsion barriers. The simulated emission spectra recorded in DCM for the two limiting situations are shown in Fig. 4.
Estimation of the relative photoluminescence (PL) quantum yields obtained from the integration of the emission profiles (Table S5†), either considering ground or excited state Boltzmann populations (Fig. 4a and b respectively), indicates the D0 dimer as the stronger emitter with a fluorescence efficiency three to four times larger than D1 and D2. This result is in complete disagreement to the experimental observations, i.e. a much larger PL quantum yield (about one order of magnitude or more) in D2 with respect to the D0 and D1 dimers.9 At this point, we must conclude that different state distributions over the computed S1 minima cannot account for the different PL efficiencies between the sulfur-bridged naphthalene dimers and that one or more non-radiative relaxation pathways (not considered so far) may play an important role in the deactivation of the D0 and D1 dimers. Furthermore, we explore the potential non-radiative S1 → S0 decays and rationalize how these mechanisms are favored in D0 and D1, but not in D2, resulting in the much larger emission intensity found for the latter.
3.4. Non-radiative relaxation pathways
First, we consider the possibility of efficient internal conversion (IC) in the D1 dimer following the pyramidal inversion mechanism on the excited state PES, as it has been proposed as a viable photo-induced process in aryl sulfoxides.30 The energy barriers computed for the inversion of the syn and antiD1 conformers are 3.5 and 3.0 kcal mol−1, respectively, which are much lower than the energy required for the same structural rearrangement in the ground state (39 kcal mol−1). Hence, it seems that the inversion may be thermally available after photo-excitation of the sulfoxide dimer. However, for such a mechanism to result in an efficient IC to the ground state, a strong non-adiabatic coupling between the two states is required. Since the interstate couplings are inversely proportional to the energy gap, a small energy difference between the two PESs is necessary. Estimation of the S0/S1 energy gaps at the inversion TS were computed to be 32 and 50 kcal mol−1 for the syn and anti conformers, respectively (Table S6†). Hence, despite the availability of the photo-induced pyramidal inversion in D1, the magnitude of the S0/S1 gaps forced us to rule out the efficient non-radiative decay via IC at the inversion TS. Although the computed tetrahedral inversion barrier for D2 in the lowest excited state is also considerably much lower than the ground state value, i.e. 41 vs. 97 kcal mol−1, the barrier is still too large to allow for photo-induced tetrahedral inversion. Furthermore, the S0/S1 energy gap at the TS is estimated at 29 kcal mol−1, blocking the non-radiative decay to the ground state via IC. Similar results have been obtained for the energy difference between the two states of D0 at the inversion TS, i.e. 14 kcal mol−1 and 11 kcal mol−1 for the syn- and anti-conformers, respectively.In an attempt to find potential efficient non-radiative mechanisms for the photo-excited naphthalene dimers, we explore regions of the PES where the gap between the ground and lowest excited singlet state becomes small or where the two states become degenerate, that is S0/S1 intersections. For clarity, herein, we only discuss the results regarding the most stable conformations, that is, the syn (D0 and D1) and anti (D2) forms. Motivated by the lowering of the S0/S1 gap at the TS of the pyramidal inversion for D1, we search for energy crossings from the trigonal planar arrangement of the SO1 bridge. Indeed, we identify a molecular geometry structurally related to the TS where the ground and excited PESs intersect with non-vanishing non-adiabatic couplings at the proximity of the crossing (ESI†), i.e. a conical intersection (CI). At this intersection, labelled as sym-CI, there is a symmetric elongation of the S–C bonds between SO1 and the naphthalene units and an important increase in the bridge C–S–C angle (Table 4). More importantly, the sym-CI point lies ∼0.66 eV below the S1 state at the FC region, and thus it is energetically accessible upon photo-excitation, providing a clear molecular mechanism to relax back to the ground state without photoemission. Similarly, we obtain a symmetric state crossing for the D0 dimer, which exhibits a similar geometrical pattern (long C–S bonds and linear C–S–C angle). However, in this case, the sym-CI is obtained energetically above the FC S1 energy. For both dimers, D0 and D1, at the sym-CI, the ground state crosses with the n(SOn) → σ* state, which is stabilized by the elongation of the two S–C bonds (Fig. 5). Moreover, in D1, the planarization of the sulfoxide group destabilizes the n(SO) due to π anti-bonding interactions with the pz orbital of the oxygen atom. In the D0 dimer, the n(S) destabilization comes from the interaction with the π-orbitals of the coplanar naphthalene fragments. On the other hand, the lack of electron lone-pairs in the sulfone bridge inhibits the presence of a low energy sym-CI in the D2 dimer.
| Dimer | State | r(C–S) | α(C–S–C) | ΔE(rel) | |
|---|---|---|---|---|---|
| a The geometries for the sym-CI and asym-CI can be found in Fig. S7. | |||||
| D0 | inv-TS | 1.80/1.80 | 178 | +0.60 | |
| sym-CI | 2.17/2.12 | 178 | +0.68 | ||
| asym-CI | 2.26/1.77 | 176 | −0.23 | ||
| D1 | inv-TS | 1.76/1.76 | 115 | +0.15 | |
| sym-CI | 1.96/1.88 | 155 | −0.66 | ||
| asym-CI | 2.32/1.78 | 107 | −1.03 | ||
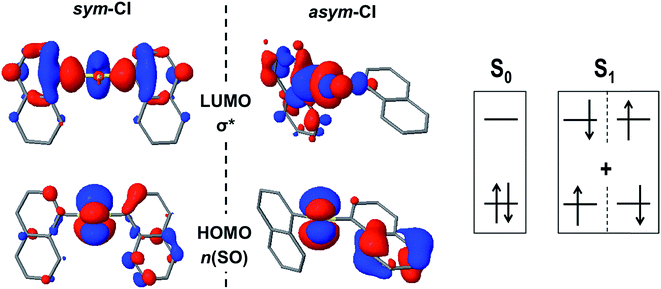 | ||
| Fig. 5 The frontier molecular orbitals n(SO) and σ* at the S0/S1sym-CI and asym-CI points for the sulfoxide naphthalene dimer (D1). | ||
Excited state optimization within the CI subspace, i.e. minimal energy CI (MECI), of the sulfide and sulfoxide dimers results in non-symmetric molecular geometries (asym-CI) with one rather long S⋯C distance and a short S–C bond. As a result, σ* localizes on one side of the dimer (at the long S⋯C separation). This structural arrangement suggests a path towards molecular fragmentation. Furthermore, the computed asym-CI energies lie below the S1 energy in the FC region (and below the sym-CI point) and are therefore energetically available for both dimers. Hence, we identify the non-adiabatic relaxation of D0 and D1 dimers through asym-CI as the mechanism describing reversible molecular fragmentation (although the molecule has not been effectively fragmented in asym-CI), where there is an elongation and shrinking of a S–C bond resulting in a fast decay to the electronic ground state. The reverse fragmentation mechanism has been proposed as the main inversion of aryl sulfoxides bearing a primary alkyl group.29,31,32,34 Moreover, we find that such a mechanism can be potentially photoinduced in the sulfide and sulfoxide aromatic dimers and proceeds through a MECI.
By gathering the results discussed above regarding the photoexcitation and different deactivation paths of the naphthalene dimers studied, it is possible to draw a general picture for the photophysical properties of Dn. The main photophysical mechanisms explored are represented in the Jablonski diagram in Fig. 6. Relaxation of the photoexcited sulfur-bridged naphthalene dimers allows the formation of strongly emissive localized excitations and weakly emitting excimers. Moreover, sulfide and sulfoxide dimers exhibit non-radiative decay back to the ground state, which actually dominate their excited state dynamics in solution. On the other hand, the lack of electron lone-pairs in D2 blocks the presence of low-lying ground-excited state crossings, resulting in much larger PL yields with respect to D0 and D1.
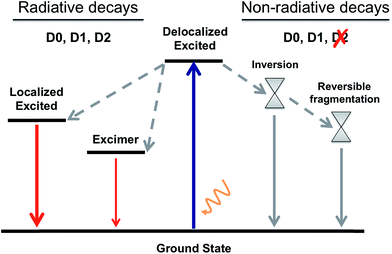 | ||
| Fig. 6 A general Jablonski diagram for the deactivation mechanisms after photoexcitation of the D0, D1 and D2 naphthalene dimers. | ||
4. Conclusions
In this study we have identified and characterized both the radiative and non-radiative deactivation mechanisms occurring in sulfur-bridged naphthalene dimers. The different PL efficiencies upon oxidation of the sulfur-bridge have been rationalized by the existence of energetically available non-radiative decays for the sulfide and sulfoxide bridges. The lack of electron lone-pairs in the sulfone linker is the origin of the much stronger PL with respect to the S and SO1 cases.Although the computed vertical transition energies and their intensities in the FC structures are very similar for the three naphthalene dimers, there are significant differences in the character of the transition to the lowest excited singlet between D0, D1 and D2, which is the larger involvement of the n(SOn) orbitals in terms of bridge → naphthalene CT with lower oxidation state of the sulfur atom. Geometrical relaxation to the local minima of the excited state PESs cannot account for the very weak PL of D0 and D1, pointing towards the existence of efficient non-radiative decays, not present from the excited D2. We identify energy crossing regions in D0 and D1 dimers that allow the conversion of the photo-excited molecules back to the ground state with no fluorescence emission. Our calculations indicate that while two types of S0/S1 state crossings, i.e. symmetric and asymmetric, may be reached along the excited state decay of D1, only the asymmetric intersection is energetically available for the D0 dimer. The identification of energetically available asymmetric CI pointing towards the reversible molecular fragmentation suggests a photoinduced roaming mechanism35 as a potential non-radiative deactivation path of D0 and D1. Finally, it is important to notice that in our calculations, due to the nature of the studied chromophores, we have not considered the role of ISC as one of the main deactivation channels.
The present results suggest that, differently to the terthiophene dimers, the SOn bridged naphthalene bichromophores do not require efficient ISC to limit the fluorescence emission. On the other hand, our results reinforce the generality of the electron lone-pair screening concept for sulfur-bridged chromophore dimers. The obtained results and conclusions are general enough to be extrapolated to other sulfur-bridged conjugated dimers, therefore proportionating novel strategies for the design of strongly luminescent organic molecules with controlled charge transfer. Investigations in this direction are currently underway in our laboratories.
Acknowledgements
We are grateful to Professors Pere Alemany and Josep Maria Bofill and Dr Miquel Huix-Rotllant for valuable insights and fruitful discussions. This study has been supported by the Basque Government (project IT588-13) and the Spanish Government MINECO/FEDER (projects CTQ2016-80955 and CTQ2015-64579-C3-3-P). D. C. thanks IKERBASQUE, Basque Foundation for Science for financial support. C. C. is indebted to the Spanish “Ministerio de Economía y Competitividad” for a pre-doctoral FPI grant and an EEBB mobility grant for a research stay in Marseille. M. B. thanks the support of the A*MIDEX grant (No. ANR-11-IDEX-0001-02) and of the project Equip@Meso (ANR-10-EQPX-29-01), both funded by the French Government “Investissements d'Avenir” program. C. J. B. acknowledges support from the National Science Foundation, grant DMR1508099. M. O. W. thanks the Natural Sciences and Engineering Research Council of Canada and the Peter Wall Foundation for funding.References
- Heliatek, http://www.heliatek.com.
- NREL Best Research-Cell Efficiencies, http://www.nrel.gov/ncpv/images/efficiency_chart.jpg.
- W. Rettig, Charge Separation in Excited States of Decoupled Systems—TICT Compounds and Implications Regarding the Development of New Laser Dyes and the Primary Process of Vision and Photosynthesis, Angew. Chem., Int. Ed., 1986, 25, 971 CrossRef.
- Z. R. Grabowski, K. Rotkiewicz and W. Rettig, Structural Changes Accompanying Intramolecular Electron Transfer:
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Focus on Twisted Intramolecular Charge-Transfer States and Structures, Chem. Rev., 2003, 103, 3899 CrossRef PubMed.
Focus on Twisted Intramolecular Charge-Transfer States and Structures, Chem. Rev., 2003, 103, 3899 CrossRef PubMed. - A. N. Bartynski, M. Gruber, S. Das, S. Rangan, S. Mollinger, C. Trinh, S. E. Bradforth, K. Vandewal, A. Salleo, R. A. Bartynski, W. Bruetting and M. E. Thompson, Symmetry-Breaking Charge Transfer in a Zinc Chlorodipyrrin Acceptor for High Open Circuit Voltage Organic Photovoltaics, J. Am. Chem. Soc., 2015, 137, 5397 CrossRef CAS PubMed.
- D. Fyfe, LED Technology: Organic displays come of age, Nat. Photonics, 2009, 3, 453 CrossRef CAS.
- C. Santato, F. Cicoira and R. Martel, Organic photonics: Spotlight on organic transistors, Nat. Photonics, 2011, 5, 392 CrossRef CAS.
- A. P. Kulkarni, C. J. Tonzola, A. Babel and S. A. Jenekhe, Electron Transport Materials for Organic Light-Emitting Diodes, Chem. Mater., 2004, 16, 4556 CrossRef CAS.
- P. R. Christensen, J. K. Nagle, A. Bhatti and M. O. Wolf, Enhanced Photoluminescence of Sulfur-Bridged Organic Chromophores, J. Am. Chem. Soc., 2013, 135, 8109 CrossRef CAS PubMed.
- C. D. Cruz, P. R. Christensen, E. L. Chronister, D. Casanova, M. O. Wolf and C. J. Bardeen, Sulfur-Bridged Terthiophene Dimers: How Sulfur Oxidation State Controls Interchromophore Electronic Coupling, J. Am. Chem. Soc., 2015, 137, 12552 CrossRef CAS PubMed.
- R. S. Becker, J. Seixas de Melo, A. L. Maçanita and F. Elisei, Comprehensive Evaluation of the Absorption, Photophysical, Energy Transfer, Structural, and Theoretical Properties of α-Oligothiophenes with One to Seven Rings, J. Phys. Chem., 1996, 100, 18683 CrossRef CAS.
- R. Rossi, M. Ciofalo, A. Carpita and G. Ponterini, Singlet—triplet intersystem crossing in 2,2′:5′,2′′-terthiophene and some of its derivatives, J. Photochem. Photobiol., A, 1993, 70, 59 CrossRef CAS.
- R. G. Parr, in Horizons of Quantum Chemistry: Proceedings of the Third International Congress of Quantum Chemistry Held at Kyoto, Japan, October 29-November 3, 1979, ed. K. Fukui and B. Pullman, Springer Netherlands, Dordrecht, 1980, p. 5 Search PubMed.
- T. Ziegler, Approximate density functional theory as a practical tool in molecular energetics and dynamics, Chem. Rev., 1991, 91, 651 CrossRef CAS.
- M. E. Casida, Time-Dependent Density-Functional Response Theory for Molecules, World Scientific, Singapure, 1995, vol. 1 Search PubMed.
- E. Runge and E. K. U. Gross, Density-Functional Theory for Time-Dependent Systems, Phys. Rev. Lett., 1984, 52, 997 CrossRef CAS.
- J.-D. Chai and M. Head-Gordon, Long-range corrected hybrid density functionals with damped atom-atom dispersion corrections, Phys. Chem. Chem. Phys., 2008, 10, 6615 RSC.
- P. C. Hariharan and J. A. Pople, The influence of polarization functions on molecular orbital hydrogenation energies, Theor. Chim. Acta, 1973, 28, 213 CrossRef CAS.
- M. M. Franci, W. J. Pietro and W. J. Hehre, Self-consistent molecular orbital methods. XXIII. A polarization-type basis set for second-row elements, J. Chem. Phys., 1982, 77, 3654 CrossRef.
- T. Clark, J. Chandrasekhar, G. W. Spitznagel and P. V. R. Schleyer, Efficient diffuse function-augmented basis sets for anion calculations III. The 3-21+G basis set for first-row elements, Li–F, J. Comput. Chem., 1983, 4, 294 CrossRef CAS.
- D. M. Chipman, Reaction field treatment of charge penetration, J. Chem. Phys., 2000, 112, 5558 CrossRef CAS.
- E. Cancès and B. Mennucci, Comment on “Reaction field treatment of charge penetration”, J. Chem. Phys., 2000, 112, 5558 ( J. Chem. Phys. , 2001 , 114 , 4744 ) CrossRef.
- J. E. Subotnik, R. J. Cave, R. P. Steele and N. Shenvi, The initial and final states of electron and energy transfer processes: diabatization as motivated by system-solvent interactions, J. Chem. Phys., 2009, 130, 234102 CrossRef PubMed.
- Y. Shao, M. Head-Gordon and A. I. Krylov, The spin–flip approach within time-dependent density functional theory: theory and applications to diradicals, J. Chem. Phys., 2003, 118, 4807 CrossRef CAS.
- A. D. Becke, Density-functional exchange-energy approximation with correct asymptotic behavior, Phys. Rev. A, 1988, 38, 3098 CrossRef CAS.
- C. Lee, W. Yang and R. G. Parr, Development of the Colle–Salvetti correlation-energy formula into a functional of the electron density, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785 CrossRef CAS.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr, J. E. Peralta, F. Ogliaro, M. J. Bearpark, J. Heyd, E. N. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. P. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, N. J. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, Ö. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian, Inc., Wallingford, CT, USA, 2009.
- Y. Shao, Z. Gan, E. Epifanovsky, A. T. B. Gilbert, M. Wormit, J. Kussmann, A. W. Lange, A. Behn, J. Deng, X. Feng, D. Ghosh, M. Goldey, P. R. Horn, L. D. Jacobson, I. Kaliman, R. Z. Khaliullin, T. Kuś, A. Landau, J. Liu, E. I. Proynov, Y. M. Rhee, R. M. Richard, M. A. Rohrdanz, R. P. Steele, E. J. Sundstrom, H. L. Woodcock, P. M. Zimmerman, D. Zuev, B. Albrecht, E. Alguire, B. Austin, G. J. O. Beran, Y. A. Bernard, E. Berquist, K. Brandhorst, K. B. Bravaya, S. T. Brown, D. Casanova, C.-M. Chang, Y. Chen, S. H. Chien, K. D. Closser, D. L. Crittenden, M. Diedenhofen, R. A. DiStasio, H. Do, A. D. Dutoi, R. G. Edgar, S. Fatehi, L. Fusti-Molnar, A. Ghysels, A. Golubeva-Zadorozhnaya, J. Gomes, M. W. D. Hanson-Heine, P. H. P. Harbach, A. W. Hauser, E. G. Hohenstein, Z. C. Holden, T.-C. Jagau, H. Ji, B. Kaduk, K. Khistyaev, J. Kim, J. Kim, R. A. King, P. Klunzinger, D. Kosenkov, T. Kowalczyk, C. M. Krauter, K. U. Lao, A. D. Laurent, K. V. Lawler, S. V. Levchenko, C. Y. Lin, F. Liu, E. Livshits, R. C. Lochan, A. Luenser, P. Manohar, S. F. Manzer, S.-P. Mao, N. Mardirossian, A. V. Marenich, S. A. Maurer, N. J. Mayhall, E. Neuscamman, C. M. Oana, R. Olivares-Amaya, D. P. O'Neill, J. A. Parkhill, T. M. Perrine, R. Peverati, A. Prociuk, D. R. Rehn, E. Rosta, N. J. Russ, S. M. Sharada, S. Sharma, D. W. Small and A. Sodt, Advances in molecular quantum chemistry contained in the Q-Chem 4 program package, Mol. Phys., 2015, 113, 184 CrossRef CAS.
- J. W. Cubbage and W. S. Jenks, Computational Studies of the Ground and Excited State Potentials of DMSO and H2SO:
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Relevance to Photostereomutation, J. Phys. Chem. A, 2001, 105, 10588 CrossRef CAS.
Relevance to Photostereomutation, J. Phys. Chem. A, 2001, 105, 10588 CrossRef CAS. - R. S. Kathayat, L. Yang, T. Sattasathuchana, L. Zoppi, K. K. Baldridge, A. Linden and N. S. Finney, On the Origins of Nonradiative Excited State Relaxation in Aryl Sulfoxides Relevant to Fluorescent Chemosensing, J. Am. Chem. Soc., 2016, 138, 15889 CrossRef CAS PubMed.
- K. Mislow, M. Axelrod, D. R. Rayner, H. Gotthardt, L. M. Coyne and G. S. Hammond, Light-Induced Pyramidal Inversion of Sulfoxides1, J. Am. Chem. Soc., 1965, 87, 4958 CrossRef CAS.
- Y. Guo and W. S. Jenks, Photolysis of Alkyl Aryl Sulfoxides:
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) α-Cleavage, Hydrogen Abstraction, and Racemization1, J. Org. Chem., 1997, 62, 857 CrossRef CAS.
α-Cleavage, Hydrogen Abstraction, and Racemization1, J. Org. Chem., 1997, 62, 857 CrossRef CAS. - B. A. Lindquist, D. E. Woon and T. H. Dunning, Electronic Structure of H2S, SF2, and HSF and Implications for Hydrogen-Substituted Hypervalent Sulfur Fluorides, J. Phys. Chem. A, 2014, 118, 1267 CrossRef CAS PubMed.
- B. W. Vos and W. S. Jenks, Evidence for a Nonradical Pathway in the Photoracemization of Aryl Sulfoxides1, J. Am. Chem. Soc., 2002, 124, 2544 CrossRef CAS PubMed.
- A. G. Suits, Roaming Atoms and Radicals: A New Mechanism in Molecular Dissociation, Acc. Chem. Res., 2008, 41, 873 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c7sc01285c |
| This journal is © The Royal Society of Chemistry 2017 |