Beam sampling: taking samples at the micro-scale
Analytical Methods Committee, AMCTB No. 84
First published on 27th February 2018
Abstract
When using a beam to make a measurement in situ, irrespective of scale, the process implicitly includes the taking of a sample. Therefore, the uncertainty of the measurement result needs to include the uncertainty generated by the sampling process, which is usually dominated by the heterogeneity of the analyte at that scale. Reliable estimates of the uncertainty of beam measurements are essential to judge their fitness-for-purpose (FFP) and hence to enable their rigorous interpretation. This approach can be applied to a wide range of techniques for the analytical assessments of materials, from handheld portable X-ray Fluorescence (pXRF) at the millimeter scale, to Secondary Ion Mass Spectrometry (SIMS) at the micron scale.
Introduction to sampling
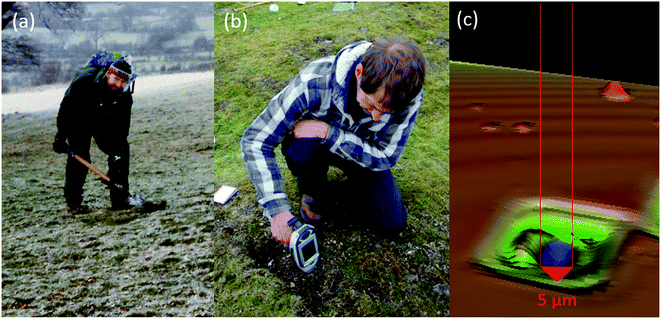
Sampling has traditionally been undertaken at the macro-scale, using tools such as a spade for soil (Fig. 1a) or a bucket for water, to try and get a mass of primary sample that is ‘sufficiently representative’ of the bulk material to be characterised (i.e., the sampling target). The mass of the primary sample is selected with the aim of being large enough to make the effect of the heterogeneity of the analyte distribution negligible within that sampling target. The subsequent physical preparation of the sample, such as grinding, mixing and splitting, can then be used to enable a small test portion (e.g., 0.1 g) to be taken for chemical analysis that is ‘sufficiently representative’ of that primary sample.What is beam sampling?
Many forms of modern analytical instrumentation designed for micro-analysis use ‘beams’ to take samples at the micro-scale. It should, however, be noted that these techniques often have their own terminology for the ‘beam’ being utilised. For example: ‘excitation volume’ is commonly used for hand-held portable X-ray Fluorescence (pXRF); ‘ion beam’ or ‘spot’ for Secondary Ion Mass Spectrometry (SIMS), ‘laser beam’ for Laser Ablation-Inductively Coupled Plasma Mass Spectrometry (LA-ICPMS) and, ‘electron micro-beam’ or ‘probe’ for Electron Probe Microanalysis (EPMA).The scale of the measurements, and the mass of the primary sample taken, varies between analytical procedures. For example, pXRF interrogates a test portion mass in the milligram range, with a ‘beam’ diameter of around 5 mm (Fig. 1b). By contrast SIMS often has a test portion in the picogram range, with a beam diameter of around 5 μm (Fig. 1c). In such situations, this test portion for a single beam sample is the same as the primary sample. When pXRF or SIMS are applied in situ, they are in effect taking a sample of the test material, as well as making a chemical analysis. For some procedures (e.g. pXRF and EPMA) the sample is ‘interrogated’ in place, non-destructively, but for others (e.g. SIMS and LA-ICPMS) the sample is removed and destroyed. In all these cases, this process can be usefully described as ‘beam sampling’.
Beam sampling usually produces much quicker results than macro-sampling, and automation is often possible, but it is susceptible to small-scale heterogeneity within the sampling target. Macro-sampling can effectively eliminate the effects of small-scale heterogeneity, given sufficient physical preparation. For example, it is usually possible to increase the mass of the primary sample, within limits of logistics and cost. In contrast, the mass interrogated by beam sampling is defined by the analytical procedure used, and is fixed within relatively small limits, as described above for pXRF and SIMS. It is therefore typical that beam measurements have more uncertainty than measurements made on bulk materials. This is because heterogeneity adds to the uncertainty associated with a measurement and arises from very small samples showing variation due to small-scale heterogeneity that is effectively averaged by macro-sampling.
Terminology of beam sampling and beam measurements
The terminology already used for sampling and measurement at the macro-scale3 can also be used to discuss beam sampling and measurement at the micro-scale. A single beam sample can be used as a primary sample, that aims to represent a larger sample target. The analytical measurement made on that primary sample quantifies the analyte concentration in that sample and thereby gives an estimate of the measurand. The measurand (the quantity intended to be measured)4 is usually taken to be the mean concentration in the target. An alternative approach is to take ‘composite measurements’ which use multiple beam samples to form a composite primary sample, that aims to reduce the uncertainty of the measurement of the mean analyte concentration within a sample target. In macro-sampling, measurements are usually made on the composite sample. However, in beam sampling, measurements are typically made on each of the increments within the composite sample, and the mean value calculated to estimate the concentration in the target. For many beam methods (e.g., SIMS), the test portion used for each measurement is the same as the single primary beam sample. However, it should be noted that for some other analytical techniques (e.g. LA-ICPMS), not all of the material that is dislodged by the beam necessarily enters the measurement system.Estimation of the uncertainty of beam measurements, including heterogeneity
The repeatability of the analytical measurement and the heterogeneity of the analyte for a particular test portion mass at a specified scale can be quantified using the ‘duplicate method’. In this method, a certain proportion of the beam measurements (e.g. 10%, n ≥ 8) is duplicated, by taking a second beam measurement a small distance from the original. This separation distance is selected to represent a fresh reinterpretation of the sampling protocol, for example after moving the beam centre to another point within the intended sampling target. The distance also reflects the effect that the heterogeneity at that scale has on the measurement uncertainty.Application of the duplicate method to beam sampling can be problematic if the beam measurement is locally destructive, which precludes placing duplicate samples within a given distance (e.g. 50 μm for SIMS). When this is the case, the duplicate sample has to be taken at the minimum distance to avoid interaction between the two measurements. Heterogeneity then has to be assumed to be negligible at that scale, although ideally that assumption should be subsequently verified.
The resultant measurements are interpreted using analysis of variance (ANOVA). Variance within the duplicate pairs gives an estimate of the analytical measurement repeatability. Variance between the pairs gives an estimate of the heterogeneity. The sum-squares of these two components gives an estimate of the measurement repeatability at the larger scale, e.g., of a whole crystal fragment if that is the sampling target. The full measurement uncertainty can then be estimated by including other factors such as bias against matched certified reference materials (CRMs), and between-lab variance.
Why do we need to know the uncertainty of beam measurements?
The value of the uncertainty can be used to judge whether the beam measurements are suitable for a particular stated objective (i.e., fit-for-purpose, FFP),3 discussed below. If the uncertainty is too large to enable this objective to be achieved, then it can be reduced. For bulk sampling, as already noted, this can be achieved by substantially increasing the mass of each primary sample, but this is not feasible for most beam sampling procedures. However, the overall sample mass can be effectively increased by taking composite measurements, where ‘n’ replicated beam measurements are located across the sampling target. The mean value can then be calculated, and the measurement uncertainty expressed as the standard error of the mean value (SEM). The high level of replication often required is feasible for some beam procedures (e.g. 100-fold for SIMS), but is somewhat less practical for others (e.g. pXRF). The uncertainty of the resultant composite measurements can be predicted by use of the equation SEM = s/√n, where s is the standard deviation. For example, a ten-fold composite measurement would be predicted to reduce the uncertainty on the mean value by a factor of 3.2 (i.e., √10). The validity of this prediction depends on the nature of the heterogeneity, and is best estimated empirically, also using the duplicate method.The concept of beam sampling can therefore be applied to a wide variety of different analytical procedures and objectives. One possible objective is to estimate the overall mean composition of a sampling target (e.g. a specified volume of a crystal fragment), in which case composite beam measurements can be applied. A particular example of this is the characterisation of a candidate reference material (RM) for beam analysis. One objective in this situation is to certify the mean concentration and its uncertainty for the required analytes in the material. This uncertainty on the mean has to include the contribution from the analyte heterogeneity at the relevant spatial scale. There are two published examples of quantifying heterogeneity for this purpose: one using SIMS on quartz fragments,2 and the other using pXRF on RM powders,5 both employing the ‘duplicate method’.
Alternatively, a second possible objective is to spatially resolve the variation of the analyte concentration across the crystal fragment, when an estimate of the uncertainty of the beam measurement at each location is needed. For example, if the aim is to establish whether the analyte concentration at the core of the crystal is different to that at its rim, then that difference must be shown to be significantly greater than can be explained by the measurement uncertainty. This situation is equivalent to the aim of traditional geochemical mapping at any scale (e.g. usually 10 m to 1000 km). For this purpose, an estimate of the measurement uncertainty is required, and reliable geochemical mapping requires that the measurement uncertainty should account for less than 20% of the total variance.3 This is one approach to judging the fitness of measurements (beam or bulk) for this particular purpose. A more general approach to judging FFP at the macro-scale balances the overall measurement uncertainty against the costs that might result from decisions based upon measurements with uncertainty that is too high, or too low.3 This particular method also differentiates the relative contributions made by sampling and analysis to the total uncertainty, thus enabling the most cost-effective approach to reducing the overall uncertainty. At the micro-scale, this approach appears to be equally applicable. Reducing the uncertainty from sampling at the macro-scale would normally be achieved by increasing either the mass of samples, or the number of increments in a composite sample. This could be similarly applied to the use of composite measurements at the micro-scale to reduce the effects of small-scale heterogeneity.
Conclusion
Beam sampling is already being used as part of many micro-analytical techniques, such as in situ pXRF, SIMS, LA-ICPMS, EPMA. However, it is often unrecognised as a type of sampling. Recognition of beam sampling as part of the micro-analytical measurement process requires the uncertainty from sampling to be included in the estimate of measurement uncertainty. This uncertainty primarily arises from heterogeneity of the analyte in the test material, at the particular scale of the measurements produced by the instrument. If this more rigorous estimate of measurement uncertainty is used, it then becomes possible to judge whether a beam measurement value is fit for its intended purpose, even if its uncertainty is higher than is usual for bulk analysis. With an increasing use of in situ measurement devices, reliable methods of estimating the FFP of beam procedures can enable the application of faster and potentially less expensive measurements in many sectors of society.References and further reading
1 A. Argyraki, M. H. Ramsey and P. J. Potts, Evaluation of portable XRF for in-situ measurements of lead on contaminated land, Analyst, 1997, 122, 743–749, DOI: 10.1039/A700746I2 M. H. Ramsey and M. Wiedenbeck, Quantifying isotopic heterogeneity of candidate reference materials at the picogram sampling scale, Geostand. Geoanal. Res., 2018, 42(1), 5–24, DOI: 10.1111/ggr.12198.
3 Measurement uncertainty arising from sampling: a guide to methods and approaches, ed. M. H. Ramsey and S. L. R. Ellison, Eurachem, 2007, https://eurachem.org/images/stories/Guides/pdf/UfS_2007.pdf.
4 Joint Committee for Guides in Metrology, International Vocabulary of Metrology – Basic and General Concepts and Associated Terms, VIM, 3rd edn, 2008, p. 136.
5 P. D. Rostron and M. H. Ramsey, Quantifying heterogeneity of small test portion masses of geological reference materials by PXRF: implications for uncertainty of reference values, Geostand. Geoanal. Res., 2017, 41(3), 459–473, DOI: 10.1111/ggr.12162.
This Technical Brief was prepared by the Subcommittee for Sampling Uncertainty and Sampling Quality (Chair Prof M. H. Ramsey) and approved by the Analytical Methods Committee on 02/02/2018.
| This journal is © The Royal Society of Chemistry 2018 |