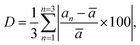Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Electrical conductivity and dielectric behaviour of nanocrystalline La0.6Gd0.1Sr0.3Mn0.75Si0.25O3
Ah Dhahri *ab,
E. Dhahri
*ab,
E. Dhahri b and
E. K. Hlilc
b and
E. K. Hlilc
aFaculté des Sciences, Monastir, Université de Monastir, Avenue de l'environnement 5019, Monastir, Tunisia
bLaboratoire de Physique Appliquée, Faculté des Sciences de Sfax, Université de Sfax, 3000, BP 1171, Tunisia. E-mail: Dhahri.ahmad@gmail.com; Tel: +216 20 20 45 55
cInstitut Néel, CNRS et Université Joseph Fourier, B.P. 166, 38042 Grenoble, France
First published on 1st March 2018
Abstract
An La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 ceramic was prepared via a solution-based chemical technique. X-ray diffraction study confirms the formation of the compound in the orthorhombic structure with the Pnma group space. Dielectric properties have been investigated in the temperature range of 85–290 K with the frequency range 40 Hz to 2 MHz. The conductivity spectra have been investigated by the Jonscher universal power law: σ(ω)![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) =
= ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) σdc
σdc![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) +
+ ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Aωn, where ω is the frequency of the ac field, and n is the exponent. The deduced exponent ‘n’ values prove that a hopping model is the dominating mechanism in the material. Based on dc-electrical resistivity study, the conduction process is found to be dominated by a thermally activated small polaron hopping (SPH) mechanism. Complex impedance analysis (CIA) indicates the presence of a relaxation phenomenon and allows us to modelize the sample in terms of an electrical equivalent circuit. Moreover, the impedance study confirms the contribution of grain boundaries to the electrical properties.
Aωn, where ω is the frequency of the ac field, and n is the exponent. The deduced exponent ‘n’ values prove that a hopping model is the dominating mechanism in the material. Based on dc-electrical resistivity study, the conduction process is found to be dominated by a thermally activated small polaron hopping (SPH) mechanism. Complex impedance analysis (CIA) indicates the presence of a relaxation phenomenon and allows us to modelize the sample in terms of an electrical equivalent circuit. Moreover, the impedance study confirms the contribution of grain boundaries to the electrical properties.
1. Introduction
The exploration of properties of inorganic materials has been a long-standing goal in the development of functional materials. There exists a close relationship among structure, morphology and physical properties. Extensive studies have been devoted to understanding the formation mechanisms from both theoretical and experimental point of views to synthesize better functional materials. Perovskite manganites have been the subject of intense research efforts; they exhibit a variety of electronic transport, magnetic and magnetocaloric properties. These properties open up a large field of applications1,2. In recent years, several studies have been reported for the dielectric properties of these materials such as of Li3(Mg0.95A0.05)2NbO6,3 CaCu3Ti4O12,4 Li2Mg4TiO7 (ref. 5) and Sr0.5Ca0.5TiO3:xPr3+ (ref. 6) ceramics. Perovskite manganite materials are present in several technological applications. In the last few years, various studies7,8 have suggested that doping at the Mn site influences the polaronic transport. The effect of substituting Mn by Ga, Ru, Fe, Co, Cr and Ti is well studied.9–14 The physical properties are successfully explained by a double-exchange (DE) mechanism based on a strong exchange interaction between Mn4+ and Mn3+ ions through intervening filled oxygen 2p states. It is believed that the interaction between the pairs of Mn4+ and Mn3+ ions is responsible for the electrical properties in these manganese oxides.15Recently, direct current (dc) and alternating current (ac) transport processes have been investigated in low-hole-doped Ln-based manganite-type perovskites such as La1−xCaxMnO3,16 La1−xSrxMnO3,17 La1−xAxMn1−yFeyO3,18 La0.7Sr0.25Na0.05Mn0.9Ti0.1O3,19 La0.5Ca0.5−xAgxMnO3,20 Pr0.67A0.33MnO3 (ref. 21) and RMnO3 (R = Eu; Gd; Tb; and Dy),22 where manganese is in a mixed valence state (Mn3+–Mn4+). Pairs of Mn4+ and Mn3+ can be controlled by changing the doping level or oxygen stoichiometry. Therefore, it seems plausible that the doping element and its content will be important for the electrical properties in these materials. To optimize the properties of this material, a systematic study of the electrical properties should be conducted over a wide temperature range and with different doping levels. Therefore, several investigations have been carried out to understand the correlation among the structure, magnetic and electrical properties, and the magnetoresistance of Re1−xMxMnO3 by doping with elements such as Cr, Ni, V, Ga, Co, Mg and Al at the Mn site.23–25 However, doping of silicon at the Mn site in Re1−xMxMnO3 (where Re = La, Nd, Pr, Sm, Y, … and M = Pb, Ba, Sr, Ca, …) has not been investigated so far. Therefore, to better understand the role of Mn and its local environment in La0.6Gd0.1Sr0.3MnO3, we have studied the effects of replacing some of Mn with Si. To decrease the critical temperature Tc (Tc = 378 K)26 of the parent compound La0.7Sr0.3MnO3, we have substituted lanthanum (La) with 10% gadolinium (Gd). The choice of the ion Si4+ is based on the fact that the ionic radius (0.4 Å) is smaller than that of Mn4+ (0.53 Å). In view of this information, the authors have taken up the present research with an objective to study the effect of silicate at the Mn site on the transport and dielectric properties of La0.6Gd0.1Sr0.3Mn0.75Si0.25O3. In the present study, we investigate the dielectric and electrical properties of La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 at different temperatures (77 K to 320 K) and frequencies (40 Hz to 10 MHz). Impedance spectroscopy is successfully employed to obtain clear information about the transport and dielectric properties of this material. Such technique can resolve the conduction components from polycrystalline electrical ceramics, particularly in differentiating the transport characteristics in grains and grain boundaries.
2. Experimental
A solution based sol–gel method was used to prepare the La0.63+Gd0.13+Sr0.32+(Mn0.73+![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Mn0.054+)Si0.254+O32− fine powder. As starting materials, stoichiometric amounts of La(NO3)3·6H2O (2.5981 g, 6 mmol); Sr(NO3)2·6H2O (0.9592 g, 3 mmol); Mn(NO3)2·4H2O (1.8826 g, 7.5 mmol); Gd(OOCCH3)3·xH2O (0.3344 g, 1 mmol); and SiCl4 (0.4247 g, 2.5 mmol) were used owing to their high solubility in water. These precursors were weighed in the desired proportions and dissolved with small amounts of deionized water. Citric acid was used as a polymerization/complexation agent, forming a stable solution. Metallic salt solution (100 mL) was added to 300 mL of a solution containing a mixture of citric acid (60 g) and ethylene glycol (13 mL), which dispersed the cations homogeneously forming a cation–polymer network. This stable solution was then heated on a thermal plate under constant stirring where polymerization occurred in the liquid solution and led to a homogeneous sol. This solution was then heated at a temperature of ∼100–140 °C till a dry thick brown-coloured sol was formed. The resin was further decomposed in an oven at a temperature of ∼300 °C in air for 3 h to get a fairly porous polymeric precursor in the form of a black resin-like material. After milling in an agate mortar, the precursor was calcined at 600 °C for 7 h to give a fine powder. Then, the obtained powder was pressed into circular pellets under 4 t cm−2 (to about 2 mm thickness) and finally sintered in air at 800 °C for 10 h. The sample was proved to be single phase by powder X-ray diffraction experiments performed at room temperature using a Siemens D-5000 with a graphite monochromatized CuKα radiation (λ = 1.54056 Å). The data collection was performed by step-scan modes in a 2θ range between 20° and 90° with a step-size of 0.0167° and a count time of 18 s per step. This system was able to detect up to a minimum of 3% of impurities according to our measurements. The structural parameters were obtained by fitting the experimental data from XRD using the Rietveld structural refinement program FULLPROF soft-ware (Version 1.9c-May 2001-LLB-JRC).27 The compound was pressed into polished pellets with a diameter of 10 mm and a thickness of about 1 mm and then sintered. For electrical measurements, two indium plots separated by a distance of 5 mm were deposited on the pellets to ensure ohmic contact. The other side was bound to the cold finger of liquid nitrogen cooled cryostat to vary the sample temperature between 77 and 320 K. The two indium pads were connected to the electrodes of an Agilent 4294A impedance analyzer to measure the sample conductance. The samples were modeled with a parallel circuit and excited by an alternating signal with an amplitude of 50 mV.
Mn0.054+)Si0.254+O32− fine powder. As starting materials, stoichiometric amounts of La(NO3)3·6H2O (2.5981 g, 6 mmol); Sr(NO3)2·6H2O (0.9592 g, 3 mmol); Mn(NO3)2·4H2O (1.8826 g, 7.5 mmol); Gd(OOCCH3)3·xH2O (0.3344 g, 1 mmol); and SiCl4 (0.4247 g, 2.5 mmol) were used owing to their high solubility in water. These precursors were weighed in the desired proportions and dissolved with small amounts of deionized water. Citric acid was used as a polymerization/complexation agent, forming a stable solution. Metallic salt solution (100 mL) was added to 300 mL of a solution containing a mixture of citric acid (60 g) and ethylene glycol (13 mL), which dispersed the cations homogeneously forming a cation–polymer network. This stable solution was then heated on a thermal plate under constant stirring where polymerization occurred in the liquid solution and led to a homogeneous sol. This solution was then heated at a temperature of ∼100–140 °C till a dry thick brown-coloured sol was formed. The resin was further decomposed in an oven at a temperature of ∼300 °C in air for 3 h to get a fairly porous polymeric precursor in the form of a black resin-like material. After milling in an agate mortar, the precursor was calcined at 600 °C for 7 h to give a fine powder. Then, the obtained powder was pressed into circular pellets under 4 t cm−2 (to about 2 mm thickness) and finally sintered in air at 800 °C for 10 h. The sample was proved to be single phase by powder X-ray diffraction experiments performed at room temperature using a Siemens D-5000 with a graphite monochromatized CuKα radiation (λ = 1.54056 Å). The data collection was performed by step-scan modes in a 2θ range between 20° and 90° with a step-size of 0.0167° and a count time of 18 s per step. This system was able to detect up to a minimum of 3% of impurities according to our measurements. The structural parameters were obtained by fitting the experimental data from XRD using the Rietveld structural refinement program FULLPROF soft-ware (Version 1.9c-May 2001-LLB-JRC).27 The compound was pressed into polished pellets with a diameter of 10 mm and a thickness of about 1 mm and then sintered. For electrical measurements, two indium plots separated by a distance of 5 mm were deposited on the pellets to ensure ohmic contact. The other side was bound to the cold finger of liquid nitrogen cooled cryostat to vary the sample temperature between 77 and 320 K. The two indium pads were connected to the electrodes of an Agilent 4294A impedance analyzer to measure the sample conductance. The samples were modeled with a parallel circuit and excited by an alternating signal with an amplitude of 50 mV.
3. Results and discussion
3.1 Microstructure analysis
To check the existence of all the elements in the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 (LGSMSiO) compound, energy dispersive X-ray analysis was performed. The EDX spectra shown in Fig. 1 reveals the presence of the elements La, Sr, Gd, Si, and Mn, which confirmed that there was no loss of any integrated elements during the sintering. The EDX analysis showed that the chemical composition of the compound was close to that of the nominal one (La![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Gd
Gd![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Sr
Sr![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Mn
Mn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Si = 0.6
Si = 0.6![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0.1
0.1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0.30
0.30![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0.75
0.75![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 0.25). The typical cationic composition for the sample is represented in Table 1. The result was very close to that of the nominal one within experimental uncertainties, and since no secondary phases were seen in the XRD patterns, it was reasonable to assume that silicon (Si) had been substituted for Mn in this sample.
0.25). The typical cationic composition for the sample is represented in Table 1. The result was very close to that of the nominal one within experimental uncertainties, and since no secondary phases were seen in the XRD patterns, it was reasonable to assume that silicon (Si) had been substituted for Mn in this sample.
 | ||
| Fig. 1 EDX spectra of the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 compound. The inset shows a typical scanning electron micrography (SEM) image. | ||
| Typical La | Cationic Gd | Composition Sr | From Mn | EDX Si | Nominal composition |
|---|---|---|---|---|---|
| 0.61 | 0.11 | 0.31 | 0.74 | 0.26 | La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 |
The SEM image of the fractured surface of the Si-doped sample (inset of Fig. 1) reveals the presence of a large distribution of grains that connect with each other. The average grain size in the sample is estimated to be about ∼120–126 nm. The presence of the microstructural characteristics can be related to the matter transport mechanism between the grains during the sintering process.
The average crystallite size values have been estimated from the full width at half maxima of the X-ray diffraction peaks. The effects of synthesis and instrumental and processing conditions have been taken into consideration while making the calculation of crystallite sizes. The broadening of the Bragg reflections due to micro strains is considered to have angular dependence and is given by: βstrain = 4ε![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) tan
tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ, where βstrain is the peak shift due to the strain,
θ, where βstrain is the peak shift due to the strain, 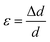 is a coefficient related to the strain and θ is the Bragg angle. The micro strains include the effects of structural defects such as dislocations, stacking faults, twin boundaries, and inter growths. The dependence of the size effect is given by the Scherer formula:
is a coefficient related to the strain and θ is the Bragg angle. The micro strains include the effects of structural defects such as dislocations, stacking faults, twin boundaries, and inter growths. The dependence of the size effect is given by the Scherer formula: 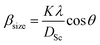 , where K is the grain shape factor (for a spherical grain K = 0.89), λ is the wavelength of the X-rays used (λ = 1.5406 Å), DSc is the crystal thickness and θmax is the corresponding incident angle. The value of (DSc) is 30 nm. In the present investigation, only the prominent peaks have been considered. The instrument broadening effect has been eliminated by subtracting the full width at half maxima (β0) values from the β size at respective Bragg peaks of a standard Si sample. Finally, the complete expression for the full width at half maximum (FWHM) of the X-ray diffraction peaks is given by:
, where K is the grain shape factor (for a spherical grain K = 0.89), λ is the wavelength of the X-rays used (λ = 1.5406 Å), DSc is the crystal thickness and θmax is the corresponding incident angle. The value of (DSc) is 30 nm. In the present investigation, only the prominent peaks have been considered. The instrument broadening effect has been eliminated by subtracting the full width at half maxima (β0) values from the β size at respective Bragg peaks of a standard Si sample. Finally, the complete expression for the full width at half maximum (FWHM) of the X-ray diffraction peaks is given by: 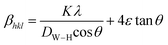 . A plot between βhkl
. A plot between βhkl![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos
cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ and sin
θ and sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ gives a straight line, and from the value of the intercept on the y-axis, the average crystal size is calculated and is found to be 45 nm. The crystallite size, calculated in the present system using the Williamson–Hall technique, is larger as compared to the crystallite size from the Debye–Scherer method (DSc) because the broadening effect due to the strain (ε = 0.14%) is completely excluded in the Debye–Scherer technique. Obviously, the particle sizes observed by SEM are several times larger than those calculated by XRD, which indicates that each particle observed by SEM consists of several crystallized grains.28 Fig. 2 shows the X-ray diffraction patterns of LGSMSiO at room temperature. The sample is single phase without detectable secondary phases within the sensitivity limits of the experiment (a few percent). The structure refinement is performed in the orthorhombic setting of the Pnma (Z = 4) space group (no. 62) (inset of Fig. 2) in which the (La/Gd/Sr) atoms are at 4c (x, 0.25, z) position, (Mn/Si) atoms are at 4b (0.5,0,0) position, O(1) is at 4c (x,0.25, z) position and O(2) is at 8d (x, y, z) position. Table 2 summarizes the relevant structural parameters obtained by the Rietveld analysis of the powder XRD pattern. This table also reports the residuals for the weighted pattern Rwp, the pattern Rp, the structure factor RF and the goodness of fit χ2. The tolerance factor, which is the geometric measure of size mismatch of the perovskite:
θ gives a straight line, and from the value of the intercept on the y-axis, the average crystal size is calculated and is found to be 45 nm. The crystallite size, calculated in the present system using the Williamson–Hall technique, is larger as compared to the crystallite size from the Debye–Scherer method (DSc) because the broadening effect due to the strain (ε = 0.14%) is completely excluded in the Debye–Scherer technique. Obviously, the particle sizes observed by SEM are several times larger than those calculated by XRD, which indicates that each particle observed by SEM consists of several crystallized grains.28 Fig. 2 shows the X-ray diffraction patterns of LGSMSiO at room temperature. The sample is single phase without detectable secondary phases within the sensitivity limits of the experiment (a few percent). The structure refinement is performed in the orthorhombic setting of the Pnma (Z = 4) space group (no. 62) (inset of Fig. 2) in which the (La/Gd/Sr) atoms are at 4c (x, 0.25, z) position, (Mn/Si) atoms are at 4b (0.5,0,0) position, O(1) is at 4c (x,0.25, z) position and O(2) is at 8d (x, y, z) position. Table 2 summarizes the relevant structural parameters obtained by the Rietveld analysis of the powder XRD pattern. This table also reports the residuals for the weighted pattern Rwp, the pattern Rp, the structure factor RF and the goodness of fit χ2. The tolerance factor, which is the geometric measure of size mismatch of the perovskite: 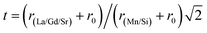 , is equal to 0.95 and is in the stable range for the perovskite structure 0.75 < t < 1.02.29
, is equal to 0.95 and is in the stable range for the perovskite structure 0.75 < t < 1.02.29
| LGSMSiO | Space group | Pnma |
|---|---|---|
a Density (exp.): 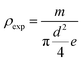 . Density(theor.). . Density(theor.). |
||
| Cell parameters | a (Å) | 5.4563(2) |
| b (Å) | 7.7241(3) | |
| c (Å) | 5.4961(5) | |
| V (Å3) | 231.63 | |
| Atoms | La/Gd/Srx | 0.0031(6) |
| z | 0.0046(2) | |
| Biso (Å2) | 0.32(3) | |
| Mn/Si Biso (Å2) | 0.26(3) | |
| (O1)x | 0.4837(2) | |
| y | 0.963(6) | |
| Biso (Å2) | 1.75(2) | |
| (O2)x | 0.31(1) | |
| y | 0.0019(5) | |
| z | 0.681(3) | |
| Biso (Å2) | 1.82(2) | |
| Structural parameters | dMn–O1 (Å) | 1.966(4) |
| θMn–O1–Mn (°) | 160.12(2) | |
| dMn–O2 (Å) | 1.962(1) | |
| θMn–O2–Mn (°) | 166.88(3) | |
| 〈dMn–O〉 (Å) | 1.964(4) | |
| 〈θMn–O–Mn〉 (Å) | 163.50(5) | |
| Density (theo.) (g cm−3) | 6.35 | |
| Density (exp.) (g cm−3) | 6.223 | |
| Compactness: C | 0.98 | |
| Agreement factors | Rp (%) | 3.55 |
| Rwp (%) | 2.12 | |
| RF (%) | 2.75 | |
| χ2 | 1.65 | |
The percentage of orthorhombic deformation, D%, can be obtained using the formula:30
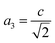 ,
, 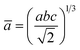 and a, b, c are the lattice parameters. The D% value for the sample estimated by using this equation is found to be 4%.
and a, b, c are the lattice parameters. The D% value for the sample estimated by using this equation is found to be 4%.
The experimental density is determined from the weight and geometrical dimensions of the cylindrical pellets and then compared to the theoretical density. The compactness is thus calculated as the ratio. Table 2 shows the values of ρexp, ρtheor and C.
3.2 Electrical conductivity analysis
Electrical conductivity in the materials is a thermally activated process that takes place due to the ordered motion of weakly bound charged particles under the influence of an electric field. It is one of the significant properties of the materials to be characterized and depends on the nature of charge carriers that dominate the conduction process, such as electron/holes or cations/anions and their response as a function of temperature and frequency. For the LGSMSiO materials, the electrical conductivity is mainly due to the hopping of electrons between the ions of the same element that are present in more than one valence state (Mn3+ → Mn4+).31 The charges can migrate under the influence of the applied field and contribute to the electrical response of the system. The frequency dependence of the conductivity generally obeys Jonscher's power law:32
σ(ω)![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) = = ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) σdc σdc![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) + +![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Aωn Aωn
| (1) |
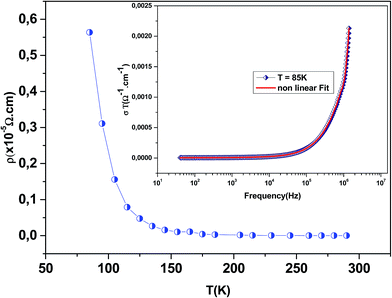
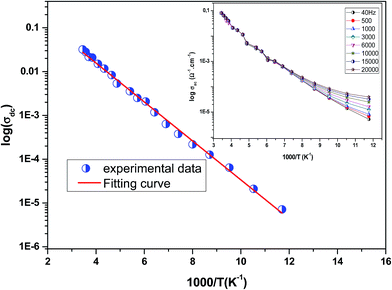
Fig. 3 shows the variation of AC conductivity as a function of frequency at different temperatures of the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 sample. It is characterized by the following points: (i) a plateau region at low frequencies corresponds to σdc. In this frequency region, the conductivity, σdc, increases with the increasing temperature. Such behavior indicates that the electrical conductivity in the material is a thermally activated process. (ii) At high frequency, the conductivity is governed by Aωn, where n is a constant and ω is the angular frequency. According to Jonscher, the origin of the frequency dependence of conductivity can be due to the relaxation phenomena of the ionic atmosphere arising from the mobile charge carriers.33 The experimental conductivity spectra of the sample are fitted using eqn (1). The fitting results are summarized in Table 3. From this table, we can conclude that the exponent n increases with the increasing temperature. This change of n with temperature corresponds to a thermally activated process. The temperature dependence of n gives information to specify the suitable mechanism involved for the AC conductivity. In most cases, the frequency exponent, n, is found to be between 0.6 and 1 for ionic conducting compounds.34 In our study, n is lower than 1 for temperatures lower than T = 205 K. This can be attributed to the hopping conduction of mobile charge carriers over the barrier between two sites, which is similar to that observed in amorphous semiconductors and glasses.35 However, for temperatures above 205 K, n is larger than one. This may be attributed to the motion of mobile charge carriers from site to site with quantum mechanical tunneling between asymmetric double-well potentials as is proposed by K. S. Gilroy et al.36 The inset of Fig. 4 represents an illustration of this fitting for the temperature T = 85 K. It can be seen that the fit (red solid line) matches well with the experimental values. Fig. 4 shows the plots of the electrical resistivity (ρ) versus temperature deduced from the dc conductivity (σdc) using the following relation: 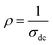 . This curve indicates that this compound exhibits semiconductor behavior across all the studied temperature ranges. Fig. 5 shows the variation of dc conductivity (σdc) versus 103/T used for the calculation of activation energy, and the plot clearly obeys the Arrhenius relation σdc
. This curve indicates that this compound exhibits semiconductor behavior across all the studied temperature ranges. Fig. 5 shows the variation of dc conductivity (σdc) versus 103/T used for the calculation of activation energy, and the plot clearly obeys the Arrhenius relation σdc![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) =
=![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) σ0
σ0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(−Eg/kBT), where σ0 is the pre-exponential factor corresponding to 1/T = 0, kB is the Boltzmann constant (=8.617 × 10−5 eV K−1), Eg is the conduction activation energy and T is the absolute temperature. For the sample, the activation energy (Eg) calculated from the slope of the graph is ∼(0.0383 ± 0.006) eV at 85–290 K.
exp(−Eg/kBT), where σ0 is the pre-exponential factor corresponding to 1/T = 0, kB is the Boltzmann constant (=8.617 × 10−5 eV K−1), Eg is the conduction activation energy and T is the absolute temperature. For the sample, the activation energy (Eg) calculated from the slope of the graph is ∼(0.0383 ± 0.006) eV at 85–290 K.
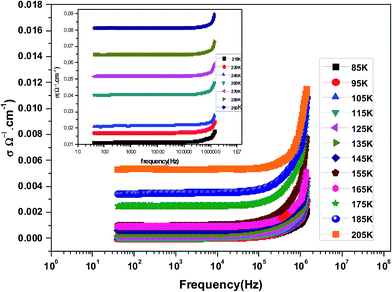 | ||
| Fig. 3 The variation at room temperature of the ac conductivity (σ) versus frequency for La0.6Gd0.1Sr0.3Mn0.75Si0.25O3. | ||
| Temperature (K) | σdc (×10−5 Ω−1 cm−1) | A (×10−9) | n | R2 (%) |
|---|---|---|---|---|
| 85 | 1.774 | 1.099 | 0.8856 | 99.99 |
| 95 | 3.218 | 7.907 | 0.8932 | 99.98 |
| 105 | 6.415 | 6.499 | 0.9165 | 99.99 |
| 115 | 12.670 | 3.785 | 0.9264 | 99.99 |
| 125 | 21.015 | 2.885 | 0.9322 | 99.99 |
| 135 | 37.727 | 5.751 | 0.9453 | 99.97 |
| 145 | 63.580 | 4.939 | 0.9561 | 99.99 |
| 155 | 96.416 | 1.328 | 0.9643 | 99.98 |
| 165 | 97.557 | 1.861 | 0.9723 | 99.99 |
| 175 | 250 | 3.584 | 0.9801 | 99.99 |
| 185 | 354 | 2.641 | 0.9857 | 99.99 |
| 205 | 535 | 4.361 | 0.9984 | 99.98 |
| 215 | 1145 | 7.914 | 1.0165 | 99.99 |
| 230 | 1723 | 2.021 | 1.0328 | 99.97 |
| 245 | 2140 | 7.812 | 1.0443 | 99.98 |
| 260 | 4054 | 9.342 | 1.0730 | 99.96 |
| 270 | 5173 | 5.898 | 1.1122 | 99.97 |
| 280 | 6486 | 3.425 | 1.1323 | 99.99 |
| 290 | 8112 | 2.547 | 1.2321 | 99.98 |
Inset of Fig. 5 shows the variation of σac![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) with temperature at different frequencies. It was observed that σac
with temperature at different frequencies. It was observed that σac![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) increased with the increase in temperature, and it obeyed the thermally activated relation: σac
increased with the increase in temperature, and it obeyed the thermally activated relation: σac ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) =
= ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) σ0
σ0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(−Eg/kBT), where σ0 is the pre-exponential factor, kB is the Boltzmann constant, Eg is the activation energy and T is the absolute temperature.37 It was also seen that the slope of the curve decreased with the increase in frequency. This indicated a reduction in Eg with increase in frequency due to enhanced electronic jumps between localized states.37 Furthermore, a merge of all of the curves at high temperatures may be due to intrinsic conductivity of the material at these temperature regions.38
exp(−Eg/kBT), where σ0 is the pre-exponential factor, kB is the Boltzmann constant, Eg is the activation energy and T is the absolute temperature.37 It was also seen that the slope of the curve decreased with the increase in frequency. This indicated a reduction in Eg with increase in frequency due to enhanced electronic jumps between localized states.37 Furthermore, a merge of all of the curves at high temperatures may be due to intrinsic conductivity of the material at these temperature regions.38
3.3 Modulus analysis
The complex electric modulus formalism can easily distinguish between electrode polarization effects and grain boundary conduction processes. It is also useful in detecting the bulk properties that appear from relaxation time.39 The variations of the imaginary part of the electrical modulus ‘M’ at different temperatures are shown in the inset of Fig. 6 from which we can note that the positions of the relaxation peaks shift toward higher frequencies as the temperature is increased. The low frequency side of the imaginary part of the modulus determines the range in which the charge carriers are mobile over long distances (the charge carriers suggest the possibility of ion migration via hopping from one site to the neighboring site). At a frequency above the peak maximum ‘M’ (high frequency), the carriers are spatially confined to potential wells being mobile over short distances and thus can be made to have localized motion within the well. The electric modulus M* represents the real dielectric relaxation process and should be replaced by a frequency dependent electric modulus which can be expressed by the following relation:40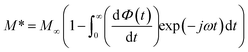 , where M∞ = 1/ε∞ is the asymptotic value of M′(ω) and ϕ(t) = exp(−(t/τM)β) represents the time evolution of the electric field within the material where β (0 < β < 1) is the stretched exponent and τM is the conductivity relaxation time.
, where M∞ = 1/ε∞ is the asymptotic value of M′(ω) and ϕ(t) = exp(−(t/τM)β) represents the time evolution of the electric field within the material where β (0 < β < 1) is the stretched exponent and τM is the conductivity relaxation time.
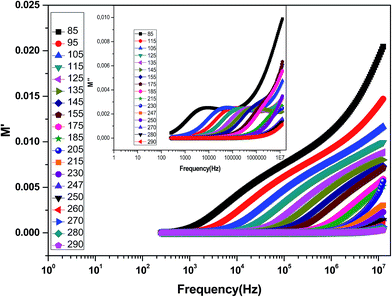 | ||
| Fig. 6 Variation of M′ with frequency at different temperatures for the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 compound. The inset shows the variation of M′′ with frequency at different temperatures. | ||
Fig. 6 shows the variation of the real part of the electrical modulus ‘M’ versus frequencies at different temperatures. We can note a very low ‘M’ value (close to zero) in the low-frequency region that increases with the increasing frequency, ultimately approaching the M∞ value. This may be attributed to a conduction phenomenon due to short-range mobility of charge carries. Herein, the relaxation frequency (fmax) corresponding to the ‘M’ peak denotes the transition from long-range to short-range motion with the increasing frequency. The temperature dependence of the frequency fmax in the low-frequency side of the dielectric relaxation can be described by the Arrhenius law. Thus the activation energy (Erelax) is calculated from 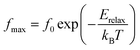 , where f0 is the pre-exponential term. Fig. 7 presents the variation of the logarithmic relaxation time log(τ) versus 103/T and log(fmax) versus 103/T in the temperature range of 85–215 K. The main values of the relaxation energy and the relaxation time obtained from the equation τ0
, where f0 is the pre-exponential term. Fig. 7 presents the variation of the logarithmic relaxation time log(τ) versus 103/T and log(fmax) versus 103/T in the temperature range of 85–215 K. The main values of the relaxation energy and the relaxation time obtained from the equation τ0 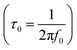 are 42.92 meV and 1.2 × 10−9 s, respectively.
are 42.92 meV and 1.2 × 10−9 s, respectively.
 | ||
| Fig. 7 (a) Variation of logarithmic relaxation time log(τ) versus 103/T and (b) plot of log(fmax) versus 103/T. | ||
3.4 Complex impedance spectroscopy
To understand the effect of substitution of manganese by the non-magnetic Si ion on the electrical properties over a wide range of frequencies and temperatures, impedance spectroscopy was employed. This technique is one of the most useful investigation techniques because the impedance of grains can be separated from the order sources of impedance, namely grain boundaries and electrode effects.41 In complex impedance diagrams (Nyquist or Cole–Cole), the imaginary part of the impedance (Z′′) was plotted against the real part of impedance (Z′). The response of an ideal parallel circuit of resistance and capacitance ‘C’ was a semicircle centered on the real axis. C was calculated from the frequency of the semicircle maximum, whereas R was determined from the diameter of the semicircle. The impedance analysis of a compound is based on an idealized circuit model with discrete electrical components. This analysis is mainly accomplished by fitting the impedance data to an equivalent circuit, which is representative of the material under investigation.Fig. 8 shows the imaginary part of the impedance (Z′′) versus the real part (Z′) over a wide range of frequencies and at different temperatures. These plots are characterized by the appearance of semicircular arcs whose maxima decease with the increasing temperature. The appearance of a single semicircle at all the temperatures means that the electrical processes obey a single relaxation mechanism.42 The diameter of the semicircle decreases with the increasing temperature, demonstrating a pronounced increase in dc conduction. To interpret such a diagram, it is necessary to model the compound.43,44 The experimental data are fitted using the Zview software and the best fit (in Fig. 8, it is presented as a red solid line) is obtained when employing an equivalent circuit formed by a resistance R1 (grain resistance Rg) in series with a parallel combination of a constant phase element impedance (ZCPE) and resistance R2 (grain boundary resistance Rgb). The equivalent configuration is of the type (R1 + (R2//ZCPE)) as shown in the inset of Fig. 8. The values of all fitted parameters are tabulated in Table 4. As the grain resistance (Rg) is too weak, the total resistance (RT), which is the sum of grain and grain boundary resistances, is approximately equal to the grain boundary contribution.45
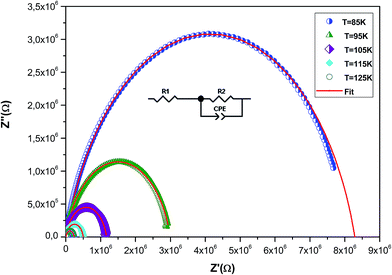 | ||
| Fig. 8 Complex impedance spectrum (Nyquist plot) for the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 sample at different temperatures with the electrical equivalent circuit (see the inset). | ||
| T (K) | R1 = Rg (Ω) | R2 = Rgb × 105 (Ω) | CPE (nF) | α |
|---|---|---|---|---|
| 85 | 680.3 | 8.2857 | 0.228 | 0.836 |
| 95 | 658 | 2.995 | 0.2347 | 0.828 |
| 105 | 629 | 1.1684 | 0.549 | 0.825 |
| 115 | 550 | 0.5005 | 0.2842 | 0.821 |
| 125 | 465.3 | 0.2395 | 0.3568 | 0.819 |
| 135 | 461.12 | 0.1220 | 0.358 | 0.819 |
| 145 | 419 | 0.06760 | 0.3933 | 0.817 |
| 155 | 395 | 0.04220 | 0.999 | 0.815 |
| 175 | 384 | 0.01510 | 1.285 | 0.812 |
| 185 | 372 | 0.0123 | 1.448 | 0.806 |
| 215 | 366 | 0.0106 | 1.690 | 0.799 |
| 230 | 357 | 0.0102 | 1.978 | 0.798 |
| 245 | 251 | 0.0098 | 2.122 | 0.789 |
| 260 | 130 | 0.0085 | 2.462 | 0.796 |
| 270 | 92 | 0.0064 | 3.025 | 0.792 |
| 280 | 73 | 0.0058 | 3.326 | 0.798 |
| 290 | 60 | 0.0049 | 4.651 | 0.795 |
The grain boundary resistance decreases with the increasing temperature, indicating a semi-conducting behavior for the compound (Table 4). This result is in good agreement with that of ρ(T) curves (Fig. 4). Such behavior has also been reported in other studies.46 It is revealed that the effect of grain boundaries helps lower the barrier to movement of the load carriers, resulting in increased electrical transport with an increase in temperature.
Fig. 9 shows the variation of the real part of impedance (Z′) with frequency at different temperatures. It is clear from the Z′ = f(freq) curves that the impedance value is higher at lower temperatures in the low-frequency domain and then, it decreases gradually with the increasing frequency. It is seen that Z′ decreases with an increase in frequency, which signifies the enhancement of ac conductivity with the increase in frequency. Moreover, Z′ decreases with an increase in temperature. It is observed that Z′ values merge at the high frequency side, which is due to a possible release of space charge.47
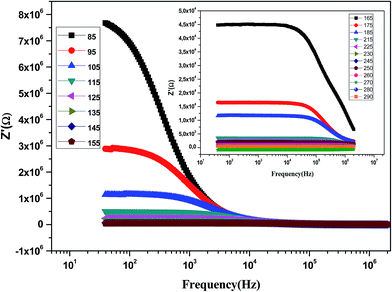 | ||
| Fig. 9 Variation of the real part of the impedance (Z′) of the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 sample as a function of frequency for different temperatures. | ||
The variation of the imaginary part of impedance (Z′′) of the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 sample as a function of frequency for different temperatures is shown in Fig. 10. It is found that the Z′′ value decreases with the increase in both temperature and frequency, and it shifts to higher frequencies as the temperature increases. This behavior describes both the type and the strength of the thermal relaxation processes in the material. This process is probably due to the presence of electrons and/or immobile species at lower temperatures and defects and vacancies at higher temperatures.47 The inset in Fig. 10a shows the normalized imaginary parts of the impedance 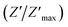 as a function of frequency at the selected temperatures. The peaks are observed with a slight symmetric broadening at each temperature, especially at higher temperature. The asymmetric broadening of the peaks suggests the presence of electrical processes in the material with a spread of relaxation times.48
as a function of frequency at the selected temperatures. The peaks are observed with a slight symmetric broadening at each temperature, especially at higher temperature. The asymmetric broadening of the peaks suggests the presence of electrical processes in the material with a spread of relaxation times.48
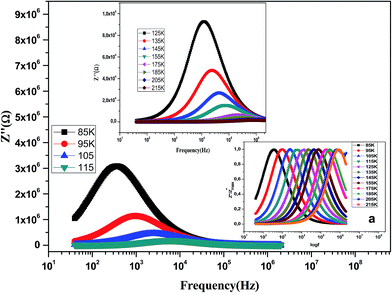 | ||
Fig. 10 Variation of the imaginary part of the impedance (Z′′) of the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 compound as a function of frequency for different temperatures. Inset: variation of 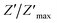 with log(f). with log(f). | ||
The position of these peaks allows for the determination of the relaxation frequency value (fmax) and the relaxation time (τ) using the relation: τ ![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) =
=![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1/2π
1/2π![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) fmax. The variation of log(τ) versus 103/T is shown in Fig. 11. We can see that the value of τ decreases with the increase in temperature, which suggests a thermally activated process. The dynamics of the relaxation process can be analyzed by the mean relaxation time τ expressed by the Arrhenius law
fmax. The variation of log(τ) versus 103/T is shown in Fig. 11. We can see that the value of τ decreases with the increase in temperature, which suggests a thermally activated process. The dynamics of the relaxation process can be analyzed by the mean relaxation time τ expressed by the Arrhenius law 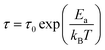 . This relaxation process is characterized by the activation energy (Ea) and a relaxation time (τ0) of about 41.7 meV and 1.55 × 10−9 s, respectively. The Ea value is in good agreement with that deduced from the modulus analysis, Erelax, which is about 42.92 meV. It is interesting to note that the energy deduced Erelax is equal to the activation energy Ea, which signifies that the relaxation process and therefore the electrical conductivity, is attributed to the effects of the grain boundaries. Indeed, the modulus analysis paragraph has reveals that the dielectric relaxation is assigned to the grain boundary effect.
. This relaxation process is characterized by the activation energy (Ea) and a relaxation time (τ0) of about 41.7 meV and 1.55 × 10−9 s, respectively. The Ea value is in good agreement with that deduced from the modulus analysis, Erelax, which is about 42.92 meV. It is interesting to note that the energy deduced Erelax is equal to the activation energy Ea, which signifies that the relaxation process and therefore the electrical conductivity, is attributed to the effects of the grain boundaries. Indeed, the modulus analysis paragraph has reveals that the dielectric relaxation is assigned to the grain boundary effect.
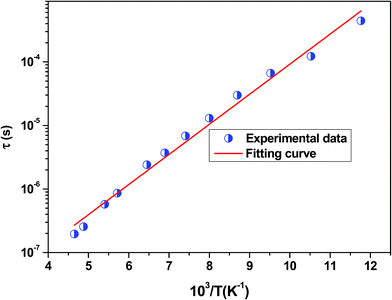 | ||
| Fig. 11 Variation of log(τ) versus 103/T for the La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 sample. Red solid line is the linear fit for our data. | ||
4. Conclusions
A La0.6Gd0.1Sr0.3Mn0.75Si0.25O3 ceramic was prepared by a solution-based sol–gel method, and its electrical properties were studied using the CIS technique. X-ray diffraction data confirmed that the sample was single phase with no detectable impurities and crystallized in the orthorhombic structure with the space group Pnma. The electrical properties were found to be strongly dependent on temperature and frequency. The ac conductivity spectra were found to obey the Jonscher's power law at different temperatures. The increase of the exponent, n, with the increasing temperature revealed a hopping process that occurred at longer distances for lower temperatures and between neighboring sites for higher temperatures. The impedance analysis revealed that low conductivity and high impedance values were observed at low temperatures. A broad peak observed in Z′′ vs. frequency plots at a particular temperature was sensitive to the concentration of silicon, and the peak shifts towards higher frequencies with the increasing temperatures demonstrated the multiple relaxation processes in the material. The relaxation time of dipoles was found to decrease with the increasing temperature.Conflicts of interest
There are no conflicts to declare.References
- N. Raju, D. Roja Sree, S. Shravan Kumar Reddy, Ch. Gopal Reddy, P. Yadagiri Reddy, K. Rama Reddy, V. Raghavendra Reddy and G. Reddy Turpu, J. Magn. Magn. Mater., 2014, 368, 308–311 CrossRef CAS.
- H. Rahmouni, R. Jemai, N. Kallel, A. Selmi and K. Khirouni, J. Alloys Compd., 2010, 497, 1–5 CrossRef CAS.
- Y. Zhao and P. Zhang, J. Alloys Compd., 2016, 658, 744–748 CrossRef CAS.
- X. Huang, H. Zhang, M. Wei, Y. Lai and J. Li, J. Alloys Compd., 2017, 708, 1026–1032 CrossRef CAS.
- J. X. Bi, C. C. Li, Y. H. Zhang, C. F. Xing, C. H. Yang and H. T. Wu, Mater. Lett., 2017, 196, 128–131 CrossRef CAS.
- V. Vidyadharan, E. Sreeja, A. Santhosh Kumar, C. Joseph, N. V. Unnikrishnan and P. R. Biju, Ceram. Int., 2017, 43, 6268–6275 CrossRef CAS.
- S. M. Yusuf, M. Sahana, K. Dorr, U. K. Robler and K. H. Muller, Phys. Rev. B, 2002, 66, 064430 CrossRef.
- J. L. Alonso, L. A. Fernandez, F. Guinea, V. Laliena and V. Martin-Mayor, Phys. Rev. B, 2002, 66, 104430 CrossRef.
- A. Omri, M. Bejar, E. Dhahri, M. Es-Souni, M. A. Valente, M. P. F. Graça and L. C. Costa, J. Alloys Compd., 2012, 536, 173–178 CrossRef CAS.
- M. Abassi, Z. Mohamed, J. Dhahri and E. K. Hlil, J. Alloys Compd., 2015, 639, 197–202 CrossRef CAS.
- N. Zaidi, S. Mnefgui, J. Dhahri and E. K. Hlil, Ceram. Int., 2016, 42, 17687–17692 CrossRef CAS.
- G. Mohamed Amara, Ah. Dhahri, J. Dhahri and E. K. Hlil, Mater. Res. Bull., 2017, 88, 91–97 CrossRef.
- Ah. Dhahri, M. Jemmali, E. Dhahri and E. K. Hlil, Dalton Trans., 2015, 44, 5620–5627 RSC.
- P. T. Phong, L. V. Bau, L. C. Hoan, D. H. Manh, N. X. Phuc and I.-J. Lee, J. Alloys Compd., 2016, 656, 920–928 CrossRef CAS.
- P. G. de Gennes, Phys. Rev., 1960, 118, 141 CrossRef CAS.
- J. L. Cohn, M. Peterca and J. J. Neumeier, Phys. Rev. B, 2004, 70, 214433 CrossRef.
- A. Pimenov, Ch. Hartinger, A. Loidl, A. A. Mukhin, V. Y. Ivanov and A. M. Balbashov, Phys. Rev. B, 1999, 59, 12419 CrossRef CAS.
- J. R. Sun, G. H. Rao, B. G. Shen and H. Wong, Appl. Phys. Lett., 1998, 73, 2998 CrossRef CAS.
- S. EL Kossi, F. I. H. Rhouma, J. Dhahri and K. Khirouni, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 440, 118–123 CrossRef CAS.
- H. Rahmouni, M. Smari, B. Cherif, E. Dhahri and K. Khirouni, Dalton Trans., 2015, 44, 10457–10466 RSC.
- S. Hcini, S. Khadhraoui, A. Triki, S. Zemni, M. Boudard and M. Oumezzine, J. Supercond. Novel Magn., 2014, 27, 195–201 CrossRef CAS.
- T. Goto, T. Kimura, G. Lawes, A. P. Ramirez and Y. Tokura, Phys. Rev. Lett., 2004, 92, 25720 CrossRef PubMed.
- S. Mnafgui, N. Zaidi, A. Dhahri, E. K. Hlil and J. Dhahri, J. Solid State Chem., 2014, 215, 193–200 CrossRef.
- M. Amara Gdaiem, S. Ghodhbane, Ah. Dhahri, J. Dhahri and E. K. Hlil, J. Alloys Compd., 2016, 681, 547–554 CrossRef.
- E. Tka, K. Cherif, J. Dhahri and E. Dhahri, J. Alloys Compd., 2011, 509, 8047–8055 CrossRef CAS.
- Y. W. Duan, X. L. Kou and J. G. Li, Phys. Rev. B: Condens. Matter Mater. Phys., 2005, 355, 250–254 CrossRef CAS.
- H. M. Rietveld, J. Appl. Crystallogr., 1969, 2, 65 CrossRef CAS.
- Ah. Dhahri, M. Jemmali, M. Hussein, E. Dhahri, A. Koumina and E. K. Hlil, J. Alloys Compd., 2015, 618, 788–794 CrossRef CAS.
- H. L. Ju, H. C. Sohn and K. M. Krishnan, Phys. Rev. Lett., 1997, 79, 3230s CrossRef.
- M. W. Shaikh and D. Varshney, Mater. Sci. Semicond. Process., 2014, 27, 418 CrossRef CAS.
- H. Rahmouni, M. Nouiri, R. Jemai, N. Kallel, F. Rzigua, A. Selmi, K. Khirouni and S. Alaya, J. Magn. Magn. Mater., 2007, 316, 23–28 CrossRef CAS.
- A. K. Jonscher, Universal Relaxation Law, Chelsea Dielectics Press, London, 1996 Search PubMed.
- E. Barsoukov and J. Ross Macdonald, Impedance Spectroscopy Theory, Experiment and Applications, 2nd edn, Wiley Interscience, New York, 2005, p. 14 Search PubMed.
- W. K. Lee, J. F. Liu and A. S. Nowick, Phys. Rev. Lett., 1994, 67, 1559 CrossRef PubMed.
- (a) S. R. Elliott, Adv. Phys., 1987, 36, 135 CrossRef CAS; (b) S. R. Elliott, Solid State Ionics, 1994, 70–71, 27 CrossRef CAS.
- K. S. Gilroy and W. A. Phillips, Philos. Mag. B, 1981, 43, 735 CAS.
- M. A. Afifi, A. E. Bekheet, E. A. Elwahabb and H. E. Atyia, Vacuum, 2001, 61, 9 CrossRef CAS.
- K. S. Rao, D. M. Prasad, P. M. Krishna, B. Tilak and K. C. Varadarajulu, Mater. Sci. Eng., B, 2006, 133, 141 CrossRef CAS.
- S. Hcini, S. Zemni, A. Triki, H. Rahmouni and M. Boudard, J. Alloys Compd., 2011, 509, 1394–1400 CrossRef CAS.
- N. Chihaoui, R. Dhahri, M. Bejar, E. Dharhi, L. C. Costa and M. P. F. Graça, Solid State Commun., 2011, 151, 133 CrossRef.
- J. T. S. Irvine, D. C. Sinclair and A. R. West, Adv. Mater., 2004, 2–3, 132–138 Search PubMed.
- A. Shukla, R. N. P. Choudhary and A. K. Thakur, J. Phys. Chem. Solids, 2009, 70, 1401 CrossRef CAS.
- D. K. Pradhan, B. K. Samantary, R. N. P. Chaudhary and A. K. Thakur, Mater. Sci. Eng., B, 2005, 116, 7 CrossRef.
- M. Nadeem, M. J. Akhtar and A. Y. Khan, Solid State Commun., 2005, 134, 431 CrossRef CAS.
- D. K. Pradhan, B. K. Samantary, R. N. P. Chaudhary and A. K. Thakur, Mater. Sci. Eng., B, 2005, 116, 7 CrossRef.
- H. Rahmouni, M. Nouiri, R. Jemai, N. Kallel, F. Rzigua, A. Selmi, K. Khirouni and S. Alaya, J. Magn. Magn. Mater., 2007, 316, 23–28 CrossRef CAS.
- Lily, K. Kumari, K. Prasad and R. N. P. Choudhary, J. Alloys Compd., 2008, 453, 325 CrossRef CAS.
- C. K. Suman, K. Prasad and R. N. P. Choudahary, J. Mater. Sci., 2006, 41, 369–375 CrossRef CAS.
| This journal is © The Royal Society of Chemistry 2018 |