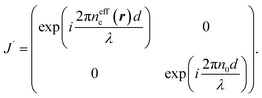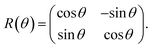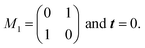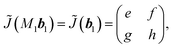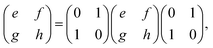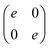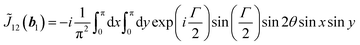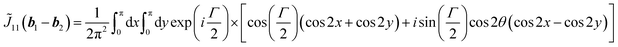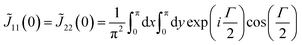Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Tunable two-dimensional polarization grating using a self-organized micropixelated liquid crystal structure†
Reo Amanoa,
Péter Salamon bc,
Shunsuke Yokokawaa,
Fumiaki Kobayashia,
Yuji Sasaki
bc,
Shunsuke Yokokawaa,
Fumiaki Kobayashia,
Yuji Sasaki *a,
Shuji Fujii
*a,
Shuji Fujii a,
Ágnes Bukab,
Fumito Araoka
a,
Ágnes Bukab,
Fumito Araoka c and
Hiroshi Orihara
c and
Hiroshi Orihara a
a
aDivision of Applied Physics, Faculty of Engineering, Hokkaido University, North 13 West 8, Kita-ku, Sapporo, Hokkaido 060-8628, Japan. E-mail: yuji.sasaki@eng.hokudai.ac.jp; Tel: +81 (0)11 7066642
bInstitute for Solid State Physics and Optics, Wigner Research Centre for Physics, Hungarian Academy of Sciences, H-1525 Budapest, P. O. Box 49, Hungary
cRIKEN Center for Emergent Matter Science (CEMS), 2-1 Hirosawa, Wako, Saitama 351-0198, Japan
First published on 12th December 2018
Abstract
Utilization of the self-organizing nature of soft materials is promising for fabricating micro- and nano-structures, which can be applied for optics. Because of the high birefringence, liquid crystals are especially suitable for optoelectronic applications such as beam steering and polarization conversion. On the other hand, most self-organized patterns in liquid crystals are one-dimensional and there are only a few examples of two dimensional systems. Here we study the light diffraction from a micro-pixelated pattern of a nematic liquid crystal which is formed by self-organization of topological defects. We demonstrate that the system works as a tunable two dimensional optical grating, which splits the incident laser beam and changes the polarization property. The intensity can be controlled by electrical voltages, which cause extinction of the zeroth-order beam. The polarization properties depend on the location of spots. The numerical calculation and the theoretical analysis not only support the experimental results but also unveil the uniqueness of the pixelated structure.
Introduction
Nematic liquid crystals (NLCs) are anisotropic fluids in which elongated molecules are aligned in a preferred direction called the director, n. Because the optical anisotropy can be controlled by electric fields, NLCs are suitable for optoelectronic applications such as displays. LC gratings are interesting examples, which are targeted to beam steering and optical filters.1–8 In particular, LC gratings can show high first-order diffraction efficiency and excellent polarization–separation properties.1,3,5,9–12 In order to fabricate gratings using LCs, a periodic modulation of director field in a small area is essentially required. Several methods have been proposed so far to realize high-resolution control of n for various LCs applications. Common approaches are to use top-down lithographic processes such as pre-patterned electrodes,1,11,13,14 scribing the substrate by AFM,15 and photo-alignment.9,16–18 Another candidate is the bottom-up approach that uses a self-organized periodic pattern.2,6,19–21 It is known that LCs generally exhibit rich pattern formation22–24 under external stimuli such as the electro-hydrodynamic effect25,26 and by the helical structure of the chiral NLCs.27–29 However these patterns are mostly limited to one-dimensional striped structure. Two dimensional patterns are relatively rare23–26 and moreover it is difficult to obtain a mono-domain structure in a large area. Thus, the diffraction properties of two dimensional self-organized patterns have not been investigated thoroughly. In this regard, recently, by doping an NLC with ionic additives, we have found a two-dimensional micropattern, which is self-organized by applying electric fields.30 The pattern consists of a square array of topological defects, which are often called umbilics.31,32 The umbilical defect structure is induced when homeotropically aligned NLC molecules with negative dielectric anisotropy are reoriented. Because this system does not require a pre-patterned surface, it is possible to change both the size of the unit cell and the birefringence with external fields. Thus, such a self-organized system is interesting for optical applications. One particular exploitation is the generation of an optical vortex from the defect structure.33,34 We have recently reported optical vortex generation using this two-dimensional grid pattern.35 There, we have simultaneously observed light diffraction. However, in the earlier paper, the diffraction has not been understood in detail.In this paper, we present the results of our comprehensive investigation aiming to understand light diffraction from the micro-pixelated LC pattern. We uncover how the diffraction pattern depends on the effective birefringence and on the frequency of the driving AC electrical voltage. The polarization state of the diffracted light is also studied. The experiments show that the intensity of diffraction spots can be controlled by the reorientation of the director field. Particularly, high diffraction-efficiency is realized under a moderate electrical voltage, which extincts the zeroth order spot. We also find that the polarization of diffracted light is converted differently depending on the diffraction order. In addition to experiments, we perform numerical calculations to understand the voltage dependence, which shows a qualitative agreement with the experimental results. The presented experimental and numerical results are understood theoretically, which also reveals the uniqueness of this pixelated structure.
Experimental
Fig. 1 shows the schematic illustration of a sample cell. Using two ITO (indium tin oxide)-coated glass plates, standard sandwich type cells are prepared. The ITO coating is stripe patterned with 1.2 mm wide and the substrates are spin-coated with a perfluoropolymer (CYTOP, Asahi Glass Co.). The thickness of the alignment layer is around 120 nm. Two substrates are overlapped so that the ITO stripes cross orthogonally each other. The mono-dispersed micro-spheres are used to maintain a proper thickness of the sample. From our earlier work, it is known that the obtained grid size is proportional to the sample thickness.30 Here, we use the sample cells with the gap of 13 μm and 21 μm. An NLC (4α,4′α-propylheptyl-1α,1′α-bicyclohexyl-4β-carbonitrile, CCN-37, Fig. 1(b)) doped with 1 wt% of an ionic additive (tetrabutylammonium benzoate, TBABE) is filled into the cell by capillary action. CCN-37 possesses a negative dielectric anisotropy (Δε ∼ −7) and the birefringence (Δn) is 0.03.36 At room temperature, CCN-37 shows the homeotropic alignment at the CYTOP surface.37 Then, by applying an ac electrical voltage V = V0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos(2πft), the director reorientation leads to the formation of a square array of umbilical defects in our system. A monodomain can be obtained by adjusting the electrical voltage. We use a He–Ne laser (633 nm in wavelength) to investigate the diffraction property. The monodomain formed in the area of 1.2 mm × 1.2 mm is large enough compared to the beam size of 0.7 mm in diameter. If necessary, wave plates and/or polarizers are placed in front of and behind the sample cell. The power of the diffracted light is measured with a power meter (Thorlabs PM100 USB) for each spot which is seen on the screen. The diffraction is captured with a CMOS camera (Thorlabs DCC1240C). Before starting the diffraction measurements, both the texture of the pattern and the irradiation position of laser beam are checked with polarized optical microscopy (POM). The microscopy is installed on the optical path so that the POM observation is possible without disarranging the optical setup.
cos(2πft), the director reorientation leads to the formation of a square array of umbilical defects in our system. A monodomain can be obtained by adjusting the electrical voltage. We use a He–Ne laser (633 nm in wavelength) to investigate the diffraction property. The monodomain formed in the area of 1.2 mm × 1.2 mm is large enough compared to the beam size of 0.7 mm in diameter. If necessary, wave plates and/or polarizers are placed in front of and behind the sample cell. The power of the diffracted light is measured with a power meter (Thorlabs PM100 USB) for each spot which is seen on the screen. The diffraction is captured with a CMOS camera (Thorlabs DCC1240C). Before starting the diffraction measurements, both the texture of the pattern and the irradiation position of laser beam are checked with polarized optical microscopy (POM). The microscopy is installed on the optical path so that the POM observation is possible without disarranging the optical setup.
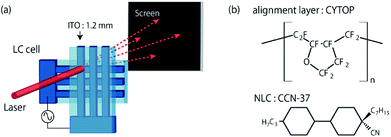 | ||
| Fig. 1 (a) Schematic illustration of the sample cell and the diffraction experiments. (b) The chemical structures of the alignment layer (CYTOP) and the NLC (CCN-37). | ||
Results and discussion
Experimental results
A POM image of the pattern is displayed in Fig. 2(a). The cell thickness is 13 μm. To induce the director reorientation, we increase the frequency, f under a constant amplitude, V0. The range of f is chosen not to break the pattern. The change in the micrographic appearance by the increase of f is shown in Fig. 2(b) and (c). Due to the enhanced retardation, the brightness changes under the same illumination condition. After preparing the suitable monodomain, a laser beam is irradiated to the sample cell. The inset of Fig. 2(b) is an image at which a linearly polarized laser beam is irradiated. The position of the laser beam on the pattern is checked. We see that the texture of the irradiated area is not damaged, indicating that the heating effect is negligible. The diffraction pattern obtained by a circularly polarized light is shown in Fig. 2(d)–(f). We can see a square array of spots whose direction is tilted by 45° from the horizontal axis. This clearly indicates that the primitive cell of the diffraction pattern corresponds to the square region displayed in Fig. 2(g) as already suggested in our recent study.35 We denote the primitive cell vectors by a1, a2. The primitive reciprocal vectors b1 and b2 are defined as ai·bj = 2πδij: ai is parallel to bi in our case. With b1 and b2, the spot (![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) 1,
1, ![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) 2) is expressed as
2) is expressed as ![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) 1b1 +
1b1 + ![[small script l]](https://www.rsc.org/images/entities/i_char_e146.gif) 2b2 as shown in Fig. 2(e). Here we focus on nine spots {(0, 0), (±1, 0), (0, ±1), (±1, ±1), (±1, ∓1)}. We do not study higher order spots because their intensity is quite weak. With a constant V0, the effect of the frequency for the diffraction pattern is shown in Fig. 2(d)–(f). It is seen that the intensity at each spot exhibits the frequency dependence. In the low frequency, i.e., in the beginning of the pattern formation from the homeotropic alignment, the zeroth-order spot I(0, 0) is dominant. This is obvious because the director field is almost perpendicular to the cell substrate. As the frequency increases, the intensity I(0, 0) decreases, while surrounding spots become reinforced. For ∼20 Hz, the zeroth-order diffraction spot almost disappears (Fig. 2(f)), i.e., a high diffraction efficiency is realized. Further increase of f does not change the brightness of the spots. This indicates that the director in the cell is well oriented and the retardation cannot be increased further in this cell. To obtain the quantitative data, the intensity of the spots is plotted as a function of frequency. (Fig. 2(h)) The intensity is normalized with that of the incident light (40 μW). As seen from the diffraction pattern, I(0, 0) decreases. For higher frequency (≥20 Hz), the change in the intensity is small. A crude estimation is made for how much light is split. We approximate the total power of the diffracted light as I(0, 0) + 4(I(1, 0) + I(1, 1)). The behavior of 4(I(1, 0) + I(1, 1)) which is the surrounding bright spots, demonstrates that I(0, 0) is diffracted to the surrounding spots efficiently. Considering that the transmitted light behind the substrate amounts around 80%, we find that 87% of the transmitted light is diffracted for the surrounding eight spots when I(0, 0) takes the minimum value and the rest amount is used for higher-order spots.
2b2 as shown in Fig. 2(e). Here we focus on nine spots {(0, 0), (±1, 0), (0, ±1), (±1, ±1), (±1, ∓1)}. We do not study higher order spots because their intensity is quite weak. With a constant V0, the effect of the frequency for the diffraction pattern is shown in Fig. 2(d)–(f). It is seen that the intensity at each spot exhibits the frequency dependence. In the low frequency, i.e., in the beginning of the pattern formation from the homeotropic alignment, the zeroth-order spot I(0, 0) is dominant. This is obvious because the director field is almost perpendicular to the cell substrate. As the frequency increases, the intensity I(0, 0) decreases, while surrounding spots become reinforced. For ∼20 Hz, the zeroth-order diffraction spot almost disappears (Fig. 2(f)), i.e., a high diffraction efficiency is realized. Further increase of f does not change the brightness of the spots. This indicates that the director in the cell is well oriented and the retardation cannot be increased further in this cell. To obtain the quantitative data, the intensity of the spots is plotted as a function of frequency. (Fig. 2(h)) The intensity is normalized with that of the incident light (40 μW). As seen from the diffraction pattern, I(0, 0) decreases. For higher frequency (≥20 Hz), the change in the intensity is small. A crude estimation is made for how much light is split. We approximate the total power of the diffracted light as I(0, 0) + 4(I(1, 0) + I(1, 1)). The behavior of 4(I(1, 0) + I(1, 1)) which is the surrounding bright spots, demonstrates that I(0, 0) is diffracted to the surrounding spots efficiently. Considering that the transmitted light behind the substrate amounts around 80%, we find that 87% of the transmitted light is diffracted for the surrounding eight spots when I(0, 0) takes the minimum value and the rest amount is used for higher-order spots.
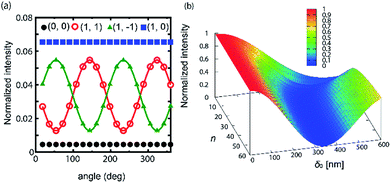
In addition to the effect of the electrical voltage for the light intensity, the polarization properties are studied by placing an analyzer behind the sample cell. Typical results are summarized in Fig. 3, which uses a cell with the thickness of 21 μm. POM observation under crossed-polarizers shows that the size of the grid becomes large compared to Fig. 2 because it is proportional to the sample thickness.30 By increasing the frequency, POM images show a significant color alternation due to the change of the retardation. (Fig. 3(b) and (c)) Considering the birefringence of Δn ∼ 0.03, the possible maximum value of Δnd is ∼600 nm if the director configuration is planar. This can be seen as a blue color under POM, which qualitatively agrees with the observation. This suggests that the director is substantially tilted in the sample cell around 25 Hz. The incident light is circularly polarized as used in Fig. 2. The diffraction pattern exhibits a similar trend to that of 13 μm cell. When the director tilts from the homeotropic alignment, the value of I(0, 0) approaches zero because the light is split. (ESI Fig S1†) An additional feature observed for the 21 μm-thick cell is that the decreased I(0, 0) is reinforced from the minimum value by increasing the frequency. This is because the retardation can be varied over a wide range compared to the 13 μm-thick cell. Fig. 3(d) and (e) show that the brightness changes depending on the direction of analyzer. We notice that there are two types of spots whose intensity is independent {(0, 0), (±1, 0), (0, ±1)} and dependent {(±1, ±1), (±1, ∓1)} from the direction of analyzer. This means that the polarization of the diffracted light is converted differently depending on the spots. We also see that the quality of the diffracted spots is slightly low compared to the 13 μm-thick cell. For example, the zeroth order spot is distorted with the direction of the analyzer. However, this is due to the imperfection of the grid-like structure and the decreased number of the grids in the irradiated area. In order to investigate details, the frequency is adjusted at a moderate value so that all the spots exhibit comparable brightness. The intensity at each spot is plotted as a function of the direction of analyzer in Fig. 3(f). We note that the low value in the normalized intensity is due to the presence of the polarizers which absorb the light. For the spots of {(0, 0), (±1, 0), (0, ±1)}, we can expect that the diffracted light is circularly polarized. On the other hand, the spots of {(±1, ±1), (±1, ∓1)} indicate that the polarization is elliptical. For these four spots, the angular dependence of the intensity of (±1, ±1) and (±1, ∓1) is opposite, i.e., if I(±1, ±1) decrease, I(±1, ∓1) increase. The phase shift for {(±1, ±1), (±1, ∓1)} varies by the applied electric field. Further experiments are carried out using a linearly polarized light. The diffraction pattern is studied under cross- and parallel-polarized conditions. (Fig. 3(g)–(i)) The zeroth-order spot can be erased as far as the cross-polarized condition is kept. For the other diffraction spots, the brightness changes by rotating crossed-polarizers. If a polarizer is set parallel to x-axis, four spots at (±1, 0), (0, ±1) are obtained. On the other hand, when the crossed-polarizers are rotated by 45°, the spots (±1, 0), (0, ±1) disappear and the spots of (±1, ±1), (±1, ∓1) appear. Thus, I(0, 0) and other spots (±1, 0), (0, ±1) which are independent of the direction of analyzer (Fig. 3(f)), have different property of polarization conversion. The different conversion can be found under parallel polarized condition. We find that I(±1, 0) and I(0, ±1) cannot be observed. These observations indicate that the conversion at (±1, 0) and (0, ±1) is the same as that of the half-wave plate whose optical axis is directed to the origin.
Numerical calculation
To understand the observation, we calculate the diffraction numerically by using the Jones matrix method. This approach is valid because the diffraction angle of this experiment is typically around 0.01 rad. Firstly, we estimate the effective retardation in two dimension and map it to the projected director field. It is to be noted that the maximum retardation which is the value at the center of each grid, increases together with the spatial change of the director field by increasing the frequency. Then, to describe the frequency dependence, we express the retardation as Δnd = (neeff(r) − n0)d = δ0F(r) = δ(r) where r = (x, y). δ0 is the maximum value of the retardation at the center of each grid and F(x, y) is a function which qualitatively reproduces the effect of the director tilts. Supposing that the cell thickness is 20 μm, δ0 ≤ 600 nm and 0 ≤ F(r) ≤ 1 are imposed. Using Jones matrix J(r) for the LC cell, the diffraction pattern at the screen is calculated with E(k) ∝ ∫J(r)E(r)exp(−ik·r)dr. We assume that the incoming laser beam E(r) is uniform over the irradiated area, i.e., E(r) = Ein. Then E(k) ∝ ∫J(r)exp(−ik·r)dr·Ein. When two optical axes of the director field are parallel to x- and y-axis, the Jones matrix J′ is given asneeff(r) varies depending on r. Then we obtain
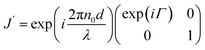 | (1) |
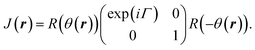 | (2) |
Here θ(r) is the rotation angle of the director from x-axis. R(θ) is the rotation matrix, which is given as
Using (nx, ny), we obtain tan−1(ny/nx). To describe the director field shown in Fig. 2(g), we use a vector field of
(nx, ny) ∝ (sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) x, sin x, sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) y). y). |
First, we examine the case for the constant retardation of F(x, y) = 1, i.e., Δnd = δ0. This basically corresponds to the planar alignment with defects. Some calculated diffraction patterns are plotted in Fig. 4(a) and (b), where circularly polarized input light is used. The appearance seems to reproduce the experiments. We change the value of the retardation, which qualitatively corresponds to the application of the electric field. (Fig. 4(c)) We find that the diffraction efficiency changes depending on the retardation of the sample. For small δ0, the zeroth-order spot I(0, 0) is dominant and the surrounding spots are weak. At 2δ0 = λ, I(0, 0) vanishes and the diffraction efficiency reaches 100%. The surrounding spots increase the intensity. When δ0 is increased further, I(0, 0) increases again and the surrounding spots become darker. The intensity for (1, 0) is higher than that for (1, 1). The tendency agrees with the experiments. On the other hand, a contrast is found in the polarization properties (Fig. 4(d)). The intensity at each spot is obtained by placing analyser behind the sample. In contrast to the experiments, the calculation does not show the angular dependence of the rotating analyser for all the spots. This means that the split light is circularly polarized even for (1, ±1) and (−1, ±1) when the alignment is planar. The calculated diffraction pattern under crossed- and parallel-polarizers is shown in Fig. 4(e) and (f). The appearance is similar to that of the experiment. Thus, we notice that the spots of (±1, 0) and (0, ±1) behave as a half wave plate whose optical axis is directed to x- and y-axes. Thus, as suggested from the experiments, when the polarization of the incident light is parallel to x-axis, it can pass through the analyzer even in a cross-polarized condition. From the above, we find that the planar alignment condition qualitatively explains the experiments, while the obtained numerical results are incomplete in part.
In our experimental system, the retardation varies depending on the reorientation of the director. To evaluate the influence of umbilical defects qualitatively, we use a function F(x, y) = (2 −cosn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) x − cosn
x − cosn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) y)/2 for effective value. n takes an even number. For a small n, this corresponds to the beginning of the formation of the grid-like texture, while further tilted configuration can be described with a larger n. We consider the effects by changing δ0 and n. In Fig. 5(a), we show a calculation which is made with δ0 = 350 nm and n = 40. An important finding is that for (±1, ±1), (±1, ∓1), the polarization property is modified, which agrees with the experiments. This means that the elliptically polarized light at these spots is due to the spatial modulation of the birefringence. Moreover, from the simulated results, we can elucidate the director field of the sample. For the presented case, it is suggested that the director field changes suddenly near the core of the defect and the other region has a uniform tilt. We also check the behavior of I(0, 0), which is plotted as the function of δ0 and n. (Fig. 5(b)) When n has a larger value, the graph shows a minimum. In particular, I(0, 0) becomes close to 0 for a high value of n. Similar to the case of the planar configuration (Fig. 4(c)), we notice that I(0, 0) exhibits a minimum point around δ0 ∼ λ/2. This demonstrates that our experimental system can also realize nearly 100% diffraction efficiency.
y)/2 for effective value. n takes an even number. For a small n, this corresponds to the beginning of the formation of the grid-like texture, while further tilted configuration can be described with a larger n. We consider the effects by changing δ0 and n. In Fig. 5(a), we show a calculation which is made with δ0 = 350 nm and n = 40. An important finding is that for (±1, ±1), (±1, ∓1), the polarization property is modified, which agrees with the experiments. This means that the elliptically polarized light at these spots is due to the spatial modulation of the birefringence. Moreover, from the simulated results, we can elucidate the director field of the sample. For the presented case, it is suggested that the director field changes suddenly near the core of the defect and the other region has a uniform tilt. We also check the behavior of I(0, 0), which is plotted as the function of δ0 and n. (Fig. 5(b)) When n has a larger value, the graph shows a minimum. In particular, I(0, 0) becomes close to 0 for a high value of n. Similar to the case of the planar configuration (Fig. 4(c)), we notice that I(0, 0) exhibits a minimum point around δ0 ∼ λ/2. This demonstrates that our experimental system can also realize nearly 100% diffraction efficiency.
Theoretical consideration
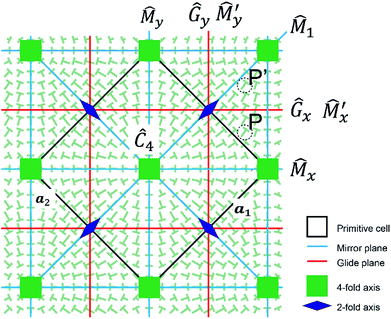
We discuss the results of diffraction patterns from a symmetrical point of view. Taking into account that two director states (nx, ny, nz) and (nx, ny, −nz) are optically the same, i.e., the corresponding Jones matrices are the same, the grid-like pattern belongs to the two-dimensional space group P4m. The symmetry elements are shown in Fig. 6. The symmetry operation can be expressed as r′ =![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) r = Tr + t, where T is a matrix representing a rotation or a mirror (reflection) with respect to the origin, and t is a translation vector. A Jones matrix J(r) is transformed to TJ(r)T−1 by
r = Tr + t, where T is a matrix representing a rotation or a mirror (reflection) with respect to the origin, and t is a translation vector. A Jones matrix J(r) is transformed to TJ(r)T−1 by ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) as the Jones matrices are second rank tensors, and r is moved to Tr + t. Since
as the Jones matrices are second rank tensors, and r is moved to Tr + t. Since ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) is a symmetry operator, we have
is a symmetry operator, we have| J(Tr + t) = TJ(r)T−1 | (3) |
The Jones matrix in the Fourier space is defined as
 | (4) |
![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (Tk)exp(−iTk·t) = T (Tk)exp(−iTk·t) = T![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (k)T−1 (k)T−1
| (5) |
In the following, we examine the symmetry of ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (k) at some k based on eqn.5 For simplicity, we use the director field of Fig. 2(g) because the following results are valid independent of specific director fields as far as the symmetry is P4m. First, we consider a symmetry operation with a mirror
(k) at some k based on eqn.5 For simplicity, we use the director field of Fig. 2(g) because the following results are valid independent of specific director fields as far as the symmetry is P4m. First, we consider a symmetry operation with a mirror ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) 1 (i.e.,
1 (i.e., ![[T with combining circumflex]](https://www.rsc.org/images/entities/i_char_0054_0302.gif) r =
r = ![[M with combining circumflex]](https://www.rsc.org/images/entities/i_char_004d_0302.gif) 1r = M1r + t) which is the diagonal direction in Fig. 6. Here, M1 and t are given as
1r = M1r + t) which is the diagonal direction in Fig. 6. Here, M1 and t are given as
For k = b1, we notice that M1b1 = b1. By using
which leads to
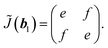 | (6) |
The symmetric form of Jones matrix indicates that for the diffracted light at b1 the sample plays a role of a wave-plate whose principal axes (the optical axes) are diagonal between x- and y-axes. To obtain ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b2), we use another symmetry operation which rotates the director by
(−b2), we use another symmetry operation which rotates the director by 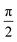 with respect to z-axis. (Ĉ4) Taking k = b1, we obtain from eqn (5)
with respect to z-axis. (Ĉ4) Taking k = b1, we obtain from eqn (5)
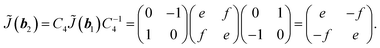 | (7) |
In the same way, the operation of Ĉ4 can be applied for ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b1) and
(−b1) and ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b2), which leads to
(−b2), which leads to
![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b1) = (−b1) = ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b1) (b1)
| (8) |
![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b2) = (−b2) = ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b2) (b2)
| (9) |
Second, we consider k = b1 − b2. Using a mirror operator in x-direction (![[M with combining tilde]](https://www.rsc.org/images/entities/i_char_004d_0303.gif) x), eqn (5) gives
x), eqn (5) gives
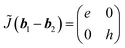 | (10) |
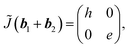 | (11) |
![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b1 + b2) = (−b1 + b2) = ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b1 − b2), (b1 − b2),
| (12) |
![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b1 − b2) = (−b1 − b2) = ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b1 + b2). (b1 + b2).
| (13) |
At these diffraction spots, the optical axes are in x- and y-directions.
Last, we show only the result at k = 0,
From experiments and numerical calculations, for k = b1, the property of the polarization conversion is the same as that of the half-wave plate whose optical axes are directed to the origin. This indicates that e of ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b1) in eqn (6) should vanish. The above-mentioned symmetry never leads to the expected result. However, we notice another symmetry (this is not usual symmetry) in Fig. 6, that is, the tilt angles at P and P′ are the same, which is mutually exchanged by the mirror
(b1) in eqn (6) should vanish. The above-mentioned symmetry never leads to the expected result. However, we notice another symmetry (this is not usual symmetry) in Fig. 6, that is, the tilt angles at P and P′ are the same, which is mutually exchanged by the mirror  (note that
(note that 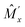 is different from the glide Ĝx, although they are in the same line.).
is different from the glide Ĝx, although they are in the same line.). 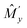 is also this type of operator. In general, these operators do not exist although they do in our case of (nx, ny) ∝ (sin
is also this type of operator. In general, these operators do not exist although they do in our case of (nx, ny) ∝ (sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) x, sin
x, sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) y). It is easily confirmed that they exist for (nx, ny) ∝ (g(x), g(y)) with
y). It is easily confirmed that they exist for (nx, ny) ∝ (g(x), g(y)) with 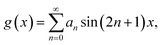 where an are arbitrary constants. For
where an are arbitrary constants. For  the following equation is valid instead of eqn (5) as
the following equation is valid instead of eqn (5) as ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) does not change by
does not change by 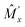
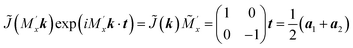 | (14) |
Substitution of k = b1 into eqn (14) yields −![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (−b2) =
(−b2) = ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (b1). Using eqn (6), (7) and (9), we finally obtain e = 0, that is, the Jones matrix of the half-wave plate:
(b1). Using eqn (6), (7) and (9), we finally obtain e = 0, that is, the Jones matrix of the half-wave plate:
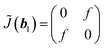 | (15) |
The symmetry also allows us to reduce ![[J with combining tilde]](https://www.rsc.org/images/entities/i_char_004a_0303.gif) (k), when we calculate the integration in eqn (4) by using eqn (2). We assume that θ(r) and δ(r) have the symmetry of P4m. The non-vanishing elements are given as
(k), when we calculate the integration in eqn (4) by using eqn (2). We assume that θ(r) and δ(r) have the symmetry of P4m. The non-vanishing elements are given as
when δ is constant and tan
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ = sin
θ = sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) y/sin
y/sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) x, corresponding to high voltage states, the above equations are reduced;
x, corresponding to high voltage states, the above equations are reduced;
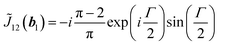 | (16) |
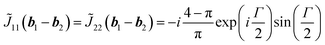 | (17) |
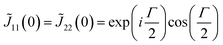 | (18) |
Particularly, from eqn (17), it is seen that the diffraction at k = b1 − b2 plays a role of the half-wave plate as well as that at k = b1. In other words, a feature of the spot at b1 − b2 is that the elliptical polarization can be tuned by the electrical voltage. However, since the grid-like structure simultaneously becomes unstable under a high-voltage, the realization of the tunability is a future work.
Conclusions
To conclude, we have reported how light is diffracted on a two-dimensional micro-structure of nematic liquid crystals. Because the structure is obtained by self-organization, the pattern works as an electrically tunable as well as switchable optical grating. Under a moderate electrical voltage, the intensity of the zeroth-order diffraction spot can be tuned, which realizes a high diffraction efficiency. In addition, it is demonstrated that the polarization of the incident light can be converted to different kinds depending on the location of spots. The numerical and theoretical calculations also reproduce well the experiments. Particularly, at some spots, this pattern has a function that inverts the handedness of the circularly polarized light. From the theoretical analysis, it is found that the symmetry of the director field plays important roles for the type of polarization of the diffracted light. These results suggest that further investigations can be performed by changing the birefringence of NLCs and by changing the pattern. Moreover, the combination with the polymer-stabilization technique is interesting. This allows us not only to maintain the director field but also tune the optical properties by external stimuli such as temperature and electric fields,4,7 which can be applied in the present system.38Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work is supported by the “Hattori-Hokokai” Foundation, the JSPS KAKENHI 18H01851, the NKFIH PD 121019 and FK 125134 grants. P. S., A. B., and F. A. are grateful to the JSPS-HAS bilateral joint research program for the support for their collaboration.Notes and references
- J. Chen, P. J. Bos, H. Vithana and D. L. Johnson, Appl. Phys. Lett., 1995, 67, 2588–2590 CrossRef CAS.
- D. Subacius, P. J. Bos and O. D. Lavrentovich, Appl. Phys. Lett., 1997, 71, 1350–1352 CrossRef CAS.
- N. Kawatsuki, T. Hasegawa, H. Ono and T. Tamoto, Adv. Mater., 2003, 15, 991–994 CrossRef CAS.
- H. Ren, Y.-H. Fan and S.-T. Wu, Appl. Phys. Lett., 2003, 82, 3168–3170 CrossRef CAS.
- H. Ono, A. Emoto, F. Takahashi, N. Kawatsuki and T. Hasegawa, J. Appl. Phys., 2003, 94, 1298–1303 CrossRef CAS.
- B. I. Senyuk, I. I. Smalyukh and O. D. Lavrentovich, Opt. Lett., 2005, 30, 349 CrossRef CAS PubMed.
- J. Yan, Y. Li and S.-T. Wu, Opt. Lett., 2011, 36, 1404 CrossRef PubMed.
- H. Chen, G. Tan, Y. Huang, Y. Weng, T.-H. Choi, T.-H. Yoon and S.-T. Wu, Sci. Rep., 2017, 7, 39923 CrossRef CAS PubMed.
- J.-H. Park, C.-J. Yu, J. Kim, S.-Y. Chung and S.-D. Lee, Appl. Phys. Lett., 2003, 83, 1918–1920 CrossRef CAS.
- M. Le Doucen and P. Pellat-Finet, Opt. Commun., 1998, 151, 321–330 CrossRef CAS.
- D. Xu, G. Tan and S.-T. Wu, Opt. Express, 2015, 23, 12274 CrossRef CAS PubMed.
- A. Ryabchun, A. Bobrovsky, Y. Gritsai, O. Sakhno, V. Shibaev and J. Stumpe, ACS Appl. Mater. Interfaces, 2015, 7, 2554–2560 CrossRef CAS PubMed.
- Z. He, T. Nose and S. Sato, Jpn. J. Appl. Phys., Part 1, 1996, 35, 3529–3530 CrossRef CAS.
- J.-L. Zhu, J.-G. Lu, J. Qiang, E.-W. Zhong, Z.-C. Ye, Z. He, X. Guo, C.-Y. Dong, Y. Su and H.-P. D. Shieh, J. Appl. Phys., 2012, 111, 033101 CrossRef.
- B. Wen, R. G. Petschek and C. Rosenblatt, Appl. Opt., 2002, 41, 1246 CrossRef PubMed.
- W. M. Gibbons and S. Sun, Appl. Phys. Lett., 1994, 65, 2542–2544 CrossRef CAS.
- H. Yoshida, K. Asakura, J. Fukuda and M. Ozaki, Nat. Commun., 2015, 6, 7180 CrossRef CAS PubMed.
- Y. Guo, M. Jiang, C. Peng, K. Sun, O. Yaroshchuk, O. Lavren-tovich and Q. H. Wei, Adv. Mater., 2016, 28, 2353–2358 CrossRef CAS PubMed.
- H.-C. Jau, T.-H. Lin, Y.-Y. Chen, C.-W. Chen, J.-H. Liu and A. Y.-G. Fuh, Appl. Phys. Lett., 2012, 100, 131909 CrossRef.
- Y. Xiang, H.-Z. Jing, Z.-D. Zhang, W.-J. Ye, M.-Y. Xu, E. Wang, P. Salamon, N. Éber and Á. Buka, Phys. Rev. Appl., 2017, 7, 064032 CrossRef.
- N. Vaupotič, M. Ali, P. W. Majewski, E. Gorecka and D. Pociecha, ChemPhysChem, 2018, 19, 2566–2571 CrossRef PubMed.
- R. B. Meyer, Phys. Rev. Lett., 1969, 22, 918–921 CrossRef CAS.
- P. Pieranski, E. Dubois-Violette and E. Guyon, Phys. Rev. Lett., 1973, 30, 736–739 CrossRef CAS.
- M. R. Kuzma, Phys. Rev. Lett., 1986, 57, 349–352 CrossRef CAS PubMed.
- A. Buka and L. Kramer, Pattern formation in liquid crystals, Springer New York, New York, NY, 1996 Search PubMed.
- L. K. Migara and J.-K. Song, NPG Asia Mater., 2018, 10, e459 CrossRef.
- H.-C. Yeh, G.-H. Chen, C.-R. Lee and T.-S. Mo, J. Chem. Phys., 2007, 127, 141105 CrossRef PubMed.
- S.-W. Kang and L.-C. Chien, Appl. Phys. Lett., 2007, 90, 221110 CrossRef.
- A. Ryabchun and A. Bobrovsky, Adv. Opt. Mater., 2018, 6, 1800335 CrossRef.
- Y. Sasaki, V. Jampani, C. Tanaka, N. Sakurai, S. Sakane, K. V. Le, F. Araoka and H. Orihara, Nat. Commun., 2016, 7, 13238 CrossRef CAS PubMed.
- A. Rapini, J. Phys., 1973, 34, 629–633 CrossRef.
- A. Rapini, L. Léger and A. Martinet, J. Phys. Colloq., 1975, 36, C1-189–C1-196 CrossRef.
- E. Brasselet and C. Loussert, Opt. Lett., 2011, 36, 719 CrossRef PubMed.
- E. Brasselet, Phys. Rev. Lett., 2012, 108, 087801 CrossRef PubMed.
- P. Salamon, N. Éber, Y. Sasaki, H. Orihara, Á. Buka and F. Araoka, Phys. Rev. Appl., 2018, 10, 044008 CrossRef.
- P. Oswald, G. Poy and A. Dequidt, Liq. Cryst., 2017, 44, 969–988 CrossRef CAS.
- S. Dhara, J. K. Kim, S. M. Jeong, R. Kogo, F. Araoka, K. Ishikawa and H. Takezoe, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2009, 79, 060701(R) CrossRef PubMed.
- Y. Sasaki, M. Ueda, K. V. Le, R. Amano, S. Sakane, S. Fujii, F. Araoka and H. Orihara, Adv. Mater., 2017, 29, 1703054 CrossRef PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c8ra08557a |
| This journal is © The Royal Society of Chemistry 2018 |