Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Dynamics of recombination via conical intersection in a semiconductor nanocrystal†
Wei-Tao
Peng
a,
B. Scott
Fales
 bc,
Yinan
Shu
bc,
Yinan
Shu
 d and
Benjamin G.
Levine
d and
Benjamin G.
Levine
 *a
*a
aDepartment of Chemistry, Michigan State University, East Lansing, MI 48824, USA. E-mail: levine@chemistry.msu.edu
bDepartment of Chemistry, The PULSE Institute, Stanford University, Stanford, CA 94305, USA
cSLAC National Accelerator Laboratory, Menlo Park, CA 94025, USA
dDepartment of Chemistry, University of Minnesota, Minneapolis, MN 55455, USA
First published on 13th November 2017
Abstract
Conical intersections are well known to introduce nonradiative decay pathways in molecules, but have only recently been implicated in nonradiative recombination processes in materials. Here we apply excited state ab initio molecular dynamics simulations based on a multireference description of the electronic structure to defective silicon nanocrystals up to 1.7 nm in diameter to search for accessible nonradiative recombination pathways. Dangling bond defects are found to induce conical intersections between the ground and first excited electronic states of five systems of various sizes. These defect-induced conical intersections are accessible at energies that are in the visible range (2.4–2.7 eV) and very weakly dependent on particle size. The dynamic simulations suggest that these intersections are accessed 40–60 fs after creation of a defect-localized excitation. This ultrafast recombination is attributed to the fact that Jahn–Teller distortion on the first excited state drives the defect directly towards a conical intersection with the ground electronic state.
Introduction
Conical intersections (CIs) are points of degeneracy between the potential energy surfaces (PESs) of two or more adiabatic electronic states.1–6 It is now well established that molecules that undergo efficient, ultrafast nonradiative transitions between electronic states of the same spin often do so by passing through CIs connecting those states. CIs can therefore be thought of in analogy to transition states; transition states are representative of paths that connect reactant and product wells on the same PES, whereas CIs are representative of nonradiative pathways connecting different electronic states. Theoretical predictions of CIs have provided insights into many important photochemical phenomena, e.g. photoisomerization,4,7 photodissociation,8–10 vision,11,12 and nonradiative decay of nucleic acids.13,14 Identification of CIs is now a routine part of the computational molecular photochemistry toolbox.15 However, only recently and through the use of advanced computing technology16 has the role that CIs play in the nonradiative recombination of excitations in semiconductors come to light.17,18Here we investigate the role of CIs in the photophysics of silicon nanocrystals (SiNCs) with dangling bond defects. SiNCs and other low-dimensional silicon systems have received intense attention due to their unique photophysical properties. Unlike bulk silicon, which has an indirect band gap, low-dimensional silicon materials can efficiently emit visible light19 with a wavelength that can be tuned via quantum confinement20–23 or surface modification.24–27 This tunable emission enables their application in optoelectronic devices,28–30 biological imaging,31 and silicon lasers.32,33 Under ambient conditions, however, SiNCs are prone to oxidize quickly upon exposure to O2 and/or H2O. Oxidation generates various defects on the surfaces of SiNCs, including dangling bonds (DBs) and some silicon oxide species (Si–O–Si bridges and Si–OH). Among them, silicon dangling bond defects have been studied extensively.34–38 In general, there are two common types of dangling bond defects: Pb centers, which are dangling bonds on a three-coordinated silicon atom on the surface, and D centers, which are DB defects located in amorphous silicon.39 DB defects have been known to degrade the performance of silicon-based devices for both photovoltaic40,41 and light emission38,42–44 applications.
Thus, it is well-known that silicon DB defects are nonradiative centers in SiNCs. It is also established that electronic movement at DB centers is strongly coupled to local vibrational motions.45,46 The widely accepted mechanism for recombination involves the sequential capture of electron and hole into the non-bonding orbital of the Pb center. Each change in the oxidation state of the Pb center is accompanied by nuclear relaxation along a bending mode that maintains local C3v symmetry; reduction results in the defect silicon atom taking on a less planar structure (i.e. less sp2-like) while oxidation results in a more planar structure. The line of thinking that yields this mechanism arises from the assumption that each change in charge state is instantaneous, however. It neglects (a) the fact that electrons and holes are strongly confined in SiNCs and therefore may interact strongly with one another and with the defect site before localization to the Pb center, and (b) the interaction of electron and hole with the defect is not instantaneous and may, instead, involve complex electron-nuclear dynamics. The CI theory of recombination considers these interactions and dynamics explicitly, therefore it would be instructive to reinvestigate this recombination process from a CI point of view.
In this work we (a) investigate whether nonradiative dynamics at Pb centers can be attributed to CIs between the ground and first excited electronic states, and (b) inform our fundamental physical intuition for recombination processes in general by analysis of the CIs associated with the dangling bond defect. To these ends we will bring to bear novel ab initio molecular dynamics (AIMD) tools capable of modeling the dynamics of electronically excited SiNCs as they approach CIs with the ground electronic state. In AIMD simulations, the nuclear dynamics are computed on PESs that are solved on the fly via electronic structure calculations. AIMD has recently become a tool of choice for the theoretical study of the photophysics of nanomaterials when either direct knowledge of excited state dynamics or extensive thermodynamic sampling are required, shedding light on various aspects of the charge carrier dynamics of SiNCs.47–56 The current study is the first to apply an AIMD approach based on a multireference description of the electronic structure to a true nanocrystal (diameter 1.7 nm). The advantage of multireference electronic structure approaches such as the complete active space configuration interaction (CASCI) approach used here57,58 is that they can accurately describe the PES in the vicinity of CIs between the ground and first excited electronic states. This is in contrast to single reference electronic structure methods—such as time-dependent density functional theory—which cannot accurately describe the potential energy surface near CIs involving the ground electronic state.59 The below study illustrates how applying CASCI-AIMD to model the dynamics of a semiconductor nanocluster from excitation to the neighborhood of a conical intersection can inform our fundamental understanding of nonradiative recombination.
Results and discussion
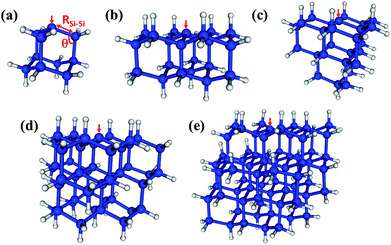
The methodological and computational specifics of our CASCI-AIMD simulations are presented in ESI,† but here we outline our study. We have performed a single CASCI-AIMD simulation for each of a series of five silicon clusters. Each cluster has a single Pb defect on the surface. The clusters (pictured in Fig. 1) range in size from a single sila-adamatane unit (Si10H15) to a 1.7 nm particle (Si72H63). All simulations were run on the first excited electronic PES starting from a structure in the Franck–Condon region. (Structures are presented in ESI.†) All surface silicon atoms aside from the defect site were capped with hydrogen atoms. We emphasize that these simulations are performed in the Born–Oppenheimer approximation.Geometries of near-zero energy gap were drawn from the AIMD trajectories and the minimal energy CIs (MECIs; the local minima on the CI seam) were optimized. Because CASCI lacks dynamic electron correlation, complete active space second-order perturbation theory (CASPT2) calculations60 were performed on the smallest cluster to estimate errors in our CASCI energies. Static CASCI and CASCI-AIMD calculations were performed in the TeraChem software package,16,61–63 which enables these demanding calculations through the use of graphics processing units—high performance computer processors designed for graphical applications such as video games. CASPT2 calculations were performed in MolPro,64–68 coupled cluster calculations were performed in GAMESS,69–71 and conical intersection optimizations were performed with CIOpt.72 Through this work we prefer adiabatic state labels: D0 and D1 to indicate the ground and first excited spin doublet electronic states of the clusters, respectively. However, when useful and appropriate we also include term symbols 2A1 and 2E to reflect the approximate symmetry of the states with respect to the local C3v symmetry of the defect site.
The D0 (2A1) minimum energy structures of our five clusters are presented in Fig. 1. Bond lengths and bond angles around the Pb defect (as indicated in Fig. 1a) at these structures are listed in the Table 1, labeled FC (Franck–Condon point). In these initial structures the three Si–Si bond lengths surrounding the DB and the associated bond angles are symmetric. The vertical excitation energies (Table 1) of the five clusters are very similar to one another, varying by only 0.3 eV, and there is not a strong trend with system size. This absence of quantum confinement effects suggests the locality of the excitation. This locality can be seen in the orbitals involved in the excitation (pictured in Fig. 2). The excitation occurs from the highest doubly occupied molecular orbital (HDOMO) to the singly occupied molecular orbital (SOMO). The HDOMO is a Si–Si σ bonding orbital (σSi–Si; e), which is relatively local to the region of the defect, while the SOMO is the dangling bond itself: the nonbonding (n; a1) sp3 orbital of the defect silicon atom. Note that there is a nearly degenerate pair of HDOMOs due to the local C3v symmetry of the defect. We present only one of the two degenerate σSi–Si orbitals in Fig. 2.
| FC | MECI | |||||
|---|---|---|---|---|---|---|
| R Si–Si/Å | θ/degree | D1 energy/eV | R Si–Si/Å | θ/degree | D1 energy/eV | |
| Si10H15 | 2.34 | 107.2 | 4.23 (3.53) | 2.43 | 123.8 | 2.67 (2.69) |
| 2.34 | 107.2 | 2.45 | 123.7 | |||
| 2.34 | 107.2 | 2.94 | 124.0 | |||
| Si22H27 | 2.36 | 105.2 | 4.25 | 2.46 | 122.6 | 2.52 |
| 2.36 | 105.2 | 2.46 | 121.9 | |||
| 2.36 | 105.2 | 2.89 | 123.5 | |||
| Si26H31 | 2.36 | 104.9 | 4.29 | 2.43 | 122.0 | 2.52 |
| 2.36 | 104.9 | 2.51 | 123.2 | |||
| 2.36 | 104.9 | 2.83 | 123.6 | |||
| Si47H49 | 2.36 | 105.6 | 3.99 | 2.43 | 122.4 | 2.44 |
| 2.36 | 105.6 | 2.54 | 123.6 | |||
| 2.36 | 105.6 | 2.81 | 123.6 | |||
| Si72H63 | 2.35 | 105.7 | 4.00 | 2.44 | 122.4 | 2.38 |
| 2.35 | 105.7 | 2.48 | 122.9 | |||
| 2.35 | 105.7 | 2.82 | 124.3 | |||
Now we consider the results of the excited state AIMD simulations initiated on the D1 (2E; σSi–Si → n) state. This is the lowest electronic state with nonzero transition dipole moment. Note that D1 and D2 are degenerate by symmetry, as will be discussed below, and D3 is considerably higher in energy (0.9 eV above D2 in the sila-adamantane cluster). The time-dependent D1 and D0 potential energies, Si–Si bond lengths (RSi–Si as defined in Fig. 1a), and bond angles (θ as illustrated in Fig. 1a) of the 1.7 nm SiNC are presented in Fig. 3. The dynamics of the smaller clusters were nearly identical; similar graphs for these cases are reported in Fig. S1–S12.† In all five cases the D1/D0 energy gap approaches zero (<0.1 eV) in the first 40–60 fs after excitation (Fig. 3a). The vanishing energy gaps strongly suggest the existence of low-lying D1/D0 CIs.73
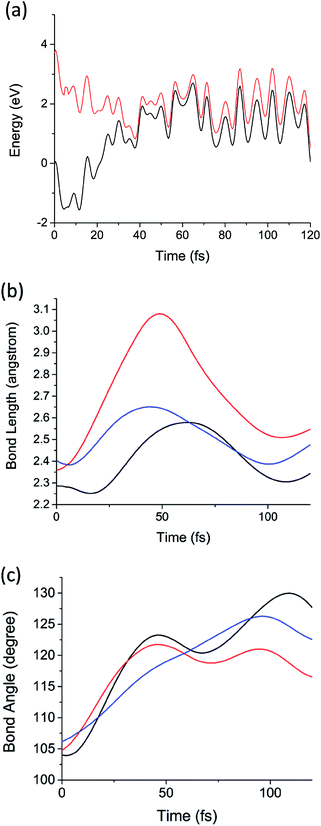 | ||
| Fig. 3 (a) The potential energies of the D1 (red) and D0 (black) electronic states as a function of time from the D1 AIMD simulation of Si72H63. (b) The three Si–Si bond lengths (RSi–Si) adjacent to the Pb defect as a function of time from the same AIMD calculation. (c) The three Si–Si–Si angles (θ, illustrated in Fig. 1a) as a function of time from the same AIMD calculation. Each color represents one of the three symmetry equivalent bond lengths or angles in (b) and (c), respectively. | ||
Using low-gap structures from the AIMD trajectories as starting guesses, MECIs were optimized in all five systems. The energies and structural details of these MECIs are reported in Table 1. Full structures are presented in ESI.† Comparing the structures of MECIs to the Franck–Condon points, one can see that both bond lengths and bond angles around the DB defects increase at the MECIs. The three Si–Si bonds surrounding the DB are asymmetrically stretched in all five clusters; one Si–Si bond grows longer (2.81–2.94 angstrom) than the other two (2.43–2.54 angstrom). Similar asymmetric stretching is observed in the AIMD simulations of all five systems (Fig. 3b and S5–S8†) on the same 40–60 fs time scale on which the D1/D0 energy gap approaches zero. Much smaller changes in bond length are observed for Si–Si bonds not immediately adjacent to the Pb defect. Consistent with past work on dangling bond defects,45 symmetric bending motion is also observed to be important; the θ angles are observed to increase significantly both in the AIMD simulations (Fig. 3c and S9–S12†) and in the optimized MECI structures (Table 1). Taken together, these calculations suggest that upon excitation of the lowest defect-localized excited state, the Pb defect moves ballistically to the CI region in 40–60 fs. It is also noteworthy that the trajectories remain in a region of small energy gap after 40–60 fs, suggesting that it may pass over the intersection multiple times, enabling efficient decay.
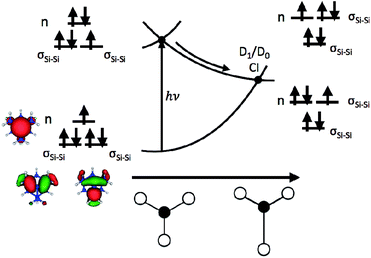
The role that symmetry breaking plays in these nonradiative dynamics can be intuitively understood through analysis of the orbitals occupied during excitation. Fig. 4 is a schematic diagram summarizing these dynamics. As described above, excitation to D1 involves the promotion of an electron from one of the degenerate σSi–Si orbitals to the n orbital. This reduces the Si–Si bond order, resulting in a lengthening of one of the Si–Si bonds (as observed in the AIMD simulations of all five clusters). In the locally C3v-symmetric FC structure the D1 (2E) state is doubly degenerate by symmetry, thus this symmetry-breaking motion is a Jahn–Teller distortion. The lengthening of a single Si–Si bond (moving from left to right along the D1 PES in Fig. 4) brings the molecule towards the MECI structure. This symmetry breaking raises the energy of one of the σSi–Si orbitals into near degeneracy with the n orbital, bringing about a CI between the D0 and D1 states. That a Jahn–Teller distortion in D1 drives the molecules directly towards the D1/D0 CI provides a straightforward explanation for the ultrafast nonradiative process that follows creation of the defect-localized excitation.
The MECIs of the defective silicon clusters studied here are all accessible at energies in the visible range (2.38–2.67 eV above the ground state minimum structure; Table 1) and therefore capable of quenching visible light emission. The MECIs show a slight decrease in energy with increasing system size; the energy decreases from 2.67 eV for the small sila-adamantane cluster to 2.38 eV for the 1.7 nm SiNC. This small energy decrease of 0.29 eV is consistent with the localized nature of the defect-localized excited state. Calculations at the dynamically correlated CASPT2 level confirm the accuracy of the MECI energies predicted by CASCI, though vertical excitation energies are somewhat overestimated. All five clusters have D1 minimum energy structures distinct from the MECIs. In all cases this minimum is 0.05–0.06 eV below the MECI, compared to the 1.6–1.9 eV released during relaxation on the excited states. Thus the MECI is energetically accessible upon excitation. Energies and structures of D1 minima are presented in ESI.†
The existence of defect-induced CIs with energies in the 2.4–2.7 eV range is consistent with several experimental observations of the photoluminescence (PL) of SiNCs after oxidation. The MECI energies suggest that the PL of SiNCs with emission energies larger than ∼2.4–2.7 eV is likely to be quenched by the DB defects. Indeed the quantum yield of PL from oxidized SiNCs is observed to drop with increasing energy,74 and single particle experiments on oxidized SiNCs show no emissive particles with PL maxima above 2.5 eV.75 In addition, the PL lifetime of oxidized SiNCs decreases with increasing PL energy, and a dramatic decrease is observed in the 2.0–2.2 eV energy range.76 This decreasing PL lifetime suggests the existence of an efficient nonradiative recombination pathway accessible above these energies, consistent with our computed CI energies. Finally, as noted in our previous studies of oxygen-containing defects, the unusual size-insensitive orange (S-band) emission of oxidized SiNCs observed in ensemble PL measurements77 is consistent with the presence of CIs accessible in this energy range. We argue that the size-insensitivity of the observed ensemble emission arises not because the emission energy of individual oxidized SiNCs becomes insensitive to particle size, but instead because the rate of nonradiative recombination is strongly size sensitive, dramatically reducing the PL yields of smaller SiNCs with shorter wavelengths. This argument reconciles the observation of size-insensitive emission with PL lifetime, linewidth, and polarization measurements suggesting that the S-band arises from quantum-confined excitons.75,78,79
Conclusions
Thus, we have elucidated the mechanism of nonradiative recombination via a dangling bond defect by application of AIMD simulations based on a multireference description of the electronic structure to SiNCs up to 1.7 nm in diameter. Within 40–60 fs after excitation of a defect-localized electronic excited state, a CI between the D0 and D1 states is accessed. This CI is accessible at energies in the 2.4–2.7 eV range, and thus is detrimental to visible PL, consistent with the fact that DBs are well-known nonradiative centers. The ultrafast recombination process is driven both by Jahn–Teller distortion in the D1 state and by totally symmetric bending of the DB center. The role that symmetry-breaking plays in this mechanism underlines the importance of treating coupled electron-nuclear dynamics in the study of recombination.Conflicts of interest
There are no conflicts to declare.Acknowledgements
We gratefully acknowledge support from the National Science Foundation under grant CHE-1565634. We acknowledge fruitful discussion with Rémi Beaulac.References
- J. Michl and V. Bonacic-Koutecky, Electronic Aspects of Organic Photochemistry, Wiley, New York, 1990 Search PubMed.
- D. R. Yarkony, Rev. Mod. Phys., 1996, 68, 985–1013 CrossRef CAS.
- F. Bernardi, M. Olivucci and M. A. Robb, Chem. Soc. Rev., 1996, 25, 321–328 RSC.
- B. G. Levine and T. J. Martinez, Annu. Rev. Phys. Chem., 2007, 58, 613–634 CrossRef CAS PubMed.
- S. Matsika and P. Krause, Annu. Rev. Phys. Chem., 2011, 62, 621–643 CrossRef CAS PubMed.
- D. R. Yarkony, Chem. Rev., 2012, 112, 481–498 CrossRef CAS PubMed.
- R. Gonzalez-Luque, M. Garavelli, F. Bernardi, M. Merchan, M. A. Robb and M. Olivucci, Proc. Natl. Acad. Sci. U. S. A., 2000, 97, 9379–9384 CrossRef CAS.
- M. L. Hause, Y. H. Yoon, A. S. Case and F. F. Crim, J. Chem. Phys., 2008, 128, 104307–104309 CrossRef PubMed.
- K. R. Yang, X. F. Xu, J. J. Zheng and D. G. Truhlar, Chem. Sci., 2014, 5, 4661–4680 RSC.
- X. L. Zhu and D. R. Yarkony, J. Chem. Phys., 2014, 140, 21 Search PubMed.
- D. Polli, P. Altoe, O. Weingart, K. M. Spillane, C. Manzoni, D. Brida, G. Tomasello, G. Orlandi, P. Kukura, R. A. Mathies, M. Garavelli and G. Cerullo, Nature, 2010, 467, 440–U488 CrossRef CAS PubMed.
- M. Liebel, C. Schnedermann and P. Kukura, Phys. Rev. Lett., 2014, 112, 198302 CrossRef CAS PubMed.
- S. Matsika, J. Phys. Chem. A, 2004, 108, 7584–7590 CrossRef CAS.
- M. Merchan and L. Serrano-Andres, J. Am. Chem. Soc., 2003, 125, 8108–8109 CrossRef CAS PubMed.
- I. Schapiro, F. Melaccio, E. N. Laricheva and M. Olivucci, Photochem. Photobiol. Sci., 2011, 10, 867–886 CAS.
- B. S. Fales and B. G. Levine, J. Chem. Theory Comput., 2015, 11, 4708–4716 CrossRef CAS PubMed.
- Y. Shu, B. S. Fales and B. G. Levine, Nano Lett., 2015, 15, 6247–6253 CrossRef CAS PubMed.
- Y. Shu, B. S. Fales, W.-T. Peng and B. G. Levine, J. Phys. Chem. Lett., 2017, 8, 4091–4099 CrossRef CAS PubMed.
- L. T. Canham, Appl. Phys. Lett., 1990, 57, 1046–1048 CrossRef CAS.
- V. Kocevski, O. Eriksson and J. Rusz, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87, 245401 CrossRef.
- M. L. Mastronardi, F. Hennrich, E. J. Henderson, F. Maier-Flaig, C. Blum, J. Reichenbach, U. Lemmer, C. Kubel, D. Wang, M. M. Kappes and G. A. Ozin, J. Am. Chem. Soc., 2011, 133, 11928–11931 CrossRef CAS PubMed.
- B. Delley and E. F. Steigmeier, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 47, 1397–1400 CrossRef CAS.
- S. Furukawa and T. Miyasato, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 38, 5726–5729 CrossRef CAS.
- Z. Y. Zhou, L. Brus and R. Friesner, Nano Lett., 2003, 3, 163–167 CrossRef CAS.
- D. Konig, J. Rudd, M. A. Green and G. Conibeer, Sol. Energy Mater. Sol. Cells, 2009, 93, 753–758 CrossRef.
- M. Dasog, G. B. De los Reyes, L. V. Titova, F. A. Hegmann and J. G. C. Veinot, ACS Nano, 2014, 8, 9636–9648 CrossRef CAS PubMed.
- H. S. Li, Z. G. Wu, T. L. Zhou, A. Sellinger and M. T. Lusk, Phys. Chem. Chem. Phys., 2014, 16, 19275–19281 RSC.
- B. Gelloz and N. Koshida, J. Appl. Phys., 2000, 88, 4319–4324 CrossRef CAS.
- C. Y. Liu, Z. C. Holman and U. R. Kortshagen, Nano Lett., 2009, 9, 449–452 CrossRef CAS PubMed.
- J. Valenta, N. Lalic and J. Linnros, Opt. Mater., 2001, 17, 45–50 CrossRef CAS.
- J. H. Park, L. Gu, G. von Maltzahn, E. Ruoslahti, S. N. Bhatia and M. J. Sailor, Nat. Mater., 2009, 8, 331–336 CrossRef CAS PubMed.
- L. Pavesi, L. Dal Negro, C. Mazzoleni, G. Franzo and F. Priolo, Nature, 2000, 408, 440–444 CrossRef CAS PubMed.
- D. Liang and J. E. Bowers, Nat. Photonics, 2010, 4, 511–517 CrossRef CAS.
- M. A. Tischler, R. T. Collins, J. H. Stathis and J. C. Tsang, Appl. Phys. Lett., 1992, 60, 639–641 CrossRef CAS.
- C. Delerue, G. Allan and M. Lannoo, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 48, 11024–11036 CrossRef CAS.
- M. Schoisswohl, J. L. Cantin, H. J. Vonbardeleben and G. Amato, Appl. Phys. Lett., 1995, 66, 3660–3662 CrossRef CAS.
- R. N. Pereira, D. J. Rowe, R. J. Anthony and U. Kortshagen, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 83, 155327 CrossRef.
- N. P. Brawand, M. Voros and G. Galli, Nanoscale, 2015, 7, 3737–3744 RSC.
- E. H. Poindexter, P. J. Caplan, B. E. Deal and R. R. Razouk, J. Appl. Phys., 1981, 52, 879–884 CrossRef CAS.
- S. Niesar, A. R. Stegner, R. N. Pereira, M. Hoeb, H. Wiggers, M. S. Brandt and M. Stutzmann, Appl. Phys. Lett., 2010, 96, 193112 CrossRef.
- H. S. Li, Z. G. Wu and M. T. Lusk, J. Phys. Chem. C, 2014, 118, 46–53 CAS.
- B. K. Meyer, V. Petrovakoch, T. Muschik, H. Linke, P. Omling and V. Lehmann, Appl. Phys. Lett., 1993, 63, 1930–1932 CrossRef CAS.
- B. G. Fernandez, M. Lopez, C. Garcia, A. Perez-Rodriguez, J. R. Morante, C. Bonafos, M. Carrada and A. Claverie, J. Appl. Phys., 2002, 91, 798–807 CrossRef.
- X. D. Pi, L. Mangolini, S. A. Campbell and U. Kortshagen, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 75, 177 CrossRef.
- C. Delerue and M. Lannoo, Nanostructure: Theory and Modelling, Springer-Verlag, Berlin, 2004 Search PubMed.
- M. Lannoo, C. Delerue and G. Allan, J. Lumin., 1993, 57, 243–247 CrossRef CAS.
- J. C. Chen, A. Schmitz, T. Inerbaev, Q. G. Meng, S. Kilina, S. Tretiak and D. S. Kilin, J. Phys. Chem. Lett., 2013, 4, 2906–2913 CrossRef CAS.
- J. Liu, A. J. Neukirch and O. V. Prezhdo, J. Chem. Phys., 2013, 139, 164303 CrossRef PubMed.
- Y. Shu and B. G. Levine, J. Chem. Phys., 2013, 139, 081102 CrossRef PubMed.
- J. Liu, A. J. Neukirch and O. V. Prezhdo, J. Phys. Chem. C, 2014, 118, 20702–20709 CAS.
- Y. Shu and B. G. Levine, J. Phys. Chem. C, 2014, 118, 7669–7677 CAS.
- Y. Han, D. A. Micha and D. S. Kilin, Mol. Phys., 2015, 113, 327–335 CrossRef CAS.
- K. G. Reeves, A. Schleife, A. A. Correa and Y. Kanai, Nano Lett., 2015, 15, 6429–6433 CrossRef CAS PubMed.
- Y. Shu and B. G. Levine, J. Phys. Chem. C, 2015, 119, 1737–1747 CAS.
- Y. Shu and B. G. Levine, J. Phys. Chem. C, 2016, 120, 23246–23253 CAS.
- N. P. Brawand, M. B. Goldey, M. Voros and G. Galli, Chem. Mater., 2017, 29, 1255–1262 CrossRef CAS.
- G. Granucci, M. Persico and A. Toniolo, J. Chem. Phys., 2001, 114, 10608–10615 CrossRef CAS.
- P. Slavicek and T. J. Martinez, J. Chem. Phys., 2010, 132, 234102 CrossRef PubMed.
- B. G. Levine, C. Ko, J. Quenneville and T. J. Martinez, Mol. Phys., 2006, 104, 1039–1051 CrossRef CAS.
- J. Finley, P. A. Malmqvist, B. O. Roos and L. Serrano-Andres, Chem. Phys. Lett., 1998, 288, 299–306 CrossRef CAS.
- I. S. Ufimtsev and T. J. Martinez, J. Chem. Theory Comput., 2009, 5, 2619–2628 CrossRef CAS PubMed.
- E. G. Hohenstein, M. E. F. Bouduban, C. C. Song, N. Luehr, I. S. Ufimtsev and T. J. Martinez, J. Chem. Phys., 2015, 143, 014111 CrossRef PubMed.
- C. C. Song, L. P. Wang and T. J. Martinez, J. Chem. Theory Comput., 2016, 12, 92–106 CrossRef CAS PubMed.
- P. J. Knowles and H. J. Werner, Chem. Phys. Lett., 1985, 115, 259–267 CrossRef CAS.
- H.-J. Werner and P. J. Knowles, J. Chem. Phys., 1985, 82, 5053–5063 CrossRef CAS.
- H. J. Werner, Mol. Phys., 1996, 89, 645–661 CrossRef CAS.
- P. Celani and H. J. Werner, J. Chem. Phys., 2000, 112, 5546–5557 CrossRef CAS.
- H. J. Werner, P. J. Knowles, G. Knizia, F. R. Manby and M. Schutz, Wires Comput. Mol. Sci., 2012, 2, 242–253 CrossRef CAS.
- M. W. Schmidt, K. K. Baldridge, J. A. Boatz, S. T. Elbert, M. S. Gordon, J. H. Jensen, S. Koseki, N. Matsunaga, K. A. Nguyen, S. Su, T. L. Windus, M. Dupuis and J. A. Montgomery, J. Comput. Chem., 1993, 14, 1347–1363 CrossRef CAS.
- J. R. Gour, P. Piecuch and M. Wloch, J. Chem. Phys., 2005, 123, 134113 CrossRef PubMed.
- J. R. Gour and P. Piecuch, J. Chem. Phys., 2006, 125, 234107 CrossRef PubMed.
- B. G. Levine, J. D. Coe and T. J. Martinez, J. Phys. Chem. B, 2008, 112, 405–413 CrossRef CAS PubMed.
- D. G. Truhlar and C. A. Mead, Phys. Rev. A: At., Mol., Opt. Phys., 2003, 68, 032501 CrossRef.
- D. Jurbergs, E. Rogojina, L. Mangolini and U. Kortshagen, Appl. Phys. Lett., 2006, 88, 233116 CrossRef.
- T. Schmidt, A. I. Chizhik, A. M. Chizhik, K. Potrick, A. J. Meixner and F. Huisken, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 125302 CrossRef.
- F. Huisken, G. Ledoux, O. Guillois and C. Reynaud, Adv. Mater., 2002, 14, 1861–1865 CrossRef CAS.
- M. V. Wolkin, J. Jorne, P. M. Fauchet, G. Allan and C. Delerue, Phys. Rev. Lett., 1999, 82, 197–200 CrossRef CAS.
- C. Garcia, B. Garrido, P. Pellegrino, R. Ferre, J. A. Moreno, J. R. Morante, L. Pavesi and M. Cazzanelli, Appl. Phys. Lett., 2003, 82, 1595–1597 CrossRef CAS.
- I. Sychugov, R. Juhasz, J. Valenta and J. Linnros, Phys. Rev. Lett., 2005, 94, 087405 CrossRef PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c7sc04221c |
| This journal is © The Royal Society of Chemistry 2018 |