Structural evolution of LiN_n⁺ (n = 2, 4, 6, 8, and 10) clusters: mass spectrometry and theoretical calculations

Abstract

Mixed nitrogen-lithium cluster cations LiN_n⁺ were generated by laser vaporization and analyzed by time-of-flight mass spectrometry. It is found that LiN₈⁺ has the highest ion abundance among the LiN_n⁺ ions in the mass spectrum. Density functional calculations were conducted to search for the stable structures of the Li–N clusters. The theoretical results show that the most stable isomers of LiN_n⁺ clusters are in the form of Li⁺(N₂)_n/2, and the order of their calculated binding energies is consistent with that of Li–N₂ bond lengths. The most stable structures of LiN_n⁺ evolve from one-dimensional linear type (C_∞v, n = 2; D_∞h, n = 4), to two-dimensional branch type (D_3h, n = 6), then to three-dimensional tetrahedral (T_d, n = 8) and square pyramid (C_4v, n = 10) types. Further natural bond orbital analyses show that electrons are transferred from the lone pair on N_α of every N₂ unit to the empty orbitals of lithium atom in LiN_2–8⁺, while in LiN₁₀⁺, electrons are transferred from the bonding orbital of the Li–N_α bonds to the antibonding orbital of the other Li–N_α bonds. In both cases, the N₂ units become dipoles and strongly interact with Li⁺. The average second-order perturbation stabilization energy for LiN₈⁺ is the highest among the observed LiN_n⁺ clusters. For neutral LiN_2–8 clusters, the most stable isomers were also formed by a Li atom and n/2 number of N₂ units, while that of LiN₁₀ is in the form of Li⁺(N₂)₃(η¹-N₄).

---

1 Introduction

Nitrogen clusters have been predicted to be potential high energy density materials (HEDM)^1,2 by numerous theoretical^3,4 studies. Since the identification of N₅⁺, N₄, and N₅⁻,^5–7 the search for new polynitrogen species by a variety of experimental techniques has received intense attention. However, only a few efforts have been successful due to the low stability of these clusters. Metal doped nitrogen clusters MN_n have advantages in designing new polynitrogen structural groups because the metal–nitrogen interactions may be able to stabilize polynitrogen groups in MN_n with respect to the corresponding isolated N_n species.⁸ Many novel polynitrogen structural groups such as cyclic N₄,^9–12 N₅,¹³ N₆,¹⁴ and N₇ (ref. 15) have been predicted to exist in this form. Thus, it is interesting to generate metal-doped nitrogen clusters, gradually increase their nitrogen content and study their structural characteristics.

To date, extensive experimental works have been undertaken to investigate mixed nitrogen–metal clusters. Numerous homoleptic azides of the type M(N₃)_n (M = Ti, Nb, Ta, Mo, W, etc.)^16–27 and their derived salts have been prepared and characterized to understand the properties of highly energetic materials. Nb⁺(N₂)_n and V⁺(N₂)_n complexes were investigated using photodissociation spectroscopy.^28,29 Rh(N₂)₄⁺ complexes were studied by infrared laser photodissociation spectroscopy and theoretical calculations.³⁰ TiN₁₂⁺ was generated by laser ablation, and the most stable structure was found to be Ti(N₂)₆⁺ with O_h symmetry.³¹ The VN_n⁺ (n = 8, 9, and 10) clusters were generated and their most stable isomers were found to be in the form of V(N₂)₄⁺, (η²-N₄)V⁺N(N₂)₂ and (η⁴-N₄)V⁺(N₂)₃, respectively.³²

Lithium is the lightest metal. It is highly reactive and can react with nitrogen gas to form lithium nitride.³³ In addition, lithium atoms have been successfully used as dopants in many clusters, such as silicon,³⁴ germanium,³⁵ boron³⁶ and aluminum³⁷ clusters, to tailor their structural and electronic properties. Although the experimental studies on lithium-doped nitrogen clusters were very scarce, these clusters have been investigated in preliminary studies by many theoretical calculations. Cheng and Li predicted that lithium and N₄²⁻ ring could form bipyramidal Li₂N₄ structures³⁸ with significant barriers for isomerization and dissociation. Glukhovtsev and Schleyer studied the structure and stability of N₅Li and found that N₅Li favours a planar C_2v structure.³⁹ Investigation of lithium–nitrogen clusters may provide useful information for understanding the lithium–nitrogen interactions and for the design of high nitrogen content species.

In this work, we investigated lithium–nitrogen binary clusters by laser ablation experiments coupled with theoretical calculations to gain insights into the geometric structures and electronic properties of LiN_n⁺ and their neutral counterparts.

2 Experimental and theoretical methods

2.1 Experimental method

The experiments were conducted using a home-built apparatus equipped with a laser vaporization supersonic cluster source and a reflectron time-of-flight mass spectrometer (RTOF-MS) that has been described elsewhere.⁴⁰ The laser vaporization source was cooled by liquid nitrogen. The disk targets made of LiCl, LiF, LiF/ZrN (mole ratio 4 : 1), LiF/AlN (mole ratio 2 : 1) or LiF/BN (mole ratio 2 : 1) were used in the experiments to provide Li⁺ ions. The LiN_n⁺ (n = 2, 4, 6, 8, and 10) cluster cations were generated in the laser vaporization source by laser ablation the rotating and translating disk targets in N₂ gas with the second harmonic of a nanosecond Nd:YAG laser (Continuum Surelite II-10). Nitrogen carrier gas with ∼4 atm backing pressure was allowed to expand through a pulsed valve (General Valve Series 9) into the source to provide nitrogen and to cool the formed clusters. The typical laser power used in this work was approximately 10 mJ per pulse. The generated cluster cations were mass-analysed by the RTOF-MS.

2.2 Theoretical methods

The geometry optimization and frequency calculations were performed with Gaussian 09 program package using density functional theory (DFT) at the M06-2X/6-311+G(d,p) level.^41–43 Every stationary point on the potential energy surface (PES) was confirmed to be local minimum-energy structure by all positive harmonic frequencies. The bond length of the N₂ molecule was calculated to verify the accuracy of the theoretical method. The calculated N–N bond length is about 1.090 Å, which is consistent with an experimental value of 1.097 Å.⁴⁴ The binding energies of nitrogen to Li^+/0 were calculated for each LiN_n^+/0 species. The binding energy is defined as:

E_b1 = 2[E(Li) + n/2E(N₂) − E(LiN_n)]/n (for the neutrals) (I)

E_b2 = 2[E(Li⁺) + n/2E(N₂) − E(LiN_n⁺)]/n (for the cations)

To get further insight into the interactions between N₂ molecule and Li^+/0, we performed natural bond orbital (NBO) analysis⁴⁵ where the electronic wave function is interpreted in terms of a set of occupied Lewis orbitals and a set of unoccupied non-Lewis delocalized orbitals. For each donor NBO (i) and acceptor NBO (j), the stabilization energy E₂ associated with charge transfer i → j is given by

E(2) = ΔE_ij = q_iF(i, j)²/(ε_i − ε_j) (II)

where q_i is the donor orbital occupancy, and ε_i and ε_j are diagonal elements (orbital energies) and F(i, j) is the off-diagonal NBO Fock matrix element.

The average E(2) of LiN_n⁺ is defined as:

3 Experimental results

Fig. 1 shows a typical mass spectrum of the clusters generated in the experiment with LiCl as the substrate and N₂ as the carrier gas. It is observed that the main series of lithium–nitrogen clusters are LiN₂⁺, LiN₄⁺, LiN₆⁺, LiN₈⁺ and LiN₁₀⁺. The mass intensity of LiN₈⁺ is predominant compared to those of the other LiN_n⁺ species. No mass peak of LiN_n⁺ with odd numbers of nitrogen atoms has been detected. In addition to the mass peaks of lithium–nitrogen clusters, the mass peaks of LiN₂(H₂O)⁺, LiN₄(H₂O)⁺, LiN₆(H₂O)⁺ and LiN₈(H₂O)⁺ are also observed. The formation of LiN_n(H₂O)⁺ more likely is due to the presence of trace amounts of water in the carrier gas. In laser ablation experiments with LiF, LiF/ZrN, LiF/AlN and LiF/BN as substrates, similar lithium–nitrogen clusters were obtained (ESI, Fig. S1–S4†).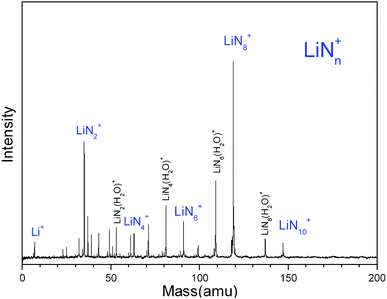

Fig. 1 Typical mass spectrum of Li–N clusters generated by laser ablation of a LiCl target with N₂ as the carrier gas.

4 Theoretical results

To search for the stable structures of LiN_n⁺ and LiN_n (n = 2, 4, 6, 8, and 10), we have conducted calculations for nine different categories of structures, from LiN₂ to LiN₁₀. Starting from the simplest one containing only two nitrogen atoms and a lithium atom, by adding one nitrogen atom at a time to the system, we obtained an increasing number of geometrical configurations as the system became larger. By placing the nitrogen atoms at different locations, we constructed the initial geometrical structures with a series of units such as N₂ molecules, N₃ chain and ring, N₄ chain and ring, and N₅, N₆, and N₇ rings and so on containing lithium atoms. To ensure that the geometry optimizations located the true minima on the potential surfaces, frequency calculations were conducted for all optimized structures. For each initial geometrical configuration, we also considered many spin multiplicities (2, 4, and 6 for neutral species and 1, 3, and 5 for positive ions). After full relaxation, a rather large number of low-lying isomers of LiN_n⁺ and LiN_n (n = 2, 4, 6, 8, and 10) were found. Here, we show the typical isomers of LiN_n⁺ (n = 2, 4, 6, 8, and 10) in Fig. 2 with the most stable structures on the left and those of LiN_n (n = 2, 4, 6, 8, and 10) in Fig. 3.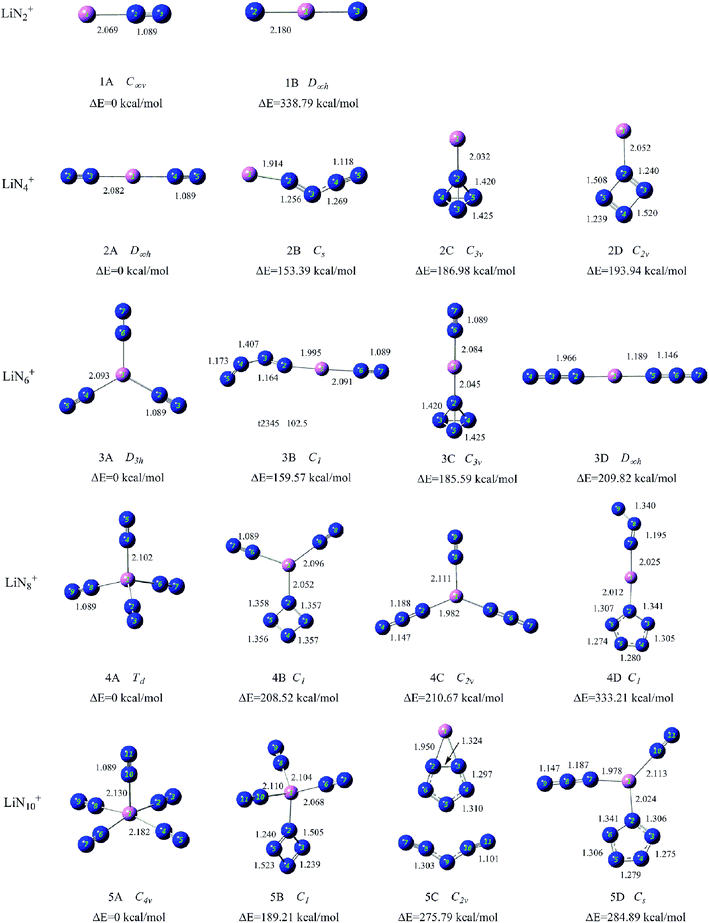

Fig. 2 Typical low-lying isomers of LiN_n⁺ (n = 2, 4, 6, 8, and 10) clusters. The bond lengths are given in angstroms.

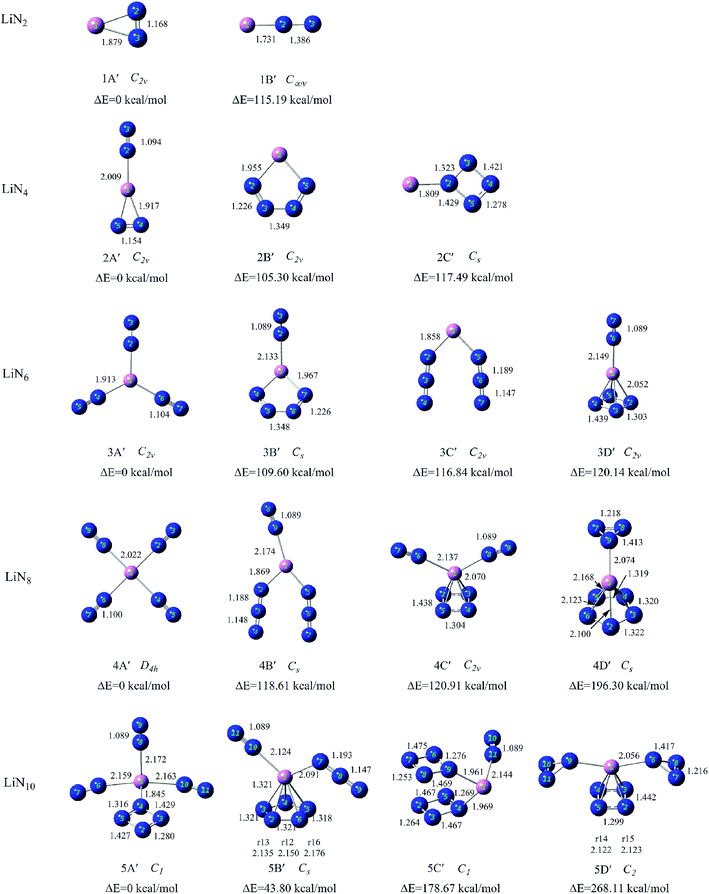Fig. 3 Structures and relative energies of the low-lying isomers of neutral LiN_n (n = 2, 4, 6, 8, and 10) clusters. The bond lengths are given in angstroms.

4.1 Structures of LiN₂⁺ and LiN₂

The ground state structure of LiN₂⁺ (1A) is a C_∞v symmetry linear structure formed by attaching an end-on N₂ molecule to a Li atom. The N–N bond lengths in the N₂ unit are 1.089 Å. The second isomer (1B) is a D_∞h symmetry linear structure formed by inserting a Li atom between two N atoms. It is much higher in energy than 1A.

For neutral LiN₂, the most stable isomer 1A′ has a C_2v symmetry triangular structure consisting of a side-on N₂ molecule and an Li atom. The N–N bond length in the side-on N₂ unit is 1.168 Å, which is longer than that of isolated N₂ molecule. The second stable structure 1B′ has one end-on N₂ unit and one Li atom, similar to 1A of LiN₂⁺. However, the N–N bond length in the end-on N₂ unit increases to 1.386 Å.

4.2 Structures of LiN₄⁺ and LiN₄

The ground state structure of LiN₄⁺ (2A) is a D_∞h symmetry linear structure, in which two N₂ molecules are attached directly to the central Li atom. The N–N bond lengths in the N₂ units are 1.089 Å. In the other low-lying isomers of LiN₄⁺ (2B–2D), N₄ units were found and they interact with Li atoms as a whole. For example, in isomers 2B, 2C and 2D, the N₄ units exhibit chain, tetrahedral, and cyclic configurations, respectively, and connect to Li atom via one nitrogen atom. These N₄ units have been reported in many previous studies and the interaction between Li⁺ and N₄ ring was also predicted by Li et al.^38,46–48

For neutral LiN₄, isomer 2A′ with one end-on and one side-on distances of the end-on and side-on N₂ units are 1.094 and 1.154 Å, respectively. A N₄ chain is found in isomer 2B′ and is connected to the Li atom through two terminal nitrogen atoms. Isomer 2C′ has a Li atom interacting with a η¹-N₄ ring similar to isomer 2D LiN₄⁺. We could not find any isomer with a tetrahedral N₄(T_d) unit in neutral LiN₄.

4.3 Structures of LiN₆⁺ and LiN₆

For LiN₆⁺, the lowest energy isomer (3A) has a planar structure with D_3h symmetry, in which three N₂ molecules interact equally with the central Li atom via their terminal nitrogen atoms. The N–N bond lengths in the N₂ units are 1.089 Å. The low-lying isomers containing other all-nitrogen units are also found and their energies are much higher than that of 3A. Isomer 3B has one end-on bound N₂ molecule and one N₄ chain coordinating to the Li atom via their terminal nitrogen atoms. The N₄ chain can be regarded as a complex of two N₂ sub-units because the bond linking the two N₂ sub-units is relatively weak. Isomer 3C contains one end-on bound N₂ molecule and one tetrahedral N₄ unit that coordinates to the Li atom through one vertex nitrogen atom. Isomer 3D is a D_∞h symmetry linear structure formed by attaching two N₃ ligands to the Li atom.

The ground state structure of LiN₆ (3A′) is similar to that of LiN₆⁺ (3A). It was also formed by attaching three N₂ molecules to the central Li atom, but its symmetry changes to C_2v. In the other low-lying isomers, N₃, N₄ and N₆ units are the main building blocks. Isomer 3B′ contains one end-on bound N₂ molecule and one N₄ chain that connects to the Li atom through its two terminal nitrogen atoms. Isomer 3C′ has two end-on N₃ units. Isomer 3D′ has one end-on N₂ molecule and one η⁴-N₄ ring.

4.4 Structures of LiN₈⁺ and LiN₈

The most stable structure of LiN₈⁺ (4A) is a T_d symmetry structure formed by attaching four end-on N₂ molecules to a Li atom. The N–N bond lengths in the N₂ units are 1.089 Å. The other low-lying isomers are much higher in energy than 4A. Isomer 4B has one η¹-N₄ ring and two end-on bound N₂ molecules. Isomer 4C contains two N₃ units and one end-on bound N₂ molecule. 4D has one η¹-N₅ ring and one N₃ unit. The planar structure Li-η¹-N₅ in 4D was also studied by Glukhovtsev and considered as a possible isomer of LiN₅.³⁹

For LiN₈, the lowest energy isomer (4A′) has a planar structure with D_4h symmetry in which four N₂ molecules interact equally with the central Li atom via their terminal nitrogen atoms. The N–N bond lengths in the N₂ units are 1.089 Å. Isomer 4B′ was formed by attaching two N₃ units and one end-on bound N₂ molecule to a Li atom. Isomer 4C′ has one η⁴-N₄ ring and two end-on bound N₂ molecules. Isomers 4B′ and 4C′ could be considered to evolve from isomers 3C′ and 3D′ by the addition of one end-on bound N₂ molecule. Isomer 4D′ has one η⁵-N₅ ring and one η¹-N₃ units. This pyramidal structure formed by N₅ ring and metal ions has been proposed in many theoretical studies.^39,49,50 [N₃MN₅]^q structure was also calculated by Jin et al., and their results suggested that the [N₃MN₅]^q in heterodecked form are thermodynamically more stable than the sandwich-like isomers [N₄MN₄]^q(D_4d) with even-membered N₄²⁻ ring [(M, q) = (Ni, 0), (Co, −1), (Fe, −2)].⁵¹

4.5 Structures of LiN₁₀⁺ and LiN₁₀

The most stable isomer of LiN₁₀⁺ (5A) consists of five N₂ molecules placed around the Li atom and exhibits C_4v symmetry. 5B is the second stable structure and has three N₂ molecules and one η¹-N₄ ring and its energy is much higher than that of 5A. The third isomer 5C has one N₁₀ unit consisting of an N₅ ring and an N₅ chain. This N₁₀ unit was similar to the N₅⁺N₅⁻ ionic compound.⁵² Isomer 5D is the fourth stable structure and has one η¹-N₅ ring, one N₂ and one N₃ unit.

The low-lying isomers of neutral LiN₁₀ are different from those of its positive charged counterpart. As shown in Fig. 3, 5A′ has three N₂ molecules and one η¹-N₄ ring. 5B′ consists of one N₂ molecule, one N₃ unit and one η⁵-N₅ ring. It is higher in energy than 5A′ by 43.80 kcal mol⁻¹. Isomer 5C′ consists of two face-to-face η¹-N₄ rings and one N₂ unit. The adjacent N₄ rings anchored on the Li atom in 5C′ are a precursor for the formation of other all-nitrogen structures. The most stable isomer with the N₃ ring is 5D′ that has two η¹-N₃ rings and one η⁴-N₄ ring. The nitrogen rings in 5D′ make it a highly energetic cluster.

5 Discussion

5.1 Structure of Li–N clusters

In our experiments, it is found that LiN_n⁺ clusters (n = 2, 4, 6, 8, and 10) can be generated by laser ablation of LiCl without any solid nitrogen source, indicating that the carrier gas (N₂) used in the experiments participated in the formation of Li–N clusters. The LiN_n⁺ clusters generated in our experiment all have even numbers of nitrogen atoms, suggesting that the generated LiN_n⁺ clusters may be formed by N₂ sub-units.

As shown in Fig. 3, the most stable isomers of LiN_n⁺ (1A–5A) obtained by theoretical calculations are formed by a lithium cation and a number of end-on bound N₂ units, that is in the form of Li⁺(N₂)_n/2. This is consistent with the facts that the generated LiN_n⁺ cluster cations all have even number of nitrogen atoms and their numbers of nitrogen atoms differ by multiples of 2. An examination of the most stable isomers 1A–5A shows that the structures of these observed LiN_n⁺ (n = 2, 4, 6, 8, and 10) cluster cations evolve from one-dimensional linear type (C_∞v, D_∞h) to two-dimensional branch type (D_3h) to three-dimensional tetrahedral (T_d) and square pyramid (C_4v) types with increasing number of N₂ units. LiN₈⁺ has a highly symmetrical structure with T_d symmetry. It also has high nitrogen content (94.1%) and may be used as a potential precursor for production of poly-nitrogen species.

Among the low-lying isomers of LiN_n^+/0 (n = 2, 4, 6, 8, and 10) presented in Fig. 2 and 3, the end-on bound N₂ units are the most common with the N–N bond lengths of approximately 1.09 Å, which are nearly the same as those in isolated nitrogen molecules. The side-on bound N₂ units are found in isomers 1A′ and 2A′, and the N–N bonds in these clusters are clearly weakened by coordination and the N–N bond lengths increase to approximately 1.16 Å. In addition, the isomers with a high number of N₂ units commonly have relatively lower energies than those with the other all-nitrogen units. This is very similar to the results obtained in our previous studies.^31,32

Other all-nitrogen groups, such as linear N₃, circular N₃, chain-shaped N₄, circular N₄, tetrahedral N₄, circular N₅, circular N₆ and so on, were also found in the low-lying isomers of LiN_n^+/0 (n = 2, 4, 6, 8, and 10) clusters. This suggests that the doping of lithium atom could promote the stability of the above all-nitrogen groups, and these energetic LiN_n^+/0 (n = 2, 4, 6, 8, and 10) isomers may exist in principle. Many N₅ units found in LiN_n^+/0 isomers were in the form of the η¹-N₅ ring, which is consistent with the coordination mode of compounds synthesized to date such as [M(H₂O)₄(N₅)₂]·4H₂O (M = Mn, Fe and Co).^53,54

5.2 Binding energies of the most stable isomers

To estimate the strength of the interactions between the Li atom and nitrogen ligands, we calculated the binding energies of the most stable isomers of LiN_n^+/0 (n = 2, 4, 6, 8, and 10). The calculation results are shown in Table 1 and Fig. 4. The Li–N₂ distances in LiN_n^+/0 (n = 2, 4, 6, 8, and 10) are also listed in Table 1 for comparison.

Table 1 Binding energies and Li–N₂ distances of the most stable isomers of LiN_n^+/0 (n = 2, 4, 6, 8, and 10)

Positive cluster		Symmetry	Multiplicity	Li–N2 distance	Eb (eV)	Neutral cluster		Symmetry	Multiplicity	Li–N2 distance	Eb (eV)
a The average Li–N2 distance.
Li–N2^+	1A	C∞v	1	2.069	0.57	Li–N2	1A′	C2v	2	1.879	−0.12
Li–N4^+	2A	D∞h	1	2.082	0.54	Li–N4	2A′	C2v	2	2.009, 1.917	0.12
Li–N6^+	3A	D3h	1	2.093	0.51	Li–N6	3A′	C2v	2	1.913	0.23
Li–N8^+	4A	Td	1	2.102	0.49	Li–N8	4A′	D4h	2	2.022	0.26
Li–N10^+	5A	C4v	1	2.172^a	0.45	Li–N10	5A′	C1	2	2.165^a	−0.79

---

Fig. 4 Binding energies of the most stable isomers of LiN_n^+/0 (n = 2, 4, 6, 8, and 10) clusters.

An examination of the results presented in Table 1 and Fig. 4 shows that binding energies of the ground states of LiN_n⁺ (n = 2, 4, 6, 8, and 10) are positive, suggesting a substantial energy stabilization of the LiN_n⁺ (n = 2, 4, 6, 8, and 10) species compared to the bare Li cation and N₂ molecules. The stabilizing effects decrease slowly as the numbers of end-on bound N₂ units increase. Considering that the N–N distances in the N₂ units of LiN_n⁺ (n = 2, 4, 6, 8, and 10) are all equal to that of the N₂ molecules calculated in this work, we wish to stress that the calculated binding energies reflect the Li–N₂ bond strengths in the clusters. Moreover, as shown in Table 1, the Li–N₂ distances were found to be in the order of LiN₂⁺ < LiN₄⁺ < LiN₆⁺ < LiN₈⁺ < LiN₁₀⁺, which is consistent with the order of the binding energies.

For neutral LiN_n (n = 2, 4, 6, 8, and 10) clusters, with the exception of the 1A′ and 5A′ clusters, the binding energies of the ground states are also positive, indicating the presence of similar stabilizing effects. However, for 1A′ with only one side-on bound N₂ unit, the binding energy is negative, possibly because the side-on bound N₂ unit has a higher energy than the N₂ molecule due to the weakening of the N–N bond (1.168 Å). The binding energy of 5A′ with N₄ rings is also negative and is much lower than those of the other stable Li–N isomers. This is because the energetic N₄ ring drastically increases the relative energy of isomer 5A′.

5.3 NBO analyses for the most stable isomers of LiN_n⁺

To further understand the binding and electronic structures of the Li–N clusters observed in the mass spectra, we performed NBO analyses for the most stable isomers of LiN_n⁺ (n = 2, 4, 6, 8, and 10), and the calculated results are presented in Tables S1–S5.† In the NBO model, the strength of the interaction of the electron donor and electron acceptor NBOs is defined by the stabilization energy E(2). To evaluate the general contribution of all of the interactions between the electron donors and electron acceptors to the stabilization energy of the LiN_n⁺ clusters, the sum of all of the stabilization energies, ∑E(2), was divided by the number of N₂ units, as shown in eqn (III), to obtain the average stabilization energy,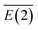 . Table 2 shows the NPA charge distributions and average second-order perturbation stabilization energies of the most stable isomers of the LiN_n⁺ clusters.

Table 2 The NPA charge distributions and second-order perturbation stabilization energies of the most stable isomers of the Li⁺–(N_α≡N_β)_n/2 clusters

Cluster		q (Li)	q (Nα)	q (Nβ)	Charge transfer	(kcal mol^−1)
Li–N2^+	1A	0.97607	−0.19289	0.21682	LP(1)Nα → LP*Li	8.93
Li–N4^+	2A	0.89635	−0.15482	0.20665	LP(1)Nα → LP*Li	22.65
Li–N6^+	3A	0.76153	−0.11126	0.19075	LP(1)Nα → LP*Li	37.36
Li–N8^+	4A	0.59306	−0.07581	0.17754	LP(1)Nα → LP*Li	50.48
Li–N10^+	5A	0.47380	−0.05275	0.15651	BD(1)Li–Nα → BD*(1)Li–Nα	31.32
			−0.05449	0.16567

---

Based on the NBO data, it was concluded that for LiN_n⁺ (n = 2, 4, 6, 8), electrons are transferred from the lone pair (LP) on N_α of every N₂ unit to the empty orbital LP* of the lithium atom, LP(1)N_α → LP*Li, leading to a lowering in the average stabilization energy by 8.93, 22.65, 37.36 and 50.48 kcal mol⁻¹, respectively. Meanwhile, for LiN₁₀⁺, the interaction is occurs mainly between the Li₁–N₂, Li₁–N₄, Li₁–N₆ and Li₁–N8 bonds, and the electrons are transferred from the bonding orbital (BD) of the Li–N_α bonds to the antibonding orbital (BD*) of the other Li–N_α bonds, giving rise to the values of approximately 31.32 kcal mol⁻¹. In both cases, the N_α and N_β in N₂ units carry negative and positive charges, respectively, forming a dipole that strongly interacts with Li⁺. The average stabilization energy, 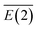, of LiN₈⁺ is the highest among the LiN_n⁺ (n = 2, 4, 6, 8, and 10) clusters, providing a partial explanation for the highest abundance of LiN₈⁺ in the mass spectra.

6 Conclusions

LiN_n⁺ (n = 2, 4, 6, 8, and 10) clusters were generated by laser ablation. LiN₈⁺ is found to be the most abundant. Density functional calculations were conducted to search for the stable structures of LiN_n^+/0. The theoretical results show that the most stable isomers of LiN_n⁺ (n = 2, 4, 6, 8, and 10) are in the form of Li⁺(N₂)_n/2, and their structures evolve from one-dimensional linear type (C_∞v, n = 2; D_∞h, n = 4), to two-dimensional branch type (D_3h, n = 6) to three-dimensional tetrahedral (T_d, n = 8) and square pyramid (C_4v, n = 10) types. The calculated binding energies suggest a substantial energy stabilization of the LiN_n⁺ (n = 2, 4, 6, 8, and 10) species compared to the bare Li cation and N₂ molecules. Further NBO analyses show that the N₂ units in LiN_n⁺ are actually dipoles that could interact with Li⁺ effectively, and LiN₈⁺ has the highest average stabilization energy. For neutral LiN_n (n = 2, 4, 6, and 8) clusters, the most stable isomers were also formed by a Li atom and the corresponding number of N₂ units, while the most stable isomer of LiN₁₀ is in the form of Li⁺(N₂)₃(η¹-N₄).

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (Grant No. 21103202 and 21273246).

Notes and references

1. W. J. Lauderdale, J. F. Stanton and R. J. Bartlett, J. Phys. Chem., 1992, 96, 1173–1178.
2. H. Östmark, New Trends in Research of Energetic Materials Czech Republic, 2006, pp. 231–250.
3. P. C. Samartzis and A. M. Wodtke, Int. Rev. Phys. Chem., 2006, 25, 527–552.
4. M. N. Glukhovtsev, H. Jiao and P. v. R. Schleyer, Inorg. Chem., 1996, 35, 7124–7133.
5. K. O. Christe, W. W. Wilson, J. A. Sheehy and J. A. Boatz, Angew. Chem., Int. Ed., 1999, 38, 2004–2009.
6. F. Cacace, G. de Petris and A. Troiani, Science, 2002, 295, 480–481.
7. A. Vij, J. G. Pavlovich, W. W. Wilson, V. Vij and K. O. Christe, Angew. Chem., Int. Ed., 2002, 41, 3051–3054.
8. L. Gagliardi and P. Pyykkö, J. Phys. Chem. A, 2002, 106, 4690–4694.
9. G. von Zandwijk, R. A. J. Janssen and H. M. Buck, J. Am. Chem. Soc., 1990, 112, 4155–4164.
10. Q. S. Li and L. P. Cheng, J. Phys. Chem. A, 2003, 107, 2882–2889.
11. L. P. Cheng and Q. S. Li, J. Phys. Chem. A, 2005, 109, 3182–3186.
12. J. M. Mercero, J. M. Matxain and J. M. Ugalde, Angew. Chem., Int. Ed., 2004, 43, 5485–5488.
13. L. Jin and Y.-h. Ding, J. Phys. Chem. A, 2009, 113, 13645–13650.
14. A. C. Tsipis and A. T. Chaviara, Inorg. Chem., 2004, 43, 1273–1286.
15. M. Lein, Chem.–Eur. J., 2001, 7, 4155–4163.
16. R. Haiges, J. A. Boatz, S. Schneider, T. Schroer, M. Yousufuddin and K. O. Christe, Angew. Chem., Int. Ed., 2004, 43, 3148–3152.
17. R. Haiges, J. A. Boatz, T. Schroer, M. Yousufuddin and K. O. Christe, Angew. Chem., Int. Ed., 2006, 45, 4830–4835.
18. R. Haiges, J. A. Boatz, M. Yousufuddin and K. O. Christe, Angew. Chem., Int. Ed., 2007, 46, 2869–2874.
19. R. Haiges, J. A. Boatz and K. O. Christe, Angew. Chem., Int. Ed., 2010, 49, 8008–8012.
20. R. Haiges, J. A. Boatz, R. Bau, S. Schneider, T. Schroer, M. Yousufuddin and K. O. Christe, Angew. Chem., Int. Ed., 2005, 44, 1860–1865.
21. A. C. Filippou, P. Portius, D. U. Neumann and K.-D. Wehrstedt, Angew. Chem., Int. Ed., 2000, 39, 4333–4336.
22. P. Portius, A. C. Filippou, G. Schnakenburg, M. Davis and K.-D. Wehrstedt, Angew. Chem., Int. Ed., 2010, 49, 8013–8016.
23. A. C. Filippou, P. Portius and G. Schnakenburg, J. Am. Chem. Soc., 2002, 124, 12396–12397.
24. A. Villinger and A. Schulz, Angew. Chem., Int. Ed., 2010, 49, 8017–8020.
25. C. Knapp and J. Passmore, Angew. Chem., Int. Ed., 2004, 43, 4834–4836.
26. T. M. Klapötke, B. Krumm, M. Scherr, R. Haiges and K. O. Christe, Angew. Chem., Int. Ed., 2007, 46, 8686–8690.
27. T. M. Klapötke, B. Krumm, P. Mayer and I. Schwab, Angew. Chem., Int. Ed., 2003, 42, 5843–5846.
28. E. D. Pillai, T. D. Jaeger and M. A. Duncan, J. Am. Chem. Soc., 2007, 129, 2297–2307.
29. E. D. Pillai, T. D. Jaeger and M. A. Duncan, J. Phys. Chem. A, 2005, 109, 3521–3526.
30. A. D. Brathwaite, H. L. Abbott-Lyon and M. A. Duncan, J. Phys. Chem. A, 2016, 120, 7659–7670.
31. K. W. Ding, X. W. Li, H. G. Xu, T. Q. Li, Z. X. Ge, Q. Wang and W. J. Zheng, Chem. Sci., 2015, 6, 4723–4729.
32. K. W. Ding, H. G. Xu, Y. Yang, T. Q. Li, Z. Q. Chen, Z. X. Ge, W. L. Zhu and W. J. Zheng, J. Phys. Chem. A, 2018, 122, 4687–4695.
33. A. M. Sapse and P. V. R. Schleyer, Lithium Chemistry: Theoretical and Experimental Overview, Wiley Interscience, New York, USA, 1995.
34. D. Hao, J. Liu and J. Yang, J. Phys. Chem. A, 2008, 112, 10113–10119.
35. T. B. Tai and M. T. Nguyen, Chem. Phys. Lett., 2010, 492, 290–296.
36. L. F. Gong, W. L. Guo, X. M. Wu and Q. S. Li, Chem. Phys. Lett., 2006, 429, 326–331.
37. X. An, H. Zhuo, W. Tian, Q. Li, W. Li and J. Cheng, Mol. Phys., 2014, 112, 2954–2962.
38. Q. S. Li and L. P. Cheng, J. Phys. Chem. A, 2003, 107, 2882–2889.
39. M. N. Glukhovtsev, P. v. R. Schleyer and C. Maerker, J. Phys. Chem., 1993, 97, 8200–8206.
40. Y. C. Zhao, Z. G. Zhang, J. Y. Yuan, H. G. Xu and W. J. Zheng, Chin. J. Chem. Phys., 2009, 22, 655–662.
41. M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr, J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, J. M. M. N. Rega, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, O. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 09, Revision A.02, Gaussian, Inc., Wallingford, CT, 2009.
42. Y. Zhao and D. G. Truhlar, Theor. Chem. Acc., 2008, 120, 215–241.
43. R. Huenerbein, B. Schirmer, J. Moellmann and S. Grimme, Phys. Chem. Chem. Phys., 2010, 12, 6940–6948.
44. CRC Handbook of Chemistry and Physics, ed. W. M. Haynes, 95th edn, 2014–2015.
45. A. E. Reed, L. A. Curtiss and F. Weinhold, Chem. Rev., 1988, 88, 899–926.
46. M. N. Glukhovtsev, H. Jiao and P. v. R. Schleyer, Inorg. Chem., 1996, 35, 7124–7133.
47. M. T. Nguyen, Coord. Chem. Rev., 2003, 244, 93–113.
48. A. Korkin, A. Balkova, R. J. Bartlett, R. J. Boyd and P. R. Schleyer, J. Phys. Chem., 1996, 100, 5702.
49. L. Gagliardi and P. Pyykko, J. Phys. Chem. A, 2002, 106, 4690–4694.
50. M. Lein, J. Frunzke, A. Timoshkin and G. Frenking, Chem.–Eur. J., 2001, 7, 4155–4163.
51. L. Jin and Y. Ding, J. Phys. Chem. A, 2009, 113, 13645–13650.
52. S. Fau, K. J. Wilson and R. J. Bartlett, J. Phys. Chem. A, 2002, 106, 4639–4644.
53. Y. Xu, Q. Wang, C. Shen, Q. Lin, P. Wang and M. Lu, Nature, 2017, 549, 78–81.
54. C. Zhang, C. Yang, B. Hu, C. Yu, Z. Zheng and C. Sun, Angew. Chem., Int. Ed., 2017, 56, 1–4.

---

Footnote

† Electronic supplementary information (ESI) available: Similar mass spectra and NBO data of the Li–N clusters. See DOI: 10.1039/c9ra00439d

---