Open Access Article
Open Access ArticleEffect of vacancies on the structural and electronic properties of Ti2CO2
Li Xiao-Hong *,
Su Xiang-Ying and
Zhang Rui-Zhou
*,
Su Xiang-Ying and
Zhang Rui-Zhou
College of Physics and Engineering, Henan University of Science and Technology, Luoyang 471003, China. E-mail: lorna639@126.com
First published on 3rd September 2019
Abstract
Ti2CO2 MXene is widely considered as a potential candidate material for sensors and optical devices. In this paper, first-principles calculations are performed to investigate the structural and electronic properties of pristine and vacancy defect Ti2CO2 monolayer. The results indicate that C-vacancy is energetically more favorable than Ti-vacancy and O-vacancy because of the smaller formation energy of C vacancy. The introduction of vacancy defects results in the transition from semiconductor to metal, and improves the electronic conductivities of Ti2CO2 monolayer. The introduction of C and O vacancies causes the Ti-d state to split into several peaks in the energy range of 0 to 2 eV, while the introduction of Ti vacancy makes the Ti-d state weaker and the C-p state stronger. Furthermore, the work function can be effectively engineered by vacancy defects.
1 Introduction
Two-dimensional (2D) materials have been an active research area since the discovery of graphene.1 Graphene is a significant 2D material2 with zero-band gap semi-metallic properties, which hampers its application in highly integrated electronic components. We can tune the band gap of graphene, but the available range of the band gap is not enough to satisfy the requirement of the application of nanoelectronic, optoelectronic devices. Therefore, it is necessary to design or search for new layered materials with intrinsic bandgaps, high thermal stability and conductivity, and high carrier mobility.MXene is a new group of low-dimensional materials3–5 and has attracted intensive attention in the field of supercapacitors,6,7 lead adsorption,8 catalysis9 and so on. MXene is obtained by selectively etching layers of A elements from its host, layered ternary MAX materials (Mn+1AXn), where n = 1, 2, 3; M is a transition metal, and X is C or N.10 The frequently used etchant is hydrofluoric acid (HF), HF-containing etchants and so on. F, O, and/or OH functional groups are often generated on the external surface of the exfoliated layers. So functionalized MXenes are denoted as Mn+1XnTx, in which T stands for the terminating F, O, and/or OH functional groups, x is the termination number.11 These functional groups are very important in the applications of energy storage, catalysis etc.12–14
Defects have been investigated in 2D materials and can influence the performance of materials and devices.15,16 It is important to understand the layered structure and point defects, which are crucial for exploration of the physiochemical properties of MXenes. The properties of 2D materials can be engineered by defects. For the low-dimensional materials, defects have a much stronger impact, when compared to their bulk counterparts. And defect engineering has attracted extensive attention17 and is often used to modify the electronic structures of many layered structures.18 Zhang et al.19 theoretically investigated the carbon-vacancy ordering in Nb4AlC3−x, and reported the importance roles of carbon vacancies in the structure stability and order–disorder phase transformation. Carbon vacancies also have important influences on the superconducting properties of materials.20–22
Vacancies are universally present in the MAX phases.23,24 So it is believed that MXenes inherit their hosts' defect. Ti2CO2 is the thinnest O-functionalized Ti-based MXene, and has many promising applications, such as catalyst, optical devices, and gas sensor.25–27 Wang et al.28 investigated the stabilities and electronic properties of vacancy-doped Ti2CO2. Their results indicated that the formation energies of C-vacancy are relatively small. Hu et al.29 reported the influence of C-vacancy on the structural stability, electronic properties of a 2 × 2 × 1 Ti2CT2 (T = O, F, and OH) supercell. They thought that C-vacancy in MXene is much easier to form when compared with graphene and other two-dimensional material. Bandyopadhyay et al.30 investigated the structural and magnetoelectronic behavior of a 4 × 4 × 1 Ti2CO2 supercell with single vacancy and double vacancies. They thought that the defect formation energies are greatly dependent on local chemical bonding. Sang et al.31 reported the atomic defects in monolayer Ti3C2Tx MXene. They reported that the formation energy of Ti-vacancy on Ti3C2Ox (7.74 eV), and the defects can't strongly influence the metallic conductivity, but can influence the surface morphology and termination groups. To the best of our knowledge, limited researches report the impact of different vacancy defect on the properties of Ti2CO2 monolayer.
Work function (WF) is an important parameter for electrode materials. A material with higher WF can be as an anode, and a material with low WF can be treated as a cathode. Research32 indicates that the materials with higher work function can reduce the Schottky barrier, which can cause increased contact resistances and limit the performance of devices. Therefore, the modulation of WF is important to improve the device performance. Up to now, the report on the modulation of WF of Ti2CO2 monolayer is unavailable.
Here, we investigated the effect of carbon vacancy (VC), Ti vacancy (VTi), and oxygen vacancy (VO) on structure, electronic properties, and work function of Ti2CO2 monolayer. The comparisons of related properties between pristine and vacancy Ti2CO2 monolayer and the modulation of vacancy defect on WF are performed in our study, which are not discussed in other people's works.29–31 Our investigation will provide an alternative method to engineer the physical and chemical properties of MXenes.
2 Computational details
All density functional theory (DFT) calculations are carried out using the Dmol3 code.33 The Perdew–Burke–Ernzerhof (PBE) functional34 generalized gradient approximation (GGA)35 is used. The used basis set is double numerical plus polarization (DNP), which corresponds to a double-ζ quality basis set with p-type polarization functions added to hydrogen, and d-type polarization functions added to heavier atoms. DNP basis set is comparable with the Gaussian 6-31G (d, p) basis set and exhibits a better accuracy.36 A 3 × 3 × 1 Ti2CO2 supercell is constructed with one carbon, titanium, or oxygen removal to highlight the influence of VC, VTi, and VO, respectively. The three models of Ti2CO2-VC, Ti2CO2-VTi, and Ti2CO2-VO monolayers are presented in Fig. 1. In order to have a comparison, the structure of pristine Ti2CO2 monolayer (PTM) is also presented in Fig. 1.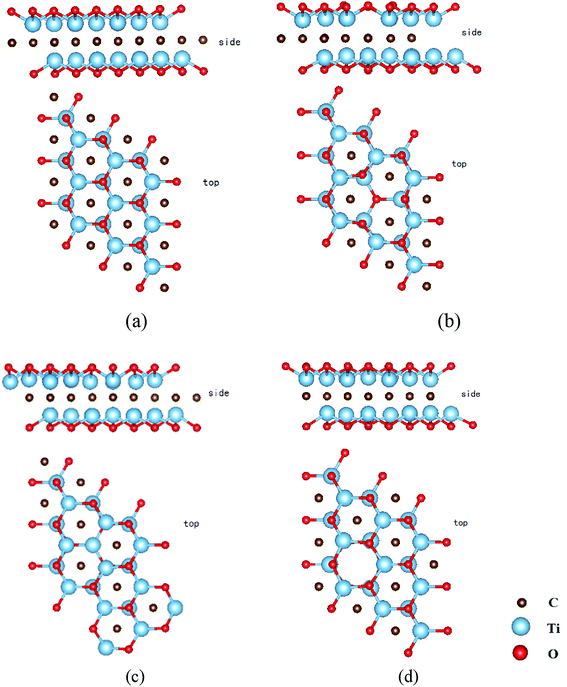 | ||
| Fig. 1 The atomic structures of pristine Ti2CO2 (a), Ti2CO2-VTi (b), Ti2CO2-VO (c), and Ti2CO2-VC (d) from top and side view. | ||
In order to simulate the isolated monolayer, a large vacuum space of 25 Å was set in the direction normal to MXene layers to avoid any unnecessary interaction between the MXene layers. In our calculation, the Brillouin zone (BZ) integration was sampled by using a 5 × 5 × 1 k-mesh according to Monkhorst–Pack method.37 The convergence in energy, force and displacement were 1 × 10−6 Ha, 0.001 Ha Å−1, and 0.005 Å, respectively. A denser 9 × 9 × 1 mesh is used to calculate the electronic density of states (DOS).
3 Results and discussion
3.1 The structure of pristine and defect-Ti2CO2 monolayer
In order to determine what defects are likely to form, the vacancy formation energies Eform for Ti, C, or O defect are obtained by the following equation:| Eform = Evac + Uatom − Epristine | (1) |
The calculated crystal parameter of PTM is 9.0483 Å, while the crystal parameters of Ti2CO2 monolayers with Ti, C, or O defect are 9.0737, 9.0719, and 9.0636 Å, respectively. So the crystal parameters of Ti2CO2-VC, Ti2CO2-VTi, and Ti2CO2-VO monolayers change slightly when compared with that of PTM. The volume of PTM is 1665.59 Å3. Compared with the volume of PTM, the volume of Ti2CO2-VC monolayer increases 1.99%. The creation of C vacancy makes the Ti atoms near C vacancy away from the vacancy by the remaining strengthened pd bonds, which results in the volume increase of Ti2CO2-VC. Compared with the volume of PTM, the volume of Ti2CO2-VTi increases by 2.01%. The reason is that the creation of Ti vacancy makes the formation of unsaturated electrons on nearest C/O atoms, which result in the stronger interactions between C/O atoms and their nearest Ti atoms. The volume of Ti2CO2-VO increases by 1.90% when compared to that of PTM. The creation of O vacancy on the surface of Ti2CO2 monolayer makes the charge redistribution, and results in the formation of the stronger Ti–O and Ti–C bonds near O vacancy.
3.2 The electronic properties of pristine and defect-Ti2CO2 monolayers
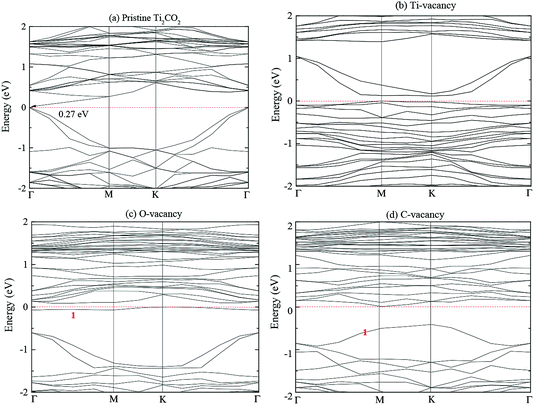
Fig. 2 presents the structures of pristine and defect Ti2CO2 monolayer along the symmetry directions Γ–M–K–Γ. The valence band maximum (VBM) and conduction band minimum (CBM) are labelled in Fig. 2. PTM is an M–Γ indirect semiconductor with the band gap of 0.27 eV, which is close to the results of Xie et al. (0.24 eV by PBE)41 and Zha et al. (0.261 eV by PBE).42 The introduction of vacancy defect results in the transition from semiconductor to metal.From Fig. 2(b), the introduction of Ti-vacancy makes the Fermi energy level shift downward compared with that of PTM, and the valence band passes through the Fermi level, which results in the metallic character of Ti2CO2-VTi. Ti defect level lies in the valence band region of PTM. From Fig. 2(c), the introduction of O vacancy defect makes the Fermi energy level shift upward. The defect energy level (band 1 in Fig. 2(c)) appears because of the removal of one oxygen atom, which passes through the Fermi energy level and results in the metallization of Ti2CO2-VO. This indicates that the O vacancy has greater effect on the photocatalyst properties of Ti2CO2 monolayer. In addition, it is noted that the defect energy level fluctuates slightly and changes gently, which indicates that the electrons in this energy level have larger effective mass, stronger localization and electron binding. From Fig. 2(d), the introduction of C vacancy makes the Fermi level shift upward compared with that of PTM. The defect energy level (band 1 in Fig. 2(d)) is introduced below the Fermi level. So the vacancy defect can effectively engineer the band structure of Ti2CO2 monolayer.
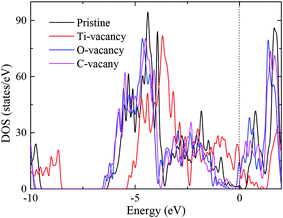
Fig. 3 presents the total density of state (DOS) of pristine and vacancy defect Ti2CO2 monolayer. It is noted that the introduction of vacancy defect imposes the significant impact on the electronic structure of Ti2CO2 monolayer. The improved electronic conductivities of Ti2CO2-VTi, Ti2CO2-VO, and Ti2CO2-VC monolayers are observed due to the significantly increased DOS at the Fermi level (EF). So the introduction of vacancy defect can improve the electronic conductivities of functionalized MXenes.
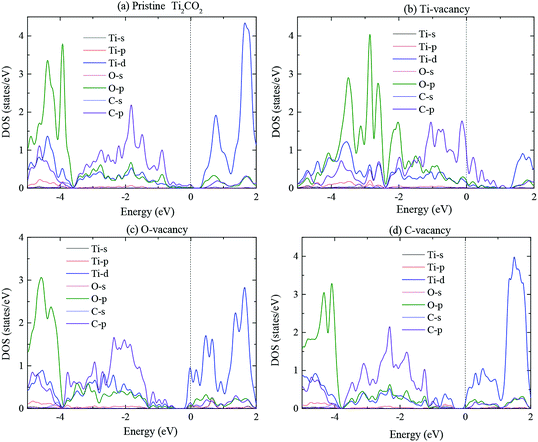
Fig. 4 presents the partial density of state (PDOS) of Ti, O, and C atoms of vacancy defect Ti2CO2 monolayer in order to illustrate the origin of electronic conductivity. The PDOS of PTM is also plotted in order to have a comparison. For PTM, the valence band is mainly from the contribution of Ti-d, C-p and O-p states, while the conduction band is mainly from the contribution of Ti-d states. Similar to PTM, Ti-d, O-p, and C-p states provide the mainly contribution of valence band for Ti2CO2-VTi monolayer, while C-p states mainly contribute to the conduction band. For Ti2CO2-VO and Ti2CO2-VC monolayers, Ti-d, O-p and C-p states provide the mainly contribution to valence band and conduction band. Combined Fig. 2 and 4, for Ti2CO2-VTi monolayer, the band at Γ point is mainly localized on C-p state, the introduction of Ti vacancy results in the unsaturated C and O atoms. For Ti2CO2-VO monolayer, the introduction of O vacancy results in three unsaturated Ti-3d states, which have the main contribution to the band at Γ point. The introduction of C vacancy results in the six unsaturated Ti atoms for Ti2CO2-VC monolayer, so Ti-d state have the main contribution to the band at Γ point and VBM from Fig. 4(d).
It is noted that the introduction of C and O vacancy makes Ti-d state split into several peaks in the energy range of 0 to 2 eV because of the structural modification. While the introduction of Ti vacancy makes Ti-d state weaker and C-p state stronger. Combined with Fig. 3 and 4, the valley of the total DOS at −3.5 eV in Fig. 3 is mainly from Ti-d state for Ti2CO2-VTi monolayer, Ti-d, O-p and C-p states for Ti2CO2-VO and Ti2CO2-VC monolayers. In the energy range of −3 to 0 eV, there exist strong interactions between Ti-d and C-p states, Ti-d and O-p states for all studied Ti2CO2 monolayers.
3.3 The analysis of work function and atomic charge
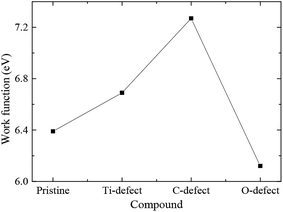
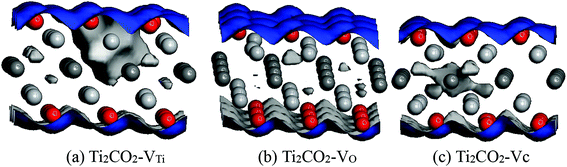
Work function (WF) is an important physical parameter of materials and plays the significant role in the light emitting diodes and the field effect transistors. The work function of pristine Ti2CO2 monolayer is 6.39 eV, while the work functions of Ti2CO2-VC, Ti2CO2-VTi, and Ti2CO2-VO monolayers are 7.27, 6.69, and 6.12 eV, respectively. This indicates that the work function can be effectively engineered by vacancy defect. According to the report of Ma et al.,32 Ti2CO2-VC and Ti2CO2-VTi monolayers with higher WFs can reduce the Schottky barrier and decrease the field emission performance, while Ti2CO2-VO monolayer with lower WF can improve the field emission performance. In the other hand, Ti2CO2-VC and Ti2CO2-VTi monolayers can be considered as anode because of the higher WFs, while Ti2CO2-VO monolayer can be considered as cathode due to the lower WF (Fig. 5).The analysis of Mulliken charge is performed in order to further investigate the effect of vacancy defect on the charge distribution. Fig. 6 presents the electrostatic potential of Ti2CO2-VTi, Ti2CO2-VO, and Ti2CO2-VC monolayers. For PTM, the charge of Ti atom in supercell is 1.202e−, while the charges of the adjacent C and O atoms are −0.980 and −0.712e−, respectively. For Ti2CO2-VTi monolayer, the removal of Ti atom results in the charge localization on the defect site, adjacent unsaturated O and C atoms. The negative charge accumulation is exhibited around Ti vacancy defect. The charge aggregation is exhibited around O atoms, while the loss of negative charge is exhibited around C atoms. The charges of the adjacent C and O atoms are −0.704 and −0.584e−, respectively. For Ti2CO2-VO monolayer, the positive charge accumulation is exhibited around O vacancy defect. The charge of the adjacent Ti atom is 1.187e−, while the charge of the adjacent C atom has little change because of the longer distance from O vacancy. From Fig. 6(c), there is a clear charge accumulation around C vacancy defect for Ti2CO2-VC monolayer. The charge of the adjacent Ti atom is 1.189e−, while the charge of adjacent O atom increases and is −0.708 e−. The introduction of C vacancy weakens the adjacent Ti–O bonds.
4 Conclusion
In this paper, the structure, electronic properties of pristine and vacancy defect Ti2CO2 monolayer have been investigated by the first principles calculation. The analysis of formation energies indicates that C-vacancy is energetically more favorable than Ti-vacancy and O-vacancy. PTM is a semiconductor. The introduction of vacancy defect results in the metallic character of Ti2CO2 monolayer and improves the electronic conductivity of Ti2CO2 monolayer. The work function can be effectively engineered by vacancy defect. O vacancy defect can improve the field emission performance, while Ti or C vacancy defect can decrease the field emission performance for Ti2CO2 monolayer. The analysis of Mulliken charge is further performed.Conflicts of interest
There are no conflicts to declare.Acknowledgements
We gratefully thank the National Natural Science Foundation of China (Grant U1304111), and the Program for Science & Technology Innovation Talents in the Universities of Henan Province (No. 14HASTIT039) and the Innovation Team of Henan University of Science and Technology (2015XTD001) for their support of this work.References
- K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, M. I. Katsnelson, I. V. Grigorieva, S. V. Dubonos and A. A. Firsov, Nature, 2005, 438, 197 CrossRef CAS PubMed.
- K. S. Novoselov, D. Jiang, F. Schedin, T. J. Booth, V. V. Khotkevich, S. V. Morozov and A. K. Geim, Proc. Natl. Acad. Sci. U. S. A., 2005, 102, 10451 CrossRef CAS PubMed.
- M. Naguib, V. N. Mochalin, M. W. Barsoum and Y. Gogotsi, Adv. Mater., 2014, 26, 992 CrossRef CAS PubMed.
- Y. Gogotsi, Nat. Mater., 2015, 14, 1079 CrossRef CAS PubMed.
- M. Naguib and Y. Gogotsi, Acc. Chem. Res., 2015, 48, 128 CrossRef CAS PubMed.
- M. Ghidiu, M. R. Lukatskaya, M. Q. Zhao, Y. Gogotsi and M. W. Barsoum, Nature, 2014, 516, 78 CrossRef CAS PubMed.
- M. Hu, Z. Li, G. Li, T. Hu, C. Zhang and X. Wang, Adv. Mater. Technol., 2017, 2, 1700143 CrossRef.
- Q. M. Peng, J. X. Guo, Q. R. Zhang, J. Y. Xiang, B. Z. Liu, A. G. Zhou, R. P. Liu and Y. J. Tian, J. Am. Chem. Soc., 2014, 136, 4113 CrossRef CAS PubMed.
- X. Zhang, Z. Zhang, J. Li, X. Zhao, D. Wu and Z. Zhou, J. Mater. Chem. A, 2017, 5, 12899 RSC.
- M. Naguib, V. N. Mochalin, M. W. Barsoum and Y. Gototsi, Adv. Mater., 2014, 26, 992 CrossRef CAS PubMed.
- D. Magne, V. Mauchamp, S. Celerier, P. Chartier and T. Cabioch, Phys. Rev. B: Condens. Matter Mater. Phys., 2015, 91, 201409 CrossRef.
- Y. Xie, M. Naguib, V. N. Mochalin, M. W. Barsoum, Y. Gogotsi, X. Yu, K.-W. Nam, X.-Q. Yang, A. I. Kolesnikov and P. R. C. Kent, J. Am. Chem. Soc., 2014, 136, 6385 CrossRef CAS PubMed.
- C. Eames and M. S. Islam, J. Am. Chem. Soc., 2014, 136, 16270 CrossRef CAS PubMed.
- Y. Xie, Y. Dall'Agnese, M. Naguib, Y. Gogotsi, M. W. Barsoum, H. L. Zhuang and P. R. C. Kent, ACS Nano, 2014, 8, 9606 CrossRef CAS PubMed.
- R. Bourrellier, S. Meuret, A. Tararan, O. Stéphan, M. Kociak and L. H. G. Tizei, Nano Lett., 2016, 16, 4317 CrossRef CAS PubMed.
- D. Wong, J. Velasco, L. Ju, J. Lee, S. Kahn, H.-Z. Tsai, C. Germany, T. Taniguchi, K. Watanabe, A. Zettl, F. Wang and M. F. Crommie, Nanotechnol, 2015, 10, 949 CAS.
- T. T. Tran, K. Bray, M. J. Ford, M. Toth and I. Aharonovich, Nat. Nanotechnol., 2015, 11, 37 CrossRef PubMed.
- W. M. Parkin, A. Balan, L. Liang, P. M. Das, M. Lamparski, C. H. Naylor, J. A. RodriguezManzo, A. T. Johnson, V. Meunier and M. Drndic, ACS Nano, 2016, 10, 4134 CrossRef CAS PubMed.
- H. Zhang, T. Hu, X. H. Wang, Z. J. Li, M. M. Hu, E. D. Wu and Y. C. Zhou, Sci. Rep., 2015, 5, 14192 CrossRef CAS PubMed.
- A. I. Gusev, Disorder and Order in Strongly Nonstoichiometric Compounds: Transition Metal Carbides, Nitrides and Oxides, Springer, New York, 2001 Search PubMed.
- J. Nguyen, N. Glandut, C. D. Jaoul and P. Lefort, Langmuir, 2013, 29, 12036 CrossRef CAS PubMed.
- X. X. Yu, G. B. Thompson and C. R. Weinberger, J. Eur. Ceram. Soc., 2015, 35, 95 CrossRef CAS.
- H. Wang, H. Han, G. Yin, C.-Y. Wang, Y.-Y. Hou, J. Tang, J.-X. Dai, C.-L. Ren, W. Zhang and P. Huai, Materials, 2017, 10(2), 103 CrossRef PubMed.
- H. Han, D. Wickramaratne, Q. Huang, J. X. Dai, T. W. Li, H. Wang, W. Zhang and P. Huai, RSC Adv., 2016, 6, 84262 RSC.
- X.-F. Yu, Y.-C. Li, J.-B. Cheng, Z.-B. Liu, Q.-Z. Li, W.-Z. Li, X. Yang and B. Xiao, ACS Appl. Mater. Interfaces, 2015, 7, 13707 CrossRef CAS PubMed.
- X.-F. Yu, J.-B. Cheng, Z.-B. Liu, Q.-Z. Li, W.-Z. Li, X. Yang and B. Xiao, RSC Adv., 2015, 5, 30438 RSC.
- X. Zhang, Z. Zhang, J. Li, X. Zhao, D. Wu and Z. Zhou, J. Mater. Chem. A, 2017, 5, 12899 RSC.
- C. Wang, H. Han and Y. Guo, Comput. Mater. Sci., 2019, 159, 127 CrossRef CAS.
- T. Hu, J. Yang and X. Wang, Phys. Chem. Chem. Phys., 2017, 19, 31773 RSC.
- A. Bandyopadhyay, D. Ghoshb and K. Swapan Pati, Phys. Chem. Chem. Phys., 2018, 20, 4012 RSC.
- X. Sang, Y. Xie, M. W. Lin, M. Alhabeb, K. L. Van Aken, Y. Gogotsi, P. R. Kent and K. Xiao, ACS Nano, 2016, 10, 9193 CrossRef CAS PubMed.
- Y. Ma, C. Shen, A. Zhang, L. Chen, Y. Liu, J. Chen, Q. Liu, Z. Li, R. M. Amer, T. Nilges, N. Ahmad Abbas and C. Zhou, ACS Nano, 2017, 11, 7126 CrossRef CAS PubMed.
- B. Delley, J. Chem. Phys., 1990, 92, 508 CrossRef CAS.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865 CrossRef CAS PubMed.
- P. E. Blöchl, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953 CrossRef PubMed.
- M. Wang, H. Liu, Z.-H. Huang and F. Kang, Chem. Eng. J., 2014, 256, 101 CrossRef CAS.
- H. J. Monkhorst and J. D. Pack, Phys. Rev. B: Solid State, 1976, 13, 5188 CrossRef.
- H. Han, D. Wickramaratne, Q. Huang, J. X. Dai, T. W. Li, H. Wang, W. Zhang and P. Huai, RSC Adv., 2016, 6, 84262 RSC.
- J. M. Gibson, Phys. Today, 1997, 50, 56 CrossRef CAS.
- H. P. Komsa, J. Kotakoski, S. Kurasch, O. Lehtinen, U. Kaiser and A. V. Krasheninnikov, Phys. Rev. Lett., 2012, 109, 035503 CrossRef PubMed.
- Y. Xie and P. R. C. Kent, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87, 235441 CrossRef.
- X.-H. Zha, K. Luo, Q. Li, Q. Huang, J. He, X. Wen and S. Du, Europhys. Lett., 2015, 111, 26007 CrossRef.
| This journal is © The Royal Society of Chemistry 2019 |