Impact of metallic trace elements on relaxivities of iron-oxide contrast agents

Abstract

In this work, well-defined 3 nm-sized Ca²⁺, Fe³⁺, Na⁺, Mg²⁺, Zn²⁺, Ni²⁺, Co²⁺, and Cd²⁺ cation-adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles were used as prototype systems to investigate the influence of metallic trace elements in body fluids on the relaxivities of iron-oxide contrast agents. It was found that surface-adsorbed cations formed a deterioration layer to induce pronounced relaxivity loss. Theoretical study showed that such relaxivity loss can be well described by a modified GCAS function, taking into account the harmonic cation oscillations around Fe₃O₄/γ-Fe₂O₃ nanoparticles. Quantum mechanics analyses revealed that even-parity and odd-parity states of harmonic oscillations are dominant in r₁ and r₂ relaxivities, respectively. Moreover, the harmonic oscillations of Na⁺ and Mg²⁺ cations around Fe₃O₄/γ-Fe₂O₃ nanoparticles are found to be classical forbidden, which are quite different from their counterparts located in the classical permissive area.

---

Introduction

During the last decade or so, magnetic resonance imaging (MRI) has become a vital non-invasive technique that aims to obtain high-resolution anatomical images for diagnosing various kinds of diseases.^1–6 In this scenario, contrast agents are widely employed based on the fact that they can enhance the image contrast in designated regions with brighter or darker signals,^7,8 and hence greatly boosting imaging sensitivity in MRI. Despite strenuous and unremitting efforts towards exploring new contrast agents, two tough issues have still been lingering around and plaguing academia. The first one is MRI artifacts, which are always undesirable but unavoidable due to signals yielding from calcification, haemorrhage, blood clot, fat, air, and so forth.^9,10 These artifacts are sometimes indistinguishable from the MRI signals of the applied contrast agents, and probably result in the inaccurate interpretation for the diagnosis. Although various techniques have been developed to suppress artifacts by means of resonant radio frequency tuning,^11,12 successful applications of these techniques are very limited.^13–16 The second pressing issue is mainly related to in vivo surroundings, in which the adsorption of macroelements and trace elements onto the contrast agents in body fluids may greatly alter their MRI properties determined from in vitro tests. This means that the well-defined MRI performance of as-prepared contrast agents may not be realized or even real in clinical application due to the presence and adsorption of elements in complex fluid environments. This is an important but poorly understood matter open to discussion.

Given that these two tough issues are mainly related to the complicated in vivo environments, therefore, in this work, we study the influence of metallic trace elements in fluids on relaxivities of iron-oxide contrast agents. Basically, trace elements in human body can be divided into three types: (i) essential trace elements, including iodine, zinc, selenium, copper, molybdenum, chromium, cobalt, and iron elements; (ii) possibly essential trace elements, including manganese, silicon, boron, vanadium, and nickel elements; (iii) potentially toxic elements but essential in their low dose, including fluorine, lead, cadmium, mercury, arsenic, aluminium, and tin elements. In this study, Fe³⁺, Zn²⁺, Co²⁺ (type i), Mg²⁺, Ni²⁺ (type ii), Cd²⁺ (type iii) cations together with two macroelements (Ca²⁺ and Na⁺ cations) were chosen as typical elements to separately adsorb onto negatively charged 3 nm-sized Fe₃O₄/γ-Fe₂O₃ contrast agents. Relaxivity measurements were then performed to demonstrate relaxivity loss and its variation rule after adsorption of various metallic cations.

Results and discussion

The XRD pattern of the as-synthesized pristine product in Fig. 1(a) shows the presence of Fe₃O₄ (JCPDS no. 85-1436) phase or γ-Fe₂O₃ (JCPDS no. 39-1346) phase, or both. Further, XPS spectrum in Fig. 1(b) gives two distinct peaks at ∼711 and ∼724 eV corresponding to Fe2p_3/2 and Fe2p_1/2 levels, respectively.¹⁷ Two satellite peaks around 718 and 732 eV demonstrate the existence of γ-Fe₂O₃ phase,¹⁸ indicating that the pristine product is a hybrid of both Fe₃O₄ phase and γ-Fe₂O₃ phase. The TEM study shows that pristine Fe₃O₄@γ-Fe₂O₃ particles are near spherical [inset of Fig. 1(a)], with an average diameter around 3 nm. These 3 nm-sized Fe₃O₄@γ-Fe₂O₃ particles possess negatively charged surfaces with a zeta potential value of −27.6 mV, which is of benefit to cation adsorption in aqueous solutions. Prior to the impregnation of these bare nanoparticles into various cation stock solutions, control experiments on relaxivities were carried out for these pristine Fe₃O₄/γ-Fe₂O₃ nanoparticles. Fig. 1(c) shows r₁ and r₂ relaxivities are determined (from slopes) to be 3.86 and 17.41 s⁻¹ mmol⁻¹ L, respectively, with an r₂/r₁ ratio of 4.51. Although these values are barely comparable to Magnevist (a commercial gadolinium complex, r₁ = 4.6 s⁻¹ mmol⁻¹ L, r₂ = 4.5 s⁻¹ mmol⁻¹ L, r₂/r₁ ∼ 1)^19,20 and much inferior to commercial Feridex (r₂ = 109.4 s⁻¹ mmol⁻¹ L),¹ the optimization of relaxivities and development of new contrast agents are outside the scope of this work.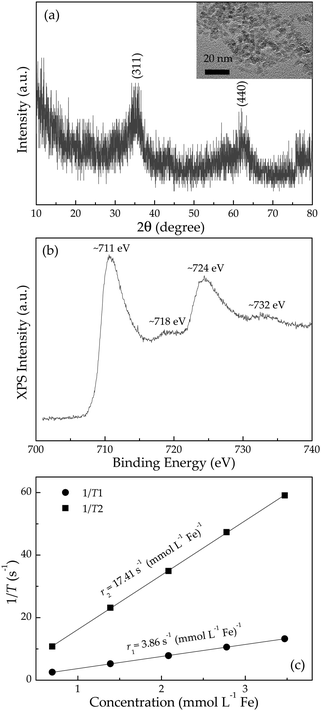

Fig. 1 (a) XRD pattern, TEM image (inset), (b) XPS spectrum and (c) proton longitudinal r₁ and transverse r₂ relaxivities (measured at 1.41 T and 37 °C) of pristine Fe₃O₄/γ-Fe₂O₃ nanoparticles.

After various cation adsorptions, all r₁ relaxivities and most r₂ relaxivities drastically fall into decline (Fig. 2), indicating the degradation of MRI performance of Fe₃O₄/γ-Fe₂O₃ nanoparticles. That is to say, the inevitable adsorption of ions in body fluids in clinical application may well deteriorate the as-designed MRI properties from in vitro tests. In this scenario, the quantitative research on the variation of r₁ and r₂ relaxivities after adsorption of various metallic cations is of fundamental importance to predicting MRI performance for in vivo applications.

Fig. 2 Proton longitudinal r₁ and transverse r₂ relaxivities (measured at 1.41 T and 37 °C) of (a) Ca²⁺, (b) Fe³⁺, (c) Na⁺, (d) Mg²⁺, (e) Zn²⁺, (f) Ni²⁺, (g) Co²⁺, and (h) Cd²⁺ adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles.

Based on observations in Fig. 2, the surface-adsorbed metallic cations form a deterioration layer on relaxivities of Fe₃O₄/γ-Fe₂O₃ nanoparticles. Therefore, simplifications like independency between adsorbed cationic shell and interior iron-oxide core will not yield the accurate results and are full of fallacies. Along this line, it is essential to consider synergetic interactions between adsorbed cations and particle surfaces from a microscopic standpoint, and even to consider stochastic dependence between electrons and ions at a more microcosmic level. We can describe this idea more precisely in the following way: consider an electrostatically adsorbed cation vibrating at an iron-oxide particle surface. It becomes common sense that any three-dimensional vibration can always be decomposed into numerous one-dimensional harmonic vibrations. In this sense, we draw lessons from conclusions in quantum mechanics and use Hermite polynomials, which belong to a classical orthogonal polynomial sequence, to make the association between final relaxivities and a defined parameter Xr/Z of adsorbed cations. Here, X, r and Z denote electronegativity, radius and atomic number of the cation under discussed (Table 1), respectively. The constructed function of Xr/Z-dependent relaxivity distribution is based on the probability density function utilized in Gram–Charlier A series (GCAS) with some modifications. As known, GCAS function can also be expressed by using Hermite polynomials but it is not guaranteed to be positive, and is therefore not a valid probability distribution. It does not converge in many cases of interest, making it not a true asymptotic expansion, because it is not possible to estimate the error of the expansion. Based on the preceding analyses, the relaxivities r_k (k = 1 or 2) can be given by

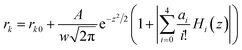wherein z has an expression of (x − x_c)/w (x = Xr/Z), a_i are cumulants and A, w are constants. The low-order Hermite polynomials involved in eqn (1) are H₀ = 1, H₁ = 2z, H₂ = 4z² − 2, H₃ = z³ − 3z, and H₄ = z⁴ − 6z³ + 3.

Table 1 Electronegativity, radius and atomic number of the adsorbed cations

Parameters	Ca^2+	Fe^3+	Na^+	Mg^2+	Zn^2+	Ni^2+	Co^2+	Cd^2+
Atomic number	20	26	11	12	30	28	27	48
Electronegativity	11.30	15.38	9.44	17.13	10.38	9.60	9.10	9.79
Radius (Å)	0.99	0.64	0.95	0.65	0.74	0.72	0.74	0.97

---

By applying eqn (1), the experimental data of both r₁ and r₂ relaxivities for various adsorbed cations can be separately connected with smooth curves, as shown in Fig. 3(a) and (b), respectively. The correlation coefficient R² values of the fitting plots are beyond 0.95, indicating the applicability of eqn (1) to make an association between relaxivities and the characteristic parameter Xr/Z of adsorbed cations. Most strikingly, the cumulants a_i are close to zero at i = 1, 3 in longitudinal case and at i = 0, 2, 4 in transverse case (Table 2). This intriguing finding reminds us of the parity of Hermite polynomials, that is, H_i(−z) = (−1)ⁱH_i(z). Along this line, we boldly propose a general formula of cumulants a_i according to the harmonic oscillator theory in quantum mechanics:

Here, α denotes the coefficient of x in the expression of z. Based on eqn (2), the values of a₀, a₂, and a₄ are calculated to be about 0.75, 0.53 and 0.92, respectively, and the values of a₁ and a₃ are calculated to be 0.53 and 0.65, respectively. Obviously, these calculated values are in excellent consistency with the corresponding fitting values in Table 2, and further validating the applicability of harmonic oscillator theory in our system. In this scenario, the nearly null value of a₁ and a₃ in longitudinal case reveals that the even-parity states of harmonic oscillators are dominant. Likewise, the odd-parity states of harmonic oscillators determines the transverse relaxivities due to a₀ ≈ a₂ ≈ a₄ ≈ 0.

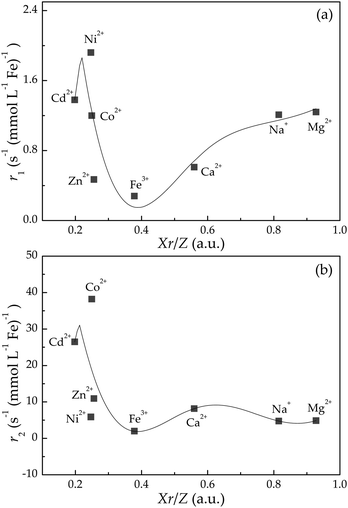Fig. 3 Dependence of (a) r₁ and (b) r₂ values on Xr/Z with regard to various cation-adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles. The experimental data are plotted as solid squares, and the solid lines are fitting results according to eqn (1).

Table 2 Fitting parameters according to eqn (1)

Relaxivity	r k0	A	w	x c	a 0	a 1	a 2	a 3	a 4
Longitudinal	3.95	−1.6 × 10^−3	0.37	0.26	0.73	4.5 × 10^−5	0.53	2.5 × 10^−6	0.89
Transverse	17.86	−19.33	0.38	0.29	1.2 × 10^−3	0.58	1.7 × 10^−6	0.66	8.9 × 10^−8

---

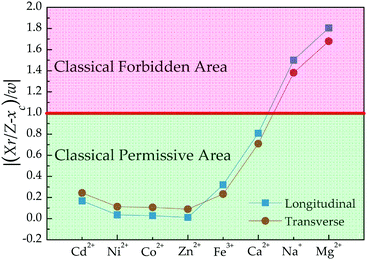
In addition, the quantum mechanics gives the characteristic length (i.e., 1/α) dividing classical forbidden area (|z| > 1/α) and classical permissive area (|z| ≤ 1/α) for harmonic oscillators. In this work, z values can be calculated based on the fitting values of x_c and w according to the equation of z = (Xr/Z − x_c)/w. Fig. 4 shows that z values of Na⁺ and Mg²⁺ cations are beyond 1/α = 1, indicating that the harmonic oscillations of Na⁺ and Mg²⁺ cations around Fe₃O₄/γ-Fe₂O₃ nanoparticles are classical forbidden. By contrast, Ca²⁺, Fe³⁺, Zn²⁺, Ni²⁺, Co²⁺, and Cd²⁺ cations can oscillate around Fe₃O₄/γ-Fe₂O₃ nanoparticles within classical permissive area.

Fig. 4 Dependence of (Xr/Z − x_c)/w values for various cation-adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles.

Experimental

Synthesis and characterization

The pristine Fe₃O₄/γ-Fe₂O₃ nanoparticles were synthesized according to the method reported elsewhere^21,22 with little modification. Typically, 10 mL of Na₂CO₃ aqueous solution (0.6 mol L⁻¹) was added into 50 mL of FeCl₃·6H₂O aqueous solution (0.04 mol L⁻¹) and vigorously stirred for five minutes. After that, ascorbic acid (0.5 g) was added into the above solution and stirred for another ten minutes. Finally, the mixture was transferred into a Teflon-lined stainless-steel autoclave with a capacity of 100 mL for hydrothermal treatment at 160 °C for 3 h. After the autoclave had cooled down to room temperature naturally, the Fe₃O₄/γ-Fe₂O₃ precipitate was separated by centrifugation, washed successively with distilled water and absolute ethanol, and dried in the air at 60 °C for 4 h.

To mimic the actual element concentration in human body, the concentration of Ca²⁺, Fe³⁺, Na⁺, Mg²⁺, Zn²⁺, Ni²⁺, Co²⁺, and Cd²⁺ cations was uniformly set to be 2 mmol L⁻¹. In a typical adsorption experiment, eight sets of 50 mg of the as-prepared Fe₃O₄/γ-Fe₂O₃ nanoparticles were separately soaked into 100 mL of CaCl₂, FeCl₃, NaCl, MgCl₂, ZnCl₂, NiCl₂, CoCl₂, and CdCl₂ aqueous solutions for 1 h. After the above adsorption, all precipitates were separated by centrifugation, washed successively with distilled water and absolute ethanol, and dried in the air at 60 °C for 4 h.

The X-ray diffraction (XRD) pattern of the sample was recorded with a Rigaku D/max-γB diffractometer equipped with a rotating anode and a Cu Kα source (λ = 0.154056 nm) at 40 kV and 150 mA between 10° and 80° (2θ) with a step size of 0.02°. The phase composition was further confirmed by X-ray photoelectron spectroscopy (XPS), which was performed on VG ESCALAB 220i-XL system. The morphology and structure of the sample was characterized by transmission-electron microscope (TEM) with an operating voltage of 200 kV (JEM-2100). The zeta potential values of the samples were determined by dynamic light scattering (DLS, Zetasizer Nano, Malvern Instrument). Iron concentrations in relaxivity experiments were determined by inductively coupled plasma-optic emission spectrometry (ICP-OES, PerkinElmer Optima 8000).

Relaxivity measurement

A mq-60 NMR analyzer (Bruker Minispec) was applied to measure proton transverse (r₂) and longitudinal (r₁) relaxivities of the samples. Specifically, a certain amount of sample was dissolved in deionized water at 37 °C and laid in a magnetic field of 1.41 T with a frequency of 60 MHz. Then a Carr–Purcell–Meiboom–Gill spin-echo pulse sequence was adopted and the interecho time and repetition time were set to be 1 ms and 6 s, respectively. The as-measured signal data was fitted by a monoexponential decay curve to yield the T₂ relaxation time. On the other hand, an inversion recovery (IR) pulse sequence was adopted by using 10 inversion times between 0.05 and 10 s. Then a monoexponential recovery curve was utilized to fit the signal data to finally obtain T₁ relaxation time. All data were presented as arithmetic means of 3 replicates. After the measurements of T₁ and T₂, the relaxivities r₁ and r₂ could be obtained by fitting respective relaxation rate (s⁻¹) versus iron concentration (mmol L⁻¹ Fe).

Conclusions

In summary, Ca²⁺, Fe³⁺, Na⁺, Mg²⁺, Zn²⁺, Ni²⁺, Co²⁺, and Cd²⁺ cation-adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles are synthesized by a facile soaking method to mimic actual element adsorption in human body. The r₁ and r₂ relaxivities of bare Fe₃O₄/γ-Fe₂O₃ nanoparticles are 3.86 and 17.41 s⁻¹ mmol⁻¹ L, respectively. However, after cation adsorption, nearly all relaxivity values show a great decrease, manifesting surface-adsorbed cations greatly degenerate the MRI capability of iron-oxide contrast agents. Such relaxivity loss can be described by a modified GCAS function, taking into account of numerous harmonic oscillations of various cations around Fe₃O₄/γ-Fe₂O₃ nanoparticles. The quantum mechanics analyses show that even-parity and odd-parity states of harmonic oscillators are dominant in r₁ and r₂ relaxivities of cation-adsorbed Fe₃O₄/γ-Fe₂O₃ nanoparticles, respectively. Moreover, the harmonic oscillations of Na⁺ and Mg²⁺ cations around Fe₃O₄/γ-Fe₂O₃ nanoparticles are found to be classical forbidden, which are quite different from their counterparts located in the classical permissive area.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The authors are grateful to the financial aid from the National Natural Science Foundation of China (NSFC No. 51472133).

Notes and references

1. T. Shin, J. Choi, S. Yun, I. Kim, H. Song, Y. Kim, K. I. Park and J. Cheon, ACS Nano, 2014, 8, 3393.
2. J. Y. Park, M. J. Baek, E. S. Choi, S. Woo, J. H. Kim, T. J. Kim, J. C. Jung, K. S. Chae, Y. Chang and G. H. Lee, ACS Nano, 2009, 3, 3663.
3. Q. Wang, A. Xiao, Y. Liu, Q. Zou, Q. Zhou, H. Wang, X. Yang, C. Zheng, Y. Yang and Y. Zhu, Nanomedicine, 2018, 14, 2551.
4. Y. Li, Y. Chang, R. Yuan and Y. Chai, ACS Appl. Mater. Interfaces, 2018, 10, 25213.
5. I. Monaco, P. Armanetti, E. Locatelli, A. Flori, M. Maturi, S. Del Turco, L. Menichetti and M. C. Franchini, J. Mater. Chem. B, 2018, 6, 2993.
6. Z. Zhao, C. Sun, J. Bao, L. Yang, R. Wei, J. Cheng, H. Lina and J. Gao, J. Mater. Chem. B, 2018, 6, 401.
7. H. B. Na, I. C. Song and T. Hyeon, Adv. Mater., 2009, 21, 2133.
8. Q. A. Pankhurst, J. Connolly, S. K. Jones and J. Dobson, J. Phys. D: Appl. Phys., 2003, 36, R167.
9. H. U. Ahmed, A. Kirkham, M. Arya, R. Illing, A. Freeman, C. Allen and M. Emberton, Nat. Rev. Clin. Oncol., 2009, 6, 197.
10. J. W. M. Bulte and D. L. Kraitchman, NMR Biomed., 2004, 17, 484.
11. W. Liu, H. Dahnke, E. K. Jordan, T. Schaeffter and J. A. Frank, NMR Biomed., 2008, 21, 242.
12. C. A. Helms, Am. J. Roentgenol., 1999, 173, 234.
13. W. S. Seo, J. H. Lee, X. Sun, Y. Suzuki, D. Mann, Z. Liu, M. Terashima, P. C. Yang, M. V. McConnell and D. G. Nishimura, Nat. Mater., 2006, 5, 971.
14. C. Richard, B. T. Doan, J. C. Beloeil, M. Bessodes, E. Tóth and D. Scherman, Nano Lett., 2008, 8, 232.
15. J. Shin, R. M. Anisur, M. K. Ko, G. H. Im, J. H. Lee and I. S. Lee, Angew. Chem., Int. Ed., 2009, 48, 321.
16. J. S. Choi, J. H. Lee, T. H. Shin, H. T. Song, E. Y. Kim and J. Cheon, J. Am. Chem. Soc., 2010, 132, 11015.
17. Z. Li, J. F. Godsell, J. P. O'Byrne, N. Petkov, M. A. Morris, S. Roy and J. D. Holmes, J. Am. Chem. Soc., 2010, 132, 12540.
18. T. Yamashita and P. Hayes, Appl. Surf. Sci., 2008, 254, 2441.
19. T. Kim, E. Momin, J. Choi, K. Yuan, H. Zaidi, J. Kim, M. Park, N. Lee, M. T. McMahon, A. Quinones-Hinojosa, J. W. M. Bulte, T. Hyeon and A. A. Gilad, J. Am. Chem. Soc., 2011, 133, 2955.
20. W. A. Seo, J. H. Lee, X. Sun, Y. Suzuki, D. Mann, Z. Liu, M. Terashima, P. C. Yang, M. V. McConnell, D. G. Nishimura and H. Dai, Nat. Mater., 2006, 5, 971.
21. J. Ma, L. Cheng and K. Chen, J. Appl. Phys., 2015, 117, 154701.
22. J. Ma and K. Chen, Sci. Rep., 2017, 7, 42312.

---