Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
One-dimensional vs. two-dimensional proton transport processes at solid–liquid zinc-oxide–water interfaces†
Matti
Hellström
 ab,
Vanessa
Quaranta
ab,
Vanessa
Quaranta
 b and
Jörg
Behler
b and
Jörg
Behler
 *ab
*ab
aUniversität Göttingen, Institut für Physikalische Chemie, Theoretische Chemie, Tammannstr. 6, 37077 Göttingen, Germany. E-mail: joerg.behler@uni-goettingen.de
bLehrstuhl für Theoretische Chemie, Ruhr-Universität Bochum, 44780 Bochum, Germany
First published on 5th November 2018
Abstract
Long-range charge transport is important for many applications like batteries, fuel cells, sensors, and catalysis. Obtaining microscopic insights into the atomistic mechanism is challenging, in particular if the underlying processes involve protons as the charge carriers. Here, large-scale reactive molecular dynamics simulations employing an efficient density-functional-theory-based neural network potential are used to unravel long-range proton transport mechanisms at solid–liquid interfaces, using the zinc oxide–water interface as a prototypical case. We find that the two most frequently occurring ZnO surface facets, (10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and (11
0) and (11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), that typically dominate the morphologies of zinc oxide nanowires and nanoparticles, show markedly different proton conduction behaviors along the surface with respect to the number of possible proton transfer mechanisms, the role of the solvent for long-range proton migration, as well as the proton transport dimensionality. Understanding such surface-facet-specific mechanisms is crucial for an informed bottom-up approach for the functionalization and application of advanced oxide materials.
0), that typically dominate the morphologies of zinc oxide nanowires and nanoparticles, show markedly different proton conduction behaviors along the surface with respect to the number of possible proton transfer mechanisms, the role of the solvent for long-range proton migration, as well as the proton transport dimensionality. Understanding such surface-facet-specific mechanisms is crucial for an informed bottom-up approach for the functionalization and application of advanced oxide materials.
Introduction
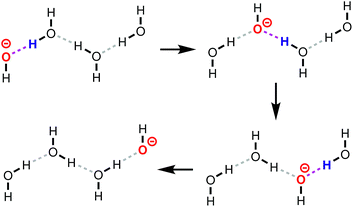
Proton transfer (PT) is the process in which a proton (H+) is transferred from one molecule to another. PT reactions play an important role for acid/base chemistry, heterogeneous and homogeneous catalysis, corrosion, biochemistry, and applications like proton exchange membrane fuel cells, where the protons act as charge carriers.The term proton transport is often used to refer to the long-scale diffusion of protons in a system. In aqueous solutions containing hydronium (H3O+) and hydroxide (OH−) ions, proton transport proceeds via the Grotthuss mechanism (reviewed in, for example, ref. 1), in which charge and mass transport are largely decoupled. A schematic representation of this mechanism for OH−(aq) is given in Fig. 1, where protons are transferred from water molecules to hydroxide ions. One sometimes uses a different perspective, namely that proton “holes”, i.e., missing protons, are transferred from the hydroxide ion to the water molecule.2,3 The Grotthuss mechanism then becomes a series of proton hole transfer events. The transport mechanisms of protons and hydroxide ions in aqueous solution have been extensively studied,2–9 but only little is known about the proton transport mechanisms at solid–liquid interfaces. For instance, recent molecular dynamics (MD) studies10,11 have shown that OH− diffuses both vehicularly (mass transport) and via a Grotthuss-like mechanism in anion exchange membranes. Further, Muñoz-Santiburcio and Marx3 explored OH− diffusion in nanoconfined slit pores, and concluded that the transport mechanism depends on the degree of confinement. Achtyl et al.12 showed that protons can diffuse through hydroxyl-terminated atomic defects in single-layer graphene.
Metal oxides are abundant in the environment and have found many applications in chemistry. On metal oxide surfaces, water molecules often spontaneously dissociate, for example by transferring a proton to the oxide ion of the substrate. The populations of dissociated and molecular water molecules depend on the substrate, the presence or absence of defects, the coverage of water, the pH, and many other factors. Often, a mixed molecular/dissociated water layer forms at the surface.
A typical metal oxide with mixed molecular/dissociated water adsorption is zinc oxide, ZnO. The interface between ZnO and water appears in, for example, medicinal chemistry,13 biosensors14 as well as pH sensors,15 and photocatalysts.16–18 Zinc oxide nanoparticles can be selectively grown into many different shapes and sizes.19 The most common crystal structure of ZnO is hexagonal wurtzite (space group P63mc), in which each Zn2+ and O2− ion is approximately tetrahedrally coordinated. The c crystal direction, with Miller indices [0001], is polar. Thus, the [0001] and [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ] crystal directions are not equivalent. The polar ±(0001) surfaces are less stable than the two most stable nonpolar (10
] crystal directions are not equivalent. The polar ±(0001) surfaces are less stable than the two most stable nonpolar (10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and (11
0) and (11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces:20,21 for this reason, ZnO nanowires are normally longer along the ±[0001] crystal directions, exposing mainly (10
0) surfaces:20,21 for this reason, ZnO nanowires are normally longer along the ±[0001] crystal directions, exposing mainly (10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and/or (11
0) and/or (11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surface facets.19 The present work addresses proton transfer and proton transport at those two surfaces of ZnO.
0) surface facets.19 The present work addresses proton transfer and proton transport at those two surfaces of ZnO.
The interaction of water with ZnO surfaces has been the subject of numerous theoretical studies, for water coverages ranging from sub-monolayer (sub-ML),22–24 to one22,23,25–28 and a few MLs,24,29,30 to the liquid.31–35 In general, it has been found that an increase of the water coverage leads to a greater degree of water dissociation at the surface. Water molecules adsorbed on surface Zn ions (Zns2+), here denoted O*H2, can dissociate and recombine by transferring a proton to/from a nearby surface oxide ion (Os2−):
| OsH− + O*H− ⇌ Os2− + O*H2 | (1) |
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), numerous studies32–34 have indicated that PT can also happen between an adsorbed hydroxide ion (O*H−) and an adsorbed water molecule (“adlayer-PT”):
0), numerous studies32–34 have indicated that PT can also happen between an adsorbed hydroxide ion (O*H−) and an adsorbed water molecule (“adlayer-PT”):| O*H2 + O*H− ⇌ O*H− + O*H2 | (2) |
Our previous work34 investigated the above two types of PT mechanisms at the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0)–liquid-water interface using MD simulations. We found that at ZnO(10
0)–liquid-water interface using MD simulations. We found that at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), the rate of adlayer-PT is greater than the rate of surface-PT. Moreover, we and others33 found that the PT reactions are aided by hydrogen-bond fluctuations in the immediate environment around the dissociating water molecules, similar to PT reactions in NaOH solutions of high concentrations.36
0), the rate of adlayer-PT is greater than the rate of surface-PT. Moreover, we and others33 found that the PT reactions are aided by hydrogen-bond fluctuations in the immediate environment around the dissociating water molecules, similar to PT reactions in NaOH solutions of high concentrations.36
On other metal oxides, such as TiO2,37 ZrO2,38 Fe2O3 (ref. 39) and CeO2,40 and other materials like GaN,41 InP,42 and GaP,42 it has been demonstrated that also solvent water molecules, i.e., water molecules that are not directly adsorbed on the surface, can participate in proton transfer reactions near the interface. In this work, we will show that such solvent-assisted PT reactions are possible also at the ZnO–water interface.
Although the mechanisms governing single PT events have been extensively studied at a number of metal oxides, much less is known about how, and to what extent, multiple subsequent PT events at the solid–liquid interface collectively contribute to long-range proton transport or proton diffusion as we will call it from now on. The distinction between single and multiple events is important, as multiple proton transfer events do not necessarily lead to any proton diffusion, since protons can jump back and forth, or “rattle”, between the same pair of donors/acceptors multiple times. Nevertheless, proton diffusion via the Grotthuss mechanism consists of a series of concatenated PT events. To what extent the short-range local structure, crystalline long-range order, and structural anisotropies of metal oxide surfaces determine the possible pathways for proton transport at the solid–liquid interface, is still completely unknown. Here, we will address these points by obtaining atomic-level insights into the structure and dynamics of the ZnO–liquid-water interface using molecular dynamics simulations.
Proton diffusion at the metal-oxide–liquid-water interface is a challenging case for molecular simulation methods, since the underlying framework must be capable of describing with high accuracy an ionic crystal, a molecular liquid, the interface between them, as well as proton transfer events. Moreover, in order to minimize the influence of finite-size effects, which can be particularly pronounced for diffusion phenomena,43 a large system, both with respect to the area of the interface, as well as the thickness of the liquid phase, is needed. At the same time, although individual proton transfer events can be quite fast, long-scale proton diffusion is potentially much slower, thus requiring long trajectories. For these reasons, a computationally efficient method is needed. In this work, we use a reactive density-functional-theory-based high-dimensional neural network potential,44,45 which provides a computationally inexpensive way of evaluating the total energy and atomic forces in a system maintaining first principles accuracy. Neural networks, one of the most widely used machine learning techniques, are in many ways ideal for simulating complicated processes like proton diffusion at solid–liquid interfaces, since they can be parameterized to reproduce density-functional-theory-calculated potential energy surfaces of arbitrary systems very accurately at a fraction of the computational cost:44,45 in a previous work, we created and validated such a neural network potential for ZnO–liquid-water interfaces.34 The training and validation sets included ample numbers of structures for both the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces in contact with liquid water. Additional validation of the neural network was performed by comparing, for example, proton transfer free energy landscapes as calculated by the neural network MD simulations to landscapes calculated directly from ab initio MD simulations for small systems (see ref. 34, as well as the ESI† to the present work). The comparison to ab initio MD revealed satisfactory agreement between the NN and the DFT results, although the NN proton transfer barriers were somewhat underestimated compared to the DFT reference. Moreover, in ref. 34 we highlighted that running a short 25 ps trajectory was not enough for equilibrating the proton transfer free energy landscapes, thus further justifying the need for an atomistic potential (in our case a neural network potential) that can be used to tackle large length and time scales.
0) surfaces in contact with liquid water. Additional validation of the neural network was performed by comparing, for example, proton transfer free energy landscapes as calculated by the neural network MD simulations to landscapes calculated directly from ab initio MD simulations for small systems (see ref. 34, as well as the ESI† to the present work). The comparison to ab initio MD revealed satisfactory agreement between the NN and the DFT results, although the NN proton transfer barriers were somewhat underestimated compared to the DFT reference. Moreover, in ref. 34 we highlighted that running a short 25 ps trajectory was not enough for equilibrating the proton transfer free energy landscapes, thus further justifying the need for an atomistic potential (in our case a neural network potential) that can be used to tackle large length and time scales.
Here, we investigate proton transfer and proton diffusion at the two most prevalent surface facets of ZnO particles, namely ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
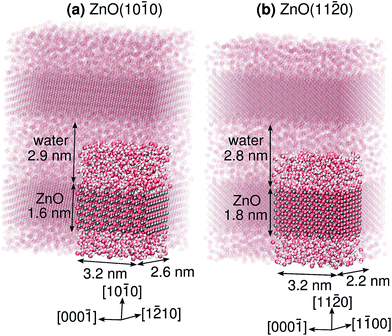
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), in contact with a thick liquid water film. The slab models for these two systems are shown in Fig. 2. In both cases, the polar ±[0001] directions run parallel to the surface. We will for the first time decipher (i) differences and similarities between the individual PT mechanisms (short-range proton transfer) and how they relate to the structures of the two surfaces, (ii) to what extent the PT barriers along the polar [0001] and [000
0), in contact with a thick liquid water film. The slab models for these two systems are shown in Fig. 2. In both cases, the polar ±[0001] directions run parallel to the surface. We will for the first time decipher (i) differences and similarities between the individual PT mechanisms (short-range proton transfer) and how they relate to the structures of the two surfaces, (ii) to what extent the PT barriers along the polar [0001] and [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ] directions differ, and (iii) whether there are preferred proton diffusion directions along the surface. We will demonstrate that proton diffusion, i.e., long-range proton transport, on ZnO(10
] directions differ, and (iii) whether there are preferred proton diffusion directions along the surface. We will demonstrate that proton diffusion, i.e., long-range proton transport, on ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) is “pseudo-one-dimensional” with hardly any diffusion along the polar crystal directions, whereas it is two-dimensional on ZnO(11
0) is “pseudo-one-dimensional” with hardly any diffusion along the polar crystal directions, whereas it is two-dimensional on ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), with significant diffusion along both the polar and non-polar crystal directions.
0), with significant diffusion along both the polar and non-polar crystal directions.
Results
The ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces are mixed-terminated, meaning that they expose an equal amount of Zn2+ and O2− ions in the outermost surface layer. As compared to the atoms in the bulk, the atoms in the surface layer have each lost one of their four nearest neighbors. For ZnO(10
0) surfaces are mixed-terminated, meaning that they expose an equal amount of Zn2+ and O2− ions in the outermost surface layer. As compared to the atoms in the bulk, the atoms in the surface layer have each lost one of their four nearest neighbors. For ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), only one of the remaining three nearest neighbors is in the surface layer (the remaining two are in the subsurface layer), whereas for ZnO(11
0), only one of the remaining three nearest neighbors is in the surface layer (the remaining two are in the subsurface layer), whereas for ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), two of the nearest neighbors are in the surface layer (and one in the subsurface layer). Thus, the ZnO(10
0), two of the nearest neighbors are in the surface layer (and one in the subsurface layer). Thus, the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) surface consists of an array of isolated Zn–O “surface dimers” in which two surface Zn atoms are only connected via a nearest neighbor O in the subsurface layer. On the contrary, the ZnO(11
0) surface consists of an array of isolated Zn–O “surface dimers” in which two surface Zn atoms are only connected via a nearest neighbor O in the subsurface layer. On the contrary, the ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surface consists of extended zig-zagged “surface rows” that consist of nearest-neighbors within in the surface layer; these surface rows extend along the polar ±[0001] directions. The rows are separated by trenches and connected by atoms in the subsurface layer.
0) surface consists of extended zig-zagged “surface rows” that consist of nearest-neighbors within in the surface layer; these surface rows extend along the polar ±[0001] directions. The rows are separated by trenches and connected by atoms in the subsurface layer.
Similar to the perspective of “proton hole” diffusion for exploring the Grotthuss mechanism of OH− diffusion in water, we here adopt the convention of exploring “proton hole diffusion” at the ZnO–water interface. To this end, we define “proton hole centers” (PHCs) to be typical proton acceptors, i.e. “free” (unprotonated) surface oxide ions, Os2−, as well as the combination of adsorbed hydroxide ions (O*H−) and the corresponding surface Zn ion. We use the term proton hole in this context simply as an indicator for a “missing” proton; our use of this term does not imply that the proton hole diffusion mechanism on the surface, which will be explored below, is equivalent to the archetypal proton hole diffusion mechanism for OH− in water (for a full account of the differences between proton and proton hole diffusion mechanisms in water, see ref. 1). In our analysis, we could in principle have explored proton diffusion instead, but this would have led to rather complicated definitions of “proton centers” (see ESI†).
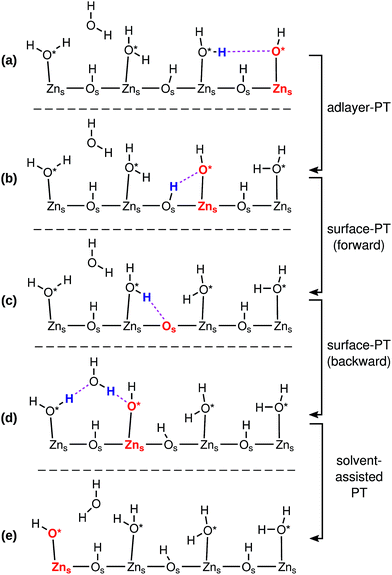
Fig. 3 indicates how a PHC (red) can diffuse on a ZnO surface via a series of PT events. The ZnO surface is depicted only schematically; the true two and three-dimensional structures of the surfaces influence the possible sequence of PT events, and the directions in which the PHC diffuses, as we will explore in more detail below.
Fig. 3 illustrates the four different kinds of PT events that occur at the interface: adlayer-PT (eqn (2)), forward surface-PT (where a water molecule recombines, eqn (1)), backward surface-PT (where a water molecule dissociates, eqn (1)), as well as solvent-assisted PT. Fig. 3 marks both the O in O*H− as well as the Zns2+ on which it is adsorbed as constituting the “proton hole center”. Both viewpoints have advantages, and we will switch between them when convenient. Associating the Zns2+ with the PHC will give us a better overview of the network of proton diffusion pathways on the surface. The surface-PT reaction in eqn (1) can thus also be written as (cf.Fig. 3b–d)
| PHC(Zns2+) ⇌ PHC(Os2−). | (3) |
Similarly, the adlayer-PT (eqn (2)) and solvent-assisted PT reactions can be written as
| PHC(Zns2+{1}) ⇌ PHC(Zns2+{2}) | (4) |
Surface-PT events affect the degree of surface hydroxylation α, which we define as the fraction of surface oxygen species that form a surface hydroxide, OsH−, i.e., 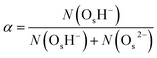 , where N(·) is the number of species. We find that the ZnO(11
, where N(·) is the number of species. We find that the ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surface has a larger degree of hydroxylation (α = 0.764 ± 0.009; the error bar indicates the 95% confidence interval around the mean after block-averaging the hydroxylation level over 20 equal-sized portions of the trajectory) than the ZnO(10
0) surface has a larger degree of hydroxylation (α = 0.764 ± 0.009; the error bar indicates the 95% confidence interval around the mean after block-averaging the hydroxylation level over 20 equal-sized portions of the trajectory) than the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) surface (α = 0.706 ± 0.002); a greater hydroxylation level at the ZnO(11
0) surface (α = 0.706 ± 0.002); a greater hydroxylation level at the ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0)–liquid-water interface was also observed in a previous molecular dynamics study employing a reactive force field.31 For ZnO(10
0)–liquid-water interface was also observed in a previous molecular dynamics study employing a reactive force field.31 For ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), ab initio MD simulations32,33 have predicted a smaller equilibrium hydroxylation level (α ≈ 0.5) than what the neural network potential predicts, which we have previously explained as arising from the different density functionals employed in those simulations compared to ours (with our present neural network potential being parameterized to RPBE-D3 reference data, see also Methods).34
0), ab initio MD simulations32,33 have predicted a smaller equilibrium hydroxylation level (α ≈ 0.5) than what the neural network potential predicts, which we have previously explained as arising from the different density functionals employed in those simulations compared to ours (with our present neural network potential being parameterized to RPBE-D3 reference data, see also Methods).34
Fig. 4 shows the calculated proton transfer free-energy landscapes (PTFELs) for the surface-PT and adlayer-PT coordinates at the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces. Further, top views of the surfaces with only the species participating in the PT reaction are given. The PTFELs give the free-energy barriers ΔF‡ for transferring the proton from the donor to the acceptor, given that the corresponding donor–acceptor pair has already formed and that the proton is deemed active for PT (see Methods). The PTFEL is presented as a one-dimensional function of the PT coordinate δmin, which is the difference between the covalent and noncovalent O–H distances; this geometric viewpoint is sufficient for our present purposes. However, the PTFEL is a many-body function, and other relevant coordinates that can be used to characterize it are, for example, the O–O distances,33 the number of hydrogen bonds that are donated and accepted by the participating species,34,36 and the hydrogen-bonding distances to molecules that are not involved in the PT itself.33 Using both δmin and the O–O distances as coordinates, ESI Fig. 6 and 7† show that the PT barriers are smaller for shorter O–O distances, as previously shown also for many other PT reactions.1,33
0) surfaces. Further, top views of the surfaces with only the species participating in the PT reaction are given. The PTFELs give the free-energy barriers ΔF‡ for transferring the proton from the donor to the acceptor, given that the corresponding donor–acceptor pair has already formed and that the proton is deemed active for PT (see Methods). The PTFEL is presented as a one-dimensional function of the PT coordinate δmin, which is the difference between the covalent and noncovalent O–H distances; this geometric viewpoint is sufficient for our present purposes. However, the PTFEL is a many-body function, and other relevant coordinates that can be used to characterize it are, for example, the O–O distances,33 the number of hydrogen bonds that are donated and accepted by the participating species,34,36 and the hydrogen-bonding distances to molecules that are not involved in the PT itself.33 Using both δmin and the O–O distances as coordinates, ESI Fig. 6 and 7† show that the PT barriers are smaller for shorter O–O distances, as previously shown also for many other PT reactions.1,33
The value of the relative free energy ΔF at the maximum of the PTFEL can be interpreted as a measure of the rate of PT along a particular PT coordinate.36 It is not a measure of the absolute rate (the number of PT events per time unit), but rather, a measure of the relative rate of different PT mechanisms in the same MD trajectory. The assumption is that for a PT event to happen, the PT coordinate δmin must pass through the value where ΔF is the highest (at δmin ≈ 0 Å). A greater value of the free-energy maximum implies a lower PT rate along that particular coordinate. Although the PT barriers can be different for the forward and backward reactions of any PT mechanism, the rates for the forward and backward reactions are equal because of the different population of these states, since the system is in chemical equilibrium.
Fig. 4a shows the surface-PT and adlayer-PT free energies at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0). For a full discussion of those results, we refer the reader to our previous work.34 Here, we simply note, that for ZnO(10
0). For a full discussion of those results, we refer the reader to our previous work.34 Here, we simply note, that for ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0): (i) the rate of adlayer-PT is greater than the rate of surface-PT, (ii) the left and right hand sides (LHS and RHS) of the adlayer-PT coordinate are equivalent, and (iii) for surface-PT, the LHS [dissociated water, PHC(Zns2+)] is more stable than the RHS [molecular water, PHC(Os2−)].
0): (i) the rate of adlayer-PT is greater than the rate of surface-PT, (ii) the left and right hand sides (LHS and RHS) of the adlayer-PT coordinate are equivalent, and (iii) for surface-PT, the LHS [dissociated water, PHC(Zns2+)] is more stable than the RHS [molecular water, PHC(Os2−)].
At the other nonpolar surface, ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) (see Fig. 4b–c), there is greater variety of the possible PT coordinates. For both surface-PT and adlayer-PT, the PHC can be transferred between surface atoms that lie within the same surface row (intra-surface-PT and intra-adlayer-PT), or between surface atoms that lie on different rows (inter-surface-PT and inter-adlayer-PT). The greatest PT rate is obtained for the inter-adlayer-PT coordinate, followed by intra-surface-PT, inter-surface-PT, and finally intra-adlayer-PT.
0) (see Fig. 4b–c), there is greater variety of the possible PT coordinates. For both surface-PT and adlayer-PT, the PHC can be transferred between surface atoms that lie within the same surface row (intra-surface-PT and intra-adlayer-PT), or between surface atoms that lie on different rows (inter-surface-PT and inter-adlayer-PT). The greatest PT rate is obtained for the inter-adlayer-PT coordinate, followed by intra-surface-PT, inter-surface-PT, and finally intra-adlayer-PT.
Unlike what was the case at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) (Fig. 4a), the LHS and RHS of the two adlayer-PT coordinates at ZnO(11
0) (Fig. 4a), the LHS and RHS of the two adlayer-PT coordinates at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) (Fig. 4b) are not equivalent. This is because the direction of adlayer-PT at ZnO(11
0) (Fig. 4b) are not equivalent. This is because the direction of adlayer-PT at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) has a component along the polar [0001] direction of the crystal substrate. The PT barriers for the relatively rare intra-adlayer-PT coordinate depend very strongly on whether the proton is transferred along [0001] or [000
0) has a component along the polar [0001] direction of the crystal substrate. The PT barriers for the relatively rare intra-adlayer-PT coordinate depend very strongly on whether the proton is transferred along [0001] or [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ], while for the more common inter-adlayer-PT coordinate, the direction of PT has less of an influence (amounting to only about 0.2kBT) on the PT barriers.
], while for the more common inter-adlayer-PT coordinate, the direction of PT has less of an influence (amounting to only about 0.2kBT) on the PT barriers.
We also found cases of the “solvent-assisted” proton transfer schematically indicated in Fig. 3d–e. Fig. 5 shows actual snapshots from the MD trajectories for this type of PT. Unlike the PT mechanisms in Fig. 4, which involved the transfer of only a single proton, the solvent-assisted PT involves the concerted transfer of two protons: one proton is donated to the solvent H2O by an adsorbed O*H2, and one proton is donated by the solvent H2O to an adsorbed O*H−. Solvent-assisted PT proceeds via transiently formed solvent OH− and OH3+ species, that are not stable intermediates (ESI Fig. 3 and 5†). The events are quite rare. For example, in the 44 ns long simulation for ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), only 41 solvent-assisted PT events were observed.
0), only 41 solvent-assisted PT events were observed.
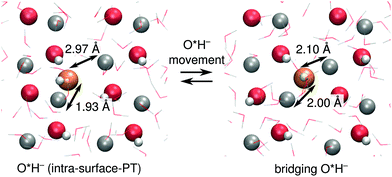
At ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), O*H− in the intra-surface-PT coordinate (Fig. 4c) can move towards the nearest Zns2+ in the neighboring surface row, ending up in a configuration where the O*H− bridges two surface rows (see Fig. 6). Although such bridging species accept a hydrogen bond from OsH−, they are mostly inactive for PT, as the PT reaction from a bridging O*H2 is barrierless (ESI Fig. 4†). Bridging O*H− thus disappear by moving back along [0001] to the intra-surface-PT configuration. About 12% of O*H− at ZnO(11
0), O*H− in the intra-surface-PT coordinate (Fig. 4c) can move towards the nearest Zns2+ in the neighboring surface row, ending up in a configuration where the O*H− bridges two surface rows (see Fig. 6). Although such bridging species accept a hydrogen bond from OsH−, they are mostly inactive for PT, as the PT reaction from a bridging O*H2 is barrierless (ESI Fig. 4†). Bridging O*H− thus disappear by moving back along [0001] to the intra-surface-PT configuration. About 12% of O*H− at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) are in such a bridging configuration. In the coming analysis, we assign the location of the PHC for such bridging configurations to be the location of the O*H−.
0) are in such a bridging configuration. In the coming analysis, we assign the location of the PHC for such bridging configurations to be the location of the O*H−.
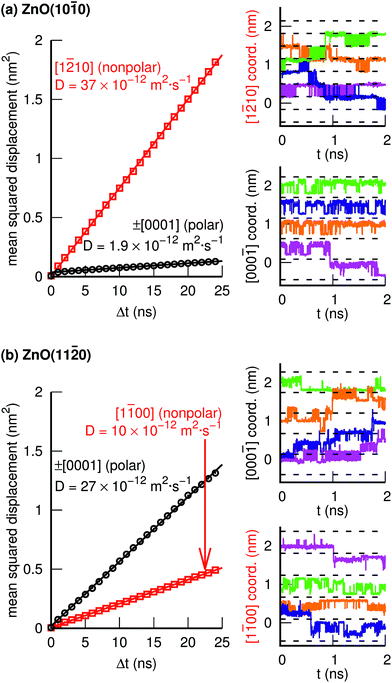
Fig. 4 and 5 illustrate individual PT events, but give no information about if such PT events can be concatenated to yield Grotthuss-like diffusion of the PHC along the surface. In order to explore proton hole diffusion, we followed the positions of the individual PHCs in time and calculated the mean squared displacement projected onto the two primary surface crystal directions (±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] and ±[0001] for ZnO(10
10] and ±[0001] for ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), and ±[1
0), and ±[1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 00] and ±[0001] for ZnO(11
00] and ±[0001] for ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0)). Fig. 7 shows the calculated mean squared displacements and some example trajectories of the PHCs.
0)). Fig. 7 shows the calculated mean squared displacements and some example trajectories of the PHCs.
At ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), the calculated diffusion coefficient along the nonpolar direction [1
0), the calculated diffusion coefficient along the nonpolar direction [1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10],
10], 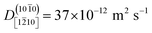 , is 20 times greater than the diffusion coefficient along the polar direction [0001],
, is 20 times greater than the diffusion coefficient along the polar direction [0001], 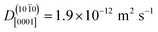 . Here, we note that the latter value is quite uncertain because of the small number of solvent-assisted PT events which contribute to PHC diffusion along [0001] (the small number of such events does not give rise to a “noisy” MSD, since the MSD is averaged over all PHCs); in fact, the biggest contribution to the calculated mean squared displacement along [0001], even for a correlation time of 25 ns (Fig. 7a), comes from individual surface-PT events, which do not contribute to PHC diffusion (see also Discussion). The calculated
. Here, we note that the latter value is quite uncertain because of the small number of solvent-assisted PT events which contribute to PHC diffusion along [0001] (the small number of such events does not give rise to a “noisy” MSD, since the MSD is averaged over all PHCs); in fact, the biggest contribution to the calculated mean squared displacement along [0001], even for a correlation time of 25 ns (Fig. 7a), comes from individual surface-PT events, which do not contribute to PHC diffusion (see also Discussion). The calculated 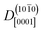 is therefore likely overestimated.
is therefore likely overestimated.
At ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), in contrast, diffusion along the polar directions is dominant:
0), in contrast, diffusion along the polar directions is dominant: 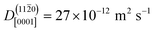 . However, the directionality is not as anisotropic as was the case for the other surface. Diffusion along the polar direction is only about 3 times faster than diffusion along the nonpolar direction:
. However, the directionality is not as anisotropic as was the case for the other surface. Diffusion along the polar direction is only about 3 times faster than diffusion along the nonpolar direction: 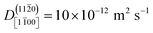 .
.
Discussion
Proton hole center diffusion network
The Grotthuss proton hole transport mechanism for OH− in aqueous solution (Fig. 1) relies on the fact that an OH− can participate in a proton transfer event with multiple (at least two) neighboring H2O molecules. If there were only one neighboring H2O molecule, the proton hole would have reached a “dead end”, forcing the next PT event to form again the previous OH− (barring hydrogen bond fluctuations). In dilute aqueous solutions, OH− accepts hydrogen bonds from three or four different H2O molecules,2 providing different pathways for the Grotthuss mechanism.
Fig. 8 illustrates what we call the “proton hole center diffusion networks” at the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces in contact with liquid water, by graphing all possible pathways that the PHC at any given position can take, for both proton transfer reactions (colored lines) and vehicular movement (black dotted lines). The thicknesses of the colored lines qualitatively indicate the rate of the individual PT processes, with thicker lines indicating greater rates. For lines with gradients, transferring the PHC from the dark end to the light end is associated with a larger barrier than the reverse reaction, as also explained in the dashed rectangles in Fig. 8. There is no PHC diffusion into the bulk liquid water, other than the transient species formed during solvent-assisted PT (which is not explicitly indicated in Fig. 8).
0) surfaces in contact with liquid water, by graphing all possible pathways that the PHC at any given position can take, for both proton transfer reactions (colored lines) and vehicular movement (black dotted lines). The thicknesses of the colored lines qualitatively indicate the rate of the individual PT processes, with thicker lines indicating greater rates. For lines with gradients, transferring the PHC from the dark end to the light end is associated with a larger barrier than the reverse reaction, as also explained in the dashed rectangles in Fig. 8. There is no PHC diffusion into the bulk liquid water, other than the transient species formed during solvent-assisted PT (which is not explicitly indicated in Fig. 8).
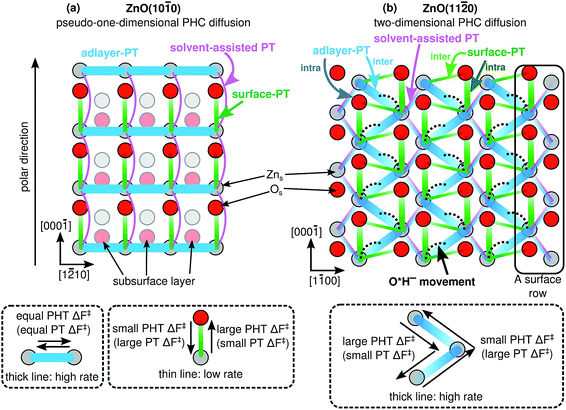 | ||
Fig. 8 Calculated proton hole center (PHC) diffusion networks at the ZnO–liquid-water interface, for (a) the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) surface, and (b) the ZnO(11 0) surface, and (b) the ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surface. The proton transfer (PT) and proton hole transfer (PHT) processes take place between PHCs, that can be located at surface O atoms, surface Zn atoms, or between two surface Zn atoms. Thicker solid lines indicate processes with greater rates. For lines with gradients, transferring the PHC from the dark end to the light end has a greater barrier than the reverse reaction. The dotted lines indicate movement of the PHC (Fig. 6), i.e., a process without proton transfer. 0) surface. The proton transfer (PT) and proton hole transfer (PHT) processes take place between PHCs, that can be located at surface O atoms, surface Zn atoms, or between two surface Zn atoms. Thicker solid lines indicate processes with greater rates. For lines with gradients, transferring the PHC from the dark end to the light end has a greater barrier than the reverse reaction. The dotted lines indicate movement of the PHC (Fig. 6), i.e., a process without proton transfer. | ||
For example, at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), a PHC centered at Zns2+ has five possible next locations: either of the two the neighboring Zns2+ ions along the nonpolar ±[1
0), a PHC centered at Zns2+ has five possible next locations: either of the two the neighboring Zns2+ ions along the nonpolar ±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] direction (thick blue lines, corresponding to adlayer-PT events, cf.Fig. 4a), the neighboring Os2− at greater coordinate along [000
10] direction (thick blue lines, corresponding to adlayer-PT events, cf.Fig. 4a), the neighboring Os2− at greater coordinate along [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ] (green lines, corresponding to surface-PT events, cf.Fig. 4a), or either of the two neighboring Zns2+ along ±[0001] (thin pink lines, corresponding to rare solvent-assisted PT events, cf.Fig. 5a). PHC(Zns2+) thus constitutes a “hub” with several connections to neighboring surface ions, and can therefore potentially contribute to Grotthuss-like PHC diffusion at ZnO(10
] (green lines, corresponding to surface-PT events, cf.Fig. 4a), or either of the two neighboring Zns2+ along ±[0001] (thin pink lines, corresponding to rare solvent-assisted PT events, cf.Fig. 5a). PHC(Zns2+) thus constitutes a “hub” with several connections to neighboring surface ions, and can therefore potentially contribute to Grotthuss-like PHC diffusion at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0). In contrast, PHC(Os2−) constitutes a “dead end” in the sense that a PHC centered at Os2− only has one possible “next location”, namely the neighboring Zns2+ with smaller coordinate along [000
0). In contrast, PHC(Os2−) constitutes a “dead end” in the sense that a PHC centered at Os2− only has one possible “next location”, namely the neighboring Zns2+ with smaller coordinate along [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ]. If the network in Fig. 8a is considered as a mathematical graph, then the PHC(Os2−) vertices have degree 1. Thus, PHC(Os2−) cannot contribute to Grotthuss-like PHC diffusion at ZnO(10
]. If the network in Fig. 8a is considered as a mathematical graph, then the PHC(Os2−) vertices have degree 1. Thus, PHC(Os2−) cannot contribute to Grotthuss-like PHC diffusion at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0).
0).
Fig. 8a reveals the source of the very different calculated PHC diffusion coefficients (Fig. 7a) for diffusion along ±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] (horizontal) and ±[0001] (vertical) at ZnO(10
10] (horizontal) and ±[0001] (vertical) at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0). Diffusion along ±[1
0). Diffusion along ±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] is driven by consecutive adlayer-PT events (thick blue horizontal lines in Fig. 8a). Although the other major type of PT mechanism, surface-PT (green in Fig. 8a), transfers the PHC along the polar ±[0001] direction, it gives no long-range diffusion along ±[000
10] is driven by consecutive adlayer-PT events (thick blue horizontal lines in Fig. 8a). Although the other major type of PT mechanism, surface-PT (green in Fig. 8a), transfers the PHC along the polar ±[0001] direction, it gives no long-range diffusion along ±[000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ], since surface-PT transfers the PHC between Zns2+ and Os2− (eqn (3)), and PHC(Os2−) constitutes a dead end. Thus, diffusion along the polar ±[0001] direction can only happen via the solvent-assisted PT mechanism (Fig. 5), which we found to be very rare. The “jump” at around t = 1 ns for the pink PHC trajectory along [000
], since surface-PT transfers the PHC between Zns2+ and Os2− (eqn (3)), and PHC(Os2−) constitutes a dead end. Thus, diffusion along the polar ±[0001] direction can only happen via the solvent-assisted PT mechanism (Fig. 5), which we found to be very rare. The “jump” at around t = 1 ns for the pink PHC trajectory along [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ] in Fig. 7a corresponds to such a solvent-assisted PT event (depicted in Fig. 5a). However, for the vast majority of the time, the PHC positions simply oscillated between the Os2− and Zns2+ positions on neighboring surface “dimers” (see for example the green, blue, and orange trajectories along [000
] in Fig. 7a corresponds to such a solvent-assisted PT event (depicted in Fig. 5a). However, for the vast majority of the time, the PHC positions simply oscillated between the Os2− and Zns2+ positions on neighboring surface “dimers” (see for example the green, blue, and orange trajectories along [000![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ] for ZnO(10
] for ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) in Fig. 7a). In contrast, along the nonpolar [1
0) in Fig. 7a). In contrast, along the nonpolar [1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] direction, several trajectories spanned several nm; in the examples in Fig. 7a, the PHCs indicated by the green and blue lines diffused about 1 nm (or about three lattice parameters along [1
10] direction, several trajectories spanned several nm; in the examples in Fig. 7a, the PHCs indicated by the green and blue lines diffused about 1 nm (or about three lattice parameters along [1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10]) in 2 ns.
10]) in 2 ns.
We thus conclude that the proton hole diffusion at the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/i_char_0031_0304.gif) 0)–water interface is pseudo-one-dimensional, with a high rate of adlayer-PT events yielding diffusion along the nonpolar ±[1
0)–water interface is pseudo-one-dimensional, with a high rate of adlayer-PT events yielding diffusion along the nonpolar ±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] direction, and a very low rate of solvent-assisted PT events contributing to the relatively small diffusion along the polar [0001] direction.
10] direction, and a very low rate of solvent-assisted PT events contributing to the relatively small diffusion along the polar [0001] direction.
At ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), the proton hole center diffusion network (Fig. 8b) is in several ways different to what is the case at ZnO(10
0), the proton hole center diffusion network (Fig. 8b) is in several ways different to what is the case at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0). Firstly, PHC(Os2−) does not constitute a “dead end”, since there are two possible next locations of the PHC, corresponding to intra-surface-PT and inter-surface-PT events, respectively. Consequently, PHC(Os2−) can contribute to Grotthuss-like diffusion of the PHC. Moreover, the black dotted lines indicate the O*H− movement from Fig. 6. However, vehicular O*H− movement does not contribute to any long-scale diffusion of the PHC, since the bridging O*H− species (Fig. 6) are very inactive for PT (ESI Fig. 4†).
0). Firstly, PHC(Os2−) does not constitute a “dead end”, since there are two possible next locations of the PHC, corresponding to intra-surface-PT and inter-surface-PT events, respectively. Consequently, PHC(Os2−) can contribute to Grotthuss-like diffusion of the PHC. Moreover, the black dotted lines indicate the O*H− movement from Fig. 6. However, vehicular O*H− movement does not contribute to any long-scale diffusion of the PHC, since the bridging O*H− species (Fig. 6) are very inactive for PT (ESI Fig. 4†).
The PT process at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) with the highest rate is inter-adlayer-PT (Fig. 4b), and Fig. 8b reveals that the concatenation of such PT events can yield a “zig-zag-like” diffusion of the PHC with net movement along the polar ±[0001] direction. For each individual inter-adlayer-PT event, the barrier to transfer the PHC from the dark end to the light end (downwards in the figure) is greater than the reverse process (see also Fig. 4). For the purpose of long-scale diffusion via the Grotthuss mechanism, i.e., concatenated PT events, once a PHC has been transferred (for example one step downwards in the figure), there is an energy barrier associated with changing the proton transfer coordinate so that the next PT event along the same direction (as opposed to the opposite direction) can occur. For this reason, there is no perpetual energy gain from transferring the PHC along the polar direction. Instead, the amount of long-scale diffusion along both polar directions (up and down) are equivalent, since the system is in equilibrium.
0) with the highest rate is inter-adlayer-PT (Fig. 4b), and Fig. 8b reveals that the concatenation of such PT events can yield a “zig-zag-like” diffusion of the PHC with net movement along the polar ±[0001] direction. For each individual inter-adlayer-PT event, the barrier to transfer the PHC from the dark end to the light end (downwards in the figure) is greater than the reverse process (see also Fig. 4). For the purpose of long-scale diffusion via the Grotthuss mechanism, i.e., concatenated PT events, once a PHC has been transferred (for example one step downwards in the figure), there is an energy barrier associated with changing the proton transfer coordinate so that the next PT event along the same direction (as opposed to the opposite direction) can occur. For this reason, there is no perpetual energy gain from transferring the PHC along the polar direction. Instead, the amount of long-scale diffusion along both polar directions (up and down) are equivalent, since the system is in equilibrium.
Similarly, the PT processes with the second and third highest rates, namely intra-surface-PT and inter-surface-PT, can also be concatenated to yield net diffusion along the polar ±[0001] direction (“rectangular” diffusion along the green lines in Fig. 8b). Diffusion along ±[0001] thus occurs when the PHC alternately jumps back and forth between two surface rows. Net diffusion along the nonpolar ±[1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 00] directions occurs via intra-adlayer-PT events and solvent-assisted PT events (thin blue and pink lines in Fig. 8b). Such PT events transfer the PHC from one Zns2+ to another Zns2+ on the “other side” of the same surface row. Unlike the case at ZnO(10
00] directions occurs via intra-adlayer-PT events and solvent-assisted PT events (thin blue and pink lines in Fig. 8b). Such PT events transfer the PHC from one Zns2+ to another Zns2+ on the “other side” of the same surface row. Unlike the case at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), it is not only solvent-assisted PT events that enable PHC diffusion along the “minor” direction, but intra-adlayer-PT events also contribute to such diffusion. For this reason, it is not surprising that the one-dimensional diffusion coefficients at ZnO(11
0), it is not only solvent-assisted PT events that enable PHC diffusion along the “minor” direction, but intra-adlayer-PT events also contribute to such diffusion. For this reason, it is not surprising that the one-dimensional diffusion coefficients at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) in both directions are much more similar in magnitude (differing only by a factor of 3, Fig. 7b) than what is the case at ZnO(10
0) in both directions are much more similar in magnitude (differing only by a factor of 3, Fig. 7b) than what is the case at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0).
0).
Thus, although the PHC diffusion at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) is anisotropic, the similar magnitude of the one-dimensional diffusion coefficients lead us to the conclusion that PHC diffusion at ZnO(11
0) is anisotropic, the similar magnitude of the one-dimensional diffusion coefficients lead us to the conclusion that PHC diffusion at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/i_char_0032_0304.gif) 0) is two-dimensional.
0) is two-dimensional.
Influence of pH
The proton hole diffusion coefficients, or equivalently, the proton diffusion coefficients, give a measure of the proton conductivity at the interface. In this work, the substrate (ZnO) is in contact with pure liquid water. This can be contrasted to previous simulation work exploring long-range proton and proton hole diffusion at solid/liquid interfaces (for example ref. 3, 10 and 11), where the diffusing species was explicitly added to the system. In such simulations, the substrate can interact with the diffusing species by influencing its preferred direction of diffusion, as well as aiding or inhibiting Grotthuss-like diffusion (as opposed to vehicular diffusion), but the surface has not “created” the diffusing species from reactions with the solvent. In the present work, we did not introduce any additional (“extrinsic”) protons or proton holes to the system, but instead followed the proton hole diffusion arising from the spontaneous dissociation and recombination of water on the surface. As a result, there is no net charge on the surface, and the present results for proton hole diffusion effectively concern ZnO surfaces at the point of zero charge (pzc), in the absence of specifically adsorbed counterions. The pzc of ZnO particles depends on the method of preparation, but is normally found at slightly basic pH, around pH 9.46 A higher pH would result in more deprotonation of OsH− and O*H2 groups, and a lower pH in the protonation of Os2− and O*H− groups. Thus, the concentrations of species that contribute to proton conductivity at the interface depend on the pH. Adlayer-PT, which is the major contributor to PHC diffusion at both ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), requires that a mixture of O*H− and O*H2 be present at the interface; thus, the pH may neither be too low nor too high for proton conduction to be efficient using the mechanisms presented in this work. Such pH-dependent differences in the physical behavior of the interface is of great interest for example for the application of ZnO-based materials as pH sensors.15 At low pH, other proton transfer mechanisms may become important; for example, proton conduction could potentially occur via O*H3+, which in our simulations at the pzc only transiently forms (ESI Fig. 2–5†). Characterizing the proton diffusion network at different pH values would serve as an excellent avenue for further exploration.
0), requires that a mixture of O*H− and O*H2 be present at the interface; thus, the pH may neither be too low nor too high for proton conduction to be efficient using the mechanisms presented in this work. Such pH-dependent differences in the physical behavior of the interface is of great interest for example for the application of ZnO-based materials as pH sensors.15 At low pH, other proton transfer mechanisms may become important; for example, proton conduction could potentially occur via O*H3+, which in our simulations at the pzc only transiently forms (ESI Fig. 2–5†). Characterizing the proton diffusion network at different pH values would serve as an excellent avenue for further exploration.
It is also possible for the surface to affect the pH of the surrounding solvent. The surface acidity can, in principle, be evaluated from the kinds of simulations that were performed in this work. For example, Wang et al.41 performed ab initio MD simulations for the GaN(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0)–water interface and calculated that surface to have pKa = 3.0 ± 0.1, by considering the free-energy proton transfer barrier from the surface into the bulk liquid. However, in the present work, we did not observe the transfer of any PHCs, other than those during transient solvent-assisted PT events, into the bulk liquid. For this reason, we cannot explicitly evaluate the surface acidity from the present simulations.
0)–water interface and calculated that surface to have pKa = 3.0 ± 0.1, by considering the free-energy proton transfer barrier from the surface into the bulk liquid. However, in the present work, we did not observe the transfer of any PHCs, other than those during transient solvent-assisted PT events, into the bulk liquid. For this reason, we cannot explicitly evaluate the surface acidity from the present simulations.
Implications for photocatalysis and nanomaterial design
The short-range and long-range proton transport properties of a surface are of fundamental importance to, for example, photoelectrochemistry and corrosion. In a recent first-principles simulation-based work, Wood et al.42 investigated short-range proton transfer reactions at the InP(001) and GaP(001) surfaces in contact with water, and provided an excellent discussion about how water diffusion and proton transfer reactivity determine the photoelectrochemical hydrogen evolution activity of these materials. In particular, the point on the surface at which a reactant (e.g., H+ or H) forms need not be the same as the point at which it participates in the hydrogen evolution reaction.ZnO and ZnO-derived materials have been shown to be promising materials for photocatalytic water splitting16,17 and photocatalytic degradation of polluting organic compounds in water.18 In the present study, we have shown that proton conduction on ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) is pseudo-one-dimensional, whereas it is two-dimensional at ZnO(11
0) is pseudo-one-dimensional, whereas it is two-dimensional at ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0). This opens up interesting opportunities for the design of advanced ZnO-based nanomaterials in which such facet-dependent proton transport dimensionalities can be exploited. For example, our results would indicate that ZnO(11
0). This opens up interesting opportunities for the design of advanced ZnO-based nanomaterials in which such facet-dependent proton transport dimensionalities can be exploited. For example, our results would indicate that ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0), where proton diffusion is two-dimensional and comparatively fast, would allow for efficient, almost isotropic, proton diffusion from the place of the formation to the place of the reaction. In contrast, at ZnO(10
0), where proton diffusion is two-dimensional and comparatively fast, would allow for efficient, almost isotropic, proton diffusion from the place of the formation to the place of the reaction. In contrast, at ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0), the formation and reaction must take place at the same “coordinate” along the polar direction, thus limiting the probability that two reactants can meet to form the product. These results are particularly interesting in light of the fact that ZnO(10
0), the formation and reaction must take place at the same “coordinate” along the polar direction, thus limiting the probability that two reactants can meet to form the product. These results are particularly interesting in light of the fact that ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) is a more stable surface than ZnO(11
0) is a more stable surface than ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0).20
0).20
Diffusion coefficients
Finally, we comment on the magnitudes of the calculated diffusion coefficients. Nuclear quantum effects (NQEs) are particularly pronounced for light elements like H, and have been shown to lower the proton transfer barriers of, for example, H3O+ and OH− ions in aqeuous solution.2,5,8 Consequently, a consideration of NQEs leads to increased proton transfer rates and higher diffusion coefficients of these ions in water. It is not unreasonable to assume that similar considerations of NQEs would lead also to greater diffusion coefficients of the PHCs on the nonpolar ZnO surfaces. Although NQEs may affect the PT barriers, and, consequently, the PT rates and diffusion coefficients, they would not necessarily affect the relative diffusion coefficients along the polar and nonpolar crystal directions. An explicit treatment of NQEs lies outside the scope of the current work, but would give a more quantitatively accurate picture of the pertinent diffusion coefficients.In simulations, the PT barriers and diffusion coefficients also depend on the underlying computational method.6 Here, we used a neural network potential fitted to reproduce a dispersion-corrected density-functional-theory-calculated potential energy surface. In a recent work, Chen et al.9 compared several different computational methods for the estimated diffusion coefficients of H3O+ and OH− in aqueous solution, and found that a particular dispersion-corrected flavor of density functional theory overestimated the diffusion coefficients somewhat. Thus, although our present simulations on the one hand underestimate the diffusion coefficients because of the lack of nuclear quantum effects, they may well overestimate the diffusion coefficients as a result of the chosen reference method for the neural network parameterization, resulting in a partial cancellation of errors.
The one-dimensional proton hole diffusion coefficients in this work, for the crystal directions where the diffusion is significant, lie approximately in the range 10 to 37 × 10−12 m2 s−1 (Fig. 7). The room-temperature diffusion coefficients for the H3O+ and OH− in bulk (3-D) water in dilute solution are 9.6 × 10−9 m2 s−1 and 5.4 × 10−9 m2 s−1, respectively. Because the diffusion of these ions in water is isotropic, the diffusion coefficients per spatial direction are a third of the quoted values, i.e., 3.2 × 10−9 m2 s−1 and 1.8 × 10−9 m2 s−1, respectively. Thus, even the fastest (1-D) proton diffusion at one of the two ZnO–water interfaces in this work 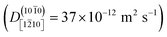 , is considerably slower than the diffusion of H3O+ and OH− in water, amounting to only about 1–2% of the corresponding H3O+ and OH− diffusion coefficients.
, is considerably slower than the diffusion of H3O+ and OH− in water, amounting to only about 1–2% of the corresponding H3O+ and OH− diffusion coefficients.
Conclusions
The spontaneous dissociation of water near metal oxide interfaces, and the proton transport properties of the interface, are of fundamental interest for catalysis, electrochemistry, and geochemistry. Here, on the basis of large-scale molecular dynamics simulations employing a first-principles-quality neural network potential, we explored Grotthuss-like proton diffusion at the two most stable surfaces of zinc oxide in contact with liquid water, revealing fundamentally different surface properties for different surface geometries. In these simulations, no “extrinsic” protons or proton holes were introduced; instead, the protons diffuse via spontaneous water dissociation and recombination events at the interface. This is, to the best of our knowledge, the first time that such an analysis has been carried out for any metal-oxide–liquid-water interface. We found that, whereas proton diffusion at the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0)–liquid-water interface is pseudo-one-dimensional, occurring mainly along the nonpolar ±[1
0)–liquid-water interface is pseudo-one-dimensional, occurring mainly along the nonpolar ±[1![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 10] directions, it is two-dimensional at the ZnO(11
10] directions, it is two-dimensional at the ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0)–liquid-water interface, occurring both along the nonpolar ±[1
0)–liquid-water interface, occurring both along the nonpolar ±[1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 00] directions and the polar ±[0001] directions. For both surfaces, proton diffusion is considerably slower than the diffusion of H3O+ and OH− in aqueous solution. These results highlight the importance of surface-specific properties of zinc oxide, with possible implications for applications of nanowires and nanoparticles, used for example as biosensors and photocatalysts.
00] directions and the polar ±[0001] directions. For both surfaces, proton diffusion is considerably slower than the diffusion of H3O+ and OH− in aqueous solution. These results highlight the importance of surface-specific properties of zinc oxide, with possible implications for applications of nanowires and nanoparticles, used for example as biosensors and photocatalysts.
Methods
The potential energy surfaces of the ZnO–liquid-water interfaces were described by a high-dimensional neural network potential (NNP), that we previously developed and validated for the ZnO–liquid-water interface using training data for both the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surface in contact with liquid water.34 The NNP was parameterized to reproduce dispersion-corrected DFT-calculated energies and forces at the RPBE-D3 level of theory.47,48 RPBE-D3 has been shown to describe liquid water,49 as well as proton transfer reactions in NaOH solutions,36 very well.
0) surface in contact with liquid water.34 The NNP was parameterized to reproduce dispersion-corrected DFT-calculated energies and forces at the RPBE-D3 level of theory.47,48 RPBE-D3 has been shown to describe liquid water,49 as well as proton transfer reactions in NaOH solutions,36 very well.
The ZnO slabs were roughly 1 nm thick and had 48 Zns and 48 Os surface atoms per side of the slab, and were separated by about 2.8 nm of liquid water (Fig. 2 and ESI†). The middle half of the ZnO slab was kept fixed during the simulations. The density of the liquid water was allowed to equilibrate in the NPT ensemble. The production simulations were run in the NVT ensemble for 44 ns using a timestep of 0.5 fs, following an equilibration period of 1 ns. The simulations were run using a custom module49 implemented in the LAMMPS program.50 Snapshots of the two systems are provided as ESI.†
Each H atom is assigned to be “covalently bound” to its nearest O atom. O atoms that do not belong to the ZnO crystal, but that are within 2.35 Å of a surface Zn ion, are considered to be adsorbed on the surface and are denoted with an asterisk.
We employed a method where we assign a “proton hole center”, PHC, to be located at each “free” Os2− (surface O without any bound H), as well as at either the Zn or O position of O*H−. This assignment is useful because the number of surface atoms does not change during the simulation, whereas the number of water molecules adsorbed on the surface, which are the sources of the protons, fluctuates during the simulation as a result of exchange events with the liquid water film. Thus, in our two surface ZnO models of the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11
0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces, there are 48 Zns2+ and 48 Os2− surface ions per side of the slab, and thus there is a total of 48 PHCs per side of the slab.
0) surfaces, there are 48 Zns2+ and 48 Os2− surface ions per side of the slab, and thus there is a total of 48 PHCs per side of the slab.
The location of a PHC is followed from one timestep to the next by minimizing the sum of squared distances moved by each PHC (obeying the minimum image convention):
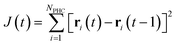 | (5) |
By following the location of the PHCs, the mean squared displacement projected onto a direction x was calculated as
| MSDx(t) = 〈|x(t) − x(0)|2〉 | (6) |
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ], and where x(t) is the x-position of the PHC at time t, and the average 〈…〉 is taken over all time origins and all PHCs. The one-dimensional diffusion coefficient was then calculated as
], and where x(t) is the x-position of the PHC at time t, and the average 〈…〉 is taken over all time origins and all PHCs. The one-dimensional diffusion coefficient was then calculated as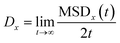 | (7) |
The proton-transfer free-energy landscapes (PTFELs) were calculated as follows: for each possible acceptor species (Os2−, OsH−, O*H−, O*H2, and solvent OH2), we scan through all of the donated hydrogen bonds, where a hydrogen bond OdHd⋯Oa exists if the distance d(Od–Oa) < 3.5 Å and the angle ∠OaOdHd < 30°.52 For each hydrogen bond, δ is calculated as d(Hd⋯Oa) − d(OdHd), where d(AB) is the distance between A and B. The donated hydrogen bond with the smallest value of δ is the one deemed “active” for PT, and the corresponding value of δ is called δmin. Depending on the nature and position of the active proton donor, the donor–acceptor pair is assigned to belong to a particular proton transfer coordinate (for example, “inter-adlayer-PT”; see ESI† for details). For each proton transfer coordinate, a histogram is created with a bin width of 0.1 Å, where the counter W is used for different intervals of δmin in the simulation. The PTFEL is then constructed as
| ΔF(δmin)/kBT = −ln(W(δmin)) | (8) |
Although δmin ≥ 0. Å, for convenience the left hand sides of the PTFELs in Fig. 4 are plotted for the corresponding negative values of δmin.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work was supported by the Cluster of Excellence RESOLV (EXC 1069) funded by the Deutsche Forschungsgemeinschaft, and the DFG Heisenberg professorship Be3264/11-2.References
- D. Marx, Proton Transfer 200 Years after von Grotthuss: Insights from Ab Initio Simulations, ChemPhysChem, 2006, 7, 1848–1870 CrossRef CAS PubMed.
- M. E. Tuckerman, D. Marx and M. Parrinello, The nature and transport mechanism of hydrated hydroxide ions in aqueous solution, Nature, 2002, 417, 925–929 CrossRef CAS PubMed.
- D. Muñoz-Santiburcio and D. Marx, On the complex structural diffusion of proton holes in nanoconfined alkaline solutions within slit pores, Nat. Commun., 2016, 7, 12625 CrossRef PubMed.
- M. Tuckerman, K. Laasonen, M. Sprik and M. Parrinello, Ab initio molecular dynamics simulation of the solvation and transport of hydronium and hydroxyl ions in water, J. Chem. Phys., 1995, 103, 150–161 CrossRef CAS.
- D. Marx, M. E. Tuckerman, J. Hutter and M. Parrinello, The nature of the hydrated excess proton in water, Nature, 1999, 397, 601–604 CrossRef CAS.
- D. Marx, A. Chandra and M. E. Tuckerman, Aqueous Basic Solutions: Hydroxide Solvation, Structural Diffusion, and Comparison to the Hydrated Proton, Chem. Rev., 2010, 110, 2174–2216 CrossRef CAS PubMed.
- A. Hassanali, F. Giberti, J. Cuny, T. D. Kühne and M. Parrinello, Proton transfer through the water gossamer, Proc. Natl. Acad. Sci. U. S. A., 2013, 110, 13723–13728 CrossRef CAS PubMed.
- N. Agmon, H. J. Bakker, R. K. Campen, R. H. Henchman, P. Pohl, S. Roke, M. Thämer and A. Hassanali, Protons and Hydroxide Ions in Aqueous Systems, Chem. Rev., 2016, 116, 7642–7672 CrossRef CAS PubMed.
- M. Chen, L. Zheng, B. Santra, H.-Y. Ko, R. A. DiStasio Jr, M. L. Klein, R. Car and X. Wu, Hydroxide diffuses slower than hydronium in water because its solvated structure inhibits correlated proton transfer, Nat. Chem., 2018, 10, 413–419 CrossRef CAS PubMed.
- C. Chen, Y.-L. S. Tse, G. E. Lindberg, C. Knight and G. A. Voth, Hydroxide Solvation and Transport in Anion Exchange Membranes, J. Am. Chem. Soc., 2016, 138, 991–1000 CrossRef CAS PubMed.
- D. Dong, W. Zhang, A. C. T. van Duin and D. Bedrov, Grotthuss versus Vehicular Transport of Hydroxide in Anion-Exchange Membranes: Insight from Combined Reactive and Nonreactive Molecular Simulations, J. Phys. Chem. Lett., 2018, 9, 825–829 CrossRef CAS PubMed.
- J. L. Achtyl, R. R. Unocic, L. Xu, Y. Cai, M. Raju, W. Zhang, R. L. Sacci, I. V. Vlassiouk, P. F. Fulvio and P. Ganesh, et al., Aqueous proton transfer across single-layer graphene, Nat. Commun., 2015, 6, 6539 CrossRef CAS PubMed.
- Y. Zhang, T. R. Nayak, H. Hong and W. Cai, Biomedical Applications of Zinc Oxide Nanomaterials, Curr. Mol. Med., 2013, 13, 1633–1645 CrossRef CAS PubMed.
- S. K. Arya, S. Saha, J. E. Ramirez-Vick, V. Gupta, S. Bhansali and S. P. Singh, Recent advances in ZnO nanostructures and thin films for biosensor applications: Review, Anal. Chim. Acta, 2012, 737, 1–21 CrossRef CAS PubMed.
- S. Al-Hilli and M. Willander, The pH Response and Sensing Mechanism of n-Type ZnO/Electrolyte Interfaces, Sensors, 2009, 9, 7445–7480 CrossRef CAS PubMed.
- K. Maeda, K. Teramura, D. Lu, T. Takata, N. Saito, Y. Inoue and K. Domen, Photocatalyst releasing hydrogen from water, Nature, 2006, 440, 295 CrossRef CAS PubMed.
- K. Maeda and K. Domen, Photocatalytic Water Splitting: Recent Progress and Future Challenges, J. Phys. Chem. Lett., 2010, 1, 2655–2661 CrossRef CAS.
- K. M. Lee, C. W. Lai, K. S. Ngai and J. C. Juan, Recent developments of zinc oxide based photocatalyst in water treatment technology: A review, Water Res., 2016, 88, 428–448 CrossRef CAS PubMed.
- Z. L. Wang, Zinc oxide nanostructures: growth, properties and applications, J. Phys.: Condens. Matter, 2004, 16, R829–R858 CrossRef CAS.
- C. Wöll, The chemistry and physics of zinc oxide surfaces, Prog. Surf. Sci., 2007, 82, 55–120 CrossRef.
- D. Mora-Fonz, T. Lazauskas, M. R. Farrow, C. R. A. Catlow, S. M. Woodley and A. A. Sokol, Why Are Polar Surfaces of ZnO Stable?, Chem. Mater., 2017, 29, 5306–5320 CrossRef CAS.
- B. Meyer, H. Rabaa and D. Marx, Water adsorption on ZnO(10
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0): from single molecules to partially dissociated monolayers, Phys. Chem. Chem. Phys., 2006, 8, 1513–1520 RSC.
0): from single molecules to partially dissociated monolayers, Phys. Chem. Chem. Phys., 2006, 8, 1513–1520 RSC. - M. Hellström, K. Jorner, M. Bryngelsson, S. E. Huber, J. Kullgren, T. Frauenheim and P. Broqvist, An SCC-DFTB Repulsive Potential for Various ZnO Polymorphs and the ZnO–Water System, J. Phys. Chem. C, 2013, 117, 17004–17015 CrossRef PubMed.
- S. Kenmoe and P. U. Biedermann, Water aggregation and dissociation on the ZnO(10
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) surface, Phys. Chem. Chem. Phys., 2017, 19, 1466–1486 RSC.
0) surface, Phys. Chem. Chem. Phys., 2017, 19, 1466–1486 RSC. - B. Meyer, D. Marx, O. Dulub, U. Diebold, M. Kunat, D. Langenberg and C. Wöll, Partial dissociation of water leads to stable superstructures on the surface of zinc oxide, Angew. Chem., Int. Ed., 2004, 43, 6641–6645 CrossRef CAS PubMed.
- D. J. Cooke, A. Marmier and S. C. Parker, Surface Structure of (1010) and (1120) Surfaces of ZnO with Density Functional Theory and Atomistic Simulation, J. Phys. Chem. B, 2006, 110, 7985–7991 CrossRef CAS PubMed.
- D. Raymand, A. C. T. van Duin, D. Spångberg, W. A. Goddard and K. Hermansson, Water adsorption on stepped ZnO surfaces from MD simulation, Surf. Sci., 2010, 604, 741–752 CrossRef CAS.
- F. Haque, S. Chenot, F. Vines, F. Illas, S. Stankic and J. Jupille, ZnO powders as multi-facet single crystals, Phys. Chem. Chem. Phys., 2017, 19, 10622–10628 RSC.
- H. F. Wilson and A. S. Barnard, Water bilayers on ZnO(10
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) surfaces: data-driven structural search, RSC Adv., 2016, 6, 30928–30936 RSC.
0) surfaces: data-driven structural search, RSC Adv., 2016, 6, 30928–30936 RSC. - S. Kenmoe and P. U. Biedermann, Water adsorbate phases on ZnO and impact of vapor pressure on the equilibrium shape of nanoparticles, J. Chem. Phys., 2018, 148, 054701 CrossRef PubMed.
- D. Raymand, A. C. T. van Duin, W. A. Goddard, K. Hermansson and D. Spångberg, Hydroxylation Structure and Proton Transfer Reactivity at the Zinc Oxide–Water Interface, J. Phys. Chem. C, 2011, 115, 8573–8579 CrossRef CAS.
- N. Kharche, M. S. Hybertsen and J. T. Muckerman, Computational investigation of structural and electronic properties of aqueous interfaces of GaN, ZnO, and a GaN/ZnO alloy, Phys. Chem. Chem. Phys., 2014, 16, 12057–12066 RSC.
- G. Tocci and A. Michaelides, Solvent-Induced Proton Hopping at a Water–Oxide Interface, J. Phys. Chem. Lett., 2014, 5, 474–480 CrossRef CAS PubMed.
- V. Quaranta, M. Hellström and J. Behler, Proton-Transfer Mechanisms at the Water–ZnO Interface: The Role of Presolvation, J. Phys. Chem. Lett., 2017, 8, 1476–1483 CrossRef CAS PubMed.
- V. Quaranta, M. Hellström, J. Behler, J. Kullgren, P. D. Mitev and K. Hermansson, Maximally resolved anharmonic OH vibrational spectrum of the water/ZnO(10
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) interface from a high-dimensional neural network potential, J. Chem. Phys., 2018, 148, 241720 CrossRef PubMed.
0) interface from a high-dimensional neural network potential, J. Chem. Phys., 2018, 148, 241720 CrossRef PubMed. - M. Hellström and J. Behler, Concentration-Dependent Proton Transfer Mechanisms in Aqueous NaOH Solutions: From Acceptor-Driven to Donor-Driven and Back, J. Phys. Chem. Lett., 2016, 7, 3302–3306 CrossRef PubMed.
- A. Tilocca and A. Selloni, Structure and Reactivity of Water Layers on Defect-Free and Defective Anatase TiO2(101) Surfaces, J. Phys. Chem. B, 2004, 108, 4743–4751 CrossRef CAS.
- R. Sato, S. Ohkuma, Y. Shibuta, F. Shimojo and S. Yamaguchi, Proton Migration on Hydrated Surface of Cubic ZrO2: Ab initio Molecular Dynamics Simulation, J. Phys. Chem. C, 2015, 119, 28925–28933 CrossRef CAS.
- G. F. von Rudorff, R. Jakobsen, K. M. Rosso and J. Blumberger, Hematite(001)–liquid water interface from hybrid density functional-based molecular dynamics, J. Phys.: Condens. Matter, 2016, 28, 394001 CrossRef PubMed.
- M. Farnesi Camellone, F. Negreiros Ribeiro, L. Szabov, Y. Tateyama and S. Fabris, Catalytic Proton Dynamics at the Water/Solid Interface of Ceria-Supported Pt Clusters, J. Am. Chem. Soc., 2016, 138, 11560–11567 CrossRef CAS PubMed.
- J. Wang, L. S. Pedroza, A. Poissier and M. V. Fernández-Serra, Water Dissociation at the GaN(10
![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) Surface: Structure, Dynamics and Surface Acidity, J. Phys. Chem. C, 2012, 116, 14382–14389 CrossRef CAS.
0) Surface: Structure, Dynamics and Surface Acidity, J. Phys. Chem. C, 2012, 116, 14382–14389 CrossRef CAS. - B. C. Wood, E. Schwegler, W. I. Choi and T. Ogitsu, Hydrogen-Bond Dynamics of Water at the Interface with InP/GaP(001) and the Implications for Photoelectrochemistry, J. Am. Chem. Soc., 2013, 135, 15774–15783 CrossRef CAS PubMed.
- B. Dünweg and K. Kremer, Molecular dynamics simulation of a polymer chain in solution, J. Chem. Phys., 1993, 99, 6983–6997 CrossRef.
- J. Behler and M. Parrinello, Generalized Neural-Network Representation of High-Dimensional Potential-Energy Surfaces, Phys. Rev. Lett., 2007, 98, 146401 CrossRef PubMed.
- J. Behler, First Principles Neural Network Potentials for Reactive Simulations of Large Molecular and Condensed Systems, Angew. Chem., Int. Ed., 2017, 56, 12828–12840 CrossRef CAS PubMed.
- L. Ma, B. Liu, P.-J. J. Huang, X. Zhang and J. Liu, DNA Adsorption by ZnO Nanoparticles near Its Solubility Limit: Implications for DNA Fluorescence Quenching and DNAzyme Activity Assays, Langmuir, 2016, 32, 5672–5680 CrossRef CAS PubMed.
- B. Hammer, L. B. Hansen and J. K. Nørskov, Improved adsorption energetics within density-functional theory using revised Perdew–Burke–Ernzerhof functionals, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 7413–7421 CrossRef.
- S. Grimme, J. Antony, S. Ehrlich and H. Krieg, A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu, J. Chem. Phys., 2010, 132, 154104 CrossRef PubMed.
- T. Morawietz, A. Singraber, C. Dellago and J. Behler, How van der Waals interactions determine the unique properties of water, Proc. Natl. Acad. Sci. U. S. A., 2016, 113, 8368–8373 CrossRef CAS PubMed.
- S. Plimpton, Fast Parallel Algorithms for Short-Range Molecular Dynamics, J. Comp. Physiol., 1995, 117, 1–19 CrossRef CAS.
- H. W. Kuhn, The Hungarian method for the assignment problem, Nav. Res. Logist. Q., 1955, 2, 83–97 CrossRef.
- A. Luzar and D. Chandler, Effect of Environment on Hydrogen Bond Dynamics in Liquid Water, Phys. Rev. Lett., 1996, 76, 928–931 CrossRef CAS PubMed.
Footnote |
† Electronic supplementary information (ESI) available: Snapshots (Cartesian coordinates of the atoms including periodic lattice parameters) of the ZnO(10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and ZnO(11 0) and ZnO(11![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 0) surfaces in contact with liquid water. Details for the automated PT coordinate assignment. Calculated PTFELs for all proton transfer coordinates, and two-dimensional PTFELs for the PT coordinates in Fig. 4. Validation of the neural network by comparison to ab initio molecular dynamics simulations. See DOI: 10.1039/c8sc03033b 0) surfaces in contact with liquid water. Details for the automated PT coordinate assignment. Calculated PTFELs for all proton transfer coordinates, and two-dimensional PTFELs for the PT coordinates in Fig. 4. Validation of the neural network by comparison to ab initio molecular dynamics simulations. See DOI: 10.1039/c8sc03033b |
| This journal is © The Royal Society of Chemistry 2019 |