Exciton-band tuning induced by the width of the cation in 2D lead iodide perovskite hybrids†
Marie-Hélène
Tremblay
a,
John
Bacsa
ab,
Stephen
Barlow
 a and
Seth R.
Marder
*a
a and
Seth R.
Marder
*a
aSchool of Chemistry and Biochemistry, and Center for Organic Photonics and Electronics (COPE), Georgia Institute of Technology GA, Atlanta 30332-0400, USA. E-mail: seth.marder@chemistry.gatech.edu
bCrystallography Lab, Emory University, 201 Dowman Drive, Atlanta, GA 30322, USA
First published on 29th May 2020
Abstract
It was previously demonstrated that the exciton energy of 2D hybrid organic–inorganic A2PbI4 perovskites is correlated with the Pb–I–Pb bond angle of the inorganic layer. We demonstrate that this angle can be tuned by changing the width of the cation and that the exciton band energy can be varied over ca. 100 meV, at least within a limited series of A2PbI4 compounds where A is a 2-(3,5-disubstitutedphenyl)ethylammonium or 2-(3-substitutedphenyl)ethylammonium ion and where the substituents are H, F, Cl, Br, or Me. Seven new crystal structures are presented here.
Introduction
Recently, two-dimensional (2D) hybrid organic inorganic perovskites (HOIP) have been used as the active layer for solar cells1–10 and light emitting diodes.11,12 Compared with their 3D counterparts (such as MAPbI3, where MA = methylammonium), they can offer superior stability and a much wider structural diversity, since the cation size is not limited by the volume of the cuboctahedral cavity in the 3D inorganic lattice. The optical properties of the perovskite can be tuned by different methods, for example, by changing the connectivity of the octahedra13 or the halide14–16 used. The optical characteristics of these materials are particularly relevant to solar-cell and light-emitting diode applications. Previous work, both computational17,18 and experimental,19 on 3D lead iodide structures showed a relationship between the Pb–I–Pb bond angles and the onset of the optical absorption of the material.20–24 On increasing the size of the cation (Cs+ < MA+ < FA+, where FA = formamidinium), the Pb–I–Pb angle decreases due to more distortion of the network of PbI6 octahedra, resulting in poorer Pb-Hal orbital overlap and an increased band gap.25,26 In 2D A2MX4 (A = organic cation, M = metal, X = halide) HOIPs, there is considerably more pronounced excitonic character than in their 3D counterparts. However, trends in the exciton energy often closely follow those in the band gap. Moreover, in some instances, the exciton energy has been found to also be correlated with the M–I–M angle.8,27–31 The steric bulk of the cation has also been found to correlate with the Sn–I–Sn angle in some studies of A2SnI4 perovskites.23,32,33Knowing that the M–I–M angle correlates with the properties of the material, we were interested to probe whether the width of 2-arylethylammonium cations (defined as the distance between meta and meta′ substituents) was related to this bond angle. We report the synthesis and crystal growth of a series of 2D perovskites where the aryl group have different widths; the meta substituents on phenylethylammonium (PEA) are systematically changed in the series (dY-PEA)2PbI4 where Y = H, F, Cl, Br and Me (Fig. 1). We describe the structural and optical properties of these materials and compare them to those of other A2PbI4 species reported in the literature.
Results and discussion
We investigated the structure and optical characteristics of lead iodide HOIPs with aromatic cations of increasing width. The width, defined here as the Y⋯Y distance, is incrementally enlarged by adding substituents at the 3,5-position of the PEA ring. The synthesis of the different cations is presented in the ESI.† The HOIPs were crystallized as pale to dark orange plates from a 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 solution of PbI2 and A+I− in HI/MeOH (see ESI† for detailed synthesis). We abbreviate these HOIPs as PEA (2-phenylethylammonium), dF-PEA (2-(3,5-difluorophenyl)ethylammonium), dCl-PEA (2-(3,5-dichlorophenyl)ethylammonium), dBr-PEA (2-(3,5-dibromophenyl)ethylammonium), and dMe-PEA (2-(3,5-dimethylphenyl)ethylammonium). Or course, the increase of halogen atom size also results in an increase in polarizability, whereas dMe-PEA has approximately the same width as dBr-PEA, but is anticipated to be less polarizable. The purity of the crystals was assessed by elemental analysis and powder XRD; in each case, XRD patterns for powder samples were consistent with the corresponding single-crystal structures (see Fig. S1, ESI†; CCDC 1986784–1986789 and 1987208†). In all cases, we obtained PbI42− layers of corner-sharing octahedra separated by bilayers of the organic cations (Fig. 2). In all compounds, there is hydrogen bonding between the iodides and the ammonium groups, which is typical for A2PbI4 HOIP structures. Based on the offset of successive inorganic layers, we can categorize dF-PEA, PEA, dCl-PEA and dMe-PEA as near-Ruddlesden–Popper structure and dBr-PEA as monocation-near-Dion-Jacobson structures (see offset in Table S3, ESI†).28
2 solution of PbI2 and A+I− in HI/MeOH (see ESI† for detailed synthesis). We abbreviate these HOIPs as PEA (2-phenylethylammonium), dF-PEA (2-(3,5-difluorophenyl)ethylammonium), dCl-PEA (2-(3,5-dichlorophenyl)ethylammonium), dBr-PEA (2-(3,5-dibromophenyl)ethylammonium), and dMe-PEA (2-(3,5-dimethylphenyl)ethylammonium). Or course, the increase of halogen atom size also results in an increase in polarizability, whereas dMe-PEA has approximately the same width as dBr-PEA, but is anticipated to be less polarizable. The purity of the crystals was assessed by elemental analysis and powder XRD; in each case, XRD patterns for powder samples were consistent with the corresponding single-crystal structures (see Fig. S1, ESI†; CCDC 1986784–1986789 and 1987208†). In all cases, we obtained PbI42− layers of corner-sharing octahedra separated by bilayers of the organic cations (Fig. 2). In all compounds, there is hydrogen bonding between the iodides and the ammonium groups, which is typical for A2PbI4 HOIP structures. Based on the offset of successive inorganic layers, we can categorize dF-PEA, PEA, dCl-PEA and dMe-PEA as near-Ruddlesden–Popper structure and dBr-PEA as monocation-near-Dion-Jacobson structures (see offset in Table S3, ESI†).28
Thermogravimetric analysis of the four HOIPs based on 2-(3,5-disubstitutedphenyl)ethylammonium ions (see Fig. S2, ESI†) shows two distinct weight losses: one begins at ca. 230 °C, while our reference (PEA)2PbI4 starts exhibiting weight loss at 200 °C.12 This initial weight lost is likely due to the organic cation decomposition and loss, while the second period of weight loss is presumably due to the sublimation of the lead iodide.
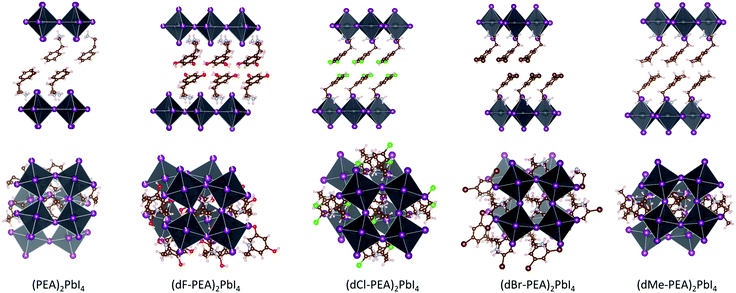 | ||
| Fig. 2 Crystal structure of (dY-PEA)2PbI4, Y = H, F, Cl, Br, Me, viewed along the plane of the inorganic sheet and after +90° rotation around the long in-layer axis. The structure of (PEA)2PbI4 is taken from the literature.34 | ||
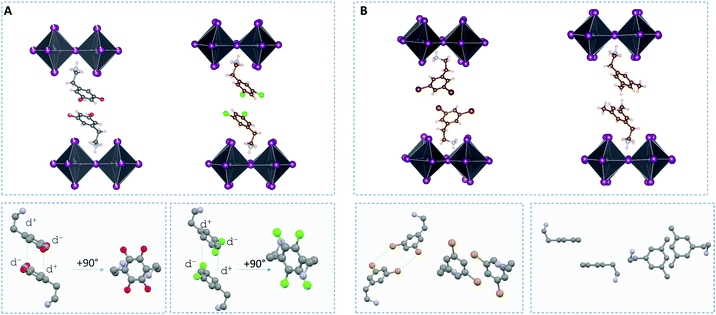
Multiple cation–cation interactions can be found in these HOIPs single crystals (see Fig. S3–S5, ESI†). For dF-PEA and dCl-PEA, the benzene rings from the two different organic layers are found to lay on top of each other. The distance between the centroid of one benzene to the plane made by another benzene is 3.343 Å for Y = F and 3.508 Å for Y = Cl, which could arise from a dipole–dipole interaction between the two cations (see Fig. 3A). In the case of dBr-PEA, there is a close approach between the bromine atoms from the two layers. This may be due to a Br⋯Br van der Waals interaction (see Fig. 3B). A close approach between the methyl groups is also seen for dMe-PEA.
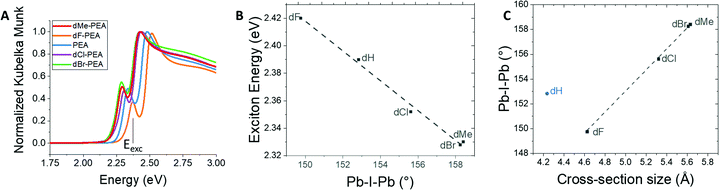
All compounds exhibited narrow linewidths for the photoluminescence feature characteristic of free exciton emission (see Fig. S5, ESI†). Fig. 4A shows the Kubelka–Munk transformation of the diffuse reflectance of the different HOIP powders and indicate the presence of two distinct bands. The energy of the low-energy peak is assigned to the exciton band energy (see Fig. 4A). As expected, there is a linear relationship between the Pb–I–Pb angle and the exciton energy; the exciton band is red shifted by ca. 100 meV from dBr-PEA to dF-PEA (see Fig. 4B).27 The relationships of Pb–I–Pb angle to the band gap in 3D HOIPs20–24 and to the exciton-band energy in 2D HOIPs8,27–31 have been observed in many instances as stated before. It has been shown that, in 3D HOIPs, band gap significantly opens upon octahedral tilting, mainly as a result of a decrease of the overlap integral, resulting in reducing antibonding character, and thus stabilization, of the valence band maximum.21 Since trends in the exciton energy often closely follow those in the band gap, this rule can be extrapolated for the exciton energy of 2D HOIPs.
There is also a linear trend between the Pb–I–Pb angle and the width of the cation (see Fig. 4C). What emerges, based on these 5 compounds, is that, even if the reference compound (PEA, smallest cross-section size) does not follow the trend, we are able to show that the width of the cation is a fair indicator of the Pb–I–Pb angle in the HOIPs.
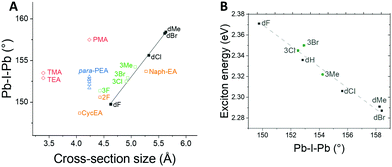
In order to establish whether this trend holds true for cations other than 3,5-disubstituted PEAs, we extended the study to other families of cations (see Fig. 5). First, we synthesized meta-substituted phenylethylammonium-based HOIPs (3Y-PEA, where Y = Cl, Br, Me) and determined the structures. Another member of this series, (3F-PEA)2PbI4, has previously been reported;35 thus structural data are now available for both dY-PEA and 3Y-PEA families for the same set of substituents (Y = F, Cl, Br, Me). Again, the Pb–I–Pb bond angle increases with the width of the cation: the data points for the three new compounds essentially lie on the same line as those for the dY-PEA series, while the point for 3F-PEA compound still lies close to this line. Moreover, data for three newly synthesized compounds fit the same linear plot between the exciton energy and the Pb–I–Pb bond angle as the dY-PEA derivatives discussed above (see Fig. 5B).
We then examined additional structures available from literature reports. para-Substituted 2-phenylethylammonium-based perovskites (in which the cross-section size is constant) have similar Pb–I–Pb bond angles (A2PbI4 where A = PEA 152.9°,34 4F-PEA 151.5°,36 4Cl-PEA 152.48°,36 4Br-PEA 152.15°,36 4Ph-PEA 153.1°,31 4Cl-Ph-CH(CH3)NH3 152.7°37), reinforcing the hypothesis that cross-section size is an important factor in determining the Pb–I–Pb bond angle, although the data points for these derivatives do not fall on the same line as the disubstituted and meta-substituted derivatives. A2PbI4 perovskites for A = TMA38 and TEA39 cations (TMA = 2-(2-thienyl)methylammonium, TEA = 2-(2-thienyl)ethylammonium), which have the same cross-section size but different alkyl length, have similar Pb–I–Pb bond angles to one another, but larger than one would expect based on the trend in the disubstituted and meta-substituted compounds. On the other hand, Pb–I–Pb angle and cross-section size data for A2PbI4 perovskites based on several other cations including 2-(naphth-2-yl)ethylammonium (NEA),34 2-(1-cyclohexenyl)ethylammonium (CycEA),40 and 2-fluorophenylethylammonium (2F-PEA)41 do lie close to the line defined by the (dY-PEA)2PbI4 derivatives (and other (3Y-PEA)2PbI4 derivatives) reported here.
There are cases of families of 2D A2PbI4 structures reported in which the cross-section and Pb–I–Pb angle do not linearly correlate: (1) when the two layer of cations interpenetrate one another, instead of being separated in two sheets (para-substituted PMAs);28 (2) when the 2D structure is not planar, but corrugated instead (as in the case of A = 2-(4-nitrophenyl)ethylammonium);42 and (3) when the core of the cation is much larger than that of 2-(phenyl)ethylammonium (pyrene and perylene derivatives).5,43,44
Conclusion
We report a total of 7 new 2D HOIPs containing 3,5-disubstituted-phenylethylammonium cations, dY-PEA (Y = F, Cl, Br, Me) and 3-substituted-phenylethylammonium cations, 3Y-PEA (Y = Cl, Br, Me). We were able to demonstrate that, at least for some limited series of compounds, the width of the cation correlates with the distortion of the inorganic sheet through Pb–I–Pb bond angle variation, which in turn correlates with the exciton energy. We note that while the second of these correlations has been discussed in other systems and can be understood in terms of effects on the perovskite band structure,21 the first is purely an empirical observation. That is, the mechanism by which the cation width affects the bond angle is not clear, although, it is expected that changes in steric demands associated with variations of the cation width will affect the optimum packing in the interlayer region; these changes in packing may be accommodated by structural changes in both organic and inorganic portions of the structure. Given the complex energetic balance of interactions and the conformational flexibility of both the organic cations and the inorganic layers in these structures, the specific structural changes observed are not easily rationalized, as is underscored by the observed non-universality of the width/angle correlation. However, although width is not a universal factor determining the Pb–I–Pb angle and multiple other factors must likely be taken into account, the observation of this relation for at least some families of cations brings us closer to developing an understanding between the structure of the organic cation and the structure of the corresponding 2D HOIP. The deviations observed from the proposed relation may afford insight into other descriptors that could be looked at in the future.Conflicts of interest
There are no conflicts of interest to declare.Acknowledgements
This work was supported by NSERC (ES. D. Scholarship for MHT) and the AFOSR (FA9550-18-1-0499). We thank Khaled Al Kurdi for his help with thermal measurements and Ningxin Jiang, Dr Alexander Steiner and Tom Pickel for help with crystal measurement. This work was performed in part at the Georgia Tech Institute for Electronics and Nanotechnology, a member of the National Nanotechnology Coordinated Infrastructure (NNCI), which is supported by the National Science Foundation (Grant ECCS-1542174).References
- I. C. Smith, E. T. Hoke, D. Solis-Ibarra, M. D. McGehee and H. I. Karunadasa, A layered hybrid perovskite solar-cell absorber with enhanced moisture stability, Angew. Chem., Int. Ed., 2014, 53, 11232–11235 Search PubMed.
- J. Yan, W. Qiu, G. Wu, P. Heremans and H. Chen, Recent progress in 2D/quasi-2D layered metal halide perovskites for solar cells, J. Mater. Chem. A, 2018, 6, 11063–11077 RSC.
- Y. Chen, Y. Sun, J. Peng, W. Zhang, X. Su, K. Zheng, T. Pullerits and Z. Liang, Tailoring organic cation of 2D air-stable organometal halide perovskites for highly efficient planar solar cells, Adv. Energy Mater., 2017, 7, 1700162 CrossRef.
- D. H. Cao, C. C. Stoumpos, O. K. Farha, J. T. Hupp and M. G. Kanatzidis, 2D Homologous perovskites as light-absorbing materials for solar cell applications, J. Am. Chem. Soc., 2015, 137, 7843–7850 CrossRef CAS PubMed.
- C. Ma, D. Shen, T.-W. Ng, M.-F. Lo and C.-S. Lee, 2D Perovskites with short interlayer distance for high-performance solar cell application, Adv. Mater., 2018, 30, 1800710 CrossRef PubMed.
- H. Lai, B. Kan, T. Liu, N. Zheng, Z. Xie, T. Zhou, X. Wan, X. Zhang, Y. Liu and Y. Chen, Two-dimensional Ruddlesden–Popper perovskite with nanorod-like morphology for solar cells with efficiency exceeding 15%, J. Am. Chem. Soc., 2018, 140, 11639–11646 CrossRef CAS PubMed.
- H. Tsai, W. Nie, J.-C. Blancon, C. C. Stoumpos, R. Asadpour, B. Harutyunyan, A. J. Neukirch, R. Verduzco, J. J. Crochet, S. Tretiak, L. Pedesseau, J. Even, M. A. Alam, G. Gupta, J. Lou, P. M. Ajayan, M. J. Bedzyk, M. G. Kanatzidis and A. D. Mohite, High-efficiency two-dimensional Ruddlesden–Popper perovskite solar cells, Nature, 2016, 536, 312 CrossRef CAS PubMed.
- L. Mao, W. Ke, L. Pedesseau, Y. Wu, C. Katan, J. Even, M. R. Wasielewski, C. C. Stoumpos and M. G. Kanatzidis, Hybrid Dion–Jacobson 2D lead iodide perovskites, J. Am. Chem. Soc., 2018, 140, 3775–3783 CrossRef CAS PubMed.
- C. C. Stoumpos, D. H. Cao, D. J. Clark, J. Young, J. M. Rondinelli, J. I. Jang, J. T. Hupp and M. G. Kanatzidis, Ruddlesden–Popper hybrid lead iodide perovskite 2D homologous semiconductors, Chem. Mater., 2016, 28, 2852–2867 CrossRef CAS.
- S. Ahmad, P. Fu, S. Yu, Q. Yang, X. Liu, X. Wang, X. Wang, X. Guo and C. Li, Dion-Jacobson phase 2D layered perovskites for solar cells with ultrahigh stability, Joule, 2019, 3, 794–806 CrossRef CAS.
- M. Yuan, L. N. Quan, R. Comin, G. Walters, R. Sabatini, O. Voznyy, S. Hoogland, Y. Zhao, E. M. Beauregard, P. Kanjanaboos, Z. Lu, D. H. Kim and E. H. Sargent, Perovskite energy funnels for efficient light-emitting diodes, Nat. Nanotechnol., 2016, 11, 872 CrossRef CAS PubMed.
- H. Tsai, W. Nie, J.-C. Blancon, C. C. Stoumpos, C. M. M. Soe, J. Yoo, J. Crochet, S. Tretiak, J. Even, A. Sadhanala, G. Azzellino, R. Brenes, P. M. Ajayan, V. Bulović, S. D. Stranks, R. H. Friend, M. G. Kanatzidis and A. D. Mohite, Stable light-emitting diodes using phase-pure Ruddlesden–Popper layered perovskites, Adv. Mater., 2018, 30, 1704217 CrossRef PubMed.
- M. E. Kamminga, G. A. de Wijs, R. W. A. Havenith, G. R. Blake and T. T. M. Palstra, The role of connectivity on electronic properties of lead iodide perovskite-derived compounds, Inorg. Chem., 2017, 56, 8408–8414 CrossRef CAS PubMed.
- K. Tanaka, T. Takahashi, T. Ban, T. Kondo, K. Uchida and N. Miura, Comparative study on the excitons in lead-halide-based perovskite-type crystals CH3NH3PbBr3 CH3NH3PbI3, Solid State Commun., 2003, 127, 619–623 CrossRef CAS.
- J. H. Noh, S. H. Im, J. H. Heo, T. N. Mandal and S. I. Seok, Chemical management for colorful, efficient, and stable inorganic–organic hybrid nanostructured solar cells, Nano Lett., 2013, 13, 1764–1769 Search PubMed.
- J. Xing, F. Yan, Y. Zhao, S. Chen, H. Yu, Q. Zhang, R. Zeng, H. V. Demir, X. Sun, A. Huan and Q. Xiong, High-efficiency light-emitting diodes of organometal halide perovskite amorphous nanoparticles, ACS Nano, 2016, 10, 6623–6630 CrossRef CAS PubMed.
- M. R. Filip, G. E. Eperon, H. J. Snaith and F. Giustino, Steric engineering of metal-halide perovskites with tunable optical band gaps, Nat. Commun., 2014, 5, 5757 CrossRef CAS PubMed.
- I. Borriello, G. Cantele and D. Ninno, Ab initio investigation of hybrid organic-inorganic perovskites based on tin halides, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 235214 CrossRef.
- G. E. Eperon, S. D. Stranks, C. Menelaou, M. B. Johnston, L. M. Herz and H. J. Snaith, Formamidinium lead trihalide: a broadly tunable perovskite for efficient planar heterojunction solar cells, Energy Environ. Sci., 2014, 7, 982–988 RSC.
- C. C. Stoumpos and M. G. Kanatzidis, The renaissance of halide perovskites and their evolution as emerging semiconductors, Acc. Chem. Res., 2015, 48, 2791–2802 CrossRef CAS PubMed.
- C. Katan, N. Mercier and J. Even, Quantum and dielectric confinement effects in Lower-Dimensional Hybrid Perovskite Semiconductors, Chem. Rev., 2019, 119, 3140–3192 CrossRef CAS PubMed.
- L. Pedesseau, D. Sapori, B. Traore, R. Robles, H.-H. Fang, M. A. Loi, H. Tsai, W. Nie, J.-C. Blancon, A. Neukirch, S. Tretiak, A. D. Mohite, C. Katan, J. Even and M. Kepenekian, Advances and promises of layered halide hybrid perovskite semiconductors, ACS Nano, 2016, 10, 9776–9786 CrossRef CAS PubMed.
- J. L. Knutson, J. D. Martin and D. B. Mitzi, Tuning the band gap in hybrid tin iodide perovskite semiconductors using structural templating, Inorg. Chem., 2005, 44, 4699–4705 CrossRef CAS PubMed.
- T. Umebayashi, K. Asai, T. Kondo and A. Nakao, Electronic structures of lead iodide based low-dimensional crystals, Phys. Rev. B: Condens. Matter Mater. Phys., 2003, 67, 155405 CrossRef.
- S. Meloni, G. Palermo, N. Ashari-Astani, M. Grätzel and U. Rothlisberger, Valence and conduction band tuning in halide perovskites for solar cell applications, J. Mater. Chem. A, 2016, 4, 15997–16002 RSC.
- R. Prasanna, A. Gold-Parker, T. Leijtens, B. Conings, A. Babayigit, H.-G. Boyen, M. F. Toney and M. D. McGehee, Band gap tuning via lattice contraction and octahedral tilting in perovskite materials for photovoltaics, J. Am. Chem. Soc., 2017, 139, 11117–11124 CrossRef CAS PubMed.
- L. Mao, C. C. Stoumpos and M. G. Kanatzidis, Two-dimensional hybrid halide perovskites: principles and promises, J. Am. Chem. Soc., 2019, 141, 1171–1190 CrossRef CAS PubMed.
- M.-H. Tremblay, J. Bacsa, B. Zhao, F. Pulvirenti, S. Barlow and S. R. Marder, Structures of (4-Y-C6H4CH2NH3)2PbI4 {Y = H, F, Cl, Br, I}: tuning of hybrid organic inorganic perovskite structures from Ruddlesden–Popper to Dion–Jacobson limits, Chem. Mater., 2019, 31, 6145–6153 CrossRef CAS.
- L. Mao, Y. Wu, C. C. Stoumpos, M. R. Wasielewski and M. G. Kanatzidis, White-light emission and structural distortion in new corrugated two-dimensional lead bromide perovskites, J. Am. Chem. Soc., 2017, 139, 5210–5215 CrossRef CAS PubMed.
- L. Mao, P. Guo, M. Kepenekian, I. Spanopoulos, Y. He, C. Katan, J. Even, R. D. Schaller, R. Seshadri, C. C. Stoumpos and M. G. Kanatzidis, Organic cation alloying on intralayer A and interlayer A′ sites in 2D hybrid Dion-Jacobson lead bromide perovskites (A′)(A)Pb2Br7, J. Am. Chem. Soc., 2020, 142(18), 8342–8351 Search PubMed.
- N. R. Venkatesan, A. Mahdi, B. Barraza, G. Wu, M. L. Chabinyc and R. Seshadri, Enhanced yield-mobility products in hybrid halide Ruddlesden–Popper compounds with aromatic ammonium spacers, Dalton Trans., 2019, 48, 14019–14026 RSC.
- Z. Xu, D. B. Mitzi, C. D. Dimitrakopoulos and K. R. Maxcy, Semiconducting perovskites (2-XC6H4C2H4NH3)2SnI4 (X = F, Cl, Br):
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Steric Interaction between the organic and inorganic layers, Inorg. Chem., 2003, 42, 2031–2039 CrossRef CAS PubMed.
Steric Interaction between the organic and inorganic layers, Inorg. Chem., 2003, 42, 2031–2039 CrossRef CAS PubMed. - D. B. Mitzi, C. D. Dimitrakopoulos and L. L. Kosbar, Structurally tailored organic−inorganic perovskites:
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) optical properties and solution-processed channel materials for thin-film transistors, Chem. Mater., 2001, 13, 3728–3740 Search PubMed.
optical properties and solution-processed channel materials for thin-film transistors, Chem. Mater., 2001, 13, 3728–3740 Search PubMed. - K.-Z. Du, Q. Tu, X. Zhang, Q. Han, J. Liu, S. Zauscher and D. B. Mitzi, Two-dimensional lead(II) halide-based hybrid perovskites templated by acene alkylamines: crystal structures, optical properties, and piezoelectricity, Inorg. Chem., 2017, 56, 9291–9302 Search PubMed.
- J. Hu, I. W. H. Oswald, S. J. Stuard, M. M. Nahid, N. Zhou, O. F. Williams, Z. Guo, L. Yan, H. Hu, Z. Chen, X. Xiao, Y. Lin, J. Huang, A. M. Moran, H. Ade, J. R. Neilson and W. You, CCDC 1893384: Experimental crystal structure determination, 2019, DOI: 10.5517/ccdc.csd.cc21k6xr.
- D. B. Straus, N. Iotov, M. R. Gau, Q. Zhao, P. J. Carroll and C. R. Kagan, Longer cations increase energetic disorder in excitonic 2D hybrid perovskites, J. Phys. Chem. Lett., 2019, 10, 1198–1205 Search PubMed.
- C.-K. Yang, W.-N. Chen, Y.-T. Ding, J. Wang, Y. Rao, W.-Q. Liao, Y.-Y. Tang, P.-F. Li, Z.-X. Wang and R.-G. Xiong, The first 2D homochiral lead iodide perovskite ferroelectrics: [R- and S-1-(4-chlorophenyl)ethylammonium]2PbI4, Adv. Mater., 2019, 31, 1808088 CrossRef PubMed.
- X.-H. Zhu, N. Mercier, A. Riou, P. Blanchard and P. Frère, (C4H3SCH2NH3)2(CH3NH3)Pb2I7: non-centrosymmetrical crystal structure of a bilayer hybrid perovskite, Chem. Commun., 2002, 2160–2161, 10.1039/B205543K.
- H. Dammak, S. Elleuch, H. Feki and Y. Abid, Synthesis, crystal structure, vibrational spectra, optical properties and theoretical investigation of a two-dimensional self-assembled organic–inorganic hybrid material, Solid State Sci., 2016, 61, 1–8 Search PubMed.
- D. G. Billing and A. Lemmerer, Poly[bis[2-(1-cyclohexenyl)ethylammonium]di-μ-iodo-diodoplumbate(II)], Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 2006, 62, m269–m271 CrossRef PubMed.
- J. Hu, I. W. H. Oswald, S. J. Stuard, M. M. Nahid, N. Zhou, O. S. Williams, Z. Gui, L. Yan, H. Hu, Z. Chen, X. Xiao, Y. Lin, J. Huang, A. M. Moran, H. Ade, J. R. Neilson and W. You, CCDC 1893383: Experimental crystal structure determination, 2019, DOI: 10.5517/ccdc.csd.cc21k6wq.
- M.-H. Tremblay, F. Thouin, J. Leisen, J. Bacsa, A. R. Srimath Kandada, J. M. Hoffman, M. G. Kanatzidis, A. D. Mohite, C. Silva, S. Barlow and S. R. Marder, (4NPEA)2PbI4 (4NPEA = 4-nitrophenylethylammonium): structural, NMR, and optical properties of a 3 × 3 corrugated 2D hybrid perovskite, J. Am. Chem. Soc., 2019, 141, 4521–4525 CrossRef CAS PubMed.
- Since the PEA compounds does not fit on the same plot of I–Pb–I angle vs. cross-section as other derivatives (dF-PEA is larger than PEA but has a smaller Pb−I−Pb bond angle; Fig. 2) we looked at other characteristics of the cations. Interestingly, the increase of the Pb−I−Pb angle correlates with the polarizability of Ar–H (i.e., that of the disubstituted phenyl ring minus the ethylammonium chain, see ref. 44 and Fig. S6, ESI†). Using this indicator, the PEA and dF-PEA are in the right order, showing that multiple factors influences the Pb−I−Pb bond angle; however, overall, this plot also shows some scatter and some anomalies (e.g., dMe-PEA and dCl-PEA have similar polarizabilities but quite different bond angles).
- R. Bosque and J. Sales, Polarizabilities of solvents from the chemical composition, J. Chem. Inform. Comput. Sci., 2002, 42, 1154–1163 Search PubMed.
Footnote |
| † Electronic supplementary information (ESI) available: Experimental procedures and crystallographic details; additional figures showing structural features. CCDC 1986784–1986789 and 1987208. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/d0qm00118j |
| This journal is © the Partner Organisations 2020 |