Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Fluorenone imidazolium salts as novel de Vries materials†
Korinna Badera,
Carsten Müllerb,
Yann Molard c,
Angelika Baroa,
Philipp Ehnia,
Jakob Knellesa and
Sabine Laschat
c,
Angelika Baroa,
Philipp Ehnia,
Jakob Knellesa and
Sabine Laschat *a
*a
aInstitut für Organische Chemie, Universität Stuttgart, Pfaffenwaldring 55, 70569 Stuttgart, Germany. E-mail: sabine.laschat@oc.uni-stuttgart.de
bInstitut für Physikalische Chemie, Universität Stuttgart, Pfaffenwaldring 55, 70569 Stuttgart, Germany
cCNRS, ISCR-UMR 6226, ScanMAT-UMS 2001, University Rennes, 35000 Rennes, France. E-mail: yann.molard@univ-rennes1.fr
First published on 23rd June 2020
Abstract
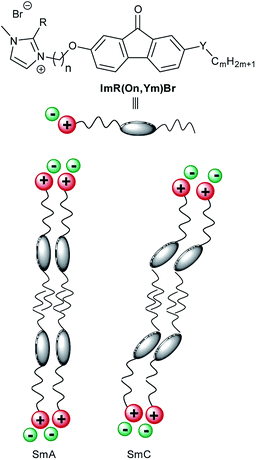
In ionic liquid crystals (ILCs) tilted mesophases such as SmC required for electro-optic devices are quite rare. We report a design concept that induced the SmC phase and enabled de Vries-like behaviour in ILCs. For this purpose, we synthesized and characterized a library of ILC derivatives ImR(On,Ym)X which consist of a rigid central fluorenone core containing an alkoxy or thioether side chain and connected via a flexible spacer to an imidazolium head group. The mesomorphic properties were studied by differential scanning calorimetry (DSC), polarizing optical microscopy (POM) and X-ray diffraction (XRD). Temperature-dependent measurements of smectic layer spacing d by small-angle X-ray scattering (SAXS) and of optical tilt angles by POM demonstrate that ILCs ImR(On,Ym)X undergo SmA–SmC phase transitions with maximum layer contraction values between 0.4% and 2.1%. The lowest reduction factor R of 0.2 at the reduced temperature T − TAC = −10 K was calculated for Im(O12,S14)Br. Electron density calculations indicated a bilayer structure. Furthermore, temperature dependent emission studies show that self-assembling has a strong influence on the emission intensity of these ILCs.
Introduction
Ionic liquid crystals (ILCs) combine the physical properties of ionic liquids with those of thermotropic liquid crystals1–8 and thus provide highly desirable materials for anisotropically ordered 1D ion conductors in organic solar cells9,10 and as solid electrolytes in lithium ion batteries.11 While ILCs share many similarities with neutral thermotropic liquid crystals, they differ in the occurrence of certain mesophases.1–8 The strong tendency of ILCs to nanosegregate ionic and non-charged segments during liquid crystalline self-assembly leads to a strong preference of the non-tilted SmA phase as their archetypal mesophase, while for example the tilted SmC phase is much less common among ILCs.12–16 We have previously identified ILCs 1, 2 showing rare SmC phases (Scheme 1), which consist of a calamitic core unit carrying one side chain and a flexible spacer connected to a cationic head group.15–18 Upon examination of the order parameter of the SmA phase of these compounds it was found that the long range orientational order (S2) in the SmA phase was much smaller as compared to the values obtained for SmA phases from non-ionic liquid crystals.19–22 On the other hand, the 1D translational order (smectic order parameter ε) of the SmA phase of ILCs is much larger17,18 as compared to non-ionic SmA phases.23–26 Thus ILCs possess a high lamellar order but only a low long range orientational order as compared to neutral liquid crystals and in that respect behave similar to the so called de Vries materials.26–29 De Vries materials have created much interest recently, because ferroelectric displays based on de Vries materials might overcome limitations of current nematic LC displays such as limited resolution and low optical efficiency.30–47 One of the characteristic features of de Vries materials is their tilting transition without defect generation. These materials show no or only very low layer contraction upon the SmA to SmC phase transition, enabling ferroelectric displays without zig–zag defects caused by the chevron-like arrangement of the molecules in the meso- phase. With regard to the design of calamitic liquid crystals displaying SmA and SmC phases and de Vries behaviour most previous work has focused on 5-phenylpyrimidines,39,41,43,48–53 2-phenylpyrimidines,54–58 biphenyls,40,44,59–64 thiadiazoles,54,65 aroylhydrazones66 and bent-core mesogens.37,67–74 Lemieux and Ivanov have reported independently for non-charged liquid crystals 3–5 that a rigid fluorenone core promotes formation of the SmC phase.75–80 As mentioned above, in contrast to neutral calamitic liquid crystals ILCs are rather reluctant to form SmC phases. Despite the few known examples,12–18 it is still an ongoing challenge to develop design principles for ILCs displaying both SmA and SmC phases. Moreover, ILCs with de Vries properties should provide a general insight into the driving forces for layer contraction and layer tilting of ionic mesogens and thus are relevant for electro-optic devices.81 Thus, we were curious, whether combination of a SmA-promoting unit, such as an imidazolium head group with a SmC-promoting calamitic core, i.e. fluorenone, tethered together via a flexible alkyl spacer would eventually lead to ILCs displaying both SmA and SmC phases and a minimal layer contraction, i.e. de Vries-like behaviour. Our results reveal that such merging of concepts is indeed successful and provides ILCs with a very broad mesophase stability, unique de Vries behaviour and solid state luminescence. The results are discussed below.Results and discussion
Synthesis of fluorenone imidazolium ILCs
In order to obtain structure–property relationships, a library of fluorenone ILCs Im(On,Om)X and ImR(On,Sm)Br was prepared (Scheme 2), where the following structural parameters were varied: alkoxy vs. thioether side chain with different chain lengths m, spacer lengths n, C-1 substituent R at the imidazolium head group and counterion. These target structures required a synthetic approach providing access to unsymmetrical fluorenones.As shown in Scheme 3, the synthesis commenced with a sequential Suzuki cross coupling82 of methyl 6-bromo-3-methoxybenzoate 6 with known borolanes 7a–f83–85 to give the biphenyl esters 8a–f.
Subsequent saponification86 provided biphenyl carboxylic acids 9a–f, which were submitted to Friedel–Crafts acylation87 via activation with thionyl chloride and reaction with AlCl3 to give the fluorenones 10a–f. For the selective deprotection of the methyl ether 10a–e two protocols employing thiols were examined.
According to a method by Magano88 N,N-diethyl-aminoethanethiol was deprotonated with NaOtBu in DMF at room temperature and then treated with the respective fluorenone 10a–e to give the hydroxyfluorenones 11a–e in moderate yields (method A). Alternatively, dodecanethiol was used together with NaH in DMF at 80 °C (method B) following a procedure by Chae89 resulting in higher yields of the desired hydroxyfluorenones 11b–e with alkoxy side chains.
For the corresponding hydroxy fluorenones 13 with thioethers, a nucleophilic displacement of aryl fluoride by thionucleophiles as reported by Kaszyński90 was planned as a key step. Upon treatment of 10f with 2.0 equiv. of dodecanethiol and 1.3 equiv. of NaH in DMF at 80 °C,90 the desired nucleophilic displacement competed with demethylation resulting in a mixture of 63% 12c and 22% of 13c (Scheme 3). This one-pot nucleophilic substitution/demethylation sequence could be optimized by employing equimolar amounts of thiol and NaH, e.g. giving 6% of 12c and 82% of the target alcohol 13c.
The hydroxyfluorenones were submitted to Williamson etherification with 1,ω-dibromoalkanes91 to provide the corresponding ω-bromoalkoxyfluorenones Br(On,Om) and Br(On,Sm) in good yields, followed by reaction with the methylimidazoles 16a–c (Scheme 3).16 While the 2-H-substituted imidazolium salts Im(On,Om)Br, Im(On,Sm)Br could be isolated in pure form after chromatography through HBr-treated silica, the corresponding 2-methyl- and 2-ethyl-substituted imidazolium bromides ImR(On,Sm)Br (R = Me, Et) required an additional recrystallization step, and thus lower overall yields were obtained. For a selected series Im(On,Om)Br the bromide counterion was replaced by triflate via salt metathesis92 (Scheme 3).
Mesomorphic properties of the ω-bromoalkoxyfluorenones
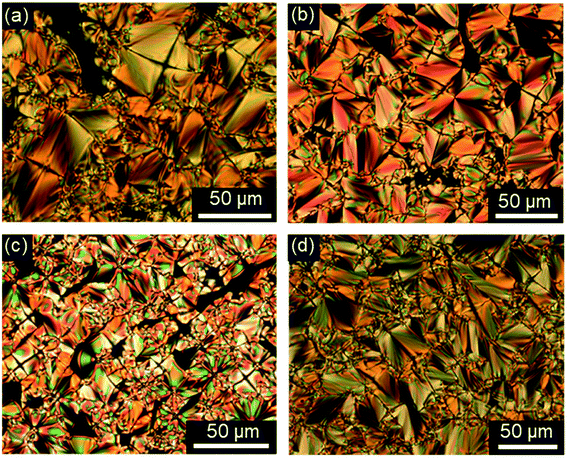
First, the mesomorphic properties of the ILC precursors, i.e. the ω-bromoalkoxyfluorenones Br(On,Ym) were examined by differential scanning calorimetry (DSC), polarizing optical microscopy (POM) and X-ray diffraction (WAXS, SAXS). The DSC data are summarized in Fig. S1–S3, Tables S1 and S2 (see ESI†). Both linking atoms Y, side chain lengths m and spacer length n had a pronounced influence on the phase behaviour of Br(On,Ym).All ω-bromoalkoxyfluorenones Br(On,Om) showed enantiotropic mesomorphism. The DSC curves reveal supercooling for the mesophase-to-crystalline transition upon cooling from the isotropic liquid while clearing temperatures are maintained in heating/cooling scans. It should be noted that supercooling is quite common among ILCs93–97 and was recently rationalized by cationic clustering.98 Under the POM Br(On,Om) displayed focal-conic fan textures, which are characteristic of SmA phases (Fig. 1a, b and d). The broadest SmA phase in the series Br(O6,Om) with various chain lengths m was found for Br(O6,O10). Upon variation of the spacer lengths n in the series Br(On,O12) the broadest mesophase widths of 21–22 K were detected for n = 8, 10. Thus both very short and very long spacers seem to disfavour lamellar mesophases. In contrast, only ω-bromoalkoxyfluorenones Br(O6,S10), Br(O8,S10), Br(O8,S12), and Br(O10,S14) with a thioether side chain showed monotropic mesomorphism upon cooling. The clearing transitions ranged between 55 and 63 °C and melting temperature between 30–58 °C. The decreased clearing temperatures and resulting destabilization of the SmA phase by thioethers agree well with previous reports on calamitic phenylpyrimidines.99,100 However, the increase of melting temperatures of Br(On,Sm) in comparison with Br(On,Om) is in contrast to these reports where thioethers led to lowering of melting points.
XRD experiments gave further insight into the phase geometry. The WAXS profile of an oriented sample of Br(O6,O8) showed a strong (001) layer reflection and a diffuse halo, which was tilted by 90° with respect to the (001) reflex, indicating an orthogonal SmA phase (Fig. 2a). In the SAXS profile a weak second order (002) reflection was visible besides the intense (001) reflection (Fig. 2b). From the (001) reflex the layer distance d was calculated according to equation nλ = 2d![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin
sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ and plotted against the temperature (Fig. S23, ESI†). Typical for SmA phases, layer distances d decreased with increasing temperature due to decreased orientational order with increasing temperature. Furthermore, with increasing chain lengths the d values increased by 2.5 Å, which is equivalent to two CH2 units. The exclusive formation of SmA phases of the ω-bromoalkoxyfluorenones Br(On,Ym) can be rationalized by the presence of SmA-promoting halogens as suggested by Giesselmann and Lemieux,101 i.e. reduced electrostatic repulsion between alkyl chains and improved van der Waals interaction between the aryl units rather than polar interactions at the interfaces between the smectic layers as was proposed by Goodby.102
θ and plotted against the temperature (Fig. S23, ESI†). Typical for SmA phases, layer distances d decreased with increasing temperature due to decreased orientational order with increasing temperature. Furthermore, with increasing chain lengths the d values increased by 2.5 Å, which is equivalent to two CH2 units. The exclusive formation of SmA phases of the ω-bromoalkoxyfluorenones Br(On,Ym) can be rationalized by the presence of SmA-promoting halogens as suggested by Giesselmann and Lemieux,101 i.e. reduced electrostatic repulsion between alkyl chains and improved van der Waals interaction between the aryl units rather than polar interactions at the interfaces between the smectic layers as was proposed by Goodby.102
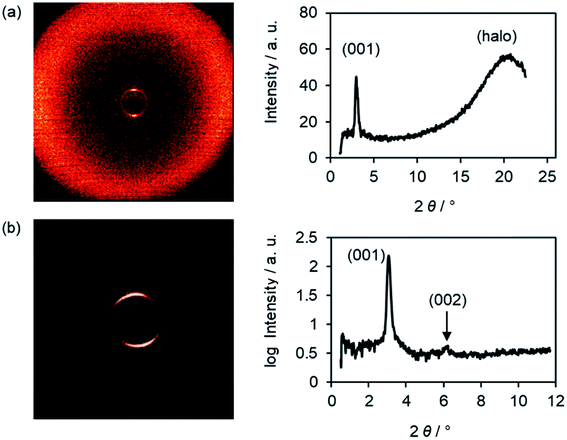 | ||
| Fig. 2 (a) Wide-angle scattering (WAXS) and (b) small-angle scattering (SAXS) profile and the corresponding diffraction pattern of Br(O6,O8) at 45 °C. | ||
Mesomorphic properties of the fluorenone ILCs
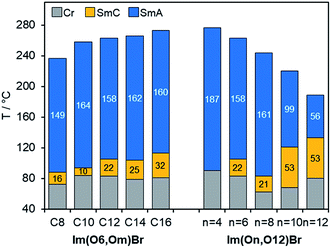
Phase transition temperatures and enthalpies of fluorenone ILCs Im(On,Om)X were determined by DSC from the first heating scans (Table S3 and Fig. S4, ESI†). Due to thermal decomposition of the samples caused by high isotropization temperatures in subsequent heating/cooling cycles no further transitions were detectable. In most cases SmC–SmA phase transitions were undetectable by DSC but were visible under the POM. Only in a few cases weak first order transitions with very small enthalpy changes were detected by DSC, which is in good agreement with previous work (Fig. S13–S17, ESI†).53,103 As exemplified for Im(O6,Om)Br in Fig. 3, the melting points remained relatively constant at 72–84 °C, while the clearing points were much stronger affected by the alkyl chain lengths m and increased from 237 °C for Im(O6,O8)Br up to 273 °C for Im(O6,O16)Br resulting in the broadest mesophase (192 K) for Im(O6,O16)Br. All ILCs within this series showed small SmC phases (10–32 K) and relatively broad SmA phases (149–164 K).In contrast, Im(O12,Om)Br (m = 10,12) with a long C12 spacer showed no evidence for decomposition and thus DSC curves of three heating/cooling cycles were fully reproducible (Fig. S4, ESI†). The small transition enthalpies of 0.5–0.6 kJ mol−1 are typical for SmC–SmA transitions.18
As shown for Im(On,O12)Br with increasing spacer lengths n clearing temperatures decreased considerably (Fig. 3), which can be rationalized by the increased flexibility of the ILC molecules with larger spacers resulting in a decreased lamellar order. This trend is in agreement with previous observations on azobenzene ILCs.17 It should be noted that the behaviour of ILCs consisting of cationic group–spacer–mesogenic core–side chain is more complex as compared to ILCs containing side chain–cationic group–side chain.
Melting temperatures changed only little (68–90 °C), however, the stability of the SmC phase was strongly affected by variation of the spacer lengths n. While Im(O4,O12)Br showed only a monotropic SmA phase, derivatives with n > 4 displayed also enantiotropic SmC phases under the POM. Furthermore, the stability increased with increasing spacer lengths n, resulting in the broadest SmC phase (53 K) for n = 10, 12, while SmC phase widths of 21–22 K were found for derivatives with n = 6, 8 (Fig. 3).
Various experimental studies from the literature20,104 including very recent theoretical work by Saielli105 described a strong influence of the counterion on transition temperatures and mesophase type. Therefore, we surmised that an exchange of the counterion might be helpful to overcome the thermal decomposition. However, the triflate counterion decreased the clearing temperature significantly to 114–130 °C only for Im(O6,O8)OTf and Im(O8,O10)OTf. The triflate was stable and the complete phase sequence was reproducible over three heating/cooling cycles (Fig. S5, ESI†). ILCs Im(On,O14)OTf (n = 4, 6) showed only a slight decrease of the clearing temperature and thus isotropization was still accompanied by thermal decomposition. Most importantly, the triflate counterion affected the mesophase type, resulting in loss of the SmC phase. Similar results were reported by Trbojevic for guanidinium ILCs connected via a flexible alkyl spacer to a rod-like biphenyl unit,15 where replacement of chloride by triflate decreased the clearing transition but suppressed the SmC phase in favor of the SmA phase.
The phase behaviour of fluorenone imidazolium bromides Im(On,Sm)Br carrying thioether side chains was similar to that of the corresponding Im(On,Om)Br. As shown for Im(On,S12)Br with dodecylthio side chain, clearing temperatures decreased with increasing spacer lengths n (R = H, Fig. 4). Thioethers Im(On,S12)Br with short spacers n = 4, 6, 8 formed only SmA phases with broad phase widths of 154–206 K, whereas the homologous ILCs with longer spacers (n = 10, 12) displayed additional SmC phases. However, the phase stability was not increased by longer spacers. The replacement of oxygen with sulfur resulted in a significant destabilization of the SmC phase, and the phase ranges decreased to 12–17 K as compared to the corresponding ILCs Im(On,O12)Br (53 K).
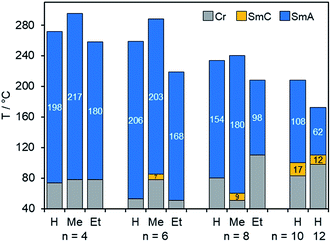 | ||
| Fig. 4 Comparison of mesophase stabilities of ILCs ImR(On,S12)Br with variable spacer lengths n upon heating. The values in the bar diagram correspond to the mesophase temperature ranges. Phase transitions were determined by DSC (1st heating) and POM (SmA–SmC transition), for Im(On,S12)Br (n = 10, 12) 3rd heating (see Tables S4 and S5, ESI†). | ||
The influence of substituent R at C-1 of the imidazolium head group on the mesophase stability of ILCs ImR(On,S12)Br is also shown in Fig. 4. Bromides ImR(On,S12)Br displayed broad SmA phases with phase widths ranging from 62–217 K. Within this series only the ILCs with H- or methyl-substituted imidazolium head group ImH(On,S12)Br and ImMe(On,S12)Br formed enantiotropic SmC phases with minimum spacer lengths of n = 10 (for R = H) and n = 6 (for R = Me). The corresponding ILCs ImEt(On,S12)Br showed only monotropic SmC phases under the POM upon cooling (Fig. 7 and S18–S20, ESI†). The clearing points were affected by substituent R. For example, for ImR(O4,S12)Br with a short C4 spacer the clearing point increased from 272 °C (R = H) to 295 °C (R = Me) and decreased to 258 °C (R = Et). It should be noted that ethyl-substituted imidazolium ILCs were still prone to thermal decomposition. These results are in good agreement with previous work by Swager,106 Butschies92 and Kapernaum.17
To obtain further information on the thermal stability, ILCs Im(On,O14)Br (n = 4, 6) and Im(O8,O16)Br with clearing transitions >270 °C were submitted to thermogravimetric analysis (TGA) (Fig. 5). The ILCs were stable up to 200 °C (black curves). Upon further heating thermal decomposition was accompanied by loss of mass. The clearing points (grey zone) are located above the decomposition temperatures. As compared to the bromides, imidazolium triflate Im(O4,O14)OTf showed a slightly improved thermal stability (red curve), however, the clearing point (243 °C) is still within the decomposition range. Thus, TGA experiments confirmed the DSC results. Presumably the triflate anion is less nucleophilic than bromide and does not cause thermally induced dealkylations of the imidazolium unit. When comparing the DSC results of Im(On,Om)Br with Im(On,Om)OTf (Table S3, ESI†), it is remarkable that for imidazolium salts with large length differences between spacer n and side chain m, the clearing temperature decreased by ∼50 K, whereas the clearing temperature of imidazolium salts with similar lengths n, m decreased by >100 K.
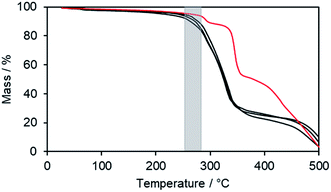 | ||
| Fig. 5 TGA thermograms of imidazolium bromides Im(On,O14)Br (n = 4,6), Im(O8,O16)Br (black lines) and triflate Im(O4,O14)OTf (red line). The grey zone illustrates the clearing temperature range. | ||
Investigations of fluorenone imidazolium bromides Im(On,Om)Br with alkoxy side chains under the POM showed large areas of homeotropic alignment. Birefringent areas could only be observed at phase boundaries and air bubbles (Fig. S13–S17, ESI†). ILCs Im(O4,Om)Br only showed fan textures (Fig. S13, ESI†), while imidazolium bromides forming SmA and SmC phases displayed both fan textures characteristic of SmA and at lower temperatures broken fan and Schlieren textures typical for SmC phases,107 as shown in Fig. 6 for Im(O10,O12)Br.
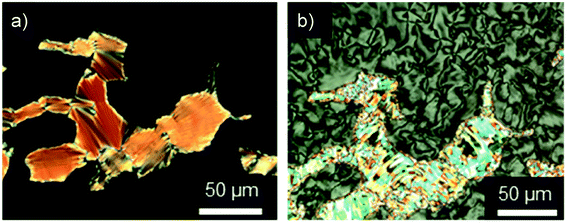 | ||
| Fig. 6 Textures of Im(O10,O12)Br as seen between crossed polarizers at (a) 132 °C and (b) 121 °C upon cooling from the isotropic liquid (cooling rate 5 K min−1, magnification ×200). | ||
The POM studies provided initial hints for de Vries-like behaviour. The color change of the fan (SmA)/broken fan (SmC) texture of Im(O10,O12)Br during SmA–SmC phase transition from orange brown to pale blue indicated an increase in birefringence in the SmC phase and thus an increased orientational order, which is reported to be characteristic for de Vries-like materials.30,54,55,65,103,108
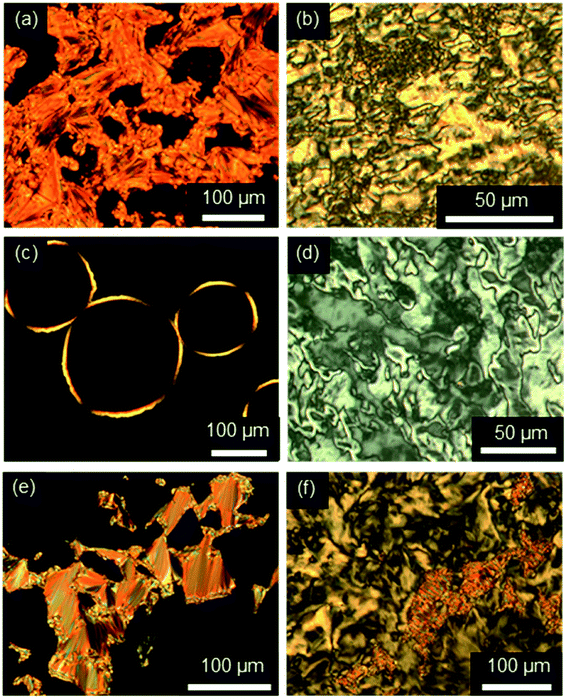
Fluorenone imidazolium bromides ImR(On,Sm)Br with thio-ether side chains formed fan and Bâtonnet textures or large homeotropic areas in the SmA phase and at lower temperatures Schlieren textures typical for SmC phases under the POM (Fig. 7). While for ILCs ImR(On,Sm)Br with R = H Schlieren textures occurred at a minimum spacer length n = 8 and side chain length m = 14, for the corresponding ILCs ImMe(On,Sm)Br and ImEt(On,Sm)Br Schlieren textures appeared already for spacer lengths n = 6.
The strong tendency of the ILCs for homeotropic alignment in the SmA phase could be suppressed by using nylon-coated glass cells (1.6 μm). For example, upon cooling from the isotropic liquid ILCs Im(O10,Y10)Br displayed well-developed fan textures (Fig. 8a and c), which transformed into broken fan textures upon further cooling (Fig. 8b and d).
As expected from temperature-dependent SAXS experiments, layer distances d in the SmA phase of Im(On,Om)X and ImR(On,Sm)Br decreased with increasing temperature (Fig. S23 and S24, ESI†). The experimentally determined layer distances d were almost twice as large as compared to the calculated molecular lengths Lcalc (molecular mechanics, force field: MMFF94s; software Avogadro109) of an all-trans fully extended conformation. The ratios d/Lcalc were approximately 1.7–1.8 suggesting the presence of partially interdigitated smectic bilayers, that are also very prominent in de Vries-like materials (Tables S7 and S8, ESI†).
Investigation of de Vries-like properties of the fluorenone ILCs
In the WAXS pattern of the SmA phase of Im(O12,O10)Br at 169 °C three sharp reflexes in the small angle region and a diffuse maximum (halo) in the wide angle section are visible (Fig. 9a). The XRD pattern remained similar during SmA–SmC transition (Fig. 9b), because ILCs ImR(On,Ym)X did not give oriented samples.17,18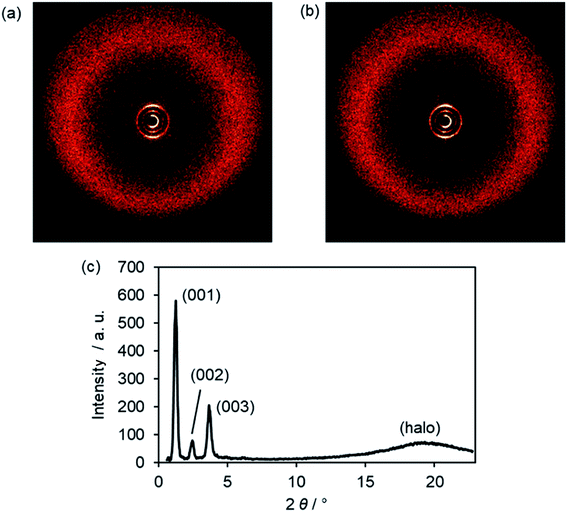 | ||
| Fig. 9 (a) WAXS diffraction image of Im(O12,O10)Br at 169 °C in the SmA phase, (b) WAXS diffraction image and (c) the corresponding scattering profile of Im(O12,O10)Br at 83 °C in the SmC phase. | ||
High order layer reflections up to 4th order (004) could be observed for ILCs ImR(On,Ym)X (Table S8, ESI†), suggesting that the smectic phases of the ILCs possess a high degree of translational order presumably due to the strong nano-segregating effect of the ionic head group.21,30,110
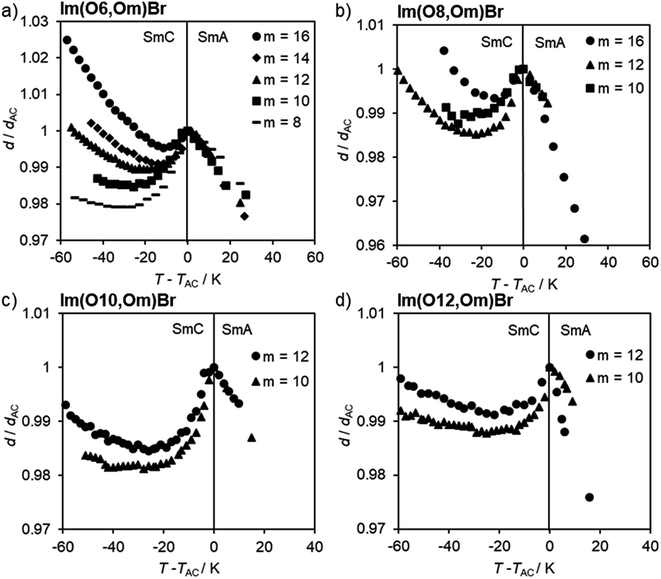
One parameter for de Vries-like behaviour is the maximum layer contraction at the SmA–SmC phase transition. Therefore, the smectic layer spacing d of ILCs ImR(On,Ym)Br as a function of temperature was measured by small-angle X-ray scattering (SAXS). The layer distances d were calculated from the (001) reflex at different temperatures, where dAC denotes the layer distance at the SmA–SmC transition temperature TAC. At the temperature where the largest layer shrinkage occurred, the maximum layer contraction Smax was determined.
The profiles of normalized layer spacings d/dAC vs. reduced temperature T − TAC for ILCs Im(On,Om)Br with an alkoxy side chain are summarized in Fig. 10 and Table 1. Estimated standard deviations were <5%. The temperature-dependent d/dAC curves are similar in this series. Upon cooling an expansion in the SmA phase until the transition temperature was observed. At transition into the SmC phase, the layer spacing initially decreased and then increased upon further cooling. This behaviour is typical for de Vries-like materials, while in conventional liquid crystals the layer thickness decreases continuously in the SmC phase resulting in larger layer contractions.50 As seen in Fig. 10a, the maximum layer contraction Smax decreases with the length m of the alkoxy side chain, from 2.1% for Im(O6,O8)Br to 0.5% for Im(O6,O16)Br (Table 1).
| Compound | T − TAC/K | dAC/Å | dC/Å | Smax/% |
|---|---|---|---|---|
| Im(O6,O8)Br | −30 | 57.7 | 56.5 | 2.1 |
| Im(O6,O10)Br | −26 | 63.8 | 62.8 | 1.5 |
| Im(O6,O12)Br | −19 | 63.7 | 63.1 | 1.1 |
| Im(O6,O14)Br | −14 | 69.9 | 69.3 | 0.9 |
| Im(O6,O16)Br | −11 | 70.3 | 70.0 | 0.5 |
| Im(O8,O10)Br | −31 | 66.0 | 65.2 | 1.4 |
| Im(O8,O12)Br | −23 | 66.3 | 65.3 | 1.5 |
| Im(O8,O16)Br | −14 | 74.1 | 73.6 | 0.7 |
| Im(O10,O10)Br | −28 | 64.7 | 63.4 | 1.9 |
| Im(O10,O12)Br | −26 | 68.0 | 67.0 | 1.5 |
| Im(O12,O10)Br | −25 | 66.7 | 65.9 | 1.2 |
| Im(O12,O12)Br | −22 | 72.0 | 71.4 | 0.9 |
| Im(O8,S14)Br | −10 | 72.9 | 72.6 | 0.4 |
| Im(O10,S10)Br | −13 | 68.9 | 68.1 | 1.1 |
| Im(O10,S12)Br | −14 | 72.1 | 71.4 | 0.9 |
| Im(O10,S14)Br | −6 | 72.8 | 72.4 | 0.5 |
| Im(O12,S10)Br | −25 | 71.1 | 70.4 | 1.0 |
| Im(O12,S12)Br | −6 | 74.5 | 73.8 | 0.9 |
| Im(O12,S14)Br | −10 | 76.8 | 76.5 | 0.4 |
| ImMe(O6,S12)Br | −17 | 69.4 | 68.8 | 0.9 |
| ImMe(O6,S14)Br | −11 | 71.1 | 70.6 | 0.7 |
| ImMe(O8,S12)Br | −20 | 70.7 | 70.0 | 1.0 |
| ImMe(O8,S14)Br | −19 | 71.9 | 71.2 | 1.1 |
| ImEt(O6,S12)Br | −14 | 66.5 | 65.7 | 1.1 |
| ImEt(O8,S10)Br | −11 | 67.3 | 66.5 | 1.2 |
| ImEt(O8,S12)Br | −17 | 70.7 | 69.8 | 1.4 |
A similar trend was observed for Im(O10,Om)Br and Im(O12,Om)Br (Fig. 10c and d). However, in the series of Im(O8,Om)Br the trend was less clearcut. ILC Im(O8,O16)Br exhibited a maximum layer contraction Smax = 0.7%, but Im(O8,Om)Br with m = 10, 12 gave similar Smax values of 1.5% and 1.4% (Fig. 10b and Table 1).
According to the d/dAC vs. T − TAC profiles, ILCs Im(On,Sm)Br with thioether side behaved similarly, i.e. the maximum layer contractions decrease with increasing side chain length m (Fig. 11a, b and Table 1). In contrast, the maximum layer contraction of the ILCs ImMe(On,Sm)Br and ImEt(On,Sm)Br with methyl or ethyl substituents at the imidazolium unit (R = Me, Et) did not show any clear trend (Fig. 11c, d and Table 1).
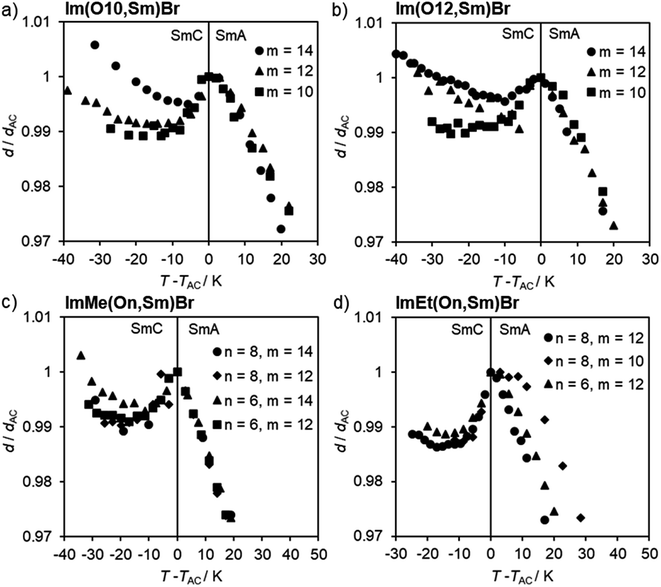 | ||
| Fig. 11 Layer spacing d/dAC vs. reduced temperature T − TAC profiles of ImR(On,Sm)Br with R = H (a and b), Me (c), Et (d). | ||
The alkyl chain lengths effect on the layer contraction might be rationalized by the interdigitation with longer chains showing a higher degree of interdigitation as compared to shorter chains in agreement with recent studies by Wöhrle on SmA phases of MIDA boronates.111 The determined d/Lcalc ratios, however, did not show a clear trend (Tables S7 and S8, ESI†). For example, the Im(O6,Om)Br series revealed dAC/Lcalc ratios at the SmA–SmC phase transition of 1.79 (m = 8), 1.84 (m = 10), 1.71 (m = 12), 1.76 (m = 14), and 1.67 (m = 16). The pronounced color change of the textures observed for ImR(On,Ym)Br by POM during SmA–SmC transition indicated an increase in birefringence, which correlates with an increase in orientational order parameter S2.45–47,53 Therefore, we assume that the orientational order increased in the SmC phase and compensation for a large portion of the maximum layer contraction. However, due to unavailability of oriented samples of fluorenone ILCs ImR(On,Ym)Br for XRD studies we failed to clarify which effect compensates layer shrinkage and is responsible for the de Vries-like properties.
An alternative method to rationalize the beneficial influence of the alkyl chain lengths on the layer contraction is the so-called zig–zag model, originally proposed by Bartolino, Doucet and Durand,112 and later confirmed by Böffel113 and Clark.114 In the zig–zag model the rigid cores are tilted to larger extent than the aliphatic chains in the SmC phase, i.e. the tilt of the layers is mainly caused by the tilted orientation of the rigid aromatic cores rather than the alkyl chains (Fig. 12).
The zig–zag model has also been implemented in the Boulder model by Walba and Clark to explain the polarization of ferroelectric liquid crystals.115–117 According to this model an increase of the non-tilted alkyl chains should lead to a smaller layer contraction at the SmA–SmC phase transition.
The de Vries-like behaviour can be quantified by the reduction factor R, which considers both maximum layer contraction and optical tilt angle θopt in the SmC phase measured as a function of temperature by POM.18,53,55,65,103 As the samples were filled in ITO glass cells with rubbed nylon alignment layer (1.6 μm thickness) by isotropic heating, only those ILCs could be investigated, which were thermally stable above the isotropization temperature based on their reproducible DSC curves. As an example, planar textures of Im(O12,O10)Br are shown in Fig. 13. In the SmA phase uniform domains are visible due to a parallel orientation of the director n with respect to the layer normal k (Fig. 13a), while in the SmC phase two different domain structures with different brightness are visible (Fig. 13b and c).
Optical tilt angles θopt were measured by POM as function of reduced temperature T − TAC in the absence of an electrical field upon cooling. In the SmA phase the director n is parallel to the layer normal k and thus θopt = 0 (Fig. 14).
 | ||
| Fig. 14 Optical tilt angle θopt vs. reduced temperature T − TAC for (a) Im(On,Om)Br and (b) Im(On,Sm)Br. For θopt(T) profiles fitted to the power law according to ref. 103 see Fig. S25, ESI.† | ||
At T − TAC = 0, the optical tilt angle θopt of the SmC phase increased significantly and then steadily increased upon further cooling. The abrupt increase of θopt indicates a 1st order SmA–SmC transition. This is in accordance with the DSC measurements, where for several compounds small peaks at the transitions from SmA to SmC were observed. In the series of ILCs with alkoxy side chain Im(On,Om)Br θopt increased up to 36° (Fig. 14a). The optical tilt angle profile of ILCs with thioether side chain Im(On,Sm)Br reveals slightly lower optical tilt angles (28–32°) (Fig. 14b). No general trend regarding spacer lengths n or alkyl chain lengths m could be detected.
From the maximum layer contraction and the optical tilt angle θopt the reduction factor R was calculated according to equation R = δ(T)/θopt(T) = cos−1[dC(T)/dAC]/θopt(T) for a given temperature.53,118 The R values, maximum layer contraction Smax and optical tilt angle θopt at the reduced temperature T − TAC are summarized in Table 2.
| Compound | T −;TAC/K | dAC/Å | dC/Å | Smax/% | θopt/° | R |
|---|---|---|---|---|---|---|
| Im(O10,O10)Br | −28 | 64.7 | 63.4 | 1.9 | 28 | 0.40 |
| Im(O12,O10)Br | −25 | 66.7 | 65.9 | 1.2 | 30 | 0.30 |
| Im(O12,O12)Br | −22 | 72.0 | 71.4 | 0.9 | 29 | 0.26 |
| Im(O10,S10)Br | −13 | 68.9 | 68.1 | 1.1 | 24 | 0.35 |
| Im(O10,S12)Br | −14 | 72.1 | 71.4 | 0.9 | 23 | 0.33 |
| Im(O12,S10)Br | −25 | 71.1 | 70.4 | 1.0 | 31 | 0.26 |
| Im(O12,S12)Br | −6 | 74.5 | 73.8 | 0.9 | 19 | 0.41 |
| Im(O12,S14)Br | −10 | 76.8 | 76.5 | 0.4 | 27 | 0.20 |
The ILCs have R values ranging from 0.20 to 0.41 indicating a high de Vries-like behaviour. Thus, our design concept not only induced the rare SmC phase but also enabled de Vries behaviour with layer contractions Smax and R values, which are comparable to those of known neutral liquid crystalline de Vries materials50,53,65 and further extends the scaffolds of ILCs previously developed by Kapernaum.17,18 Moreover, it confirms that fluorenones are beneficial for de Vries ILCs. In agreement with the procedure reported by Lemieux103 the θopt(T) profiles were fitted to the power law,103 which provided the order parameter β related to the SmA–SmC transition (see also ref. 24 in Lemieux103). The ILCs possessed β values of 0.19–0.24, i.e. indicating weak 1st order transitions or tricritical point transitions (in case of β = 0.25) in agreement with Lemieux' observations (for details see Fig. S25, ESI†).
Calculation of the electron density
Based on layer reflections from SAXS experiments the electron density distribution in the smectic phase was obtained. The electron density profile ρ(z) along the director n was calculated by using eqn (1).119
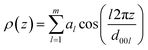 | (1) |
From the intensity of the Bragg reflections (00l) and subsequent correction via the Lorentz factor120 the square values |al|2 were determined, while the signs of the al coefficients remained indefinite.121 Therefore, all possible combinations of signs of the different al coefficients must be generated and submitted to a plausibility check. The imidazolium bromides Im(On,Ym)Br showed three layer reflections and therefore three coefficients a1, a2 and a3 could be determined. The results are summarized in Table 3.
| T/°C | d001/Å | d002/Å | d003/Å | a1 | a2 | a3 | |
|---|---|---|---|---|---|---|---|
| Im(O12,O10)Br | 167 | 66.7 | 33.3 | 22.1 | 1 | 0.31 | 0.52 |
| Im(O12,S10)Br | 160 | 67.7 | 33.8 | 22.4 | 1 | 0.32 | 0.60 |
| Im(O12,O12)Br | 176 | 71.1 | 35.6 | 23.5 | 1 | 0.29 | 0.41 |
| Im(O12,S12)Br | 164 | 69.9 | 34.8 | 23.1 | 1 | 0.38 | 0.55 |
The electron density profile and the packing model of Im(O12,O10)Br are shown in Fig. 15. A large maximum of the electron density ρ(z) is visible in the region of the ionic head groups, forming a charged sublayer. In the region of the aliphatic spacer a local minimum of the electron density ρ(z) is visible and a local maximum due to the aromatic fluorenone core. Along the terminal alkoxy side chains, the electron density ρ(z) shows a global minimum. For the other fluorenones in Table 3 analogous density profiles were obtained (Fig. S26, ESI†).
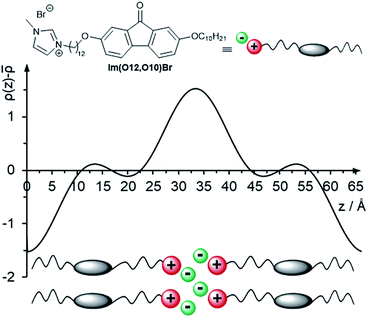 | ||
| Fig. 15 Electron density profile of Im(O12,O10)Br in the SmA phase at 167 °C indicating a bilayer structure and its suggested packing model. | ||
Absorption and emission properties of fluorenone precursors and ILCs
UV/vis data of imidazolium salts Im(On,Ym)X and their precursors are shown in Fig. 16. All fluorenone derivatives with an alkoxy side chain displayed absorption maxima at λmax = 272–274 nm with a shoulder at lower wavelengths and two smaller bands at 302 nm and 314 nm, respectively (Fig. 16a).122 The intense bands at 272–274 nm were assigned to the π–π* transition of the fluorenone core.123,124 The UV/vis spectra were neither affected by different donor groups at the fluorenone core, i.e. methoxy, alkoxy or hydroxy nor different anions (bromide vs. triflate). However, the replacement of alkoxy by thioether side chains resulted in a bathochromic shift of the absorption maxima to λmax = 282–283 nm (Fig. 16b).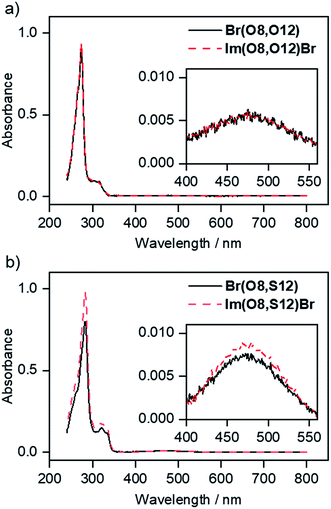 | ||
| Fig. 16 Absorbance spectra (in CHCl3) of (a) precursor fluorenone Br(O8,O12) and ILC Im(O8,O12)Br with alkoxy side chains and (b) precursor fluorenone Br(O8,S12) and ILC Im(O8,S12)Br with thioether side chains. For details of the fluorescence spectra in solution see Fig. S28 and S29 and the corresponding text in the ESI.† | ||
This effect has been used for organic solar cells and field effect transistors to decrease the HOMO–LUMO gap and to improve the charge carrier mobility.125 In contrast, the strongly electron-withdrawing fluoro substituent in 10f shifted the absorption maximum hypsochromically to λmax = 267 nm (Fig. S27†). All studied fluorenones were orange-red amorphous solids due to a low absorption in the visible region. A weak band around 400 nm was assigned to symmetry-forbidden n–π* transition of the carbonyl group (Fig. 16, inset).123,124
For potential applications bulk emission properties are particularly interesting. Therefore, selected samples were studied by solid state fluorescence spectroscopy utilizing a polarizing optical microscope equipped with UV light source (λexc = 350–380 nm) and photodetector. The method is limited to thermally stable compounds, because the samples required isotropization and thermal annealing to obtain uniform thin films for solid state emission spectra. The solid state emission spectra of Br(O6,O16), exemplified in Fig. 17a, displayed at 25 °C a strong band at λem = 604 nm with two shoulders at longer wavelengths caused by the excimer emission due to fluorenone–fluorenone interactions.122 At higher temperatures the intensity of the shoulder at longer wavelengths (635 nm) resulting from vibronic coupling increased.
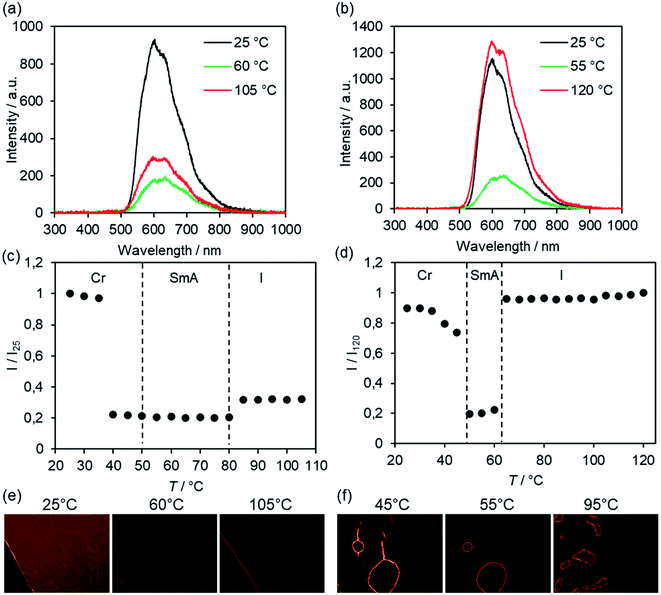 | ||
| Fig. 17 Emission spectra of (a) Br(O6,O16) and (b) Br(O8,S12) at different temperatures (λexc = 350–380 nm). Temperature-dependent emission intensity of (b) Br(O6,O16) and (d) Br(O8,S12) upon cooling from the isotropic liquid (cooling rate 5 K min−1). Transition temperatures (dashed lines) were determined from the 3rd cooling scan by DSC (Tables S1 and S2, ESI†). POM pictures of (e) Br(O6,O16) and (f) Br(O8,S12) under irradiation with UV light at the given temperatures, irradiation time 8 s (e) and 4 s (f). POM pictures were taken without analyzer in order to detect sufficient emission intensity. | ||
The emission intensity was measured as a function of temperature upon cooling from the isotropic liquid (Fig. 17c). In the isotropic liquid the emission intensity was only 30% of the intensity at 25 °C (I25). The I80/I25 value decreased to 0.2 (20%) in the SmA phase and remained constant throughout the whole temperature range of the phase. Upon transition into the crystalline phase at 35 °C a strong increase of the emission was visible. It should be noted, that the SmA–Cr transition was detected at 51 °C by DSC (dashed line in Fig. 17c). The different emission intensities could also be observed via the POM pictures under irradiation with UV light (Fig. 17e).
A different behaviour of the bulk emission was observed for fluorenone Br(O8,S12) with thioether side chain (Fig. 17b, d and f). A strong emission at λem = 600 nm was observed in the isotropic liquid. Upon cooling and at the I–SmA transition the intensity decreased only slightly, but at 60 °C in the SmA phase, the intensity decreased steeply to 20% of the intensity of the isotropic phase. At the SmA–Cr transition again a steep increase of the emission was detected, with the intensity being slightly below the emission intensity of the isotropic phase. In other words, the strongest fluorescence was observed at 120 °C. This result is remarkable, because the strongest emission would be expected at low temperature in the highly ordered crystalline phase rather than in the disordered isotropic phase. Presumably, the observed band at 600 nm corresponds to the excimer emission and thus, comparison of ether and thioether derivatives suggests that the interaction between fluorenones in the thioether derivative is more pronounced in the isotropic and crystalline phase as compared to the SmA phase.126
The bulk emission behaviour of fluorenone imidazolium triflate Im(O6,O8)OTf resembled that of Br(O6,O16). Upon heating of the crystalline phase the emission intensity decreased slightly, but decreased steeply at 80 °C at the Cr–SmA transition to a 10 times lower intensity than that in the Cr phase and remained constant upon further heating and did not increase upon isotropic clearing (Fig. S30, ESI†).
Conclusion
We developed a synthetic strategy to a series of ILCs ImR(On,Ym)X consisting of a SmC-promoting central fluorenone core with one alkoxy or thioether side chain and a flexible spacer connected to a SmA-promoting imidazolium head group. Precursor fluorenone bromides Br(On,Ym) formed only SmA mesophases, while the fluorenone ILCs Im(On,Om)Br carrying an alkoxy side chain indeed displayed both SmA and SmC mesophases. Thereby spacer lengths n > 10 increased the stability of the SmC phase up to phase widths of 53 K. Attempts to overcome the high clearing points of ILCs Im(On,Om)Br, being close to the thermal decomposition temperature via exchange of the bromides against triflate counterions resulted indeed in decreased clearing temperatures. However, the desired effect was accompanied by the loss of the SmC phase and thus triflate was not further considered in these ILCs. ILCs ImR(On,Sm)Br with thioether side chain behaved similarly as compared to the corresponding ether derivatives Im(On,Om)Br. In this series enantiotropic SmC mesophases were observed for the H- and Me-substituted imidazolium group with minimum spacer lengths n = 10 (R = H) and n = 6 (R = Me), while the ethyl substituent (R = Et) caused monotropic SmC phases upon cooling. Fluorenone ILCs Im(On,Sm)Br carrying thioethers displayed broader SmC phases as compared to the corresponding ether derivatives Im(On,Om)Br, while the opposite trend was observed for the temperature range of the SmA phase, suggesting that S–S interactions are beneficial for SmC phases in ILCs. Alternatively, the larger sulfur in the thioether side chains might be better accommodated in a tilted than a non-tilted orientation.POM observations of a color change of the textures during the SmA–SmC phase transition indicated an increase in the birefringence of the SmC phase which is correlated with an increased orientational order as expected for de Vries-like materials.30,108 In XRD measurements higher order reflections were visible suggesting a high degree of translational order in the smectic phase which may originate from strong nano-segregation of the ionic imidazolium head group.30 From d/dAC vs. T − TAC profiles maximum layer contraction values Smax ranging from 0.4 to 2.1% were obtained. The optical tilt angles θopt measured by POM, and finally the calculation of the reduction factor R based on layer spacings d/dAC and θopt values gave further evidence for de Vries-like behaviour. The R values of ILCs ImR(On,Ym)Br ranged from 0.2 to 0.41 and are comparable to those of known neutral liquid crystalline compounds.50,53,65 The most promising de Vries ILC was thioether derivative Im(O12,S14)Br (Smax = 0.4%, R = 0.20).
Emission spectra of selected derivatives revealed “off–on” effects because the emission intensity strongly depended on the phase type (crystalline, liquid crystalline, isotropic). For bromides Br(On,Om) with an alkoxy side chain, the highest intensity observed in the crystalline state steeply decreased at the Cr–SmA transition and increased in the isotropic liquid. Bromide Br(O8,S12) with thioether side chain showed a reverse behaviour, i.e. a steep decrease of highest intensity in the isotropic liquid upon SmA transition followed by an increase at the SmA–Cr phase transition. The emission intensity profile of ILC Im(O6,O8)OTf resembled that of Br(On,Om) with the strongest intensity in the crystalline state. After abrupt reduction at the Cr–SmA transition, however, the intensity remained nearly constant also in the isotropic liquid.
In conclusion, we have demonstrated that the combination of charged imidazolium head groups with calamitic fluorenones carrying a lateral polar group and a flexible tether generates ILCs with remarkably low layer shrinkage during SmA–SmC transition. Moreover, fluorenone ILCs and their precursor bromides possess a strong solid state luminescence, which is switched off in the SmA phase and in case of Br(O8,S12) is recovered in the isotropic phase. The emission observed in the solid state is the excimer emission corresponding to the interaction between fluorenone moieties, indicating aggregation-induced emission (AIE) behaviour,127,128 which is much stronger in the isotropic and crystalline phase than in the SmA phase for Br(O8,S12). The phenomenon is less pronounced for the fully oxygenated compound. Thus, our approach has provided access to novel de Vries as well as thermoluminescent materials.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work was supported by the Deutsche Forschungsgemeinschaft (LA 907/17-1 and LA 907/20-1, project SNAPSTER), the ANR (ANR-18-CE92-0026-01), the Deutsche Akademische Austauschdienst (DAAD Procope project PLISE), Ministère de l'Europe et des Affaires étrangères, Ministère de l'Enseignement supérieur, de la Recherche et de l'Innovation (PHC Procope), the Ministerium für Wissenschaft, Forschung und Kunst des Landes Baden-Württemberg and the Carl-Schneider-Stiftung Aalen (shared instrumentation grant). We would like to thank Prof. Frank Giesselmann for helpful discussions.References
- K. Goossens, K. Lava, C. W. Bielawski and K. Binnemans, Chem. Rev., 2016, 116, 4643–4807 CrossRef CAS PubMed.
- M. Mansueto and S. Laschat, in Handbook of Liquid Crystals, ed. J. W. Goodby, P. J. Collings, T. Kato, C. Tschierske, H. Gleeson and P. Raynes, Wiley-VCH, Weinheim, 2nd edn, 2014, vol. 6, pp. 231–280 Search PubMed.
- S. Chen and S. H. Eichhorn, Isr. J. Chem., 2012, 52, 830–843 CrossRef CAS.
- K. V. Axenov and S. Laschat, Materials, 2011, 4, 206–259 CrossRef CAS PubMed.
- L. Douce, J.-M. Suisse, D. Guillon and A. Taubert, Liq. Cryst., 2011, 38, 1653–1661 CrossRef CAS.
- K. Binnemans, Chem. Rev., 2005, 105, 4148–4204 CrossRef CAS PubMed.
- V. Causin and G. Saielli, in Green Solvents II: Properties and Applications of Ionic Liquids, ed. A. Mohammad and D. Imamuddin, Springer, Dordrecht, 2012, pp. 79–118 Search PubMed.
- S. K. Pal and S. Kumar, in Biosensors Nanotechnology, ed. A. Tiwani and A. P. F. Turner, Scrivener Publishing, Berkeley, MA 2014, pp. 267–314 Search PubMed.
- D. Högberg, B. Soberats, R. Yatagai, S. Uchida, M. Yoshio, L. Kloo, H. Segawa and T. Kato, Chem. Mater., 2016, 28, 6493–6500 CrossRef.
- R. Atasiei, M. Raicopol, C. Andronescu, A. Hanganu, A. L. Alexe-Ionescu and G. Barbero, J. Mol. Liq., 2018, 267, 81–88 CrossRef CAS.
- F. Yuan, S. Chi, S. Dong, X. Zou, S. Lv, L. Bao and J. Wang, Electrochim. Acta, 2019, 294, 249–259 CrossRef CAS.
- K. Stappert and A.-V. Mudring, RSC Adv., 2015, 5, 16886–16896 RSC.
- S. Kohmoto, Y. Hara and K. Kishikawa, Tetrahedron Lett., 2010, 51, 1508–1511 CrossRef CAS.
- K. Goossens, K. Lava, P. Nockemann, T. Van Hecke, L. Van Meervelt, P. Pattison, K. Binnemans and T. Cardinaels, Langmuir, 2009, 25, 5881–5897 CrossRef CAS PubMed.
- N. Trbojevic, J. C. Haenle, T. Wöhrle, J. Kirres and S. Laschat, Liq. Cryst., 2016, 43, 1135–1147 CrossRef CAS.
- G. F. Starkulla, S. Klenk, M. Butschies, S. Tussetschläger and S. Laschat, J. Mater. Chem., 2012, 22, 21987–21997 RSC.
- N. Kapernaum, E. Wuckert, W. Frey, S. Marino, M. Wahl, F. Giesselmann and S. Laschat, J. Phys. Org. Chem., 2018, 31, e3779 CrossRef.
- N. Kapernaum, C. Müller, S. Moors, M. C. Schlick, E. Wuckert, S. Laschat and F. Giesselmann, ChemPhysChem, 2016, 17, 4116–4123 CrossRef CAS PubMed.
- A. Sanchez-Castillo, M. A. Osipov, S. Jagiella, Z. H. Nguyen, M. Kašpar, V. Hamplova, J. Maclennan and F. Giesselmann, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2012, 85, 061703 CrossRef CAS PubMed.
- W. Haase, Z. X. Fan and H. J. Müller, J. Chem. Phys., 1988, 89, 3317–3322 CrossRef CAS.
- A. J. Leadbetter and E. K. Norris, Mol. Phys., 1979, 38, 669–686 CrossRef CAS.
- W. L. McMillan, Phys. Rev. A: At., Mol., Opt. Phys., 1972, 6, 936–947 CrossRef CAS.
- N. Kapernaum and F. Giesselmann, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2008, 78, 062701 CrossRef PubMed.
- J. Watanabe and M. Hayashi, Macromolecules, 1989, 22, 4083–4088 CrossRef CAS.
- E. F. Gramsbergen and W. H. De Jeu, Liq. Cryst., 1989, 4, 449–455 CrossRef CAS.
- Y. Takanishi, A. Ikeda, H. Takezoe and A. Fukuda, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 1995, 51, 400–406 CrossRef CAS PubMed.
- D. Nonnenmacher, S. Jagiella, Q. X. Song, R. P. Lemieux and F. Giesselmann, ChemPhysChem, 2013, 14, 2990–2995 CrossRef CAS PubMed.
- M. V. Gorkunov, M. A. Osipov, N. Kapernaum, D. Nonnenmacher and F. Giesselmann, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2011, 84, 051704 CrossRef CAS PubMed.
- S. T. Lagerwall, P. Rudquist and F. Giesselmann, Mol. Cryst. Liq. Cryst., 2009, 510, 148–157 CAS.
- J. P. F. Lagerwall and F. Giesselmann, ChemPhysChem, 2006, 7, 20–45 CrossRef CAS PubMed.
- A. de Vries, J. Chem. Phys., 1979, 71, 25–31 CrossRef CAS.
- A. de Vries, Mol. Cryst. Liq. Cryst., 1979, 49, 179–185 CrossRef CAS.
- A. de Vries, A. Ekachai and N. Spielberg, Mol. Cryst. Liq. Cryst., 1979, 49, 143–152 CrossRef CAS.
- V. Swaminathan, V. P. Panov, A. Panov, D. Rodriguez-Lojo, P. J. Stevenson, E. Gorecka and J. K. Vij, J. Mater. Chem. C, 2020, 8, 4859–4868 RSC.
- N. Yadav, V. Swaminathan, V. P. Panov, R. Dhar and J. K. Vij, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2019, 100, 052704 CrossRef CAS PubMed.
- Y. Yamada, W. Sano and A. Fukuda, Mol. Cryst. Liq. Cryst., 2019, 682, 1–7 CrossRef CAS.
- A. A. S. Green, M. R. Tuchband, R. Shao, Y. Shen, R. Visvanathan, A. E. Duncan, A. Lehmann, C. Tschierske, E. D. Carlson, E. Guzman, M. Kolber, D. M. Walba, C. S. Park, M. A. Glaser, J. E. Maclennan and N. A. Clark, Phys. Rev. Lett., 2019, 122, 107801 CrossRef CAS PubMed.
- D. Goswami, P. K. Mandal, O. Gutowski and A. Sarma, Liq. Cryst., 2019, 46, 2115–2126 CrossRef CAS.
- S. P. Sreenilayam, D. Rodriguez-Lojo, D. M. Agra-Kooijman, J. K. Vij, V. P. Panov, A. Panov, M. R. Fisch, S. Kumar and P. J. Stevenson, Phys. Rev. Mater., 2018, 2, 025603 CrossRef CAS.
- W. M. Zoghaib, C. Carboni, F. A. Kashoub, J. S. Al-Rushidi, B. Y. Al-Jabri, A. S. Al-Alawi and H. F. Al-Mendhry, Mol. Cryst. Liq. Cryst., 2018, 666, 54–64 CrossRef CAS.
- V. Swaminathan, V. P. Panov, A. Kocot and J. K. Vij, J. Chem. Phys., 2019, 150, 084901 CrossRef CAS PubMed.
- V. Swaminathan, V. P. Panov, S. P. Sreenilayam, Y. P. Panarin and J. K. Vij, Liq. Cryst., 2019, 46, 1246–1251 CrossRef CAS.
- S. P. Sreenilayam, D. Rodriguez-Lojo, V. P. Panov, V. Swaminathan, J. K. Vij, Y. P. Panarin, E. Gorecka, A. Panov and P. J. Stevenson, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2017, 96, 042701 CrossRef CAS PubMed.
- N. Yadav, V. P. Panov, V. Swaminathan, S. P. Sreenilayam, J. K. Vij, T. S. Perova, R. Dhar, A. Panov, D. Rodriguez-Lojo and P. J. Stevenson, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2017, 95, 062704 CrossRef PubMed.
- W. H. de Jeu, Physical Properties of Liquid Crystalline Materials, Gordon and Breach, New York, 1980 Search PubMed.
- S. Chandrasekhar, Liquid Crystals, Cambridge University Press, Cambridge, 2003 Search PubMed.
- P. G. de Gennes, The Physics of Liquid Crystals, Clarendon Press, Oxford, 1975 Search PubMed.
- Z. Ahmed, C. Müller, J. J. Johnston, K. Nguyen, C. P. J. Schubert, K. Abitaev, S. Marino, F. Giesselmann and R. P. Lemieux, Liq. Cryst., 2019, 46, 896–904 CrossRef CAS.
- C. Müller, C. P. J. Schubert, R. P. Lemieux and F. Giesselmann, ChemPhysChem, 2018, 19, 2703–2708 CrossRef PubMed.
- C. P. J. Schubert, C. Müller, A. Bogner, F. Giesselmann and R. P. Lemieux, Soft Matter, 2017, 13, 3307–3313 RSC.
- C. P. J. Schubert, C. Müller, F. Giesselmann and R. P. Lemieux, J. Mater. Chem. C, 2016, 4, 8483–8489 RSC.
- C. P. J. Schubert, C. Müller, M. D. Wand, F. Giesselmann and R. P. Lemieux, Chem. Commun., 2015, 51, 12601–12604 RSC.
- C. P. J. Schubert, A. Bogner, J. H. Porada, K. Ayub, T. Andrea, F. Giesselmann and R. P. Lemieux, J. Mater. Chem. C, 2014, 2, 4581–4589 RSC.
- Q. Song, D. Nonnenmacher, F. Giesselmann and R. P. Lemieux, J. Mater. Chem. C, 2013, 1, 343–350 RSC.
- J. C. Roberts, N. Kapernaum, F. Giesselmann and R. P. Lemieux, J. Am. Chem. Soc., 2008, 130, 13842–13843 CrossRef CAS PubMed.
- L. Li, C. D. Jones, J. Magolan and R. P. Lemieux, J. Mater. Chem., 2007, 17, 2313–2318 RSC.
- M. Thompson, C. Carkner, A. Bailey, N. J. Mosey, N. Kapernaum and R. P. Lemieux, Liq. Cryst., 2014, 41, 1246–1260 CrossRef CAS.
- M. Thompson, C. Carkner, N. J. Mosey, N. Kapernaum and R. P. Lemieux, Soft Matter, 2015, 11, 3860–3868 RSC.
- S. P. Sreenilayam, D. M. Agra-Kooijman, V. P. Panov, V. Swaminathan, J. K. Vij, Y. P. Panarin, A. Kocot, A. Panov, D. Rodriguez-Lojo, P. J. Stevenson, M. R. Fisch and S. Kumar, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2017, 95, 032701 CrossRef CAS PubMed.
- W. M. Zoghaib, A. K. George, C. Carboni, M. Surekha, A. V. N. Ashok Kumar, P. V. Chalapathy and D. M. Potukuchi, Soft Mater., 2018, 16, 166–185 CrossRef CAS.
- W. M. Zoghaib, C. Carboni, J. Al-Rawahi, F. Al-Rubaiei, H. Al-Bulushi, M. Al-Aufi, M. Al-Harrasi, S. Al-Kalbani and A. Al-Kiyumi, Mol. Cryst. Liq. Cryst., 2016, 632, 114–123 CAS.
- M. Kohout, A. Bubnov, J. Šturala, V. Novotná and J. Svoboda, Liq. Cryst., 2016, 43, 1472–1485 CrossRef CAS.
- W. M. Zoghaib, C. Carboni, H. Al-Hinai, S. Al-Abri, S. Al-Kasbi, E. Al-Nasseri, M. Al-Masroori, M. Al-Yahyaee and S. Al-Busaidi, Mol. Cryst. Liq. Cryst., 2015, 612, 183–190 CrossRef CAS.
- G.-Y. Yeap, T.-N. Chan, W.-S. Yam, K. Madrak, D. Pociecha and E. Gorecka, Liq. Cryst., 2012, 39, 1041–1047 CrossRef CAS.
- K. M. Mulligan, A. Bogner, Q. Song, C. P. J. Schubert, F. Giesselmann and R. P. Lemieux, J. Mater. Chem. C, 2014, 2, 8270–8276 RSC.
- H. K. Singh, S. K. Singh, R. Nandi, D. S. Shankar Rao, S. K. Prasad, R. K. Singh and B. Singh, RSC Adv., 2016, 6, 57799–57802 RSC.
- Z. Zhang, S. Kaur, B. Kundu, B. K. Sadashiva and H. F. Gleeson, J. Mater. Chem. C, 2017, 5, 1195–1205 RSC.
- M. Alaasar, M. Prehm, M.-G. Tamba, N. Sebastián, A. Eremin and C. Tschierske, ChemPhysChem, 2016, 17, 278–287 CrossRef CAS PubMed.
- S. H. Ryu, T. J. Shin, T. Gong, Y. Shen, E. Korblova, R. Shao, D. M. Walba, N. A. Clark and D. K. Yoon, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2014, 89, 032502 CrossRef PubMed.
- Y. Shen, L. Wang, R. Shao, T. Gong, C. Zhu, H. Yang, J. E. Maclennan, D. M. Walba and N. A. Clark, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2013, 88, 062504 CrossRef PubMed.
- D. M. Walba, H. Yang, P. Keller, C. Zhu, R. Shao, D. A. Coleman, C. D. Jones and N. A. Clark, Macromol. Rapid Commun., 2009, 30, 1894–1899 CrossRef CAS PubMed.
- N. Kapernaum, D. M. Walba, E. Korblova, C. Zhu, C. Jones, Y. Shen, N. A. Clark and F. Giesselmann, ChemPhysChem, 2009, 10, 890–892 CrossRef CAS PubMed.
- D. M. Walba, E. Korblova, L. Eshdat, M. C. Biewer, H. Yang, C. Jones, M. Nakata, M. Talarico, R. Shao and N. A. Clark, J. Soc. Inf. Disp., 2007, 15, 585–588 CrossRef CAS.
- J. P. F. Lagerwall, D. Coleman, E. Körblova, C. Jones, R. Shao, J. M. Otón, D. M. Walba, N. Clark and F. Giesselmann, Liq. Cryst., 2006, 33, 17–23 CrossRef CAS.
- J. A. McCubbin, X. Tong, Y. Zhao, V. Snieckus and R. P. Lemieux, Chem. Mater., 2005, 17, 2574–2581 CrossRef CAS.
- J. A. McCubbin, V. Snieckus and R. P. Lemieux, Liq. Cryst., 2005, 32, 1195–1203 CrossRef.
- J. A. McCubbin, X. Tong, R. Wang, Y. Zhao, V. Snieckus and R. P. Lemieux, J. Am. Chem. Soc., 2004, 126, 1161–1167 CrossRef CAS PubMed.
- K. Takatoh, K. Sunohara and M. Sakamoto, Mol. Cryst. Liq. Cryst., 1988, 164, 167–178 CrossRef.
- M. D. Harjung, C. P. J. Schubert, F. Knecht, J. H. Porada, R. P. Lemieux and F. Giesselmann, J. Mater. Chem. C, 2017, 5, 7452–7457 RSC.
- A. V. Ivanov, S. A. Lyakhov, M. Y. Yarkova, A. I. Galatina and A. V. Mazepa, Russ. J. Gen. Chem., 2002, 72, 1435–1438 CrossRef CAS.
- R. B. Lemieux, Acc. Chem. Res., 2001, 34, 845–853 CrossRef CAS PubMed.
- A. Schultz, S. Laschat, A. Saipa, F. Giesselmann, M. Nimtz, J. L. Schulte, A. Baro and B. Miehlich, Adv. Funct. Mater., 2004, 14, 163–168 CrossRef CAS.
- Y. Wang, Y. Liu, J. Luo, H. Qi, X. Li, M. Nin, M. Liu, D. Shi, W. Zhu and Y. Cao, Dalton Trans., 2011, 40, 5046–5051 RSC.
- S. V. Subrahmanyam, P. V. Chelapathi, S. Mahabaleshwara, M. Srinivasulu, A. K. George and D. M. Potukuchi, Phys. B, 2014, 450, 173–184 CrossRef CAS.
- M.-C. Yeh, Y.-L. Su, M.-C. Tzeng, C. W. Ong, T. Kajitani, H. Enozawa, M. Takata, Y. Koizumi, A. Saeki, S. Seki and T. Fukushima, Angew. Chem., 2013, 125, 1065–1068 (Angew. Chem. Int. Ed., 2013, 52, 1031–1034) CrossRef.
- J. R. Epperson, M. A. Bruce, J. D. Catt, J. A. Deskus, D. B. Hodges, G. N. Karageorge, D. J. Keavy, C. D. Mahle, R. J. Mattson, A. A. Ortiz, M. F. Parker, K. S. Takaki, B. T. Watson and J. P. Yevich, Bioorg. Med. Chem., 2004, 12, 4601–4611 CrossRef CAS PubMed.
- S. R. D. George, T. E. Elton and J. B. Harper, Org. Biomol. Chem., 2015, 13, 10745–10750 RSC.
- J. Magano, M. H. Chen, J. D. Clark and T. Nussbaumer, J. Org. Chem., 2006, 71, 7103–7105 CrossRef CAS PubMed.
- J. Chae, Arch. Pharm. Res., 2008, 31, 305–309 CrossRef CAS PubMed.
- A. Jankowiak, Z. Debska, J. Romański and P. Kaszyński, J. Sulfur Chem., 2012, 33, 1–7 CrossRef CAS.
- S. Choi, M. A. Larson, S. H. Hinrichs and P. Narayanasamy, Bioorg. Med. Chem. Lett., 2016, 26, 1997–1999 CrossRef CAS PubMed.
- M. Butschies, S. Sauer, E. Kessler, H.-U. Siehl, B. Claasen, P. Fischer, W. Frey and S. Laschat, ChemPhysChem, 2010, 11, 3752–3765 CrossRef CAS PubMed.
- J. Mars, B. Hou, H. Weiss, H. Li, O. Konovalov, S. Festersen, B. M. Murphy, U. Rütt, M. Bier and M. Mezger, Phys. Chem. Chem. Phys., 2017, 19, 26651–26661 RSC.
- Y. Nozaki, K. Yamaguchi, K. Tomida, N. Taniguchi, H. Hara, Y. Takikawa, K. Sadakane, K. Nakamura, T. Konishi and K. Fukao, J. Phys. Chem. B, 2016, 120, 5291–5300 CrossRef CAS PubMed.
- F. Nemoto, M. Kofu and O. Yamamuro, J. Phys. Chem. B, 2015, 119, 5028–5034 CrossRef CAS PubMed.
- K. Nishikawa, in Ionic Liquids Further UnCOILed, ed. N. V. Plechkova and K. R. Seddon, Wiley, Hoboken, 2014, pp. 59–85 Search PubMed.
- A. Abate, A. Petrozza, G. Cavallo, G. Lanzani, F. Matteucci, D. W. Bruce, N. Houbenov, P. Metrangolo and G. Resnati, J. Mater. Chem. A, 2013, 1, 6572–6578 RSC.
- T. Niemann, D. Zaitsau, A. Strate, A. Villinger and R. Ludwig, Sci. Rep., 2018, 8, 1–7 CrossRef CAS PubMed.
- H. Liu and H. Nohira, Liq. Cryst., 1998, 24, 719–726 CrossRef CAS.
- V. F. Petrov, V. A. Vinokurov and V. V. Belyaev, Mol. Cryst. Liq. Cryst., 2010, 518, 40–59 CrossRef CAS.
- I. Rupar, K. M. Mulligan, J. C. Roberts, D. Nonnenmacher, F. Giesselmann and R. P. Lemieux, J. Mater. Chem. C, 2013, 1, 3729–3735 RSC.
- J. W. Goodby, I. M. Saez, S. J. Cowling, V. Görtz, M. Draper, A. W. Hall, S. Sia, G. Cosquer, S.-E. Lee and E. P. Raynes, Angew. Chem., 2008, 120, 2794–2828 (Angew. Chem. Int. Ed., 2008, 47, 2754–2787) CrossRef.
- Q. Song, D. Nonnenmacher, F. Giesselmann and R. P. Lemieux, Chem. Commun., 2011, 47, 4781–4783 RSC.
- M. M. Neidhardt, M. Wolfrum, S. Beardsworth, T. Wöhrle, W. Frey, A. Baro, C. Stubenrauch, F. Giesselmann and S. Laschat, Chem.–Eur. J., 2016, 22, 16494–16504 CrossRef CAS PubMed.
- W. Cao, B. Senthilkumar, V. Causin, V. P. Swamy, Y. Wang and G. Saielli, Soft Matter, 2020, 16, 411–420 RSC.
- P. H. J. Kouwer and T. M. Swager, J. Am. Chem. Soc., 2007, 129, 14042–14052 CrossRef CAS PubMed.
- I. Dierking, Textures of Liquid Crystals, Wiley-VCH, Weinheim, 2003 Search PubMed.
- R. G. Horn, J. Phys., 1978, 39, 105–109 CrossRef CAS.
- M. D. Hanwell, D. E. Curtis, D. C. Lonie, T. Vandermeersch, E. Zurek and G. R. Hutchison, J. Cheminf., 2012, 4, 17 CAS.
- A. J. Leadbetter and P. G. Wrighton, J. Phys., Colloq., 1979, 40, 234–242 Search PubMed.
- T. Wöhrle, R. Gündemir, W. Frey, F. Knecht, A. Köhn and S. Laschat, Chem.–Eur. J., 2017, 23, 4149–4159 CrossRef PubMed.
- R. Bartolino, J. Doucet and G. Durand, Ann. Phys., 1978, 3, 389–395 CAS.
- E. N. Keller, E. Nachaliel, D. Davidov and C. Böffel, Phys. Rev. A: At., Mol., Opt. Phys., 1986, 34, 4363–4369 CrossRef CAS PubMed.
- W. G. Jang, M. A. Glaser, C. S. Park, K. H. Kim, Y. Lansac and N. A. Clark, Phys. Rev. E: Stat., Nonlinear, Soft Matter Phys., 2001, 64, 051712 CrossRef CAS PubMed.
- D. M. Walba and N. A. Clark, Proc. SPIE, 1987, 825, 81–87 CrossRef.
- D. M. Walba and N. A. Clark, Ferroelectrics, 1988, 84, 65–72 CrossRef.
- D. M. Walba, H. A. Razavi, A. Horiuchi, K. F. Eidman, B. Otterholm, R. C. Haltiwanger, N. A. Clark, R. Shao, D. S. Parmar, M. D. Wand and R. T. Vohra, Ferroelectrics, 1991, 113, 21–36 CrossRef CAS.
- M. D. Radcliffe, M. L. Brostrom, K. A. Epstein, A. G. Rappaport, B. N. Thomas, R. Shao and N. A. Clark, Liq. Cryst., 1999, 26, 789–794 CrossRef CAS.
- P. Davidson and L. Strzelecki, Liq. Cryst., 1988, 3, 1583–1595 CrossRef CAS.
- S. Aeffner, T. Reusch, B. Weinhausen and T. Salditt, Eur. Phys. J. E, 2009, 30, 205–214 CrossRef CAS PubMed.
- P. Davidson and A. M. Levelut, Liq. Cryst., 1992, 11, 469–517 CrossRef CAS.
- F. Xu, H. Wang, X. Du, W. Wang, D.-E. Wang, S. Chen, X. Han, N. Li, M.-S. Yuan and J. Wang, Dyes Pigm., 2016, 129, 121–128 CrossRef CAS.
- T.-H. Huang, X.-C. Li, Y.-H. Wang, Z.-H. Kang, R. Lu, E.-L. Miao, F. Wang, G.-W. Wang and H.-Z. Zhang, Opt. Mater., 2013, 35, 1373–1377 CrossRef CAS.
- A. Poe, A. Della Pelle, S. Byrnes and S. Thayumanavan, Chem.–Eur. J., 2015, 21, 7721–7725 CrossRef CAS PubMed.
- S. Sergeyev, W. Pisula and Y. H. Geerts, Chem. Soc. Rev., 2007, 36, 1902–1929 RSC.
- S. Diring, F. Camerel, B. Donnio, T. Dintzer, S. Toffanin, R. Capelli, M. Muccini and R. Ziessel, J. Am. Chem. Soc., 2009, 131, 18177–18185 CrossRef CAS PubMed.
- J. Luo, Z. Xie, J. W. Y. Lam, L. Cheng, H. Chen, C. Qiu, H. S. Kwok, X. Zhan, Y. Liu, D. Zhu and B. Z. Tang, Chem. Commun., 2001, 1740–1741 RSC.
- J. Mei, N. L. C. Leung, R. T. K. Kwok, J. W. Y. Lam and B. Z. Tang, Chem. Rev., 2015, 115, 11718–11940 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/d0ra04650g |
| This journal is © The Royal Society of Chemistry 2020 |