Main-group metal cyclophane complexes with high coordination numbers

Abstract

Density functional theory calculations using the PBE0-D3BJ hybrid functional have been employed to investigate the complexation of main-group metal-cations with [2.2.2]paracyclophane and deltaphane. Geometry optimization under symmetry constraints was performed to observe the mode of coordination that a metal-cation adopts when it resides inside the cyclophane cavity. Thermodynamic properties were investigated to note the trends of stability along a group of metals. To further investigate the bonding properties, Morokuma–Ziegler energy decomposition analysis, natural bond orbital analysis and Bader's analysis were employed. It was observed that most of the main-group metal complexes with cyclophanes prefer an η⁶η⁶η⁶ coordination mode where the metal-cation sits in the centre of the cyclophane cavity. There is an increased thermodynamic stability in [2.2.2]paracyclophane complexes compared to their deltaphane analogues while the reverse is true regarding the strength of coordination based on interaction energy.

---

Introduction

Cyclophanes consist of two or more aromatic rings connected through aliphatic bridges, to form a cyclic cavity.¹ The aromatic rings are characterized by a planar geometry but the strain imposed by the aliphatic components renders the geometry of the cyclophanes twisted.²

There is a growing interest in the donor–acceptor complexes of cyclophanes with metals due to their various applications such as the development of ion-selective electrodes,³ catalysis⁴ and chelation.⁵ Metal-chelating agents involving cyclophanes are potential candidates to be used in waste-water treatment since they are known for metal-ion scavenging.^3,6 Host molecules undergo conformational changes upon incorporation of the guest species in their macrocyclic cavity and these differences may be observed sometimes through fluorescence. This allows recognition of targetted guest metal-cations.⁷ Moreover, derivatives of metallacyclophane hosts have been reported as potential candidates for optical biomolecular recognition.⁸ Luminescent metal-complexes of cyclophanes have been reported to have their potential applications in thin-film nonoporous materials.⁹ All these applications are due to the potential of the π-rich cyclophane cavity to host electrophilic or cationic guests by making inclusion complexes, despite the fact that some smaller cyclophanes are also known to form exclusion complexes.¹⁰

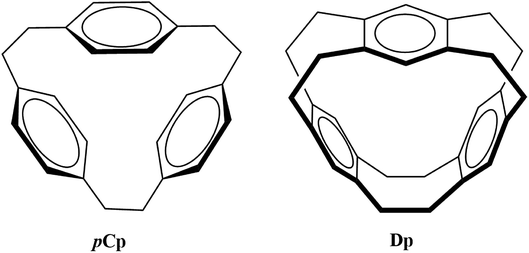
The selectivity and sensitivity of cyclophanes can be improved as evident from the efforts in the past such as derivatization and functionalization using different coordination groups,^4,11,12 controlling the size of the cavity to capture guest species¹³ and replacing the usual phenyl ring with heterocyclic aromatic rings such as imidazolium.¹⁴ Owing to the variety of their potential applications, it is important to explore the bonding properties of different possible metallacyclophanes. In the current study, the two cyclophanes [2.2.2]paracyclophane (pCp) and deltaphane (Dp) given in Fig. 1 were selected for this purpose.

Fig. 1 Cyclophane ligands of interest in the current study.

pCp was first synthesized by Pierre and co-workers where they called it a π-prismand due to its π-rich prism-shaped cavity¹⁵ and demonstrated its complexation with silver triflate. Extending the concept and introducing an increased rigidity compared to that of pCp, Kang et al. reported the synthesis of Dp.¹⁶ As the systematic name of the latter i.e. [2,2,2,2,2,2](1,2,4,5)cyclophane suggests, Dp has six ethano bridges compared to three in pCp. pCp and Dp consist of three phenyl rings each bridged through aliphatic chains. However, pCp is more flexible as each aromatic ring is attached to two ethyl bridges unlike its attachment to four in case of Dp.

Due to the presence of phenyl rings, both these molecules contain π-rich cavities that can coordinate to the metal-cations to form stable complexes. It was shown earlier that the silver triflate complex of pCp is much more stable compared to other such complexes with aromatic systems.¹⁵ Similarly, the crystal structure of silver triflate complex of Dp was also reported.¹⁶ During the same period (mid to late 1980s), Schmidbaur and co-workers reported the groundbreaking complexes of pCp with some of the main-group metals.^17–19 Despite these contributions in synthesis of metallacyclophanes of pCp and Dp, a lot of effort is still required to explore the bonding properties of these complexes to make use of these promising π-donating ligands on industrial scale.

In this regard, we previously reported the bonding properties of coinage metal complexes of pCp and Dp.²⁰ Earlier, the groups of Frenking and Castro carried out a computational study on the coordination mode and bonding properties of inclusion complexes of Sn²⁺ and Ag⁺ with pCp.²¹ They further extended the concept to computationally understand the role of formal charge of a cation in π–cation interactions by comparing the complexes of isoelectronic In⁺ and Cd²⁺ with pCp.²² In further instances, Castro et al. investigated helicenes²³ and Dp²⁴ as potential π-donors to form various π–cation interactions through relativistic DFT approach. A review of the use of relativistic computational tools to study the structural and bonding properties of these π–cation interactions has been published recently²⁵ which shows that different avenues in this field have been opened by the groups of Castro and Frenking over the last few years. The current work is aimed at investigating the coordination and bonding properties of the complexes of group 13 (Ga⁺, In⁺ and Tl⁺), 14 (Ge²⁺, Sn²⁺ and Pb²⁺) and 15 (As³⁺, Sb³⁺ and Bi³⁺) with the π-rich cavity of pCp and Dp using density functional theory (DFT).

Computational details

All calculations related to geometry optimization were performed with the Gaussian 09 suite of programs (Revision D.01).²⁶ The PBE0 hybrid functional²⁷ in conjunction with Grimme's empirical D3 correction with Becke–Johnston damping (D3BJ)²⁸ was used in combination with the def2-TZVP basis-set²⁹ of triple-ζ quality in all these calculations.

The optimized structures were further subject to the Morokuma–Ziegler Energy Decomposition Analysis (MZEDA)³⁰ that was carried out with the ADF2014 program.³¹ The TZ2P (Slater Type Orbital) basis set³² was employed along with the relativistic ZORA Hamiltonian.^33,34 MZEDA involves the decomposition of total energy (ΔE) of a molecule as:

ΔE = (ΔE₁ + ΔE₂) + ΔE^int (1)

In eqn (1), ΔE^int is the instantaneous interaction between the two molecular fragments and (ΔE₁ +ΔE₂) is the sum of their individual energies. The above equation implies that ΔE^int is the difference between the total energy of a molecule and its fragments. ΔE^int can be further subdivided as in eqn (2):

ΔE^int = ΔE^Pauli + ΔE^elstat + ΔE^orb (2)

Here, ΔE^elstat is the energy due to electrostatic interactions (mostly attractive in nature) between the molecular fragments. ΔE^Pauli is the repulsion term and it arises due to the electrons with same spin. ΔE^orb indicates the interactions involving charge transfer polarization effects.

Bader's analysis based on quantum theory of atoms in molecules (QTAIM)³⁵ given by Richard Bader was performed using Multiwfn software.³⁶ NBO analysis was carried out with NBO 6.0 program³⁷ as interfaced with Gaussian09.

Molecular graphics were rendered with GaussView 5.0.9.³⁸

Results and discussion

Structural features

Un-complexed cyclophanes. pCp possesses a rigid geometry and has an internal cavity with diameter 2.5 Å.³⁹ The phenyl rings are bridged at the para positions through ethyl chains. Dp is even more rigid as the phenyl rings are doubly connected to each other at the ortho and meta positions through ethano bridges. The D₃ symmetric geometries were optimized at PBE0-def2TZVP level of DFT and have been characterized as minima on the potential energy surface. Additionally, the two have D_3h symmetric transition structures optimized previously at the same level.²⁰ Calculated structural data of both of these ligands is consistent with their crystal structural information^16,39 except the fact that gas-phase calculated bond lengths are sometimes longer than those in a crystal structure (solid-phase) due to crystal packing forces that are absent in a gas-phase calculation.

In pCp, the C–C bond lengths in bridging ethyl units and that for bridgeheads are 1.54 Å and 1.51 Å compared to the experimental mean bond lengths of 1.43 Å and 1.52 Å respectively. The calculated C–C bond lengths in the phenyl rings are equivalent at 1.39 Å compared to the experimental bond lengths i.e. 1.37 Å. Dp presents a different case with a small variation of bond lengths in phenyl rings. Here, the calculated C–C bond length of the two sides of each phenyl ring attached to ethyl bridges is 1.40 Å compared to its experimental value of 1.390(2) Å while the other four bonds are calculated to be 1.39 Å compared to the experimental 1.387(3) Å bond length.

pCp–Mⁿ⁺ and Dp–Mⁿ⁺ complexes

Inclusion complexes of pCp (pCp–Mⁿ⁺) and Dp (Dp–Mⁿ⁺) with nine metal-cations of interest were optimized in their D₃ and C₃ symmetry and confirmed as either minima or transition structures through vibrational analysis. In the former case, the group 13 complexes and pCp–As³⁺ are C₃ symmetric minima while group 14 and the rest of group 15 pCp–Mⁿ⁺ complexes have D₃ symmetry in their ground state structures. In case of group 13 pCp–M⁺ complexes and pCp–As³⁺, the D₃ symmetry was possible for the transition structures only. The D₃ symmetric pCp–Mⁿ⁺ complexes show η⁶η⁶η⁶ metal–phenyl rings coordination. However, pCp–Ga⁺ and pCp–As³⁺ present a case with C₃ symmetry showing η⁶η⁶η⁶ coordination while its counterparts i.e. pCp–In⁺ and pCp–Tl⁺ exhibit η²η²η² coordination. As for the Dp complexes, all the nine structures were optimized as minima in C₃ as well as D₃ symmetry. Dp–Mⁿ⁺ complexes with C₃ show η¹η¹η¹ coordination of a metal ion staying on the top of Dp cavity in each complex. D₃ symmetric complexes, on the other hand, exhibit η⁶η⁶η⁶ coordination of the metal-cation present inside the Dp cavity. These results suggest that despite few exceptions, the pCp and Dp complexes of main-group metal-cations under discussion differ from the transition metal complexes where the metal-cation tends to come out of the cavity^20,21 preferring peripheral coordination unlike current complexes where central (η⁶η⁶η⁶) coordination is preferred.

Some key structural parameters of pCp–Mⁿ⁺ and Dp–Mⁿ⁺ of interest are given in Table 1. The experimental evidence is available only for pCp–Ga⁺, pCp–In⁺, pCp–Ge²⁺, pCp–Sn²⁺ and pCp–As³⁺ where it can be seen that computational results align well with the experimental ones. However, the distance of metal ion from the center of the pCp cavity in experimental and computational instances differs, which can be attributed to the proximity of corresponding counter anion(s) to the metal cation in experimentally reported complexes. This is in line with the computational findings reported earlier in case of pCp–Sn²⁺.¹⁸ The difference is highly pronounced in case of pCp–As³⁺ where both the calculated conformations (C₃ and D₃) exhibit η⁶η⁶η⁶ coordination mode, as mentioned earlier, with As³⁺ in C₃ symmetry displaced by 0.266 Å from the center of the host cavity. On the other hand, the experimentally reported pCp–As³⁺ shows η²η²η² coordination where AsCl₃ coordinates from the top of the cavity. In addition to pCp–Sn²⁺, the computational findings for pCp–In⁺ have also been reported earlier²² which, despite small numerical differences, show a great deal of similarity with the pCp–In⁺ calculated in our case.

Table 1 Key structural features of pCp–Mⁿ⁺ and Dp–Mⁿ⁺. Exp. shows experimentally reported pCp–Ga⁺, pCp–In⁺, pCp–Ge²⁺, pCp–Sn²⁺ and pCp–As³⁺. The computational results for pCp–In⁺ and pCp–Sn²⁺ reported earlier given in parentheses. Avg. C–M, Cent–M and C=C–M denote average metal–carbon distances, distance of metal cation from the center of the cavity, and average distance between C=C of phenyl rings (upper and lower in pCp–Mⁿ⁺ and sideways in Dp–Mⁿ⁺) from metal ion, respectively

		Avg. C–M	Cent–M	Internal Radius	C=C–M
pCp–Ga^+	C3	2.993	0.466	2.603	2.896
	D3	2.972	0.000	2.625	2.895
	Exp.^17	2.985	0.417	2.628	2.910
pCp–In^+	C3	3.130 (3.170)	1.192 (1.698)	2.644	3.052 (3.091)
	D3	3.029 (3.101)	0.000 (0.000)	2.689 (2.765)	2.963 (3.042)
pCp–Tl^+	C3	3.144	1.351	2.640	3.066
	D3	3.040	0.000	2.701	2.976
pCp–Ge^2+	D3	2.843	0.000	2.476	2.746
	Exp.^18	3.062	0.994	2.449	3.017
pCp–Sn^2+	D3	2.952 (2.975)	0.000 (0.000)	2.599 (2.595)	2.872 (2.877)
	Exp.^18	2.958	0.382	2.581	2.877
pCp–Pb^2+	D3	2.957	0.000	2.605	2.879
pCp–As^3+	C3	2.851	0.266	2.498	2.742
	D3	2.829	0.000	2.418	2.685
	Exp.^19	3.458	2.799	2.578	3.390
pCp–Sb^3+	D3	2.866	0.000	2.498	2.769
pCp–Bi^3+	D3	2.900	0.000	2.537	2.810
Dp–Ga^+	C3	2.637	2.354	2.365	2.744
	D3	2.836	0.000	2.467
Dp–In^+	C3	2.863	2.788	2.374	2.779
	D3	2.877	0.000	2.512
Dp–Tl^+	C3	2.863	2.788	2.374	2.779
	D3	2.877	0.000	2.512
Dp–Ge^2+	C3	2.910	2.866	2.377	2.792
	D3	2.891	0.000	2.528
Dp–Sn^2+	C3	2.526	2.219	2.345	2.732
	D3	2.823	0.000	2.449
Dp–Pb^2+	C3	2.590	2.286	2.360	2.750
	D3	2.843	0.000	2.472
Dp–As^3+	C3	2.206	2.060	2.230	2.658
	D3	2.724	0.000	2.343
Dp–Sb^3+	C3	2.378	2.190	2.311	2.670
	D3	2.784	0.000	2.400
Dp–Bi^3+	C3	2.460	2.253	2.331	2.715
	D3	2.803	0.000	2.421

---

The structural parameters of C₃ and D₃ symmetric conformers of pCp–Ga⁺ are identical except the displacement of Ga⁺ from the center of the cavity by 0.466 Å in C₃ conformer in contrast to its exactly central location in the D₃ counterpart. This is supported by identical ΔE^int of both the conformers in Table 4. However, in case of pCp–In⁺ and pCp–Tl⁺, the metal ion is located significantly further from the center in C₃ symmetry while it is exactly in the center in D₃ analogue. In both of these complexes, cavity size increases upon inclusion of metal cation for η⁶η⁶η⁶ coordination as evident from internal radius C=C–M (Table 1) while it shrinks when the metal cation approaches from the top of the pCp cavity for η²η²η² interaction. In line with these observations, C₃ conformers of pCp–In⁺ (ΔE^int = −51.51 kcal mol⁻) and pCp–Tl⁺ (ΔE^int = −46.78 kcal mol⁻) are energetically favourable by −4.73 kcal mol⁻ and −5.2 kcal mol⁻ than their D₃ (ΔE^int = −46.78 kcal mol⁻ for pCp–In⁺ and −41.37 kcal mol⁻ for pCp–Tl⁺) counterparts. Similarly, the D₃ symmetric pCp–As³⁺ is less favourable by 8.47 kcal mol⁻ than its C₃ analogue as evidenced by ΔE^int (Table 4).

Table 2 Gas-phase enthalpies (kcal mol⁻¹) of formation of metal complexes pCp–Mⁿ⁺ and Dp–Mⁿ⁺

	pCp	Dp		pCp	Dp		pCp	Dp
Ga^+	−110.1	−66.7	Ge^2+	−304.0	−284.1	As^3+	−683.7	−682.8
In^+	−95.7	−37.8	Sn^2+	−259.7	−227.8	Sb^3+	−566.7	−559.0
Tl^+	−92.5	−30.3	Pb^2+	−243.9	−207.5	Bi^3+	−524.8	−511.7

---

Table 3 Ionic radii (reported here from literature^40–42) of metal cations of interest in the current study. All the values are in Å

Ga^+	0.81 (ref. 40)	Ge^2+	0.73 (ref. 41)	As^3+	0.53 (ref. 42)
In^+	1.04 (ref. 40)	Sn^2+	0.93 (ref. 41)	Sb^3+	0.76 (ref. 42)
Tl^+	1.15 (ref. 40)	Pb^2+	0.98 (ref. 41)	Bi^3+	0.93 (ref. 42)

---

Table 4 Results of the MZEDA analysis for pCp–Mⁿ⁺ complexes in D₃ symmetry (results for the possible C₃ symmetric conformers in parentheses) at the PBE0/TZ2P level. Results for pCp–In⁺ and pCp–Sn²⁺ at BP86-D3/TZ2P+ level published earlier^21,22 given in square brackets for comparison. The percentage shows the contribution of an energy term in the total attraction energy which is the sum of ΔE^ele and ΔE^orb. All values in kcal mol⁻¹

	Ga		In		Tl
ΔE^prep	−3.32	(−3.09)	34.55 [10.00]	(−6.80 [4.50])	33.72	(−6.87)
ΔE^Pauli	66.40	(61.73)	89.40 [73.20]	(49.24 [39.10])	88.67	(44.47)
ΔE^ele	−59.47	(−56.98)	−70.74 [−49.10]	(−49.34 [−31.00])	−68.25	(−45.62)
	46.1%	(45.8%)	52.00% [40.80%]	(48.97% [36.90%])	52.50%	(50.10%)
ΔE^orb	−69.46	(−67.40)	−65.44 [−71.20]	(−51.42 [−53.10])	−61.79	(−45.43)
	53.9%	(54.2%)	48.00% [59.20%]	(51.03% [63.10%])	47.5%	(49.9%)
ΔE^int	−62.53	(−62.66)	−46.78 [−58.50]	(−51.51 [−62.00])	−41.37	(−46.57)

---

	Ge	Sn	Pb
ΔE^prep	−2.66	−3.77 [2.1]	−3.96
ΔE^Pauli	75.88	88.57 [86.0]	96.88
ΔE^ele	−88.12	−93.57–66.5	−96.56
	26.7%	32.3% [22.4%]	34.2%
ΔE^orb	−242.16	−205.31 [−229.9]	−193.83
	73.3%	68.7% [77.6%]	66.8%
ΔE^int	−254.41	−210.30 [−217.10]	−193.50

---

	As		Sb	Bi
ΔE^prep	−10.29	(−7.20)	−4.80	−4.91
ΔE^Pauli	78.98	(130.07)	103.33	106.87
ΔE^ele	−118.37	(−132.27)	−127.73	−129.40
	16.7%	(17.2%)	20.7%	22.4%
ΔE^orb	−589.09	(−634.78)	−488.72	−447.91
	83.3%	(82.8%)	79.3%	77.6%
ΔE^int	−628.51	(−636.98)	−512.72	−470.42

---

In case of Dp–Mⁿ⁺, all the C₃ conformers exhibit η¹η¹η¹ coordination with the metal cation located on the top of the cavity where their D₃ analogues are perfectly η⁶η⁶η⁶ coordinated. A comparison of ΔE^int (Table 5) shows that in most of the cases, D₃ conformers of Dp–Mⁿ⁺ are energetically favourable compared to their C₃ counterparts except Dp–In⁺, Dp–Tl⁺ and Dp–As³⁺ where η¹η¹η¹ coordination is more favourable.

Table 5 Results of the MZEDA analysis for Dp–Mⁿ⁺ complexes in D₃ symmetry (results for the possible C₃ symmetric conformers in parentheses) at the PBE0/TZ2P level. The percentage shows the contribution of an energy term in the total attraction energy which is the sum of ΔE^ele and ΔE^orb. All values in kcal mol⁻¹

	Ga		In		Tl
ΔE^prep	−3.76	(−6.20)	−4.81	(−11.67)	−4.78	(−14.01)
ΔE^Pauli	105.14	(72.51)	149.89	(57.30)	149.34	(51.92)
ΔE^ele	−80.18	(−48.98)	−102.52	(−39.82)	−99.57	(−36.36)
	48.4%	(39.3%)	55.4%	(40.3%)	55.9%	(41.3%)
ΔE^orb	−85.48	(−75.66)	−82.92	(−58.98)	−78.54	(−51.59)
	51.6%	(60.7%)	44.6%	(59.7%)	44.1%	(58.7%)
ΔE^int	−60.52	(−52.13)	−35.52	(−41.50)	−28.78	(−36.03)

---

	Ge		Sn		Pb
ΔE^prep	−2.10	(−11.11)	−5.80	(−9.57)	−8.16	(−8.98)
ΔE^Pauli	100.36	(126.50)	140.15	(130.24)	146.76	(120.82)
ΔE^ele	−102.48	(−90.84)	−119.12	(−94.34)	−121.11	(−90.38)
	27.6%	(24.2%)	33.4%	(28.6%)	35.17%	(30.0%)
ΔE^orb	−268.68	(−283.79)	−237.98	(−235.22)	−223.23	(−210.59)
	72.4%	(75.8%)	66.6%	(71.4%)	64.83%	(70.0%)
ΔE^int	−270.81	(−248.13)	−216.92	(−199.32)	−197.60	(−180.15)

---

	As		Sb		Bi
ΔE^prep	−4.48	(−41.84)	−5.16	(−28.21)	−6.11	(−23.29)
ΔE^Pauli	100.30	(201.07)	140.55	(186.07)	154.08	(168.78)
ΔE^ele	−132.95	(−142.09)	−147.66	(−140.83)	−152.61	(−134.77)
	17.4%	(16.2%)	21.6%	(19.7%)	23.5%	(21.8%)
ΔE^orb	−633.37	(−734.81)	−535.01	(−575.48)	−496.03	(−510.29)
	82.6%	(83.8%)	78.4%	(80.3%)	76.5%	(78.2%)
ΔE^int	−666.07	(−675.84)	−542.09	(−530.24)	−494.61	(−476.28)

---

Thermodynamic parameters

The trends of thermodynamic stability were established by calculating the enthalpies of reaction for the complexes under discussion given by the reaction as given in eqn (3).

Lg + Mⁿ⁺ → LgMⁿ⁺ (3)

Here, Lg (ligand) denotes pCp or Dp as the case may be and Mⁿ⁺ represents the respective metal cation with n = 1, 2, 3 for group 13, 14 and 15 respectively. LgMⁿ⁺ shows the resulting cyclophane–metal complex.

It can be seen in Table 2 that while moving from left to right in a period, thermodynamic feasibility of pCp or Dp complexes is enhanced as indicated by increasing exothermic enthalpy from group 13 through 14 to 15 in the same period. On the other hand, there is a trend of decreasing thermodynamic feasibility while moving from lighter to heavier elements in a group. These trends can be attributed to the size of ionic radii and formal charges on metal-cations. The smaller the ionic radius (as on the top of a group and the left side of a period), the greater the thermodynamic feasibility of the corresponding complex. Ionic radii of the metal cations under discussion are given in Table 3. Moreover, the role of formal charge has been found crucial in determining the strength of interaction and stability previously in such complexes.²² Upon moving from group 13 to 15 in a period, the formal charge in our case increases from +1 to +3 and so does the exothermic enthalpy of reaction. The trends of thermodynamic feasibility can be correlated with the results obtained from EDA and are discussed in the next section.

Bonding properties

The nature of bonding in the inclusion complexes under discussion was carried out using MZEDA technique, Bader's and NBO analyses. The former decomposes total interaction energy into various energy terms and hence it explains the strength of different interactions. NBO and Bader's analyses predict the type of bonding.

Morokuma–Ziegler energy decomposition analysis

The results for MZEDA of pCp–Mⁿ⁺ complexes are given in Table 4. It is to be noted that ΔE^orb indicates the strength of covalent interaction while ΔE^ele shows the strength of electrostatic attraction. The ratio of ΔE^orb to ΔE^ele explains the relative importance of covalent and ionic interactions i.e. the greater the ratio, the higher the percentage of ΔE^orb will be. The total steric repulsion present in a complex is depicted as ΔE^Pauli. The sum of the above-mentioned three terms accounts for the instantaneous interaction energy ΔE^int. However, it is advised in the literature to describe the overall interaction in terms of three separate quantities; ΔE^Pauli, ΔE^ele and ΔE^orb.⁴³

Table 4 shows that in group 13 pCp–M⁺ complexes, interaction energy is highest on the top of group and lowest in case of pCp–Tl⁺. This is in line with the trends of enthalpy of reaction (Table 2) that depicts a decrease in thermodynamic feasibility down the group. It can also be argued that increasing cationic radius (Table 3) down the group makes the π-donation from cyclophane cavity to metal less convenient. It can be seen that the C₃ symmetric pCp–Ga⁺ has an η⁶η⁶η⁶ coordination with the three aromatic rings of pCp which facilitates an overall stronger interaction. The metal-cation in pCp–In⁺ and pCp–Tl⁺ is located further from the centre of the cavity building an η²η²η² coordination in each case, thus contributing to a comparatively lower interaction energy.

A deeper insight into the EDA results (Table 4) shows that D₃ symmetric η⁶η⁶η⁶ transition structures of group 13 experiences a greater repulsion than their C₃ symmetric complexes as indicated by ΔE^Pauli. This is then compensated by a greater ΔE^prep in case of the former compared to the latter. However, both the C₃ and D₃ conformers of pCp–Ga⁺ are η⁶η⁶η⁶ with Ga⁺ locating a little further from the center in C₃ symmetric complex while ΔE^int is identical for both. Moreover, coordination in pCp–Ga⁺ is facilitated by a higher percentage of orbital interaction (ΔE^orb) than the electrostatic interaction (ΔE^ele). In case of pCp–In⁺ and pCp–Tl⁺, the percentage of ΔE^orb is less compared to that in pCp–Ga⁺ and ΔE^ele fraction substantially increases which ultimately accounts for a decrease in strength of coordination down the group as suggested by a decreasing ΔE^int. The strength of both the attraction terms may be expressed as the ratio of ΔE^orb to ΔE^ele which is 1.2 for pCp–Ga⁺ while it is 1.0 for each of pCp–In⁺ and pCp–Tl⁺. The trends of EDA results for group 14 and 15 are identical to those of group 13.

It is important to note that Table 4 includes EDA results reported earlier for pCp–In⁺²² and pCp–Sn²⁺ (ref. 21) calculated at BP86/TZ2P+ level. Although there is difference between the numerical values of those earlier and current studies which may be attributed to the different methodologies (BP86/TZ2P+ earlier versus PBE0/TZ2P current) used in both studies, the trends are identical. For instance, the η²η²η²-coordinated pCp–In⁺ is favoured compared to its η⁶η⁶η⁶ analogue based on their ΔE^int in both the cases. Similarly, ΔE^orb is a major attractive term in η⁶η⁶η⁶-coordinated pCp–Sn²⁺ in earlier and current studies as evidenced by the percentages of their ΔE^orb and ΔE^ele (Table 4).

The trends of EDA in the case of the Dp complexes under discussion (Table 5) are similar to that for pCp complexes. There occurs a decrease in overall interaction energy from top to bottom in a group. However, Dp–In⁺ and Dp–Tl⁺ exhibit relatively stronger electrostatic interactions compared to their pCp analogues. This is evident from the ratio of ΔE^orb to ΔE^ele that is 0.8 for both of these complexes while the same is 1.0 for both of their pCp counterparts. All Dp complexes demonstrate the presence of strong coordination as evident from the interaction energy. However, Table 5 shows that ΔE^int of D₃ symmetric η⁶η⁶η⁶-coordinated Dp–Mⁿ⁺ complexes is comparatively higher than that of their C₃ symmetric η¹η¹η¹-coordinated counterparts. This difference can be correlated to the greater contribution of covalent interactions (ΔE^orb) compared to that of electrostatic interactions (ΔE^ele) in overall ΔE^int in case of D₃ complexes. On the other hand, C₃ symmetric complexes are characterized by higher contribution of ΔE^ele than that of ΔE^orb in the overall ΔE^int which causes a comparatively weaker coordination in C₃ complexes than their D₃ analogues.

It can be deduced from Tables 4 and 5 that on moving from group 13 to 15 in a period, ΔE^int increases to a great extent from one metal ion to the next (Table 4). This trend can be attributed to the formal charge of a cation as witnessed previously.²² In our case, the formal charge is +1, +2 and +3 on group 13, 14, and 15 metal ions whereas ΔE^int in a period increases in the order of group 13 < group 14 < group 15. Moreover, the percentage of ΔE^orb successively increases and that of ΔE^ele subsequently decreases from left to right in a period. This whole discussion suggests that an increase in formal charge of a metal ion strengthens its coordination with the cyclophane host characterized by an increasing ΔE^orb which further accounts for an increase in overall ΔE^int along a period.

QTAIM (Bader's) analysis

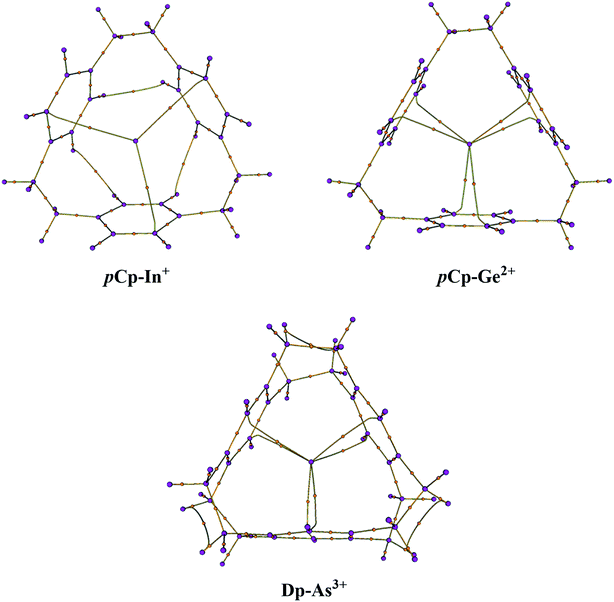
The molecular graphs were extracted from the Bader's analysis results that show the bond paths for all the electron pairs that would be expected for the host molecules i.e. CC and CH bonds. Additionally, there are bond critical paths (BCPs) connecting a metal-cation to the host (Fig. 2). For η²η²η² complexes i.e. pCp–In⁺ and pCp–Tl⁺, there is single BCP between the metal and one carbon atom of each of the aromatic rings toward the surface of the cavity that coordinates with the metal-cation as in the case of pCp–In⁺ in Fig. 2 where the coordination is on the top of the cavity. For all the pCp and Dp D₃ symmetric complexes there are two BCPs connecting the metal from the centre of the cyclophane cavity with two carbon atoms one each on top and bottom sides of the cavity as in the case of pCp–Ge²⁺ and Dp–As³⁺ (Fig. 2). The graphs for C₃ complexes are similar to each other and same is the case with D₃ complexes. This suggests a similar bonding in these complexes.

Fig. 2 Molecular graphs of C₃ symmetric pCp–In⁺ (representing all the complexes with C₃ symmetry) and D₃ symmetric pCp–Ge²⁺ and Dp–As³⁺ (representing all the D₃ symmetric complexes) calculated at PBE0-B3BJ/def2TZVP. BCPs are shown as orange-coloured dots.

The results for Bader's analysis of all the complexes are given in Table 6 which may be used to classify the types of interaction in these complexes. For that, Popelier has recently devised a mechanism based on electron density (ρ) and its various other functions.⁴⁴ According to the set of these rules, a small ρ accompanied by a negative Laplacian L (or ∇²ρ > 0 since L = −∇²ρ) usually denotes a depletion of electron density along a BCP and is the characteristic of either closed-shell or donor–acceptor interactions while a small ρ along with L approaching zero indicates a shared interaction. On the other hand, a large electron density and a positive L (or ∇²ρ < 0) shows that electron density is concentrated along a BCP and the interaction will usually be classified as covalent or intermediate. To further confirm the nature of interaction, some additional parameters such as local energy density and the ratio of kinetic energy (G) to ρ are also advised by Popelier.⁴⁴

Table 6 Electron density (ρ) and its Laplacian (L), ratio of kinetic energy to electron density (G/ρ) and local energy density (H) calculated through Bader's analysis of the complexes of interest. These QTAIM parameters may be used as criteria to characterize the type of interaction in the current metal–cyclophane complexes based on Table 8.1 from ref. 44 see discussion in the text. The group 13 pCp–M⁺ and pCp–As³⁺ are C₃ symmetric while all others have D₃ symmetric minima. All values in a.u.

	pCp				Dp
	ρ	L	G/ρ	H	ρ	L	G/ρ	H
Ga^+	0.017	0.036	0.572	−4.0 × 10^−4	0.024	0.046	0.574	−2.1 × 10^−3
In^+	0.016	0.032	0.545	−2.0 × 10^−4	0.023	0.057	0.634	−1.6 × 10^−3
Tl^+	0.018	0.042	0.618	3.0 × 10^−4	0.027	0.073	0.716	−1.0 × 10^−3
Ge^2+	0.025	0.046	0.532	−1.7 × 10^−3	0.029	0.050	0.540	−2.9 × 10^−3
Sn^2+	0.023	0.046	0.565	−1.4 × 10^−3	0.029	0.058	0.598	−2.8 × 10^−3
Pb^2+	0.024	0.059	0.651	−7.0 × 10^−4	0.030	0.074	0.681	−1.9 × 10^−3
As^3+	0.055	0.045	0.428	−1.2 × 10^−2	0.034	0.056	0.519	−3.6 × 10^−3
Sb^3+	0.030	0.050	0.524	−2.8 × 10^−3	0.034	0.057	0.542	−4.2 × 10^−3
Bi^3+	0.028	0.060	0.588	−1.9 × 10^−3	0.034	0.072	0.625	−3.4 × 10^−3

---

A careful classification based on Table 6 suggests that the complexes under discussion possess shared interactions i.e. electron sharing is enabled between the cyclophane hosts and the cationic guests. These findings are in line with the results of EDA where ΔE^orb in most of the cases is a major contributor in total attraction energy compared to ΔE^ele. All these complexes have a small ρ and its laplacian (L), a G/ρ less than 1 and a negative value of H except pCp–Tl⁺ that has a positive H suggesting that it has interaction between a shared and a donor–acceptor interaction. The EDA results of pCp–Tl⁺ support this assumption since ΔE^ele has a greater percentage than ΔE^orb.

Molecular orbital analysis

The electronic structure of the pCp and Dp complexes under consideration was further investigated based on natural population analyses (Table 7). Quantitative molecular orbital (MO) diagrams of pCp–Ga⁺ (C₃ η⁶η⁶η⁶), pCp–In⁺ (C₃ η²η²η²), pCp–Sb³⁺ (D₃ η⁶η⁶η⁶) and Dp–Sn²⁺ (D₃ η⁶η⁶η⁶) are given in Fig. S2 in ESI† to examine bonding and antibonding interactions between the cation and ligand fragments of a complex. The π bonding and antibonding orbitals of a cyclophane ring correspond to those of an aromatic system and have been labelled as π₁, π₂ and π₃. In all the η⁶η⁶η⁶ complexes where the metal cation is centrally located inside the ligand cavity, π₁ orbital of the cyclophane ligand coordinates with ns orbital of metal cation (with n = 4, 5, 6 for 4th, 5th and 6th row of elements respectively) giving rise to fully occupied bonding and antibonding π₁ns interactions as in Fig. S2(a)† thus not taking part in overall bonding (‘n’ has the value 4, 5, 6 for 4th, 5th, and 6th-row elements respectively). However, np orbital of the metal cation can be bonded to π₂ and π₃ orbitals of the aromatic system such that p_z interacts with π₂ based on orientation while π₃ has an equal chance of interaction with p_x and p_y orbitals of the metal cation. In pCp–In⁺ and pCp–Tl⁺ complexes, the bonding scheme is somewhat different as both have η²η²η² coordination mode. We propose in these two cases that only π₂ and π₃ of the cyclophane cavity coordinate with ns orbital and one of the np sub-orbitals, respectively leaving behind π₁ without any interaction as in Fig. S2(b).† This is also supported by the comparison of the amount of ligand to metal charge transfer (LMCT) in these two complexes (Table 7) with η⁶η⁶η⁶-coordinated pCp–Ga⁺ where LMCT is double the amount of that in its other two counterparts.

Table 7 NBO data for metal-cations in selected cyclophane–metal complexes. NC shows NBO charges of metal-cations, NEC denotes natural electronic configuration while LMCT is for ligand to metal charge transfer

		pCp	Dp
Ga^+	NC	0.61	0.57
	NEC	4s^1.984p^0.39	4s^1.984p^0.43
	LMCT	0.39	0.43
In^+	NC	0.80	0.63
	NEC	5s^1.995p^0.19	5s^1.975p^0.37
	LMCT	0.20	0.37
Tl^+	NC	0.78	0.64
	NEC	6s^1.986p^0.24	6s^1.986p^0.36
	LMCT	0.22	0.36
Ge^2+	NC	0.96	0.97
	NEC	4s^1.994p^1.03	4s^1.984p^1.02
	LMCT	1.04	1.03
Sn^2+	NC	1.16	1.37
	NEC	5s^1.995p^0.85	5s^1.985p^0.58
	LMCT	0.84	0.63
Pb^2+	NC	1.26	1.40
	NEC	6s^1.996p^0.73	6s^1.986p^0.55
	LMCT	0.74	0.60
As^3+	NC	0.97	0.92
	NEC	4s^1.994p^2.06	4s^1.984p^2.07
	LMCT	2.03	2.08
Sb^3+	NC	1.29	1.66
	NEC	5s^1.995p^1.71	5s^1.985p^1.28
	LMCT	1.71	1.34
Bi^3+	NC	1.37	1.78
	NEC	6s^1.996p^1.62	6s^1.986p^1.16
	LMCT	1.63	1.22

---

It can be seen in Fig. S2(a)† in MO diagram of pCp–Ga⁺ that π₁ of pCp and 4s Ga⁺ mix to give fully occupied bonding and antibonding MOs where bonding orbital has 15.87% contribution of Ga⁺ while the remaining 84.13% come from pCp. The contribution of Ga⁺ in antibonding MO, however, increases to 50.90%. Next, π₂ and 4p_z mix with a contribution of 6.86% and 83.79% from Ga⁺ in the resultant bonding and antibonding MOs, respectively. Moreover, π₃ mixes equally with 4p_x and 4p_y (as discussed above) to give bonding (1.93% from Ga⁺) and antibonding (60.62% from Ga⁺) interactions. In contrast, we can observe an overall lesser contribution of 5p orbital of In⁺ in Fig. S2(b) compared to 4p of Ga⁺ in (a).† This is in with a higher LMCT in case of η⁶η⁶η⁶-coordinated pCp–Ga⁺ compared to that in η²η²η²-coordinated pCp–In⁺ (Table 7). A comparison of (a), (b), (c) and (d) in Fig. S2† suggests that the greater the contribution of p orbital of corresponding metal cation, the larger the LMCT would be.

Comparison of pCp and Dp complexes

pCp and Dp have π-rich cavities and can efficiently host the main-group metal-cations making inclusion complexes with them. However, both possess different structures that cause these π-prismands to behave somewhat differently from each other. Although pCp has a rigid geometry as stated earlier, it is still somewhat flexible due to three ethano-bridges linking the three aromatic rings compared to Dp which has six aliphatic chains bridging the three phenyl rings. It readily undergoes conformational changes and tends to adjust its geometry accordingly to accommodate the metal-cations more conveniently. Hence, the pCp complexes are thermodynamically more feasible compared to the Dp complexes.

The comparison is not so simple when it comes to the strength of bonding interaction based on an overall interaction energy. Although, there is no definite trend followed while comparing pCp complexes with their Dp analogues, there are instances where Dp complexes have an increased coordination strength. For example, group 13 pCp–M⁺ complexes (Table 4) have higher interaction energy than corresponding Dp–M⁺ complexes (Table 5) which follows the same trend as in their thermodynamic feasibility (Table 2). However, the interaction energy in case of most of the group 14 and 15 pCp–Mⁿ⁺ is lower than their Dp analogues. This anomaly can be attributed to the collective effects of ΔE^orb and ΔE^prep. As a general trend, ΔE^orb is greater while ΔE^prep is smaller for Dp–Mⁿ⁺ complexes compared to pCp–Mⁿ⁺ complexes.

Conclusion

Quantum chemical calculations were employed to investigate the pCp and Dp complexes of main-group metals. Geometry optimization under symmetry constraints shows that an η⁶η⁶η⁶ mode of coordination is preferred in most of the cases. These complexes present excellent examples of host–guest interactions unlike the previously reported transition metal complexes of cyclophanes where a metal-cation takes peripheral position on top of the cavity. Most of the complexes are minima in D₃ symmetry whereas group 13 pCp–M⁺ and pCp–As³⁺ have only C₃ symmetric minima. MZEDA shows based on an overall interaction energy that all the complexes of interest bear strong metal–cyclophane coordination. The thermodynamic stability of pCp complexes is higher than their Dp analogues which is as expected due the more flexible and “adjustable” structure of pCp compared to Dp. However, the trends of coordination strength in both the cases are mixed. For example, group 13 pCp complexes have higher interaction energy than their Dp counterparts (a trend that is in line with their thermodynamic stability) while the majority of the rest of Dp complexes have an increased coordination strength compared to the corresponding pCp complexes (thus opposing the trend of thermodynamic stability). This can be correlated with the fact that generally ΔE^prep is lower and ΔE^orb is higher for Dp–Mⁿ⁺ complexes than corresponding pCp–Mⁿ⁺ except group 13 complexes which result in an overall higher ΔE^int for Dp–Mⁿ⁺ than pCp–Mⁿ⁺ of group 14 and 15. NBO analysis provided the basis for explaining in detail the electronic structure of complexes. Different parameters of Bader's analysis suggest the shared nature of M–C interactions in all these complexes except pCp–Tl⁺ that has a donor–acceptor type of interaction.

Conflicts of interest

The authors declare that they have no conflict of interest.

Acknowledgements

The authors thank Dr Matthias Lein, Professor Martyn P. Coles, Dr Ralf Tonner, Dr Khurshid Ayub and Dr Julia Schaht for useful discussions. One of the authors (Yasir Altaf) acknowledges Victoria Doctoral Scholarship to pursue this research. Additional computer time was provided by the Victoria University of Wellington High Performance Computer Facilities Raapoi and Heisenberg.

References

1. P. M. Keehn and S. M. Rosenfeld, Cyclophanes, Academic Press, New York, 1983, vol. I and II.
2. F. N. Diedrich, Cyclophanes, Royal Society of Chemistry, Cambridge, 1991.
3. J. Gross, G. Harder, A. Siepen, J. Harren, F. Vögtle, H. Stephan, K. Gloe, B. Ahlers, K. Cammann and K. Rissanen, Concave hydrocarbons, Chem.–Eur. J., 1996, 2, 1585–1595.
4. Y. Murakami, Functionalized cyclophanes as catalysts and enzyme models, Cyclophanes II, Berlin, Heidelberg, 1983; pp. 107–155.
5. J. C. Garrison, R. S. Simons, W. G. Kofron, C. A. Tessier and W. J. Youngs, Synthesis and structural characterization of a silver complex of a mixed-donor N-heterocyclic carbene linked cyclophane, ChemComm, 2001, 1780–1781.
6. T. Seppälä, E. Wegelius and K. Rissanen, [2.2.2]m, p, p-and [2.2.2]m, m, p-Cyclophane-Ag-triflate: new π-prismand complexes, New J. Chem., 1998, 22, 789–791.
7. B. Valeur and I. Leray, Design principles of fluorescent molecular sensors for cation recognition, Coord. Chem. Rev., 2000, 205, 3–40.
8. D. Ramaiah, P. P. Neelakandan, A. K. Nair and R. R. Avirah, Functional cyclophanes: Promising hosts for optical biomolecular recognition, Chem. Soc. Rev., 2010, 39, 4158–4168.
9. R. V. Slone, K. D. Benkstein, S. Bélanger, J. T. Hupp, I. A. Guzei and A. L. Rheingold, Luminescent transition-metal-containing cyclophanes (“molecular squares”): covalent self-assembly, host-guest studies and preliminary nanoporous materials applications, Coord. Chem. Rev., 1998, 171, 221–243.
10. H. Schmidbaur, W. Bublak, B. Huber, G. Reber and G. Müller, Coordination-Polymeric Paracyclophane Complexes of Copper and Silver, Angew. Chem., Int. Ed. Engl., 1986, 25, 1089–1090.
11. M. B. Inoue, P. Oram, G. Andreu-de Riquer, M. Inoue, P. Borbat, A. Raitsimring and Q. Fernando, Transition Metal Complexes of 12-and 13-Membered Functionalized Macrocycles, Dioxotetraazacycloalkanediacetates, Inorg. Chem., 1995, 34, 3528–3535.
12. M. B. Inoue, I. C. Muñoz, L. Machi, M. Inoue and Q. Fernando, Structural and spectroscopic studies of binuclear Cu²⁺ and Co²⁺ complexes with an amide-based naphthalenophane, Inorg. Chim. Acta, 2000, 311, 50–56.
13. P. Saarenketo, R. Suontamo, T. Jödicke and K. Rissanen, Ab Initio MO Study of Silver Ion Complexation in [2.2.2] Cyclophane π-Prismands, Organometallics, 2000, 19, 2346–2353.
14. E. Alcalde, C. Ayala, I. Dinarès, N. Mesquida and F. Sánchez-Ferrando, Novel [1_n]-meta-Heterophane Frameworks with a Bis-Betaine Nature, J. Org. Chem., 2001, 66, 2281–2290.
15. J. L. Pierre, P. Baret, P. Chautemps and M. Armand, [2.2.2]paracyclophane, a novel type of metal cation complexing agent (. pi.-prismand), J. Am. Chem. Soc., 1981, 103, 2986–2988.
16. H. C. Kang, A. Hanson, B. Eaton and V. Boekelheide, [26](1, 2, 4, 5) Cyclophane (deltaphane) and related compounds. Simultaneous. pi.-electron interaction among three benzene rings, J. Am. Chem. Soc., 1985, 107, 1979–1985.
17. H. Schmidbaur, R. Hager, B. Huber and G. Müller, Arene Complexes with Weak Interactions: A Macrocyclic Tris(arene) Complex of Gallium(I) with η¹⁸ Coordination, Angew. Chem., Int. Ed. Engl., 1987, 26, 338–340.
18. T. Probst, O. Steigelmann, J. Riede and H. Schmidbaur, Ge^II and Sn^II Complexes of [2.2.2]Paracyclophane with Threefold Internal η⁶ Coordination, Angew. Chem., Int. Ed. Engl., 1990, 29, 1397–1398.
19. T. Probst, O. Steigelmann, J. Riede and H. Schmidbaur, Arsen (III)-, Antimon (III)-und Bismuth (III)-halogenid-Komplexe des [2.2. 2] Paracyclophans: Vom lockeren van-der-Waals-Addukt zu stark gerichteten π-Komplexen mit zwei-und dreifacher externer η6-Koordination, Chem. Ber., 1991, 124, 1089–1093.
20. Z. X. Wong and M. Lein, Guest-Host Interaction of Coinage Metals in π-Rich Cavities, Aust. J. Chem., 2016, 69, 969–974.
21. C. O. Ulloa, M. Ponce-Vargas, R. de Mattos Piccoli, G. F. Caramori, G. Frenking and A. Munoz-Castro, [2.2.2]Paracyclophane, preference for η⁶ or η¹⁸ coordination mode including Ag(I) and Sn(II): a survey into the cation-π interaction nature through relativistic DFT calculations, RSC Adv., 2015, 5, 7803–7811.
22. A. O. Ortolan, G. F. Caramori, G. Frenking and A. Muñoz-Castro, Role of the cation formal charge in cation-π interaction. A survey involving the [2.2.2]paracyclophane host from relativistic DFT calculations, New J. Chem., 2015, 39, 9963–9968.
23. A. O. Ortolan, G. F. Caramori, R. L. T. Parreira and A. Muñoz-Castro, Helicenes as Molecular Tweezers in the Formation of Cation-π Complexes. Bonding and Circular Dichroism Properties from Relativistic DFT Calculations, ChemPhysChem, 2018, 19, 2321–2330.
24. A. O. Ortolan, N. D. Charistos, R. Guajardo-Maturana, C. O. Ulloa, G. F. Caramori, R. L. T. Parreira and A. Muñoz-Castro, On the cation-π capabilities of small all sp2-carbon host structures. Evaluation of [6.8]3cyclacene from relativistic DFT calculations, Int. J. Quantum Chem., 2019, 119, e25811.
25. D. Macleod-Carey, G. F. Caramori, R. Guajardo-Maturana, D. Paez-Hernandez, A. Muñoz-Castro and R. Arratia-Perez, Advances in bonding and properties of inorganic systems from relativistic calculations in Latin America, Int. J. Quantum Chem., 2019, 119, e25777.
26. M. J. Frisch, et al., Gaussian 09 Revision D.01, Gaussian Inc., Wallingford CT, 2009.
27. (a) J. P. Perdew, K. Burke and M. Ernzerhof, Generalized gradient approximation made simple, Phys. Rev. Lett., 1996, 77, 3865–3868; (b) J. P. Perdew, K. Burke and M. Ernzerhof, Errata: Generalized gradient approximation made simple, Phys. Rev. Lett., 1997, 78, 1396; (c) C. Adamo and V. Barone, Toward reliable density functional methods without adjustable parameters: The PBE0 model, J. Chem. Phys., 1999, 110, 6158–6169.
28. (a) S. Grimme, J. Antony, S. Ehrlich and H. Krieg, A consistent and accurate ab initio parameterization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu, J. Chem. Phys., 2010, 132, 154104; (b) S. Grimme, S. Ehrlich and L. Goerigk, Effect of the damping function in dispersion corrected density functional theory, J. Comput. Chem., 2011, 32, 1456–2465.
29. F. Weigend and R. Ahlrichs, Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence, Phys. Chem. Chem. Phys., 2005, 7, 3297.
30. (a) K. Kitaura and K. Morokuma, A new energy decomposition scheme for molecular interactions within the Hartree-Fock approximation, Int. J. Quantum Chem., 1976, 10, 325–340; (b) T. Ziegler and A. Rauk, A theoretical study of the ethylene-metal bond in complexes between copper(1+), silver(1+), gold(1+), platinum(0) or platinum(2+) and ethylene, based on the Hartree-Fock-Slater transition-state method, Inorg. Chem., 1979, 18, 1558–1565; (c) T. Ziegler and A. Rauk, Carbon monoxide, carbon monosulfide, molecular nitrogen, phosphorus trifluoride, and methyl isocyanide as sigma donors and pi acceptors. A theoretical study by the Hartree-Fock-Slater transition-state method, Inorg. Chem., 1979, 18, 1755–1759; (d) F. M. Bickelhaupt and E. J. Baerends, in Rev. Comput. Chem., ed. K. B. Lipkowitz and D. B. Boyd, Wiley, New York, 2000, vol. 15, pp. 1–86.
31. (a) G. te Velde, F. M. Bickelhaupt, E. J. Baerends, C. Fonseca Guerra, S. J. A. van Gisbergen, J. G. Snijders and T. Ziegler, Chemistry with ADF, J. Comput. Chem., 2001, 22, 931–967; (b) C. Fonseca Guerra, J. G. Snijders, G. te Velde and E. J. Baerends, Towards an order-N DFT method, Theor. Chem. Acc., 1998, 99, 391–403; (c) E. Baerends, et al., ADF2014. SCM, Theoretical Chemistry, Vrije Universiteit, Amsterdam, The Netherlands, http://www.scm.com/, 2014.
32. E. Van Lenthe and E. J. Baerends, Optimized Slater-type basis sets for the elements 1-118, J. Comput. Chem., 2003, 24, 1142–1156.
33. E. v. Lenthe, E. J. Baerends and J. G. Snijders, Relativistic regular two-component Hamiltonians, J. Chem. Phys., 1993, 99, 4597–4610.
34. E. van Lenthe, E. J. Baerends and J. G. Snijders, Relativistic total energy using regular approximations, J. Chem. Phys., 1994, 101, 9783–9792.
35. R. W. Bader, Atoms in Molecules: A Quantum Theory, Oxford University Press, Clarendon Press, 1990.
36. T. Lu and F. M. Chen, A multifunctional wavefunction analyzer, J. Comput. Chem., 2012, 33, 580–592.
37. A. E. Reed, L. A. Curtiss and F. Weinhold, Intermolecular interactions from a natural bond orbital, donor-acceptor viewpoint, Chem. Rev., 1988, 88, 899–926.
38. R. Dennington, T. Keith and J. Millam, GaussView Version 5.0.9, Semichem Inc., Shawnee Mission, KS, 2009.
39. C. Cohen-Addad, P. Baret, P. Chautemps and J.-L. Pierre, Structures cristallines du [2.2.2]paracyclophane (I) (C₂₄H₂₄) et de son complexe avec le perchlorate d’argent (II) (C₂₄H₂₄.AgClO₄), Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 1983, 39, 1346–1349.
40. L. Pauling, The Nature of the Chemical Bond and the Structure of Molecules and Crystals: An Introduction to Modern Structural Chemistry, Cornell university press Ithaca, NY, 1960, vol. 260.
41. L. H. Ahrens, The use of ionization potentials Part 1. Ionic radii of the elements, Geochim. Cosmochim. Acta, 1952, 2, 155–169.
42. R. D. Shannon, Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides, Acta Crystallogr., Sect. A: Cryst. Phys., Diffr., Theor. Gen. Crystallogr., 1976, 32, 751–767.
43. L. Zhao, M. von Hopffgarten, D. M. Andrada and G. Frenking, Energy decomposition analysis, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2018, 8, e1345.
44. P. L. A. Popelier, The Chemical Bond: Fundamental Aspects of Chemical Bonding, John Wiley & Sons, Ltd, 2014, ch. 8, pp. 271–308.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/d0ra05303a

---