Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Probing anisotropic mechanical behaviour in carbamazepine form III†
Benjamin P. A.
Gabriele
a,
Craig J.
Williams
b,
Matthias E.
Lauer
c,
Brian
Derby
 b and
Aurora J.
Cruz-Cabeza
b and
Aurora J.
Cruz-Cabeza
 *a
*a
aDepartment of Chemical Engineering and Analytical Science, University of Manchester, UK. E-mail: aurora.cruzcabeza@manchester.ac.uk
bDepartment of Materials, University of Manchester, UK
cRoche Innovation Center Basel, Basel, Switzerland
First published on 11th January 2021
Abstract
Nanoindentation measurements of the mechanical properties of the (020), (002) and (101) crystal faces in carbamazepine (CBZ) form III revealed that the (020) face had a greater elastic modulus and hardness than the (002) and (101) faces, which had similar modulus and hardness values. Atomic force microscopy (AFM) imaging of the indents showed that whilst no surface plastic displacement was observed around the residual indents on the (020) face, indents on both (002) and (101) faces nucleated cracks in multiple orientations and showed slip bands parallel to (020). The computed compliance matrix of CBZ form III was in good agreement with the experimental results and predicted anisotropic stiffness, with E(020) > E(002) > E(101). Modelling also revealed that the molecular flexibility of CBZ results in a less stiff material than that of an equivalent rigid compound. Indentation in one direction results in the opening of the CBZ-butterfly wings whilst in the other two results in their closing. Molecular dynamics simulations confirmed the primary (020) slip plane, consistent with the AFM images of the indents. It was also found that shear on the primary (020) slip plane, requires the breaking and forming of CBZ hydrogen bonded dimers. The slip plane, (020), was identified as having the lowest structural rugosity and hydrogen bonding density but not the lowest attachment energy.
1. Introduction
Beyond their required biological activities, active pharmaceutical ingredients (APIs) need to have appropriate further physicochemical properties that enable their development into effective drug products. For example, APIs need to have suitable aqueous solubilities and kinetics of dissolution,1 be able to grow from solution into adequately sized crystals with good filterability2 and have the required mechanical properties for tabletting. If the physical properties of an API are poor, attempts to improve them are often sought through the design of alternative solid forms such as cocrystals,3 hydrates,4–6 or polymorphs.7 This approach requires the development of new solid forms often involving different chemical compositions, which may require new and costly solid form screenings together with corresponding stability studies. Another route to modify properties is through the design, addition and fine tuning of various processes used for the production of the API. For example, sonocrystallisation,8 temperature cycling9 or appropriate use of milling10 are processes shown to enable modifications of particle size and shape distributions in crystalline powders which in turn can impact their filtration behaviour11,12 or mechanical properties.8Tablets contain a mixture of crystalline powders of the API and excipients. This mixture is referred to as the formulation and the exact proportion of the powder components is usually established on a trial-and-error basis working around the mechanical behaviour of the API powders so as to achieve a powder mixture that can be tabletted. Tablets formulated directly by compacting the API in isolation (without excipients) have the advantage of being smaller, not needing the formulation step and thus being cheaper to produce. Ultimately, the mechanical properties of the material API in powder form are primarily dictated by the mechanical properties of its single crystals. In that context, the study of the mechanical properties of single crystals directly using nanoindentation13,14 offers the opportunity to determine an API's mechanical behaviour at early stages of drug development (when the material is only available in small quantities) and to probe a number of other solid-state phenomena upon exposing the material to tabletting (i.e. stressed induce phase transformations,15 dehydration4,16…). The early acquisition of this information would provide extra tools to influence process design and control, allowing improvements to the drug's mechanical behaviour prior to its transition to formulation and tabletting.
Molecular crystals, particularly those with low symmetry forms, are well known to be highly anisotropic, with their properties varying along different crystal axes and faces. Growth rates,2 dissolution17 and mechanical properties18 are, amongst others, properties known to be face and direction dependent. Of particular interest, nanoindentation enables the evaluation of anisotropy of mechanical properties in molecular crystals, when combined with basic face indexing pre-experiments. It can probe specific crystallographic surfaces over penetration depths <1 μm and requires an exposed area of a few μm2. For this, however, large single crystals (hundreds of μm for at least two dimensions) are required so that repeated measurements on different {hkl} faces are possible. Most molecular crystals only expose one perhaps two {hkl} families of faces, hence it remains very challenging to perform nanoindentation experiments along different crystal directions. Despite these difficulties, the number of reports on anisotropic mechanical behaviour in molecular crystals measured with nanoindentation has increased considerably in recent years. Examples of these include works on saccharin,19L-alanine,20 sucrose,21 difluoroboron avobenzone,22 piroxicam15 or a series of amino-acids23 amongst others. Nanoindentation allows for the determination of a reduced modulus ER (related to the modulus of elasticity E(hkl) ≈ ER,(hkl)(1 − ν),24–26 with ν the Poisson's ratio) and hardness H of materials.
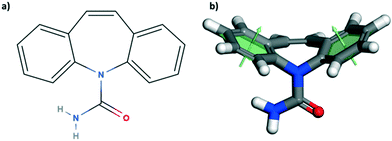
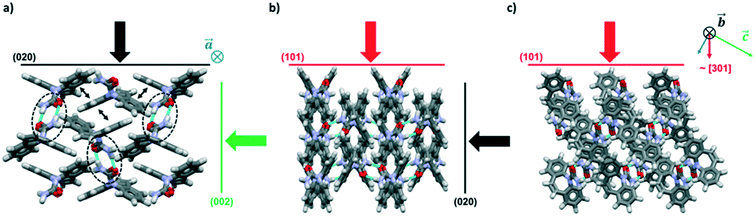
Here, we have studied the mechanical properties of carbamazepine (CBZ) form III (Fig. 1) along a number of crystallographic directions. CBZ, a drug used to treat epilepsy, has an interesting solid form landscape with five polymorphs27–31 and a wide range of cocrystals.32 We have chosen the stable form III28 for our nanoindentation studies since it readily crystallises as equant large crystals under various crystallisation conditions. Growth of appropriately sized crystals of CBZ-III from methanol allows for nanoindentation of three distinct faces: (020), (101) and (002). Nanoindentation combined with imaging analysis reveals distinct mechanical behaviour in the three directions. These experiments are supported by computer simulations to aid our understanding of plastic deformation of CBZ-III along the three directions studied. Overall, the study provides an enhanced understanding of the anisotropic mechanical behaviour in form III carbamazepine from a combined experimental and computational perspective.
2. Experimental section
Sample preparation
Slurries of carbamazepine in methanol (anhydrous, 99.8%, Sigma Aldrich) were heated to 40 °C. Solutions were then filtered and transferred into petri dishes which were left to cool (naturally from 40 °C to room temperature, ∼21 °C) and slowly evaporate under ambient conditions over 48 to 96 hours. The harvesting of the CBZ-III single crystals from their mother liquor was performed as follows. Due to the very high solubility of CBZ in methanol, a layer of hexane (HPLC, >95%, Fisher Chemical) was poured gently on top of the methanolic solution. The two solvents being immiscible, it allowed for the removal of the saturated solution from the crystals' surface while harvesting them with tweezers from solution, preventing secondary nucleation. Handling of crystals was always done with the aid of PEEK tweezers. Crystals retained for the nanoindentation experiments had dimensions of 0.5 × 0.5 × 0.25 mm, or larger. After face identifications, crystals were glued in the correct orientation using cyanoacrylate glue.Face indexing
Representative single crystals of CBZ-III grown from methanol were face indexed by means of a goniometer mounted on a single crystal X-ray diffractometer (XCalibur-2, Rigaku Oxford Diffraction) with a MoKα X-ray source (λ = 0.71073 Å). Photographs of the single crystals were taken before each X-ray diffraction measurement (whilst rotating the crystals) and were used for subsequent face indexing. The unit cell parameters and the space group were determined and verified to match those of CBZ-III as reported in the Cambridge Structural Database33 (CSD) refcode CBMZPN02.28 Thus, the Miller indices used throughout the manuscript refer specifically to the unit cell parameters defined in this refcode. Indexing of the faces was carried out using the software CrysAlisPro (v. 171.40.14d, Rigaku Oxford Diffraction).34Nanoindentation
Experiments were carried out using a TI 950 Triboindenter (Bruker Nanomechanical Test Instruments, Minneapolis, USA) equipped with a diamond Berkovich probe. Indents were carried out on selected single crystals whose crystal shape clearly matched those identified during face indexing. Indentations were conducted under load-control mode using a unique trapezoid load function which is presented in the ESI.† Crystals of carbamazepine were large enough to allow for them to be firmly glued on a glass slide prior to the indentation experiments. 10 crystals were indented per crystal face tested, 9 indents were carried out per crystal and the distance between individual indents was set to 20 μm. Reduced modulus and hardness were extracted from the indentation curves as stated in ISO 14577.35 Calibration of the tip was made using a PS-1 sheet sample (ER = 2.91 GPa, VPG Micro-measurements, Malvern, USA), which was indented in between each single crystal tested to verify for possible tip contamination and calibration errors. As previously described in detail,14 prior to indentation we investigated the optimal maximum displacement, load rate and holding times which were fixed to 5 second load, 2 second hold and 5 seconds unload segments. The maximum load PMax used was fixed to 3 mN as no further changes in the values of ER and H were observed for higher loads.14Imaging
Crossed polarised light was used to identify single crystals of good quality using an Axioplan2 Zeiss Microscope in transmission mode. Imaging of the indents was carried out using an atomic force microscope (AFM, Multimode8, Bruker), using PeakForce QNM in Air mode, with a TESP-V2 probe (k = 42 N m−1).Computation
All simulations were done with Materials Studio 2019 (ref. 36) using the COMPASS-II37 forcefield with its own atomic charges, known to be a reliable forcefield for the simulation of molecular crystals.38 Energy values of all simulations were converted to kJ mol−1 and reported per molecule of CBZ.3. Results
3.1. Experimental results
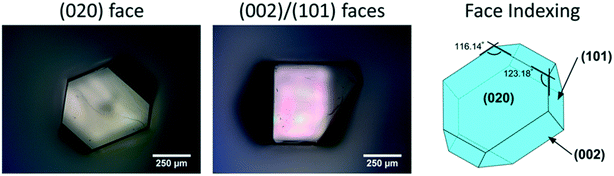 | ||
| Fig. 2 Micrographs of a single crystal of CBZ form III grown from methanol, with its associated face indexing. | ||
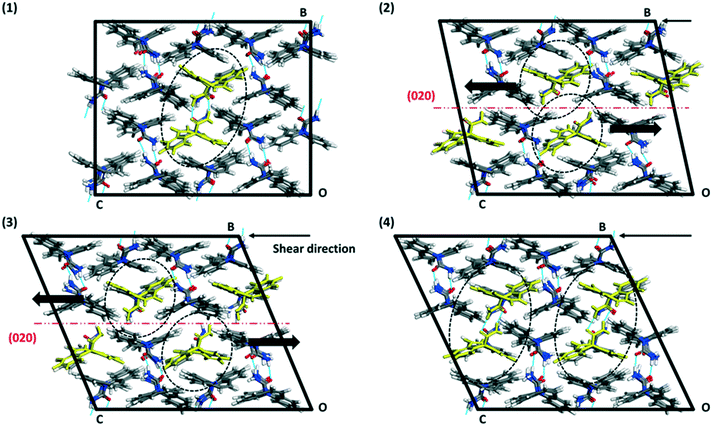
The crystal packing of CBZ-III along the three directions indented is presented in Fig. 3. Hydrogen bonded dimers (R22(8) amide⋯amide) and aromatic interactions are highlighted in Fig. 3a (black dashed circles and black arrows respectively). The lattice energy of CBZ-III ULATT was calculated to be −145.6 kJ mol−1 with contributions of around 20% of electrostatic interactions and 80% of van der Waals interactions (see ESI†). Dimers are oriented very closely along [010] (Fig. 3a) whilst van der Waals interactions are dominated mostly from the aromatic interactions between neighbouring aromatic rings of CBZ.
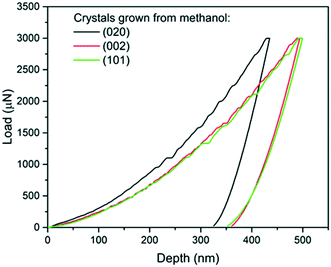 | ||
| Fig. 4 Load-depth curves from indentation carried out on a (020), a (101) and a (002) face of single crystals of carbamazepine form III crystals. | ||
The associated averages and standard deviations of ER and H obtained from these nanoindentation experiments are given in Table 1, whilst associated histograms of these datasets are presented in ESI.† The reduced modulus measured on the (020) faces is higher than those measured on the (002) and (101) by 26% and 29% respectively. Similarly, the hardness measured on the (020) face is higher than that of the two other faces, with an increase of approximately 20%. Differences between the (002) and (101) faces are less prominent and overall averages of both ER and H on these faces are extremely similar.
| Face | E R ± stdv (GPa) | H ± stdv (MPa) |
|---|---|---|
| (020) | 13.87 ± 0.26 | 470 ± 17 |
| (002) | 11.01 ± 0.22 | 396 ± 13 |
| (101) | 10.74 ± 0.28 | 394 ± 17 |
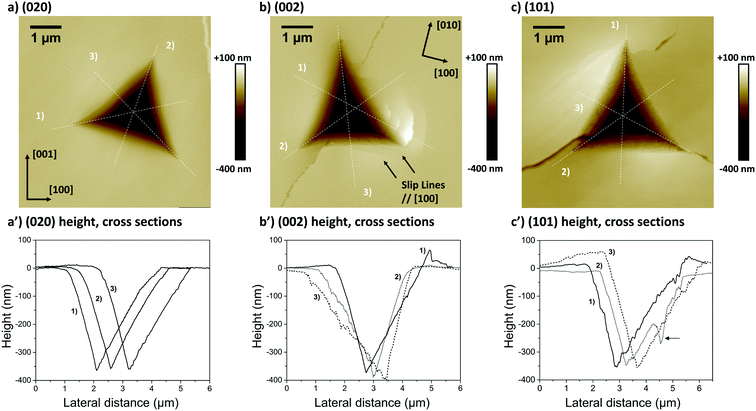
The second and third AFM images (Fig. 5b and c) show indents carried out on the softer (002) and (101) faces respectively. Plastic deformations of several nature are observed in both cases with evidence of slips, radial cracks, small pile-ups and sink-ins. On the (002) face, slip lines run along [100] and individual cracks propagate along a direction close to (but not quite) [010] with cracks having a zig-zag shape. On the (101) face, similar deformation features are observed, however it was not possible to unequivocally determine the direction of the slip bands and radial cracks. We note that cracks on the (101) face also propagated alternatively along two directions, as seen in Fig. 5c. The presence of small pile-ups and sink-ins along some indent edges is visible in the cross-sections shown in Fig. 5b′ and c′. Such dramatic differences in the plastic response of CBZ crystals across different crystallographic faces are consistent with the differences in the values of ER and H in Table 1.
We note that whilst indentation of the (002) and (101) faces resulted in cracks at the working PMax of 3000 μN (which may result in an artificial reduction of the derived stiffness or hardness),43,44 we did not observe a significant impact of these cracks on the resulting ER or H values calculated at different maximum loads (Fig. 6). If cracks were to have a significant effect on these derived values, an increase in load should result in more cracks and thus a significant lowering of the calculated ER and H values. As discussed previously,14 the maximum load PMax used in nanoindentation measurements needs to be above a minimum value below which the data becomes inconsistent due to calibration issues. In this case, the plateau for acquisition of consistent data is reached in all three faces at around 2000 μN. At loads above 2000 μN, the derived values of ER and H as a function of maximum load remain constant within the expected experimental variability for these measurements. For example at PMax of 3000 μN, the derived mean ER value for the (101) face on 90 independent indents on 9 different crystals is 10.74 GPa with a measured maximum and minimum values of 10.25 and 11.45 GPa respectively (ESI†). Similarly, the derived mean H value for the (101) face on 90 independent indents on 9 different crystals is 394 MPa with a measured maximum and minimum values of 359 and 428 MPa respectively. All measurements of ER and H on PMax values above 2000 μN (Fig. 6) lie within these ranges. This suggests that cracking, in this case, has little impact on the derived stiffness and hardness values. We also note that since the pile-ups and sink-ins observed in the (002) and (101) indents were small, no corrections were applied to the indentation area calculations.
3.2. Computational results
| Plane indented | E EXP (GPa) | E COMP-RT (GPa) | E COMP-0K (GPa) | E COMP-AVG (GPa) | Poisson's ratio ν |
|---|---|---|---|---|---|
| (020) | 13.16 ± 0.24 | 10.22 | 15.32 | 12.77 | 0.25 |
| (002) | 10.37 ± 0.20 | 7.42 | 12.35 | 9.89 | 0.26 |
| (101) | 9.59 ± 0.25 | 7.94 | 10.24 | 9.09 | 0.34 |
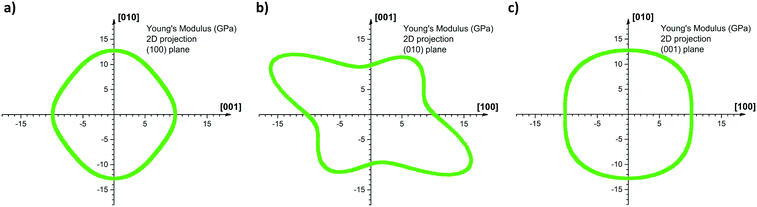
Fig. 7 shows the 2D projection of the “averaged-E” matrix on the (100), (010) and (001) planes, in which the distance of each data point from the centre of the graph corresponds to the absolute value of E computed for a given crystallographic direction. The highest computed value read is 19.0 GPa along [−302] and the lowest is 9.1 GPa along the [301] as shown in Fig. 7b. If we define here the anisotropy ratio (AR) as the ratio of the largest over the smallest value of a property, hence, for the Young's modulus, ARCOMP-AVG = 2.1. From Fig. 7a, we can extract EZ = E(002) = 9.9 GPa and EY = E(020) = 12.8 GPa, thus the calculations confirm that E(002) < E(020) as observed experimentally. Moreover, the computed value for the direction normal to (101) (i.e. direction of indentation, ≈[301] as explained in ESI†) can be read in Fig. 7b as E(101) = 9.1 GPa. Again, E(101) < E(020) whilst E(101) is slightly lower in value than E(002) as observed experimentally. We note that the computed values for E are close to the measured values and predict the correct anisotropy of mechanical behaviour in CBZ-III, which is encouraging given the simplicity of the model (a classic COMPASS-II forcefield).
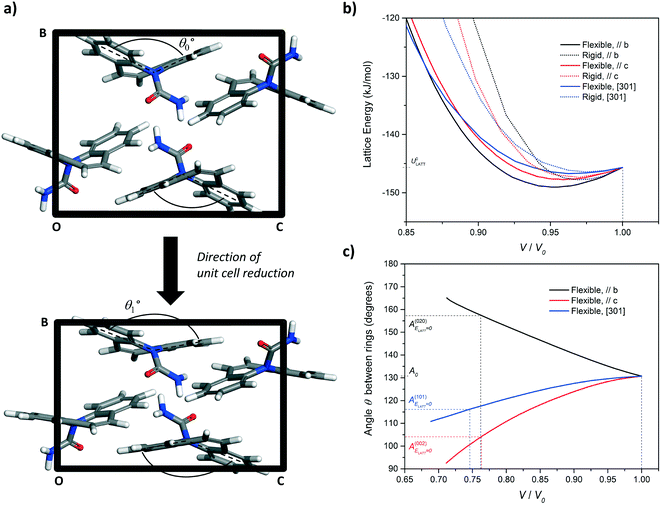 | ||
| Fig. 8 (a) Simulation of unit cell compression via a progressive reduction of the b-axis of CBZ-III. The evolution of the angle made by the two benzene rings of the CBZ molecule (Fig. 1) was monitored as a function of the unit-cell volume reduction. (b) Evolution of the lattice energy and (c) the butterfly wing angle as a function of unit cell compression along the [010], [001], and [301] directions for CBZ-III. Solid lines and dashed lines correspond to simulations treating the CBZ molecules as flexible and rigid respectively. | ||
In Fig. 8b, the lattice energy functions can be conveniently fitted to a second order polynomial function. The modulus of elasticity along a crystal direction is theoretically proportional to the second derivative of the corresponding function, when assessed at ULATT = ULATT,MIN. Full details of the equation linking E and ULATT, obtained for these derivatives, including numerical values and statistical measures of fit, are reported in ESI.† Here, we draw one simple conclusion: the second derivative is always lower for the potential energy functions of flexible molecules as compared to those for rigid molecules, which indicates that flexibility makes the material less stiff in CBZ-III.
The results from the shearing simulations are presented in Table 3. At least one slip event occurred over the 1500 ps simulation time along the (020) plane in four simulations, which include all three indented faces. A single simulation showed slip along the (002) plane. One of the simulations showed no slip events prior to structural collapse (loss of long-range order in the supercell). Slip events are abrupt, occurring over a short time <10 ps. It is notable that only two simulations revealed repeating slip events (simulations 3 and 6, Table 3), indicating (020) is the most likely slip plane. These simulations only show repetitive crystal slips when indentation is carried out on the (002) or (101) face. We further note that the intensity of the shear (distance made by the (020)-molecules per slip event) is not an integer of a unit cell axis (1/3 of c-axis ∼4.6 Å, 1/4 of [301], indicating metastable intermediate states for a full slip to occur – partial dislocations).
| Indented face | Indenting/shear direction | Simulation number | Simulation static plane | Slip plane | Intensity × [direction] of slip | Comments |
|---|---|---|---|---|---|---|
| (020) | [010] | 1 | (100) | (020) | 1 × [100] | One slip event, eventually collapse |
| 2 | (001) | (020) | K × [001], K = 1/3 | One slip event, eventually collapse | ||
| (002) | [001] | 3 | (010) | (020) | K × [001], K = 1/3 | Repetition slip events over entire simulation |
| 4 | (100) | (002) | [100] | One slip event, eventually collapse | ||
| (101) | [301] | 5 | (−103) | — | — | No slip, the system ends collapsing |
| 6 | (010) | (020) | K × [301], K = 1/4 | Repetitive slip events over entire simulation |
| Family | U ATT (kJ mol−1) | Rugosity (Å) |
|---|---|---|
| {020} | −83.4 | +0.09 |
| {200} | −122.9 | −2.20 |
| {002} | −71.2 | −3.10 |
| {011} | −64.2 | −0.33 |
| {101} | −87.2 | −2.93 |
{10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) } } |
−78.1 | +0.08 |
One may state that the (011) plane is the most likely slip plane based on attachment energy computations or that the (020) and the (10![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) ) planes are the most likely slip planes based on the surface rugosities (these are the smoothest surfaces). Table 4 shows thus that the rugosity predictor seems to be in agreement with the experimental observations and the MD-shear simulations for the (020) face, however it shows that the attachment energy model fails.
) planes are the most likely slip planes based on the surface rugosities (these are the smoothest surfaces). Table 4 shows thus that the rugosity predictor seems to be in agreement with the experimental observations and the MD-shear simulations for the (020) face, however it shows that the attachment energy model fails.
We note that these are simple predictive tools and should be used with care. In Fig. 10, PBCs in CBZ-III are shown in a 3 × 2 × 2 supercell (only interactions that are more stabilising than −20 kJ mol−1 shown). This reveals a 3D network in CBZ-III, made up of hydrogen bonds (blue tubes) corrugated with aromatic interactions of various strengths (purple and red tubes). Such 3D-network materials have been reported to i) exhibit brittle mechanical responses47 and ii) possess higher mechanical properties than their 2D counterparts.49 Concerning this latter point, values reported in Table 1 are significantly higher than those reported for smaller 2D-network molecules such as in benzoic acid derivatives, even if systems with higher mean molecular volumes like carbamazepine should have reduced mechanical properties.49
4. Discussion
We have studied CBZ-III experimentally with nanoindentation and have interpreted its mechanical behaviour using a number of simulation methods using the COMPASS-II forcefield. For the experimentation, we note that appropriate crystal growth is needed to obtain morphologies allowing nanoindentation of multiple crystal faces and thus assessment of the mechanical properties along independent directions for this system. Here, we found that growth from methanol provided a suitable chunky block morphology allowing indentation on three distinct faces.For the computations, since calculations of mechanical properties (i.e. stiffness matrix) require the computation of the second derivatives of the system forces, the model describing the force/potential needs to be very accurate. A very accurate method based on DFT-d, however, would require of significant computational time and would not allow for MD or shearing simulations involving supercell models. It is thus challenging to find an accurate method that is also affordable computationally for the prediction of mechanical properties in molecular crystals.45 Here the COMPASS-II model seemed to perform relatively well when both results – with and without unit cell parameter optimisation – of E were averaged. Further to this, the model allowed for the PBC calculations which revealed that CBZ-III is made of strong 3D continuous interactions (absolute values higher than 20 kJ mol−1), so the material was expected to be harder than other comparable 2D or 1D interacting systems, and the identification of soft/slip planes was thus more complex. We also used this model to gain insights as to the mechanisms of inter and intra molecular deformations upon compression. Whilst there are a number of studies looking at the impact of intermolecular interactions on the mechanical properties of molecular crystals, to the best of our knowledge this is the first study which has studied the contribution of molecular flexibility.
Combining the simulation results and experimental data, we can assess the extent of anisotropy of the mechanical properties in CBZ-III in various ways. We extend here the definition of the anisotropy ratio (AR) as the ratio of the largest value of a property over the smallest for values measured/computed in a number of directions (i.e. EMAX/EMIN or HMAX/HMIN). A large AR would indicate a highly anisotropic behaviour whilst an AR = 1 would indicate a perfectly isotropic material. CBZ-III crystals had experimental AR values for E and H equal to 1.3 and 1.2 respectively (using data from Table 1). These AR values are in good agreement with typical AR results measured experimentally and reported in the literature. In molecular crystals, these ARs range from 1 to ∼2 for Young's modulus and from 1 to ∼1.6 for hardness.50 From this, CBZ-III appears to be at the middle-lower end range of ARs for molecular crystals. For comparison, in crystalline metals ARs of the Young's modulus were reported to range from 1.12 (magnesium) to 3.42 (zinc).51
The anisotropy in mechanical behaviour was also manifest through the imaging of the indents on the three different crystal faces studied. This revealed that, upon indentation of the (020) face, neither significant surface displacement around the indent nor cracking was seen. However, indents on both the (002) and the (101) faces clearly displayed small material pile-up, slip traces and cracks. Our ER values calculated at different PMax and depth of penetrations (Fig. 6) show that for this system, the cracking seems to have no or little impact on the derived mechanical properties. This is also corroborated in the computations of E: the predicted stiffness for the studied faces match well the experimentally derived values. Interestingly, the faces that showed some degree of cracking, (002) and (101), were softer than the face that did not show cracking, (020). Normally, brittle faces are expected to be harder but we observed the opposite behaviour for CBZ-III.
It was difficult to accurately identify the slip planes from the images of the slip traces but, with the aid of simulations, these could unequivocally be assigned to the (020) planes. This result is surprising because i) the slip of (020) (Fig. 3a) requires of the breakage of hydrogen bonds and ii) (020) does not have the lowest energy of attachment (three other planes have a lower UATT). However, the associated rugosity of (020) predicts the highest probability of slip amongst the potential slip planes computed (Fig. 9). Hence, in this case it seems that interplanar rugosity is the most significant predictor of the primary slip plane.
To enable the slip of planes with negative rugosities, one can think of two alternatives. First, a large and local increase of the interplanar spacing dhkl (repulsive intermolecular interactions) would make the rugosity positive, enabling a slip or possibly a crack. A second option would require the modification of molecular flexibility (angles between intramolecular bonds, for example θ1 in Fig. 8) upon external stress in such a manner that a negative rugosity becomes positive. Both alternatives would probably require significantly more energy than that required to break the hydrogen bonds between two (020) planes. We note that the three options discussed (slicing and slip of planes with positive rugosity, local enlargement of dhkl or modification of angles between intramolecular bonds) all require energy. To correctly identify slip planes in molecular systems, the challenge would be hence to quantify which one is the most favourable energetically. The shearing experiments do indeed predict easiest slip on the (020) plane even upon indentation of the (020) plane. This again confirms this plane contains the easiest/lowest energy deformation mechanism. However, upon indentation of (020), the (020) slip must be partly hindered because (020) is orthogonal to the indentation (shearing) direction, leading to higher values of hardness in this direction than in the other two. We note that the shearing simulations only afforded one slip event on the (020) simulations whilst several events occurred on the other faces for simulations of the same length of time.
Coming back to the rugosity calculations, planes with similar rugosities can indeed behave differently. For example, the rugosity calculations show that whilst (020) and (−101) faces have similar rugosities (+0.09 and +0.08 Å repectively), only the (020) plane serves as a slip plane as shown by the MD simulations. Here, the hydrogen bonds that need breaking for this slip to occur are important. In previous works, the breaking of hydrogen bonds has been acknowledged as playing an inhibitting role in slippage but it has not been quantified.40 Here, we propose the calculation of what we define as the (hkl) plane hydrogen bond density (HB-density-(hkl)). For this, a 2D-unit cell on the plausible slip plane under consideration is calculated by identifying the shortest translations along two perpendicular directions to the plane direction. This is, crystallographically speaking, the 2D-unit cell on the plane of interest. The number of HBs that need breaking in this area for the plane to slip are then counted and the HB-density (number of bonds per unit of area) is calculated. Table 5 presents the rugosity data together with the HB-density for the most important planes. When seen together then, the combination of low rugosity (flat surface) and low HB-density-{hkl} (less energy required to break the HBs during slippage) does indeed unambigously reveal the (020) plane as the most likely slip plane in agreement with the MD simulations.
| Slip plane family | Rugosity (Å) | Plane 2D unit cell dimensions (Å × Å) | Number of HBs in 2D unit cell | HB-density-{hkl} (−/Å2) |
|---|---|---|---|---|
| {020} | +0.09 | 7.5 × 13.9 | 2 | 0.019 |
| {−101} | +0.08 | 15.4 × 11.5 | 4 | 0.023 |
| {011} | −0.33 | 7.5 × 17.8 | 4 | 0.029 |
| (200) | −2.20 | — | 0 | 0.000 |
| (101) | −2.93 | — | 0 | 0.000 |
| (002) | −3.10 | — | 0 | 0.000 |
The simulation of unit cell compression also demonstrated that molecular deformation of CBZ is markedly different, depending on the deformation orientation. Compression of the hardest face, (020), leads to an opening of the CBZ butterfly wings whilst compression of the softer faces results in their closing. In both cases, this flexibility results in a softer material than that of an equivalent rigid molecule (Fig. 6b and ESI†).
Finally, we note that whilst this is the first study of mechanical behaviour in CBZ-III single crystals upon nanoindentation, previous works by Rowe and Roberts reported the mechanical properties of CBZ-III powder compacts with different levels of porosities. However measured on powder compacts, they reported a value of 13.2 GPa for the Young's modulus of CBZ-III.52,53 Their reported value is close to those we obtained using nanoindentation on different faces.
5. Concluding remarks
The large size of crystals obtained by crystallising carbamazepine form III from methanol enabled nanoindentation testing along more than one crystal orientation. Nanoindentation showed that the (020) face of CBZ-III is stiffer and harder than the two other faces tested, (002) and (101). Imaging the indents using AFM revealed different plastic deformation processes between the (020) face and the (002) and (101) faces, with slip lines observed parallel to (020). On the surface around the indents performed on (020), there is no resolvable features or cracking that could be attributed to the nanoindentation experiment, whilst the latter two faces show indents around which nucleated slip bands and cracks as well as small pile up of the material.Overall, whilst CBZ has a strong 3D network of interactions, the system deforms plastically through slip on the (020) plane, which requires the breaking (and reforming) of hydrogen bonds. The material also shows some brittleness and surface cracking was observed on indentation. MD-Shearing simulations showed results consistent with our experimental observations of the slip plane, whilst energy of attachment failed at predicting such a slip plane. Rugosity data combined with a new parameter we defined as the HB-density of the slip plane of interest, were able to reveal the (020) as the most likely slip plane in agreement with the experiments and the MD simulations. Computed Young's moduli gave a good picture of the anisotropy of mechanical behaviour in CBZ-III. Of particular interest, MD-shearing simulation add to a set of computable parameters such as attachment energy, planes rugosity, whose efficiency at predicting weak planes has often been challenged.
Conflicts of interest
The authors declare no conflicts of interest.Acknowledgements
AJCC thanks the Royal Society for an Industry Fellowship and a Research Grant. This work was supported by the Henry Royce Institute for Advanced Materials, funded through EPSRC grants EP/S009493/1, EP/R00661X/1, EP/P025021/1 and EP/P025498/1. BG thanks Roche and the University of Manchester for funding, and the staff in the Department of Chemistry XRD Facility in the School of Natural Sciences at the University of Manchester for their assistance. The authors thank Dr Pirmin Hidber for helpful discussions and Andrew Forrest for his help with the nanoindenter. The authors also thank the referees for their helpful comments.References
- M. L. Cheney, D. R. Weyna, N. Shan, M. Hanna, L. Wojtas and M. J. Zaworotko, Coformer Selection in Pharmaceutical Cocrystal Development: A Case Study of a Meloxicam Aspirin Cocrystal That Exhibits Enhanced Solubility and Pharmacokinetics, J. Pharm. Sci., 2011, 100, 2172–2181 CrossRef CAS.
- Y. Liu, J. Black, K. F. Boon, A. J. Cruz-Cabeza, R. J. Davey, R. Dowling, N. George, A. Hutchinson and R. Montis, When Crystals Don't Grow – the Growth Dead Zone, Cryst. Growth Des., 2019, 19, 4579–4587 CrossRef CAS.
- C. C. Sun and H. Hou, Improving Mechanical Properties of Caffeine and Methyl Gallate Crystals by Cocrystallization, Cryst. Growth Des., 2008, 8, 1575–1579 CrossRef CAS.
- F. Liu, D. E. Hooks, N. Li, N. A. Mara and J. A. Swift, Mechanical Properties of Anhydrous and Hydrated Uric Acid Crystals, Chem. Mater., 2018, 30, 3798–3805 CrossRef CAS.
- M. S. R. N. Kiran, S. Varughese, U. Ramamurty and G. R. Desiraju, Effect of Dehydration on the Mechanical Properties of Sodium Saccharin Dihydrate Probed with Nanoindentation, CrystEngComm, 2012, 14, 2489–2493 RSC.
- S. Y. Chang and C. C. Sun, Superior Plasticity and Tabletability of Theophylline Monohydrate, Mol. Pharmaceutics, 2017, 14, 2047–2055 CrossRef CAS.
- P. Di Martino, A. M. Guyot-Hermann, P. Conflant, M. Drache and J. C. Guyot, A New Pure Paracetamol for Direct Compression: The Orthorhombic Form, Int. J. Pharm., 1996, 128, 1–8 CrossRef.
- D. K. Bučar, J. A. Elliott, M. D. Eddleston, J. K. Cockcroft and W. Jones, Sonocrystallization Yields Monoclinic Paracetamol with Significantly Improved Compaction Behavior, Angew. Chem., Int. Ed., 2015, 54, 249–253 CrossRef.
- H. Eisenschmidt, N. Bajcinca and K. Sundmacher, Optimal Control of Crystal Shapes in Batch Crystallization Experiments by Growth-Dissolution Cycles, Cryst. Growth Des., 2016, 16, 3297–3306 CrossRef CAS.
- F. Salvatori and M. Mazzotti, Manipulation of Particle Morphology by Crystallization, Milling, and Heating Cycles – A Mathematical Modeling Approach, Ind. Eng. Chem. Res., 2017, 56, 9188–9201 CrossRef CAS.
- R. Wakeman, The Influence of Particle Properties on Filtration, Sep. Purif. Technol., 2007, 58, 234–241 CrossRef CAS.
- G. Perini, F. Salvatori, D. R. Ochsenbein, M. Mazzotti and T. Vetter, Filterability Prediction of Needle-like Crystals Based on Particle Size and Shape Distribution Data, Sep. Purif. Technol., 2019, 211, 768–781 CrossRef CAS.
- U. Ramamurty and J. I. Jang, Nanoindentation for Probing the Mechanical Behavior of Molecular Crystals-a Review of the Technique and How to Use It, CrystEngComm, 2014, 16, 12–23 RSC.
- B. P. A. Gabriele, C. J. Williams, M. E. Lauer, B. Derby and A. J. Cruz-Cabeza, Nanoindentation of Molecular Crystals: Lessons Learnt from Aspirin, Cryst. Growth Des., 2020, 20(9), 5956–5966 CrossRef CAS.
- P. Manimunda, E. Hintsala, S. Asif and M. K. Mishra, Mechanical Anisotropy and Pressure Induced Structural Changes in Piroxicam Crystals Probed by In Situ Indentation and Raman Spectroscopy, JOM, 2017, 69, 57–63 CrossRef.
- S. Seethalekshmi, M. S. R. N. Kiran, U. Ramamurty and S. Varughese, Phase Transitions and Anisotropic Mechanical Response in a Water-Rich Trisaccharide Crystal, Cryst. Growth Des., 2020, 20, 442–448 CrossRef CAS.
- A. Danesh, S. D. Connell, M. C. Davies, C. J. Roberts, S. J. B. Tendler, P. M. Williams and M. J. Wilkins, An in Situ Dissolution Study of Aspirin Crystal Planes (100) and (001) by Atomic Force Microscopy, Pharm. Res., 2001, 18, 299–303 CrossRef CAS.
- D. Olusanmi, K. J. Roberts, M. Ghadiri and Y. Ding, The Breakage Behaviour of Aspirin under Quasi-Static Indentation and Single Particle Impact Loading: Effect of Crystallographic Anisotropy, Int. J. Pharm., 2011, 411, 49–63 CrossRef CAS.
- M. S. R. N. Kiran, S. Varughese, C. M. Reddy, U. Ramamurty and G. R. Desiraju, Mechanical Anisotropy in Crystalline Saccharin: Nanoindentation Studies, Cryst. Growth Des., 2010, 10, 4650–4655 CrossRef CAS.
- R. M. Mohamed, M. K. Mishra, L. M. Al-Harbi, M. S. Al-Ghamdi and U. Ramamurty, Anisotropy in the Mechanical Properties of Organic Crystals: Temperature Dependence, RSC Adv., 2015, 5, 64156–64162 RSC.
- K. J. Ramos and D. F. Bahr, Mechanical Behavior Assessment of Sucrose Using Nanoindentation, J. Mater. Res., 2007, 22, 2037–2045 CrossRef CAS.
- G. R. Krishna, M. S. R. N. Kiran, C. L. Fraser, U. Ramamurty and C. M. Reddy, The Relationship of Solid-State Plasticity to Mechanochromic Luminescence in Difluoroboron Avobenzone Polymorphs, Adv. Funct. Mater., 2013, 23, 1422–1430 CrossRef CAS.
- I. Lubomirsky, I. Azuri, E. Meirzadeh, D. Ehre, S. R. Cohen, A. M. Rappe, M. Lahav and L. Kronik, Unusually Large Young's Moduli of Amino Acid Molecular Crystals, Angew. Chem., Int. Ed., 2015, 54, 13566–13570 CrossRef.
- S. P. Timoshenko and J. Goodier, Theory of Elasticity, McGraw-Hill, New York, 2nd edn, 1951 Search PubMed.
- N. A. Stilwell and D. Tabor, Elastic Recovery of Conical Indentations, Proc. Phys. Soc., London, 1961, 78, 169–179 CrossRef.
- A. C. Fischer-Cripps, Contact Mechanics, in Nanoindentation, 2011, pp. 1–19 Search PubMed.
- M. M. J. Lowes, M. R. Caira, A. P. Lotter and J. G. D. Van Watt, Physicochemical Properties and X-ray Structural Studies of the Trigonal Polymorph of Carbamazepine, J. Pharm. Sci., 1987, 76, 744–752 CrossRef CAS.
- J. N. Lisgarten, R. A. Palmer and J. W. Saldanha, Crystal and Molecular Structure of 5-Carbamyl-5H-Dibenzo[b,f] Azepine, J. Crystallogr. Spectrosc. Res., 1989, 19, 641–649 CrossRef CAS.
- M. Lang, J. W. Kampf and A. J. Matzger, Form IV of Carbamazepine, J. Pharm. Sci., 2002, 91, 1186–1190 CrossRef CAS.
- A. L. Grzesiak, M. Lang, K. Kim and A. J. Matzger, Comparison of the Four Anhydrous Polymorphs of Carbamazepine and the Crystal Structure of Form I, J. Pharm. Sci., 2003, 92, 2260–2271 CrossRef CAS.
- J. B. Arlin, L. S. Price, S. L. Price and A. J. Florence, A Strategy for Producing Predicted Polymorphs: Catemeric Carbamazepine Form V, Chem. Commun., 2011, 47, 7074–7076 RSC.
- T. Gelbrich and M. B. Hursthouse, Systematic Investigation of the Relationships between 25 Crystal Structures Containing the Carbamazepine Molecule or a Close Analogue: A Case Study of the XPac Method, CrystEngComm, 2006, 8, 448–460 RSC.
- C. R. Groom, I. J. Bruno, M. P. Lightfoot and S. C. Ward, The Cambridge Structural Database, Acta Crystallogr., Sect. B: Struct. Sci., Cryst. Eng. Mater., 2016, 72, 171–179 CrossRef CAS.
- P. R. O. CrysAlis, Version 171.40.14d, Oxford Diffraction/Agilent Technologies UK Ltd, England, Yarnton, 2018 Search PubMed.
- ISO 14577: Instrumented Indentation Test for Hardness and Materials Parameters, in Metallic Materials, Int. Organ. Stand. Geneva, Switz, 2015 Search PubMed.
- Dassault Systèmes BIOVIA, Materials Studio, Release 2019, Dassault Systèmes, San Diego, 2019 Search PubMed.
- H. Sun, Z. Jin, C. Yang, R. L. C. Akkermans, S. H. Robertson, N. A. Spenley, S. Miller and S. M. Todd, COMPASS II: Extended Coverage for Polymer and Drug-like Molecule Databases, J. Mol. Model., 2016, 22, 1–10 CrossRef.
- R. L. M. Robinson, D. Geatches, C. Morris, R. MacKenzie, A. G. P. Maloney, K. J. Roberts, A. Moldovan, E. Chow, K. Pencheva and D. R. M. Vatvani, Evaluation of Force-Field Calculations of Lattice Energies on a Large Public Dataset, Assessment of Pharmaceutical Relevance, and Comparison to Density Functional Theory, J. Chem. Inf. Model., 2019, 59, 4778–4792 CrossRef.
- A. Marmier, Z. A. D. Lethbridge, R. I. Walton, C. W. Smith, S. C. Parker and K. E. Evans, ElAM: A Computer Program for the Analysis and Representation of Anisotropic Elastic Properties, Comput. Phys. Commun., 2010, 181, 2102–2115 CrossRef CAS.
- M. J. Bryant, A. G. P. Maloney and R. A. Sykes, Predicting Mechanical Properties of Crystalline Materials through Topological Analysis, CrystEngComm, 2018, 20, 2698–2704 RSC.
- S. Varughese, M. S. R. N. Kiran, K. A. Solanko, A. D. Bond, U. Ramamurty and G. R. Desiraju, Interaction Anisotropy and Shear Instability of Aspirin Polymorphs Established by Nanoindentation, Chem. Sci., 2011, 2, 2236–2242 RSC.
- M. K. Mishra, U. Ramamurty and G. R. Desiraju, Solid Solution Hardening of Molecular Crystals: Tautomeric Polymorphs of Omeprazole, J. Am. Chem. Soc., 2015, 137, 1794–1797 CrossRef CAS.
- M. L. Oyen and R. F. Cook, A Practical Guide for Analysis of Nanoindentation Data, J. Mech. Behav. Biomed. Mater., 2009, 2, 396–407 CrossRef.
- A. C. Burch, J. D. Yeager and D. F. Bahr, Indentation Fracture Behavior of Energetic and Inert Molecular Crystals, J. Mater. Res., 2019, 34, 3954–3963 CrossRef CAS.
- C. Wang and C. C. Sun, Computational Techniques for Predicting Mechanical Properties of Organic Crystals: A Systematic Evaluation, Mol. Pharmaceutics, 2019, 16, 1732–1741 CrossRef CAS.
- C. C. Sun and Y.-H. Kiang, On the Identification of Slip Planes in Organic Crystals Based on Attachment Energy Calculation, J. Pharm. Sci., 2008, 97, 3456–3461 CrossRef CAS.
- M. J. Turner, S. P. Thomas, M. W. Shi, D. Jayatilaka and M. A. Spackman, Energy Frameworks: Insights into Interaction Anisotropy and the Mechanical Properties of Molecular Crystals, Chem. Commun., 2015, 51, 3735–3738 RSC.
- C. Wang and C. C. Sun, Identifying Slip Planes in Organic Polymorphs by Combined Energy Framework Calculations and Topology Analysis, Cryst. Growth Des., 2018, 18, 1909–1916 CrossRef CAS.
- B. Gabriele, C. Williams, M. Lauer, B. Derby and A. Cruz-Cabeza, Isomechanical Groups in Molecular Crystals and Role of Aromatic Interactions, Cryst. Growth Des., 2020, 20(11), 7516–7525 CrossRef CAS.
- C. Wang and C. C. Sun, The Landscape of Mechanical Properties of Molecular Crystals, CrystEngComm, 2020, 20, 1149–1153 RSC.
- B. C. A. Hutchinson, 16: Anisotropy in Metals, Mater. Sci. Technol., 2015, 31, 1393–1401 CrossRef CAS.
- R. J. Roberts and R. C. Rowe, Influence of Polymorphism on the Young's Modulus and Yield Stress of Carbmazepine, Sulfathiazole and Sulfanilamide, Int. J. Pharm., 1996, 129, 79–94 CrossRef CAS.
- R. J. Roberts, R. S. Payne and R. C. Rowe, Mechanical Property Predictions for Polymorphs of Sulphathiazole and Carbamazepine, Eur. J. Pharm. Sci., 2000, 9, 277–283 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/d0ce01659d |
| This journal is © The Royal Society of Chemistry 2021 |