Metal-centered monocyclic carbon wheel clusters with record coordination numbers in planar species

Abstract

The highest coordination number identified to date in planar species is CN = 10 in metal-centered monocyclic boron wheel clusters D_10h M©B₁₀⁻ (M = Ta and Nb) (Galeev et. al., Angew. Chem. Int. Ed., 2012, 51, 2101). Extensive global minimum searches and first-principles theory calculations performed herein indicate that the experimentally observed LaC₁₃⁺ and LaC₁₄⁺ possess the well-defined global minima of perfect metal-centered monocyclic carbon wheel D_13h La©C₁₃⁺ (1) and slightly off-centered C_2v La©C₁₄⁺ (4) (¹A₁) with record coordination numbers of CN = 13 and 11 in planar structures, respectively, further pushing the boundary of our understanding of chemical structures and bonding. Detailed molecular orbital, nucleus-independent chemical shift, and ring current analyses indicate that D_13h La©C₁₃⁺ (1) is σ + π dually aromatic in nature, with 14 totally delocalized in-plane σ electrons and 14 totally delocalized out-of-plane π electrons each matching the 4N + 2 aromatic rule (N_σ = N_π = 3). Similar σ + π dually aromatic metal-centered monocyclic wheel clusters D_13h Ca©C₁₃ (2), C_13v Ac©C₁₃⁺ (3), C_2v Y©B₆C₆⁺ (5), and C_2v Sc©B₅C₆ (6) have also been obtained with CN = 13, 13, 12, and 11, respectively. The results obtained in this work effectively enrich the chemical structures and bonding patterns of planar hypercoordinated complexes.

---

1. Introduction

Searching for the maximum coordination number in planar species has fascinated chemists for many years and continuously pushed the boundary of our understanding of chemical structures and bonding.^1,2 The central atom and periphery atoms around it in the ligand in stable planar hypercoordinated structures must match both geometrically and electronically, i.e., they must have the right atomic sizes and electronic configurations. The experimentally observed boron-centered monocyclic boron wheel clusters D_7h B©B₇²⁻ and D_8h B©B₈⁻ are good examples in which the central B atoms have the coordination numbers of CN = 7 and 8, respectively.³ These boron cluster monoanions prove to be σ + π dually aromatic in nature with six delocalized σ and six delocalized π electrons (6 σ + 6 π) each conforming to the 4N + 2 aromatic rule (N_σ = N_π = 1). Based on the double aromaticity requirement in bare boron wheel clusters, a general electronic design principle x + n + k = 12 or 16 was developed for metal-centered monocyclic boron wheel clusters M©B_n^k− by the groups of Wang and Boldyrev,^1,4–6 where x stands for the formal valence of the metal center M. This design principle has been successfully applied to the experimentally characterized octacoordinated D_8h Co©B₈⁻ with CN = 8,⁵ nonacoordianted D_9h Ru©B₉⁻, D_9h Rh©B₉⁻, and D_9h Ir©B₉⁻ with CN = 9,^7,8 and, finally, to the decacoordinated D_10h Ta©B₁₀⁻ and D_10h Nb©B₁₀⁻ with CN = 10 which has proven to be the highest coordination number in planar species observed to date.^1,2

However, planar hypercoordination chemistry may go well beyond metal-centered monocyclic boron wheel clusters. The recent experimental characterization of perfect planar D_9h C₁₈ by atom manipulations and high-resolution atomic force microscopy⁹ inspires us to coordinate transition metal centers with bare cyclo[n]carbon ring-like clusters (C_n, n ≥ 11) to form metal-centered monocyclic carbon wheel complexes M©C_n^k+. Very recently, the electronic properties of metal-carbon ring complexes such as Li@C₁₈ and MC₁₆ have been reported.^10,11 Carbon ([He]2s²2p²) is known to be effective ligand to various transition metals with a smaller covalent radius than boron ([He]2s²2p¹), while group IIIB metals La, Y, and Sc with the valence electronic configurations of (n-1)d¹ns² possess the largest covalent radii in the periodic table.¹² Metal-centered monocyclic carbon wheel clusters M©C_n^k+ (M = La, Y, Sc) are thus possible to have even higher coordination numbers than their boron counterparts M©B_n^k−. In fact, two families of LaC_n⁺ monocations (n = 12–40) were observed in mobility measurements as early as in 1994, with a La atom inserted into the carbon ring for even-numbered clusters or attached to the inside or outside of the carbon ring for odd-numbered clusters.¹³ More detailed mass spectra were reported late on LaC_n⁺ in which LaC₁₃⁺ appeared to be a prominent species with the La center most likely attached inside a C₁₃ ring (ring Ib), while LaC₁₄⁺ was the only LaC_2n⁺ cluster with even number of C atoms for which ring Ib structure had a higher mass intensity than its competing isomer with a La atom inserted into a C₁₄ ring (ring Ia).¹⁴ Similar prominent mass peaks were also observed for YC₁₃⁺, CeC₁₃⁺, and ScC₁₃⁺.¹⁵ Early density functional theory (DFT) calculations indicated that the most stable isomer of LaC₁₃⁺ possessed a nearly-cumulenic structure with the La atom located at the center of the carbon ring.^16,17 However, the unique role these experimentally observed LaC_n⁺ species (n = 13, 14) play in planar hypercoordination chemistry has largely omitted in previous investigations and their accurate geometrical and electronic structures and detailed La–C coordination bonding patterns remain to be fully evaluated using the state-of-the-art theoretical approaches to interpret their behaviors observed in experiments.

Detailed first-principles theory calculations performed in this work indicate that the experimentally observed La©C₁₃⁺ (1) with a perfect D_13h symmetry and slightly off-centered La©C₁₄⁺ (4) with a C_2v geometry achieve the record coordination numbers of CN = 13 and 11 in planar species reported to date. The enhanced stability of La©C₁₃⁺ (1) originates from its σ + π dual aromaticity with 14 delocalized σ electrons and 14 delocalized π electrons (14 σ + 14 π) each matching the 4N + 2 aromatic rule (N_σ = N_π = 3). σ + π dually aromatic D_13h Ca©C₁₃ (2), C_13v Ac©C₁₃⁺ (3), C_2v Y©B₆C₆⁺ (5), and C_2v Sc©B₅C₆ (6) have also been obtained at first-principles theory level with CN = 13, 13, 12, and 11, respectively. The highly stable La©C₁₃⁺ (1) is found to behave like a super-hydrogen monocation (H⁺) in its substituted complex compounds.

2. Theoretical procedure

Extensive global-minimum (GM) searches were performed on LaC₁₃⁺, LaC₁₄⁺, CaC₁₃, AcC₁₃⁺, YB₆C₆⁺, ScB₅C₆, and CeC₁₃^+/2+ using the TGmin2 code¹⁸ at DFT level based on the constraint basin-hopping algorithm.¹⁹ In total, more than 1000 stationary points with different spin multiplicities were probed for each species at PBE/DZVP level using the CP2K program.^20,21 Low-lying isomers were then fully optimized at the M06-2X and PBE0 level,^22,23 with the 6-311+G(d,p) basis sets for C, B, N, Ca, and Sc²⁴ and Stuttgart relativistic small-core pseudopotentials for La, Y, Ce, and Ac,^25,26 using the Gaussian-16 program suite.²⁷ Frequency analyses were performed to make sure all the optimized structures are true minima of the systems. Relative energies for the five lowest-lying isomers were further refined using the more accurate coupled cluster method with triple excitations CCSD(T)^28–30 implemented in Molpro³¹ with the basis set of cc-pVTZ for C, B, N, Ca, and Sc and the Stuttgart small-core pseudopotential for La, Y, and Ac. The optimized GM structures are summarized in Fig. 1 and more alternative isomers tabulated in Fig. S1–S6.† Natural bonding orbital (NBO) analyses were performed using the NBO 6.0 program.³² Born–Oppenheimer molecular dynamics (BOMD) simulations were performed on LaC₁₃⁺ (1) for 30 ps using the CP2K software suite at 300, 800, and 1000 K.²¹ The anisotropy of the current-induced density (ACID)³³ analyses were performed using the ACID code, with the ring-current maps generated using POV-Ray 3.7.³⁴ The iso-chemical shielding surfaces (ICSSs)^35,36 were generated with the Multiwfn 3.8 code.³⁷ The UV-vis spectra were simulated using the time-dependent TD-DFT-M06-2X approach.³⁸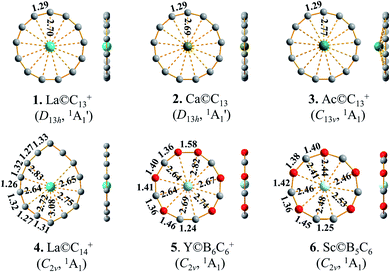

Fig. 1 Top and side views of the optimized La©C₁₃⁺ (1), Ca©C₁₃ (2), Ac©C₁₃⁺ (3), La©C₁₄⁺ (4), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6) at M06-2X level, with bond lengths indicated in Å.

3. Results and discussion

We start from LaC₁₃⁺, the most concerned species observed in LaC_n⁺ series (n = 12–40).¹³ Encouragingly and interestingly, as shown in Fig. 1 and S1,† extensive GM searches indicate that LaC₁₃⁺ possesses the well-defined perfect planar GM of D_13h La©C₁₃⁺ (1) which contains a La atom located exactly at the center of the C₁₃ wheel ligand, with the optimized La–C coordination bond lengths of r_La–C = 2.70 Å which are slightly longer than the sum of the self-consistent single-bond covalent radii of La and C (2.55 Å)¹² and C–C bond lengths of r_C–C = 1.29 Å which lie between C=C double-bond (1.34 Å) and C≡C triple bond (1.20 Å), setting up the highest coordination number of CN = 13 in planar species reported to date. La©C₁₃⁺ (1) possesses the huge HOMO–LUMO gap of ΔE_gap = 5.33 eV at M06-2X level, well underlying its high chemical stability. The slightly distorted triplet C_s LaC₁₃⁺ (³A′) appears to be the second lowest-lying isomer lying 2.41 eV above the GM at CCSD(T). The seventh C_2v isomer with a La inserted into the C₁₃ ring and the fifth C_2v isomer with a La attached to the outside of the C₁₃ ring are found to lie 3.36 eV and 3.09 eV higher in energy than the GM at M06-2X, respectively. Extensive BOMD simulations indicate that La©C₁₃⁺ (1) is highly dynamically stable at both 800 K and 1000 K, with the small average root-mean-square-deviations of RMSD = 0.11 and 0.13 Å and maximum bond length deviations of MAXD = 0.31 and 0.36 Å, respectively (Fig. S7†). No high-lying isomers were observed during the dynamical simulations.

Replacing the La center in La©C₁₃⁺ (1) with a Ca atom generates the charge-transfer neutral complex D_13h Ca©C₁₃ (2). The Ca center in Ca©C₁₃ (2) matches the C₁₃ wheel ligand perfectly both electronically and geometrically though it has a much lower Wiberg bond index of WBI_Ca = 0.26 than La in La©C₁₃⁺ (1) where WBI_La = 1.76 (Table 1). The Ca–C coordination interaction with the low bond order of WBI_Ca–C = 0.02 in Ca²⁺©C₁₃²⁻ (2) is thus almost purely ionic. Using an Ac atom which has a larger atomic radius than La to replace La in La©C₁₃⁺ (1), the slightly buckled C_13v Ac©C₁₃⁺ (3) is generated in which the Ac atom lies 0.59 Å above the C₁₃ ring, with the periphery C–C distances of r_C–C = 1.29 Å remaining basically unchanged. The experimentally observed prominent ScC₁₃⁺ and YC₁₃⁺ monocations¹⁵ have severely off-centered C_2v Sc©C₁₃⁺ (¹A₁) (with CN = 8) and C_2v Y©C₁₃⁺ (¹A₁) (with CN = 9) GM structures due to strong ring strains, respectively, while the open-shell CeC₁₃⁺ possesses a slightly distorted GM C_2v Ce©C₁₃⁺ (²B₂) which has practically a D_13h symmetry (Fig. S8†). With one more valence electron detached, the Ce©C₁₃²⁺ dication isovalent with La©C₁₃⁺ (1) has indeed a perfect D_13h GM (Fig. S8†).

Table 1 Calculated HOMO–LUMO gaps ΔE_gap/eV, natural atomic charges q_M, electronic configurations, and Wiberg bond indexes WBI_M of the metal centers M and Wiberg bond indexes of the M–C (WBI_M–C) and M–B (WBI_M–B) coordination interactions and NICS values 1.0 Å above the metal centers (NICS (1)) of the M^(x)©B_mC_n^k± complex series (1–6) at M06-2X level

M^(x)©BmCn^k±	CN	ΔEgap/eV	qM	Electronic configurations of central metal M	WBIM	WBIM–C	WBIM–B	NICS (1)
D13h La©C13^+ (1)	13	5.33	2.08	[Xe] 4f^0.435d^0.416s^0.02	1.76	0.14	—	−58.54
D13h Ca©C13 (2)	13	5.63	1.87	[Ar] 3d^0.094s^0.034p^0.01	0.26	0.02	—	−44.68
C13v Ac©C13^+ (3)	13	5.26	2.38	[Rn] 5f^0.196d^0.357s^0.03	1.22	0.10	—	−55.16
C2v La©C14^+ (4)	11	3.72	2.15	[Xe] 4f^0.285d^0.506s^0.03	1.64	0.07–0.16	—	−29.21
C2v Y©B6C6^+ (5)	12	5.70	2.12	[Kr] 5s^0.064d^0.705p^0.01	1.67	0.10–0.22	0.09–0.12	−24.08
C2v Sc©B5C6 (6)	11	5.32	1.94	[Ar] 3d^0.874s^0.074p^0.02	1.94	0.13–0.29	0.13–0.14	−29.62

---

It is natural to ask at current stage whether it is possible to form metal-centered monocyclic carbon wheel clusters with coordination numbers greater than thirteen (i.e., CN > 13). We carefully checked the hypercoordination chemistry of the experimentally observed La©C₁₄⁺ in this work. Although a bare C₁₄ has a perfect C_7h acetylenic structure,³⁹ with a La atom added in, the La-doped LaC₁₄⁺ possesses the off-centered planar GM of C_2v La©C₁₄⁺ (4) which has the actual coordination number of CN = 11 (Fig. 1 and S4†). The three C atoms on the top part of La©C₁₄⁺ (4) with La–C distances great than 3.1 Å have the practically negligible La–C coordination bond orders (with WBI_La–C ≈ 0.00). Using Ac, the largest actinide metal in the periodical table, to replace La,¹² an elongated planar D_2h Ac©C₁₄⁺ (Fig. S8†) with CN = 12 is generated. A C₁₄ ring is obviously too big in size to host a transition-metal atom at its geometrical center comfortably to form a perfect wheel complex D_nh M©C_n with the same M–C coordination bonding distances. We conclude that CN = 13 is the highest coordination number in metal-centered monocyclic carbon wheel clusters M©C_n⁺ with effective M–C coordination interactions.

Introducing certain numbers of B atoms into the carbon rings generates more structural diversities in B–C binary wheel ligands B_mC_n with CN ≤ 13. As examples, the metal-centered monocyclic C–B binary wheel complexes Y©B₆C₆⁺ (5) and Sc©B₅C₆ (6) in Fig. 1 and C_2v La©BC₁₂ in Fig. S8† as the GMs of the systems possess the coordination numbers of CN = 12, 11, and 13, respectively. These planar C–B binary wheel complexes have larger C–B and B–B periphery distances than the corresponding C–C distances in La©C₁₃⁺ (1) because B has a larger covalent radius than C.

To interpret the high stabilities of these hypercoordinated planar species, we performed detailed NBO and molecular orbital analyses on La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6). As tabulated in Table 1, the La center in La©C₁₃⁺ (1) possesses the natural atomic charge of q_La = + 2.08 |e|, electronic configuration of La[Xe]4f^0.435d^0.416s^0.02, and total Wiberg bond order of WBI_La = 1.76. The C atoms on the C₁₃ wheel ligand have the total Wiberg bond orders of WBI_C = 3.99 and C–C bond orders of WBI_C–C = 1.73, revealing the cumulenic nature of the complex. Obviously, the La center donates its 6s electron almost completely to the C₁₃ ligand. The La–C₁₃ coordination interactions mainly originate from contributions involving the 5d and 4f atomic orbitals of the La center, as indicated in the degenerated π-HOMO/HOMO′, π-HOMO-2/HOMO-2′, σ-HOMO-1/HOMO-1′, and σ-HOMO-3/HOMO-3 in Fig. 2(a). Although each La–C coordination interaction in La©C₁₃⁺ (1) has a relatively low bond order (WBI_La–C = 0.14), the thirteen equivalent La–C coordination bonds function together to effectively stabilize the hypercoordinated cluster, making it the well-defined GM of the system observed solely in gas-phase experiments.^13,14 Similar situations happen in Ac©C₁₃⁺ (3), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6).

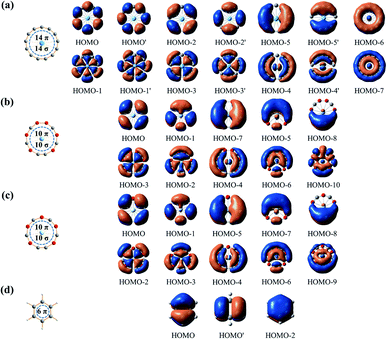Fig. 2 Delocalized π- and σ-CMOs of (a) La©C₁₃⁺ (1), (b) Y©B₆C₆⁺ (5), and (c) Sc©B₅C₆ (6), in comparison with the delocalized π-CMOs of (d) D_6h C₆H₆.

The 14 totally delocalized canonical molecular orbitals (CMOs) of La©C₁₃⁺ (1) are collectively shown in Fig. 2(a), including 7 delocalized π-CMOs and 7 delocalized σ-CMOs. Its remaining 13 σ-CMOs correspond to 13 two-center-two-electron (2c-2e) C–C σ bonds along the periphery of the C₁₃ ligand (Fig. S9†). The degenerate f-type HOMO/HOMO′, d-type HOMO-2/HOMO-2′ and p-type HOMO-5/HOMO-5′ and non-degenerate s-type HOMO-6 possess three, two, one, and zero nodal surfaces, respectively, forming an out-of-plane 14 π electron system matching the 4N_π + 2 aromatic rule with N_π = 3. Similarly, the degenerate HOMO-1/HOMO-1′, HOMO-3/HOMO-3′, and HOMO-4/HOMO-4′ and non-degenerate HOMO-7 form an in-plane 14 σ electron system matching the 4N_σ + 2 aromatic rule with N_σ = 3. Such a unique 14 σ + 14 π electronic configuration renders σ + π dual aromaticity to La©C₁₃⁺ (1), effectively stabilizing the monocation observed in experiments, similar to the situation in the σ + π dually aromatic D_10h Ta©B₁₀⁻ which possesses a 10 σ + 6 π electronic configuration.¹ It is noticed that metal-centered wheel D_13h La©C₁₃⁺ (1) has the same numbers of delocalized σ and π electrons (14 σ + 14 π) as the highly stable ring-like acetylenic C_7h C₁₄.³⁹

As shown in Fig. 2(b) and (c), both the metal-centered monocyclic C–B binary wheel clusters Y©B₆C₆⁺ (5) and Sc©B₅C₆ (6) have 5 delocalized π-CMOs and 5 delocalized σ-CMOs, forming an out-of-plane 10 π electron system conforming to the 4N_π + 2 π-aromatic rule (N_π = 2) and an in-plane 10 σ electron system conforming to 4N_σ + 2 σ aromatic rule (N_σ = 2). Such 10 σ + 10 π electronic configurations make both Y©B₆C₆⁺ (5) and Sc©B₅C₆ (6) σ + π dually aromatic in nature, similar to but with more delocalized electrons than the previously reported Co©B₈⁻ and Ru©B₉⁻ with 6 σ + 6 π delocalized electrons and Ta©B₁₀⁻ and Nb©B₁₀⁻ with 10 σ + 6 π delocalized electrons.^1,5–8

The La–C₁₃ coordination interactions in La©C₁₃⁺ (1) mainly originate from d–p and f–p σ-coordination interactions involving the in-plane La 5d_x²−y²/5d_xy and 4f_{x(x²−3y²)}/4f_{y(y²−3x²)} atomic orbitals and C 2p_x/2p_y hybridized atomic orbitals, as demonstrated in the degenerated HOMO-3/HOMO-3′ (5d–2p σ-coordination) and HOMO-1/HOMO-1′ (4f–2p σ-coordination) in Fig. 2(a), respectively. Quantitatively, the La center contributes more to the delocalized in-plane σ-CMOs (2.81–7.63%) than to the delocalized out-of-plane π-CMOs (0.08–3.66%). The delocalized in-plane σ-CMOs thus dominate the La–C coordination bonding interactions. Similarly, the in-plane 4d–2p σ-coordination interactions (HOMO-2 and HOMO-3) in Y©B₆C₆⁺ (5) and 3d–2p σ-coordination interactions (HOMO-2 and HOMO-3) in Sc©B₅C₆ (6) dominate the metal–ligand interactions in the two 10 σ + 10 π systems.

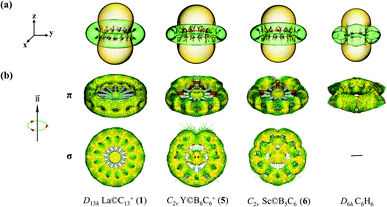
The aromatic nature of La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6) is further evidenced by their calculated nucleus-independent chemical shift (NICS) values. Based on the calculated NICS-ZZ components, Fig. 3(a) depicts the ICSS surfaces of 1, 5, and 6 with the Z-axis perpendicular to the molecular planes to illuminate the chemical shielding around the metal centers, in comparison with that of the prototypical aromatic benzene (C₆H₆). Obviously, the space inside the C₁₃, B₆C₆, or B₅C₆ rings in horizontal direction or within about 1.0 Å above the metal centers in vertical direction belong to chemical shielding regions with negative NICS-ZZ values (highlighted in yellow), while the chemical de-shielding areas with positive NICS values (highlighted in green) are located outside the wheel ligands in horizontal direction. Fig. 3(a) clearly shows the ICSS surfaces of these metal-centered planar complexes are exactly similar to that of the aromatic benzene.

Fig. 3 (a) Calculated iso-chemical shielding surfaces (ICSSs) of La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6), compared with that of aromatic benzene C₆H₆. Yellow and green regions stand for chemical shielding and de-shielding areas, respectively. (b) Calculated π- and σ-ring current maps of La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6), respectively, in comparison with the π-ring current map of D_6h C₆H₆. The external magnetic field is perpendicular to the wheel plane. The red arrows represent directions and magnitudes of the ring currents at various positions on the ACID iso-surfaces.

Calculating the anisotropy of current-induced density (ACID)³⁴ is an effective approach to graphically display the ring currents induced by an external magnetic field in vertical directions perpendicular to the molecular planes. ACID can be decomposed into σ and π components separately. Fig. 3(b) clearly indicates the π-ring current maps of La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6) are extremely similar to the corresponding π-ring current map of π-aromatic benzene C₆H₆. Besides, in contrast to benzene which possesses no delocalized σ-electrons, La©C₁₃⁺ (1), Y©B₆C₆⁺ (5), and Sc©B₅C₆ (6) also exhibit strong σ-ring currents, rendering additional σ-aromaticity to stabilize the systems, as shown in Fig. 3(b) (see Fig. S10† for high-resolution π- and σ-ring current maps of 1, 5, and 6). The observation of both σ- and π-diatropic ring currents in 1, 5, and 6 well supports the σ + π dually aromatic nature of these planar hypercoordinated complexes.

Based on the planar hypercoordinated species discussed above, we develop a universal electronic design principle for σ + π dually aromatic metal-centered monocyclic boron, carbon, or boron-carbon binary wheel clusters M^(x)©B_mC_n^k±. There exist m + n 2c-2e σ bonds along the periphery of the monocyclic B_mC_n wheel ligand. With each C atom providing two delocalized electrons and each B atom contributing one delocalized electron, the total number of the delocalized electrons in M^(x)©B_mC_n^k± matches the requirement of σ + π dual aromaticity of the system:

m + 2n + x ± k = L (L = 12, 16, 20, or 28) (1)

where L is the total number of delocalized σ and π electrons each matching the 4N + 2 rule and x stands for the formal valence of the central atom M. Such an electronic design principle covers the previously observed D_7h B©B₇²⁻, D_8h B©B₈⁻, D_9h Co©B₈⁻, and D_9h Ru©B₉⁻ where L = 12 and x = 3, 3, 3, and 2 and D_10h Ta©B₁₀⁻ and D_10h Nb©B₁₀⁻ where L = 16 and x = 5 and 5, respectively. For the currently discussed La©C₁₃⁺ (1), Ca©C₁₃ (2), and Ac©C₁₃⁺ (3), L = 28 and x = 3, 2, and 3, while for Y©B₆C₆⁺ (5) and Sc©B₅C₆ (6), L = 20 and x = 3 and 3, respectively. The slightly buckled C_13v Ac©C₁₃⁺ (3) follows the same electronic design principle though the Ac center in it slightly mismatches the C₁₃ wheel ligand in geometry. Eqn (1) can be easily extended to σ + π dually aromatic B–C–N ternary wheel complexes M^(x)©B_mC_nN_l ^k± where it reads: m + 2n + 3l + x ± k = 28, as demonstrated in the cases of C_s La©BNC₁₁⁺ and C_s La©B₄N₄C₅⁺ (Fig. S8†) in which each N atom contributes three valence electrons to the delocalized systems. However, extensive test calculations indicate that inclusion B or N atoms in the wheel ligands does not help to improve the maximum coordination numbers of the systems.

The highly stable La©C₁₃⁺ (1) with a wide HOMO–LUMO gap and fully occupied bonding inner-shell CMOs can be used as a super-hydrogen monocation (H⁺) to form various substituted multi-nuclei complexes. Typical examples include C_13v [La©C₁₃]X (X = F, Cl, Br) (7), D_13h [La©C₁₃]⁺L₂ (L = Ar, Kr) (8), C_2v [La©C₁₃]₂O (9), and C_3v N[La©C₁₃]₃ (10) (Fig. 4) which can be derived from the parent species C_∞v HX, D_∞h H⁺L₂, C_2v H₂O, and C_3v NH₃ by substituting H⁺ monocation(s) with La©C₁₃⁺ (1) unit(s), respectively, presenting the viable possibility to form complex compounds with multiple hypercoordinated metal centers.

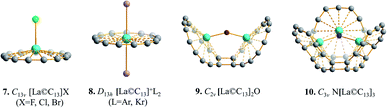Fig. 4 Optimized structures of [La©C₁₃]X (7) (X = F, Cl, Br), [La©C₁₃]⁺L₂ (8) (L = Ar, Kr), [La©C₁₃]₂O (9), and N[La©C₁₃]₃ (10) at M06-2X level.

Finally, as examples, the simulated IR, Raman, and UV-vis spectra of D_13h La©C₁₃⁺ (1) and C_2v Y©B₆C₆⁺ (5) are presented in Fig. S11† to facilitate their spectroscopic characterizations. La©C₁₃⁺ (1) possesses highly simplified spectra due to its perfect D_13h symmetry. It has two main IR peaks at 39 and 866 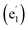cm⁻¹, three Raman peaks at 272 , 664 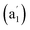, and 1280 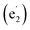cm⁻¹, and three UV absorption peaks at 156 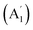, 174 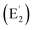, and 200 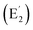nm, respectively. C_2v Y©B₆C₆⁺ (5) has more complicated spectra, with two main IR absorption peaks at 499 (b₁) and 588 (b₂) cm⁻¹, two major Raman scattering bands at 211 (b₂) and 623 (a₁) cm⁻¹, and five UV absorption bands around 134 (¹B₂), 161 (¹A₁), 191 (¹B₂), 207 (¹A₁), and 219 (¹B₂) nm, respectively.

4. Conclusions

Extensive first-principles theory calculations performed in this work unveil the highest coordination numbers of CN = 13 in La©C₁₃⁺ (1), Ca©C₁₃ (2), and Ac©C₁₃⁺ (3), CN = 12 in Y©B₆C₆⁺ (5), and CN = 11 in La©C₁₄⁺ (4) and Sc©B₅C₆ (6) reported to date in planar species, effectively enriching the structural and bonding patterns in planar hypercoordination chemistry. σ + π dually aromatic metal-centered monocyclic B–C binary wheel complexes M^(x)©B_mC_n^k± follow the electronic design principle of m + 2n + x ± k = L (L = 12, 16, 20, or 28) which can be readily extended to more complicated systems containing other periphery atoms rather than B and C. The recent experimental identification of the sp-hybridized molecular carbon allotrope, π-aromatic C₁₈, induced by atom manipulations,⁹ presents the possibility to synthesize and characterize the σ + π dually aromatic La©C₁₃⁺ (1) and its complex compounds to open a new area in planar hypercoordination chemistry, catalysis, and materials science.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The work was supported by the National Natural Science Foundation of China (21720102006 and 21973057 to S.-D. Li).

Notes and references

1. T. R. Galeev, C. Romanescu, W. L. Li, L. S. Wang and A. I. Boldyrev, Angew. Chem., Int. Ed., 2012, 51, 2101–2105.
2. T. Heine and G. Merino, Angew. Chem., Int. Ed., 2012, 51, 4275–4276.
3. H. J. Zhai, A. N. Alexandrova, K. A. Birch, A. I. Boldyrev and L. S. Wang, Angew. Chem., Int. Ed., 2003, 42, 6004–6008.
4. C. Romanescu, T. R. Galeev, W. L. Li, A. I. Boldyrev and L. S. Wang, Angew. Chem., Int. Ed., 2011, 123, 9506–9509.
5. C. Romanescu, T. R. Galeev, W. L. Li, A. I. Boldyrev and L. S. Wang, Angew. Chem., Int. Ed., 2011, 50, 9334–9337.
6. C. Romanescu, T. R. Galeev, W. L. Li, A. I. Boldyrev and L. S. Wang, Acc. Chem. Res., 2013, 46, 350–358.
7. C. Romanescu, T. R. Galeev, W. L. Li, A. I. Boldyrev and L. S. Wang, J. Chem. Phys., 2013, 138, 134315.
8. W. L. Li, C. Romanescu, T. R. Galeev, Z. A. Piazza, A. I. Boldyrev and L. S. Wang, J. Am. Chem. Soc., 2012, 134, 165–168.
9. K. Kaiser, L. M. Scriven, F. Schulz, P. Gawel, L. Gross and H. L. Anderson, Science, 2019, 365, 1299–1301.
10. Z. Liu, X. Wang, T. Lu, A. Yuan and X. Yan, ChemRxiv, 2021 DOI:10.26434/chemrxiv.14601168.v1.
11. Y. Jiang, Y. Wu, J. Deng and Z. Wang, Phys. Chem. Chem. Phys., 2021, 23, 8817–8824.
12. P. Pyykkç and M. Atsumi, Chem.–Eur. J., 2009, 15, 12770–12779.
13. D. E. Clemmer, K. B. Shelimov and M. F. Jarrold, J. Am. Chem. Soc., 1994, 116, 5971–5972.
14. K. B. Shelimov, D. E. Clemmer and M. F. Jarrold, J. Phys. Chem., 1995, 99, 11376–11386.
15. R. Klingeler, P. S. Bechthold, M. Neeb and W. Eberhardt, J. Chem. Phys., 2000, 113, 4.
16. S. Roszak and K. Balasubramanian, Chem. Phys. Lett., 1997, 264, 80–84.
17. D. L. Strout and M. B. Hall, J. Phys. Chem. A, 1998, 102, 641–645.
18. X. Chen, Y. F. Zhao, Y. Y. Zhang and J. Li, J. Comput. Chem., 2019, 40, 1105–1112.
19. D. J. Wales and H. A. Scheraga, Science, 1999, 285, 1368–1372.
20. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
21. J. VandeVondele, M. Krack, F. Mohamed, M. Parrinello, T. Chassaing and J. Hutter, Comput. Phys. Commun., 2005, 167, 103–128.
22. Y. Zhao and D. G. Truhlar, Theor. Chem. Acc., 2008, 120, 215–241.
23. C. Adamo and V. Barone, J. Chem. Phys., 1999, 110, 6158–6170.
24. R. Krishnan, J. S. Binkley, R. Seeger and J. A. Pople, J. Chem. Phys., 1980, 72, 650–654.
25. D. Feller, J. Comput. Chem., 1996, 17, 1571–1586.
26. L. Schuchardt, B. T. Didier, T. Elsethagen, L. Sun, V. Gurumoorthi, J. Chase, J. Li and T. L. Windus, J. Chem. Inf. Model., 2007, 47, 1045–1052.
27. M. J. Frisch, et al., Gaussian 16, Revision A.03, Gaussian Inc., Wallingford, CT, 2016.
28. J. Čížek, Adv. Chem. Phys., 1969, 14, 35.
29. G. D. Purvis III and R. J. Bartlett, J. Chem. Phys., 1982, 76, 1910.
30. K. Raghavachari, G. W. Trucks, J. A. Pople and M. Head-Gordon, Chem. Phys. Lett., 1989, 157, 479–483.
31. H. J. Werner, et al., Molpro, version.1., 2012, (www.%20molpro.net).
32. P. E. D. Glendening, J. K. Badenhoop, A. E. Reed, J. E. Carpenter, J. A. Bohmann, C. M. Morales, C. R. Landis and F. Weinhold, NBO 6.0, 2013.
33. D. Geuenich, K. Hess, F. Köhler and R. Herges, Chem. Rev., 2005, 105, 3758–3772.
34. Povray, Persistence of vision raytracer, POV-Ray 3.7, http://www.povray.org.
35. S. Klod and E. Kleinpeter, J. Chem. Soc., Perkin Trans. 2, 2001, 2, 1893–1898.
36. E. Kleinpeter, S. Klod and A. Koch, J. Mol. Struct., 2007, 811, 45–60.
37. T. Lu and F. W. Chen, J. Comput. Chem., 2012, 33, 580–592.
38. R. Bauernschmitt and R. Ahlrichs, Chem. Phys. Lett., 1996, 256, 454–464.
39. S. Arulmozhiraja and T. Ohno, J. Chem. Phys., 2008, 128, 114301.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/d1ra05367a

---