Aggregation of retained helium and hydrogen in titanium beryllide Be₁₂Ti: a first-principles study

Abstract

Titanium beryllide, Be₁₂Ti, has been proposed as a prospective neutron multiplier in fusion reactors. First-principles calculations have been performed to investigate the nucleation mechanism of a He bubble in bulk Be₁₂Ti. Meanwhile, the influence of the presence of H atoms on the nucleation of the He bubble, i.e., the synergistic effect of He and H atoms, has also been investigated. It has been found that the He bubble will initially nucleate around a monovacancy (V_Be2). When more He atoms have been implanted, two newly induced vacancies (V_Be1 and V_Be3) could be successively observed. The nucleation of the He bubble will occur around the divacancy of V_Be2V_Be1 and the trivacancy of V_Be2V_Be1V_Be3. Dumbbell structures in the He bubble evolve with the number of implanted He atoms and finally disappear. The presence of H atoms will significantly influence the nucleation of the He bubble. It is interesting that some tetrahedral and octahedral structures have also been observed. The maximal number of H atoms trapped by a He bubble has been obtained. These phenomena could be further explained by the continuous shrinking of the isosurface of charge density. The present results provide a microscopic physical foundation to understand the mechanism of He and H atoms retention in neutron multiplier materials. This investigation could be helpful for the design and fabrication of more promising beryllides which could withstand a severe external environment.

---

1 Introduction

As promising neutron multiplier materials, beryllium (Be) and beryllium intermetallic compounds (e.g., Be₁₂Ti) have potential applications in accelerators of boron neutron capture therapy (BNCT)¹ and solid breeding blankets in fusion demonstration (DEMO) reactors.^2,3 However, the radiogenic gas tritium (hydrogen isotope, T) and helium (He) with different concentrations simultaneously produced by neutron irradiations will inevitably accumulate in Be⁴ and beryllides,⁵ which could induce structural damage⁶ and degradation of material properties.^7,8 In particular, the retention of radioactive tritium could bring difficulties in the disposal of Be-based wastes. The retention and release of tritium and helium in neutron multiplier materials have been one of the key issues for the design and safety assessment of fusion reactors.⁶ Beryllides, such as Be₁₂Ti, are superior to pure Be due to lower irradiation swelling⁹ and smaller hydrogen (H) retention.¹⁰

Under irradiation, various defects, such as vacancies, could be produced. H or He atoms could be easily trapped by vacancies to form H/He–vacancy (abbreviated as H/He–V) complexes^11,12 owing to the attraction of vacancies to H or He atoms. With more H or He atoms binding to H/He–V complexes, small gas bubbles begin to form, and finally grow large with the coalescence of small bubbles.¹² The distribution, density and mean size of the He bubbles are dependent on the irradiation temperature, the structure of the irradiated material itself and the energy and concentration of the implanted He ions.^13,14 The synergistic effect between He and H atoms is an interesting topic of research in gas retention and release. It has been reported¹⁵ that, in Be co-deposits, a lower He concentration could promote the retention of deuterium (hydrogen isotope, D) while a higher He concentration could reduce the retention of D. He bubbles could grow larger under subsequent H irradiations.¹² The evidence for the co-existence of T and He in common bubbles has been found in research on the desorption of T and He atoms in Be.^16,17 During the evolution of mixed gas bubbles, which contain both H and He atoms, H atoms tend to be distributed on the surface of the He bubbles.^18,19 However, to our knowledge, the phenomena of mixed bubbles containing both H and He atoms are rarely reported in titanium beryllide Be₁₂Ti.

First-principles calculations could provide a feasible way to better understand the physical micromechanism of various irradiation effects.^20–22 In particular, the early stage of nucleation of He or H bubbles has been widely simulated by first-principles calculations.^23–25 For pure Be, the calculated formation energy of a monovacancy is consistent with the data deduced from experiment.²⁶ Divacancies in Be are energetically unstable,²⁷ but He atoms could stabilize the divacancies when they bind to divacancy clusters, which are in specific orientations.²⁸ A monovacancy could trap up to five H atoms or twelve He atoms in pure Be, and the formation of a H₂ molecule is impossible.²⁹ The research³⁰ has shown that the diffusion of a single H atom in Be₁₂Ti with a vacancy becomes relatively difficult due to a higher barrier energy compared with that in perfect Be₁₂Ti. This indicates that vacancies could act as traps for H atoms. It has been further investigated³¹ that three different types of Be vacancies could all trap four H atoms and a Ti vacancy could trap ten H atoms.

However, few works have been focused on the investigation of the micromechanism of He bubble nucleation, especially the influence of the presence of H atoms on the He bubbles, i.e., the synergistic effect of H and He atoms in Be₁₂Ti. The purpose of this work is to model the nucleation of He bubbles, primarily by investigating the ability of a monovacancy to trap multiple He atoms in Be₁₂Ti. Besides, the mixed bubbles containing both H and He atoms are also simulated further to explore the synergistic effect of H and He atoms, which is significant in understanding the micromechanism behind the retention of He and H atoms in Be₁₂Ti.

2 Computational methodology

In this work, all the first-principles calculations are carried out within the density functional theory (DFT) framework as implemented in the Vienna Ab initio Simulation Package (VASP).^32,33 The ion–electron interaction is described using the projector augmented-wave (PAW) method.³⁴ The generalized gradient approximation (GGA) developed by Perdew and Wang³⁵ has been employed to calculate the exchange–correlation energy. All the defect calculations are performed based on a tetragonal supercell containing 208 atoms. The plane-wave cutoff energy is set as 500 eV, and a k-point mesh of 2 × 2 × 4 is employed for Brillouin-zone integration according to the Monkhorst–Pack scheme,³⁶ which has been well tested. The shape and volume of perfect bulk Be₁₂Ti have been fully relaxed and the dimensions are kept fixed for all the defect calculations. For the geometry optimization, all atoms are relaxed until the total energy difference and forces on each atom are less than 10⁻⁶ eV and 0.001 eV Å⁻¹, respectively.

The solution energy for a single H or He atom at an interstitial site in Be₁₂Ti has the following formalism:

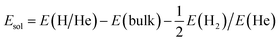where E(H/He) is the total energy of perfect Be₁₂Ti containing one H or He atom in an interstitial site, E(bulk) is the total energy of perfect Be₁₂Ti, E(H₂) and E(He) are the total energies of one H₂ molecule or one isolated He atom in the vacuum, respectively.

The formation energy of one vacancy in Be₁₂Ti has the following formalism:

E_for = E(V) − E(bulk) + μ_X (2)

where E(V) is the total energy of the supercell with one vacancy, μ_X is the chemical potential of element X (X = Be, Ti).

The binding energy between one vacancy and a single H or He atom has the following formalism:

E_bind = E(H/He,V) − E(H/He) − E(V) + E(bulk) (3)

where E(H/He,V) is the total energy of the supercell containing one vacancy and one H or He atom. A positive binding energy indicates that the interaction between the vacancy and H/He is repulsive, while it is attractive with a negative binding energy.³⁷

The solution energy for nHe and/or mH atoms (n ≥ 1, m ≥ 0) in Be₁₂Ti with one vacancy is calculated by the following formalism:

where E(nHe,V,mH) refers to the total energy of the system containing nHe atoms, one vacancy and/or mH atoms.

To interpret the degree of lattice deformation, the deformation energy induced by the incorporation of nHe and/or mH atoms into the system with one vacancy has also been calculated by the following formalism:

E_def = E[(He_n–V–H_m)–nHe–mH] − E(V) (5)

where E[(He_n–V–H_m)–nHe–mH] refers to the static energy of the distorted supercell after nHe and/or mH atoms have been removed. E(V) represents the static energy of the original undistorted supercell which contains one vacancy.

The trapping energy has been defined as the energy required to move an interstitial He atom into one vacancy or an interstitial H atom into a He_n–V complex. For He atoms trapped by one vacancy, the trapping energy has the following formalism:

E_trap = E(He_n–V) E(He_n−1–V) − E(He_I) + E(bulk) (6)

where E(He_n–V) represents the total energy of the supercell containing one He_n–V complex and E(He_I) corresponds to the total energy of the supercell with one He atom in an interstitial site.

For H atoms trapped by a He_n–V complex, the trapping energy has the following formalism:

E_trap = E(He_n–V–H_m) − E(He_n–V–H_m−1) − E(H_I) + E(bulk) (7)

where E(He_n–V–H_m) is the total energy of the supercell containing the He_n–V–H_m complex. E(H_I) represents the total energy of the supercell containing one H atom in an interstitial site.

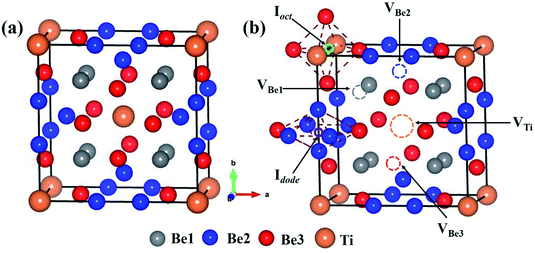
The bulk Be₁₂Ti has tetragonal symmetry in the space group of I₄/mmm.^38,39 Ti atoms occupy the Wyckoff position of the 2a lattice site (0, 0, 0), and three symmetrically different Be atoms, which are labeled as Be1, Be2 and Be3, occupy the Wyckoff positions of 8f (0.25, 0.25, 0.25), 8i (0.361, 0, 0) and 8j (0.277, 0.5, 0), respectively. The structure of the unit cell for Be₁₂Ti is shown in Fig. 1(a), where the orange spheres represent Ti atoms and the grey, blue and red spheres represent Be1, Be2 and Be3 atoms, respectively. The obtained lattice constants are a = b = 7.328 Å, and c = 4.145 Å, which are in good agreement with previous experimental^38,40 and theoretical calculation results.^31,41,42

Fig. 1 (a) Schematic diagram for the unit cell of the tetragonal Be₁₂Ti crystal structure, (b) schematic diagram for the four vacancies (V_Ti, V_Be1, V_Be2 and V_Be3) and two interstitial sites (I_oct and I_dode) in Be₁₂Ti. The orange, grey, blue and red spheres denote Ti, Be1, Be2 and Be3 atoms, respectively. The orange, grey, blue and red circles represent V_Ti, V_Be1, V_Be2 and V_Be3, respectively. The green and purple circles refer to I_oct and I_dode, respectively.

3 Results and discussion

3.1 Stability of individual H and He atoms in Be₁₂Ti

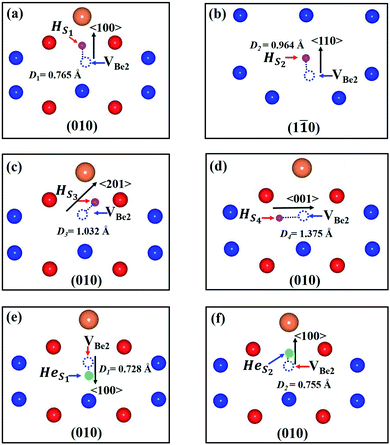
The stability of individual H and He atoms in perfect Be₁₂Ti has been investigated. There are seven energetically stable interstitial sites for single H and He atoms.³⁰ For a single H atom, it has the lowest solution energy of 0.509 eV at an octahedral interstitial site (I_oct), as shown in Fig. 1(b). For an individual He atom, it preferentially occupies a dodecahedral interstitial site (I_dode) (Fig. 1(b)) with the lowest solution energy of 4.028 eV. These results are consistent with the previous results.³⁰

There are four types of vacancies: V_Be1 (Be1 vacancy), V_Be2 (Be2 vacancy), V_Be3 (Be3 vacancy) and V_Ti (Ti vacancy), as shown in Fig. 1(b). The formation energies of these vacancies are summarised in Table 1, together with previous results for comparison. Obviously, the vacancy V_Be2 has the lowest formation energy among these vacancies. Therefore, we have only investigated the interactions between the defect of V_Be2 and H/He atoms throughout the work. A thorough search has been performed to ascertain the preferential site for both H and He atoms around V_Be2.

Table 1 Formation energies of the four different vacancies in bulk Be₁₂Ti

Configuration	VBe1 (eV)	VBe2 (eV)	VBe3 (eV)	VTi (eV)
This work	1.658	1.450	1.568	3.949
Ref. ^30	1.650	1.440	1.560	3.920
Ref. ^41	1.600	1.430	1.530	4.100

---

As we can see from Fig. 2, there are four stable sites and two stable sites distributed in the different crystallographic planes for the presence of single H and He atoms, respectively. Meanwhile, the solution energies and binding energies are presented in Table 2. V_Be2 exhibits attraction to both H and He atoms. For a single H atom, V_Be2 has the strongest attraction to a H atom, with a binding energy of −0.670 eV. H atom and V_Be2 are distributed approximately along the direction of 〈201〉 in the plane of (010), and the distance between the H atom and the center of the Be2 vacancy is 1.032 Å, as shown in Fig. 2(c). For a single He atom, the configuration shown in Fig. 2(e) corresponds to the most stable state, with a binding energy of −2.130 eV and a distance of 0.728 Å. A similar tendency could also be observed for the solution energies.

Fig. 2 (a)–(d) Four stable configurations for an individual H atom near to V_Be2. (e) and (f) Two stable configurations for an individual He atom near to V_Be2. The orange, blue, red, green and pink spheres denote Ti, Be2, Be3, He and H atoms, respectively. The blue circles represent V_Be2.

Table 2 Solution and binding energies of individual H/He atoms at the different stable sites near to V_Be2 in bulk Be₁₂Ti

Configuration	Distance (Å)	Esol (eV)	Ebind (eV)
HS1	0.765	0.074	−0.435
HS2	0.964	−0.079	−0.588
HS3	1.032	−0.160	−0.670
HS4	1.375	0.298	−0.212
HeS1	0.728	1.898	−2.130
HeS2	0.755	2.236	−1.792

---

3.2 Nucleation mechanism of a He bubble in the most stable monovacancy

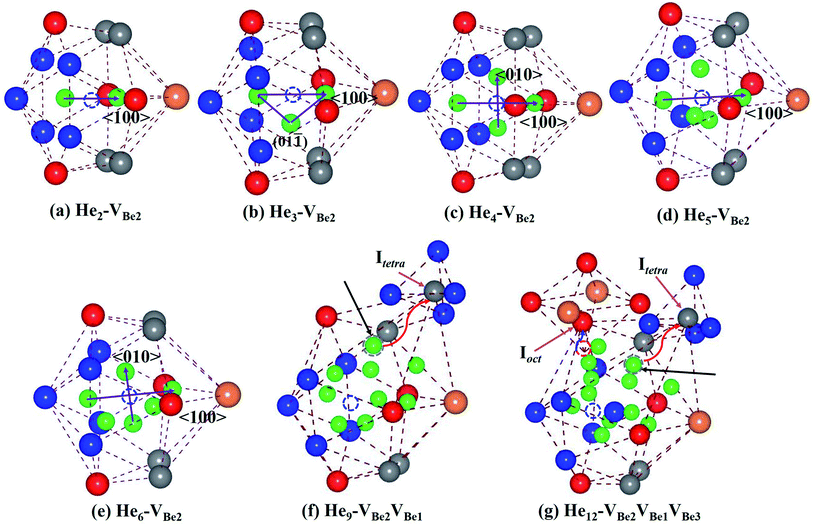
It has been revealed that monovacancy V_Be2 is preferentially formed in bulk Be₁₂Ti. It is important to get insight into the nucleation mechanism of a He bubble in V_Be2. Thus, the nucleation process of He_n–V_Be2 complexes (n is the number of He atoms, n ≥ 1) has been investigated in the following paragraph.

Considering the low He concentration, He atoms are placed near to V_Be2 one by one, that is, the “sequential way”⁴³ has been adopted to simulate the nucleation of the He bubble; this has also been applied in many investigations related to the nucleation of He or H bubbles.^44,45 The solution energies, trapping energies and deformation energies are summarised in Table 3. For the simplest He–V_Be2 complex, the most stable configuration has been obtained, as shown in Fig. 2(e). When an extra He atom is trapped by a He–V_Be2 complex, the He₂–V_Be2 complex has the configuration of a dumbbell with a trapping energy of −1.525 eV, which exactly distributes along the direction of 〈100〉, as shown in Fig. 3(a). The distance between two He atoms is 1.611 Å, and V_Be2 is located in the middle of the dumbbell. When the third He atom is implanted into the He₂–V_Be2 complex, the above mentioned dumbbell configuration is also observed, but the distance between the two He atoms increases to 1.976 Å. The distances between the third He atom and the other two He atoms are 1.499 Å and 1.524 Å. Thus, the He₃–V_Be2 complex has the approximate shape of an isosceles triangle located in the plane of (011̄), which is shown in Fig. 3(b). The trapping energy of the He atoms is −0.713 eV. In the He₄–V_Be2 complex with a trapping energy of −1.019 eV, as shown in Fig. 3(c), besides the dumbbell distributing along the direction of 〈100〉, another dumbbell configuration is also observed, which distributes along the direction of 〈010〉. In the most stable configuration of the He₅–V_Be2 complex with a trapping energy of −0.764 eV, the initial dumbbell slightly deviates from its original direction of 〈100〉. Whereas the dumbbell with a direction of 〈010〉 disappears, as shown in Fig. 3(d). In the configuration of the He₆–V_Be2 complex, there are also two dumbbells: one approximately distributes along the direction of 〈100〉, and the other is along the direction of 〈011̄〉, as shown in Fig. 3(e). The trapping energy of the He atoms is −0.851 eV. In the most stable configuration of the He₇–V_Be2 complex, dumbbells similar to those in the He₄–V_Be2 complex are also obtained. The trapping energy for the He atoms is −0.908 eV. When one new He atom is added into the He₇–V_Be2 complex, the two dumbbells deviate from their original directions. The trapping energy of the He atoms is −0.836 eV. The deformation of the lattice becomes more obvious, where some of Be atoms near the He₈–V_Be2 complex begin to deviate from their lattice sites due to the repulsion of He atoms.

Table 3 Solution energies, trapping energies and deformation energies of He_n–V_Be2 complexes (n ≤ 8), He_n–V_Be2V_Be1 complexes (n = 9, 10, and 11) and the He₁₂–V_Be2V_Be1V_Be3 complex

Configuration	Esol (eV)	Etrap (eV)	Edef (eV)
He1–VBe2	1.898	−2.130	0.191
He2–VBe2	4.402	−1.525	0.525
He3–VBe2	7.717	−0.713	1.458
He4–VBe2	10.725	−1.019	2.504
He5–VBe2	13.989	−0.764	3.602
He6–VBe2	17.167	−0.851	4.908
He7–VBe2	20.287	−0.908	6.207
He8–VBe2	23.479	−0.836	7.591
He9–VBe2VBe1	26.040	−1.467	9.631
He10–VBe2VBe1	28.594	−1.475	10.439
He11–VBe2VBe1	31.545	−1.078	11.687
He12–VBe2VBe1VBe3	34.605	−0.968	12.936

---

Fig. 3 The most stable configurations of the He_n–V_Be2 complexes (n = 2, 3, 4, 5 and 6), the He₉–V_Be2V_Be1 complex and the He₁₂–V_Be2V_Be1V_Be3 complex are presented. The orange, grey, blue, red and green spheres denote Ti, Be1, Be2, Be3 and He atoms, respectively. The grey, blue and red circles refer to Be1, Be2 and Be3 vacancies, respectively.

Things become more interesting once the most stable configuration of the He₉–V_Be2 complex has been formed. As we can see from Fig. 3(f), one Be1 atom near V_Be2 is completely pushed out from its lattice site into a tetrahedral interstitial site (I_tetra). The newly produced vacancy, V_Be1, is occupied by one He atom. The trapping energy drops dramatically to −1.467 eV. Next, the He_n–V_Be2V_Be1 complexes (n ≥ 9) will nucleate around V_Be2 and V_Be1. And V_Be1 is occupied by one He atom. In particular, in the He₁₂–V_Be2V_Be1 complex, a similar phenomenon can also be observed that one Be3 atom near to V_Be2 is exactly pushed out from its lattice site. Therefore, a new vacancy, V_Be3, is also induced without the occupation of a He atom. It is inferred that the He_n–V_Be2V_Be1V_Be3 complexes (n ≥ 12) will nucleate around the three vacancies V_Be2, V_Be1 and V_Be3. The critical configurations for the divacancy of the He_n–V_Be2V_Be1 complexes and the trivacany of the He_n–V_Be2V_Be1V_Be3 complexes have been obtained.

The solution and deformation energies increase approximately linearly with the sequential implantation of He atoms in the He_n–V_Be2 complexes (n ≤ 12), as shown in Fig. 4(a) and (b), indicating that the distortion of lattice becomes more serious as more He atoms are embedded into Be₁₂Ti. As we can see from Table 3, in the initial stage of He bubble nucleation, it is difficult for saturation to occur and for a positive trapping energy to be obtained. It is proposed that individual He atoms could bind to V_Be2 and form a He–V_Be2 complex due to the attraction of V_Be2. The subsequent He atoms are trapped by V_Be2 and the He_n–V_Be2 complexes are formed. As more He atoms are implanted, new vacancies can be produced and the capacity for He atoms can be enhanced. Thus, more He atoms can be trapped by vacancies, forming the He_n–V_i complexes (i represents the number of vacancies). Finally, He bubbles could nucleate and grow gradually.

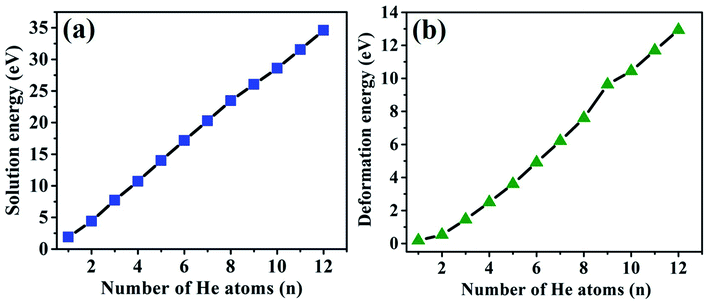Fig. 4 The relationship of solution energies and deformation energies of the He_n–V_Be2 (n ≤ 8), He_n–V_Be2V_Be1 (n = 9, 10, and 11) and He₁₂–V_Be2V_Be1V_Be3 complexes with the number of implanted He atoms.

3.3 Influence of the presence of H atoms on the nucleation of He bubbles and the trapping ability of monovacancies to H and He atoms

To explore the influence of the presence of H atoms on the nucleation of He bubbles, the capture behavior of H atoms in the He_n–V_Be2 complexes (n ≤ 6), i.e., the synergistic effect of the H and He atoms, has been exhaustively investigated in the following paragraph. The solution energies, trapping energies, deformation energies and parts of the most stable configurations of the He_n–V_Be2–H_m complexes (m is the number of H atoms) have been shown in Table 4 and Fig. 5, respectively.

Table 4 Solution energies, trapping energies and deformation energies for the He_n–V_Be2–H_m (n ≤ 6, m ≤ 6) complexes

Configuration	Esol (eV)	Etrap (eV)	Edef (eV)
He1–VBe2–H1	1.827	−0.580	0.261
He1–VBe2–H2	1.933	−0.403	0.362
He1–VBe2–H3	2.352	−0.091	0.568
He1–VBe2–H4	2.840	−0.021	0.727
He1–VBe2–H5	3.401	0.053	1.131
He2–VBe2–H1	4.711	−0.200	0.720
He2–VBe2–H2	5.053	−0.168	0.891
He2–VBe2–H3	5.540	−0.022	1.122
He2–VBe2–H4	6.044	−0.005	1.345
He2–VBe2–H5	6.637	0.084	1.670
He3–VBe2–H1	7.973	−0.253	1.712
He3–VBe2–H2	8.253	−0.230	1.904
He3–VBe2–H3	8.662	−0.100	2.174
He3–VBe2–H4	9.144	−0.026	2.549
He3–VBe2–H5	9.704	0.051	2.841
He4–VBe2–H1	10.960	−0.274	2.816
He4–VBe2–H2	11.233	−0.237	3.068
He4–VBe2–H3	11.670	−0.073	3.427
He4–VBe2–H4	12.181	0.002	3.814
He5–VBe2–H1	14.211	−0.287	3.902
He5–VBe2–H2	14.501	−0.219	4.267
He5–VBe2–H3	14.902	−0.109	4.678
He5–VBe2–H4	15.373	−0.038	4.955
He5–VBe2–H5	15.929	0.047	5.432
He6–VBe2–H1	17.406	−0.270	5.218
He6–VBe2–H2	17.742	−0.173	5.651
He6–VBe2–H3	18.199	−0.052	5.987
He6–VBe2–H4	18.674	−0.034	6.343
He6–VBe2–H5	19.176	−0.007	7.164
He6–VBe2–H6	19.807	0.122	7.390

---

Fig. 5 Parts of the most stable configurations of the He_n–V_Be2–H_m complexes (n ≤ 6). The orange, grey, blue, red, green and pink spheres denote Ti, Be1, Be2, Be3, He, and H atoms, respectively. The blue circles refer to V_Be2.

As we can see from Fig. 5, in the He₁–V_Be2–H₁ complex, the distance between the H atom and He atom is 1.733 Å. In the most stable configuration of the He₁–V_Be2–H₂ complex, the two H atoms, the He atom and V_Be2 are located in the same plane of (031). The two H atoms and the He atom form the structure of an isosceles triangle, where the distances between the He atom and each H atom are 1.773 Å. In the configuration of the He₁–V_Be2–H₄ complex, there are two H atoms distributed along the direction of 〈001〉. When another H atom is trapped by the He₁–V_Be2–H₄ complex, the trapping energy becomes positive, implying that the He₁–V_Be2 complex could accommodate four H atoms at most.

In the He₂–V_Be2–H₁ complex, the above mentioned dumbbell distributing along the direction of 〈100〉 still exists. However, the distance between two He atoms is 1.648 Å, which is larger than that in the He₂–V_Be2 complex (1.611 Å). In the He₂–V_Be2–H₂ complex, two H atoms also distribute along the direction of 〈001〉. Besides, two He atoms and two H atoms are located in the same plane of (010). In the He₂–V_Be2–H₄ complex, one new dumbbell structure along the direction of 〈010〉, which is composed of two H atoms and one V_Be2, could obviously be observed. Meanwhile, four H atoms and two He atoms form the structure of an octahedron. The maximal trapping ability of the He₂–V_Be2 complex for H atoms is four.

The dumbbell distributing along the direction of 〈100〉 has been also observed in the He₃–V_Be2–H₁ complex. Four atoms (one H atom and three He atoms) form the structure of a tetrahedron. In the He₃–V_Be2–H₂ complex, two H atoms also distribute along the direction of 〈001〉. In the configuration of the He₃–V_Be2–H₃ complex, three H atoms and three He atoms form the structure of an octahedron. It is found that the He₃–V_Be2 complex could also trap four H atoms.

The He₄–V_Be2 complex could trap three H atoms at most. The two dumbbells observed in the He₄–V_Be2 complex still exist. The structure of an octahedron, which is composed of two H atoms and four He atoms in the He₄–V_Be2–H₂ complex, has been obviously observed.

In the He₅–V_Be2–H₂ complex, two H atoms are no longer along the direction of 〈001〉. In the He₅–V_Be2–H₃ complex, the dumbbell structure along the direction of 〈100〉 disappears. In the He₅–V_Be2–H₄ complex, four H atoms form the structure of a tetrahedron. The He₅–V_Be2 complex could also trap four H atoms. However, the He₆–V_Be2 complex could trap up to five H atoms. Interestingly, in all the He_n–V_Be2–H_m complexes (n ≤ 6, m ≤ 6), the He atoms are located in the core surrounding V_Be2, while the H atoms are distributed on the surface of the core, forming a shell. This phenomenon has also been observed in W¹⁹ and Fe.⁴⁶

It can be seen from Fig. 6(a) and (b) that the solution energies and deformation energies of the He and H atoms in the He_n–V_Be2–H_m complexes (n ≤ 6, m ≤ 6) approximately linearly increase with the increase in the number of implanted He and H atoms, meaning that the degree of deformation increases with the sequential implantation of He and H atoms. The largest deformation will be induced by the He₆–V_Be2–H_m complexes. As we can see from Fig. 6(c), the He₂–V_Be2–H_m complexes have the maximal trapping energy. Besides, the trapping energy of all configurations will become positive when more than four H atoms are implanted around the He_n–V_Be2 complexes. It is inferred that, in terms of their ability to trap H atoms, the He_n–V_Be2 complexes will be saturated. The positive trapping energy means that it is difficult for the He_n–V_Be2 complexes to trap H atoms, and the retention of H atoms will occur far away from the He_n–V_Be2–H_m complexes. Besides, throughout the simulation of nucleation of He_n–V_Be2–H_m complexes, no molecular H₂ has been observed. The distances between any arbitrary two H atoms are larger than the bond length of molecular H₂, 0.74 Å. Furthermore, it has also shown that the charge density between an arbitrary two H atoms is very low. The results indicate that the interaction between the arbitrary two H atoms is very weak, and the bond of molecular H₂ cannot form. For example, in the He₆–V_Be2–H₅ system, the minimal distance between two H atoms is 2.318 Å, which is larger than the bond length of molecular H₂. On the other hand, as one can see from Fig. 7, the charge density between the two H atoms with a minimal distance between them of 2.318 Å is very low, which further indicates that no molecular H₂ has formed.

Fig. 6 Relationship of (a) solution energies, (b) deformation energies and (c) trapping energies of the He_n–V_Be2 complexes (n ≤ 6) with the number of implanted H atoms.

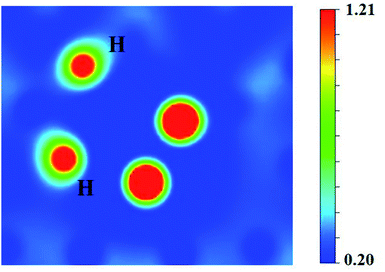Fig. 7 The charge density (e Å⁻³) between the two H atoms separated by a distance of 2.318 Å in the He₆–V_Be2–H₅ system.

To better understand the mechanism for the trapping of H atoms by He_n–V_Be2 complexes, the electronic structures of the He₁–V_Be2–H_m complexes have been calculated. As shown in Fig. 8, the purple curved surfaces represent the isosurface of charge density of 0.13 e Å⁻³. The individual He atom could not fill up the charge density hole. The surface of optimal electron density gradually shrinks as H atoms are sequentially implanted into the He₁–V_Be2 complex. When more than four H atoms are implanted, there is not enough isosurface for H atoms to combine with the complex. The residual H atoms would escape the complex due to the repulsion from other H atoms and will be trapped by other vacancies and complexes.

Fig. 8 Isosurfaces of charge density at the (a) V_Be2, (b) He₁–V_Be2 and (c)–(f) He₁–V_Be2–H_m complexes (m = 1, 2, 3, and 4). The orange, grey, blue, red, green and pink spheres denote Ti, Be1, Be2, Be3, He, and H atoms, respectively. The purple curved surfaces represent the isosurface of charge density of 0.13 e Å⁻³.

It has been firmly confirmed that beryllides are superior to pure Be in irradiation resistance.^9,10 Therefore, alloying is an effective method not only for inhibiting the irradiation damage, but also improving the mechanical and thermodynamic properties of materials. Ternary or more complex beryllides may be more excellent as neutron multiplier materials. Besides, in experiment, researchers could develop new techniques to fabricate beryllides which have nanoscale grains. In the materials with small grains, there will be a large fraction of interfaces and grain boundaries,¹⁴ which also act as traps for the segregation and aggregation of transmutation-produced He and H atoms. As such, the concentrations of He and H atoms inside the grain will be relatively reduced. The irradiation induced swelling and damage could be well mitigated.

4 Conclusions

A systematic investigation has been performed to clarify the nucleation mechanism of a He bubble around a Be vacancy in bulk Be₁₂Ti. The influence of the presence of H atoms on the nucleation of the He bubble, i.e., the synergistic effect of He and H atoms, has been further investigated. During the process of He bubble nucleation, dumbbell structures evolve with the number of implanted He atoms and finally disappear. In the He_n–V_Be2 complexes (n ≤ 8), the nucleation of the He bubble is around a V_Be2 monovacancy. It becomes interesting when another He atom is embedded into the He₈–V_Be2 complex. One new vacancy is induced and is occupied by one He atom in the He₉–V_Be2 complex. The subsequent He bubble nucleation is around the divacancy of V_Be2V_Be1. When an extra He atom is implanted into the He₁₁–V_Be2V_Be1 complex, another new vacancy V_Be3 is produced, but without the occupation of a He atom. It is inferred that the nucleation of the He bubble will be around the trivacancy of V_Be2V_Be1V_Be3. It is difficult to get to saturation of trapped He atoms.

In the study of the synergistic effect of He and H atoms, the implantation of H atoms into the He_n–V_Be2 (n ≤ 6) complexes could influence the stability of existing dumbbells. On the other hand, some tetrahedral and octahedral structures are also obtained. The He_n–V_Be2 (n ≤ 6) complexes could trap approximately four H atoms. The residual H atoms could not be accommodated due to the continuous shrinking of the isosurface of charge density, but could be trapped by other vacancies or complexes far away from the He_n–V_Be2–H₄ (n ≤ 6) complex. This simulation study provides a foundation to understand the evolution of the He bubble microstructure and the synergistic effect between He and H atoms in Be₁₂Ti, which is favorable for better understanding the retention of irradiation-induced He and H atoms in neutron multiplying materials. This investigation could be helpful for the design and fabrication of more promising beryllides which could withstand a severe external environment.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work is supported by the National Nature Science Foundation of China (Grant No. 11775102), the Strategic Priority Research Program of Chinese Academy of Sciences (Grant No. XDA21010202) and the Advanced Energy Science and Technology Guangdong Laboratory.

References

1. J. H. Kim and M. Nakamichi, J. Nucl. Mater., 2019, 519, 182–187.
2. D. V. Bachurin and P. V. Vladimirov, Intermetallics, 2018, 100, 163–170.
3. S. C. Middleburgh and R. W. Grimes, Acta Mater., 2011, 59, 7095–7103.
4. P. Vladimirov, C. Ferrero, V. Chakin, P. Kurinskiy, A. Moeslang, R. Pieritz, T. Weitkamp and E. Brun, Acta Mater., 2015, 88, 293–301.
5. V. Chakin, R. Rolli, R. Gaisin, U. H. Kramar, M. Nakamichi and M. Zmitko, Fusion Eng. Des., 2020, 161, 111938.
6. V. Chakin, R. Rolli, A. Moeslang, M. Klimenkov, M. Kolb, P. Vladimirov, P. Kurinskiy, H. C. Schneider, S. van Til, A. J. Magielsen and M. Zmitko, J. Nucl. Mater., 2013, 442, S483–S489.
7. V. Chakin, R. Rolli, A. Moeslang, P. Vladimirov, P. Kurinskiy, S. van Til, A. J. Magielsen and M. Zmitko, Fusion Eng. Des., 2013, 88, 2309–2313.
8. V. Chakin, J. Reimann, A. Moeslang, R. Latypov and A. Obukhov, Prog. Nucl. Energy, 2012, 57, 2–7.
9. K. Tsuchiya, T. Hoshino, H. Kawamura, Y. Mishima, N. Yoshida, T. Terai, S. Tanaka, K. Munakata, S. Kato, M. Uchida, M. Nakamichi, H. Yamada, D. Yamaki and K. Hayashi, Nucl. Fusion, 2007, 47, 1300–1306.
10. Y. Fujii, M. Miyamoto, J. H. Kim, M. Nakamichi, N. Murayoshi and H. Iwakiri, Nucl. Mater. Energy, 2016, 9, 233–236.
11. S. Y. Binyukova, I. I. Chernov, B. A. Kalin and T. Swe, J. Nucl. Mater., 2007, 367–370, 500–504.
12. W. H. Hu, L. P. Guo, J. H. Chen, F. F. Luo, T. C. Li, Y. Y. Ren, J. P. Suo and F. Yang, Fusion Eng. Des., 2014, 89, 324–328.
13. S. J. Leclerc, M. L. Lescoat, F. Fortuna, L. Legras, X. Li and A. Gentils, J. Nucl. Mater., 2015, 466, 646–652.
14. X. Y. Sun, F. D. Chen, H. Huang, J. W. Lin and X. B. Tang, Appl. Surf. Sci., 2019, 467–468, 1134–1139.
15. A. Založnik, M. J. Baldwin, R. P. Doerner, T. S. Selinger and S. Brezinsek, J. Nucl. Mater., 2018, 512, 25–30.
16. I. B. Kupriyanov, G. N. Nikolaev, V. V. Vlasov, A. M. Kovalev and V. P. Chakin, J. Nucl. Mater., 2007, 367–370, 511–515.
17. E. Rabaglino, J. P. Hiernaut, C. Ronchi and F. S. Argentina, J. Nucl. Mater., 2002, 307–311, 1424–1429.
18. E. Abramov and D. Eliezer, J. Mater. Sci., 1992, 27, 2595–2598.
19. N. Juslin and B. D. Wirth, J. Nucl. Mater., 2013, 438, S1221–S1223.
20. W. A. Counts, C. Wolverton and R. Gibala, Acta Mater., 2010, 58, 4730–4741.
21. P. B. Zhang, J. J. Zhao and B. Wen, J. Nucl. Mater., 2012, 429, 216–220.
22. H. F. Zhang, B. D. Yao, L. Q. Shi, D. J. O’Connor, J. Huang, J. Y. Zhang, W. Ding and Y. X. Wang, Acta Mater., 2015, 97, 50–57.
23. Z. C. Meng, C. L. Wang, J. T. Liu, Y. L. Wang, X. L. Zhu, L. Yang and L. Huang, Phys. Chem. Chem. Phys., 2020, 22, 18040–18049.
24. Y. G. Xu, X. J. Bai, X. H. Zha, Q. Huang, J. He, K. Luo, Y. H. Zhou, T. C. Germann, J. S. Francisco and S. Y. Du, J. Chem. Phys., 2015, 143, 114707.
25. J. J. Liu, C. L. Wang, X. L. Zhu, J. T. Liu, X. M. Zhang, X. Q. Gou, W. S. Duan and L. Yang, Phys. Chem. Chem. Phys., 2018, 20, 18766–18774.
26. L. Ferry, F. Virot, M. Barrachin, Y. Ferro, C. Pardanaud, D. Matveev, M. Wensing, T. Dittmar, M. Koppen and C. Linsmeier, Nucl. Mater. Energy, 2017, 12, 453–457.
27. M. G. Ganchenkova and V. A. Borodin, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 75, 054108.
28. P. V. Vladimirov and A. Moeslang, J. Nucl. Mater., 2013, 442, S694–S698.
29. P. B. Zhang, J. J. Zhao and B. Wen, J. Phys.: Condens. Matter, 2012, 24, 095004.
30. X. L. Zhu, C. L. Wang, J. J. Liu, X. M. Zhang, H. Q. Deng, W. S. Duan and L. Yang, RSC Adv., 2018, 8, 35735.
31. X. L. Zhu, C. L. Wang, Z. C. Meng, Y. L. Wang, H. Q. Deng, W. S. Duan and L. Yang, J. Nucl. Mater., 2019, 525, 7–13.
32. G. Kresse and J. Furthmüller, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169–11186.
33. G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15–50.
34. P. E. Blöchl, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953–17979.
35. J. P. Perdew and Y. Wang, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 45, 13244.
36. H. J. Monkhorst and J. D. Pack, Phys. Rev. B: Solid State, 1976, 13, 5188–5192.
37. Q. Zhao, Z. Zhang, M. Huang, X. D. Zhang and X. P. Ouyang, Nucl. Instrum. Methods Phys. Res., Sect. B, 2020, 470, 48–55.
38. E. Gillam, H. P. Rooksby and L. D. Brownlee, Acta Crystallogr., 1964, 17, 762–763.
39. M. L. Jackson, P. A. Burr and R. W. Grimes, Acta Crystallogr., Sect. B: Struct. Sci., Cryst. Eng. Mater., 2016, B72, 277–280.
40. A. Zalkin, D. E. Sands, R. G. Bedford and O. H. Krikorian, Acta Crystallogr., 1961, 14, 63–65.
41. M. L. Jackson, P. A. Burr and R. W. Grimes, Nucl. Fusion, 2017, 57, 086049.
42. X. K. Liu, Q. J. Feng, B. Tang, J. Zheng, Z. Zheng, W. Zhou, J. T. Tian and J. Wang, RSC Adv., 2019, 9, 5302.
43. A. Alkhamees, H. B. Zhou, Y. L. Liu, S. Jin, Y. Zhang and G. H. Lu, J. Nucl. Mater., 2013, 437, 6–10.
44. M. A. Tschopp, F. Gao and K. N. Solanki, Acta Mater., 2017, 124, 544–555.
45. W. Counts, C. Wolverton and R. Gibala, Acta Mater., 2011, 59, 5812–5820.
46. E. Hayward and C. Deo, J. Phys.: Condens. Matter, 2012, 24, 265402.

---

Footnote

† Contributed equally to this work.

---