Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Chemical tuning of spin clock transitions in molecular monomers based on nuclear spin-free Ni(II)†
Marcos
Rubín-Osanz
 a,
François
Lambert
b,
Feng
Shao‡
a,
François
Lambert
b,
Feng
Shao‡
 b,
Eric
Rivière
b,
Régis
Guillot
b,
Eric
Rivière
b,
Régis
Guillot
 b,
Nicolas
Suaud
b,
Nicolas
Suaud
 c,
Nathalie
Guihéry
c,
David
Zueco
c,
Nathalie
Guihéry
c,
David
Zueco
 a,
Anne-Laure
Barra
d,
Talal
Mallah
a,
Anne-Laure
Barra
d,
Talal
Mallah
 *b and
Fernando
Luis
*b and
Fernando
Luis
 *a
*a
aInstituto de Nanociencia y Materiales de Aragón, CSIC-Universidad de Zaragoza, 50009 Zaragoza, Spain. E-mail: fluis@unizar.es
bInstitut de Chimie Moléculaire et des Matériaux d'Orsay, CNRS, Université Paris-Saclay, 91405 Orsay Cedex, France. E-mail: talal.mallah@universite-paris-saclay.fr
cLaboratoire de Chimie et Physique Quantiques, Université Paul Sabatier, 31062 Toulouse Cedex 4, France
dLaboratoire National des Champs Magnétiques Intenses, CNRS-Univ. Grenoble-Alpes, 38042 Grenoble Cedex 9, France
First published on 25th February 2021
Abstract
We report the existence of a sizeable quantum tunnelling splitting between the two lowest electronic spin levels of mononuclear Ni complexes. The level anti-crossing, or magnetic “clock transition”, associated with this gap has been directly monitored by heat capacity experiments. The comparison of these results with those obtained for a Co derivative, for which tunnelling is forbidden by symmetry, shows that the clock transition leads to an effective suppression of intermolecular spin–spin interactions. In addition, we show that the quantum tunnelling splitting admits a chemical tuning via the modification of the ligand shell that determines the crystal field and the magnetic anisotropy. These properties are crucial to realize model spin qubits that combine the necessary resilience against decoherence, a proper interfacing with other qubits and with the control circuitry and the ability to initialize them by cooling.
Introduction
Magnetic molecules are attractive candidates to encode spin qubits and qudits.1–6 Each molecule consists of a core of one or several magnetic ions, surrounded by non-magnetic ligands. The interaction of these ions with their local coordination sphere determines the magnetic energy levels and states of the molecule. Tuning the molecular structure and the local coordination of the magnetic ions introduces the possibility of designing systems adapted to specific applications,7–15 and constitutes one of the most appealing characteristic traits of a chemically driven approach.In principle, any magnetic molecule with two well resolved low-lying energy levels can encode a qubit, provided that one can induce transitions between them by means of external stimuli, often resonant electromagnetic pulses. A further condition is that the qubit states are sufficiently robust against any other interaction that can perturb their coherent evolution. In magnetic molecules and at temperatures that are sufficiently low to “freeze” spin-lattice relaxation, decoherence is mainly associated with magnetic noise, arising from either nuclear spins in the ligands or, in not very diluted samples, from the coupling (e.g. dipolar) to other molecules.7,8,16–22 Finally, scaling up beyond a qubit, which is obviously necessary for developing any application of real value, implies connecting several qubits. Therefore, a crucial challenge is how to combine a good isolation from noise with the ability to externally control the molecular spin states and their mutual interactions.
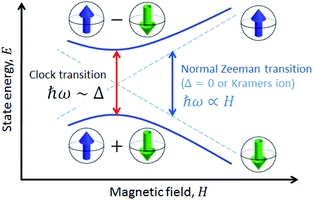
A solution to this problem, borrowed from atomic physics, is to encode the qubit in superposition states that form at level anti-crossings, also known as clock transitions (Fig. 1).23–27 These level anti-crossings arise from quantum tunnelling between opposite orientations of non-Kramers (i.e. integer) spins.28 Clock transitions have a remarkable stability against magnetic field fluctuations, as dipolar decoherence vanishes at first order. Examples found among molecular materials24–26 show that spin coherence times increase sharply near each clock transition. However, the presence of sizeable hyperfine interactions turns them into non-ideal qubit candidates. First, getting to the clock transition requires the application of an external magnetic field and second and, probably more important, the two levels involved in the clock transition might not include the actual ground spin state, thus hindering a simple qubit initialization by cooling.
In this work, we study spin clock transitions in a closer to ideal situation, afforded by molecular [M(Me6tren)Cl](ClO4) monomers, with M = Ni(II) (1) and Co(II) (2).29,30 With its S = 1 ground state and strongly uniaxial anisotropy, (1) provides a realization of the simplest non-Kramer system, with just three magnetic levels (mS = 0 and ±1). In addition, the only isotope with a nonzero nuclear spin (61Ni, I = 3/2) has a close to negligible natural abundance of 1.14%. We probe the magnetic level structure by means of heat capacity experiments. The population of quantized levels gives rise to a characteristic Schottky anomaly in the specific heat.31 This technique is therefore a relatively simple, yet direct, tool to detect small energy splittings, in the range of 0.1 K to 8 K.32,33 In addition, it allows following its dependence on magnetic field, which is one of the characteristic traits of a clock transition. In complex 2, isostructural to 1, Ni(II) is replaced by Co(II), a Kramers ion (S = 3/2) for which quantum tunnelling is forbidden by time-reversal symmetry.28 This allows us to compare both situations and experimentally probe how clock transitions affect spin–spin intermolecular interactions in the same crystal structure.
Finally, we have also studied [Ni(2-Imdipa)(NCS)](NCS) (4), a new complex with octahedral geometry close to the strict Oh symmetry that has a correspondingly weaker magnetic anisotropy. This system helps us to show that the quantum tunnelling gap Δ can be chemically tuned to make it compatible with the microwave circuits that are regarded as promising quantum computation platforms.34–36
Experimental methods
Synthesis and structures
[M(Me6tren)Cl](ClO4) with M = Ni (1), Co (2) and Zn (3); [Ni(2-Imdipa)(NCS)](NCS) (4). Molecules 1, 2 and 3 are isostructural and have been previously reported and studied,29,30 while 4 is a new complex designed and reported here as a demonstration of chemical tuning of the tunnelling gap of 1. The details of its synthesis and crystal structure are given in the accompanying ESI.† Solid solutions of, nominally, 10% 1 and 10% 2 into the diamagnetic 3 have also been prepared. They are denoted by 1d9.5% and 2d11%, respectively. The actual concentrations were determined by comparing magnetization isotherms measured on the pure and diluted samples under identical conditions (Fig. S1 of the ESI†).Heat capacity experiments
The specific heat of all complexes was measured, between T = 0.35 K and 20 K (100 K for 3), with a commercial physical property measurement system (PPMS-Quantum Design) that makes use of a relaxation method.32,33 Powder samples of 1, 2 and 3 were mixed with apiezon N grease to improve the thermal contact with the calorimeter. The powder samples were pressed into a small pellet, in the form of a thin disk which was then placed in contact with the calorimeter platform. We find that the crystallites that form the powder have a geometrical tendency to pack in some orientations under pressure. The crystal axes are therefore not randomly oriented but show some preferential orientation in these samples (see Fig. S2† for an estimate). In the case of complex 4, experiments were performed on single crystals placed onto a thin layer of apiezon N grease.Magnetic characterization
Magnetization data of 4 were measured, in sintered powder form as well as on single crystals, with a commercial Magnetic Properties Measurement System (MPMS-Quantum Design), a magnetometer based on a SQUID sensor, between 1.8 K and 300 K, magnetic fields up to 5 T and for different crystal orientations. The magnetic ac susceptibility of 1 (in powder form) and 4 (on a single crystal and on a powdered sample obtained by crushing the same crystal) was measured using the AC measuring options of the same SQUID magnetometer (for frequencies between 1 Hz and 1.4 kHz) and of the PPMS system (for frequencies between 100 Hz and 10 kHz).EPR experiments
High-frequency EPR spectra of a single crystal of 1 were recorded on a multifrequency spectrometer operating in a double-pass configuration. A 110 GHz frequency source (Virginia Diodes Inc.) was used. The power of the frequency source was varied, either with an external attenuator or with internal one. The emitted power was measured independently by a powermeter. The exciting light was propagated with a Quasi-Optical bridge (Thomas Keating) outside the cryostat, working in induction mode which provides an efficient isolation of the detector, and with a corrugated waveguide inside it. The detection was carried out with a hot electron InSb bolometer (QMC Instruments). The main magnetic field was supplied by a 16 T superconducting magnet associated with a variable temperature insert (Cryogenic).Ab initio calculations
Ab initio calculations were performed using the ORCA 4.0.1.2 quantum chemistry package.37 The D and E parameters of the new complex 4 were evaluated following the procedure already developed by some of us.38 State average CASSCF (Complete Active Space Self Consistent Field) calculations were first performed to account for non-dynamic correlations; then, dynamical correlations were accounted for using the NEVPT2 method in its strongly contracted scheme.39–41 Finally, spin–orbit (SO) couplings were calculated using the Spin Orbit State Interaction (SI–SI) method implemented in ORCA.42 The Complete Active Space (CAS) is composed of the five mainly-3d orbitals of the Ni ion and the 8 associated electrons, i.e. CAS(8,5). The averaged CASSCF molecular orbitals optimization was done over the 10 triplet and 15 singlet spin states generated by the CAS(8,5). The SO coupling was considered between all the mS components of these states, the spin-free energy (diagonal elements of the SO matrix) being evaluated at the NEVPT2 level.The DKH-def2-QZVPP basis sets were used for Ni (14s10p5d4f2g), for S atoms (11s7p4d2f1g), for N atoms (8s4p3d2f1g) belonging to the coordination sphere of Ni and for the C atoms (8s4p3d2f1g) of NCS−, while for other N and C atoms the DKH-def2-TZVP (6s3p2d1f) basis was used and a def2-SVP (2s1p) basis for H atoms. For DFT calculations, df2-SVP atomic basis sets were used for Ni (5s3p2d1f), for C and N atoms (3s2p1d), for S (4s3p1d) and for H (2s1p).
Results and discussion
Direct detection of spin clock transitions by heat capacity experiments
Fig. 2 shows the specific heat c/R of 1 measured at zero magnetic field. Above approximately 8 K, it is dominated by the contribution arising from lattice vibrations. This contribution has been determined independently from the specific heat of the diamagnetic complex 3, which is also shown in Fig. 2. At low and very low temperatures the specific heat of 1 shows an additional Schottky-like anomaly, with a maximum centred at T0 = 1.75 K.This anomaly signals the existence of a finite gap in the spectrum of magnetic energy levels of this complex.32,33 Since 98.86% of the stable Ni isotopes carry no nuclear spin, the anomaly must be associated with thermal excitations of the molecular electronic spins. In order to gain further information on its nature, we have measured a sample (1d9.5%) that contains 9.5% of 1 diluted into the diamagnetic complex 3. Despite the expected decrease in the strength of intermolecular magnetic interactions, these data show the same Schottky anomaly, even shifted to slightly higher temperatures (see also Fig. S3†). Therefore, we can safely discard that spin–spin interactions originate the Schottky-like anomaly observed in 1 and conclude that it is due to a zero-field splitting (ZFS) intrinsic to each individual molecule.
These results can be easily understood as follows. We show in Fig. 2 the specific heat of 1 (solid lines) and the energy levels (inset) calculated by using the spin Hamiltonian reported in ref. 29:
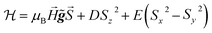 | (1) |
![[g with combining tilde]](https://www.rsc.org/images/entities/b_i_char_0067_0303.gif) is the gyromagnetic tensor that determines the Zeeman interaction with the magnetic field
is the gyromagnetic tensor that determines the Zeeman interaction with the magnetic field 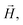 and D and E are the diagonal (or uniaxial) and off-diagonal (rhombic) magnetic anisotropy constants (ZFS parameters), respectively. Because of its very strong uniaxial anisotropy, the magnetic energy level spectrum of 1 shows a highly excited mS = 0 level above the ground mS = ±1 doublet. The Schottky anomaly associated with the population of the former level is expected to show up above 40 K, thus it is completely masked by the much higher lattice contribution and, therefore, experimentally undetectable. To all practical purposes, the behaviour of 1 at low temperatures reduces to that of a text book two-level system.31 The gap observed experimentally must then arise from the weaker off-diagonal anisotropy term E(Sx2 − Sy2). This term induces tunnelling between the mS = ±1 sub-levels and gives rise to a quantum tunnelling gap Δ = 2E and to a Schottky-like specific heat contribution, as shown in Fig. 2.
and D and E are the diagonal (or uniaxial) and off-diagonal (rhombic) magnetic anisotropy constants (ZFS parameters), respectively. Because of its very strong uniaxial anisotropy, the magnetic energy level spectrum of 1 shows a highly excited mS = 0 level above the ground mS = ±1 doublet. The Schottky anomaly associated with the population of the former level is expected to show up above 40 K, thus it is completely masked by the much higher lattice contribution and, therefore, experimentally undetectable. To all practical purposes, the behaviour of 1 at low temperatures reduces to that of a text book two-level system.31 The gap observed experimentally must then arise from the weaker off-diagonal anisotropy term E(Sx2 − Sy2). This term induces tunnelling between the mS = ±1 sub-levels and gives rise to a quantum tunnelling gap Δ = 2E and to a Schottky-like specific heat contribution, as shown in Fig. 2.
The temperature T0 of the specific heat maximum provides a simple and direct method to determine Δ. At zero field, T0 of a simple two level system is given by31,33
 | (2) |
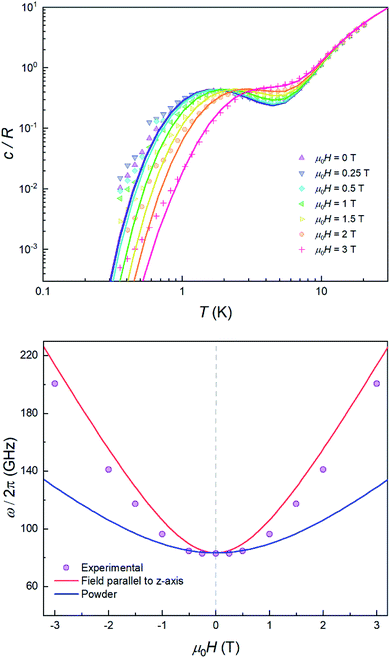
The quantum nature of the observed level splitting is confirmed by its magnetic field dependence. Fig. 3 (top) shows specific heat data of 1 measured for 0 ≤ μ0H ≤ 3 T. The low-T anomaly hardly changes for magnetic fields below 1 T. A weak dependence on magnetic field is precisely the behaviour expected for the energy gap ℏω near a spin clock transition. Near the anti-crossing, ℏω is approximately given by
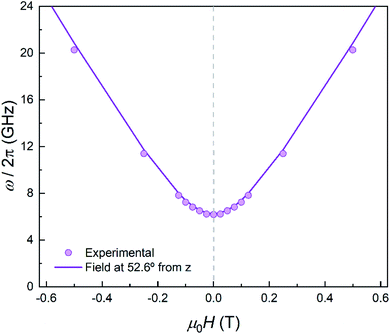
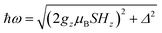 | (3) |
The temperature T0 of the specific heat maximum can then be calculated by simply replacing Δ in eqn (2) by this field-dependent ℏω. It then follows that the magnetic field has little influence on the specific heat provided that 2gzμBSHz ≪ Δ, as observed experimentally. The parabolic dependence of the quantum level splitting predicted by eqn (3) can be directly monitored by plotting the experimental T0 as a function of H. The data nicely follow this dependence, as shown by the bottom panel of Fig. 3. Yet, the experimental shift of the heat capacity anomaly is larger than what one would expect for a randomly oriented sample, suggesting that the polycrystalline sample has a preferred orientation, as expected (see methods). The orientation that best accounts for the heat capacity data is a “mix” between that of a random powder and a fully oriented sample with z parallel to the magnetic field (cf.Fig. 3 and S2†).
Comparison with a Kramers spin system: effect of spin clock transitions on spin–spin interactions
This section describes a realization, within the same molecular system, of a spin with a conventional level crossing (cf.Fig. 1), thus providing a nice comparison with the “quantum limit” that complex 1 represents. For this purpose we study the Co(II) containing complex 2. Whereas Ni(II) has an integer spin S = 1, Co(II) possesses an S = 3/2 ground state. Therefore, it is a Kramers ion. Quantum tunnelling of the electronic spin is then strictly forbidden on account of time-reversal symmetry.28 We expect to see profound differences in the physical behaviour of these two derivatives. As it is shown in the following, these differences manifest themselves neatly in the heat capacity.Fig. 4 shows the specific heat of 2 measured at zero field. The magnetic anisotropy of 2 is much weaker than that of 1.30 For this reason, in this case the anomaly associated with the ZFS between the mS = ±1/2 and mS = ±3/2 level doublets (see the inset of Fig. 4) shows up at lower temperatures and can be observed as an extra contribution to c/R over the lattice contribution. The experimental results agree with simulations performed using the spin Hamiltonian in eqn (1) with D = −8.31 cm−1 and E ≈ 0, which result in an overall ZFS of 2D. These values are in almost perfect agreement with D = −8.12 cm−1 and E = 0 determined from high-field EPR.30
The additional contribution to c/R that is observed below 1 K must therefore arise from the splitting of the ground mS = ±3/2 doublet. This contribution can, in principle, be associated with either hyperfine interactions (Co only stable isotope has I = 7/2 nuclear spin) and/or with spin–spin couplings between different molecules in the crystal. However, as it is shown in Fig. S4,† the former are expected to be too weak to account for the specific heat measured below 1 K. In order to confirm this, we have measured the heat capacity of a magnetically diluted sample 2d11% of 2 in the diamagnetic derivative 3. The low-T contribution observed for the pure compound 2 is greatly suppressed in 2d11%, i.e. not just rescaled by concentration, and virtually disappears. This result confirms that isolated molecules of 2 have no electronic gap, as expected, and suggests that the low-T specific heat reflects mainly the effects of intermolecular interactions.
For a more quantitative description, the effects of intermolecular spin–spin interactions have been calculated by performing Monte Carlo simulations on the crystal lattice. We have used the following simple model:
 | (4) |
The sign of J can be further refined by looking at the field dependence of the specific heat. Data measured on pure and magnetically diluted samples of 2 are shown in Fig. 5. The Zeeman interaction splits the mS = ±3/2 levels and competes with the spin–spin interactions. The specific heat then shows a rounded maximum, again characteristic of a two-level system, which progressively shifts towards higher temperatures as H increases. Although, with the proper scaling, data measured on the pure 2 and the magnetically diluted 2d11% samples tend to approach each other as H increases, the Zeeman splitting, as measured from the maximum temperature, remains always larger for the latter. In addition, the magnetic specific heat of 2d11% is closer, at any H, to what is expected for non-interacting molecules. These results confirm that spin–spin interactions have a predominantly antiferromagnetic character in this lattice, as magnetization hysteresis measured at very low temperatures already suggested.30
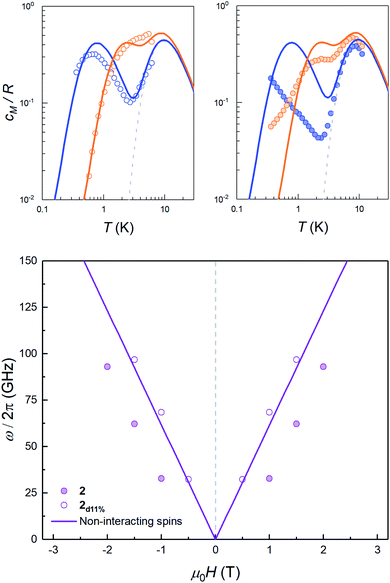 | ||
| Fig. 5 Top: magnetic specific heat cm of 2d11% (open dots, left panel) and of 2 (solid dots, right panel) at selected fields μ0H = 0.5 T (blue) and 1.5 T (orange) obtained after subtraction of the lattice contribution estimated for the diamagnetic compound 3. In both cases, cm is normalised per mole of magnetic molecules. The dashed line shows the zero field splitting contribution (associated with the 2|D| gap in the inset of Fig. 4), while solid lines show the simulation of the expected heat capacity for non-interacting molecular spins (ZFS + Zeeman interaction). Bottom: experimental energy gap ℏω = kBT0/0.42, with T0 the field-dependent temperature of the heat capacity maximum. The solid lines show the Zeeman level crossing expected for non-interacting molecules with the magnetic field applied along the z molecular axis. | ||
The results described in this and the previous section allow gaining a deeper insight on how a sizeable quantum tunnelling gap affects spin–spin interactions. Complex 2 illustrates the conventional, or classical, behaviour (cf.Fig. 4): interactions between spins in an ordered lattice break the symmetry between spin-up and spin-down states and lead to a transition towards a long-range ordered phase at sufficiently low temperatures. By contrast, and in spite of having the same crystal lattice, the specific heat of complex 1 (cf.Fig. 2) does not show, below 0.5 K, any signature of spin–spin interactions. The close to perfect scaling with spin concentration, and the dependence on magnetic field (cf.Fig. 3) suggest, instead, that these molecules behave as quasi-isolated.
In order to illustrate the essential ingredients that govern this “quantum decoupling”, we have numerically diagonalized the spin Hamiltonian 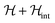 , where
, where 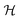 and
and 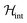 are given by eqn (1) and (4), respectively, for an affordable lattice comprising just one S = 1 spin and its six nearest neighbours located within the same crystallographic plane. Details of these “toy quantum model” calculations are given in Fig. S6.† The results reflect how quantum fluctuations influence the ground state of 1. At zero field, the wave function becomes a symmetric superposition of spin-up and spin-down states whenever the quantum tunnelling gap Δ exceeds the characteristic energy scale ε ≈ Z|J|S2/2 of spin–spin interactions. If we estimate ε of 1 by taking the same J that was determined for complex 2 and S = 1, it follows that Δ ≈ 10ε for this system. The condensation into the ground singlet state then largely suppresses intermolecular magnetic interactions.
are given by eqn (1) and (4), respectively, for an affordable lattice comprising just one S = 1 spin and its six nearest neighbours located within the same crystallographic plane. Details of these “toy quantum model” calculations are given in Fig. S6.† The results reflect how quantum fluctuations influence the ground state of 1. At zero field, the wave function becomes a symmetric superposition of spin-up and spin-down states whenever the quantum tunnelling gap Δ exceeds the characteristic energy scale ε ≈ Z|J|S2/2 of spin–spin interactions. If we estimate ε of 1 by taking the same J that was determined for complex 2 and S = 1, it follows that Δ ≈ 10ε for this system. The condensation into the ground singlet state then largely suppresses intermolecular magnetic interactions.
Chemical tuning of spin clock transitions
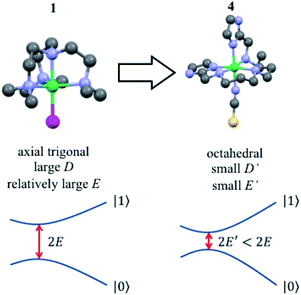
For 1, the tunnel splitting is due to the Jahn–Teller effect that lifts the orbital degeneracy of the Ni(II) electronic state. This effect distorts the trigonal plane of the complex and gives rise to the appearance of a large rhombic parameter (E = 40 GHz), as demonstrated above. A relatively straightforward strategy to drastically reduce the quantum tunnelling splitting (2E) is to globally reduce the axial ZFS parameter D, so that the |E| parameter will be restricted to an upper limit of (1/3)|D|, assuming of course that the two molecules at hand have a similar rhombicity |E/D|.
An efficient approach to achieve small |D| values, in comparison to that of complex 1, is to consider a Ni(II) complex with an octahedral geometry, as close as possible to Oh symmetry but not strictly Oh. Indeed, for Oh symmetry, the three components of the orbital moment (Lx, Ly and Lz) belong to the same irreducible representation T1g of Oh. The effect of SOC will then be the same in the three axes and the three-fold degeneracy of the ground state (3A2g) cannot be lifted (the three mS sub-levels 0 and ±1 would have the same energy). However, if the complex is slightly distorted the effect of SOC will be slightly different along each direction of space, resulting in a very low value of |D|.28,43
With this idea in mind, we prepared several octahedral Ni(II) complexes using a pentadentate organic ligand (2-Imdipa, Fig. 6 and S7–S9†) and different axial ones (NCS−, Cl−, NO3−). It turned out that the hexacoordinate complex bearing NCS− as axial ligand of formula [Ni(2-Imdipa)(NCS)](NCS) (complex 4) has all the characteristics required as summarized in Fig. 7, i.e. a similar rhombicity (|E/D|) but an axial parameter D that is nearly two orders of magnitude smaller (see below for details).
The preparation and full characterization of the organic ligand and complex 4 are detailed in the ESI.† The compound crystallizes in the space group P21/n (group 14, see Table S1†). There are two crystallographically independent molecules (Fig. S7†) that mainly differ by about 1° tilts in their relative orientations, and that have almost the same coordination sphere (Fig. S8†). We will, therefore, focus on one of them.
The geometry around Ni is a distorted octahedron (Fig. S9†). The three amine nitrogen atoms (N2, N3 and N4) and N1 belonging to one of the imidazolate groups of the pentadentate ligand 2-Imdipa lie almost in one plane (equatorial). The nitrogen atom of the other imidazolate group (N5) and that of NCS− (N6) occupy the apical positions. The Ni–N bond distances in the equatorial planes are all larger (average value 2.137 Å, see Table S2†) than the axial ones (2.060 Å). The axial bond distances are identical within experimental errors while those in the equatorial plane differ by less than 0.087 Å (Table S2†).
Because the structure does not show any intermolecular path for strong antiferromagnetic couplings, this small decrease can tentatively be attributed to the ZFS of the S = 1 state. Magnetization isotherms were measured at T = 2, 4 and 6 K.
When plotted against μ0H/T (Fig. S11†), these magnetization curves are not superimposable, which again confirms the presence of a net ZFS within the S = 1 ground state. Fixing the isotropic g factor to 2.16, as derived from χT data, it is possible to achieve very high quality fits of the data for negative and positive D values and a TIP equal to 10−4, as expected for Ni(II) octahedral complexes. The fit parameters are: D = +2.11 cm−1 and |E| = 0.09 cm−1 (|E/D| = 0.04) when starting the fit with a positive D-value and D = −2.96 cm−1 and |E| = 0.06 cm−1 (|E/D| = 0.02) when starting from a negative D value. These data show that, as expected for a Ni(II) complex with an octahedral geometry, the axial ZFS parameter (D) is very small and the rhombic parameter (E) that defines the tunnel splitting (2E) is also very small. However, magnetization data on a powder sample cannot discriminate between positive D (mS = 0 ground level) or negative D (mS = ±1 ground levels).
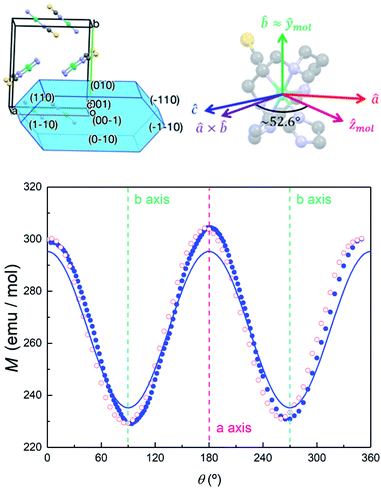
In order to further elucidate the magnetic anisotropy of complex 4, we carried out heat capacity and magnetization studies on a single crystal that has the form of a hexagonal prism (Fig. S12†). The specific heat measured for  along the perpendicular to the hexagonal faces, within the ac plane, is shown in Fig. 8. This quantity reflects the thermal population of the spin levels, thus it critically depends on the sign of D. As Fig. S13† shows, the results are incompatible with a positive D, and agree well with a uniaxial anisotropy (D < 0). The fits of these data and of those obtained by applying the magnetic field along b (see the ESI† for symmetry considerations used in these fits and Fig. S13† for the results) suggest that the easy magnetization axis z lies on the ac plane at 52.6° from the perpendicular to the ab plane as shown in Fig. 9. Besides, the Schottky anomaly centred near 2 K, which corresponds to the thermal excitation of the mS = 0 state, evidences that 4 has a weaker magnetic anisotropy than 1, and gives D ≈ −2.71 cm−1.
along the perpendicular to the hexagonal faces, within the ac plane, is shown in Fig. 8. This quantity reflects the thermal population of the spin levels, thus it critically depends on the sign of D. As Fig. S13† shows, the results are incompatible with a positive D, and agree well with a uniaxial anisotropy (D < 0). The fits of these data and of those obtained by applying the magnetic field along b (see the ESI† for symmetry considerations used in these fits and Fig. S13† for the results) suggest that the easy magnetization axis z lies on the ac plane at 52.6° from the perpendicular to the ab plane as shown in Fig. 9. Besides, the Schottky anomaly centred near 2 K, which corresponds to the thermal excitation of the mS = 0 state, evidences that 4 has a weaker magnetic anisotropy than 1, and gives D ≈ −2.71 cm−1.
Getting insight of the contribution of each state by analysing the composition of their wave function is possible, as it has recently been done on a Ni(II) complex with an octahedral geometry,44 but is out of the scope of this paper. These results confirm our reasoning that a Ni(II) hexacoordinate complex with octahedral geometry that slightly deviates from Oh symmetry leads to small D value and consequently small E (as |E| < |D|/3). However, because of the very weak deviation from Oh symmetry, it is not possible to predict without ab initio calculations the sign of D that is of major importance for using these systems as qubits.
Spin relaxation
The data shown in Fig. 3 and 10 provide a direct mapping of the anticrossings between the two lowest lying spin levels of 1 and 4. Besides, we have shown that the zero-field eigenstates become quite insensitive to environmental magnetic fields. These two properties, which are characteristic traits of a spin-clock transition, make these molecules promising spin qubit candidates. However, they might not be enough, if the spin relaxation towards thermal equilibrium, parameterized by the spin-lattice relaxation time T1, becomes also very fast. We have addressed this important question experimentally, by combining frequency-dependent ac susceptibility and EPR measurements.Illustrative ac susceptibility data are shown in Fig. S17–S19.† They show a dependence on frequency ω for both 1 and 4, signalling the presence of relatively slow relaxation processes45 (note that the characteristic time scale of these measurements is 1/ω > 16 μs). In the case of 1, however, the data do not allow a quantitative determination of T1 because the maximum of the imaginary susceptibility component χ” (that approximately corresponds to 1/ω = T1) appears to be above our highest attainable frequency. A further limitation arises from the fact that superposition spin states (Fig. 1) have a zero average magnetic moment. As a result, phonon-induced transitions between different eigenstates do not lead to any change in the susceptibility (see Fig. S20†).46 Near the clock transition (<0.1 T for complex 4, < 1 T for complex 1), the linear response becomes dominated by the fully reversible van Vleck susceptibility, which arises from the field-induced modulation of the spin wave functions. In the case of complex 4, we have been able to extract T1 for temperatures below approximately 6 K and magnetic fields above 0.1 T. We have measured both a single crystal and a powder obtained by crushing it and embedding it in apiezon N grease, in order to rule out effects associated with a poor thermal contact between the sample and the sample holder (phonon bottleneck effect47). Representative T1 data are shown in Fig. 11 (for the powder) and Fig. S21† (for the single crystal).
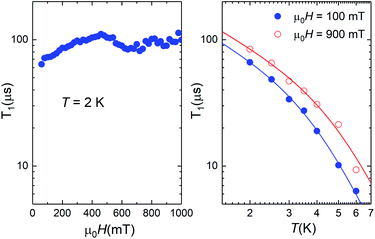 | ||
| Fig. 11 Spin-lattice relaxation time of 4 derived from frequency-dependent ac susceptibility experiments performed on a powdered sample as a function of magnetic field at T = 2 K (left) and at two different magnetic fields as a function of temperature (right). The solid lines are fits that include direct and Raman relaxation processes (cf.eqn (5)). | ||
The results show that T1 approaches 100 μs at T = 2 K. The temperature dependence is compatible with relaxation driven by a combination of Raman and direct processes,48,49 and can be described by the expression
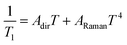 | (5) |
The exponent of the Raman term in eqn (5) has been fixed to the one that corresponds to non Kramers ions when optical phonons play a role,49 as it is likely the case for molecular spins.50 The weak dependence on magnetic field also agrees with a dominant Raman relaxation process.48 However, the direct process might take over at sufficiently strong magnetic fields (when the gap between the ground and first excited levels becomes large enough), as can be seen in the results measured on the single crystal above 0.7–0.8 T (Fig. S21†). This effect could also account for the faster relaxation of complex 1 and underlines, once more, the importance of tuning the quantum tunnelling splitting.
For complex 1, a complementary estimate of T1 has been obtained from high-frequency EPR experiments (Fig. S22†). At T = 2 K, the EPR spectrum shows saturation effects associated with a relatively slow spin-lattice relaxation (Fig. S23†). From the power dependence of the signal intensity the spin-lattice relaxation time T1 can be estimated to be in the order of tens of microseconds, thus in agreement with ac susceptibility results that point to T1 < 100 μs (see ESI† for further details).
Let us compare now the spin-lattice relaxation times with typical quantum operation time scales. At the clock transition, the Rabi frequency for quantum oscillations between the tunnel split states  , where
, where  is the resonant microwave field that must have nonzero component along the anisotropy axis z (otherwise, the transition is forbidden). For a typical bz = 1 mT, this results in qubit operation frequencies of the order of GHz (operation times of order 10−3 μs). Therefore, we can safely conclude that the spin-lattice relaxation will not limit the application of these molecules as spin qubits. The relevant time scale at low temperatures then becomes T2, which will be maximized at the clock transition thanks to its insensitiveness to magnetic field fluctuations.23–26
is the resonant microwave field that must have nonzero component along the anisotropy axis z (otherwise, the transition is forbidden). For a typical bz = 1 mT, this results in qubit operation frequencies of the order of GHz (operation times of order 10−3 μs). Therefore, we can safely conclude that the spin-lattice relaxation will not limit the application of these molecules as spin qubits. The relevant time scale at low temperatures then becomes T2, which will be maximized at the clock transition thanks to its insensitiveness to magnetic field fluctuations.23–26
Conclusions
The results discussed in the previous sections neatly show the existence of a sizeable quantum tunnel splitting Δ in mononuclear Ni(II) complexes. Heat capacity experiments enable a direct detection of this quantum gap and monitoring how it evolves with magnetic field, following the quadratic dependence that characterizes a spin clock transition. The same technique evidences also that the molecular spin qubits effectively decouple from each other at the clock transition if the gap is larger than the typical energy scale of spin–spin interactions. The latter effect is of intrinsic interest for fundamental physics, and can be understood within the framework of the quantum Ising model in a transverse magnetic field.51 This paradigmatic model describes a quantum phase transition. For Δ > Z|J|S2/2, the spin lattice enters a quantum paramagnetic state and stays there, i.e. no transition to long-range order occurs, down to T = 0. A realization of such quantum phase transition has been observed for crystals of Fe8 molecular clusters.52 However, while in this case the transition was driven by an external magnetic field applied perpendicular to the magnetic anisotropy axis, the spins of complex 1 are already in the quantum regime even at zero field on account of its large Δ. These molecules provide then model candidates, with parameters tuneable by chemical means, to investigate the dynamics of pure two-level systems53 with a well-defined quantum ground state, of which very few pure realizations exist in nature.The ability to tune the quantum tunnelling gap via the chemical design of the molecular structure is also of relevance for the development of robust building blocks for scalable quantum technologies. Two obvious reasons, already mentioned, are the possibility to select the most appropriate frequency to optimally interface with the control electronics, even at zero field, and the ability to initialize these qubits by simply cooling them down to very low temperatures. However, there is a more subtle potential advantage that deserves to be mentioned. One of the characteristic, and most appealing, aspects of molecular based spin qubits is the ability to scale up quantum resources within each molecule, e.g. by introducing several spin sites each acting as a qubit. This strategy has given rise to model systems, able to implement basic quantum gates7,11–13 or even quantum error correction algorithms15 at the molecular scale. Decoupling the qubits from each other contributes to enhance the spin coherence. However, it also has a clear downside: a set of N identical uncoupled qubits does not work as an N-qubit processor, as it lacks the possibility of individually addressing each qubit and of performing two-qubit gates. The ability to tune Δ provides both the possibility of making each qubit different (e.g. by having a different resonance frequency) and of activating the spin–spin interactions that are essential to implement conditional operations.54 Molecular structures hosting several Ni(II) centres, which are chemically feasible, could therefore encode multiple addressable qubits while preserving resilience against decoherence. An illustrative example is given in Fig. S24† for the simplest situation of two coupled S = 1 spins.55 Because of their chemical tuneability, the molecular complexes reported here are not just suitable qubits, but proper elements to build more complex quantum functionalities (a kind of “decoherence-free” quantum processor) at the molecular level.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work was supported by funds from the EU (COST Action 15128 MOLSPIN, QUANTERA project SUMO, FET-OPEN grant 862893 FATMOLS), the Spanish MICINN (grants RTI2018-096075-B-C21 and PCI2018-093116) and the Gobierno de Aragón (grant E09-17R-Q-MAD). TM thanks the IUF (Institut Universitaire de France) for financial support.Notes and references
- M. N. Leuenberger and D. Loss, Nature, 2001, 410, 789–793 CrossRef CAS PubMed.
- F. Troiani and M. Affronte, Chem. Soc. Rev., 2011, 40, 3119–3129 RSC.
- G. Aromí, D. Aguilà, P. Gamez, F. Luis and O. Roubeau, Chem. Soc. Rev., 2012, 41, 537–546 RSC.
- E. Moreno-Pineda, C. Godfrin, F. Balestro, W. Wernsdorfer and M. Ruben, Chem. Soc. Rev., 2018, 47, 501–513 RSC.
- A. Gaita-Ariño, F. Luis, S. Hill and E. Coronado, Nat. Chem., 2019, 11, 301–309 CrossRef PubMed.
- M. Atzori and R. Sessoli, J. Am. Chem. Soc., 2019, 141, 11339–11352 CrossRef CAS PubMed.
- F. Luis, et al. , Phys. Rev. Lett., 2011, 107, 117203 CrossRef CAS PubMed.
- C. J. Wedge, et al. , Phys. Rev. Lett., 2012, 108, 107204 CrossRef CAS PubMed.
- M. J. Martínez-Pérez, et al. , Phys. Rev. Lett., 2012, 108, 247213 CrossRef PubMed.
- D. Aguilà, et al. , J. Am. Chem. Soc., 2014, 136, 14215–14222 CrossRef PubMed.
- A. Ardavan, et al. , npj Quantum Inf., 2015, 1, 15012 CrossRef.
- A. Fernández, et al. , Nat. Commun., 2016, 7, 10240 CrossRef PubMed.
- J. Ferrando-Soria, et al. , Nat. Commun., 2016, 7, 11377 CrossRef CAS PubMed.
- M. Atzori, et al. , Chem. Sci., 2018, 9, 6183–6192 RSC.
- E. Macaluso, et al. , Chem. Sci., 2020, 11, 10337–10343 RSC.
- A. Ardavan, et al. , Phys. Rev. Lett., 2007, 98, 057201 CrossRef PubMed.
- A. Morello, P. C. E. Stamp and I. S. Tupitsyn, Phys. Rev. Lett., 2006, 97, 207206 CrossRef PubMed.
- S. Takahashi, et al. , Nature, 2011, 476, 76–79 CrossRef CAS PubMed.
- K. Bader, et al. , Nat. Commun., 2014, 5, 5304 CrossRef CAS PubMed.
- J. M. Zadrozny, J. Niklas, O. G. Poluetkov and D. E. Freedman, ACS Cent. Sci., 2015, 1, 488–492 CrossRef CAS PubMed.
- M. Atzori, et al. , J. Am. Chem. Soc., 2016, 138, 2154–2157 CrossRef CAS PubMed.
- K. S. Pedersen, A.-M. Ariciu, S. McAdams, H. Weihe, J. Bendix, F. Tuna and S. Piligkos, J. Am. Chem. Soc., 2016, 138(18), 5801–5804 CrossRef CAS PubMed.
- G. Wolfowicz, A. M. Tyryshkin, R. E. George, H. Riemann, N. V. Abrosimov, P. Becker, H.-J. Pohl, M. L. W. Thewalt, S. A. Lyon and J. J. L. Morton, Nat. Nanotechnol., 2013, 8, 561–564 CrossRef CAS PubMed.
- M. Shiddiq, D. Komijani, Y. Duan, A. Gaita-Ariño, E. Coronado and S. Hill, Nature, 2016, 531, 348–351 CrossRef CAS PubMed.
- J. M. Zadrozny, A. T. Gallagher, T. D. Harris and D. E. Freedman, J. Am. Chem. Soc., 2017, 139, 7089–7094 CrossRef CAS PubMed.
- C. A. Collett, K.-I. Ellers, N. Russo, K. R. Kittilstved, G. A. Timco, R. E. P. Winpenny and J. R. Friedman, Magnetochemistry, 2019, 5, 4 CrossRef CAS.
- S. Giménez-Santamarina, S. Cardona-Serra, J. M. Clemente-Juan, A. Gaita-Ariño and E. Coronado, Chem. Sci., 2020, 11, 10718–10728 RSC.
- A. Abragam and B. Bleaney, Electron Paramagnetic Resonance of Transition Ions, 1970 Search PubMed.
- R. Ruamps, R. Maurice, L. Batchelor, M. Boggio-Pasqua, R. Guillot, A. L. Barra, J. Liu, E. Bendeif, S. Pillet, S. Hill, T. Mallah and N. Guihéry, J. Am. Chem. Soc., 2013, 135, 3017–3026 CrossRef CAS PubMed.
- R. Ruamps, L. J. Batchelor, R. Guillot, G. Zakhia, A. L. Barra, W. Wernsdorfer, N. Guihéry and T. Mallah, Chem. Sci., 2014, 5, 3418–3424 RSC.
- H. B. Callen, Thermodynamics and an Introduction to Thermostatistics, 1985 Search PubMed.
- F. Luis, F. L. Mettes, J. Tejada, D. Gatteschi and L. J. de Jongh, Phys. Rev. Lett., 2000, 85, 4377–4380 CrossRef CAS PubMed.
- M. Evangelisti, F. Luis, L. J. de Jongh and M. Affronte, J. Mater. Chem., 2006, 16, 2534–2549 RSC.
- R. J. Schoelkopf and S. M. Girvin, Nature, 2008, 451, 664–669 CrossRef CAS PubMed.
- M. D. Jenkins, et al. , Dalton Trans., 2016, 45, 16682–16693 RSC.
- C. Bonizzoni, A. Ghirri, F. Santanni, M. Atzori, L. Sorace, R. Sessoli and M. Affronte, npj Quantum Inf., 2020, 6, 68 CrossRef.
- F. Neese, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2012, 2, 73–78 CAS.
- R. Maurice, R. Bastardis, C. d. Graaf, N. Suaud, T. Mallah and N. Guihéry, J. Chem. Theory Comput., 2009, 5, 2977–2984 CrossRef CAS PubMed.
- C. Angeli, R. Cimiraglia, S. Evangelisti, T. Leininger and J. P. Malrieu, J. Chem. Phys., 2001, 114, 10252 CrossRef CAS.
- C. Angeli, R. Cimiraglia and J. P. Malrieu, Chem. Phys. Lett., 2001, 350, 297–305 CrossRef CAS.
- C. Angeli, R. Cimiraglia and J. P. Malrieu, J. Chem. Phys., 2002, 117, 913 Search PubMed.
- F. Neese, J. Chem. Phys., 2005, 122, 34107 CrossRef PubMed.
- F. E. Mabbs and D. Collison, Electron Paramagnetic Resonance of d Transition Metal Compounds, 1992 Search PubMed.
- N. Suaud, G. Rogez, J. Rebilly, M. Bouammali, N. Guihéry, A. L. Barra and T. Mallah, Appl. Magn. Reson., 2020, 51, 1215–1231 CrossRef.
- K. S. Cole and R. H. Cole, J. Chem. Phys., 1941, 9, 341–352 CrossRef CAS.
- S. Gómez-Coca, A. Urtizberea, E. Cremades, P. J. Alonso, A. Camón, E. Ruiz and F. Luis, Nat. Commun., 2014, 5, 4300 CrossRef PubMed.
- J. H. Van Vleck, Phys. Rev., 1941, 59, 724 CrossRef CAS.
- R. Orbach, Proc. R. Soc. A, 1961, 264, 458 CAS.
- K. N. Shrivastava, Phys. Status Solidi, 1983, 117, 437 CrossRef CAS.
- A. Lunghi, F. Totti, S. Sanvito and R. Sessoli, Chem. Sci., 2017, 8, 6051–6059 RSC.
- R. B. Stinchcombe, J. Phys. C: Solid State Phys., 1973, 6, 2459 CrossRef CAS.
- E. Burzurí, F. Luis, B. Barbara, R. Ballou, E. Ressouche, O. Montero, J. Campo and S. Maegawa, Phys. Rev. Lett., 2011, 107, 097203 CrossRef PubMed.
- A. J. Leggett, S. Chakravarty, A. T. Dorsey, M. P. A. Fisher, A. Garg and W. Zwerger, Rev. Mod. Phys., 1987, 59, 1 CrossRef CAS.
- F. Luis, P. J. Alonso, O. Roubeau, V. Velasco, D. Zueco, D. Aguilà, J. I. Martínez, L. A. Barrios and G. Aromí, Commun. Chem., 2020, 3, 176 CrossRef CAS.
- F. El-Khatib, B. Cahier, M. Lopez-Jorda, R. Guillot, E. Riviere, H. Hafez, Z. Saad, J. J. Girerd, N. Guihery and T. Mallah, Inorg. Chem., 2017, 56, 10655–10663 CrossRef CAS PubMed.
Footnotes |
| † Electronic supplementary information (ESI) available. CCDC 2000847. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/d0sc05856d |
| ‡ Present address: Key Laboratory of Marine Chemistry Theory and Technology, Ministry of Education, College of Chemistry and Chemical Engineering, Ocean University of China, Qingdao 266100, China. |
| This journal is © The Royal Society of Chemistry 2021 |