Clustering of metal dopants in defect sites of graphene-based materials

Abstract

Single-atom catalysts are promising candidates for many industrial reactions. However, making true single-atom catalysts is an experimental dilemma, due to the difficulty of keeping dopant single atoms stable at temperature and under pressure. This difficulty can lead to clustering of the metal dopant atoms in defect sites. However, the electronic and geometric structure of sub-nanoscale clusters in single-atom defects has not yet been explored. Furthermore, recent studies have proven sub-nanoscale clusters of dopants in single-atom defect sites can be equally good or better catalysts than their single-atom counterparts. Here, a comprehensive DFT study is undertaken to determine the geometric and electronic structure effects that influence clustering of noble and p-block dopants in C₃- and N₄-defect sites in graphene-based systems. We find that the defect site is the primary driver in determining clustering dynamics in these systems.

---

Introduction

Atmospheric CO₂ levels are currently the highest that they have been in the past 14 million years.¹ One mitigation strategy is to sequester CO₂ from the atmosphere and convert it into a fuel source by cleaving the C=O bond. However, CO₂ is a particularly stable molecule and therefore, to have any hope of breaking this bond, a catalyst must be employed. Ideally, the catalyst would be simple to manufacture, cheap, stable and both selective and active for the CO₂ reduction reaction (CO₂RR). However, activity and selectivity for the CO₂RR differs depending on the catalyst, pH, electrolyte and overpotential. With all of these demands, engineering a suitable catalyst is a non-trivial task.

Catalysts have been in development for the CO₂RR for many years. The first catalysts to be considered were monometallic catalysts. Of the transition metals, Cu is the most active and selective for the CO₂RR.^2–7 However, while Cu can reduce CO₂, it does so at low efficiency⁸ and requires a high overpotential⁹ rendering Cu non-viable as an industrial catalyst.

Bimetallic catalysts were then designed in an attempt to combine the activity of one metal with the selectivity of another, thus obtaining the best of both worlds. W/Au,¹⁰ Cu/In¹¹ and Cu/Sn¹² catalysts have all been shown to be active for the CO₂RR. However, both mono- and bimetallic transition metal catalysts are fundamentally limited by so-called ‘scaling relations’^13,14 whereby the adsorption energy of key intermediates in the reaction are related and ultimately govern the catalytic activity of the material. As such, new classes of catalysts that are not governed by scaling relations need to be explored.

In 2004, graphene was discovered.¹⁵ Graphene is a two-dimensional (2D) sheet of hexagonally bound C atoms characterised by sp² hybridisation and resembling a honeycomb lattice. Graphene in isolation is inert and unreactive but has excellent electrical conductivity and high surface area.¹⁵ To improve graphene's catalytic activity, both metal and non-metal (e.g. N, B, S) elements can be doped on or into the sheet. However, elements cannot easily be adsorbed to a perfect graphene sheet, due to adsorbates having a high diffusion mobility on the surface under experimental conditions.¹⁶ As such, defect sites are required to limit the mobility of adsorbates on the surface. The two most commonly used sites for doping species into graphitic systems are the graphitic site (hereafter referred to as the C₃-site), and the pyridinic site (hereafter referred to as the N₄-site). A metal atom can then be doped into these defect sites^10,17–22 giving rise to a so-called ‘single-atom’ catalyst. The interaction of the C framework, metal dopants and, in the N₄-sites, the N atoms, give these single-atom catalysts unprecedented properties quite distinct from those of graphene, the defect site or, indeed, the metal dopant.²³ However, how these different features interact with one another to give a material novel properties is not well understood.

Unfortunately, single-atom catalysts are extremely prone to thermal deactivation. Sintering and coalescing can occur under ambient conditions,²⁴ at high temperature and low pressure,²⁵ on a range of different substrate materials²⁶ and a variety of surface coverages.²⁷ There have been many studies that develop methods of maintaining the stability of the single-atom structures^28–32 and further studies that even describe methods of producing thermally stable single-atom catalysts from nanoparticles for selected metals.³³ There are advantages to maintaining the single-atom sites, such as establishing a higher activity per gram rating.³⁴ However, it has also been found that small sub-nanoscale clusters can exhibit a higher activity than the single-atom sites with respect to time and cycles^35–38 and, thus, can be equally as important, if not more so, to the overall catalytic activity of the material. For example, a Rh sub-nanoscale cluster of 4–8 Å in diameter on a TiO₂ support was found to be 5–10 times more active than than its single-atom counterpart.³⁶ Furthermore, atomic clusters of up to nine Pt atoms doped into an N₄-site in graphene were shown to be more active than the single-atom sites after 4000 cycles and 16 hours for the hydrogen evolution reaction in acid.³⁵

The relationship between structure and function in catalytic systems is well known. For example, in heterogenous catalysis by changing the surface terminations of Pt that meet to form an edge site, the catalytic activity is altered by 5 orders of magnitude for hydrogen desorption.³⁹ A study of a Ru nanoparticle determined that available active sites have differing activities, with some sites being up to nine orders of magnitude more active than others.^40,41 These studies highlight the importance of understanding the structure of the system being utilised. Very limited work has been carried out on the structure of sub-nanoscale clusters in defect sites on extended C systems – one of the primary aims of the present study.

Much work has been carried out on the incorporation of transition metals into single-atom C-based catalysts.^42–46 Fe,⁴⁶ Co,⁴⁷ Ni,⁴⁸ and Zn⁴⁸ doped N₄-sites have been shown to catalyse the CO₂RR, producing CO as the major product. Rh-N₄ was found to be most active catalyst for producing CH₃OH from a range of transition metals.⁴² However, similar to pure transition metal catalysts, transition metal single-atom catalysts are also limited by scaling relations.^42,49 One suggested way of breaking these scaling relations is to incorporate post-transition metals into graphene-based materials.^42,50 To date, there is very limited research on post-transition metal doped C₃- and N₄-graphene based materials as single-atom catalysts for the CO₂RR.

Post-transition metals have been studied in a limited number of contexts for their catalytic promise. Sn and Al doped C₃-systems have been shown to be active for the CO₂RR.^21,51 A Bi-based metal–organic-framework, has limited propensity for the CO₂RR,⁵² but 2D bismuthene has been reported to be a highly effective catalyst for the same reaction.⁵³ Au is included in this study as it is a noble metal well-known for its highly size-dependent catalytic ability.⁵⁴

In this study, a computational approach is used to determine the structure of noble (Au) and post-transition metal (Al, Bi, Ga and Sn) doped single-atom and sub-nanoscale cluster catalysts in C₃- and N₄-sites.

Computational details

All calculations were carried out in the Vienna ab initio Simulation Package (VASP)⁵⁵ using plane wave density functional theory (DFT) with the PBE⁵⁶ exchange-correlational functional in conjunction with the projector-augmented wave (PAW)⁵⁷ method. An energy cutoff of 600 eV was used, with van der Waals (vdW) interactions included through the D3 method with Becke–Johnson damping.^58,59 Calculations were electronically converged to 1 × 10⁻⁵ eV, while ionic convergence was set to 1× 10⁻² eV to avoid minor optimisation fluctuations in the graphene sheets. Monkhorst–Pack k-point sampling of 3 × 3 × 1 was selected. Higher k-points were tested, but energies were altered by less than 2 × 10⁻⁴ eV atom⁻¹ as the k-point grid was increased (Table S1, ESI†).

The objective of this work was to understand how selected metal dopants localise in defect sites in graphene-based materials. The dopants considered in this study were Al, Au, Bi, Ga and Sn. Two different sites were considered; a C₃-site, where a single atom has been removed from the graphene sheet (Fig. S1, ESI†) and an N₄-site, where two adjacent C atoms are removed and the four under-coordinated C atoms substituted with N (Fig. S2, ESI†). Unit cell sizes of 4 × 4 hexagonal C rings were used, resulting in 50 atoms in the C₃-site system and 49 atoms in the N₄-site system. Size testing of the system was carried out by examining how the adsorption energy (E_ads) of the metal dopant to the site varied with respect to the size of the system (Fig. S3, ESI†). Periodic systems were separated by a minimum of 15 Å of vacuum in the z-dimension. Reference single atoms were calculated as an isolated atom in a vacuum, separated from the next repeating unit by no less than 12 Å.

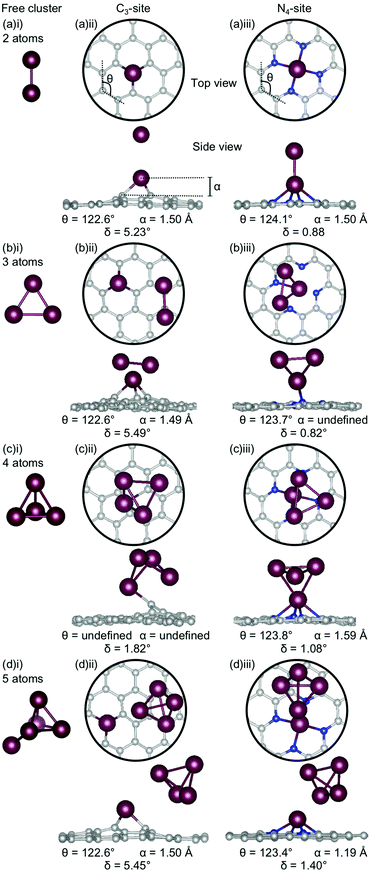
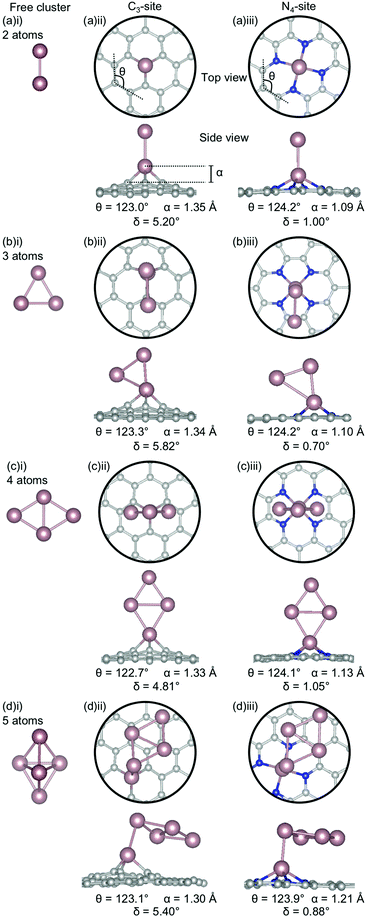
In the first section of this work, the structure of single metal atom doped C₃- and N₄-sites were examined. However, it has been experimentally proven to be extremely difficult to ensure that single-atom catalysts are truly ‘single-atom’ due to the limited control of experiments in this size regime, therefore it is likely that dopant atoms cluster in the defect sites. In the second section of this work, clustering of metal dopants in the C₃- and N₄-sites was considered. In this section, the stability of low energy free clusters, selected from exhaustive global optimisation studies from two to five atoms in size,^60–66 were calculated. From this initial examination of low energy cluster confirmations, all 2- and 3-atom configurations were doped into the sites. For the 4- and 5-atom clusters, the three lowest energy configurations from the free cluster calculations were doped into the C₃- and N₄-sites.

Comparative calculations were also carried out in TURBOMOLE⁶⁷ for the single-atom systems and for the free clusters. Similar trends were found between the two quantum chemical codes, providing an extra assurance that the results found in this study are reliable. Full discussion of the computational setup and results from these simulations can be found in Supplementary Note 6 (ESI†). The TURBOMOLE calculated spin states are taken as the minimum energy spin configuration of the VASP structures, due to the geometric and electronic similarity of these systems and the difficulty of extracting this information from VASP simulations.

Results and discussion

Metal dopants in C₃- and N₄-sites

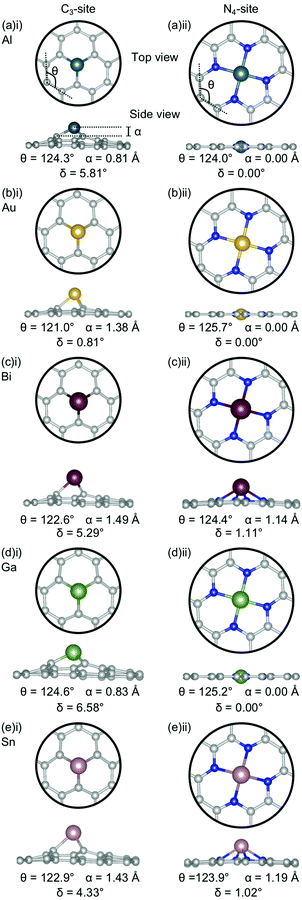
Firstly, the geometries of the single-atom doped C₃- and N₄-sites for all metals are considered (Fig. 1). In the C₃-site, the out-of-plane height is correlated to ring strain with Al and Ga having a lower out-of-plane height of 0.81–0.83 Å and a higher ring strain of 124.3–124.6° (Fig. 1(a)(i) and (d)(i)) compared to Au, Bi and Sn which all have larger out-of-plane binding heights, between 1.38–1.49 Å, and a lower ring strain of 121.0–122.9° (Fig. 1(b)(i), (c)(i) and (eii)).

Fig. 1 Optimised geometries for metal doped (i) C₃- and (ii) N₄-sites for (a) Al, (b) Au, (c) Bi, (d) Ga and (e) Sn. C atoms are grey, N atoms are blue, Al atoms are teal, Au atoms are yellow, Bi atoms are red, Ga atoms are green and Sn atoms are pink. The out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view are to used highlight the active site and not the unit cell repetitions.

For the N₄-site, of those metals that bind in-plane – Al, Au and Ga – the two with larger vdW radii, Au and Ga, (2.32 Å) exhibit an increased ring strain, with θ between 125.2–125.7° (Fig. 1(b)(ii) and (d)(ii)). However, Al (2.25 Å) has a similar ring strain (θ: 124.0°; Fig. 1(a)(ii)) to the considerably larger Sn and Bi (θ: 123.9 and 124.4°, respectively; Fig. 1(c)(ii) and (e)(ii)). For Al, this reduction in ring strain is attributed to the smaller vdW radius while for Bi and Sn, the lower ring strain is attributed to the out-of-plane binding of the metal dopant as a result of their considerably larger vdW radii (2.42 and 2.52 Å, respectively).

A third parameter for describing these systems has also been defined, δ:

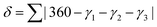where γ₁, γ₂ and γ₃ are the internal angles of the three nearest neighbours to each C atom (Fig. S4, ESI†). C atoms are excluded if they have less than 3 nearest neighbours, meaning that δ values should not be compared between C₃- and N₄-systems. Generally, δ is a descriptor for the degree of planarity in the extended C system.

In the C₃-site, the Au-doped system is considerably more planar (0.81°) than the other four metal-doped systems, which all have a similar degree of distortion (4.33–6.58°). In the N₄-site, the three metals that bind in-plane also have a planar extended C system (Al, Au and Ga) while the Bi and Sn doped N₄-systems have a small but non-zero distortion (1.02–1.11°).

The relationship between the adsorption energy, E_ads, and the vdW radius⁶⁸ of the metal dopant was then examined (Fig. 2a). E_ads is calculated as:

E_ads = E_tot − E_site − E_atom (2)

where E_tot is the total energy of the system containing the metal dopant, E_site is the energy of the optimised undoped C₃- or N₄-site in the extended C system and E_atom is the energy of the metal dopant atom in isolation. E_ads is defined such that, the more negative the value, the stronger the interaction between the metal dopant and the graphene flake. There are many different methods available for calculating the vdW radii of elements.^68–72 We select the values presented by Alvarez for the vdW radii due to their inclusion all of the metals considered in this study, meaning that all radii are calculated within the same mathematical framework.⁶⁸ Other methods of calculating the vdW radii assign the same radii to Ga and Al, with the ordering of other metals being the same as Alvarez.^70,71

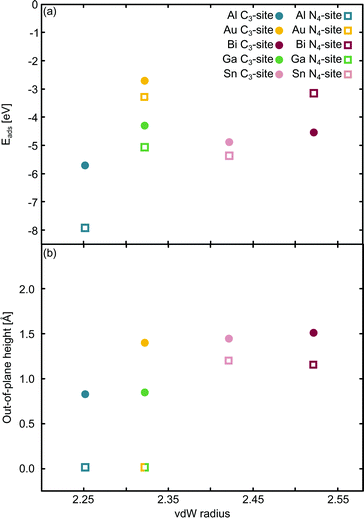Fig. 2 Single metal dopants in C₃- and N₄-sites. The relationship between the van der Waals (vdW) radius⁶⁸ and (a) E_ads and (b) out-of-plane binding height is shown. The green/yellow square shows the out-of-plane binding height for Ga and Au, which have the same vdW radius.

E _ads does not change systematically with respect to size of the dopant atom (Fig. 2a). In the C₃-site, Ga, Sn and Bi all have a similar E_ads, despite having considerably different vdW radii, while Al has a slightly stronger E_ads. Au has a comparatively small vdW radius of 2.32 Å and a particularly weak E_ads. However, size is not the only factor that dictates the interaction of the dopant with the flake; valence electrons and density of states (Fig. S5–S14, ESI†) also play a key role. Al has a valency of 3s²3p¹, Ga of 4p¹, Sn, 5p² and Bi, 6p³. The s² valence electrons for Sn and Bi, are not available for bonding due to the ‘inert pair effect’ that results from the difference in energy of the s and p orbitals for elements in the lower rows of the periodic table. Ga sits on the cusp of this transition from binding to non-binding valence s² electrons,^73,74 likely explaining the similarity in E_ads of these three dopants. Al, however, is able to utilise the valence 3s²3p¹ electrons for bonding, thus giving Al an increased flexibility in bonding configurations that it can adopt. Finally, Au, with a 6s¹ valency, is simply too noble to form strong bonds with the defect site.

In the N₄-sites, Al has a particularly strong E_ads. Bi and Au have the weakest E_ads of the metals considered here and Ga and Sn exhibit a similar E_ads, between that of Al, and Au and Bi. The strong interaction of Al with the N₄-site is not surprising as it has 3 valence electrons to form both σ and π bonds with the site and sits in-plane. Furthermore, because Al and N are close on the periodic table, their orbitals have similar energetics and thus, good overlap, ultimately leading to a strong interaction. Au is a noble metal and only has a 6s¹ electron available for binding, with the addition of a small capability for dispersive binding due to polarisation of the d-shell. When compared to, for example, Ga, with the same vdW radius, Ga has a valence configuration of 4p¹. Ga having a p valency allows for better orbital overlap with the valence N p states thus enabling a stronger adsorption interaction with the defect site.

When comparing the C₃-site to the N₄-site, the E_ads varies across a smaller range for the former. E_ads is consistently less in the C₃-site than in the N₄-site, with the exception of Bi, which, counter-intuitively, binds more strongly to the smaller C₃-site than the bigger N₄-site. Au, Ga and Sn all display a similar difference in E_ads between the C₃- and N₄-sites, while Al has a considerably stronger E_ads in the N₄-site compared to the C₃-site. The increased stability for N₄-binding over C₃-binding is likely due to the increased versatility of the N atoms compared to the C atoms. That is, N atoms provide opportunity for both σ and π bonding, while sp² hybridised C only offers σ bonding.

Subsequently, the relationship between the vdW radius of the metal dopant and the out-of-plane binding height was investigated (Fig. 2b). Two possible binding heights for the metal dopants in each of the C₃- and N₄-sites were observed; in the C₃-site, if the metal dopant has a vdW radius ≤2.32 Å (Al and Ga), it sits ∼0.8 Å out-of-plane, while if the vdW radius is ≥2.42 Å (Bi and Sn), the metal dopant sits ∼1.5 Å out-of-plane. The out-of-plane binding height of Au in the C₃-site is an anomaly as it has the same vdW radius as Ga, but an out-of-plane binding height comparable to Bi and Sn (Fig. 2b). This is likely due to Au having a full valence d-shell, making it ‘hard sphere’ compared to Ga, which has a more diffuse valence shell. In the N₄-site, however, Al, Au and Ga all fit within the cavity and bind in-plane with the extended C system, while Sn and Bi are larger and, therefore, bind at a height of ∼1.2 Å (Fig. 2b). We also highlight that the difference in the binding height between the dopants in the C₃-site is considerably less (∼0.7 Å) compared to the N₄-site (∼1.2 Å). Due to the more similar binding height of the dopants in the C₃-site, it is plausible that the E_ads is less variable for these sites. In contrast, the marked difference in binding height in the N₄-site, in conjunction with the variance in valency, is a probable explanation for the increased variance in E_ads of this site. Finally, we suggest that the particularly weak binding of Bi in the N₄-site is the result of both the large out-of-plane binding height and the doublet spin state of Bi giving rise to a comparatively destabilised structure. This is in contrast to the Sn doped N₄-site, which has a similar out-of-plane binding height to Bi but adopts a triplet spin state, thus Sn is likely able to better interact with the N p orbitals than Bi (Fig. S10 and S14, ESI†).

Chan et al. found that in the C₃-site, Al, Ga, Sn and Bi were covalently bound to the neighbouring C atoms.⁷⁵ Electron localisation function (ELF) analysis carried out in this study shows that there is a degree of covalency to the metal dopant-C bond, but that this bond is not truly covalent, when compared to, for example, the sp² hybridised C–C bond (Table S2, ESI†). It has previously been found that a covalent bonding interaction between the C and the dopant atom results in large in-plane distortions.⁷⁶ Here, we find that both the size of the metal dopant and E_ads factor into the distortion in the graphitic flake. In the C₃-site, because all of the metal dopants considered in this study are too large to site within the defect, E_ads appears to be the determining factor in the degree of distortion in the graphene flake. Au doped C₃-systems have the weakest E_ads of any metal considered here, thus, we attribute the planarity of the extended C system to the comparatively weak interaction between Au and the C system. In contrast, Al, Bi, Ga and Sn all display a similar degree of distortion within the graphene flake (δ: 4.33–6.48°). In the N₄-sites, however, the size of the metal dopant is the determining factor for the degree of planarity in the graphitic flake, with Al, Au and Ga all binding in-plane and maintaining the planarity of the graphene flake (δ: 0.00°). In contrast to this, Bi and Sn bind out-of-plane (α: 1.14–1.19 Å) which results in the graphene flake no longer being truly planar (δ: 1.02–1.11°).

In order to further probe the single-atom dopant systems, the charge density distribution was examined by means of the charge density (Table S3, ESI†) and Bader charge analyses (Tables S4 and S5, ESI†). These show that the Bader charge on all the metal dopants is lower than the formal charge, which is unsurprising due to the delocalisation of electrons. It is interesting that Ga, in the same group as Al, has a Bader charge similar to that of Bi and Sn but a very similar geometric structure to Al. However, this is likely due to the inert pair effect that needs to be taken into account for the Ga, Bi and Sn. Al, Au, Bi and Ga doped C₃- and N₄-sites all resulted in the doublet being the lowest energy spin state, while the lowest energy spin states for Sn doped systems were found to be a singlet and triplet state in the C₃- and N₄-site, respectively (Table S10, ESI†).

Free clusters (N = 2–5)

Making true ‘single-atom’ catalysts is the goal of experiments, however, in reality it is extremely difficult to control how many dopant atoms locate in the cavities under experimental conditions.^24–27 Therefore, in this study, the structure of these sub-nanoscale metal clusters doped into C₃- and N₄-sites are explicitly explored. To do this, low energy configurations of free metal clusters 2–5 atoms in size are considered (Table S6, ESI†) and the cohesive energy (E_coh) was calculated (Fig. 3). E_coh is defined as:⁷⁷where E_cluster is the total energy of the cluster, N_cluster is the number of atoms in the cluster and E_atom is the energy of the single metal atom in isolation.

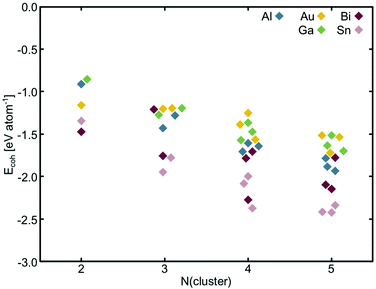Fig. 3 Cohesive energy per metal atom for selected free clusters. Points are offset on the x-axis only to make them visible and are associated with the nearest integer value.

Of the free metal clusters, Sn generally displays the strongest E_coh. Bi exhibits an intermediate E_coh between Sn, and Al, Au and Ga. It is interesting to note, that Bi has the largest range in E_coh of all of the metals considered here. Al, Au and Ga all display similar E_coh however, the range in E_coh for Au is lower across the N = 2–5 atom size range than for Al and Ga. The trends between the metal E_coh values calculated here are consistent with those calculated by various methods in the literature (Fig. S15, ESI†).

We take the spin states calculated in TURBOMOLE to be the lowest energy electronic configurations (Tables S11 and S12, ESI†). Al and Ga both have one p valence electron and when there are an uneven number of atoms in the cluster prefer a doublet spin state. However, when there are an even number of atoms in the cluster, the high spin triplet state is preferred over the singlet state, in keeping with prior studies on the spin states of Ga clusters.^61,78 Bi has three valence p electrons and Au has one valence s electron but, in contrast to Al and Ga, these metals flip between the singlet state, when the number of the atoms in the cluster is even, to the low spin doublet state, when the number of atoms in the cluster is uneven. Sn has two p valence electrons and is more stable in the high spin triplet configuration when N_cluster ≤ 3 atoms and the low spin singlet state when N_cluster ≥ 4 atoms.

Clustering of metal dopants in C₃- and N₄-sites

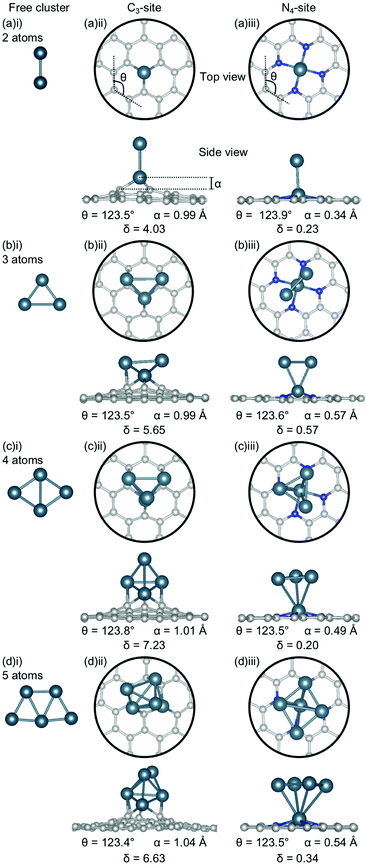
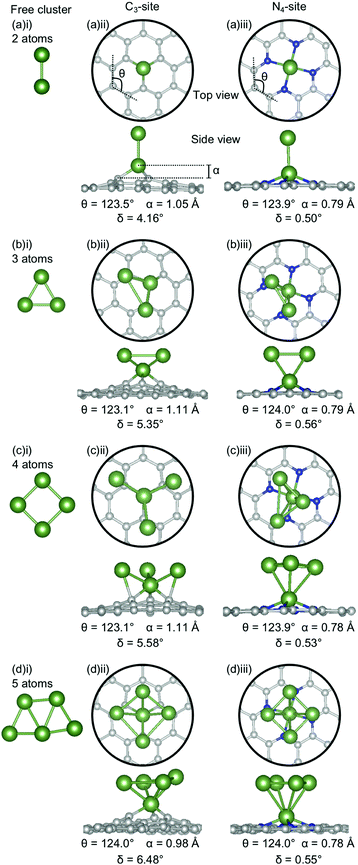
The clusters for which the E_coh was calculated were then doped into C₃- and N₄-sites to simulate dopant clustering in the cavity. What follows is a detailed analysis of the lowest energy configurations of clustered metal dopants in C₃- and N₄-sites and their relation to the lowest energy free cluster configurations. All cluster geometries and relative electronic energies can be found in the ESI† (Tables S7 and S8).

Al and Ga. The lowest energy free clusters for both Al and Ga show that a triangular, planar motif is favoured for all sizes between 3 and 5 atoms (Fig. 4 and 5(b)(i), (c)(i), (d)(i)). However for Ga, the minimum energy N = 4 atom free cluster geometry is a square, in contrast to Al for which it is a rhombus. The low energy clusters found in this study are consistent with those found by Drebov et al. and Ahlrichs et al.^60,61,79

Fig. 4 Lowest energy structures for Al systems of (a) 2-atoms, (b) 3-atoms, (c) 4-atoms and (d) 5-atoms in size as (i) a free cluster and doped in the (ii) C₃- and (iii) N₄-site. Al is shown in teal, C in grey and N in blue. The average out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view highlight the active site and not the unit cell repetitions.

Fig. 5 Lowest energy structures for Ga systems of (a) 2-atoms, (b) 3-atoms, (c) 4-atoms and (d) 5-atoms in size as (i) a free cluster and doped in the (ii) C₃- and (iii) N₄-site. Ga is shown in green, C in grey and N in blue. The out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view highlight the active site and not the unit cell repetitions.

In the C₃-sites, Al doped systems are considerably different to the free clusters of the same size. In particular, the preference for planarity is lifted. At cluster sizes of 3–5 atoms, Al is in close proximity to more than one C atom (Fig. 4(b)(ii), (c)(ii) and (d)(ii)), possibly due to the small size of Al allowing an increased proximity. The interaction of the metal cluster to the graphene flake through more than one C atom is also observed for Ga at N = 4 atoms (Fig. 5(c)(ii)). For Al, the ring strain is very consistent between all clustered sites, ranging from 123.4–123.8°, and is slightly lower than the single-atom doped system (124.3°). The out-of-plane binding height of the Al also remains very similar between the lowest energy clustered configurations (0.99–1.04 Å) but is slightly higher in all clustered cases than the single-atom doped system (α: 0.88 Å). In the Ga doped systems, a slightly higher out-of-plane binding height (0.98–1.11 Å) than the single-atom doped system (0.83 Å), and an associated lower ring strain (123.1–124.0° compared to 124.6° for the single-atom doped system) is observed. The Al doped C₃-sites have a considerably distorted extended C system with δ values ranging from 4.03–7.23°, however, this is in range of the single-atom doped system (δ: 5.81°). In the Ga-systems, high δ values are also observed (Fig. 5(a)(ii), (b)(ii), (c)(ii), (d)(ii)) in the range of 4.16–6.48°. In both the Al and Ga C₃-systems, this high degree of distortion in the extended C system could be the result of the clustered metal atoms interacting with the graphene flake through multiple atoms, and therefore, these C atoms being pulled out of plane. As a final note, the Ga systems also appear to have a preference for the clustered Ga atoms orienting over C atoms associated with the C₃-site.

There are two points of interest for clustering in the N₄-site. Firstly, in all cases, the doped metal atom is pulled out of the graphitic plane (for Al, α: 0.34–0.57 Å and, for Ga, α: ∼0.78 Å; Fig. 4 and 5(a)(iii), (b)(iii), (c)(iii), (d)(iii)), in contrast to the optimised single-atom configuration where the dopant atom sat perfectly in-plane with the extended C system for both Al and Ga. While the δ values are low for all of the clustered systems (Al; 0.20–0.57°, Ga; 0.50–0.56°), these values are, notably, non-zero. Secondly, when considering a N atom cluster, the lowest energy configuration is that of the optimised single-atom doped N₄-site with the N − 1 free cluster above the metal-doped site. For example, the lowest energy structure for the N₄-site doped with a 4-atom (N) Al cluster (Fig. 4(c)(iii)) is the metal doped N₄-site (Fig. 1(a)ii) beneath the 3-atom (N − 1) subcluster (Fig. 4(b)(i)). When clusters of 4- and 5-atoms are doped into the N₄-site, there appears to be a preference for the Al atoms locating directly over the N atoms. The ring strain in the Al-doped N₄-systems is very similar across all cluster sizes (123.5–123.9°) and also to the singly doped system (124.0°). For the clustered Ga systems, associated with an increased out-of-plane binding height is a lower ring strain (∼123.9°) compared to the single-atom doped system (125.2°).

Au. Au has the same lowest energy free cluster geometries as Al with a triangular planar motif being preferred (Fig. 6(b)(i), (c)(i), (d)(i)). However, the linear trimer for the three-atom cluster is very competitive with the triangle motif, being only 0.03 eV higher in energy (Table S6, ESI†). The lowest energy configurations found in this study agree with those found by others^64,66 except for N = 3 atoms. However, a consensus on the lowest energy configuration at this size has not yet been reached, with Assadollahzadeh et al. finding the lowest energy configuration to be a wide isosceles triangle,⁶⁴ and Jain et al. finding it to be a linear trimer,⁶⁶ which is also the competitive structure found here.

Fig. 6 Lowest energy structures for Au systems of (a) 2-atoms, (b) 3-atoms, (c) 4-atoms and (d) 5-atoms in size as (i) a free cluster and doped in the (ii) C₃- and (iii) N₄-site. Au is shown in yellow, C in grey and N in blue. The out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view highlight the active site and not the unit cell repetitions.

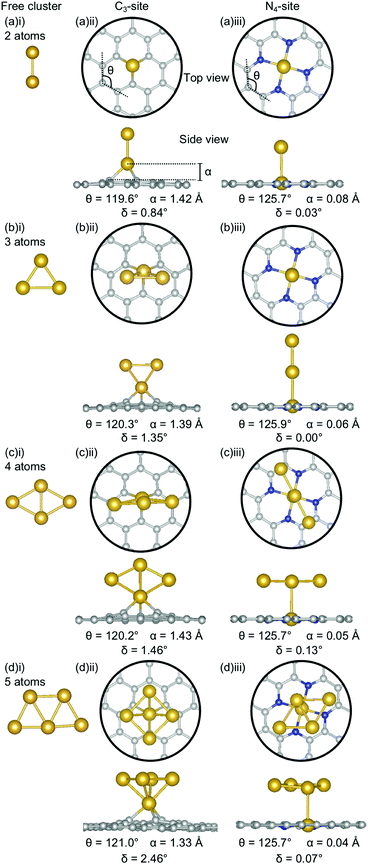
The clustering of Au in the C₃-sites is interesting, because, compared to all of the other metals, the graphitic flake is more planar (Fig. 6(a)(ii), (b)(ii), (c)(ii), and (d)(ii)) with δ values between 0.84–2.46°. It is also interesting to note, that the δ values increase monotonically as the cluster size increases. The 2-atom Au doped system has a similar δ value to that of the single-atom doped system (0.83° and 0.81°, respectively). For N = 2–4 atoms, the lowest energy cluster simply inserts into the C₃-site, and the cluster geometry of the lowest energy free cluster is retained. This is not the case for the 5-atom system, where a square planar N − 1 cluster sits above the Au doped C₃-site. The ring strain for the clustered Au systems is fairly consistent (119.6–121.0°) as is the out-of-plane binding height of the binding Au atom (1.33–1.43 Å). Both of these values are comparable to the single-atom doped system (θ: 121.0° and α: 1.38 Å).

In the N₄-site, Au generally exhibits the same trends as Al and Ga, with the lowest energy structure being the N − 1 free cluster above the metal-doped site (Fig. 6(a)(iii), (b)(iii), (c)(iii), (d)(iii)). However, for Au, the cluster binds directly through the Au atom in the N₄-site and perpendicular to the sheet. There is no preference for keeping the doped metal atoms close in proximity to the C-based system. In the N = 3 atom case, the competitive energetics of the triangle and linear trimer cluster are highlighted, with the insertion of the N − 1 subcluster adopting a linear structure rather than the lower surface area triangle geometry. Again, the Au doped N₄-systems are highly planar, with very low δ values of 0.00–0.13° and α values of 0.04–0.08 Å. The planarity of the Au-doped systems likely arises from the weak E_ads of Au with the defect sites resulting from the 6s¹ valence electron.

Bi. Bi free clusters have strong energetic preferences and prefer 3D configurations once this becomes a possibility at N > 3 atoms (Fig. 7(c)(i) (d)(i) and Table S6, ESI†). For example, N = 3 atoms, the triangle motif is 1.64 eV lower in energy than the linear trimer, while for N = 4 atoms, the trigonal pyramid is 1.97 eV lower in energy than the next lowest energy structure. Yuan et al. also find the N = 4 atoms, trigonal pyramid structure to be particularly stable, which they attributed to a closed electronic structure at this size according to the jellium model – they determine the N = 4 atoms to be a ‘magic’ size for Bi.⁶⁵ For N = 5 atoms, however, the energetic preference is less strong, with the lowest energy structure (bent trigonal bipyramid) being only 0.26 eV lower in energy than the square pyramid. At this size, Yuan et al. find the square pyramid to be the lowest energy configuration.⁶⁵

Fig. 7 Lowest energy structures for Bi systems of (a) 2-atoms, (b) 3-atoms, (c) 4-atoms and (d) 5-atoms in size as (i) a free cluster and doped in the (ii) C₃- and (iii) N₄-site. Bi is shown in red, C in grey and N in blue. The out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view highlight the active site and not the unit cell repetitions.

When Bi becomes clustered in the C₃-site, a number of the Bi-Bi bond distances become anomalously long (Fig. 7(a)(ii), (b)(ii), (c)(ii), (d)(ii)). For example, the Bi–Bi dimer has a bond length of 2.65 Å (Fig. 7(a)(i)), in good agreement with previous experimental and calculated values,^80,81 however when this dimer is doped into the C₃-site, the Bi-Bi bond distance is increased to 3.74 Å – longer than the reported non-covalent Bi-Bi bond of 3.53 Å.⁸² Furthermore, in the N = 3 atom cluster (Fig. 7(b)(ii)), the Bi-Bi bond distance between the Bi doped in the C₃-site and the 2-atom, N − 1, subcluster is 4.20 Å – 1.5 times that of the calculated Bi-Bi dimer. The N = 4 atom cluster is unique because the Bi atom is bound to the extended C system through a single C atom, rather than through the three available in the C₃-site, resulting in one of the hexagonal C rings associated with the C₃-site being altered such that a 5-membered ring is formed. In the N = 5 atom cluster, the minimum bond distance between the single-atom doped site and the N − 1 subcluster is 3.96 Å making the subcluster effectively unbound. However, covalent bonding within the subclusters is expected, due to the proximity of the Bi atoms.⁸²

The distortion (δ) in the flake is fairly consistent for the Bi doped C₃-systems (5.23–5.49°), and very similar to the singly doped Bi C₃-system, which has a δ of 5.29°. The similarity in the geometric parameters between the single-atom doped system and the clustered systems is rationalised by the clustered systems essentially being single-atom doped systems with loosely bound Bi clusters. The exception to this is N = 4 atoms, which has a much lower distortion of 1.82°. Again, the 4-atom Bi cluster is a particularly stable structure as a unit⁸² and, therefore, interacts weakly with graphene flake, thereby not distorting the flake and explaining this exception. Finally, we note that a larger out-of-plane binding height is not necessarily associated with reduced ring strain for these systems.

In the N₄-site, where N = 3 atoms, the Bi-Bi bond distance between the Bi single-atom doped flake and the 2-atom (N − 1) subcluster is ∼3.0 Å. In the 4- and 5-atom clusters the same geometry is seen for both the C₃- and N₄-sites; a point of difference between Bi, and Al, Ga and Au. All of the Bi doped N₄-systems have a similar degree of planarity, with δ values between 0.82–1.40°, similar to the singly doped Bi N₄-system, which has a δ value of 1.11°.

Sn. Free clusters for Sn have a preference for triangular motifs, (Fig. 8(b)(i), (c)(i), (d)(i)) similar to Al, Au and Ga, however, the 5-atom free cluster adopts a trigonal bipyramidal structure, rather than a planar structure. Furthermore, there is a strong energetic preference for N = 4 atoms to adopt a rhombus structure, with the next lowest energy structure at this size being 1.17 eV less stable. At N = 5 atoms, there is strong competition between the trigonal bipyramidal structure and the square pyramid structure, which is only 0.02 eV less stable (Table S6, ESI†). For N = 2–4 atoms, the lowest energy structures found here are in agreement with those found elsewhere in the literature, however, at N = 5 atoms, these studies find the square pyramid structure to be more stable than the trigonal bipyramid structure.^60,63 Furthermore, in the free clusters found here, while N ≤ 4 atoms, there is no bond length greater than 2.86 Å, in contrast to N = 5 atoms, where all the Sn–Sn bond lengths are ∼3.0 Å. This suggests that covalent bonding is preferred in the Sn clusters ≤4-atoms and metallic bonding preferred in the 5-atom Sn cluster, from comparison to the first nearest neighbour distances in covalent α-Sn and metallic β-Sn bulk networks of 2.80 Å and 3.02 Å, respectively.⁸³

Fig. 8 Lowest energy structures for Sn systems of (a) 2-atoms, (b) 3-atoms, (c) 4-atoms and (d) 5-atoms in size as (i) a free cluster and doped in the (ii) C₃- and (iii) N₄-site. Sn is shown in pink, C in grey and N in blue. The out-of-plane binding height is denoted by α, the average internal angle of the adjacent 6-atom ring is denoted by θ and the degree of distortion in the extended C system is given by δ. Note that the circles in the top view highlight the active site and not the unit cell repetitions.

Sn has a unique behaviour amongst the metals considered here. In both the C₃- and N₄-sites, the lowest energy cluster configurations are identical (Fig. 8(ii), (iii)), making it the only dopant considered in this study to be completely agnostic to site. The out-of-plane binding height for the Sn clustered C₃-sites (1.30–1.35 Å) are consistently less than for the single-atom system (1.43 Å). Interestingly, despite, the lower out-of-plane binding height of the clustered systems, the ring strain is comparable between the clustered and single-atom systems (122.70–123.3° and 122.9° respectively). However, the distortion in the extended C system is slightly higher in the clustered systems (4.81–5.82°) than for the single-atom system (4.33°).

In the N₄-sites, the out-of-plane binding between the clustered and single-atom systems is comparable (1.09–1.21 Å for the clustered sites and 1.19 Å for the singly doped site), as is the ring strain (123.9–124.2° compared to 123.9°) and the distortion in the extended C system (0.70–1.05° compared to 1.02°). There appears to be a preference for Sn–Sn interaction being through a single Sn atom and the Sn–Sn interaction being oriented perpendicular to the extended C system, excepting N = 4 atoms. In the N = 5 atom case, the Sn-Sn interaction bond length is 2.96 Å; comparable to the Sn-Sn metallic bond length in β-Sn, 3.02 Å.⁸³ It is possible that the particularly strong E_coh of Sn free clusters (Fig. 3) makes the lowest energy Sn clusters more difficult to alter thus providing a hypothesis for the consistency of Sn cluster configurations, irrespective of site.

General trends in clustering in flakes. In this section, the interaction energy (E_int) of the graphitic flake and the metal dopant atoms (Fig. 9(a)(i) and (b)(i)) and between the doped metal flake and the remaining cluster atoms (N − 1) (Fig. 9(a)(ii) and (b)(ii)) is calculated. This parameter is only calculated for the lowest energy configuration for clustered dopant atoms in the extended C system.

Fig. 9 Energy of interaction (E_int) between (i) the N-atom cluster and the graphene flake and (ii) the N − 1 atom subcluster and the metal doped graphene flake in the (a) C₃-sites (filled circles) and (b) N₄-sites (unfilled squares). Al is shown in teal, Au in gold, Bi in red, Ga in green and Sn in pink. Insets depict the definition of E_int for a generic system with the dashed line showing where the systems were differentiated.

Considering the interaction energy of a single metal atom with the flake i.e. E_int at N = 1 atom, all are stabilised by more than 3.0 eV (Fig. 9(a)(i) and (b)(i)). From the free cluster energies of the metal dimer (N = 2 atoms), it is clear that E_coh is no more than 1.5 eV atom⁻¹ (Fig. 3). This highlights that if the graphitic flakes were defect rich and metal dopant poor, the expected thermodynamic result would be single atom catalysts rather than clustered sites because it is more energetically favourable for the second metal dopant atom to locate in a defect site than it is for this atom to cluster at the site. The only exception to this is the 4-atom Bi cluster in the C₃-site, where E_coh and the E_int are competitive as this Bi cluster has a closed electronic shell and therefore is a particularly stable configuration,⁶⁵ as has been previously discussed.

The change in E_int from N = 1 atom to N = 2 atoms shows, similarly, only weak changes in comparison to the single atom interaction energies, reinforcing the potential for these systems to produce single-atom catalysts. In the case of the C₃-site, for Al, Sn and Bi there is an initial destabilisation of E_int as the number of dopant atoms is increased. In contrast, for Ga and Au, there is a slight stabilisation of E_int upon adding a second dopant atom to the defect site. By comparison, in the N₄-site, E_int is destabilised for all the dopants upon the addition of a second dopant atom to the defect site. While the changes in E_int in the C₃-site are small, they highlight that the metallic interaction energies vary more in this site, than in the N₄-site.

Finally, E_int between the doped metal flake and the remaining cluster atoms in their adsorbed configuration (N − 1) (Fig. 9(a)(ii) and (b)(ii)) was considered. Generally, the changes in E_int of the N − 1 subcluster in the C₃-site are much greater than in the N₄-site. In the N₄-site, the single metal dopant atom is able to sit in the defect site and the N − 1 subcluster is comparatively free to adopt a preferred minimum energy configuration within the N − 1 subcluster. The ability of the metal dopants to form the minimum energy N − 1 subcluster, and for all the metal dopants to behave in this way, reduces both the magnitude of E_int and the variation in this value, as is reflected in Fig. 9(b)(ii). In contrast, the C₃-site tends to encourage 3D cluster geometries that are less favoured for free clusters, as seen from the lowest energy free cluster calculations (Fig. 4–8 and Table S6, ESI†). Due to the fact that the metal dopants adopt a different cluster geometry than the minimum energy cluster, E_int of the N − 1 cluster with the metal doped flake is highly variable and metal dependent, resulting in larger magnitude and variation in E_int in the C₃-site, compared to the N₄-site.

While no explicit simulations at finite temperatures are carried out in the present research, we expect that the SACs studied here are likely to remain SACs at room temperature, and indeed, well above. Using Boltzmann's kinetic theory as an approximate gauge of average kinetic energy, kinetic effects would destabilise E_int by ∼0.04 eV at room temperature. Given that our interaction energies are between 13–230 times stronger than the destabilising kinetic effect, we conclude that typical experimental temperatures will minimally alter the behaviour described in this work.

Conclusions

In conclusion, the interaction of the metal dopant with the defect site is dictated by the valency and size of the dopant and the size and bonding flexibility of the defect site. Clustering of the dopants at the site is highly likely, experimentally. However, the considerable additional stabilisation that is gained by locating metal dopant atoms in unoccupied defect sites over clustering in an occupied site suggests that both C₃- and N₄-sites may be selective for true single-atom catalysts. Under conditions that support clustering, such as high dopant concentration, the defect site exerts considerable control over the clustering geometry; the smaller C₃-site with reduced bonding options drives dopant clusters to adopt 3D structures. In contrast, the N₄-site is larger with more bonding possibilities, thus a single metal dopant atom sits in the defect site and the N − 1 subcluster is able to sit, loosely bound, above it. As such, we conclude that if clustering at a site does occur, the C₃-site is more likely to maintain this clustering than the N₄-site.

Conflicts of interest

There are no conflicts of interest to declare.

Acknowledgements

The authors wish to acknowledge the use of New Zealand eScience Infrastructure (NeSI) high performance computing facilities and consulting support as part of this research. New Zealand's national facilities are provided by NeSI and funded jointly by NeSI's collaborator institutions and through the Ministry of Business, Innovation & Employment's Research Infrastructure programme. URL https://www.nesi.org.nz. S. L. acknowledges funding from the Deutscher Akademischer Austauschdienst to support this collaboration and Jiang Liang Low for useful discussions. The Zentraleinrichtung für Datenverarbeitung (ZEDAT) of the Freie Universität Berlin is also acknowledged for supplying computational resources.

References

1. Y. Zhang, M. Pagani, Z. Liu, S. Bohaty and R. DeConto, A 40-million-year history of atmospheric CO₂, Philos. Trans. R. Soc. A, 2013, 371, 20130096.
2. K. Schouten, Z. Qin, E. Pérez Gallent and M. Koper, Two pathways for the formation of ethylene in CO reduction on single-crystal copper electrodes, J. Am. Chem. Soc., 2012, 134, 9864–9867.
3. W. Durand, A. Peterson, F. Studt, F. Abild-Pederson and J. Nørskov, Structure effects on the energetics of the electrochemical reduction of CO₂ by copper surfaces, Surf. Sci., 2011, 605, 1354–1359.
4. M. Gattrell, N. Gupta and A. Co, A review of the aqueous electrochemical reduction of CO₂ to hydrocarbons at copper, J. Electroanal. Chem., 2006, 594, 1–19.
5. A. Peterson, F. Abild-Pederson, F. Studt, J. Rossmeisl and J. Nørskov, How copper catalyzes the electroreduction of carbon dioxide into hydrocarbon fuels, Energy Environ. Sci., 2010, 3, 1311–1315.
6. D. DeWulf, T. Jin and A. Bard, Electrochemical and surface studies of carbon dioxide reduction to methane and ethylene at copper electrodes in aqueous solutions, J. Electrochem. Soc., 1989, 136, 1686–1691.
7. Y. Hori, R. Takahashi, Y. Yoshinami and A. Murata, Electrochemical reduction of CO at a copper electrode, J. Phys. Chem. B, 1997, 101, 7075–7081.
8. L. Zhang, Z. Zhao and J. Gong, Nanostructured materials for heterogeneous electrocatalytic CO₂ reduction and their related reaction mechanisms, Angew. Chem., Int. Ed., 2017, 56, 11326–11353.
9. Y. Hori, Electrochemical CO₂ reduction on metal electrodes, 2008.
10. S. Back, H. Kim and Y. Jung, Selective heterogeneous CO₂ electroreduction to methanol, ACS Catal, 2015, 5, 965–971.
11. G. Larrazábal, A. Martín, S. Mitchell, R. Hauert and J. Pérez-Remírez, Enhanced reduction of CO₂ to CO over Cu–In electrocatalysts: catalyst evolution is the key, ACS Catal., 2016, 6, 6265–6274.
12. S. Sarfraz, A. Garcia-Esparza, A. Jedidi, L. Cavallo and K. Takanabe, Cu–Sn bimetallic catalyst for selective aqueous electroreduction of CO₂ to CO, ACS Catal., 2016, 6, 2842–2851.
13. J. Nørskov, T. Bligaard, J. Rossmeisl and C. Christensen, Towards the computational design of solid catalysts, Nat. Chem., 2009, 1, 37–46.
14. F. Abild-Pedersen, J. Greeley, F. Studt, J. Rossmeisl, T. Munter, P. Moses, E. Skúlason, T. Bligaard and J. Nørskov, Scaling properties of adsorption energies for hydrogencontaining molecules on transition-metal surfaces, Phys. Rev. Lett., 2007, 99, 016105.
15. K. Novoselov, A. Geim, S. Morozov, D. Jiang, Y. Zhang, S. Dubonos, I. Grigorieva and A. Firsov, Electric Field effect in atomically thin carbon Films, Science, 2004, 306, 666–669.
16. K. Nakada and A. Ishii, Migration of adatom adsorption on graphene using DFT calculation, Solid State Commun., 2011, 151, 13–16.
17. S. Kattel, P. Atanassov and B. Keifer, Stability, electronic and magnetic properties of inplane defects in graphene: a First principles study, J. Phys. Chem. C, 2012, 116, 8161–8166.
18. A. Titov, P. Zapol, P. Král, D. Liu, H. Iddir, K. Baishya and L. Curtiss, Catalytic Fe-xN sites in carbon nanotubes, J. Phys. Chem. C, 2009, 113, 21629–21634.
19. D. Lee, W. Lee, W. Lee, S. Kim and Y. Kim, Theory, synthesis, and oxygen reduction catalysis of Fe-porphyrin-like carbon nanotube, Phys. Rev. Lett., 2012,(106), 175502.
20. M. Kaukonen, A. Krasheninnikov, E. Kauppinen and R. Nieminen, Doped graphene as a material for oxygen reduction reaction in hydrogen fuel cells: a computational study, ACS Catal., 2012, 3, 159–165.
21. M. Esrafili and N. Saeidi, Sn-embedded graphene: an active catalyst for CO oxidation to CO₂?, Physica E, 2015, 74, 382–387.
22. Y.-H. Lu, M. Zhou, C. Zhang and Y.-P. Feng, Metal-embedded graphene: a possible catalyst with a high activity, J. Phys. Chem. C, 2009, 113, 20156–20160.
23. T. Ma, Q. Fan, X. Li, J. Qiu, T. Wu and Z. Sun, Graphene-based materials for electrochemical CO₂ reduction, J. CO₂ Util., 2019, 30, 168–182.
24. M. Asoro, D. Kovar, Y. Shao-Horn, L. Allard and P. Ferreira, Coalescence and sintering of Pt nanoparticles: in situ observation by aberration-corrected HAADF-STEM, Nanotechnology, 2009, 21, 025701.
25. S. Simonsen, I. Chorkendorff, S. Dahl, M. Skoglundh, J. Sehested and S. Helveg, Direct observations of oxygen-induced platinum nanoparticle ripening studied in situ TEM, J. Am. Chem. Soc., 2010, 132, 7968–7975.
26. T. Risse, S. Shaikhutdinov, N. Nilius, M. Sterrer and H.-J. Freund, Gold supported on thin oxide Films: from single atoms to nanoparticles, Acc. Chem. Res., 2008, 41, 949–956.
27. C. Campbell, S. Parker and D. Starr, The effects of size-dependent nanoparticle energetics on catalyst sintering, Science, 2002, 298, 811–814.
28. R. Qin, P. Liu, G. Fu and N. Zheng, Strategies for stabilizing atomically dispersed metal catalysts, Small Methods, 2018, 2, 1700286.
29. H. Tang, F. Liu, J. Wei, B. Qiao, H. Zhao, Y. Su, C. Jin, L. Li, J. Liu, J. Wang and T. Zhang, Ultrastable hydroxyapatite/titanium-dioxide-supported gold nanocatalyst with strong metal-support interaction for carbon monoxide oxidation, Angew. Chem., Int. Ed., 2016, 128, 10764–10769.
30. H. Jeong, J. Bae, J. Woo Han and H. Lee, Promoting effects of hydrothermal treatment on the activity and durability of Pd/CeO₂ catalysts for CO oxidation, ACS Catal., 2017, 7, 7097–7105.
31. S. Schmidt, C. Greczynski, C. Goyenola, C. K. Gueorguiev, X. S. Czigány, J. Jensen, I. G. Ivanov and L. Hultman, CFx thin solid Films deposited by high power impulse magnetron sputtering: synthesis and characterization, Surf. Coat. Technol., 2011, 206, 646–653.
32. G. K. Gueorguiev, C. Goyenola, S. Schmidt and L. Hultman, CFx: a First-principles study of structural patterns arising during synthetic growth, Chem. Phys. Lett., 2011, 516, 62–67.
33. S. Wei, A. Li, J.-C. Liu, Z. Li, W. Chen, Y. Gong, Q. Zhang, W.-C. Cheong, Y. Wang, L. Zheng, H. Xiao, C. Chen, D. Wang, Q. Peng, L. Gu, X. Han, J. Li and Y. Li, Direct observation of noble metal nanoparticles transforming to thermally stable single atoms, Nat. Nanotechnol., 2018, 13, 856–861.
34. S. Yang, J. Kim, Y. Joo Tak, A. Soon and H. Lee, Single-atom catalyst of platinum supported on titanium nitride for selective electrochemical reactions, Angew. Chem., Int. Ed., 2016, 55, 2058–2062.
35. M. Sun, J. Ji, M. Hu, M. Weng, Y. Zhang, H. Yu, J. Tang, J. Zheng, Z. Jiang, F. Pan, C. Liang and Z. Lin, Overwhelming the performance of single atoms with atomic clusters for platinum-catalyzed hydrogen evolution, ACS Catal., 2019, 9, 8213–8223.
36. H. Guan, J. Lin, B. Qiao, X. Yang, L. Li, S. Miao, J. Liu, A. Wang, X. Wang and T. Zhang, Catalytically active Rb sub-nanoclusters on TiO₂ for CO oxidation at cryogenic temperatures, Angew. Chem., Int. Ed., 2016, 55, 2820–2824.
37. X. Cheng, Y. Li, L. Zheng, Y. Yan, Y. Zhang, G. Chen, S. Sun and J. Zhang, Highly active, stable oxidized platinum clusters as electrocatalysts for the hydrogen evolution reaction, Energy Environ. Sci., 2017, 10, 2450–2458.
38. S. Tian, Q. Fu, W. Chen, Q. Feng, Z. Chen, J. Zhang, W.-C. Cheong, R. Yu, L. Gu, J. Gond, J. Luo, C. Chen, Q. Peng, C. Draxl, D. Wang and Y. Li, Carbon nitride support Fe₂ cluster catalysts with superior performance for alkene epoxidation, Nat. Commun., 2018, 9, 2353.
39. E. Skúlason, A. Faraj, L. Kristinsdóttir, J. Hussain, A. Garden and H. Jónsson, Catalytic activity of Pt nano-particles in H₂ formation, Top. Catal., 2014, 57, 273–281.
40. J. Gavnholt and J. Schiøtz, Structure and reactivity of ruthenium nanoparticles, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 035404.
41. S. Dahl, A. Logadottir, R. Egeberg, J. Larsen, I. Chorkendorff, E. Törnqvist and J. Nørskov, Role of steps in N₂ activation on Ru(0001), Phys. Rev. Lett., 1999, 83, 1814–1817.
42. V. Tripkovich, M. Vanin, M. Karamad, M. Björketun, K. Jacobsen, K. Thygesen and J. Rossmeisl, Electrochemical CO₂ and CO reduction on metal-functionalized porphyrinlike graphene, J. Phys. Chem. C, 2013, 117, 9187–9195.
43. H. Zhou, X. Zou, X. Wu, X. Yang and J. Li, Coordination engineering in cobalt–nitrogenfunctionalised materials for CO₂ reduction, J. Phys. Chem. Lett., 2019, 10, 6551–6557.
44. K. Ogura and I. Yoshida, Electrocatalytic reduction of CO₂ to methanol. 9. Mediation with metal porphyrins, J. Mol. Catal., 1988, 47, 51–57.
45. J. Shen, M. Kolb, A. Göttle and M. Koper, DFT study on the mechanism of the electrochemical reduction of CO₂ catalysed by cobalt porphyrins, J. Phys. Chem. C, 2016, 120, 15714–15721.
46. T. Huan, N. Ranjbar, G. Rousse, M. Sougrati, A. Zitolo, V. Mougel, F. Jaouen and M. Fontecave, Electrochemical reduction of CO₂ catalysed by Fe–N–C materials: a structureselectivity study, ACS Catal., 2017, 7, 1520–1525.
47. F. Pan, H. Zhang, K. Liu, D. Cullen, K. More, M. Wang, Z. Feng, G. Wang, G. Wu and Y. Li, Unveiling active sites of CO₂ reduction on nitrogen-coordinated and atomically dispersed iron and cobalt catalysts, ACS Catal., 2018, 8, 3116–3122.
48. H. Yang, S. Hung, S. Liu, K. Yuan, S. Miao, L. Zhang, X. Huang, H. Wang, W. Cai, R. Chen, J. Gao, X. Yang, W. Chen, Y. Huang, H. Chen, C. Li, T. Zhang and B. Liu, Atomically dispersed Ni(I) as the active site for electrochemical CO₂ reduction, Nat. Energy, 2018, 3, 140–147.
49. J. Li, P. Pršlja, T. Shinagawa, A. Fernández, F. Krumeich, K. Artyushkova, P. Atanassov, A. Zitolo, Y. Zhou, R. Garía-Muelas, N. López, J. Pérez-Ramírez and F. Jaouen, Volcano trend in electrocatalytic CO₂ reduction activity over atomically dispersed metal sites on nitrogen-doped carbon, ACS Catal., 2019, 9, 10426–10439.
50. J. Wu, T. Sharifi, Y. Gao, T. Zhang and P. Ajayan, Emerging carbon-based heterogeneous catalysts for electrochemical reduction of carbon dioxide into value-added chemicals, Adv. Mater., 2019, 31, 1804257.
51. Y. Tang, X. Dai, Z. Yang, Z. Liu, L. Pan, D. Ma and Z. Lu, Tuning the catalytic property of non-noble metallic impurities in graphene, Carbon, 2014, 71, 139–149.
52. E. Zhang, T. Wang, K. Yu, W. Chen, A. Li, H. Rong, R. Lin, S. Ji, X. Zheng, Y. Wang, L. Zheng, C. Chen, D. Wang, J. Zhang and Y. Li, Bismuth single atoms resulting from transformation of metal-organic frameworks and their use as electrocatalysts for CO₂ reduction, J. Am. Chem. Soc., 2019, 141, 16569–16573.
53. F. Yang, A. Elnabawy, R. Schimmenti, P. Song, J. Wang, Z. Peng, S. Yao, R. Deng, S. Song, Y. Lin, M. Mavrikakis and W. Xu, Bismuthene for highly efficient carbon dioxide electroreduction reaction, Nat. Commun., 2020, 11, 1088.
54. M. Haruta, When gold is not noble: catalysis by nanoparticles, Chem. Record, 2003, 3, 75–87.
55. G. Kresse and J. Furthmüller, Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169.
56. J. Perdew, K. Burke and M. Ernzerhof, Generalized gradient approximation made simple, Phys. Rev. Lett., 1996, 77, 3865–3868.
57. P. Blöchl, Projector augmented-wave method, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953–17979.
58. S. Grimme, J. Antony, S. Ehrlich and H. Krieg, A consistent and accurate ab initio parameterization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu, J. Chem. Phys., 2010, 132, 154104.
59. S. Grimme, S. Ehrlich and L. Goerigk, Effect of the damping function in dispersion corrected density functional theory, J. Comput. Chem., 2011, 32, 1456–1465.
60. N. Drebov and R. Ahlrichs, Small clusters of aluminium and tin: highly correlated calculations and validation of density functional procedures, J. Chem. Phys., 2011, 134, 124308.
61. N. Drebov, F. Weigend and R. Ahlrichs, Structures and properties of neutral gallium clusters: a theoretical investigation, J. Chem. Phys., 2011, 135, 044314.
62. X. Gong and E. Tosatti, Structure of small gallium clusters, Phys. Lett. A, 1992, 166, 369–372.
63. B. Assadollahzadeh, S. Schäfer and P. Schwerdtfeger, Electronic properties for small tin clusters Sn_n (n < 20) from density functional theory and convergence toward the solid state, J. Comput. Chem., 2009, 31, 929–937.
64. B. Assadollahzadeh and P. Schwerdtfeger, A systematic search for minimum structures of small gold clusters Au_n (n = 2–20) and their electronic properties, J. Chem. Phys., 2009, 131, 064306.
65. H. Yuan, H. Chen, A. Kuang, Y. Miao and Z. Xiong, Density-functional study of small neutral and cationic bismuth clusters Bi_n and Bi_n⁺ (n = 2–24), J. Chem. Phys., 2008, 128, 094305.
66. P. Jain, A DFT-based study of the low energy electronic structures and properties of small gold clusters, Struct. Chem., 2005, 16, 421–426.
67. F. Furche, R. Ahlrichs, C. Hättig, W. Klopper, M. Sierka and F. Weigend, Turbomole, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2014, 4, 91–100.
68. S. Alvarez, A cartography of the van der Waals territories, Dalton Trans., 2013, 42, 8617–8636.
69. S. S. Batsanov, Calculation of van der Waals radii of atoms from bond distances, J. Mol. Struct., 1999, 468, 151–159.
70. S. S. Batsanov, van der Waals radii of elements, Inorg. Mater., 2001, 37, 871–885.
71. M. Mantina, A. Chamberlin, R. Valero, C. Cramer and D. Truhlar, Consistent van der Waals radii for the whole main group, J. Phys. Chem. A, 2009, 113, 5806–5812.
72. A. Bondi, van der Waals volume and radii, J. Phys. Chem., 1964, 68, 441–451.
73. P. Power, π-bonding and the lone pair effect in multiple bonds between heavier group elements, Chem. Rev., 1999, 99, 3463–3503.
74. W. Kutzelnigg, Chemical bonding in higher main group elements, Angew. Chem., 1984, 23, 272–295.
75. K. Chan, J. Neaton and M. Cohen, First-principles study of metal adatom adsorption on graphene, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 77, 235430.
76. X. Liu, C. Wang, Y. Yao, W. Lu, M. Hupalo, M. Tringides and K. Ho, Bonding and charge transfer by metal adatom adsorption on graphene, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 83, 235411.
77. T. Pawluk, Y. Hirata and L. Wang, Studies of iridium nanoparticles using density functional theory calculations, J. Phys. Chem. B, 2005, 109, 20817–20823.
78. N. Gaston and A. Parker, On the bonding of Ga₂, structures of Gan clusters and the relation to the bulk structure of gallium, Chem. Phys. Lett., 2011, 501, 375–378.
79. R. Ahlrichs and S. Elliott, Clusters of aluminium, a density functional theory, Phys. Chem. Chem. Phys., 1998, 1, 13–21.
80. L. Gao, P. Li, H. Lu, S. Li and Z. Guo, Size- and charge-dependent geometric and electronic structures of Bi_n (Bi_n⁻) clusters (n = 2–13) by First principles simulations, J. Chem. Phys., 2008, 128, 194304.
81. C. Effantin, A. Topouzkhanian, J. Figuet, J. d'Incan, R. F. Barrow and J. Verges, Electronic states of Bi₂ studied by laser-excited uorescence, J. Phys. B: Atom. Mol. Phys., 1982, 15, 3829.
82. H. Matsushima, S.-W. Lin, S. Morin and O. M. Magnussen, In situ video-STM studies of the mechanisms and dynamics of electrochemical bismuth nanostructure formation on Au, Faraday Discuss., 2016, 193, 171–185.
83. M. Musgrave, On the relation between grey and white tin (α-Sn and β-Sn), Proc. R. Soc. A, 1963, 272, 503–528.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/d1cp05008g

---