Open Access Article
Open Access ArticleIn silico activation of dinitrogen with a light atom molecule†
Stefan
Mebs
 *a and
Jens
Beckmann
*a and
Jens
Beckmann
 b
b
aInstitut für Experimentalphysik, Freie Universität Berlin, Arnimallee 14, 14195 Berlin, Germany. E-mail: stefan.mebs@fu-berlin.de
bInstitut für Anorganische Chemie und Kristallographie, Universität Bremen, Leobener Straße 7, 28359 Bremen, Germany
First published on 15th August 2022
Abstract
The N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond can be cleaved in silico with a light atom molecule containing only the earth abundant elements C, H, Si, and P. Extensive density functional theory (DFT) computations on various classes of peri-substituted scaffolds containing Lewis acidic and basic sites in the framework of frustrated Lewis pairs (FLP) indicate that the presence of two silyl cations and two P atoms in a flexible but not too flexible arrangement is essential for energy efficient N2-activation. The non-bonding lone-pair electrons of the P atoms thereby serve as donors towards N2, whereas the lone-pairs of N2 donate into the silyl cations. Newly formed lone-pair basins in the N2-adducts balance surplus charge. Thereby, the N–N bond distance is increased by astonishing 0.3 Å, from 1.1 Å in N2 gas to 1.4 Å in the adduct, which makes this bond prone to subsequent addition of hydride ions and protonation, forming two secondary amine sites in the process and eventually breaking the N
N triple bond can be cleaved in silico with a light atom molecule containing only the earth abundant elements C, H, Si, and P. Extensive density functional theory (DFT) computations on various classes of peri-substituted scaffolds containing Lewis acidic and basic sites in the framework of frustrated Lewis pairs (FLP) indicate that the presence of two silyl cations and two P atoms in a flexible but not too flexible arrangement is essential for energy efficient N2-activation. The non-bonding lone-pair electrons of the P atoms thereby serve as donors towards N2, whereas the lone-pairs of N2 donate into the silyl cations. Newly formed lone-pair basins in the N2-adducts balance surplus charge. Thereby, the N–N bond distance is increased by astonishing 0.3 Å, from 1.1 Å in N2 gas to 1.4 Å in the adduct, which makes this bond prone to subsequent addition of hydride ions and protonation, forming two secondary amine sites in the process and eventually breaking the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond. Potential formation of dead-end states, in which the dications (“active states”) aversively form a Lewis acid (LA)–Lewis base (LB) bond, or in which the LA and LB sites are too far away from each other to be able to capture N2, are problematic but might be circumvented by proper choice of spacer molecules, such as acenaphthalene or biphenylene, and the ligands attached to the LA and LB atoms, such as phenyl or mesityl, and by purging the reaction solutions with gaseous N2 in the initial reaction steps. Charge redistributions via N2-activation and splitting were monitored by a variety of real-space bonding indicators (RSBIs) derived from the calculated electron and electron pair densities, which provided valuable insight into the bonding situation within the different reaction steps.
N triple bond. Potential formation of dead-end states, in which the dications (“active states”) aversively form a Lewis acid (LA)–Lewis base (LB) bond, or in which the LA and LB sites are too far away from each other to be able to capture N2, are problematic but might be circumvented by proper choice of spacer molecules, such as acenaphthalene or biphenylene, and the ligands attached to the LA and LB atoms, such as phenyl or mesityl, and by purging the reaction solutions with gaseous N2 in the initial reaction steps. Charge redistributions via N2-activation and splitting were monitored by a variety of real-space bonding indicators (RSBIs) derived from the calculated electron and electron pair densities, which provided valuable insight into the bonding situation within the different reaction steps.
Introduction
In the field of biological and chemical catalysis, small molecule activation plays a central role. In millions of years of biological evolution, nature facilitated water oxidation,1 oxidative hydrogen splitting as well as hydrogen formation via proton reduction,2 oxygen reduction,3,4 carbon dioxide reduction,5 and finally splitting of dinitrogen,6 – the most inert molecule known – via stepwise reduction, to name the most important ones. The underlying chemical processes are without exception realized by metalloproteins, containing typically 1–6 transition metal atoms such as Mn, Fe, Co, Ni, Cu, and Mo in the active site, since they require complex multi-step redox-reactions, most of which are still under debate so far.1–6 The resulting biogeochemical cycles of energy and matter are crucial to all forms of life, e.g. without nitrogenases, consuming gaseous N2, the soil would suffer drastically from deficiency of bio-accessible nitrogen.7 In tremendous efforts to understand and mimic nature, scientists are developing and improving crystalline or amorphous solid-state materials (heterogeneous catalysis),8,9 molecular compounds (homogeneous catalysis),10–16 and bio-inspired structures (biomimetic catalysis),17–20 which resulted i.a. in mayor-scale industrial applications in the last century without which the energy and food demands of modern culture couldn’t be maintained. Arguably the most prominent is the Haber–Bosch process for nitrogen fixation and production of ammonia, which was developed and professionalized in the first two decades of the 20th century, giving access to efficient megaton-scale production of fertilizer, and nowadays causes about 1–2% of the global energy demands and about 2–3% of the global CO2 production.21 In the flourishing field of heterogeneous N2 fixation, a diversity of solid state materials derived from metal–organic frameworks (MOFs),22 covalent organic frameworks (COFs),23 doped BN,24,25 BN nanotubes,26 BC nanosheets,27 SiC,28 single metal atoms at graphene29 or BN-graphene sheets,30 nitrogen-rich carbon materials,31 boron doped diamond,32 other 2D-networks,33 and boron carbide nitride nanotube supported single-atom catalysts34 were investigated experimentally and/or computationally. In parallel, recent developments in the field of molecular synthesis proved that N2 can be cleaved using transition-metal containing compounds,35–38 and converted to ammonia,39–41 although in low efficiencies and turn-over numbers so far (Scheme 1). A change in paradigm was introduced shortly after by the first boron-based molecular compounds being capable of nitrogen fixation, proving that transition metal atoms as part of the catalyst are not necessarily essential for nitrogen fixation, not even in homogeneous catalysis (Scheme 1).42–48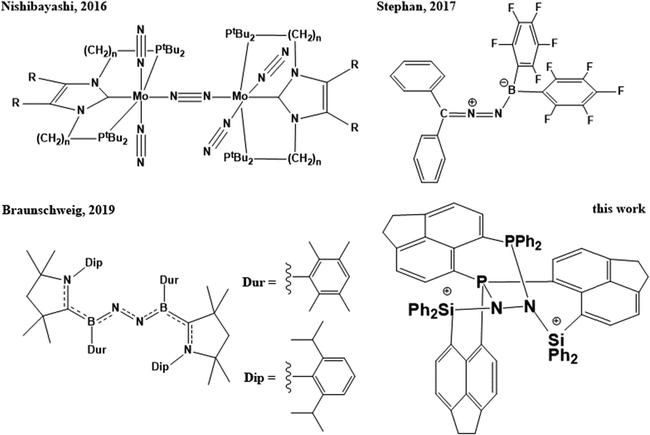 | ||
| Scheme 1 Activation of N2 by Mo-pincer dimers, intermolecular frustrated Lewis pairs, hypovalent borylene compounds, and intramolecular frustrated Lewis pairs. | ||
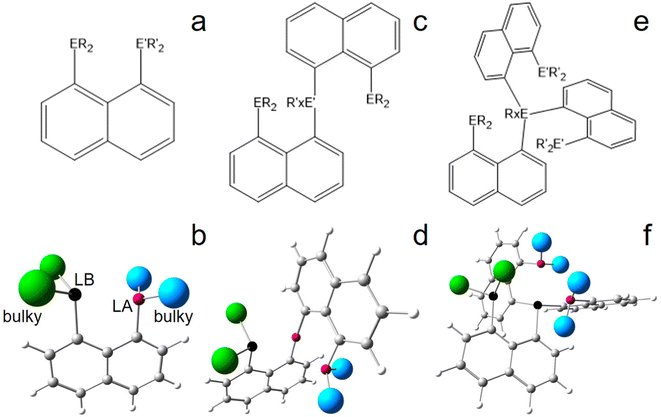
The current study follows that line of evidence, but varies in the conceptual design of the molecular system in order to not only fix and activate the inert N2 molecule, but to ultimately break the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond. The structures proposed here combine different basic chemical concepts, all of which are hypothesized to be essential for nitrogen uptake, activation, and finally splitting with a light-atom molecule: (1) Uptake & Activation: (a) the active site of the molecule follows the concept of frustrated Lewis pairs (FLPs),49–55 which combines a Lewis acidic (LA) site like B or Si+ with a Lewis basic (LB) site like N or P, fixing and polarizing the target molecule, N2. FLPs have received tremendous attention in the last decades due to their catalytic capabilities, especially since they are typically constructed of light atoms.56–60 (b) The catalyst should contain more than one LA and/or LB within one molecule to significantly enhance the gradient of the electrostatic potential in the area between LA and LB. (2) Dynamics: the ideal catalyst molecule offers (limited) rotational degrees of freedom allowing for fine-tuning of the LA–LB distance in the process of N2 splitting, a requirement for electronic accommodation of the whole system. Metalloproteins undergo series of structural and electronic transitions in catalytic reactions. Other rotations (e.g. ligand motion) should be minimized as they may block the active site or cause entropic contributions to dominate over energetic aspects. (3) Protection: concepts 1 and 2 are seemingly in conflict with each other, because spatially flexible LA/LB-pairs may come close enough to form a direct (and strong) LA–LB-bond, thereby quenching any reactivity towards small non-polarized molecules. In order to keep the LA/LB-pair dynamic but frustrated, the choice of proper spacer groups (e.g. poly-aromatic scaffolds) as well as of ligands attached to the LA/LB-atoms (e.g. phenyl vs. mesityl) is crucial. Ideally, the latter are actively preventing the formation of a direct LA–LB-bond by means of steric ligand-to-ligand repulsion, with the benefit of releasing additional energy via adduct formation.
N triple bond. The structures proposed here combine different basic chemical concepts, all of which are hypothesized to be essential for nitrogen uptake, activation, and finally splitting with a light-atom molecule: (1) Uptake & Activation: (a) the active site of the molecule follows the concept of frustrated Lewis pairs (FLPs),49–55 which combines a Lewis acidic (LA) site like B or Si+ with a Lewis basic (LB) site like N or P, fixing and polarizing the target molecule, N2. FLPs have received tremendous attention in the last decades due to their catalytic capabilities, especially since they are typically constructed of light atoms.56–60 (b) The catalyst should contain more than one LA and/or LB within one molecule to significantly enhance the gradient of the electrostatic potential in the area between LA and LB. (2) Dynamics: the ideal catalyst molecule offers (limited) rotational degrees of freedom allowing for fine-tuning of the LA–LB distance in the process of N2 splitting, a requirement for electronic accommodation of the whole system. Metalloproteins undergo series of structural and electronic transitions in catalytic reactions. Other rotations (e.g. ligand motion) should be minimized as they may block the active site or cause entropic contributions to dominate over energetic aspects. (3) Protection: concepts 1 and 2 are seemingly in conflict with each other, because spatially flexible LA/LB-pairs may come close enough to form a direct (and strong) LA–LB-bond, thereby quenching any reactivity towards small non-polarized molecules. In order to keep the LA/LB-pair dynamic but frustrated, the choice of proper spacer groups (e.g. poly-aromatic scaffolds) as well as of ligands attached to the LA/LB-atoms (e.g. phenyl vs. mesityl) is crucial. Ideally, the latter are actively preventing the formation of a direct LA–LB-bond by means of steric ligand-to-ligand repulsion, with the benefit of releasing additional energy via adduct formation.
Typically, it is a matter of months from initial ligand design on the desk to successful bench synthesis in the chemical laboratory. Failures and obstacles cause delays and are not sustainable by means of resources – energy, time, money, and material (any maybe stamina). Computational chemistry nowadays opens large capabilities for screening large numbers of potential candidates, the most promising of which may be synthesized later. In the fields of metal–organic frameworks (MOF), 2D-networks, and solid-state structures, in silico design and screening of hypothetical compounds is already based on algorithmic generation of structural databases.29,61,62 However, this approach is not easily transferable to molecular design since the variation of parameters, e.g. spacer groups or ligands attached to the active centers, typically results in significant alterations of the structural motifs, many of which are non-unique, e.g. due to rotational isomerism. Moreover, a prerequisite for in situ activation of N2 with a light-atom molecule is that the catalyst should be principally accessible by (known) synthetic routes, excluding many hypothetic candidates. The compounds chosen in the present density functional theory (DFT) study are thus derived from and related to a variety of published peri-substituted compounds comprising intramolecular interactions.63–70Peri-substituted compounds were initially synthesized to enforce direct atom–atom contacts and investigate the basic bonding characteristics.71 The structures typically employ naphthalene or acenaphthalene as spacer groups, but xanthene, dibenzofuran, biphenylene and others are also used to modulate the peri-distance. In the framework of this study only acenaphthalene (ace), and biphenylene (bip) are used with DFT-calculated H⋯H peri-distances of 2.7 and 3.9 Å in the non-substituted species, whereas naphthalene (dperi = 2.5 Å), xanthene (dperi = 4.9 Å) and dibenzofuran (dperi = 5.4 Å) are considered to be detrimental due to their short/large peri-distance and the presence of an oxygen atom in the central part of the spacer molecule in the latter two, the lone-pairs of which might potentially perturb N2-adduct formation. The focus is directed towards the ace-containing structures as they are potentially most easily available by known synthetic routes.
Limitations of the method: the Gibbs free energy (ΔG) can principally be obtained by vibrational frequency calculations, which was, however, not feasible for the investigated compounds due to their size and the high computational costs at the applied b3pw91-D3/6-311+G(2df,p) level of theory. In order not to be restricted to the electronic energy difference (ΔE) obtained from the DFT optimizations, the DFT optimization were repeated for the most relevant states at the lower b3pw91-D3/6-31+G* level. ΔE (at the higher level) and ΔG (at the lower level) showed the same trends, but considerable differences in the actual numbers. Based on the same grounds, the current study was restricted to the first three reaction steps of N2 splitting, including N2 fixation/activation by the ligand system, reduction with hydride (H−) and subsequent protonation (H+) or second reduction (H−). Since a complete transformation of one molecule N2 into two molecules NH3 require six reduction and protonation steps in potentially variable order and since both N atoms could be attacked from several topological sites by H+ or H− in each step in the systems used here, the potential number of possible reaction paths goes into the hundreds. Thus, the results presented here rather serve as prove of concept for the applicability of the employed chemical concepts than as a definite molecular design and related mechanistic study. Nevertheless, this study may guide synthetic chemists for new ligand designs of light-atom molecules being capable of energy efficient and durable homogeneous splitting of nitrogen.
In the following the molecules investigated for N2-splitting are denoted as “ligand system” as they may also serve for complexation with metal ions. The (di)cationic states of the ligand system are considered (and thus labeled) as “active” for N2 uptake and activation if the LA and LB atoms are not closer than 2.5 Å or far off by more than 4.5 Å. The latter two cases accordingly are considered as “dead-end” states. A short LA–LB distance energetically blocks N2 uptake, whereas a very large distance makes it unlikely by entropic means.
Crucial structures, such as the active ligand system, the different N2 bound states (N2-adducts), as well as relevant reaction products with H− or H+ were electronically characterized by a set of real-space bonding indicators (RSBIs) in order to monitor charge reorganization events via reduction of N2. RSBIs comprise the Atoms-In-Molecules (AIM72–74) and Electron Localizability Indicator (ELI-D75) topological approaches, as well as the Non-Covalent Interactions index (NCI76). AIM determines atomic basins from the electron density (ED, ρ(r)) providing atomic and fragmental charges and volumes as well as a bond paths motif, which transcends the Lewis picture of chemical bonding, in that it also includes secondary intramolecular chemical contacts in its topological framework. The electronic bond characteristics at the bond critical points (bcps) are indicative of the nature, e.g. degree of covalency, and strength of the interaction. Even more sensitive to the formation of very weak secondary contacts is the NCI, which gives rise to the formation of contact patches also if AIM “overlooks” them.77 ELI-D determines basins of paired electrons from the electron pair densities, providing electron populations and volumes for core (C(X)), disynaptic bonding (V2(X,Y)), and monosynaptic lone-pair (V1(X)) or protonated (“H atoms”) type of basins. ELI-D and NCI show spatial complementary, as the former is closely related to covalent bonding aspects, whereas the latter is closely related to non-covalent bonding aspects.78 The combined use of those RSBIs thus gives a comprehensive picture of chemical bonding in real-space.
DFT calculations
Structural optimizations were conducted for all compounds by density functional theory (DFT) at the b3pw91-D3/6-311+G(2df,p)79,80 level of theory using Gaussian1681 at the curta super-computer system of the Freie Universität Berlin. London dispersion was modelled using Grimme's GD3BJ parameters (b3pw91-D3).82 The COSMO solvation model was applied to mimic the dichloromethane environment.83 The full ligand systems and corresponding N2-adducts were too large to afford frequency calculations at this level of theory. Accordingly, the optimizations were repeated at the smaller b3pw91-D3/6-311+G* level for the most relevant states. Subsequent normal mode (or frequency) analysis (at 298.15 K and 1 atm) proved all but one structures to be local minima; for 3a (see below) a negligible phonic band at −5 cm−1 was observed. Peri-interaction energies (α-PIEs) were determined by an isodesmic approach, comparing the energy sum of the di-substituted spacer group and non-substituted spacer group with the two different non-substituted spacer groups, e.g. X-spacer-Y + spacer vs. X-spacer + spacer-Y (X, Y: substituents), disclosing peri-interactions to be either attractive, non-bonding, or repulsive. α-PIEs serve here as reference values to judge the energetic costs of quenching states, see ESI,† for details. Attempts to calculate the transition state (TS) of N2-adduct formation failed. A potential energy scan (PES) was thus conducted for a series of fixed N–N distances between 1.10 and 1.54 Å. The wavefunction (wfn) files of relevant structures for N2-activation were used for a topological analysis of the electron density according to the Atoms-In-Molecules (AIM72–74) space-partitioning scheme using AIM2000,84 whereas DGRID-5-185 was used to generate and analyze the Electron-Localizability-Indicator (ELI-D75) related real-space bonding descriptors applying a grid step size of 0.075 a.u. using the formatted checkpoint files (fchk). For ELI-D figures, additional grids of 0.15 a.u. step size were computed. NCI76 grids were generated with NCIplot86 with a grid step-size of 0.12 a.u. Analyses of the reduced density gradient, s(r) = [1/2(3π2)1/3]|∇ρ|/ρ4/3, according to the NCI method is used to visualize non-covalent bonding aspects. An estimation of different non-covalent contact types according to steric/repulsive (λ2 > 0, red-colored), van der Waals-like (λ2 ≈ 0, green-colored), and attractive (λ2 < 0, blue-colored) is facilitated by mapping the ED times the sign of the second eigenvalue of the Hessian (sign(λ2)ρ) on the iso-surfaces of s(r). Structures are displayed with ChemCraft,87 bond paths are displayed with AIM2000, NCI figures are displayed with VMD,88 and ELI-D figures are displayed with MolIso.89Results and discussion
Structures and energies
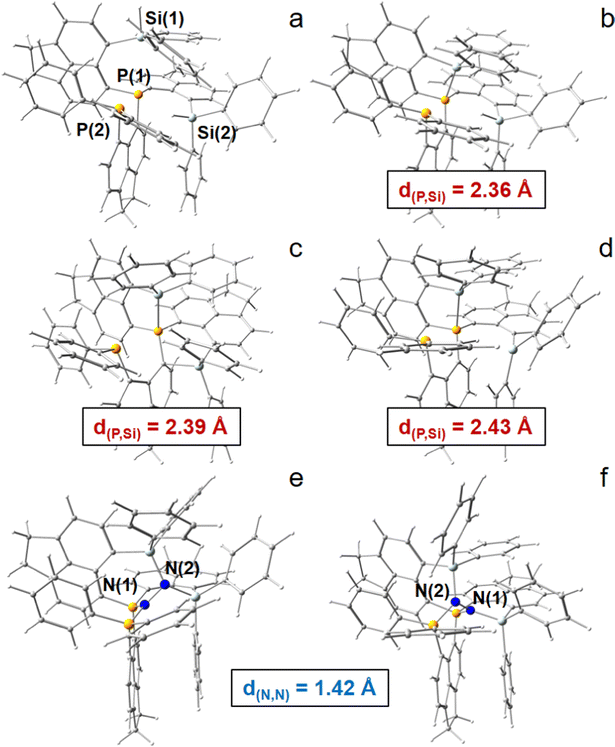
Basic peri-substituted scaffolds are constructed of one spacer group and two peri-substituents, e.g. a LA such as –BF2 and a LB such as –PPh2, see Fig. 1a and b for the conceptual design. The fixed peri-distances and limited number of LA and LB atoms, however, restrict their use for small molecule activation. The “best case” obtained by DFT is presented in the ESI.† A more flexible structural composition is given by adding a second spacer group to the LA or LB atom (Fig. 1c and d), but extensive computational studies proved that the high degree of rotational flexibility causes either the LA and LB atoms to be far away from each other or the formation of energetically low-lying quenched states, rendering this design also ineligible for small molecule activation, see the ESI† for the “best case” obtained by DFT. Accordingly, the study was extended to include three spacer groups, linked by a central LB atom (vide infra), see Fig. 1e and f for the conceptual design. One of the three peri-substituents carries another LB, each of the remaining two carries a LA fragment, resulting ideally in a 2LA–2LB active site. The third spacer group not only provides another LA or LB site, but significantly limits molecular rotations, keeping the whole system more rigid, but still flexible. In the course of finding the best candidates for the central atoms of the LA and LB fragments, only the combination of silyl cations and phosphorus atoms was found to be capable for efficient N2 fixation and activation in silico, see Fig. 2. Replacing P by N or As atoms resulted in significantly lower binding affection and strength towards N2. However, since the corresponding N-analogues are synthetically hardly accessible and As is toxic, this result is advantageous. Using B atoms as LA generates an additional energetic cost as the initially trigonal-planar R3B-fragment has to reorganize towards a tetrahedral R3B-N2 orientation via N2-adduct formation, which is unfavorable and causes the tested reactions to be endothermic (not shown). Consequently, only models comprising Si+ and P are represented in this study. Besides global abundance and the non-toxicity of the used elements, one appealing advantage of a molecular composition including only C, H, Si, and P atoms and N atoms from the target molecule is that all nuclei are sensitive to NMR, rendering detection of intermediate states and reaction products possible.A dicationic ligand system being constructed by a central P atom carrying three acenaphthtyl (ace) spacer groups, a –PPh2 group as second LB as well as two –Si+Ph2 groups as strong LA in the three peri-positions, finally turned out to be the best candidate for the 3-spacer approach, see Fig. 2. The system is energetically indeed capable to uptake N2 and extend the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond (d(N,N) = 1.091 Å for DFT) by 0.327 Å to the unprecedented value of 1.418 Å, corresponding to a N–N single bond, and releasing 161.6 kJ mol−1 of energy in the (in silico) process, see Table S4 (ESI†). Subsequent hydride uptake of the N2-adduct already breaks the N–N bond, vide infra. However, this inspiring result comes with a major setback, as already the first hydride abstraction from the neutral ligand systems (6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)2(1) to form the monocation [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)(-5-ace-6-SiPh2)]+ (2) with one –Ph2SiH and one –Ph2Si+ group results in a quenched state with a short P–Si contact of 2.361 Å, compare Fig. 2a and b. This is already close to the optimized Si–P peri-distance in Ph2P-ace-Ph2Si+ (d(P,Si) = 2.324 Å) and hints towards a stabilization (or Si–P bond strength) of larger than 150 kJ mol−1, see Fig. S4 (ESI†). Second hydride abstraction to form the [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2]2+ (3a) not only keeps this unfortunate situation (d(P,Si) = 2.393 Å), but exhibits an additional short contact of 2.105 Å between the second Si cation and an arylic C atom, which is bound to the central P atom, see Fig. 2c. A slightly more “active state” (3b, d(P,Si) = 2.428 Å, d(Si,Cπ) = 3.327 Å) in which the LA and LB parts are not interacting that strongly is already 62.2 kJ mol−1 higher in energy, compare Fig. 2c and d. Attempts to solve this problem by introduction of sterically more demanding ligands at the LA site (Mes vs. Ph) couldn’t prevent the formation of an energetically lower-lying dead-end state, although the energetic difference (ΔE = 42.4 kJ mol−1 at the 6-31+G* level) is somewhat smaller, see Fig. S5 (ESI†). Notably, the problem could indeed be solved employing the larger biphenylene (bip) molecule as spacer group for the two LA parts, see Fig. S7 (ESI†). Due to the large peri-distances in the bip parts, the dicationic (“active”) state of this ligand system does not form any direct (intra-spacer) P–Si peri-contacts, but only an indirect (inter-spacer) contact of 2.503 Å, which likely would not hamper N2-uptake. Moreover, the energetic gain and N–N bond stretching effect is even higher in the bip-variant (ΔE = −210.9 kJ mol−1, d(N,N) = 1.437 Å), but since this ligand system seems hardly accessible by synthetic means, the focus of this DFT study remains on the ace-variant. Experimentally, formation of energetically lower-lying dead-end state(s) might be avoided also for the ace-variant by bubbling the solution with gaseous N2 in the crucial (Si)H hydride abstraction steps. If not, the use of larger spacer groups such as bip is unavoidable.
N triple bond (d(N,N) = 1.091 Å for DFT) by 0.327 Å to the unprecedented value of 1.418 Å, corresponding to a N–N single bond, and releasing 161.6 kJ mol−1 of energy in the (in silico) process, see Table S4 (ESI†). Subsequent hydride uptake of the N2-adduct already breaks the N–N bond, vide infra. However, this inspiring result comes with a major setback, as already the first hydride abstraction from the neutral ligand systems (6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)2(1) to form the monocation [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)(-5-ace-6-SiPh2)]+ (2) with one –Ph2SiH and one –Ph2Si+ group results in a quenched state with a short P–Si contact of 2.361 Å, compare Fig. 2a and b. This is already close to the optimized Si–P peri-distance in Ph2P-ace-Ph2Si+ (d(P,Si) = 2.324 Å) and hints towards a stabilization (or Si–P bond strength) of larger than 150 kJ mol−1, see Fig. S4 (ESI†). Second hydride abstraction to form the [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2]2+ (3a) not only keeps this unfortunate situation (d(P,Si) = 2.393 Å), but exhibits an additional short contact of 2.105 Å between the second Si cation and an arylic C atom, which is bound to the central P atom, see Fig. 2c. A slightly more “active state” (3b, d(P,Si) = 2.428 Å, d(Si,Cπ) = 3.327 Å) in which the LA and LB parts are not interacting that strongly is already 62.2 kJ mol−1 higher in energy, compare Fig. 2c and d. Attempts to solve this problem by introduction of sterically more demanding ligands at the LA site (Mes vs. Ph) couldn’t prevent the formation of an energetically lower-lying dead-end state, although the energetic difference (ΔE = 42.4 kJ mol−1 at the 6-31+G* level) is somewhat smaller, see Fig. S5 (ESI†). Notably, the problem could indeed be solved employing the larger biphenylene (bip) molecule as spacer group for the two LA parts, see Fig. S7 (ESI†). Due to the large peri-distances in the bip parts, the dicationic (“active”) state of this ligand system does not form any direct (intra-spacer) P–Si peri-contacts, but only an indirect (inter-spacer) contact of 2.503 Å, which likely would not hamper N2-uptake. Moreover, the energetic gain and N–N bond stretching effect is even higher in the bip-variant (ΔE = −210.9 kJ mol−1, d(N,N) = 1.437 Å), but since this ligand system seems hardly accessible by synthetic means, the focus of this DFT study remains on the ace-variant. Experimentally, formation of energetically lower-lying dead-end state(s) might be avoided also for the ace-variant by bubbling the solution with gaseous N2 in the crucial (Si)H hydride abstraction steps. If not, the use of larger spacer groups such as bip is unavoidable.
Three different N2-activation modes were obtained by DFT, see parts e and f of Fig. 2, as well as Fig. S8c (ESI†). Notably, the PSiN–NSiP (Fig. 2f) coordination mode of [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2]2+ (4b) is superior to those of P2N–NSi2 of 4a (Fig. 2e) or P–N–N–Si2P of 4c (Fig. S8c, ESI†) modes as it results in significantly lower molecular energies for both investigated structural types (Table S4, ESI†). Apparently, “vertical” through-atom polarization of each N atom within a P–N–Si environment is more effective in weakening the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond than “horizontal” through-bond polarization within a P2/1–N–N–Si2P0/1 environment. In order to investigate this crucial difference in more detail, the electronic bond characteristics of two strongest N2-adducts (4a, 4b) were investigated computationally applying a set of complementary real-space bonding indicators (RSBI), see the following section.
N triple bond than “horizontal” through-bond polarization within a P2/1–N–N–Si2P0/1 environment. In order to investigate this crucial difference in more detail, the electronic bond characteristics of two strongest N2-adducts (4a, 4b) were investigated computationally applying a set of complementary real-space bonding indicators (RSBI), see the following section.
In an attempt to break the strongly activated N–N bond, the most energy efficient N2-adduct (4b, PSiN–NSiP) of the ace-variant was “exposed” to hydride attack by attaching a H− ion at one of eight potential coordination sites, that is above and below the two (SiP)N(N)-planes at N(1) and N(2), as well as attacking the Si atoms from outside (exo) or inside (endo) within their (Ph2)Si(N)-tetrahedrons. The hydride attack resulted in two states, in which the N–N bond was ultimately broken to form one Si–N–P and one Si–N(H)–P fragment (Fig. 3a and b). Note that the H− ion formally underwent an Umpolung in that process. In one of those H− reduced N2-adducts [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2H]+ (5a), the electronic bond characteristics of which are also discussed in the RSBI section, a short intramolecular PSiN–H⋯NSiP hydrogen bond is formed, which lowers the molecular energy considerably. To the contrary, although all four models including a Si–H instead of a N–H bond could be optimized to stable structures by DFT, they are not only energetically strongly unfavorable, but the N–N fixation is merely disturbed in those models, even when the Si–H bond is directed towards the inside of the molecule (endo), thereby breaking one of the two Si–N bonds, see Fig. S9 (ESI†).
Subsequently, the H− reduced N2-adduct including the PSiN–H⋯NSiP hydrogen bond was exposed computationally to proton attack at N(1) or N(2), resulting again in two different models, which now formally comprises the dicationic ligand system, one molecule of N2 and one molecule of H2, see Fig. 3c and d. The energetically lower model is denoted as [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·HN2H]2+ (6a). Stepwise reduction and protonation is a common reaction motif in biological systems. Notably, second H− attack of the H− reduced N2-adduct is energetically disfavored and thus not considered further (Table S4, ESI†).
Fig. 4 displays a provisional reaction scheme based on the ΔE values for the so far applied b3pw91-D3/6-311+G(2df,p) level as well as ΔG values for the reoptimized b3pw91-D3/6-31+G* level structures of the “best models”, that is, the energetically lowest lying structural isomers of each electronic state (see also Tables S8–S10, ESI†). Since all but one reactions in that scheme don’t change the number of involved species and rotational movements are rather limited in the sterically crowded ligand systems, entropic effects were expected and found to be rather small: the largest difference between ΔE and ΔG indeed was found for N2-adduct formation. The neutral ligand system (6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)2 (1) comprising two –SiHPh2 fragments already forms (at least) three structural isomers, depending if the Si–H bond is pointing towards the outside (denoted as “exo”) or inside (denoted as “endo”) of the molecule. As the exo–exo- and endo–endo-variants (see Fig. S6a, b and Table S4, ESI†) are higher in energy, they are not considered further and model (6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)2 (1) thus refers to the exo–endo-state (Fig. 2a). Single hydride abstraction releases considerable amounts of energy (ΔE = −232 kJ mol−1, ΔG = −217 kJ mol−1), because the monocationic model [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2H)(-5-ace-6-SiPh2)]+ (2) forms a short Si–P bond, whereas the second abstraction of the endo-hydride to form the dication [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2]2+ (3a) is almost energy neutral (ΔE = −3 kJ mol−1, ΔG = 6 kJ mol−1). Although N2-adduct formation to form [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2]2+ (4b) is energetically strongly favorable, releasing another 162 kJ (ΔE) or 75 (ΔG) mol−1, the (purple colored) 2 and 3a states are considered as dead-end states, which should be circumvented, e.g. by flushing the solution with gaseous N2via the hydride abstractions, see purple curved arrow in Fig. 4. Conversion of the 1 atm standard state 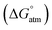 to the 1 M standard state
to the 1 M standard state 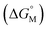 is relevant for the N2-adduct formation step, because the number of moles is changing. Using
is relevant for the N2-adduct formation step, because the number of moles is changing. Using 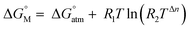 with R1 = 8.31447 J K−1 mol−1, R2 = 0.08206 L atm K−1 mol−1, T = temperature in K, and n = change in number of moles,90 a further free enthalpy expense of 7.9 kJ mol−1 has to be added to the −75 kJ mol−1, leaving −67 kJ mol−1. The basis-set superposition error (BSSE) of 4b was calculated to be 10.1 kJ mol−1, leaving finally −57 kJ mol−1. Once 4b has been formed successfully, it is prone to stepwise attack of adding hydride (ΔE = −243 kJ mol−1, ΔG = −208 kJ mol−1), ultimately splitting the N–N bond [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2H]+ (5a), and subsequent protonation (ΔE = −43 kJ mol−1, ΔG = −48 kJ mol−1) to form [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·HN2H]2+ (6a), which formally comprises the ligand system, one molecule N2 and one molecule H2, as mentioned above. The energetic cost for the heterolytic splitting of H2 to form transferable “H(−)” and “H(+)”, according to the reaction scheme: 1/2B2H6 + Tf(−) + H2 → BH4(−) + TfH (ΔG = 151.8 kJ mol−1) is overcompensated by the summed up energetic gain of adding hydride and subsequent protonation for the first hydrogen equivalent (ΔG = 255.0 kJ mol−1) forming the diazene structure 6a. Subsequent reduction and protonation steps surely will be much less beneficial. Further reactions with H− and H+, however, have not been attempted so far due to the enormous numbers of potential states. The NH3-poisoned states 3a·NH3 and 3a·2NH3 (see Fig. S10, ESI†) were calculated to be 129.2 and 162.2 kJ mol−1 lower in energy (at b3pw91-D3/6-31+G*) than the sum of 3a and one or two NH3 molecules, making it unlikely that the process can be completed to finally release NH3 with this ligand system. The reaction 6a + 2BH4− + 2TfH → 3a·2NH3 + B2H6 + 2Tf− is formally slightly endothermic (ΔG = 27.9 kJ mol−1; b3pw91-D3/6-31+G*).
with R1 = 8.31447 J K−1 mol−1, R2 = 0.08206 L atm K−1 mol−1, T = temperature in K, and n = change in number of moles,90 a further free enthalpy expense of 7.9 kJ mol−1 has to be added to the −75 kJ mol−1, leaving −67 kJ mol−1. The basis-set superposition error (BSSE) of 4b was calculated to be 10.1 kJ mol−1, leaving finally −57 kJ mol−1. Once 4b has been formed successfully, it is prone to stepwise attack of adding hydride (ΔE = −243 kJ mol−1, ΔG = −208 kJ mol−1), ultimately splitting the N–N bond [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2H]+ (5a), and subsequent protonation (ΔE = −43 kJ mol−1, ΔG = −48 kJ mol−1) to form [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·HN2H]2+ (6a), which formally comprises the ligand system, one molecule N2 and one molecule H2, as mentioned above. The energetic cost for the heterolytic splitting of H2 to form transferable “H(−)” and “H(+)”, according to the reaction scheme: 1/2B2H6 + Tf(−) + H2 → BH4(−) + TfH (ΔG = 151.8 kJ mol−1) is overcompensated by the summed up energetic gain of adding hydride and subsequent protonation for the first hydrogen equivalent (ΔG = 255.0 kJ mol−1) forming the diazene structure 6a. Subsequent reduction and protonation steps surely will be much less beneficial. Further reactions with H− and H+, however, have not been attempted so far due to the enormous numbers of potential states. The NH3-poisoned states 3a·NH3 and 3a·2NH3 (see Fig. S10, ESI†) were calculated to be 129.2 and 162.2 kJ mol−1 lower in energy (at b3pw91-D3/6-31+G*) than the sum of 3a and one or two NH3 molecules, making it unlikely that the process can be completed to finally release NH3 with this ligand system. The reaction 6a + 2BH4− + 2TfH → 3a·2NH3 + B2H6 + 2Tf− is formally slightly endothermic (ΔG = 27.9 kJ mol−1; b3pw91-D3/6-31+G*).
 | ||
| Fig. 4 ΔE (b3pw91-D3/6-311+G(2df,p)) and related ΔG (b3pw91-D3/6-31+G*) energy diagrams of the investigated reactions steps. The purple curved arrow indicates a potential shortcut from the neutral ligand system (1, energy value set to zero) to the most stable N2-adduct (4b) circumventing the formation of dead-end species 2 and 3a (colored in purple) by bubbling the solution with N2 gas via two-step hydride abstraction. For clarity, all steps and states after adding N2 are color-coded with respect to the DFT-level (higher level: blue, lower level: red). No transition states included. 1 atm to 1 M and BSSE corrections not included for 4b, see main text. The main difference between ΔE and ΔG stem from thermal corrections, and not from the basis-set level, see Fig. S11 (ESI†) for ΔE at b3pw91-D3/6-31+G*. | ||
Transition-state calculations are not included in the scheme due to the size and complexity of the ligand system, but an attempt was made to evaluate the activation barrier of the N2 molecule being located within the “active site” of 4b by conducting a potential energy scan with N–N distances fixed to values between 1.10 and 1.55 Å, see Fig. S12 (ESI†) (b3pw91-D3/6-31+G*). The observed continuous rise in energy along shorter bond distances suggests that once N2 has entered the active site of the ligand system (assuming no blocking P–Si bonds), it will immediately be stretched to about 1.4 Å.
Real-space bonding indicators
The most relevant states, 3a, 4a, 4b, 5a, and 6a, are electronically characterized applying a complementary set of real-space bonding indicators (RSBIs), which were extracted from the calculated electron densities (ED, ρ(r)) and electron pair densities, following the AIM,72–74 NCI,76 and ELI-D75 methods. Charge redistributions via N2-activation and subsequent N–N bond rupture are monitored by means of AIM atomic and fragmental charges (Table 1 and Table S11, ESI†) and electron populations of (non-)bonding ELI-D basins (Table 2 and Tables S12–S13, ESI†). In the investigated systems N2-uptake is accompanied by tremendous charge accumulation at the N2-site, as each N atom gains more than 1.4 e. The majority of this charge is delivered by the two adjacent P atoms (together more than 2.5 e), whereas the two Si atoms show minor contributions (together about 0.4 e). For the organic fragments (ace spacer and phenyl rings) charge redistributions never exceed 0.1 e and are thus neglected. In fact, the charge redistributions only happen within the Si2P2N2 fragment, which shows a stable summed up charge in 3a, 4a, and 4b, see Table 1 for details. The results highlight the essential role of the two “hard” Si+ ions to drive the activation of the inert N2 molecule as well as the essential role of the two P atoms to serve as “soft” and flexible electron donors, see Fig. S13 (ESI†) for further details. By reducing the N2-site and extending the N–N bond in the process to a value above 1.4 Å, which may be considered as an entatic state, the N–N fragment is well prepared for chemical reduction with a hydride atom and subsequent protonation to form two secondary amine sites. In many metalloproteins, Fe4O4 cubane clusters serve as reversible electron reservoirs for close-by active sites, the role of which is filled by P atoms in the light atom molecules of this study. This might be related to the observation that the boron atom, which on the one side is electron deficient, but on the other side carries non-bonded ED in the pz-orbital, is capable to activate N2 as well.44–48 Notably, 4a and 4b show similar AIM charges despite the qualitatively different P2N–NSi2 and PSiN–NSiP binding modes. Adding a hydride atom to N2-adduct [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2]2+ (4b) thereby splitting the weakened N–N bond and forming a N–H⋯N contact [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2H]+ (5a), alters all fragments in a systematical fashion: whereas the P and Si atoms are slightly more positive charged in 5a, all organic fragments are more negative charged, but with one exception those changes never exceed ±0.1 e. Drastic changes, however, are again obtained for the N(H) and N atoms gaining further 0.75 and 0.50 e, which brings both N atomic charges to values of −2 e or larger. It is thus not of surprise that further chemical reduction with another hydride atom is energetically strongly disfavored, see Table S4 (ESI†). In contrast, subsequent protonation of [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2H]+ (5a) causes the formation of two secondary amines of the SiPNH-type in [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·HN2H]2+ (6a) and affects the charges accordingly.| Q(AIM) | 3a | 4a | 4b | 5a | 6a | 4b–3a | 5a–4b | 6a–5a |
|---|---|---|---|---|---|---|---|---|
| Q AIM(N): 0.00 e (N2), −0.33 e (N2H2), −0.68 e (N2H4), −1.04 e (NH3). | ||||||||
| N(1) | −1.51 | −1.49 | −2.00 | −1.87 | −0.50 | 0.13 | ||
| N(2) | −1.43 | −1.43 | −2.18 | −1.95 | −0.75 | 0.23 | ||
| H(N) | 0.49 | 0.46 | 0.49 | −0.04 | ||||
| H(N) | 0.43 | 0.43 | ||||||
| NxHy | −2.94 | −2.93 | −3.68 | −2.94 | −0.76 | 0.75 | ||
| P(1) | 1.38 | 2.76 | 2.70 | 2.86 | 2.70 | 1.31 | 0.16 | −0.16 |
| P(2) | 1.52 | 2.73 | 2.77 | 2.81 | 2.83 | 1.25 | 0.04 | 0.03 |
| Si(1) | 2.66 | 2.89 | 2.90 | 2.93 | 2.92 | 0.24 | 0.03 | −0.01 |
| Si(2) | 2.74 | 2.90 | 2.89 | 2.95 | 2.91 | 0.14 | 0.06 | −0.04 |
| Si2P2N2Hx | 8.31 | 8.35 | 8.33 | 7.86 | 8.43 | 0.02 | −0.47 | 0.57 |
| ace(1) | −0.71 | −0.81 | −0.76 | −0.87 | −0.77 | −0.05 | −0.11 | 0.10 |
| ace(2) | −1.05 | −1.08 | −1.00 | −1.10 | −1.04 | 0.05 | −0.10 | 0.06 |
| ace(3) | −1.04 | −0.98 | −1.07 | −1.12 | −1.08 | −0.03 | −0.05 | 0.04 |
| ph(1) | −0.44 | −0.40 | −0.41 | −0.47 | −0.41 | 0.03 | −0.06 | 0.06 |
| ph(2) | −0.44 | −0.35 | −0.39 | −0.46 | −0.40 | 0.05 | −0.07 | 0.06 |
| ph(3) | −0.64 | −0.69 | −0.68 | −0.72 | −0.68 | −0.04 | −0.04 | 0.04 |
| ph(4) | −0.66 | −0.68 | −0.66 | −0.71 | −0.68 | 0.00 | −0.05 | 0.03 |
| ph(5) | −0.63 | −0.68 | −0.68 | −0.70 | −0.68 | −0.05 | −0.02 | 0.02 |
| ph(6) | −0.67 | −0.67 | −0.66 | −0.69 | −0.66 | 0.00 | −0.03 | 0.03 |
![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N, N
N, N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) N, and N–N bonds
N, and N–N bonds
| d [Å] | ρ(r)bcp [e Å−3] | ∇2ρ(r)bcp [e Å−5] | ε | d 1/d | RJI [%] | |
|---|---|---|---|---|---|---|
| ρ(r)bcp: ED at the bcp, ∇2ρ(r)bcp: Laplacian, ε: bond ellipticity d1: distance atom(1)-bcp, RJI: Raub-Jansen Index, G/ρ(r)bcp, H/ρ(r)bcp: kinetic and total energy density over ρ(r)bcp ratios, V: potential energy density, NELI, VELI: electron populations and volumes the ELI-D basin, γELI: ELI-D value at the attractor position. | ||||||
| N2 | 1.091 | 4.83 | −73.6 | 0.00 | 0.50 | 50.0 |
| N2H2 | 1.233 | 3.33 | −32.3 | 0.15 | 0.50 | 50.0 |
| 4a | 1.417 | 2.02 | −8.4 | 0.02 | 0.52 | 60.1 |
| 4b | 1.418 | 2.04 | −8.7 | 0.01 | 0.50 | 50.6 |
| N2H4 | 1.428 | 2.05 | −10.1 | 0.00 | 0.50 | 50.0 |
| G/ρ(r)bcp [a.u.] | H/ρ(r)bcp [a.u.] | G/V | N ELI [e] | V ELI [Å3] | γ ELI | |
|---|---|---|---|---|---|---|
| N2 | 0.88 | −1.94 | 0.31 | 3.50 | 6.0 | 1.46 |
| N2H2 | 0.63 | −1.31 | 0.32 | 2.45 | 5.1 | 1.52 |
| 4a | 0.53 | −0.82 | 0.39 | 1.14 | 0.8 | 1.56 |
| 4b | 0.51 | −0.81 | 0.39 | 1.15 | 0.8 | 1.56 |
| N2H4 | 0.45 | −0.80 | 0.36 | 1.33 | 1.0 | 1.54 |
The considerably weakened N–N bonds in [(6-Ph2P-ace-5-)P(-5-ace-6-SiPh2)2·N2]2+ (4a and 4b) are electronically characterized by topological and integrated bond properties (Table 2) and compared to dinitrogen, diazene and hydrazine. With almost 1.42 Å, the N–N bond distances in 4a and 4b are already very close to the N–N single bond of hydrazine (d(N,N) = 1.43 Å). Consequently, the ED at the bond critical point (bcp) is reduced from 4.8 e Å−3 in N2 to 2.0 e Å−3 in 4a, or 4b, resembling the value of 2.1 e Å−3 in hydrazine. This is accompanied by a drastic reduction of the electron populations (NELI) of the corresponding ELI-D N–N bonding basins (V2(N,N)) from 3.5 e in N2 to 1.1–1.2 e in the N2-adducts, the latter being already smaller in numbers than the 1.33 e found in the V2(N,N) basin of hydrazine. Less efficient orbital overlap between the N atoms and thus lower degrees of covalency are reflected by the Laplacian of the ED (∇2ρ(r)bcp) and the total energy density over ED ratio (H/ρ(r)bcp), which both drop down considerably (from negative values towards zero). Since the kinetic energy over ED ratio (G/ρ(r)bcp) is dropping down less “fast” (from positive values towards zero), the ratio between G and the potential energy density (V) increases slightly, hinting towards higher relevance of non-covalent bonding aspects for longer N–N distances. This goes along with slightly increased ELI-D values at the V2(N,N) attractor position (γELI), suggesting higher localizability (i.e. less electron sharing) of the bonding electron pair. Bond ellipticities (ε) of 0.0 prove the cylindrical symmetry of the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond in N2 and the N–N single bonds in 4a, 4b, and hydrazine, whereas for the N
N triple bond in N2 and the N–N single bonds in 4a, 4b, and hydrazine, whereas for the N![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) N double bond in azene, the symmetry is slightly disturbed (ε = 0.15), as expected. For the small reference models, the N atoms are indistinguishable, which causes the bcp to be located exactly at the middle of the N–N bond, i.e. d1/d = 0.5. Based on the same grounds, the Raub-Jansen Index (RJI91), which determines how electron populations with an ELI-D basin are distributed over adjacent AIM atomic basins, is 50%. For the symmetrical 4b (PSiN–NSiP) this is also valid, but slight distortions are observed for the less symmetrical 4a (P2N–NSi2), vide infra. The lone-pairs of the N atoms accommodate for charge balancing, but their ELI-D basins show a surprising trend for the investigated ligand systems (Table S12, ESI†): for N2, N2H2, N2H4, and NH3, there is a clear trend of decreasing electron populations with decreasing s-character, e.g. 3.14 e in N2 (sp-like) to 2.14 e in NH3 (sp3-like). However, in models 4a, 4b, 5a, and 6a, flatter SixPyNE (E = N, H) planes (more “sp2”-like) cause lower NELI values, whereas more tetrahedral shaped planes (more “sp3”-like) cause higher NELI-values. An exception is the “azide”-like N(2) atom in 5a, which might be considered as “sp2-like, and the lone-pair basin of which carries 2.9 e.
N double bond in azene, the symmetry is slightly disturbed (ε = 0.15), as expected. For the small reference models, the N atoms are indistinguishable, which causes the bcp to be located exactly at the middle of the N–N bond, i.e. d1/d = 0.5. Based on the same grounds, the Raub-Jansen Index (RJI91), which determines how electron populations with an ELI-D basin are distributed over adjacent AIM atomic basins, is 50%. For the symmetrical 4b (PSiN–NSiP) this is also valid, but slight distortions are observed for the less symmetrical 4a (P2N–NSi2), vide infra. The lone-pairs of the N atoms accommodate for charge balancing, but their ELI-D basins show a surprising trend for the investigated ligand systems (Table S12, ESI†): for N2, N2H2, N2H4, and NH3, there is a clear trend of decreasing electron populations with decreasing s-character, e.g. 3.14 e in N2 (sp-like) to 2.14 e in NH3 (sp3-like). However, in models 4a, 4b, 5a, and 6a, flatter SixPyNE (E = N, H) planes (more “sp2”-like) cause lower NELI values, whereas more tetrahedral shaped planes (more “sp3”-like) cause higher NELI-values. An exception is the “azide”-like N(2) atom in 5a, which might be considered as “sp2-like, and the lone-pair basin of which carries 2.9 e.
RSBIs for the Si–N and P–N bonds in 4a, 4b, 5a, and 6a are collected in Table S13 (ESI†). Both bond types become shorter via breaking the N–N bond and forming the secondary amines, accompanied by increased ρ(r)bcp and NELI values. Notably, H/ρ(r)bcp remains unaffected, whereas the already dominating G/ρ(r)bcp as well as ∇2ρ(r)bcp rise to more positive values, indicating essentially increased ionic bond contributions in the Si–N and P–N bonds of 5a and 6a. Whereas the P–N bonds can be considered as polarized-covalent, as indicated by a RJI of about 82%, the Si–N bonds are already at the border of dative bonds (RJI larger than 92%).
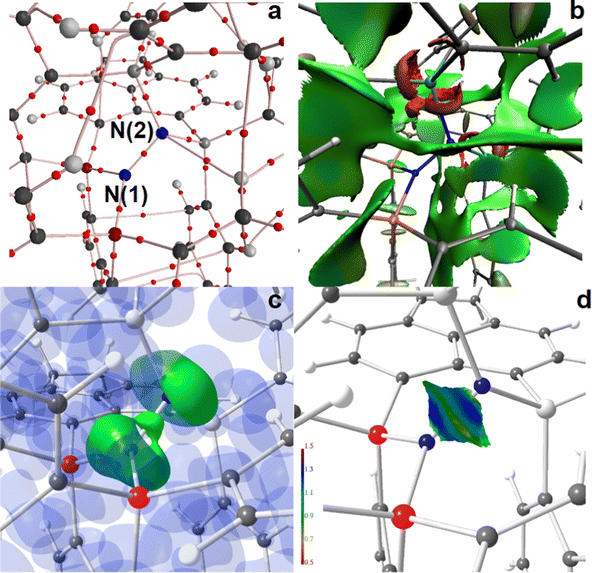
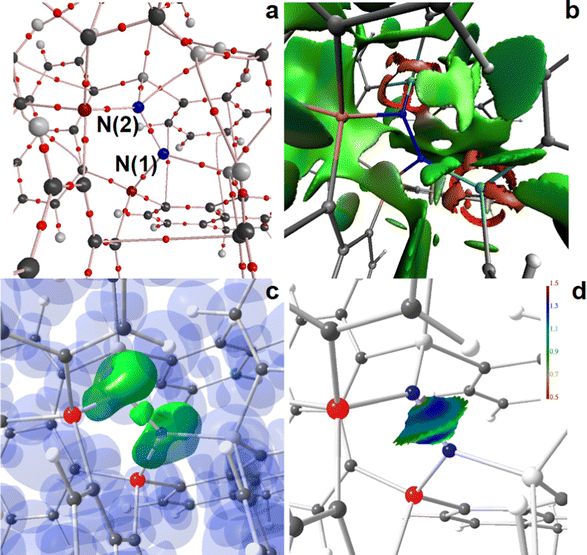
The AIM bond topology as well as NCI and ELI-D basin distributions of the “active site” areas of the two N2-adducts, 4a and 4b, are displayed in Fig. 5 and 6; full molecule representations and the results for 5a and 6a are comprised in the ESI,† (Fig. S14–S19). For both, 4a and 4b, AIM topology reveals only one long and thus weak secondary C–H⋯N contact at the N2-site, see parts a of Fig. 5 and 6, suggesting that this site is fully determined by the primary N–N, Si–N, and P–N bonds. Also the NCI, which is even more sensitive to the formation of weak non-covalent interactions, and shows extended flat greenish basins reflecting numerous attractive H⋯H, H⋯Cπ, and Cπ⋯Cπ contacts within the molecules, gives no hint for non-covalent bond contributions within the active site, including the N2- and P–N-bonds, which are dominated by covalent bond contributions, see parts b of Fig. 5 and 6. Ring-shaped and red-colored NCI-basins around the Si atoms confirm the relevance of non-covalent bonding aspects to the Si–N and Si–C bonds. Parts c and d of Fig. 5 and 6 display color-coded ELI-D iso-surface representations and the ELI-D distribution mapped on the V2(N,N) basin, respectively. For clarity, V2(N,N) and V1(N) (lone-pair basins) are given in solid-green for the iso-surfaces, whereas V2(Si,N), V2(P,N), and the remaining basin types are given in transparent-blue (Fig. 5c and 6c). At an iso-value of γ = 1.35, the V2(N,N) bonding basin is still topologically connected with the two V1(N(2)) basins of the P2N-site in 4a, but already separated from the V1(N(1)) basin of the Si2N-site. In contrast, V2(N,N) is well separated from both SiPN-sites in 4b. Apparently, the less symmetric N2-coordination mode in 4a induces the N–N bonding electron pair to move towards N(1), which results in a RJI of 60% (see above), i.e. 60% of the electron population of V2(N,N) is located within the AIM atomic basin of N(1)P2, and 40% in the basin of N(2)Si2. Nevertheless, since the differences between P2N–NSi2 and PSiN–NSiP coordination modes are not tremendous, the size and shape of V2(N,N) as well as the ELI-D distribution mapped on its surface is very much the same (Fig. 5d and 6d).
N–H⋯N bond formation further separates both N atoms in 5a and 6a, see Fig. S16–S19 (ESI†). The new secondary contact within the active site is reflected by an (N)H⋯N bcp as well as by a flat green or bluish NCI basin between the two N atoms, and it leaves a concave impression on V1(N) with slightly increased ELI-D values in the cavity area (parts d of Fig. S16–S19, ESI†).
Conclusion
The DFT study shows that – at least in silico – the inert N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N triple bond can be cleaved by molecular compounds containing only the earth abundant elements C, H, Si, and P. The combination of two silyl cations as Lewis acidic sites with the lone-pairs of two phosphorus atoms as Lewis basic sites in a flexible but not too flexible spatial arrangement thereby proved to be essential to energy efficiently drive N2-uptake, activation and finally splitting. However, the reaction path can’t be proceeded to liberate of NH3 molecules due to formation of very strong H3N–Si(+) bonds in the final steps. Nevertheless, the proposed ligand systems may serve as blueprint for related systems and approaches. Especially the role of the P atoms as “soft” electron donors should be highlighted in this respect. Different modes of N2-bonding (e.g. P2N–NSi2vs. PSiN–NSiP coordination) were encountered and examined by means of real-space bonding indicators derived from the calculated electron and electron pair densities. Minute effects of charge rearrangements via the different reaction steps were monitored by AIM atomic and fragmental charges and by electron populations of bonding and non-bonding ELI-D basins. Via N2-uptake, the N2 lone pairs (LP(N)) donate into the LA centers, and electron population is transferred from the N
N triple bond can be cleaved by molecular compounds containing only the earth abundant elements C, H, Si, and P. The combination of two silyl cations as Lewis acidic sites with the lone-pairs of two phosphorus atoms as Lewis basic sites in a flexible but not too flexible spatial arrangement thereby proved to be essential to energy efficiently drive N2-uptake, activation and finally splitting. However, the reaction path can’t be proceeded to liberate of NH3 molecules due to formation of very strong H3N–Si(+) bonds in the final steps. Nevertheless, the proposed ligand systems may serve as blueprint for related systems and approaches. Especially the role of the P atoms as “soft” electron donors should be highlighted in this respect. Different modes of N2-bonding (e.g. P2N–NSi2vs. PSiN–NSiP coordination) were encountered and examined by means of real-space bonding indicators derived from the calculated electron and electron pair densities. Minute effects of charge rearrangements via the different reaction steps were monitored by AIM atomic and fragmental charges and by electron populations of bonding and non-bonding ELI-D basins. Via N2-uptake, the N2 lone pairs (LP(N)) donate into the LA centers, and electron population is transferred from the N![[triple bond, length as m-dash]](https://www.rsc.org/images/entities/char_e002.gif) N bonding ELI-D basins towards newly formed LP(N) basins. The majority of the electron population within the newly formed P–N bonding basins (stemming from the formerly LP(P) basins of the ligand system) is located within the AIM atomic basins of the N atoms, considerably increasing their charge from zero in gaseous N2 to ca. −1.5 e in the N2-adducts. The tremendous weakening of the N–N bond (d(N,N) = 1.4 Å vs. 1.1 Å in gaseous N2) makes it prone to subsequent addition of hydride ions and protonation to form two secondary amines. A critical point for this class of peri-substituted compounds remains the potential formation of dead-end states, in which either the LA and LB prematurely form a strong dative bond or the LA and LB sites are too far away from each other to fix N2. Fine tuning of the relevant distances by combination of suitable spacer molecules, such as (ace)naphthyl, biphenylene, xanthene, or dibenzofuran with (bulky) ligand groups attached to the LA and LB atoms, such as methyl, iso-propyl, tert-butyl, phenyl, or mesityl, as well as bubbling the solution with N2 gas via hydride abstraction, may solve this problem, but not all combinations might be accessible by synthetic means in the laboratory. Practical work in the laboratory also has to show, if the bulky ligand hamper access of gaseous N2 to the active site. In silico work is ongoing in order to optimize the ligand system towards energy efficiency and practical accessibility and towards extraction of ΔG and ΔS values of all reaction states to get complete and reliable reaction schemes including transition states.
N bonding ELI-D basins towards newly formed LP(N) basins. The majority of the electron population within the newly formed P–N bonding basins (stemming from the formerly LP(P) basins of the ligand system) is located within the AIM atomic basins of the N atoms, considerably increasing their charge from zero in gaseous N2 to ca. −1.5 e in the N2-adducts. The tremendous weakening of the N–N bond (d(N,N) = 1.4 Å vs. 1.1 Å in gaseous N2) makes it prone to subsequent addition of hydride ions and protonation to form two secondary amines. A critical point for this class of peri-substituted compounds remains the potential formation of dead-end states, in which either the LA and LB prematurely form a strong dative bond or the LA and LB sites are too far away from each other to fix N2. Fine tuning of the relevant distances by combination of suitable spacer molecules, such as (ace)naphthyl, biphenylene, xanthene, or dibenzofuran with (bulky) ligand groups attached to the LA and LB atoms, such as methyl, iso-propyl, tert-butyl, phenyl, or mesityl, as well as bubbling the solution with N2 gas via hydride abstraction, may solve this problem, but not all combinations might be accessible by synthetic means in the laboratory. Practical work in the laboratory also has to show, if the bulky ligand hamper access of gaseous N2 to the active site. In silico work is ongoing in order to optimize the ligand system towards energy efficiency and practical accessibility and towards extraction of ΔG and ΔS values of all reaction states to get complete and reliable reaction schemes including transition states.
Author contributions
S. M.: conceptual design, DFT calculations and analysis, manuscript writing. J. B.: conceptual design, critical revision of the article.Conflicts of interest
The authors declare no conflict of interest.Acknowledgements
Dr Emanuel Hupf is greatly acknowledged for software support and critically reading the manuscript. The DFG is acknowledged for funding.References
-
R. E. Blankenship, Molecular mechanisms of photosynthesis, John Wiley & Sons, 3rd edn, 2021 Search PubMed
.
- W. Lubitz, H. Ogata, O. Rudiger and E. Reijerse, Hydrogenases, Chem. Rev., 2014, 114(8), 4081–4148 CrossRef CAS PubMed
.
- M. Wikstrom, K. Krab and V. Sharma, Oxygen Activation and Energy Conservation by Cytochrome c Oxidase, Chem. Rev., 2018, 118(5), 2469–2490 CrossRef CAS PubMed
.
- K. Schroder, NADPH oxidases: Current aspects and tools, Redox Biol., 2020, 34, 101512 CrossRef PubMed
.
- A. M. Appel, J. E. Bercaw, A. B. Bocarsly, H. Dobbek, D. L. DuBois, M. Dupuis, J. G. Ferry, E. Fujita, R. Hille, P. J. A. Kenis, C. A. Kerfeld, R. H. Morris, C. H. F. Peden, A. R. Portis, S. W. Ragsdale, T. B. Rauchfuss, J. N. H. Reek, L. C. Seefeldt, R. K. Thauer and G. L. Waldrop, Frontiers, Opportunities, and Challenges in Biochemical and Chemical Catalysis of CO2 Fixation, Chem. Rev., 2013, 113(8), 6621–6658 CrossRef CAS PubMed
.
- B. M. Hoffman, D. Lukoyanov, Z. Y. Yang, D. R. Dean and L. C. Seefeldt, Mechanism of Nitrogen Fixation by Nitrogenase: The Next Stage, Chem. Rev., 2014, 114(8), 4041–4062 CrossRef CAS PubMed
.
- N. Gruber and J. N. Galloway, An Earth-system perspective of the global nitrogen cycle, Nature, 2008, 451(7176), 293–296 CrossRef CAS PubMed
.
- R. Schlogl, Heterogeneous Catalysis, Angew. Chem., Int. Ed., 2015, 54(11), 3465–3520 CrossRef PubMed
.
- V. Kyriakou, I. Garagounis, A. Vourros, E. Vasileiou and M. Stoukides, An Electrochemical Haber–Bosch Process, Joule, 2020, 4(1), 142–158 CrossRef CAS
.
- S. Yadav, S. Saha and S. S. Sen, Compounds with Low-Valent p-Block Elements for Small Molecule Activation and Catalysis, ChemCatChem, 2016, 8(3), 486–501 CrossRef CAS
.
- W. Zhang, W. Z. Lai and R. Cao, Energy-Related Small Molecule Activation Reactions: Oxygen Reduction and Hydrogen and Oxygen Evolution Reactions Catalyzed by Porphyrin- and Corrole-Based Systems, Chem. Rev., 2017, 117(4), 3717–3797 CrossRef CAS PubMed
.
- R. Saha and P. K. Chattaraj, Activation of Small Molecules (H-2, CO2, N2O, CH4, and C6H6) by a Porphyrinoid-Based Dimagnesium(I) Complex, an Electride, Acs Omega, 2018, 3(12), 17199–17211 CrossRef CAS PubMed
.
- F. Moller, S. Piontek, R. G. Miller and U. P. Apfel, From Enzymes to Functional Materials-Towards Activation of Small Molecules, Chem. – Eur. J., 2018, 24(7), 1471–1493 CrossRef PubMed
.
- R. L. Melen, Frontiers in molecular p-block chemistry: From structure to reactivity, Science, 2019, 363(6426), 479–484 CrossRef CAS PubMed
.
- L. J. Taylor and D. L. Kays, Low-coordinate first-row transition metal complexes in catalysis and small molecule activation, Dalton Trans., 2019, 48(33), 12365–12381 RSC
.
- C. Weetman, A. Porzelt, P. Bag, F. Hanusch and S. Inoue, Dialumenes - aryl vs. silyl stabilisation for small molecule activation and catalysis, Chem. Sci., 2020, 11(18), 4817–4827 RSC
.
- H. T. Lei, X. L. Li, J. Meng, H. Q. Zheng, W. Zhang and R. Cao, Structure Effects of Metal Corroles on Energy-Related Small Molecule Activation Reactions, ACS Catal., 2019, 9(5), 4320–4344 CrossRef CAS
.
- E. H. Edwards and K. L. Bren, Light-driven catalysis with engineered enzymes and biomimetic systems, Biotechnol. Appl. Biochem., 2020, 67(4), 463–483 CrossRef CAS PubMed
.
- A. C. Ghosh, C. Duboc and M. Gennari, Synergy between metals for small molecule activation: Enzymes and bio-inspired complexes, Coordin. Chem. Rev., 2021, 428, 213606 CrossRef CAS
.
- L. Marchetti and M. Levine, Biomimetic Catalysis, ACS Catal., 2011, 1(9), 1090–1118 CrossRef CAS
.
- IEDT, https://www.iipinetwork.org/wp-content/Ietd/content/ammonia.html.
- Q. Wang and D. Astruc, State of the Art and Prospects in Metal-Organic Framework (MOF)-Based and MOF-Derived Nanocatalysis, Chem. Rev., 2020, 120(2), 1438–1511 CrossRef CAS PubMed
.
- S. S. Liu, M. F. Wang, T. Qian, H. Q. Ji, J. Liu and C. L. Yan, Facilitating nitrogen accessibility to boron-rich covalent organic frameworks via electrochemical excitation for efficient nitrogen fixation, Nat. Commun., 2019, 10, 3898 CrossRef PubMed
.
- J. X. Zhao and Z. F. Chen, Single Mo Atom Supported on Defective Boron Nitride Monolayer as an Efficient Electrocatalyst for Nitrogen Fixation: A Computational Study, J. Am. Chem. Soc., 2017, 139(36), 12480–12487 CrossRef CAS PubMed
.
- X. Mao, S. Zhou, C. Yan, Z. H. Zhu and A. J. Du, A single boron atom doped boron nitride edge as a metal-free catalyst for N-2 fixation, Phys. Chem. Chem. Phys., 2019, 21(3), 1110–1116 RSC
.
- C. V. S. Kumar and V. Subramanian, Can boron antisites of BNNTs be an efficient metal-free catalyst for nitrogen fixation? - A DFT investigation, Phys. Chem. Chem. Phys., 2017, 19(23), 15377–15387 RSC
.
- W. B. Qiu, X. Y. Xie, J. D. Qiu, W. H. Fang, R. P. Liang, X. Ren, X. Q. Ji, G. W. Cui, A. M. Asiri, G. L. Cui, B. Tang and X. P. Sun, High-performance artificial nitrogen fixation at ambient conditions using a metal-free electrocatalyst, Nat. Commun., 2018, 9, 3485 CrossRef PubMed
.
- M. Zheng, Y. Li, K. N. Ding, Y. F. Zhang, W. K. Chen and W. Lin, Nitrogen fixation on metal-free SiC(111) polar surfaces, J. Mater. Chem. A, 2020, 8(15), 7412–7421 RSC
.
- M. Zafari, D. Kumar, M. Umer and K. S. Kim, Machine learning-based high throughput screening for nitrogen fixation on boron-doped single atom catalysts, J. Mater. Chem. A, 2020, 8(10), 5209–5216 RSC
.
- S. B. Tang, Q. Dang, T. Y. Liu, S. Y. Zhang, Z. G. Zhou, X. K. Li, X. J. Wang, E. Sharman, Y. Luo and J. Jiang, Realizing a Not-Strong-Not-Weak Polarization Electric Field in Single-Atom Catalysts Sandwiched by Boron Nitride and Graphene Sheets for Efficient Nitrogen Fixation, J. Am. Chem. Soc., 2020, 142(45), 19308–19315 CrossRef CAS PubMed
.
- P. Sun, J. Huang, F. Xu, J. J. Xu, T. Q. Lin, W. Zhao, W. J. Dong and F. Q. Huang, Boron-Induced Nitrogen Fixation in 3D Carbon Materials for Supercapacitors, ACS Appl. Mater. Interfaces, 2020, 12(25), 28075–28082 CrossRef CAS PubMed
.
- B. Liu, Y. P. Zheng, H. Q. Peng, B. F. Ji, Y. Yang, Y. B. Tang, C. S. Lee and W. J. Zhang, Nanostructured and Boron-Doped Diamond as an Electrocatalyst for Nitrogen Fixation, ACS Energy Lett., 2020, 5(8), 2590–2596 CrossRef CAS
.
- C. W. Liu, Q. Y. Li, C. Z. Wu, J. Zhang, Y. G. Jin, D. R. MacFarlane and C. H. Sun, Single-Boron Catalysts for Nitrogen Reduction Reaction, J. Am. Chem. Soc., 2019, 141(7), 2884–2888 CrossRef CAS PubMed
.
- T. Y. Liu, Q. Dang, X. H. Zhou, J. Li, Z. Ge, H. Che, S. B. Tang, Y. Luo and J. Jiang, Synergistic Effect of Boron Nitride and Carbon Domains in Boron Carbide Nitride Nanotube Supported Single-Atom Catalysts for Efficient Nitrogen Fixation, Chem. – Eur. J., 2021, 27(23), 6945–6953 CrossRef CAS PubMed
.
- S. F. Ye, E. Bill and F. Neese, Electronic Structures of the [Fe(N-2)(SiP3iPr)](+1/0/-1) Electron Transfer Series: A Counterintuitive Correlation between
Isomer Shifts and Oxidation States, Inorg. Chem., 2016, 55(7), 3468–3474 CrossRef CAS PubMed
.
- B. D. Matson and J. C. Peters, Fe-Mediated HER vs N2RR: Exploring Factors That Contribute to Selectivity in (P3Fe)-Fe-E(N-2) (E = B, Si, C) Catalyst Model Systems, ACS Catal., 2018, 8(2), 1448–1455 CrossRef CAS PubMed
.
- M. P. Shaver and M. D. Fryzuk, Activation of molecular nitrogen: Coordination, cleavage and functionalization of N-2 mediated by metal complexes, Adv. Synth. Catal., 2003, 345(9–10), 1061–1076 CrossRef CAS
.
- J. B. Lu, X. L. Wang, J. Q. Wang, J. C. Liu, H. Xiao and J. Li, Efficient Nitrogen Fixation via a Redox-Flexible Single-Iron Site with Reverse-Dative Iron -> Boron sigma Bonding, J. Phys. Chem. A, 2018, 122(18), 4530–4537 CrossRef CAS PubMed
.
- A. Eizawa, K. Arashiba, H. Tanaka, S. Kuriyama, Y. Matsuo, K. Nakajima, K. Yoshizawa and Y. Nishibayashi, Remarkable catalytic activity of dinitrogen-bridged dimolybdenum complexes bearing NHC-based PCP-pincer ligands toward nitrogen fixation, Nat. Commun., 2017, 8, 14874 CrossRef CAS PubMed
.
- Y. Ashida and Y. Nishibayashi, Catalytic conversion of nitrogen molecule into ammonia using molybdenum complexes under ambient reaction conditions, Chem. Commun., 2021, 57(10), 1176–1189 RSC
.
- J. Fajardo and J. C. Peters, Tripodal (P3Fe)-Fe-X-N-2 Complexes (X = B, Al, Ga): Effect of the Apical Atom on Bonding, Electronic Structure, and Catalytic N-2-to-NH3 Conversion, Inorg. Chem., 2021, 60(2), 1221–1228 CrossRef PubMed
.
- C. N. Tang, Q. M. Liang, A. R. Jupp, T. C. Johnstone, R. C. Neu, D. T. Song, S. Grimme and D. W. Stephan, 1,1-Hydroboration and a Borane Adduct of Diphenyldiazomethane: A Potential Prelude to FLP-N-2 Chemistry, Angew. Chem., Int. Ed., 2017, 56(52), 16588–16592 CrossRef CAS PubMed
.
- R. L. Melen and A. Step, Closer to Metal-Free Dinitrogen Activation: A New Chapter in the Chemistry of Frustrated Lewis Pairs, Angew. Chem., Int. Ed., 2018, 57(4), 880–882 CrossRef CAS PubMed
.
- A. J. Ruddy, D. M. C. Ould, P. D. Newman and R. L. Melen, Push and pull: the potential role of boron in N-2 activation, Dalton Trans., 2018, 47(31), 10377–10381 RSC
.
- C. Hering-Junghans, Metal-Free Nitrogen Fixation at Boron, Angew. Chem., Int. Ed., 2018, 57(23), 6738–6740 CrossRef CAS PubMed
.
- M. A. Legare, G. Belanger-Chabot, R. D. Dewhurst, E. Welz, I. Krummenacher, B. Engels and H. Braunschweig, Nitrogen fixation and reduction at boron, Science, 2018, 359(6378), 896–899 CrossRef CAS PubMed
.
- M. A. Legare, C. Pranckevicius and H. Braunschweig, Metallomimetic Chemistry of Boron, Chem. Rev., 2019, 119(14), 8231–8261 CrossRef CAS PubMed
.
- M. A. Legare, M. Rang, G. Belanger-Chabot, J. I. Schweizer, I. Krummenacher, R. Bertermann, M. Arrowsmith, M. C. Holthausen and H. Braunschweig, The reductive coupling of dinitrogen, Science, 2019, 363(6433), 1329–1332 CrossRef CAS PubMed
.
- D. W. Stephan, Discovery of Frustrated Lewis Pairs: Intermolecular FLPs for Activation of Small Molecules, Top. Curr. Chem., 2013, 332, 1–44 CrossRef CAS PubMed
.
- D. W. Stephan, FRUSTRATED LEWIS PAIRS A metal-free landmark, Nat. Chem., 2014, 6(11), 952–953 CrossRef CAS PubMed
.
- D. W. Stephan, Frustrated Lewis Pairs, J. Am. Chem. Soc., 2015, 137(32), 10018–10032 CrossRef CAS PubMed
.
- D. W. Stephan, Frustrated Lewis Pairs: From Concept to Catalysis, Acc. Chem. Res., 2015, 48(2), 306–316 CrossRef CAS PubMed
.
- F. G. Fontaine and D. W. Stephan, On the concept of frustrated Lewis pairs, Philos. T. R. Soc. A, 2017, 375, 20170004 CrossRef PubMed
.
- D. W. Stephan and G. Erker, Frustrated Lewis pair chemistry, Philos. T. R. Soc. A, 2017, 375, 20170239 CrossRef PubMed
.
- A. R. Jupp and D. W. Stephan, New Directions for Frustrated Lewis Pair Chemistry, Trends Chem., 2019, 1(1), 35–48 CrossRef CAS
.
- G. Menard and D. W. Stephan, Room Temperature Reduction of CO2 to Methanol by Al-Based Frustrated Lewis Pairs and Ammonia Borane, J. Am. Chem. Soc., 2010, 132(6), 1796–1797 CrossRef CAS PubMed
.
- D. W. Stephan and G. Erker, Frustrated Lewis Pairs: Metal-free Hydrogen Activation and More, Angew. Chem., Int. Ed., 2010, 49(1), 46–76 CrossRef CAS PubMed
.
- L. Greb, P. Ona-Burgos, B. Schirmer, S. Grimme, D. W. Stephan and J. Paradies, Metal-free Catalytic Olefin Hydrogenation: Low-Temperature H2 Activation by Frustrated Lewis Pairs, Angew. Chem., Int. Ed., 2012, 51(40), 10164–10168 CrossRef CAS PubMed
.
- S. A. Weicker and D. W. Stephan, Activation of Carbon Dioxide by Silyl Triflate-Based Frustrated Lewis Pairs, Chem. – Eur. J., 2015, 21(37), 13027–13034 CrossRef CAS PubMed
.
- A. I. Briceno-Strocchia, T. C. Johnstone and D. W. Stephan, Using frustrated Lewis pairs to explore C-F bond activation, Dalton Trans., 2020, 49(4), 1319–1324 RSC
.
- G. Avci, S. Velioglu and S. Keskin, In Silico Design of Metal Organic Frameworks with Enhanced CO2 Separation Performances: Role of Metal Sites, J. Phys. Chem. C, 2019, 123(46), 28255–28265 CrossRef CAS
.
- G. H. Gu, C. Choi, Y. Lee, A. B. Situmorang, J. Noh, Y. H. Kim and Y. Jung, Progress in Computational and Machine-Learning Methods for Heterogeneous Small-Molecule Activation, Adv. Mater., 2020, 32(35), 1907865 CrossRef CAS PubMed
.
- J. Beckmann, T. G. Do, S. Grabowsky, E. Hupf, E. Lork and S. Mebs,
peri-Interactions in 8-Diphenylphosphino-1-bromonaphthalene, 6-Diphenylphosphino-5-bromoacenaphthene, and Derivatives, Z. Anorg. Allg. Chem., 2013, 639(12-13), 2233–2249 CrossRef CAS
.
- J. Beckmann, E. Hupf, E. Lork and S. Mebs,
peri-Substituted (Ace)Naphthylphosphinoboranes. (Frustrated) Lewis Pairs, Inorg. Chem., 2013, 52(20), 11881–11888 CrossRef CAS PubMed
.
- E. Hupf, E. Lork, S. Mebs and J. Beckmann, Intramolecularly Coordinated (6-(Diphenylphosphino)acenaphth-5-yl)stannanes. Repulsion vs Attraction of P- and Sn-Containing Substituents in the peri Positions, Organometallics, 2014, 33(10), 2409–2423 CrossRef CAS
.
- E. Hupf, E. Lork, S. Mebs and J. Beckmann, Sterically Congested 5-Diphenylphosphinoacenaphth-6-yl-silanes and -silanols, Organometallics, 2015, 34(15), 3873–3887 CrossRef CAS
.
- S. Furan, E. Hupf, E. Lork, S. Mebs and J. Beckmann, Insights into Frustrated and Regular peri-Substituted (Ace-)Naphthylaminoboranes and (Ace-)Naphthylphosphinoboranes, Eur. J. Inorg. Chem., 2017,(27), 3302–3311 CrossRef CAS
.
- E. Hupf, M. Olaru, C. I. Rat, M. Fugel, C. B. Hubschle, E. Lork, S. Grabowsky, S. Mebs and J. Beckmann, Mapping the Trajectory of Nucleophilic Substitution at Silicon Using a peri-Substituted Acenaphthyl Scaffold, Chem. – Eur. J., 2017, 23(44), 10568–10579 CrossRef CAS PubMed
.
- F. Kutter, A. Denhof, E. Lork, S. Mebs and J. Beckmann, 1,8-Bis(diphenylphosphino)biphenylene. A new ligand for late transition metal complexes, Z. Kristallogr. – Cryst. Mater., 2018, 233(9-10), 627–639 CrossRef CAS
.
- F. Kutter, E. Lork, S. Mebs and J. Beckmann, Intramolecular P-H center dot center dot center dot H-Si Dihydrogen Bonding in the 5-Dimethylsily1-9,9-dimethylxanthen-4-yl-diphenylphosphonium Cation, Organometallics, 2018, 37(22), 4287–4296 CrossRef CAS
.
- V. Balasubramaniyan,
peri Interaction in Naphthalene Derivatives, Chem. Rev., 1966, 66(6), 567–641 CrossRef CAS
.
- R. F. W. Bader, Subspace Quantum-Mechanics - Quantum Definition of an Atom in a Molecule, Bull. Am. Phys. Soc., 1977, 22(3), 305–306 Search PubMed
.
- R. F. W. Bader and T. T. Nguyendang, Quantum-Theory of Atoms in Molecules - Dalton Revisited, Adv. Quantum Chem., 1981, 14, 63–124 CrossRef CAS
.
- R. F. W. Bader, Atoms in Molecules, Acc. Chem. Res., 1985, 18(1), 9–15 CrossRef CAS
.
- M. Kohout, A measure of electron localizability, Int. J. Quantum Chem., 2004, 97(1), 651–658 CrossRef CAS
.
- E. R. Johnson, S. Keinan, P. Mori-Sanchez, J. Contreras-Garcia, A. J. Cohen and W. T. Yang, Revealing Noncovalent Interactions, J. Am. Chem. Soc., 2010, 132(18), 6498–6506 CrossRef CAS PubMed
.
- J. R. Lane, J. Contreras-Garcia, J. P. Piquemal, B. J. Miller and H. G. Kjaergaard, Are Bond Critical Points Really Critical for Hydrogen Bonding?, J. Chem. Theory Comput., 2013, 9(8), 3263–3266 CrossRef CAS PubMed
.
- S. Mebs, Complex modes of bonding: NCI/ELI-D vs. DORI surface analyses of hapticities and hydrogen-hydrogen contacts in zincocene related compounds, Chem. Phys. Lett., 2016, 651, 172–177 CrossRef CAS
.
- A. D. Becke, A New Mixing of Hartree-Fock and Local Density-Functional Theories, J. Chem. Phys., 1993, 98(2), 1372–1377 CrossRef CAS
.
- J. P. Perdew, J. A. Chevary, S. H. Vosko, K. A. Jackson, M. R. Pederson, D. J. Singh and C. Fiolhais, Atoms, Molecules, Solids, and Surfaces - Applications of the Generalized Gradient Approximation for Exchange and Correlation, Phys. Rev. B: Condens. Matter Mater. Phys., 1992, 46(11), 6671–6687 CrossRef CAS PubMed
.
-
M. J. T. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, G. A. Petersson, H. Nakatsuji, X. Li, M. Caricato, A. V. Marenich, J. Bloino, B. G. Janesko, R. Gomperts, B. Mennucci, H. P. Hratchian, J. V. Ortiz, A. F. Izmaylov, J. L. Sonnenberg, D. Williams-Young, F. Ding, F. Lipparini, F. Egidi, J. Goings, B. Peng, A. Petrone, T. Henderson, D. Ranasinghe, V. G. Zakrzewski, J. Gao, N. Rega, G. Zheng, W. Liang, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, K. Throssell, J. A. Montgomery, J. E. Jr. Peralta, F. Ogliaro, M. J. Bearpark, J. J. Heyd, E. N. Brothers, K. N. Kudin, V. N. Staroverov, T. A. Keith, R. Kobayashi, J. Normand, K. Raghavachari, A. P. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, J. M. Millam, M. Klene, C. Adamo, R. Cammi, J. W. Ochterski, R. L. Martin, K. Morokuma, O. Farkas, J. B. Foresman and D. J. Fox, Gaussian 16, C.01, Gaussian, Inc., Wallingford CT, 2016 Search PubMed
.
- S. Grimme, Semiempirical GGA-type density functional constructed with a long-range dispersion correction, J. Comput. Chem., 2006, 27(15), 1787–1799 CrossRef CAS PubMed
.
- S. Miertus, E. Scrocco and J. Tomasi, Electrostatic Interaction of a Solute with a Continuum - a Direct Utilization of Abinitio Molecular Potentials for the Prevision of Solvent Effects, Chem. Phys., 1981, 55(1), 117–129 CrossRef CAS
.
- F. Biegler-Konig, J. Schonbohm and D. Bayles, Software news and updates - AIM2000 - A program to analyze and visualize atoms in molecules, J. Comput. Chem., 2001, 22(5), 545–559 CrossRef
.
-
M. Kohout, DGRID, 4.6, 2015
.
- J. Contreras-Garcia, E. R. Johnson, S. Keinan, R. Chaudret, J. P. Piquemal, D. N. Beratan and W. T. Yang, NCIPLOT: A Program for Plotting Noncovalent Interaction Regions, J. Chem. Theory Comput., 2011, 7(3), 625–632 CrossRef CAS PubMed
.
-
G. A. Andirenko, ChemCraft, 1.8; CrowdStrike: https://www.chemcraftprog.com
.
- W. Humphrey, A. Dalke and K. Schulten, VMD: Visual molecular dynamics, J. Mol. Graphics Modell., 1996, 14(1), 33–38 CrossRef CAS
.
- C. B. Hübschle and P. Luger, MolIso - a program for colour-mapped iso-surfaces, J. Appl. Crystallogr., 2006, 39, 901–904 CrossRef
.
-
C. J. Cramer, Essentials of Computational Chemistry: Theories and Models, Wiley & Sons Ltd, 2nd edn, 2004 Search PubMed
.
- S. Raub and G. Jansen, A quantitative measure of bond polarity from the electron localization function and the theory of atoms in molecules, Theor. Chem. Acc., 2001, 106(3), 223–232 Search PubMed
.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d2cp02516g |
| This journal is © the Owner Societies 2022 |