Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Experimental and modeling study of ZnO:Ni nanoparticles for near-infrared light emitting diodes
Imen Elhamdi *a,
Hajer Souissia,
Olfa Taktaka,
Jaber Elghoulbc,
Souha Kammouna,
Essebti Dhahri
*a,
Hajer Souissia,
Olfa Taktaka,
Jaber Elghoulbc,
Souha Kammouna,
Essebti Dhahri a and
Benilde F. O. Costad
a and
Benilde F. O. Costad
aLaboratoire de Physique Appliquée, Groupe de Physique des Matériaux Luminescents, Faculté des Sciences, Université de Sfax, BP 1171, 3000 Sfax, Tunisia. E-mail: imen85356@gmail.com
bImam Mohammad Ibn Saud Islamic University (IMSIU), College of Sciences, Department of Physics, Riyadh 11623, Saudi Arabia
cLaboratory of Physics of Materials and Nanomaterials Applied at Environment (LaPhyMNE), Faculty of Sciences in Gabes, Gabes University, 6072 Gabes, Tunisia
dUniversity of Coimbra, CFisUC, Physics Department, P-3004-516 Coimbra, Portugal
First published on 29th April 2022
Abstract
This work is devoted to the synthesis and study of the different properties of ZnO nanoparticles (NPs) doped with the Ni element. We have used a simple co-precipitation technique for the synthesis of our samples and various structural, morphological and optical techniques for their analysis. Energy-Dispersive X-ray spectroscopy (EDX) confirms the stoichiometry of the samples. The X-Ray Diffraction (XRD) patterns reveal the hexagonal wurtzite phase of polycrystalline ZnO with a P63mc space group. Debye Scherrer and Williamson–Hall methods show that the average size of crystallites is around 40 nm. Transmission electron microscopy (TEM) images confirm the XRD results. The optical spectrum of Zn0.95Ni0.5O shows the presence of near-band-edge (NBE) ultraviolet emission. The absorption defect bands appearing near the blue–green region and near infrared emission are attributed to the Ni2+ intra-3d luminescence. The electronic structure of the Ni2+ doped ZnO NPs confirms the Td site symmetry of Ni2+ in the ZnO host crystal and leads to a perfect correlation between calculated and experimental energy levels.
1 Introduction
ZnO is an attractive wide band gap II–VI semiconductor for potential applications in photonics, electronics, biosensors, biomedicine and spintronics.1–10 Due to good properties such as wide band gap (3.37 eV) and high excitation binding energy (60 meV), ZnO is among the useful materials for applications such as nanodevice fabrication, gas sensors, light emitting diodes, dye-sensitized solar cells, light emitting devices, thin film transistors, and optoelectronic devices.10–18 Likewise, it is widely used in various medicinal, cosmetic, and food products. Due to the anti-microbial and anti-tumor activities, ZnO is included in some cosmetic lotions as it is also known to maintain UV blocking and absorbing capabilities.5 ZnO can also be used as an astringent for wound healing, hemorrhoid treatment, eczema treatment and excoriation treatment in human medicine and veterinary science. ZnO nanoparticles (NPs) have also antibacterial, anti-neoplastic and antimicrobial properties.5 In order to modify the structure of the electronic band and improve its applications in various semiconductor spintronic devices, ZnO has been doped with different transition metals (TM).6–18 The Zn2+ in the crystal lattice structure of ZnO will be replaced by metal ions from doping, thus increasing the number of free electrons. This improves the electronic properties of the oxide semiconductor. ZnO NPs have been prepared by simple, low cost, solution-based methods, such as chemical precipitation, sol–gel synthesis, solvothermal and hydrothermal methods.16–21 The co-precipitation method is among the methods that have been widely used for the synthesis of NPs. It is considered to be promising method due to the ease of control of the particle size and the need for low temperatures in the synthesis process.In the present work, the ZnO NPs doped with Nickel were synthesized by using chemical co-precipitation method. The structural, morphological and optical properties of the ZnO NPs doped with Ni2+ were studied. We present a detailed crystal field analysis of electron energy levels of Ni2+ doped ZnO NPs. A theoretical study, based on the Racah tensor algebra methods, was performed for the Ni2+ center (3d8) with Td site symmetry.
2 Experimental methods
2.1 Materials
The precursors used to prepare ZnO:Ni2+ nanopowders, purchased from Sigma Aldrich, consist of high purity chemicals without further purification which are: zinc sulfate heptahydrate ZnSO4·7H2O (ACS reagent, 99%), nickel chloride hexahydrate NiCl2·6H2O (99.9%, trace metals basis), sodium hydroxide NaOH (reagent grade, ≥98%) and ethanol C2H5OH.2.2 Synthesis of Ni-doped ZnO NPs
As it is known, the co-precipitation process does not require expensive and complex equipment and it is robust and reliable to control the shape and size of the particles.18 Therefore, we used this process to synthesize the Ni doped ZnO nanoparticles. The process consists of dissolving 5 g of zinc sulfate heptahydrate and 0.105 g of nickel chloride in 300 ml of ultra-pure water. Then, 50 ml of NaOH (10 M) was added dropwise to the solution which was stirred magnetically for 2 h at room temperature to produce a white gelatinous precipitate. Then we filtered and washed the white precipitates using ethanol and ultrapure water several times. This step was followed by drying for 4 h at 200 °C in a furnace and grinding in an agate mortar. Finally, the nanoparticles obtained were heat treated at 500 °C in air atmosphere for 2 h.2.3 Characterization techniques
The crystal structure of the Ni doped ZnO NPs was analyzed on the basis of powder X-ray diffraction (XRD) data. The powder diffraction patterns were recorded with a STOE STADI P powder diffractometer in Debye–Scherrer geometry using Ge(111) monochromatic Cu-Kα1-radiation of wavelength λ = 1.5406 Å. The XRD measurements at room temperature were carried out in the locked coupled mode in the 2θ range from 10° to 80° with a step width of 0.02°. The surface morphology, size distribution and the composition of the elements of Ni-doped ZnO NPs were realized with Transmission Electron Microscopy TEM made on a JEOL 2011 JEOL JEM 2000 Ex microscope operating at accelerating voltage of 100 kV and probe current of ∼800 pA coupled to an Energy-Dispersive X-ray analysis (EDX) device. The micrograph patterns were recorded at 80 kV and the selected area electron diffraction (SAED) pattern was recorded by keeping camera constant 100 cm. The Fourier Transform Infrared (FTIR) was used to identify the elemental constituents of the material. The FTIR spectrum was recorded at room temperature in the range of 4000–400 cm−1 using Thermo Nicolet 380 with KBr pellet technique. An integrating sphere compatible with the powder samples attached to the UV-Vis-IR spectrophotometer (Shimadzu UV-3101 PC) has been used to measure of the optical absorbance in the wavelength range from 300 to 1800 nm. Room temperature photoluminescence (PL) spectra of our NPs have been carried using a JobinYvon-Horiba Triax 190 spectrometer with a 488 nm line of an argon ion laser and a spectral resolution of 0.3 nm, coupled with a liquid nitrogen-cooled CCD detector.3 Theoretical crystal-field study
When the free transition ion Ni2+ is used as a dopant of ZnO NPs, the energy levels are subdivided. The group theory allows us to predict the subdivision of the energy levels just by specifying the symmetry of the site occupied by the transition ion. This theory determines the number and the degeneracy of these energy levels and the value of these energies will be determined only by numerical calculation. The transition ion Ni2+ with 3d8 electronic configuration occupies a Td site symmetry in ZnO. The decomposition of the D(L) reducible representations of the SO(3) group (rotation group of the free transition ion) into irreducible representations of the site group Td leads to the stark levels of the Ni2+ ions in the ZnO host matrix. Fig. 1 represents the splitting of the Ni2+ (3d8) energy level under the crystal field with Td site symmetry. The energies of the stark levels (without and with the spin–orbit interaction) are obtained only by numerical calculation when using the Racah method. In order to study the energy levels splitting of Ni2+, we diagonalize the 45 × 45 matrix associated to the following Hamiltonian:15–17,22–27| H = H0 + Hee(B,C) + HTrees(α) + HCF(Dq) + HSO(ζ) | (1) |
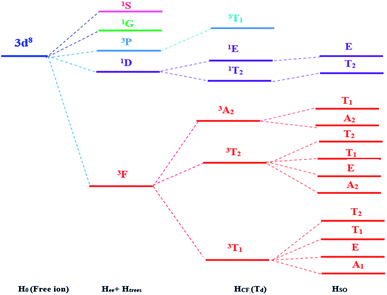 | ||
| Fig. 1 Energy levels of the Ni2+ ion (3d8) under the crystal field with Td symmetry and spin orbit coupling. | ||
H0 is the Hamiltonian configuration associated to the energy E0 which is 45 times degenerated. Hee is associated to the electron–electron repulsions which leads to the Russell-Saunders terms: 4F (ground state according to Hund rule), 4P, 2H, 2G, 2F, 2D, 2D′ and 2P excited terms. The analytical eigenvalues of Hee are a function of the Racah parameters B and C.15 The Trees correction Hamiltonian HTrees represents the two-body orbit–orbit polarization interaction. The analytical eigenvalues of HTrees are function of the Trees parameter α.15–18,22 For tetrahedral symmetry Td and following the Wybourne's notation, the crystal field Hamiltonian HCF is given by:15–18,22–28
 | (2) |
Dq is the crystal field strength parameter. HCF is a function of the Racah tensor operators Cq(k), with:15–18,22–28
 | (3) |
Ykq are the spherical harmonics.
Using the Racah tensor algebraic methods and as we know that HCF is of the intermediate strength,28 we have calculated numerically the matrix elements of Cq(k) in the basis functions 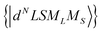 15–18,22–28
15–18,22–28
 | (4) |
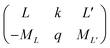 is the 3j-symbols and
is the 3j-symbols and 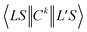 is the reduced matrix elements.
is the reduced matrix elements.
The B, C, and Dq parameters are deduced from the optical spectra. The eigenvalue of the spin–orbit coupling Hamiltonian HSO depends on ξ parameter.
The free ion Ni2+ parameters are: B0 = 1041 cm−1, C0 = 4831 cm−1, α0 = 42 cm−1 and ζ0 = 702 cm−1.16,17,22,29–31
An approximation is used between the parameters of doped and the free Ni2+ ion:26,27
| B = N4B0, C = N4C0, α = N4α0 and ζ = N2ζ0 | (5) |
The average reduction factor N is defined as:26,27
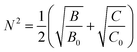 | (6) |
We created a Maple software code to determine the electronic structure of a transition ion having 3dn electronic configurations and occupying a site with symmetry determined by one of the 32-point crystallographic groups. The ref. 26 and 27 provide more information about this code. This code produces the same results as Yeung and Rudowicz's code.32
4 Results and discussion
4.1 Energy-dispersive X-ray spectroscopy analysis (EDX)
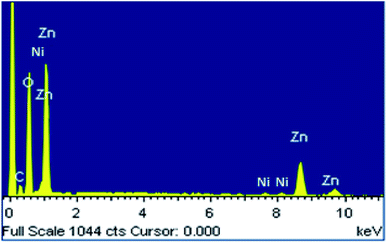
The EDX spectrum of the prepared ZnO NPs doped with Ni2+ is shown in Fig. 2. We know that peak C in the EDX spectrum is linked to the emission of the carbon ribbon used during the measurement. We note the presence of Zn and O as main components with low levels of Ni. This confirms the correct insertion of the doping element during preparation. In addition, there is no trace of peaks of external impurities in the studied compound confirming the purity of this material. The observation of low intensity peak for nickel doped ZnO in Fig. 2, confirms the proper incorporation of nickel Ni2+ in the ZnO structure. The quantitative analysis of the compositional elements presents in Ni-doped ZnO NPs using EDX spectra is presented in Table 1. From this analysis, the average Ni/Zn atomic percentage ratio is derived to be 5.01% in Ni-doped ZnO samples. This result confirms the good agreement with the experimental concentration used during the synthesis.| Spectrum for Zn0.95Ni0.05O | O | Ni | Zn |
|---|---|---|---|
| Weight (%) | 39.08 | 2.75 | 58.17 |
| Ni/Zn ratio | 0.0472 | ||
| Atomic (%) | 72.42 | 1.31 | 26.27 |
| Ni/Zn ratio | 0.0498 |
4.2 XRD diffraction
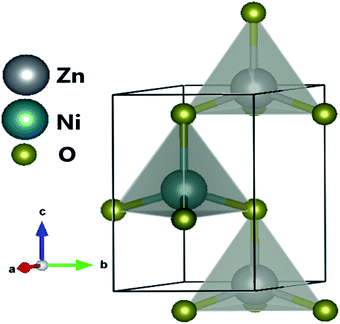
The XRD pattern of Fig. 3 clearly shows a good crystallinity of prepared samples Zn0.95Ni0.05O NPs. The XRD peaks, located at 2θ values of 31.786° (100), 34.4256° (002), 36.2574° (101), 47.5444° (102), 56.5858° (110), 62.8694° (103), 66.3757° (200), 67.9516° (112) and 69.0743° (201), have been indexed using the standard ICDD file No. 36-1451 for ZnO.33 This XRD analysis confirms the single phase with a hexagonal wurtzite structure of ZnO lattice (space group P63mc). It is noted that the wurtzite structure is not affected by the Ni transition ion doping. The Zn0.95Ni0.05O diffraction peaks show a small shift towards higher 2θ angle values compared to undoped ZnO NPs. In addition, the intensity of these peaks has decreased and the line widths broadened for the Zn0.95Ni0.05O NPs compared to the undoped ZnO NPs. The increase in lattice disorder and the deformation induced by the Ni2+ substitution can explain the change in XRD pattern. The ZnO diffraction peak (101) is the most intense peak indicating that the crystallographic preferential orientation is according to (101). In this structure, the Ni2+ ion replaces the Zn2+ ion, by substitution, in the ZnO structure and occupies a tetrahedral Td site symmetrically surrounded by four oxygen atoms with a slight distortion (Fig. 4).11,12Interplanar distance between the planes of given Miller indices h, k and l called dhkl as a function of the lattice constants (a, c) is given by the following relation:19,20
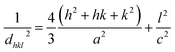 | (7) |
The plane spacing dhkl satisfies the Bragg's law:9
nλ = 2dhkl![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ θ
| (8) |
The lattice parameters a, b and c for Zn0.95Ni0.05O NPs are calculated from eqn (1) and (2) (a = b = 3.2497 Å and c = 5.2086 Å). The lattice parameters for undoped hexagonal wurtzite ZnO are a = b = 3.2513 Å and c = 5.2092 Å.21 The XRD refinements were also performed by Rietveld refinement software (Fullprof). The calculated pattern is consistent with the observed data and gives the same results for the lattice parameters a, b and c (Fig. 2). The refined crystallographic parameters are given in Table 2. The values of the parameters a and c of the Zn0.95Ni0.05O NPs are smaller compared to those of the undoped ZnO NPs, which is related to the fact that the ionic rays of the dopant Ni+2 (0.69 Å) is lower than the ionic radii of the substituted Zn+2 (0.74 Å). The value of interplanar spacing d101(2.4760 Å) for Zn0.95Ni0.05O calculated from XRD data for (101) plan shows a slightly decrease compared to d101 (2.4770 Å) for undoped ZnO. This behavior is surely due to the variation in bond lengths and angles between atoms caused by the distortion of the NiO4 tetrahedron, causing lattice stress. The tensile stress causes the peak to shift to lower 2θ values due to the increased spacing d, whereas the compressive stress plays an opposite role.
| Rp | Rwp | Rexp | χ2 |
|---|---|---|---|
| 4.75 | 6.13 | 2.49 | 6.05 |
| a (Å) | b (Å) | c (Å) | α (°) | β (°) | γ (°) |
|---|---|---|---|---|---|
| 3.2497 | 3.2497 | 5.2057 | 90.0000 | 90.0000 | 120.0000 |
| Atom | X | Y | Z | Occupation |
|---|---|---|---|---|
| Zn | 0.3333 | 0.6667 | 0.0000 | 0.9254 |
| Ni | 0.3333 | 0.6667 | 0.0000 | 0.0254 |
| O | 0.3333 | 0.6667 | 0.3833 | 1.0010 |
In the direction of c axis, the Zn–O bond length L has been calculated using the following formula:19,20
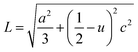 | (9) |
The positional parameter u in the wurtzite structure measures the amount which each atom gets displaced with respect to the next.33,34 The parameter u is determined by the relation:
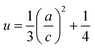 | (10) |
The value of u is of 0.375 when the network is ideal. The variation of u indicates a modification of the lattice parameters. The calculated L is of 1.9779 for Zn0.95Ni0.05O (L is of 1.9787 for undoped ZnO). A good agreement between L of this study and with those reported in literature21 is obtained which supports the results of the present study.
The volume of the unit cell for the hexagonal system has been calculated using the following relation:19
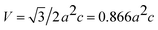 | (11) |
The volume of the unit cell is of 47.6349 Å3.
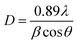 | (12) |
The incident X-ray radiation wavelength λ is of 0.15418 nm. The Bragg angle θ represents the position of the diffraction peak in the diffractograms. β is expressed in radian and represents the full width at half maxima (FWHM) of X-ray diffraction peak. The crystallite size D is calculated from the intense XRD peak and was found to be 36.06 nm.
The density of dislocation (δ) measures the defects concentration in nanostructures. The δ is considered as the ratio of the dislocation length and volume of the crystal and is calculated using the equation:36
| δ = 1/D2 | (13) |
The crystallite size D is calculated above from eqn (6). The calculated value of dislocation density (δ) for synthesized Zn0.95Ni0.05O is of 7.6870 × 10−4 nm−2.
β![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ = 0.89λ/D + 4ε θ = 0.89λ/D + 4ε![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ θ
| (14) |
By using Origin 2018, we have plotted (β![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos
cos![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ) as a function of (4
θ) as a function of (4![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin
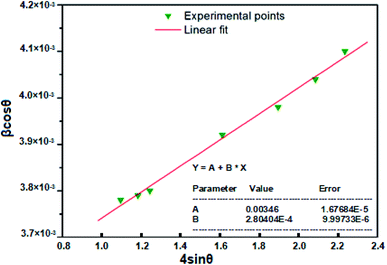
sin![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) θ) for the preferred orientation peaks in the XRD pattern (following peaks (100), (002), (101), (102), (110), (103) and (112)) of Zn0.95Ni0.05O NPs with the wurtzite hexagonal phase. From the obtained Williamson–Hall plots (Fig. 5), the crystallite size and the strain deduced from the intersection y-axis and slope of the linear fit was 39.6 nm and 2.8040 × 10−4, respectively. The crystallite size evaluated by the Williamson–Hall model is larger than that obtained by the Scherrer model. This is due to the fact that the effect of broadening of the X-ray peaks of Fig. 3, results from the size of the crystallites and the presence of deformation, and this latter is completely neglected in the Scherrer method. The strain ε obtained from the Williamson–Hall model is an important factor to consider and has a remarkable effect on the size of the crystallites.
θ) for the preferred orientation peaks in the XRD pattern (following peaks (100), (002), (101), (102), (110), (103) and (112)) of Zn0.95Ni0.05O NPs with the wurtzite hexagonal phase. From the obtained Williamson–Hall plots (Fig. 5), the crystallite size and the strain deduced from the intersection y-axis and slope of the linear fit was 39.6 nm and 2.8040 × 10−4, respectively. The crystallite size evaluated by the Williamson–Hall model is larger than that obtained by the Scherrer model. This is due to the fact that the effect of broadening of the X-ray peaks of Fig. 3, results from the size of the crystallites and the presence of deformation, and this latter is completely neglected in the Scherrer method. The strain ε obtained from the Williamson–Hall model is an important factor to consider and has a remarkable effect on the size of the crystallites.
4.3 Transmission electron microscope (TEM) studies
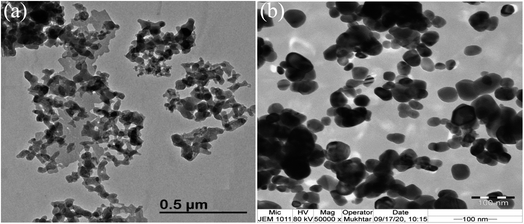
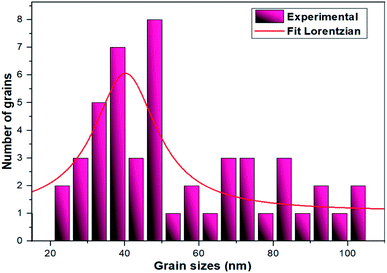
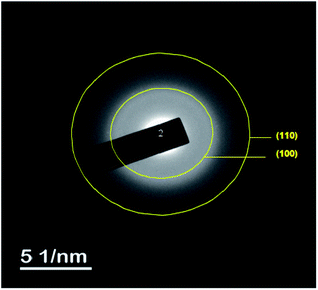
The TEM observations are is a good tool for the morphological studies of ZnO NPs and to determine the particle size and shape. TEM micrographs of Zn0.95Ni0.05O NPs, presented in Fig. 6a and b, indicate the formation of nanometric particles with a well-confined but agglomerated structure. When compared to undoped ZnO, the nickel doped ZnO samples have a random hexagonal and spherical shape with lower particle sizes. The distribution of particles is heterogeneous and the particles appear to be broadly agglomerated. Because of the increased surface to volume ratio, which results in an increase in attractive force, the particles tend to agglomerate together. This is due to nickel incorporation in the ZnO lattice. The Fig. 7 shows the particle size distribution histogram of Zn0.95Ni0.05O NPs. The particle size spans from 20 to 100 nm on the histogram, with an average size of 40.173 nm. This average value is equivalent to the estimated crystallite size calculated by Willamson–Hall method. The SAED image of Zn0.95Ni0.05O system, shown in Fig. 8, reveals a ring pattern confirming the polycrystalline structure of the sample. The indexing of SAED pattern matches well with XRD data.4.4 Fourier transform infrared (FTIR) studies
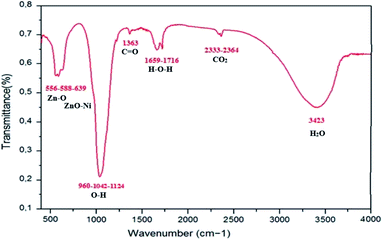
The FTIR spectrum for Zn0.95Ni0.05 NPs was recorded in the range of 400–4000 cm−1 and is shown in Fig. 9. The FTIR spectrum provides further information on functional groups. The presence and the position of the absorption bands depend on the crystal structure, chemical composition and particle morphology. FTIR measurements confirmed the creation of the wurtzite structure in Ni doped ZnO. Table 3 lists the persistent IR frequencies, as well as the vibrational assignments, that are responsible for Ni-doping in ZnO at room temperature. In the hexagonal wurtzite type crystal structure, FTIR spectra reveal the existence of characteristic peaks in the ranges of 450 to 650 cm−1, which correspond to Zn–O stretching vibrational modes.38 The change in bond lengths of Zn–O lattice when Ni ion replaces Zn ion leads to a shift of the vibration frequency of Zn–O to a frequency higher than 434 cm−1 corresponding to undoped ZnO. This confirms the incorporation of Ni2+ ions as a substitute of Zn2+ ions in ZnO structure. The vibrations of ZnO–Ni local bonds and defect states are responsible for the absorption bands that emerge about 640 and 960 cm−1, respectively.38 From literature, these frequency are absent in undoped ZnO sample. The bands around 1042 cm−1 are strong with asymmetric stretching of resonance interaction between vibration modes of oxide ions in the nanocrystals. The asymmetric and symmetric stretching of the carboxyl group (C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O) correlate to the absorption peaks seen at 1350 and 1400 cm−1. The peak around 1659 cm−1 in ZnO is ascribed to H–O–H bending vibration and is assigned to a little amount of H2O in the ZnO NPs. The absorption peaks observed in the range 2320–2400 cm−1are due to the existence of CO2 molecule in air. The broad absorption peak (2700–3500 cm−1) centered at 3443 cm−1 is ascribed to normal polymeric O–H stretching vibration of H2O molecule in Zn–O lattice.38 The appearance of these bands in manufactured nanoparticles could be attributed to atmospheric water content adsorption.
O) correlate to the absorption peaks seen at 1350 and 1400 cm−1. The peak around 1659 cm−1 in ZnO is ascribed to H–O–H bending vibration and is assigned to a little amount of H2O in the ZnO NPs. The absorption peaks observed in the range 2320–2400 cm−1are due to the existence of CO2 molecule in air. The broad absorption peak (2700–3500 cm−1) centered at 3443 cm−1 is ascribed to normal polymeric O–H stretching vibration of H2O molecule in Zn–O lattice.38 The appearance of these bands in manufactured nanoparticles could be attributed to atmospheric water content adsorption.
| Assignments | Wavenumber (cm−1) |
|---|---|
| Zn–O stretching mode | 450–650 |
| Vibrations of ZnO–Ni local bonds | 640 |
| Defect states | 960 |
| O–H asymmetric stretching | 1042 |
Symmetric stretching of the carboxyl group (C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) O) O) |
1350–1400 |
| H–O–H bending vibration | 1659 |
| CO2 molecule in air | 2320–2400 |
| O–H stretching vibration of H2O | 3443 |
4.5 Investigation of the optical spectra
The UV-Visible optical absorption spectrum of Zn0.95Ni0.05O NPs recorded at room temperature (300–1800 nm) and the PL spectrum (800–1300 nm) are illustrated in Fig. 10.| αhν = A(hν − Eg)n | (15) |
Defects commonly mentioned in ZnO-based nanostructures are:42–48
- Oxygen vacancies with different charged states (the +2 charged state (VO2+), the +1 charged state (VO+) and the neutral state (VO0)); the resulting emissions related to this type of defect usually occur near blue–green (approximately 420–550 nm).
- Zn vacancies; the resulting emissions related to this type of defect usually occur near yellow (approximately 550–610 nm).
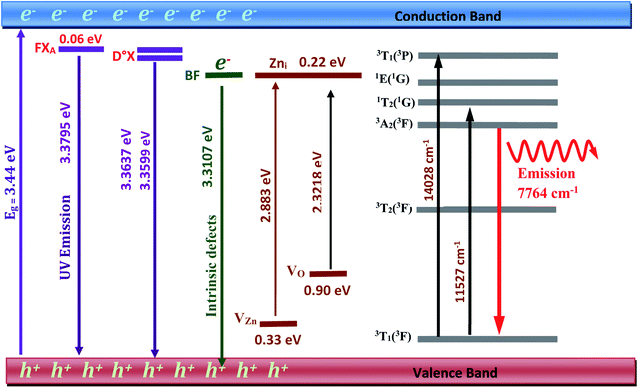
- Zn interstitials; in the wurtzite ZnO structure, Zni occupies the tetrahedral or the octahedral site. When compared to the interstitial occupying tetrahedral site, the octahedral occupancy of Zni is shown to be a stable and favorable state.17 It has been demonstrated that The Co2+ implantation generates a high concentration of additional zinc interstitials as it substitutes the Zn ions.17 This confirms that the intense donor bound exciton emission at 3.36 eV at low temperatures assigned to the D°X line is related to zinc interstitials Zni.17 We assume in this work the same behavior with the incorporation of the transition ion Ni2+. The resulting emissions related to this type of defect usually occur near orange-red (approximately 610–750 nm).
The absorption peak at 430 nm may be due to the electron transition from the valence band to Zn interstitial energy level. The absorption in the green region (511–600 nm) is related to deep level absorption (DLE). VO related defects and VZn contribute to this broad band absorption. The most common defects in ZnO are oxygen vacancies and generally act as radiative centers in luminescence processes. These oxygen vacancies are considered among the intrinsic defects of n-type ZnO. By capturing electrons they generate a new level of energy that can form ionized vacancies which act as deep defect donors. These deep defects further influence the optical properties of ZnO. The green absorption located at 534 nm is certainly related to the oxygen vacancies.
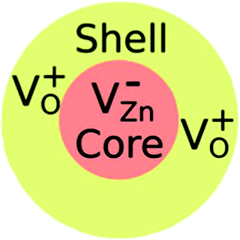
Electron paramagnetic resonance (EPR) spectroscopy is one approach for properly observing and distinguishing defects in ZnO crystals.42,46,48 According to Shankari Nadupalli et al., two EPR signals are observed for ZnO nanocrystals at g = 1.9620 and g = 2.0048, depending on the size and shape of the ZnO particle. These findings suggest that the surface/volume ratio has an impact on the sort of defects incorporated in ZnO. By using the core–shell model, core defects are assigned to EPR lines at g = 1.9620, whereas surface/shell defects are attributed to EPR lines at g = 2.0048 (Fig. 11). The EPR signal of surface/shell defects is attributed to an unpaired electron-trapped oxygen vacancy (VO) site and can either be VO+ or VO0, depending on the size and morphology of ZnO. Whereas, the EPR signal of the core defects is attributed to VZn− defect centers42 (Fig. 11). This result is specifically for ZnO nanocrystals. According to this concept and by tuning the size of the ZnO nanocrystal, one can achieve a negatively charged (n-type) core with a positively charged (p-type) surface or an exclusive p-type ZnO quantum dot. The core–shell model is only applicable to nanosized ZnO particles and cannot be used to simulate microcrystalline (>500 nm) or bulk ZnO samples.42,46,48 The research presented by Shankari Nadupalli et al. predicted that changing the size of the nanocrystal can change the concentration of intrinsic defects and their location.42 The size of the nanocrystal and the post-annealing treatments can also affect the UV, green, orange, and red emissions.42 From ref. 42, the calculated energy level for Zni is 0.22 eV below the conduction band and VZn is 0.30 eV above the valence band. The transition Zni → VZn leads to a radiative emission of 2.98 eV (415 nm). The defect level of VO is located at 0.9 eV above the valence band. Then, the transition between Zni → VO results in a radiative emission of 2.38 eV (520 nm).42
- 763 nm (13106 cm−1) on the absorption spectrum corresponding to the spin allowed transition 3T1 (3F) → 3T1 (3P).
- 859 nm (11641 cm−1) on the absorption spectrum corresponding to the spin forbidden transition 3T1 (3F) → 1T2 (1D).
- 1187 nm (8424 cm−1) on the emission spectrum corresponding to the spin allowed transition 3A2 (3F) → 3T1 (3F).
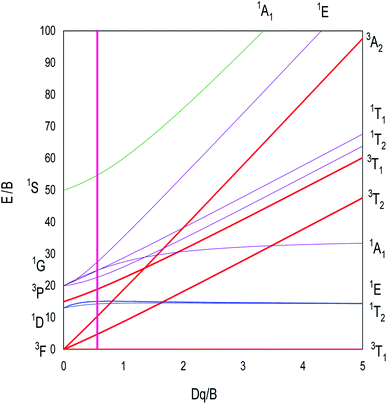
The analytical expressions of the Ni2+ energies are obtained by calculating the eigenvalues of the Hamiltonian (45 × 45) of eqn (1). The obtained eigenvalues are a function of the average reduction factor N and the crystal field strength Dq. These two parameters have been determined from the observed energies of the absorbance spectra of Zn0.95Ni0.05O NPs recorded at room temperature. We consider the ratio C/B = 4.64 of the free ion. The B, C, α and ξd parameters are calculated from eqn (5). All the parameters N, Dq, B, C, α and ξd are listed in Table 4. The calculated parameters lead to the theoretical energy levels of ZnO NPs doped with Ni2+ with the use of the code developed by S. Kammoun et al. (Table 5). The reports: βB = B/B0 = 0.71 and βC = C/C0 = 0.71 make clear the covalency effects. The Tanabe–Sugano diagram, schematized in Fig. 12 for Ni2+ ion (C/B = 4.64), indicates the suitable value for (Dq/B = 0.57) by the vertical line obtained from this theoretical analysis.
| Td | Eobserved (cm−1) | Ecalculated (cm−1) Td site symmetry | T′d | E′calculated (cm−1) |
|---|---|---|---|---|
| 3T1(3F) | 0 | 0 | A1 | 0 (1) |
| T2 | 140 (3) | |||
| T1 | 1002 (3) | |||
| E | 1535 (2) | |||
| 3T2(3F) | 3564 | A2 | 3933 (1) | |
| T1 | 4008 (3) | |||
| E | 4478 (2) | |||
| T2 | 4606 (3) | |||
| 3A2(3F) | 8424 | 7764 | T1 | 8592 (3) |
| 1T2(1D) | 11641 | 11527 (3) | T2 | |
| 1E(1D) | 12111 (2) | 1A1 | ||
| 3T1(3P) | 13106 | 14028 | A1 | 13824 (1) |
| T2 | 14110 (3) | |||
| T1 | 14685 (3) | |||
| E | 14686 (2) | |||
| 1T1(1G) | 17712 (3) | |||
| 1T2(1G) | 19310 (3) | T2 | ||
| 1A1(1G) | 19432 (1) | E | ||
| 1E(1G) | 21328 (2) | T1 | ||
| 1A1(1S) | 43752 (1) | A1 |
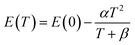 | (16) |
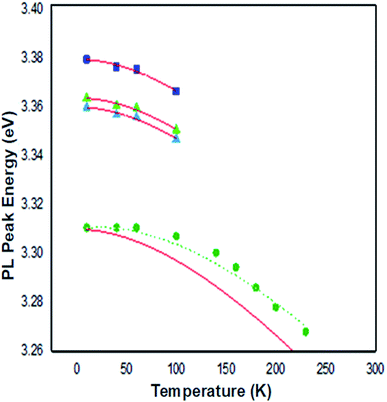
From Fig. 14, the Varshni curve is well compatible with the experimental values for the lines FXA and D°X lines. The fitting parameters for FXA and D°X lines are respectively:
α = 7.1382 10−4 eV K−1, β = 455 K and E(0) = 3.3795 eV and 3.3637 and 3.3599 eV.
The shallow zinc donor state involved in tetrahedral and octahedral interstitial sites proves the existence of two recombinations attributed to the neutral donor-bound exciton D°X (3.3637 and 3.3599 eV).
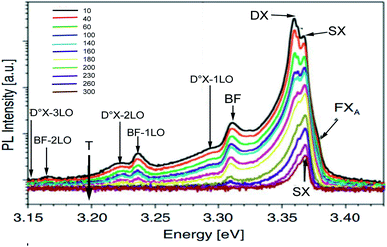
From the adjustment of the FXA emission, we can conclude that the shift of this emission with increasing temperature can be due to the shrinking of the forbidden band induced by the temperature. The recombination of both FXA and D°X at low temperatures is possible in the inter band excitonic transition.50,51 The thermal activation energy, with increasing the temperature (T > 60 K), is enough to a significant amount of D°X ionize into FXA(D°X → D° + FXA). Due to its high binding energy of 60 meV, FXA will remain up to room temperature. The D°X emission is no longer visible in the PL spectrum. The emission spectra exhibited a prominent fluorescence peak at 368 nm in visible region due intrinsic and extrinsic defects. An excitonic line, labeled as the surface excitons (SX) for ZnO, is detected between the FXA and D°X lines at 3.3676 eV. The SX line's intensity is found to be lower than its nanoscale counter-part, which has been related to surface-volume ratio effects. The line positioned at 3.3107 eV at 10 K is maintained up to room temperature and becomes dominant at 300 K, where it is located at 3.2645 eV. This line has the same characteristics as the BF emission line reported in Co2+ doped ZnO NPs at 3.314 eV at 15 K and in Co2+ doped ZnO NPs at 3.3187 eV at low temperature 10 K, according to comparison.
The recombination of defect-bound carriers with free carriers in some bands is assigned to this line. The experimental data of the BF peak position at various temperatures fit well to the curve (dotted line in Fig. 14) calculated using the formula describing the peak position of emission due to transitions from a donor to the valence band:52
| hν = Eg − ED + kBT | (17) |
Eg denotes the band-gap energy, ED is the donor's binding energy, T denotes temperature, and kB denotes the Boltzmann constant. According to Varshni's model, the position peak of the BF emission fluctuates slower with temperature than the energy band gap, as illustrated in Fig. 14.
At 10 K in Fig. 13, several additional peaks appear on the low energy side. These peaks are assigned to the longitudinal optical phonon replica (LO) of the donor bound excitons D°X and BF emission. This interpretation is based on the average spacing of 72 meV.
The peaks at 3.2922, 3.2211, and 3.1536 eV are thought to be D°X (D°X-1LO, D°X-2LO, and D°X-3LO) phonon replicas, the peaks at 3.2360 and 3.1662 eV are thought to be BF phonon replicas (BF-1LO and BF-2LO). Fig. 15 shows all the optical transitions demonstrated in this work.
5 Conclusion
Ni-doped ZnO NPs were successfully prepared by co-precipitation method. The structural and morphological properties of the synthesized nanoparticles have been studied using several techniques. EDX and XRD show a good crystallinity of prepared samples and the incorporation of Ni2+ in ZnO structure. It confirms a single phase with a hexagonal wurtzite structure of ZnO lattice (space group P63mc). The crystallite size determined by the Williamson–Hall model is bigger than that obtained by the Scherrer model. The results of the Williamson–Hall model are more precise because the constraint ε is taken into account and the data is fitted according to this method. The grain size obtained from TEM is equivalent with crystallite size calculated by Willamson–Hall method. From TEM, the morphology of the ZnO:Ni NPs shows a hexagonal structure of spherical shape with smaller grain size compared to undoped ZnO. The SAED ring pattern suggests that the ZnO NPs phase was polycrystalline in structure, and the distance between crystalline planes was consistent with the standard pattern for a wurtzite ZnO crystal structure. FTIR spectra revealed the existence of all functional groups, and this study proved that Ni2+ is occupying the Zn2+ position. From the UV photoluminescence spectra, we interpret the ZnO near-band-edge NBE ultraviolet emission. The good fit with Varshni's semi empirical formula of the peak positions versus temperature confirms the two near-band-edge NBE ultraviolet emission: the free exciton FXA and the donor bound exciton emission D°X. The observed values of the BF peak position at various temperatures fit well to the curve calculated using the formula describing the peak location of emission owing to transitions from a donor to the valence band. The blue–green absorption in ZnO NPs could be owing to an electron transition from the valence band to the Zn interstitial energy level, which is caused by defects. Another absorption in the green region is related to deep level absorption (DLE). It consists with the oxygen vacancies VO. From the theoretical crystal-field analysis and the visible region, the electronic structure of the Ni2+ doped zinc oxide nanoparticles is determined. The Td site symmetry of Ni2+ in ZnO host crystal is confirmed in this theoretical work, which leads to excellent correlations between observed and computed energy levels. According to this study, Ni doping enhances the optical properties of ZnO nanoparticles. In addition to the ultraviolet near-band transitions and the visible blue–green transition, the incorporation of Ni2+ in ZnO semiconductor with a high gap leads to the near-infrared emission at 1187 nm which is attributed to intra-3d–3d transition 3A2(3F) → 3T1(3F). The d–d transitions of Ni2+ are located in the large gap of ZnO nanoparticles. For this reason, ZnO:Ni2+ can be used for optoelectronic devices such as near-infrared light-emitting diodes (N-IR LEDs). Moreover, Ni2+ doping increases the ZnO matrix defects, which increases the luminescence of the blue–green region.Conflicts of interest
There are no conflicts of interest to declare.Acknowledgements
The authors acknowledge the support of the Tunisian Ministry of Higher Education and Scientific Research within the framework of the Tunisian-Portuguese cooperation in the field of scientific research and technology (Project of University of Sfax-University of Aveiro). This work was supported by national funds from FCT – Fundação para a Ciência e a Tecnologia, I.P., within the projects UIDB/04564/2020 and UIDP/04564/2020. Access to TAIL-UC facility funded under QREN-Mais Centro Project No. ICT_2009_02_012_1890 is gratefully acknowledged.References
- P. Sheng, L. Yao, P. Yang, D. Yang, C. Lu, K. Cao and W. Li, The origin of enhanced photoelectrochemical activity in metal-ion-doped ZnO/CdS quantum dots, J. Alloys Compd., 2020, 822, 153700, DOI:10.1016/j.jallcom.2020.153700.
- A. T. Le, M. Ahmadipour and S.-Y. Pung, A review on ZnO-based piezoelectric nanogenerators: Synthesis, characterization techniques, performance enhancement and applications, J. Alloys Compd., 2020, 844, 156172, DOI:10.1016/j.jallcom.2020.156172.
- F. Z. Bedia, A. Bedia, N. Maloufi, M. Aillerie, F. Genty and B. Benyoucef, Effect of tin doping on optical properties of nanostructured ZnO thin films grown by spray pyrolysis technique, J. Alloys Compd., 2014, 616, 312–318, DOI:10.1016/j.jallcom.2014.07.086.
- S. Agarwal, L. K. Jangir, K. S. Rathore, M. Kumar and K. Awasthi, Morphology-dependent structural and optical properties of ZnO nanostructures, Appl. Phys. A, 2019, 125, 553 CrossRef CAS . https://link.springer.com/article/10.1007/s00339-019-2852-x.
- Y. Zhang, T. R. Nayak, H. Hong and W. Cai, Biomedical Applications of Zinc Oxide Nanomaterials, Curr. Mol. Med., 2013, 13(10), 1633–1645 CrossRef CAS PubMed.
- K. Raja, P. S. Ramesh and D. Geetha, Synthesis, Structural and optical properties of ZnO and Ni-doped ZnO hexagonal nanorods by Co-precipitation method, Spectrochim. Acta, Part A, 2014, 120, 19–24, DOI:10.1016/j.saa.2013.09.103.
- J. El Ghoul, Synthesis, structural and optical properties of nanoparticles (Al, V) co-doped zinc oxide, Bull. Mater. Sci., 2016, 39, 7–12 CrossRef CAS . https://link.springer.com/article/10.1007%2Fs12034-015-1139-x.
- J. El Ghoul, M. Kraini and L. El Mir, Synthesis of Co-doped ZnO nanoparticles by sol–gel method and its characterization, J. Mater. Sci.: Mater. Electron., 2015, 26, 2555–2562, DOI:10.1007/s10854-015-2722-z.
- J. El Ghoul, Synthesis of vanadium doped ZnO nanoparticles by sol–gel method and its characterization, J. Mater. Sci.: Mater. Electron., 2016, 27, 2159–2165, DOI:10.1007/s10854-015-4006-z.
- K. Kumar, M. Chitkara, I. S. Sandhu, D. Mehta and S. Kumar, Photocatalytic, Optical and Magnetic properties of Fe-doped ZnO nanoparticles prepared by chemical route, J. Alloys Compd., 2014, 588, 681–689, DOI:10.1016/j.jallcom.2013.11.127.
- N. Hasuike, K. Nishio, H. Katoh, A. Suzuki, T. Isshiki, K. Kisoda and H. Harima, Structural and electronic properties of ZnO polycrystals doped with Co, J. Phys.: Condens. Matter, 2009, 21, 064215, DOI:10.1088/0953-8984/21/6/064215.
- T. Zou, X. Xing, Y. Yang, Z. Wang, Z. Wang, R. Zhao, X. Zhang and Y. Wang, Water-soluble ZnO quantum dots modified by (3-aminopropyl) triethoxysilane: The promising fluorescent probe for the selective detection of Cu2+ ion in drinking water, J. Alloys Compd., 2020, 825, 153904, DOI:10.1016/j.jallcom.2020.153904.
- Z. Yan, Z. Sun, K. Yue, A. Li and L. Qian, CoO/ZnO nanoclusters immobilized on N-doped 3 D reduced graphene oxide for enhancing lithium storage capacity, J. Alloys Compd., 2020, 836, 155443, DOI:10.1016/j.jallcom.2020.155443.
- S. Anjum, H. Nazli, F. Bashir and K. Mahmood, Structural, Optical and Magnetic Properties of Zn0.5CuxCo0.5-x Dilute Magnetic Semiconductors, Mater. Today: Proc., 2015, 2, 5552–5558, DOI:10.1016/j.matpr.2015.11.085.
- S. Kammoun and J. El ghoul, Structural and optical investigation of Co-doped ZnO nanoparticles for nanooptoelectronic devices, J. Mater. Sci.: Mater. Electron., 2021, 32, 7215–7225, DOI:10.1007/s10854-021-05430-7.
- A. Neffati, H. Souissi and S. Kammoun, Electronic structure of Co-doped ZnO nanorods, J. Appl. Phys., 2012, 112, 083112, DOI:10.1063/1.4757634.
- F. Mselmi, A. Neffati and S. Kammoun, Theoretical investigation of the cathodoluminescence spectra of Co-doped ZnO nanowires, J. Lumin., 2018, 198, 124–131, DOI:10.1016/j.jlumin.2018.02.018.
- J. El Ghoul and F. F. Al-Harbi, Synthesis, structural, optical and magnetic properties of Gd co-doped ZnO:V nanoparticles, Solid State Commun., 2020, 314–315, 113916, DOI:10.1016/j.ssc.2020.113916.
- N. Patra, S. K. Karuturi, D. Nakamura, M. Higashihata, V. Singh and I. A. Palani, Influence of Ni, Ti and NiTi alloy nanoparticles on hydrothermally grown ZnO nanowires for photoluminescence enhancement, J. Alloys Compd., 2019, 770, 1119, DOI:10.1016/j.jallcom.2018.08.160.
- V. Gandhi, R. Ganesan, H. H. A. Syedahamed and M. Thaiyan, Effect of Cobalt Doping on Structural, Optical, and Magnetic Properties of ZnO Nanoparticles Synthesized by Coprecipitation Method, J. Phys. Chem. C, 2014, 118, 9715–9725, DOI:10.1021/jp411848t.
- H. Sarma and K. C. Sarma, X-ray Peak Broadening Analysis of ZnO Nanoparticles Derived by Precipitation method, International Journal of Scientific and Research Publication, 2014, 4 Search PubMed ). https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.429.2767%26rep=rep1%26type=pdf.
- F. Mselmi, O. Taktak, H. Souissi and S. Kammoun, Correlation between experimental spectroscopic study and crystal-field calculations of Co2+ ions in α-ZnAl2S4 spinel, J. Lumin., 2019, 206, 319–325, DOI:10.1016/j.jlumin.2018.10.047.
- H.-M. Zhang, X. Wan and Z.-M. Zhang, Theoretical studies of the spin Hamiltonian parameters and local structures for the tetragonal Cu2+ and Ni3+ centers in Mg2TiO4, J. Alloys Compd., 2013, 549, 226–230, DOI:10.1016/j.jallcom.2012.09.078.
- H.-M. Zhang, Y.-D. Li, B.-J. Chen, C.-D. Feng and Y.-J. Fu, Theoretical studies on the Spin Hamiltonian parameters and the local structure for Cu2+ in rutile, J. Alloys Compd., 2020, 25, 154294, DOI:10.1016/j.jallcom.2020.154294.
- O. Taktak, H. Souissi and S. Kammoun, Optical absorption properties of ZnF2-RO-TeO2 (R = Pb, Cd and Zn) glasses doped with chromium (III): Neuhauser model and crystal field study, Opt. Mater., 2021, 113, 110682, DOI:10.1016/j.optmat.2020.110682.
- O. Taktak, H. Souissi and S. Kammoun, Optical properties of the phosphors Zn2SnO4:Cr3+ with near-infrared long-persistence phosphorescence for bio-imaging applications, J. Lumin., 2020, 228, 117563, DOI:10.1016/j.jlumin.2020.117563.
- H. Souissi, O. Taktak and S. Kammoun, Theoretical study by analytical equations of optical properties of the phosphors Zn2SnO4 codoped with Cr3+ and (Al3+ or Eu3+) via the crystal field control, Opt. Mater., 2021, 121, 111529, DOI:10.1016/j.optmat.2021.111529.
- D. J. Newman and B. Ng, Crystal Field Handbook, Cambridge University Press, 1st edn, 2000, pp. 28–36 Search PubMed.
- J. S. Griffith, The Theory of transition-metal ions, Cambridge university Press, Cambridge, 1961 Search PubMed.
- S. Sugano, Y. Tanabe, H. Kamimura, Multiplets of Transition-Metal Ions in Crystals, Academic Press, New York, 1st edn, 1970, pp. 106–111 Search PubMed.
- R. C. Powell, Physics of Solid-State Laser Materials, Springer-Verlag, New York, 1998, 1st edn, pp. 215–233 Search PubMed.
- Y. Y. Yeung and C. Rudowicz, Ligand field analysis of the 3dN ions at orthorhombic or higher symmetry sites, Comput. Chem., 1992, 16, 207–216, DOI:10.1016/0097-8485(92)80004-J.
- C. S. Barret and T. B. Massalski, Structure of Metals: Crystallographic Methods, Principles and Data, Pergamon Press, Oxford, 1980 Search PubMed.
- F. C. M. Vande Pol, Thin-film ZnO-properties and applications, Am. Ceram. Soc. Bull., 1990, 69, 1959–1965 CAS . https://jglobal.jst.go.jp/en/detail?JGLOBAL_ID=200902031457640468.
- G.-L. Tan, D. Tang, D. Dastan, A. Jafari, J. P. B. Silva and X.-T. Yin, Effect of heat treatment on electrical and surface properties of tungsten oxide thin films grown by HFCVD technique, Mater. Sci. Semicond. Process., 2021, 122, 105506, DOI:10.1016/j.mssp.2020.105506.
- A. S. Hassanien, A. A. Akl and A. H. Sáaedicryst, Synthesis, crystallography, microstructure, crystal defects, and morphology of BixZn1−xO nanoparticles prepared by sol–gel technique, CrystEngComm, 2018, 20, 1716–1730, 10.1039/c7ce02173a.
- M. M. Obeid, H. R. Jappor, K. Al-Marzoki, I. A. Al-Hydary, S. J. Edrees and M. M. Shukur, Unraveling the effect of Gd doping on the structural, optical, and magnetic properties of ZnO based diluted magnetic semiconductor nanorods, RSC Adv., 2019, 9, 33207–33221, 10.1039/c9ra04750f.
- M. P. Dasari, U. Godavarti and V. Mote, Structural, morphological, magnetic and electrical properties of Ni-doped ZnO nanoparticles synthesized by co-precipitation method, Process. Appl. Ceram., 2018, 12, 100–110, DOI:10.2298/pac1802100d.
- R. Mguedla, A. Ben Jazia Kharrat, O. Taktak, H. Souissi, S. Kammoun, K. Khirouni and W. Boujelben, Experimental and Theoretical investigations on optical properties of multiferroic PrCrO3 ortho-chromite compound, Opt. Mater., 2020, 101, 109742, DOI:10.1016/j.optmat.2020.109742.
- K. Joshi, M. Rawat, S. K. Gautam, R. G. Singh, R. C. Ramola and F. Singh, Band gap widening and narrowing in Cu-doped ZnO thin films, J. Alloys Compd., 2016, 680, 252–258, DOI:10.1016/j.jallcom.2016.04.093.
- R. Pappalardo, D. L. Wood and R. C. Linares Jr, Optical Absorption Spectra of Ni-Doped Oxide Systems. I, J. Chem. Phys., 1961, 35, 1460, DOI:10.1063/1.1732066.
- S. Nadupalli, S. Repp, S. Weberb and E. Erdem, About defect phenomena in ZnO nanocrystals, Nanoscale, 2021, 13, 9160–9171, 10.1039/d1nr00943e.
- H. Kaftelen, K. Ocakoglu, R. Thomann, S. Tu, S. Weber and E. Erdem, EPR and photoluminescence spectroscopy studies on the defect structure of ZnO nanocrystals, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 014113, DOI:10.1103/physrevb.86.014113.
- P. Jakes and E. Erdem, Finite size effects in ZnO nanoparticles: An electron paramagnetic resonance (EPR) analysis, Phys. Status Solidi RRL, 2011, 5(2), 56–58, DOI:10.1002/pssr.201004450.
- S. Repp, S. Weber and E. Erdem, Defect Evolution of Nonstoichiometric ZnO Quantum Dots, J. Phys. Chem. C, 2016, 120(43), 25124–25130, DOI:10.1021/acs.jpcc.6b09108.
- A. U. Ammar, I. D. Yildirim, F. Bakan and E. Erdem, ZnO and MXenes as electrode materials for supercapacitor devices, Beilstein, J. Nanotechnol., 2021, 12, 49–57, DOI:10.3762/bjnano.12.4.
- M. H. Aleinawi, A. U. Ammar, M. Buldu-Akturk, N. S. Turhan, S. Nadupalli and E. Erdem, Spectroscopic Probing Of Mn-Doped ZnO Nanowires Synthesized via a Microwave-Assisted Route, J. Phys. Chem. C, 2022, 126(8), 4229–4240, DOI:10.1021/acs.jpcc.2c00009.
- E. Erdem, Microwave power, temperature, atmospheric and light dependence of intrinsic defects in ZnO nanoparticles: A study of electron paramagnetic resonance (EPR) spectroscopy, J. Alloys Compd., 2014, 605, 34–44, DOI:10.1016/j.jallcom.2014.03.157.
- Y. P. Varshni, Temperature dependence of the energy gap in semiconductors, Physica, 1967, 34, 149–154, DOI:10.1016/0031-8914(67)90062-6.
- F.-Y. Jen, Y.-C. Lu, C.-Y. Chen, H.-C. Wang, C. C. Yang, B.-P. Zhang and Y. Segawa, Temperature-dependent exciton dynamics in a ZnO thin film, Appl. Phys. Lett., 2005, 87, 252117, DOI:10.1063/1.2150277.
- L. H. Quang, S. J. Chua, K. P. Loh and E. Fitzgerald, The effect of post-annealing treatment on photoluminescence of ZnO nanorods prepared by hydrothermal synthesis, J. Cryst. Growth, 2006, 287, 157–161, DOI:10.1016/j.jcrysgro.2005.10.060.
- G. D. Chen, M. Smith, J. Y. Lin, H. X. Jiang, A. Salvador, N. Sverdlov, A. Botchkarv and H. Morkoc, Photoluminescence studies of band-edge transitions in GaN epitaxial layers grown by plasma-assisted molecular beam epitaxy, J. Appl. Phys., 1996, 79, 2675H CrossRef.
| This journal is © The Royal Society of Chemistry 2022 |