Open Access Article
Open Access ArticleThe electronic, magnetic, and optical properties of double perovskite La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc)
Suneela Arif * and
Yasir Ali
* and
Yasir Ali
Department of Physics and Astronomy, Hazara University, Garden Campus (Main Campus), Mansehra, Pakistan. E-mail: suneela@hu.edu.pk; Tel: +92 310-050-7841
First published on 9th December 2022
Abstract
2D La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskites are considered promising potential materials for electronic, magnetic, and optoelectronic applications. Herein, we thoroughly investigated the ground state electronic, magnetic, and optical properties of half-metallic ferromagnetic (HM-FM) La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskites using the full-potential linearized augmented plane wave (FP-LAPW) method within the generalized gradient (GGA) and (GGA (+U)) approximations based on density functional theory (DFT). Full structural optimization was carried out by using the FP-LAPW method. The electronic band structure (BS) and the total density of states (DOS) calculations confirm that all these double perovskite compounds are half-metallic (HM). The total spin magnetic moments calculated confirm the half metallic and ferromagnetic nature of the materials. Optical properties were determined for their potential use in opto-electronic devices. Based on our results, we concluded that La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds are half metal ferromagnetic and have potential applications as ferromagnetic insulators in spintronic, magnetic and optoelectronic devices.
1. Introduction
Perovskites are extensively studied oxides, and exhibit potential applications as insulators/dielectrics in superconductors and a range of materials from diamagnetic to colossal magneto-resistive (CMR).1–3 BaTiO3 and PbZrO3 are well-known perovskites used in the piezoelectric, dielectric, optoelectronic and microelectronic industries.4–6 Among the different classes of perovskites are ordered double perovskites (ODP), general formula, A2B′B′′O6, where “A” is a rare-earth or alkaline-earth element, while B′ and B′′ are different transition-metal ions. These have attracted attention due to their ability to accommodate an extensive range of rare earth elements at the A-site, significantly modifying the bond length and bond angle of the octahedra, and two different types, sizes and valence states of cations at the B′ and B′′-sites, offering the opportunity to manipulate, tune and tailor their physical properties according to industrial and technological needs.7,8 ODP usually exhibit half-metallic (HM),9,10 ferrimagnetic (FiM),11,12 ferromagnetic (FM),13,14 antiferromagnetic (AF),15,16 half-metallic ferromagnetic (HM-FM),17,18 half-metallic ferrimagnetic (HM-FiM),17,19 nonmagnetic (NM),18,20 ferroelectric (FE),12,21 and semiconducting (SC)12,18,21 responses near room temperature. Half-metallic materials have well-defined band gaps in one spin state and metallic bands in the other spin channel.21 Thus, HM materials have quantization of magnetic moment, zero spin susceptibility and 100% spin polarization at the Fermi level.21–23 HM materials have potential application in data storage memory and magnetic recording due to their single spin charge carriers.24,25 J. B. Philipp et al.26 reported the structural, transport and optical properties and doping effects in half-metallic double perovskites (HM-DP) A2CrWO6 with A = Sr, Ba and Ca. The saturation magnetization and the influence of steric effects on the Curie temperature, TC, were determined by changing the alkaline earth ion on the A site and a maximum Curie temperature (TC = 458° K) was reported for Sr2CrWO6, which has an almost undistorted perovskite structure and a tolerance factor Tf ≈ 1. The researchers showed that the structural changes in Ba2CrWO6 and Ca2CrWO6 result in a reduction of the Curie temperature, suggesting that, in general for double perovskites, an optimal TC can be achieved only for Tf ≈ 1. Furthermore, they found that both the Curie temperature and saturation magnetization MS reduce due to electron doping in Sr2CrWO6 by partial substitution of Sr2+ by La2+. Room temperature colossal magneto resistance and high temperature TC transition for Sr2FeMoO6 systems confirm half-metallic (HM) behavior. Half-metallic ferromagnetic (HM-FM) nature is observed for La2CrCoO6 (LCCO),27 La2CrNiO6 (LCNO),28 La2ScNiO6 (LSNO),27 La2VNiO6 (LVNO)27 and La2VScO6 (LVSO)27 compounds, validating their potential suitability for spintronic applications such as in magnetic sensors, magneto resistive random-access memory (MRRAM) and solid fuel cells. A half-metallic antiferromagnetic (HM-AFM) response is observed in LaAVRuO6 (A = Ca, Sr, and Ba) systems using the local spin-density approximation (LSDA).29 Chen et al.30 reported that La2VMnO6 has half-metallic antiferromagnetic (HM-AFM) and ferromagnetic (HM-FM) phases and the antiferromagnetic phase is more stable by 0.1 eV per cell than the ferromagnetic phase. The magneto resistance (MR) in ordered and disordered double perovskite oxide Sr2FeMoO6 was investigated by D. D. Sarma et al.31 They concluded that the ordered sample exhibits a sharp low-field response, followed by moderate changes at higher fields, and the disordered sample was characterized in the absence of a low-field response, confirming that the low-field response depends critically on the half-metallic ferromagnetism, while the high-field response depends on the overall magnetic nature of the sample, still in the absence of the half-metallic state. Although double perovskites are widely studied materials due to their potential applications, no detailed theoretical and experimental studies are available for La based La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskite compounds. Herein, we conclusively theoretically predict the structural, electronic, magnetic and optoelectronic properties of La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskites using first principle generalized gradient GGA32–34 and GGA (+U)35,36 approximation calculations, considering the ferromagnetic state in the tetragonal structure (14/mmm, No. 139). Since La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskites are strongly electron correlated systems requiring enhanced description, GGA calculations were corrected by using a strong-correlation correction GGA (+U). We revealed that the electronic magnetic and optoelectronic properties of these materials support their potential use in capacitors, photocells, data storage devices and automobile license plates.2. Method of calculation
We performed structural, electronic, magnetic and optical property calculations for La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskite compounds using GGA32–34 and GGA (+U)35,36 (hybrid term) techniques within the framework of density functional theory (DFT).37,38 Full structural optimization was carried out by using the full-potential linearized augmented plane wave (FP-LAPW) method39,40 as implemented in WIEN2K software41 (updated UNIX versions of the original Wien2K code are called Wien93, Wien95 and Wien97). The WIEN2K software was used to calculate the lattice parameter, band structure, charge density and exchange-correlation potential (VExc). The charge density and potential were extended in terms of spherical harmonics inside the muffin-tin spheres in terms of the augmented plane wave (APW)32–36 outside the muffin-tin spheres. In WIEN2K, the basis set APW+ local is used inside the atomic sphere for chemically important orbitals, while FP-LAPW is used for others, therefore it is based on the combination of both techniques.39–41 The strong electron correlation systems, such as transition metal oxides, were described by the on-site electron correlation correction GGA (+U) method.35,36 The main control of the convergence in co-relation with the basis size is by means of the parameter, RKmax, the product of the largest plane wave vector (Kmax), the smallest radius of the sphere (R) and the cutoff value of the angular momentum (Lmax). In our calculations, for excellence convergence of the charge, a plane wave cut-off value of RMTKmax = 6 is used in the interstitial region, up to 120 K-point numbers were used for the Brillouin zone for the 14/mmm structures and Lmax = 6 was used for the charge density and potential.3. Results and discussion
3.1. Electronic band structures and density of states
The band structure (BS) and density of states (DOS) give information about the electronic properties27,28,36 of the materials, and were investigated using GGA and GGA (+U) for the La2BB′O6 (B = Cr, V and B′ = Co, Ni and Sc) double perovskite systems, as illustrated in Fig. 1 and 2. We observed that the band structures are crowded in both the valence and conduction band. GGA calculations for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 systems (Fig. 1(a–j) and Table 1) reveal overlapped valence and conduction bands around the Fermi level in spin-up states with a metallic response, whereas in the spin-down channel there was no overlapping around the Fermi level and energy gaps of 0.94 eV, 1.14 eV, 1.63 eV, 1.22 eV and 3.53 eV were exhibited, confirming a non-metallic semiconducting response, validating half-metallic (HM) behavior in good agreement with previously reported work.37–39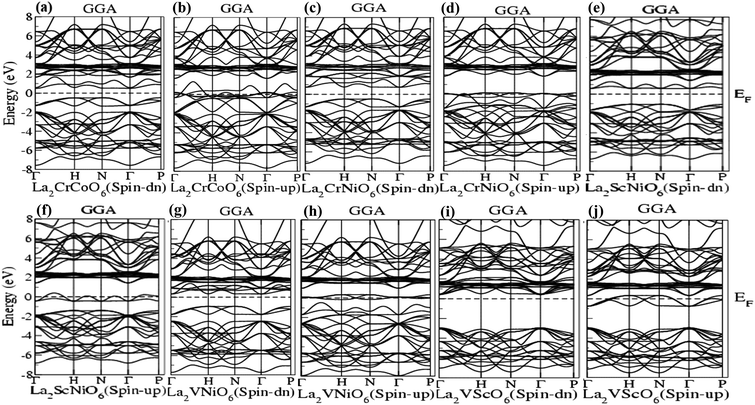 | ||
| Fig. 1 (a–j) Band structures of La2CrCoO6 (LCCO), La2CrNiO6 (LCNO), La2ScNiO6 (LSNO), La2VNiO6 (LVNO) and La2VScO6 (LVSO) using the GGA scheme. | ||
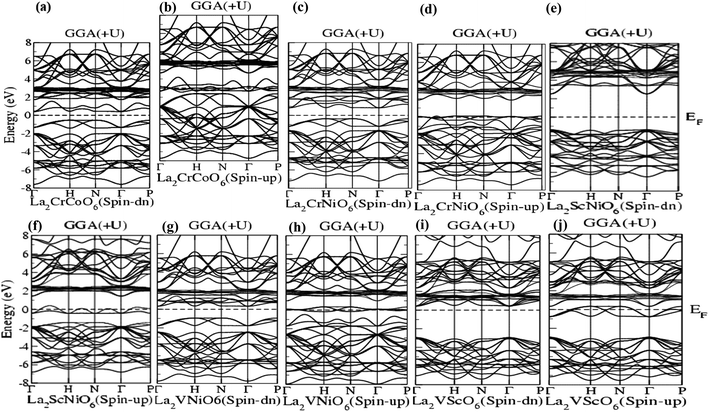 | ||
| Fig. 2 (a–j) Band structure of La2CrCoO6 (LCCO), La2CrNiO6 (LCNO), La2ScNiO6 (LSNO), La2VNiO6 (LVNO) and La2VScO6 (LVSO) using the GGA (+U) scheme. | ||
| Serial no. | Compound | Spin | Present work Eg (eV) | Other theoretical work Eg (eV) | ||
|---|---|---|---|---|---|---|
| GGA | GGA (+U) | GGA | GGA (+U) | |||
| 1 | La2CrCoO6 | Spin-up | Metallic | Metallic | Metallic | Metallic |
| Spin-down | 0.945296 | 1.667336 | 0.94 (ref. 28) | 0.762 (ref. 27) | ||
| 2 | La2CrNiO6 | Spin-up | Metallic | Metallic | Metallic | Metallic |
| Spin-down | 1.149897 | 1.211155 | 1.14 (ref. 28) | 1.007 (ref. 27) | ||
| 3 | La2ScNiO6 | Spin-up | Metallic | Metallic | Metallic | Metallic |
| Spin-down | 1.632651 | 3.855420 | 1.63 (ref. 28) | 1.497 (ref. 27) | ||
| 4 | La2VNiO6 | Spin-up | Metallic | Metallic | Metallic | Metallic |
| Spin-down | 1.225672 | 2.00800 | 1.22 (ref. 28) | 1.116 (ref. 27) | ||
| 5 | La2VScO6 | Spin-up | Metallic | Metallic | Metallic | Metallic |
| Spin-down | 3.537194 | 3.566456 | 3.53 (ref. 28) | 3.238 (ref. 27) | ||
Fig. 2(a–j) represent the GGA (+U) calculations for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds, showing that in the spin-down channel the energy gaps for these compounds were 1.66 eV, 1.21 eV, 3.85 eV, 2.00 eV and 3.56 eV, respectively. However, overlapped valence and conduction bands are observed in the spin-up channel, confirming the metallic response. Both the values of the band gap calculated via GGA and GGA (+U) calculations listed in Table 1 and represented in Fig. 1 and 2 indicate that La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 are direct bandgap materials since the maxima of the valence bands and minima of the conduction bands lie at the sigma (Γ) symmetry points.
The band structure calculated by GGA and GGA (+U) shows that the width of the band gaps increases by a specific amount of energy (0.5 eV). The band gap or energy gap (Eg) calculated for La2VScO6 is the maximum and equal to 3.537 eV and 3.566 eV whereas La2CrCoO6 has the minimum bandgap of 0.945 eV (according GGA), and the La2CrNiO6 system has the minimum band gap of 1.211 eV (according to GGA (+U)). The calculated band gaps in terms of electron volts (eV) using GGA and GGA (+U) calculations are illustrated in Table 1 and are in good agreement with other previously reported theoretical work.
The behaviors of the band structures and electronic distributions (states) of the La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds were calculated by total DOS (TDOS) using the GGA and GGA (+U) schemes as shown in Fig. 3 and 4(a–e). The TDOS calculations reveal that in all the compounds a maximum contribution above the Fermi level (EF), arises due to Tot-La in the spin-up as well as spin-down states. The valence and conduction bands overlapping in the spin-up channel validates the metallic behavior, whereas there is a clear gap between the valence and conduction bands for the spin-down channel displaying semiconducting behavior, hence confirming that all these compounds are half-metallic. The main reasons for the half metallicity are p–d hybridization and double exchange. The stronger p–d hybridization in these compounds leads to the p-state being pushed above the Fermi level (EF) in the spin-up channel, while the band extends in the spin-down channel with EF exhibiting the double exchange effect, referred to as half-metallicity. In the case of La2CrNiO6 (Fig. 3(b) and 4(b)) in both the spin-up and spin-down channels above the Fermi level, La-f has the maximum contribution, whereas below the Fermi level there is a small contribution from Cr-d and Ni-d to the total density of states (TDOS) and the peak value of the TDOS in the conduction band is nearly 48 (states per eV) at 3 eV. Similarly, we observed that in the case of the La2ScNiO6, La2VNiO6 and La2VScO6 compounds (Fig. 3(c–e) and 4(c–e)) there is a small contribution of Ni-d to the total density of states (TDOS) below the Fermi level (EF), while above the Fermi level there is a maximum contribution of La-f to the (TDOS). Furthermore, there is a negligible contribution of O-p to the total density of states (TDOS) below the Fermi level and a small contribution of Sc-d and V-d in the conduction bands, for both the spin-up and spin-down channels.
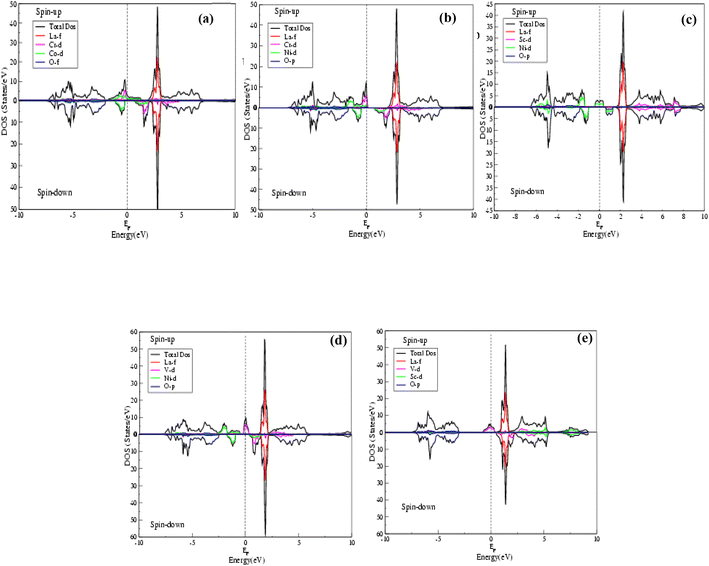 | ||
| Fig. 3 (a–e) Total density of states (TDOS) of La2CrCoO6 (LCCO), La2CrNiO6 (LCNO), La2ScNiO6 (LSNO), La2VNiO6 (LVNO) and La2VScO6 (LVSO) compounds calculated by the GGA scheme. | ||
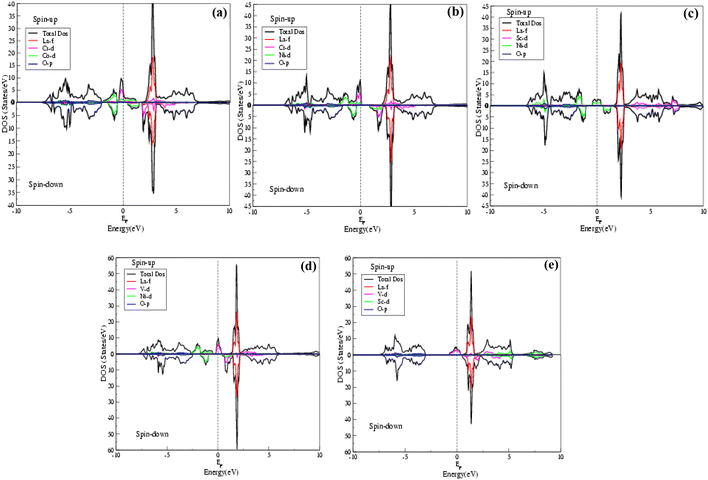 | ||
| Fig. 4 Total density of states (TDOS) of La2CrCoO6 (LCCO), La2CrNiO6 (LCNO), La2ScNiO6 (LSNO), La2VNiO6 (LVNO) and La2VScO6 (LVSO) compounds calculated by the GGA (+U) scheme. | ||
The DOS is nearly the same for both the GGA and GGA (+U) calculations and the maximum value of the density of states above the Fermi level is at approximately 1.25 eV.
3.2. Magnetic properties
The magnetic behaviors of the La2VNiO6, La2VScO6, La2ScNiO6, La2CrNiO6 and La2CrCoO6 double perovskite compounds were calculated by spin polarized GGA and GGA (+U) approximations by calculating the interstitial region (mIntst), individual atom (mS1, S2, S3, S4) and total cell (mCell) magnetic moments. The detailed values of the magnetic moments calculated are listed in Tables 2 and 3. In the generalized gradient approximation calculation, spinel compound transition metal d-state electrons with oxides are not strongly correlated, which keeps them near the Fermi level (EF). Therefore, for the transition metal d-state electrons we used GGA (+U) calculations with the U parameter changing from 1.5 eV to 3.0 eV. Choosing an appropriate value of U (ranging from 1.5 eV to 3.0 eV), splits the band structure of these compounds but the total magnetic moments remain the same and the energy gaps increase from 1.2 eV, 3.5 eV, 1.6 eV, 1.1 eV and 0.9 eV to 2.0 eV, 3.6 eV, 3.3 eV, 2.9 eV and 1.6 eV in spin-down channels. The detailed values of the magnetic moments calculated via the GGA and GGA (+U) approximations of all these compounds are illustrated in Tables 2 and 3, and confirm the half-metallic ferromagnetism.| Serial no. | Materials | mIntst | mS1 | mS2 | mS3 | mS4 | mS5 | mCell |
|---|---|---|---|---|---|---|---|---|
| 1 | La2CrCoO6 | 0.27150 | 0.01201 | 2.32582 | 0.18412 | 0.01326 | 0.01290 | 2.88359 |
| 2 | La2CrNiO6 | 0.28269 | 0.01420 | 1.99849 | 1.38930 | 0.04885 | 0.05090 | 4.00017 |
| 3 | La2ScNiO6 | 0.01170 | −0.00220 | 0.04520 | 0.77638 | 0.02802 | 0.02834 | 0.99828 |
| 4 | La2VNiO6 | 0.15326 | 0.01338 | 0.89250 | 1.48145 | 0.06907 | 0.06982 | 2.97139 |
| 5 | La2VScO6 | 0.42797 | 0.07356 | 1.42382 | 0.03688 | −0.00487 | −0.00447 | 2.00820 |
| Serial no. | Compound | mIntst | mS1 | mS2 | mS3 | mS4 | mS5 | mCell |
|---|---|---|---|---|---|---|---|---|
| 1 | La2CrCoO6 | 0.32371 | 0.02128 | 2.38831 | 0.15488 | 0.01886 | 0.01511 | 3.00761 |
| 2 | La2CrNiO6 | 0.26399 | 0.01282 | 1.97414 | 1.40242 | 0.05606 | 0.05715 | 4.00693 |
| 3 | La2ScNiO6 | −0.01631 | 0.00239 | 0.01728 | 1.31964 | −0.05725 | −0.05270 | 1.00007 |
| 4 | La2VNiO6 | 0.15399 | 0.01325 | 0.89531 | 1.48322 | 0.06848 | 0.06922 | 2.97283 |
| 5 | La2VScO6 | 0.42984 | 0.07343 | 1.42780 | 0.03685 | −0.00532 | −0.00506 | 2.01050 |
The transition metals Cr, Co, Ni, Sc, and V have the maximum contribution to the total spin magnetic moments, whereas La has the minimum contribution. In the La2CrCoO6 (LCCO) and La2CrNiO6 (LCNO) compounds, Cr has the maximum contribution to the total spin magnetic moment, whereas in the La2ScNiO6 (LSNO) and La2VNiO6 (LVNO) compounds, Ni has the maximum contribution. However, in the case of the La2VScO6 (LVSO) compound, V has the maximum contribution to the total spin magnetic moment. Furthermore, in all these compounds La2CrNiO6 (LCNO) has the maximum total spin magnetic moment of 4.0 μB, whereas La2ScNiO6 (LSNO) has the minimum total magnetic moment of 0.9 μB.
3.3. Optical properties
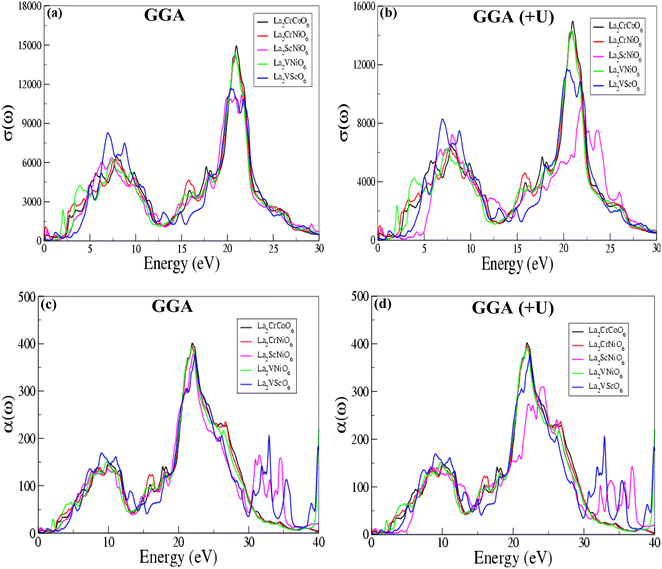
We have investigated the optical properties of the half-metallic ferromagnetic ordered double perovskite compounds La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6, including the optical conductivity σ(ω), reflectivity R(ω), refractive index n(ω), absorption co-efficient α(ω) and real ε1(ω) and imaginary ε2(ω) parts of the dielectric constant for various energies (in eV), via the GGA and GGA (+U) techniques. The calculated optical conductivity (σ(ω)) is illustrated in Fig. 5(a and b). The optical conductivity (σ(ω)) is the ratio of the current density to the electric field and depends on the frequency or energy of the incident photons, and is represented by the equation:| σ(ω) = J(ω)/E(ω) | (1) |
The calculated values of the optical conductivity (σ(ω)) are listed in Table 4, and show that the optical conductivity (σ(ω)) varies from 11.205 to 14.927. La2CrCoO6 (LCCO) has the maximum value of 14.927 at 20.993 eV, whereas La2ScNiO6 (LSNO) has the minimum value of 11.205 at 21.565 eV. The values of the optical conductivity calculated by using the GGA scheme for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds are 14.927, 14.181, 11.205, 14.377 and 11.680 at 20.993 eV, 20.911 eV, 21.565 eV, 20.830 eV and 20.367 eV. The values of the optical conductivity using the GGA (+U) scheme for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds are 14.958, 14.317, 9.286, 14.377 and 11.680 at 20.990 eV, 20.912 eV, 22.000 eV, 20.830 eV and 20.367 eV. The results obtained for the optical conductivity are nearly the same for both the GGA and GGA (+U) techniques. Furthermore, the value of the optical conductivity (σ(ω)) for La2VNiO6 (LVNO) and La2VScO6 (LVSO) is exactly same for both schemes. The absorption coefficients α(ω) calculated for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds shown in Fig. 5(c and d) quantify the incident light absorbed by the material (instead of being reflected or refracted), giving information about the transformation of electromagnetic energy into the internal energy of the absorber.
| Serial no. | Compound | GGA | GGA (+U) | GGA | GGA (+U) | ||||
|---|---|---|---|---|---|---|---|---|---|
| σ(ω) | E (eV) | σ(ω) | E (eV) | α(ω) | E (eV) | α(ω) | E (eV) | ||
| 1 | La2CrCoO6 | 14.927 | 20.993 | 14.958 | 20.990 | 401.082 | 21.973 | 401.871 | 21.973 |
| 2 | La2CrNiO6 | 14.181 | 20.911 | 14.317 | 20.912 | 394.128 | 21.918 | 395.603 | 21.918 |
| 3 | La2ScNiO6 | 11.205 | 21.565 | 9.286 | 22.000 | 378.128 | 22.136 | 376.096 | 22.204 |
| 4 | La2VNiO6 | 14.377 | 20.830 | 14.377 | 20.830 | 390.602 | 21.837 | 390.602 | 21.837 |
| 5 | La2VScO6 | 11.680 | 20.367 | 11.680 | 20.367 | 376.535 | 22.354 | 376.535 | 22.535 |
The values of the absorption coefficient α(ω) calculated via GGA and GGA (+U) listed in Table 4 indicate that of all five of these compounds, La2CrCoO6 (LCCO) has the maximum value of 401.082 at 21.973 eV, showing that this compound has more potential to absorb incident photons and can be used for absorption in optical devices.
The absorption power for incident photons in the La2VScO6 compound is the minimum (376.535 at 22.354 eV), indicating that this compound may be less useful for optical and optoelectronic applications than the other four compounds. The value of the energy observed for the maximum absorption coefficient (α(ω)) is in the range of 21 eV to 22.5 eV. Interestingly, the absorption coefficient (α(ω)) calculated for La2VNiO6 is α = 390.602, exactly the same for both schemes, and both schemes exhibit the same value of the energy (E = 21.837 eV). The values of the optical absorption coefficients α(ω) are specific for each compound, validating the potential application in optics where a specific ultraviolet radiation (UV) wavelength of absorption is required, such as in sunglasses, colored filters, dyes etc.
Fig. 6 represents the refractive indices (n(ω)) of all five compounds. The refractive index n(ω) is a critical feature of semiconductors, revealing the amount of light bent or refracted. The value of n(ω) increases with increasing energy (eV). The GGA and GGA (+U) calculations report higher values of the refractive index n(ω) for La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds of 6.005 (at 0.013 eV), 9.602 (at 0.038 eV), 4.928 (at 0.122 eV), 3.943 (at 0.013 eV) and 3.979 (at 0.013 eV), and 6.322 (at 0.013 eV), 8.732 (at 0.013 eV), 4.614 (at 0.149 eV), 3.820 (at 0.019 eV) and 3.979 (at 0.013 eV), indicating their potential use in optoelectronic applications, primarily in electronic displays, such as LCDs, OLEDs, and quantum dot (QDLED) televisions. The refractive index (n(ω)) calculated for La2CrNiO6 has the maximum value of 9.602 at 0.038 eV, whereas a minimum value of 3.943 at 0.013 eV is reported for the La2VNiO6 compound, as compared to the other compounds. There is no large difference between the refractive indices (n(ω)) calculated by GGA and GGA (+U). The refractive indices (n(ω)) measured for the La2VNiO6 and La2VScO6 compounds are nearly the same at 0.013 eV in the GGA scheme. The refractive indices (n(ω)) of La2CrCoO6 and La2ScNiO6 are equal to 6.0 and 4.9 at 0.013 eV and 0.122 eV, respectively. The n(ω) values observed for all these compounds are non-linear. Initially there is a sharp increase and then a decrease with increasing energy (eV), etc., which is in good agreement with previously reported theoretical and experimental results related to 2D double perovskites.40–44
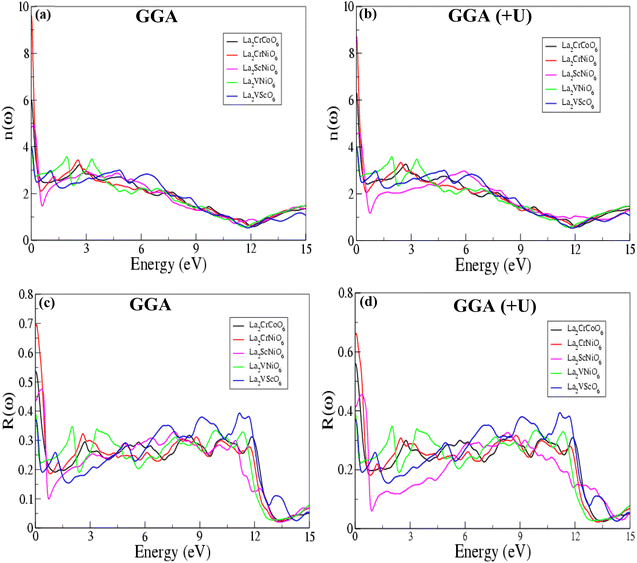 | ||
| Fig. 6 (a, b) Refractive index (n(ω)) and (c, d) reflectivity R(ω) of the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds calculated by the GGA and GGA (+U) processes. | ||
The optical reflectivity R(ω) values in relation to the incident energy calculated via GGA and GGA (+U) shown Fig. 6(c and d) confirm the reflective nature of these compounds. The optical reflectivity R(ω) is the ratio of the energy of a wave reflected from a surface to the energy possessed by the wave striking the surface. The GGA and GGA (+U) values of the reflectivity R(ω) for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds of 0.534, 0.696, 0.475, 0.389 and 0.369, and 0.558, 0.662, 0.454, 0.389 and 0.369 indicate that the surfaces of these materials are smooth and have an ordered crystalline structure. Among all five of these compounds, La2CrNiO6 has the maximum value of 0.696 at 0.04 eV, whereas La2VScO6 has the lowest value of 0.369 at 0.013 eV in both the GGA and GGA (+U) schemes.
The calculated real ε1(ω) and imaginary ε2(ω) parts of the dielectric constant as a function of energy (eV) calculated via both the GGA and GGA (+U) schemes are shown in Fig. 7. The dielectric constant, known as the relativity permittivity or specific inductive capacity, is a dimensionless number expressing a material's ability to support an electrostatic field while dissipating a minimum amount of energy in the form of heat. The calculated values of the static dielectric constants for the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds confirm that all these compounds display a dielectric response, and according to GGA approximations the values of the real parts of the dielectric constant ε1(ω) are in the range of 14.172 to 80.617, and the values of the imaginary parts are in the range of 7.867 to 78.097. Furthermore, according to the GGA (+U) scheme, the values of the real parts of the dielectric constant ε1(ω) are in the range of 14.172 to 68.613, and the values of the imaginary parts ε2(ω) are in the range of 7.867 to 58.682.
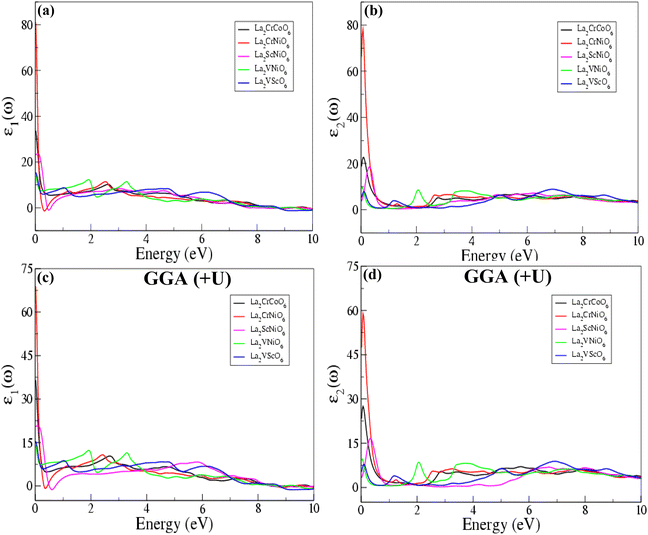 | ||
| Fig. 7 Real ε1(ω) and imaginary ε2(ω) parts of the dielectric constants of the La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 compounds calculated by the GGA and GGA (+U) processes. | ||
It is worth noting that the value of the ε2(ω) is zero until absorption starts after the photon energy reaches the band gap energy, which determines the threshold for a direct optical transition between the VBM and the CBM. The dielectric constant values describe the effect of the electric field on a material and the higher the dielectric constant, the more the material tends to reduce any field set up in it. In both the GGA and GGA (+U) processes, the calculated values of the real ε1(ω) and imaginary ε2(ω) parts of the dielectric constant for La2CrNiO6 (LCNO) are the highest, which indicates that this material is more dielectric than the others. La2VNiO6 (LVNO) is the least dielectric material, indicated by the obtained values of the dielectric constant. The materials with high dielectric constants are useful in the manufacture of high-value capacitors.
4. Conclusions
In this study, we investigated the influence of 3d transition metal incorporation at the B (Cr and V) and B′ (Co, Ni and Sc) sites of the 2D La2BB′O6 double perovskites on the electronic, magnetic, and optoelectronic properties. Full structural optimization was carried out by using the full-potential linearized augmented plane wave technique as implemented in WIEN2K software. The results are in good agreement with previously reported work. The electronic band structures in the spin-up and spin-down states reveal that all five of these compounds are half-metallic. In the case of the spin-up channel, La2CrCoO6, La2CrNiO6, La2ScNiO6, La2VNiO6 and La2VScO6 display metallic features, while they display non-metallic features in the spin-down channel. The calculated density of states gives a detailed explanation of the band structure, and the electronic distributions of these compounds drawn in terms of the total density of states verify the half-metallic nature. The pd-hybridizations, double exchange correlations (strong-correlation correction (GGA (+U))), ground-state energies and calculated total magnetic moments (2.9 μB, 2.0 μB, 0.9 μB, 4.0 μB and 2.8 μB) of these compounds confirm the ferromagnetic behavior. Therefore, the overall nature of these materials is half-metallic ferromagnetic. We have calculated the optical conductivities σ(ω) and absorption co-efficients α(ω), and the maximum values are 14.927 and 401.08 at 21.97 eV for the La2CrCoO6 compound. The refractive index (n(ω)) and optical reflectivity (R(ω)) calculations reveal that La2CrNiO6 has the highest values of 9.602 at 0.038 eV and 0.696 at 0.04 eV, whereas La2VNiO6 has the lowest values of 3.943 at 0.013 eV and 0.389 at 0.013 eV, compared to all the other compounds. The real and imaginary parts of the dielectric constant show that La2CrNiO6 has the maximum value, which means that this compound is more dielectric than the others. The fully spin-polarized strong magnetic element induces the weak magnetic element, and slightly increases the positive (or negative) magnetic moment, therefore it is regarded as the main cause of the half-metallic properties. In summary, based on our calculations, we revealed that 2D La2BB′O6 double perovskites with 3d transition metals incorporated at the B (Cr and V) and B′ (Co, Ni and Sc) sites have potential applications in spintronic, multifunctional, and optoelectronic devices.Data availability
The raw/processed data required to reproduce these findings cannot be shared at this time as the data also forms part of an ongoing study. The data may be provided on request.Conflicts of interest
I hereby confirm that the work reported in this manuscript is novel and has no conflict of interest.Acknowledgements
All my colleagues and fellows from the Material Modelling Lab are acknowledged for valuable suggestions and comments in this research work.References
- J. Zhu, H. Li, L. Zhong, P. Xiao, X. Xu, X. Yang, Z. Zhao and J. Li, Perovskite oxides: preparation, characterizations, and applications in heterogeneous catalysis, ACS Catal., 2014, 4(9), 2917–2940 CrossRef CAS.
- Properties and Applications of Perovskite-Type Oxides, ed. L. G. Tejuca and J. L. Fierro, CRC Press, 2000 Search PubMed.
- E. Grabowska, Selected perovskite oxides: characterization, preparation and photocatalytic properties—a review, Appl. Catal., B, 2016, 186, 97–126 CrossRef CAS.
- M. Acosta, N. Novak, V. Rojas, S. Patel, R. Vaish, J. Koruza, G. A. Rossetti Jr and J. Rödel, BaTiO3-based piezoelectrics: fundamentals, current status, and perspectives, Appl. Phys. Rev., 2017, 4, 041305 Search PubMed.
- P. Wang, Q. Guo, F. Li, F. Xia, H. Hao, H. Sun, H. Liu and S. Zhang, Modified Pb(Mg1/3Nb2/3)O3-PbZrO3–PbTiO3 ceramics with high piezoelectricity and temperature stability, J. Am. Ceram. Soc., 2021, 104(10), 5127–5137 CrossRef CAS.
- Q. Guo, F. Li, F. Xia, X. Gao, P. Wang, H. Hao, H. Sun, H. Liu and S. Zhang, High-performance Sm-doped Pb(Mg1/3Nb2/3)O3-PbZrO3-PbTiO3-based piezoceramics, ACS Appl. Mater. Interfaces, 2019, 11(46), 43359–43367 CrossRef CAS PubMed.
- C. J. Howard, B. J. Kennedy and P. M. Woodward, Ordered double perovskites – a group-theoretical analysis, Acta Crystallogr., Sect. B: Struct. Sci., 2003, 59(4), 463–471 CrossRef PubMed.
- A. E. Maughan, A. M. Ganose, D. O. Scanlon and J. R. Neilson, Perspectives and design principles of vacancy-ordered double perovskite halide semiconductors, Chem. Mater., 2019, 31(4), 1184–1195 CrossRef CAS.
- S. Nazir, Insulator-to-half metal transition and enhancement of structural distortions in [… formula…] double perovskite oxide via hole-doping, Sci. Rep., 2021, 11, 1240 CrossRef CAS PubMed.
- H. Kato, T. Okuda, Y. Okimoto, Y. Tomioka, K. Oikawa, T. Kamiyama and Y. Tokura, Metal-insulator transition of ferromagnetic ordered double perovskites:(Sr1−yCay)2FeReO6, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65(14), 144404 CrossRef.
- W. Prellier, V. Smolyaninova, A. Biswas, C. Galley, R. L. Greene, K. Ramesha and J. Gopalakrishnan, Properties of the ferrimagnetic double perovskites A2FeReO6 (A = Ba and Ca), J. Phys.: Condens. Matter, 2000, 12(6), 965 CrossRef CAS.
- I. V. Solovyev, Electronic structure and stability of the ferrimagnetic ordering in double perovskites, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65(14), 144446 CrossRef.
- M. Hashisaka, D. Kan, A. Masuno, M. Takano, Y. Shimakawa, T. Terashima and K. Mibu, Epitaxial growth of ferromagnetic La2NiMnO6 with ordered double-perovskite structure, Appl. Phys. Lett., 2006, 89(3), 032504 CrossRef.
- E. Carvajal, O. Navarro, R. Allub, M. Avignon and B. Alascio, Ferromagnetic transition in ordered double perovskites and related alloys, Eur. Phys. J. B, 2005, 48(2), 179–187 CrossRef CAS.
- D. D. Russell, A. J. Neer, B. C. Melot and S. Derakhshan, Long-range antiferromagnetic ordering in b-site ordered double perovskite Ca2ScOsO6, Inorg. Chem., 2016, 55(5), 2240–2245 CrossRef CAS PubMed.
- L. Ortega-San Martin, J. P. Chapman, L. Lezama, J. Sánchez Marcos, J. Rodríguez-Fernández, M. I. Arriortua and T. Rojo, Magnetic properties of the ordered double perovskite Sr2MnTeO6, Eur. J. Inorg. Chem., 2006, 7, 1362–1370 CrossRef.
- M. R. Li, M. Retuerto, Z. Deng, P. W. Stephens, M. Croft, Q. Huang, H. Wu, X. Deng, G. Kotliar, J. Sánchez-Benítez and J. Hadermann, Giant magnetoresistance in the half-metallic double-perovskite ferrimagnet Mn2FeReO6, Angew. Chem., Int. Ed., 2015, 54(41), 12069–12073 CrossRef CAS PubMed.
- F. Estrada, E. J. Guzmán, O. Navarro and M. Avignon, Curie temperature behavior in half-metallic ferromagnetic double perovskites within the electronic correlation picture, Phys. Rev. B, 2018, 97(19), 195155 CrossRef CAS.
- O. Erten, O. N. Meetei, A. Mukherjee, M. Randeria, N. Trivedi and P. Woodward, Theory of half-metallic ferrimagnetism in double perovskites, Phys. Rev. Lett., 2011, 107(25), 257201 CrossRef PubMed.
- Q. Tang and X. Zhu, Half-metallic double perovskite oxides: recent developments and future perspectives, J. Mater. Chem. C, 2022, 15301–15338 RSC.
- N. Rahmani, M. E. Ghazi, M. Izadifard, D. Wang, A. Shabani and B. Sanyal, Density functional study of structural, electronic and magnetic properties of new half-metallic ferromagnetic double perovskite Sr2MnVO6, J. Phys.: Condens. Matter, 2019, 31(47), 475501 Search PubMed.
- O. N. Meetei, O. Erten, A. Mukherjee, M. Randeria, N. Trivedi and P. Woodward, Theory of half-metallic double perovskites. I. Double exchange mechanism, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87(16), 165104 CrossRef.
- Q. Tang and X. Zhu, Half-metallic double perovskite oxides: recent developments and future perspectives, J. Mater. Chem. C, 2022, 15301–15338 RSC.
- G. M. Mustafa, M. Hassan, N. M. Aloufi, S. Saba, S. Al-Qaisi, Q. Mahmood, H. Albalawi, S. Bouzgarrou, H. H. Somaily and A. Mera, Half metallic ferroamgnetism, and transport properties of vacancy ordered double perovskites Rb2(Os/Ir)X6 (X = Cl, Br) for spintronic applications, Ceram. Int., 2022, 23460–23467 CrossRef CAS.
- T. Alshahrani, Q. Mahmood and M. Rashid, The role of 5d electrons spin in quantum ferromagnetism and transport properties of double perovskites Cs2ZCl/Br6 (Z = Ta, W) for spintronic applications, Eur. Phys. J. Plus, 2021, 136(3), 1–2 Search PubMed.
- J. B. Philipp, P. Majewski, L. Alff, A. Erb, R. Gross, T. Graf, M. S. Brandt, J. Simon, T. Walther, W. Mader, D. Topwal and D. D. Sarma, Structural and doping effects in the half-metallic double perovskite A2CrWO6 (A =Sr, Ba, and Ca), Phys. Rev. B: Condens. Matter Mater. Phys., 2003 Oct, 68, 144431 Search PubMed.
- Y. P. Liu, S. H. Chen, H. R. Fuh and Y. K. Wang, First-principle calculations of half-metallic double perovskite La2BB′O6 (B, B′ = 3d transition metal), Commun. Comput. Phys., 2013, 14(1), 174–185 CrossRef.
- U. R. Mehtab, W. Qun and Y. Yunfei, Electronic, Magnetic and Optical Properties of Double Perovskite Compounds: A First Principle Approach, Crystals, 2022, 12(11), 1597 CrossRef.
- J. H. Park, S. K. Kwon and B. I. Min, Half-metallic antiferromagnetic double perovskites: LaAVRuO6 (A = Ca, Sr, and Ba), Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65(17), 174401 Search PubMed.
- S. H. Chen, Z. R. Xiao, P. H. Lee, Y. P. Liu and Y. K. Wang, Stability of half-metallic antiferromagnet La2VMnO6, first-principles calculation study, Phys. B, 2011, 406(14), 2783–2787 CrossRef CAS.
- D. D. Sarma, E. V. Sampathkumaran, S. Ray, R. Nagarajan, S. Majumdar, A. Kumar, G. Nalini and T. G. Row, Magnetoresistance in ordered and disordered double perovskite oxide, Sr2FeMoO6, Solid State Commun., 2000, 114(9), 465–468 Search PubMed.
- J. P. Perdew, K. Burke and M. Ernzerhof, Generalized gradient approximation made simple, Phys. Rev. Lett., 1996, 77(18), 3865 CrossRef CAS PubMed.
- K. Burke, J. P. Perdew and M. Ernzerhof, Why the generalized gradient approximation works and how to go beyond it, Int. J. Quantum Chem., 1997, 61(2), 287–293 CrossRef CAS.
- Z. Wu and R. E. Cohen, More accurate generalized gradient approximation for solids, Phys. Rev. B: Condens. Matter Mater. Phys., 2006, 73(23), 235116 CrossRef.
- S. Bo and Z. Ping, First-principles local density approximation (LDA) + U and generalized gradient approximation (GGA) + U studies of plutonium oxides, Chin. Phys. B, 2008, 17(4), 1364 Search PubMed.
- Y. Guermit, M. Drief, T. Lantri, A. Tagrerout, H. Rached, N. E. Benkhettou and D. Rached, Theoretical investigation of magnetic, electronic, thermoelectric and thermodynamic properties of Fe2TaZ (Z = B, In) compounds by GGA and GGA + U approaches, Comput. Condens. Matter, 2020, 22, e00438 CrossRef.
- S. A. Mir and D. C. Gupta, Understanding the origin of half-metallicity and thermophysical properties of ductile La2CuMnO6 double perovskite, Int. J. Energy Res., 2019, 43(9), 4783–4796 CrossRef CAS.
- S. Satpathy, Z. S. Popović and F. R. Vukajlović, Density-functional studies of the electronic structure of the perovskite oxides: La1−xCax MnO3, J. Appl. Phys., 1996, 79(8), 4555–4557 CrossRef CAS.
- M. U. Rehman, Q. Wang and Y. Yu, Electronic, Magnetic and Optical Properties of Double Perovskite Compounds: A First Principle Approach, Crystals, 2022, 12(11), 1597 Search PubMed.
- S. Berri, N. Bouarissa, M. Ibrir and O. Meglali, Optical spectra and thermal properties of double perovskite Ba2LuTaO6 material, Optik, 2021, 229, 166272 CrossRef CAS.
- S. A. Dar, R. Sharma, V. Srivastava and U. K. Sakalle, Investigation on the electronic structure, optical, elastic, mechanical, thermodynamic and thermoelectric properties of wide band gap semiconductor double perovskite Ba2InTaO6, RSC Adv., 2019, 9(17), 9522–9532 Search PubMed.
- M. Hussain, M. Rashid, A. Ali, M. F. Bhopal and A. S. Bhatti, Systematic study of optoelectronic and transport properties of cesium lead halide (Cs2PbX6; X = Cl, Br, I) double perovskites for solar cell applications, Ceram. Int., 2020, 46(13), 21378–21387 CrossRef CAS.
- O. T. Ali, M. R. Mohamed, M. H. Ali, M. E. Elsayed and B. Mikhael, Optical, electrical and magnetic properties of lanthanum strontium manganite La1−xSrxMnO3 synthesized through the citrate combustion method, Phys. Chem. Chem. Phys., 2017, 19, 6878–6886 RSC.
- I. Koriba, B. Lagoun, A. Cheriet, G. Abdenacer, B. Soraya, A. Anfel, A. Linda and A. Akram, Phase stability, mechanical and optoelectronic properties of lanthanum chromite-based perovskite oxide, Appl. Phys. A, 2022, 128, 82 CrossRef CAS.
| This journal is © The Royal Society of Chemistry 2022 |