Open Access Article
Open Access ArticleUnderstanding nascent plasmons and metallic bonding in atomically precise gold nanoclusters†
Xiangsha
Du‡
,
Zhongyu
Liu‡
 ,
Tatsuya
Higaki
,
Meng
Zhou
,
Tatsuya
Higaki
,
Meng
Zhou
 and
Rongchao
Jin
and
Rongchao
Jin
 *
*
Department of Chemistry, Carnegie Mellon University, Pittsburgh, Pennsylvania 15213, USA. E-mail: rongchao@andrew.cmu.edu
First published on 13th January 2022
Abstract
The metallic bond is arguably the most intriguing one among the three types of chemical bonds, and the resultant plasmon excitation (e.g. in gold nanoparticles) has garnered wide interest. Recent progress in nanochemistry has led to success in obtaining atomically precise nanoclusters (NCs) of hundreds of atoms per core. In this work, thiolate-protected Au279(SR)84 and Au333(SR)79 NCs, both in the nascent metallic state are investigated by cryogenic optical spectroscopy down to 2.5 K. At room temperature, both NCs exhibit distinct plasmon resonances, albeit the NCs possess a gap (estimated 0.02–0.03 eV, comparable to thermal energy). Interestingly, we observe no effect on plasmons with the transition from the metallic state at r.t. to the insulating state at cryogenic temperatures (down to 2.5 K), indicating a nonthermal origin for electron-gas formation. The electronic screening-induced birth of metallic state/bonding is discussed. The obtained insights offer deeper understanding of the nascent metallic state and covalent-to-metallic bonding evolution, as well as plasmon birth from concerted excitonic transitions.
Introduction
Metallic gold nanoparticles (e.g., 3–100 nm diameter) show elegant surface plasmon resonance (SPR) in the visible wavelength range, which has stimulated great research interest since Faraday's early scientific work in the mid-19th century.1–4 In particular, the recent two decades have witnessed intensive research on shape control, hierarchical assembly, and other aspects of nanoparticles, as well as their applications in catalysis, nanoelectronics, optics, sensing and biomedicine.Toward the smaller end of gold NP sizes (i.e., <3 nm), the SPR gradually fades out with decreasing size due to the quantum size effect,5–12 hence, exhibiting a transition from the metallic to the nonmetallic state; the latter is manifested in the emergence of a distinct bandgap or a HOMO–LUMO gap (Eg).13–22 On that note, the term ‘nonmetallic’ is equivalent to molecular, semiconducting, or insulating, and all of them have been used in the literature. If one starts with the smallest cluster (i.e. diatomic Au2), the bond in Au2 is apparently a covalent bond (being nonmetallic), and that in Mg2 is van der Waals interaction.23 With increasing number of atoms in the cluster, the Eg value decreases generally (albeit with some local zig-zag behavior24), and over the size range of tens to hundreds of metal atoms the bonding orbitals become more and more closely spaced, ultimately forming a quasi-continuous band (i.e. valence band), and similarly the antibonding orbitals give rise to the conduction band. When the gap between the two bands is so small, thermal excitation of valence electrons in fully occupied levels into the upper levels becomes predominant and the metallic state is formed.
Theoretically, by setting up the one-electron Schrödinger equation for electrons and ignoring the electron–electron and electron–nuclear interactions (potential energy V = 0), one obtains the equation for a single valence electron (mass m) confined in a volume,
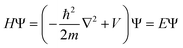 | (1) |
For a cubic system of edge length a, the equation can be readily solved to obtain the energy (En) for quantized levels of the electron,
 | (2) |
For the spherical coordinate system (radius a for the sphere), one can similarly solve the one-electron Schrödinger equation and obtain,
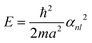 | (3) |
With the discrete values of nx,y,z or αn,l, one can construct the energy-level diagram. The average spacing (δ) between successive levels can be estimated from the reciprocal of the density of states according to eqn (2),25,26
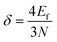 | (4) |
| Eg = δ < kBT (Kubo criterion) | (5) |
In recent years, solution phase nanochemistry has advanced significantly,2 and recent progress has indeed led to the development of atomically precise metal nanoclusters (NCs) protected by ligands.10–22 Unlike the gas phase NCs for which spectroscopic probing is quite challenging,32 solution phase NCs can be readily subjected to any spectroscopic characterization.33–37 In addition, solution phase syntheses can be readily scaled up (e.g., milligrams to grams38). The creation of size-discrete NCs in the protected form opens up opportunities for probing the properties of the nascent metallic state and metallic bond formation in ultrasmall NCs.39–41
In this work, we report the cryogenic spectroscopic probing of whether the nascent metallic state in gold NPs is temperature dependent or not by utilizing atomically precise gold NCs. Two sizes, Au279(SR)84 and Au333(SR)79, are selected for this study, together with a nonmetallic Au144(SR)60 NC for comparison. Surprisingly, although the gaps of Au279 and Au333 are of ∼kBT at room temperature (kBT at r.t. ∼25 meV), no effect on plasmon resonance from the transition to an insulating state at cryogenic temperatures is observed by monitoring the SPR (a signature of the electron-gas), even when the temperature is decreased to 2.5 K (i.e., ∼0.2 meV), at which the Eg is already >100 times larger than the NC's thermal energy (i.e., nonmetallicity by eqn (5)). Based on the result, we further discuss some insights into metallic bond formation and nascent plasmon resonance emerged from coherent excitons.
Results and discussion
The Au144(PET)60 and Au333(PET)79 NCs (where PET = SCH2CH2Ph) were synthesized by a size-focusing methodology reported in our previous work.40,42,43 Briefly, a narrow size-distributed Aux(PET)y mixture was first synthesized following the method of kinetic control, and such a crude product was then subject to size-focusing (e.g. thermal reaction with excess PET thiol at 80 °C for 2 days). The resulting Au144 and Au333 were separated by thin-layer chromatography (TLC, with CH2Cl2/methanol (6![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, v/v) as the eluent). The Au279(TBBT)84 (where TBBT = S-Ph-p-C(CH3)3) was synthesized by the ligand exchange-induced size transformation method.40,44 More experimental details, including the characterization, are provided in the ESI.†
1, v/v) as the eluent). The Au279(TBBT)84 (where TBBT = S-Ph-p-C(CH3)3) was synthesized by the ligand exchange-induced size transformation method.40,44 More experimental details, including the characterization, are provided in the ESI.†
Mass spectrometry and room temperature optical spectroscopy analyses
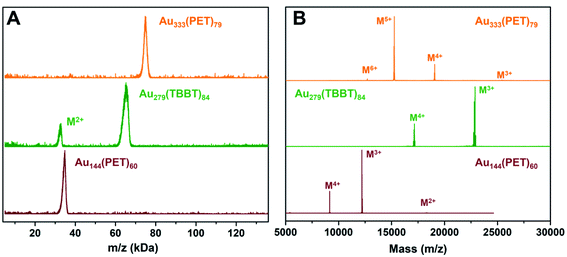
The high purity of the three NCs is confirmed by matrix-assisted laser desorption ionization (MALDI) and electrospray ionization (ESI) mass spectrometry analyses. As can be seen in Fig. 1A, MALDI analyses show a narrow peak at ∼35 kDa for Au144(PET)60 and a ∼75 kDa peak for Au333(PET)79, whereas the MALDI spectrum of Au279(TBBT)84 shows a 1+ charged peak at ∼65 kDa (intense) and also a 2+ peak at ∼32.5 kDa (weak). No impurity was observed in the wide-range MALDI spectra. The precise masses of the NCs were determined by ESI-MS (Fig. 1B). The Au144(PET)60 spectrum shows two dominant peaks of molecular ions at m/z 9149 (M4+) and 12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 199 (M3+), plus a minor peak at 18
199 (M3+), plus a minor peak at 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 297 corresponding to the 2+ ion [Au144(PET)60]2+. These ions are formed under the ESI conditions, as the native state is charge-neutral. All three peaks are consistent with the expected formula weight of Au144(PET)60 (FW = 36
297 corresponding to the 2+ ion [Au144(PET)60]2+. These ions are formed under the ESI conditions, as the native state is charge-neutral. All three peaks are consistent with the expected formula weight of Au144(PET)60 (FW = 36![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 597.48 Da). The Au279(TBBT)84 (FW = 68
597.48 Da). The Au279(TBBT)84 (FW = 68![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 835.77 Da) spectrum shows peaks at m/z 17
835.77 Da) spectrum shows peaks at m/z 17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 209 (4+) and 22
209 (4+) and 22![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 945 (3+); note that the 2+ and 1+ peaks are beyond the mass detector's range and species of higher charges are not observed due to much less abundances under our ESI conditions. Finally, the ESI spectrum of Au333(PET)79 (FW = 76
945 (3+); note that the 2+ and 1+ peaks are beyond the mass detector's range and species of higher charges are not observed due to much less abundances under our ESI conditions. Finally, the ESI spectrum of Au333(PET)79 (FW = 76![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 430.23 Da) shows predominant mass peaks at m/z 15
430.23 Da) shows predominant mass peaks at m/z 15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 286 (5+) and 19
286 (5+) and 19![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 108 (4+). The three NCs are all charge neutral in their native state.
108 (4+). The three NCs are all charge neutral in their native state.
The UV-vis absorption spectrum of Au144 (dissolved in CH2Cl2) exhibits multiple peaks at 337, 463, 517, and 700 nm (Fig. 2A), consistent with previous reports.43,45 It is worth noting that Au144 shows extra peaks in the near-infrared (NIR) range (ESI Fig. S1†). The multi-peak feature indicates the nonmetallic state of Au144, that is, Au144 possesses discrete electronic energy levels and gives rises to single-electron transitions (excitons), much like small molecules. Recent electrochemical measurements on Au144 indeed revealed a 0.17 eV HOMO–LUMO gap at room temperature (r.t.),46 and the gap became slightly larger with decreasing temperature, resembling the semiconductor behavior.33 In contrast, Au279 and Au333 both show a nascent SPR peak at ∼500 nm (Fig. 2B and C),40,47 and no measurable gap was observed in electrochemical measurements.46 According to the independent-electron theory (eqn (4)), there should be an ultrasmall gap in metallic Au279 and Au333, as well as nonmetallic Au246(SR)80 (its nonmetallicity confirmed by ultrafast spectroscopy analyses48), since they are finite systems. However, ultrasmall gaps (e.g. at the meV scale or kBT) is beyond the detection limit of electrochemical and spectroscopic measurements.46,48 Perhaps scanning tunneling spectroscopy at cryogenic temperatures or even millikelvin temperatures (e.g. using a dilution refrigerator as in quantum computing systems) may permit the measurement of the meV gaps.
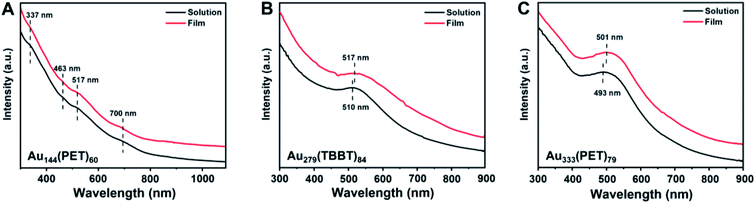 | ||
| Fig. 2 Room temperature optical absorption spectra of (A) Au144(PET)60, (B) Au279(TBBT)84, and (C) Au333(PET)79 in the solid film and solution, respectively. | ||
Cryogenic spectroscopy probing of NCs down to 2.5 K
To test the possible temperature dependence of the nascent metallic state, we carried out cryogenic optical spectroscopy measurements (from r.t. down to 2.5 K). On that note, the cuvette-based measurement was only successful down to ∼70 K but failed at lower temperatures due to shattering of cuvettes (even the cryogenic compatible ones), thus we switched to the thin-film based measurement for temperatures down to 2.5 K. The NCs are uniformly embedded in polystyrene thin films to acquire high quality spectra. At r.t., the film's optical spectra are almost superimposable to the solution spectra (Fig. 2A–C), albeit with a slight broadening of peaks. A sub-10 nm shift of absorption maxima is observed in Au279 and Au333, whereas the peak shift in Au144 is trivial. For nonmetallic Au144, the difference between the solid film and the solution spectra can be ascribed to the coupling of electronic states with extra vibrational states induced by stronger inter-molecular interactions in the film state; whereas for Au279 and Au333, a redshift of SPR is caused by the larger dielectric constant of the film, and a slight broadening of SPR due to accelerated ultrafast electron dynamics.6The absorption spectra of the three film samples from r.t. down to 2.5 K are shown in Fig. 3A–C. For nonmetallic Au144(PET)60, the bands at 517 nm and 700 nm clearly show a blueshift and become sharpened as the temperature decreases from r.t. to 2.5 K, and the peak shift fitting49,50 reveals a 15 meV vibrational mode coupled with the 700 nm peak, indicating a significant contribution of electron-phonon interaction. This phenomenon disagrees with Kubo and Kreibig's earlier prediction, i.e., they believed that the electron-phonon scattering should be suppressed in small-sized NPs (e.g. the number of Au atoms < 240).51 An explanation for this contradiction is that the interaction between the metal core and the protecting ligands provides an extra energy relaxation pathway. In our measurements, several new features appear in the absorption spectrum of Au144(PET)60 at cryogenic temperatures, which provides strong evidence for the discrete energy levels in Au144(PET)60, consistent with the cryogenic measurements by Weissker and Negishi et al. (their temperatures were only down to tens of kelvins).52,53 In contrast, the SPR peak of metallic-state Au333(PET)79 and Au279(TBBT)84 NCs shows no shift, except for a small increase in intensity and a negligible narrowing of bandwidth with the temperature down to 2.5 K. Similar trends were previously observed in larger sized, polydispersed Au NPs.51
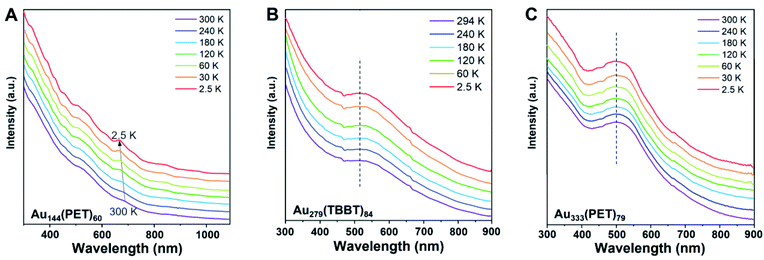 | ||
| Fig. 3 Cryogenic optical absorption spectra of (A) Au144(PET)60, (B) Au279(TBBT)84, and (C) Au333(PET)79 in solid films (r.t. to 2.5 K, NCs being embedded in thin films of polystyrene). | ||
It is interesting to observe no effect on the nascent SPR in Au333(PET)79 and Au279(TBBT)84 with their transition from the metallic to insulating state over the temperature decrease from r.t. to 2.5 K. We note that a temperature-dependent transition in a single La@C60 cluster was reported,54 in which the scanning tunneling measurements of current–voltage (I–V) curves of La@C60 on graphite revealed a metal-to-semiconductor transition at 28 K, with the semiconducting state having a 40 meV gap. Of course, a major difference in our system is that the Au333(PET)79 and Au279(TBBT)84 NCs are optically excited (i.e. much higher excitation energy than the ultrasmall meV gaps).
Here we estimate the Eg of the three NCs by using the Kubo formula, Ef/N (note: the factor of 4/3 is dropped for estimation purposes). The first estimation takes all the gold atoms in the particle to be the N value in the Kubo formula (Table 1), while the second estimation takes the difference between the number of gold atoms and the number of ligands under the assumption that each thiolate ligand consumes one valence electron of gold in forming the Au–S covalent bond. The Eg values are compiled in Table 1, from which the case of Au144 shows a deviation of a factor of ∼2 when compared with the experimental value, while there is no accurately measured Eg of Au279 and Au333 for a comparison. The estimated Eg of Au279 and Au333 are comparable to the r.t. thermal energy (0.025 eV), which seems to explain the observed SPR and metallicity at r.t., because the r.t. thermal energy can effectively excite the free valence electrons to form an electron-gas. However, when the temperature is decreased, the electron-gas state would be destroyed since the electrons can no longer be excited thermally (Scheme 1); for example, at 2.5 K, the thermal energy is only ∼0.0002 eV, about 150 times smaller than the Eg values (∼0.03 eV for Au279 and Au333), and thus it would be impossible to stir up the orbital-held electrons into an electron-gas. Therefore, one expects that the Au333 and Au279 transition to an insulating state at 2.5 K (Scheme 1), and accordingly it would exert some effects on the SPR, but no change to SPR was observed in our cryogenic probing. This implies that both Au279 and Au333 NCs, albeit with a nonzero HOMO–LUMO gap of ∼meV, can sustain the plasma state (i.e. the electron-gas) without thermal contribution (Scheme 1).
| Au144(SR)60 (1.7 nm core) | Au279(SR)84 (2.2 nm) | Au333(SR)79 (2.3 nm) | |
|---|---|---|---|
| a n.d. = not determined, i.e. beyond the detection limit of electrochemical analyses. | |||
| E g by taking the total number of gold atoms | 0.038 eV | 0.020 eV | 0.016 eV |
| E g by taking the number of gold atoms subtracting the number of ligands | 0.065 eV | 0.028 eV | 0.022 eV |
| Experimental Eg at r.t. | 0.17 eV | n.d. | n.d. |
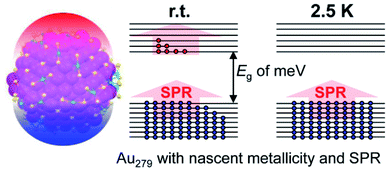 | ||
| Scheme 1 Thermal excitation of electrons and the nascent plasma state in Au279. The block arrow represents plasmon excitation as opposed to single-electron (excitonic) excitation by incident light. | ||
The one-electron treatment adopts a free-electron-gas model and does not consider the electron–electron and electron–ion interactions.26 A higher order theory (e.g. the Fermi liquid model26,55) might be able to provide more insights into the temperature-dependent metallic/insulating states in finite systems. The above cryogenic spectroscopy result implies a nonthermal origin for electron-gas formation. When the energy level spacing is on the meV scale, the broadening of energy-levels should be taken into consideration, and the potential factors are the excited state lifetime-induced broadening, electron–phonon coupling, electronic structure renormalization upon excitation, etc., all being on the meV scale, so that the meV HOMO–LUMO gap becomes smeared out, even in the absence of thermal excitation. In previous theoretical modeling using density functional theory (DFT), some researchers reported that the plasmon state would be formed in Au144 and even the smaller Au133 NC,56,57 but recent careful measurements do not support the theory because of the distinct gaps observed in these two NCs.46 In addition, both NCs also showed molecular-like electron dynamics,33 as well as luminescence,46 hence, they are not in the metallic state. For large sizes of NCs (e.g. more than one hundred atoms of gold), it is still a major challenge to perform DFT analysis with sufficient accuracy. Thus, developing new methods is highly desired, and such efforts will contribute to addressing the fundamentals of metallic-state formation with increasing size.58,59
Origin of nascent SPR
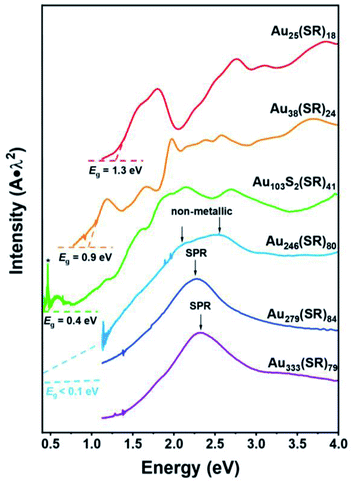
Here we further discuss the evolution of Eg and optical absorption spectra of gold nanoclusters with increasing size (Fig. 4). Smaller NCs (n < 100 or so) possess distinct Eg, such as Au103 with a 0.4 eV gap (Fig. 4, green curve). In our recent work we observed a sharp transition from nonmetallic Au246(SR)80 to metallic Au279(SR)84.40 The Au246 NC exhibits multiple peaks (i.e. multi-excitons) as revealed in both the steady-state and femtosecond transient absorption spectra, and its ultrafast electron relaxation dynamics showed no excitation power dependence, but with a mere 33-atom increase, the excitonic picture disappeared in Au279 — which exhibited a single SPR peak at ∼500 nm and power dependence in ultrafast electron dynamics.40 It seems that the excitons in NCs larger than Au246 start to “communicate” and develop a coherence, and the concerted excitonic transitions are generated and manifested as a plasmon excitation in Au279 (Fig. 4). The development of such coherence and oscillator energy merging into SPR energy (∼2.5 eV) is intriguing (Fig. 4, comparing the Au246 and Au279 spectra).In small NCs, a long-range electron–electron repulsion is present, i.e.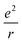 (where e is the electron charge and r is the distance). With increasing size, the electronic screening effect builds up and leads to weak short-range forces, i.e.
(where e is the electron charge and r is the distance). With increasing size, the electronic screening effect builds up and leads to weak short-range forces, i.e. , where l is the screening length which is dependent on the electronic density (i.e. the higher the electronic density, the shorter the l, hence, the electron's Coulomb interaction becomes short-ranged). Ultimately, the strong screening effect leads to the formation of nearly independent electrons (i.e. the electron-gas). From the optical absorption spectra, we observed that in NCs larger than Au103, long-wavelength absorption with relatively large oscillator strength starts to retreat toward shorter wavelengths (Fig. 4), indicating the excitons' peak energy focusing. The free propagation or ‘roaming’ of electrons in larger NCs such as Au246 leads to stronger electronic screening, hence the merging of various excitons, and finally, giving rise to plasmonic excitation in Au279. It remains to be elucidated whether the concerted excitonic excitations are solely induced by the increased electronic density with increasing size, or involve some other subtle factors. Heteroatom doping of Au279 might provide more insights.
, where l is the screening length which is dependent on the electronic density (i.e. the higher the electronic density, the shorter the l, hence, the electron's Coulomb interaction becomes short-ranged). Ultimately, the strong screening effect leads to the formation of nearly independent electrons (i.e. the electron-gas). From the optical absorption spectra, we observed that in NCs larger than Au103, long-wavelength absorption with relatively large oscillator strength starts to retreat toward shorter wavelengths (Fig. 4), indicating the excitons' peak energy focusing. The free propagation or ‘roaming’ of electrons in larger NCs such as Au246 leads to stronger electronic screening, hence the merging of various excitons, and finally, giving rise to plasmonic excitation in Au279. It remains to be elucidated whether the concerted excitonic excitations are solely induced by the increased electronic density with increasing size, or involve some other subtle factors. Heteroatom doping of Au279 might provide more insights.
From the experiments, gold NCs with an ultrasmall Eg of ∼0.02–0.03 eV (comparable to kBT at r.t.) seem to already reach the metallic state or metallic bonding. Both Au279 and Au333 should transition to an insulating state at 2.5 K according to the Kubo criterion, albeit no effect on SPR is observed in cryogenic spectroscopic probing. The nonzero Eg in finite-sized Au279 and Au333 NCs is in contrast to bulk metals in which Eg = 0. In future work, it would be worthwhile to carry out far-infrared and terahertz absorption measurements to directly probe the ultrasmall Eg, as opposed to the high energy excitation in UV-vis-NIR spectroscopy analysis. Future theoretical work may also reveal more insights into the birth of coherence among the valence electrons with a build-up of electronic screening, the emergence of nascent plasmon resonances, and also the new properties of nascent plasmons.
Conclusions
In summary, cryogenic spectroscopy of Au279 and Au333 in the size domain (2.2 to 2.5 nm) provides insights into the temperature effect on the metallic state or metallic bonding in ultrasmall particles. Au279 and Au333 show nascent metallicity at r.t. by exhibiting collective excitation of electrons (i.e. SPR), but no effect on SPR is observed from the metal-to-insulator transition (Eg ≫ kBT) when the temperature is decreased to 2.5 K, at which the thermal energy (kBT = 0.0002 eV) is already significantly smaller than the estimated Kubo gaps (0.02–0.03 eV) of Au279 and Au333. The sustained electron-gas state in these ultrasmall NPs from r.t. down to 2.5 K implies a nonthermal mechanism for electron-gas formation. The electronic density-dependent screening of Coulomb repulsions between electrons leads to the formation of short-ranged electronic interactions with increasing size, hence, the development of an electron-gas. The coherence among excitons leads to the birth of SPR. The obtained insights contribute to the understanding of the fundamental metallic bond formation and electronic interactions, and also benefit the future applications of nanocluster materials in solar energy conversion, plasmonics, photocatalysis, sensing, and other fields.Data availability
All the data have been included in the ESI.†Author contributions
X. D. and T. H. performed the synthesis, Z. L. carried out cryogenic spectroscopy measurements, M. Z. collected some of the r.t. spectra, and R. J. designed the project. All authors discussed the results and contributed to the manuscript writing.Conflicts of interest
There are no conflicts to declare.Acknowledgements
R. J. is thankful for the financial support from the National Science Foundation (DMR-1808675).References
- Y. W. C. Cao, R. Jin and C. A. Mirkin, Nanoparticles with Raman Spectroscopic Fingerprints for DNA and RNA Detection, Science, 2002, 297, 1536–1540 CrossRef CAS PubMed.
- R. Jin, C. Zeng, M. Zhou and Y. Chen, Atomically Precise Colloidal Metal Nanoclusters and Nanoparticles: Fundamentals and Opportunities, Chem. Rev., 2016, 116, 10346–10413 CrossRef CAS PubMed.
- G. V. Hartland, L. V. Besteiro, P. Johns and A. O. Govorov, What's So Hot about Electrons in Metal Nanoparticles?, ACS Energy Lett., 2017, 2, 1641–1653 CrossRef CAS.
- A. Arbouet, C. Voisin, D. Christofilos, P. Langot, N. Del Fatti, F. Vallée, J. Lermé, G. Celep, E. Cottancin, M. Gaudry, M. Pellarin, M. Broyer, M. Maillard, M. P. Pileni and M. Treguer, Electron-Phonon Scattering in Metal Clusters, Phys. Rev. Lett., 2003, 90, 177401 CrossRef CAS PubMed.
- X. Li, S. Takano and T. Tsukuda, Ligand Effects on the Hydrogen Evolution Reaction Catalyzed by Au13 and Pt@Au12: Alkynyl vs. Thiolate, J. Phys. Chem. C, 2021, 125, 23226–23230 CrossRef CAS.
- M. Zhou, T. Higaki, G. Hu, M. Y. Sfeir, Y. Chen, D.-e. Jiang and R. Jin, Three-orders-of-magnitude variation of carrier lifetimes with crystal phase of gold nanoclusters, Science, 2019, 364, 279–282 CrossRef CAS PubMed.
- M. Hesari, H. Ma and Z. F. Ding, Monitoring single Au38 nanocluster reactions via electrochemiluminescence, Chem. Sci., 2021, 12, 14540–14545 RSC.
- Z. Wang, H. T. Sun, M. Kurmoo, Q.-Y. Liu, G.-L. Zhuang, Q.-Q. Zhao, X.-P. Wang, C.-H. Tung and D. Sun, Carboxylic acid stimulated silver shell isomerism in a triple core–shell Ag84 nanocluster, Chem. Sci., 2019, 10, 4862–4867 RSC.
- M. H. Naveen, R. Khan, M. A. Abbas, E. Cho, G. J. Lee, H. Kim, E. Sim and J. H. Bang, Modulation of the photoelectrochemical behavior of Au nanocluster-TiO2 electrode by doping, Chem. Sci., 2020, 11, 6248–6255 RSC.
- T. Higaki, Q. Li, M. Zhou, S. Zhao, Y. Li, S. Li and R. Jin, Toward the Tailoring Chemistry of Metal Nanoclusters for Enhancing Functionalities, Acc. Chem. Res., 2018, 51, 2764–2773 CrossRef CAS PubMed.
- K. K. Chakrahari, R. P. B. Silalahi, J.-H. Liao, S. Kahlal, Y.-C. Liu, J.-F. Lee, M.-H. Chiang, J.-Y. Saillard and C. W. Liu, Synthesis and structural characterization of inverse-coordination clusters from a two-electron superatomic copper nanocluster, Chem. Sci., 2018, 9, 6785–6795 RSC.
- C. M. Aikens, Electronic and Geometric Structure, Optical Properties, and Excited State Behavior in Atomically Precise Thiolate-Stabilized Noble Metal Nanoclusters, Acc. Chem. Res., 2018, 51, 3065–3073 CrossRef CAS PubMed.
- R. R. Nasaruddin, T. Chen, N. Yan and J. Xie, Roles of Thiolate Ligands in The Synthesis, Properties and Catalytic Application of Gold Nanoclusters, Coord. Chem. Rev., 2018, 368, 60–79 CrossRef CAS.
- X. Kang and M. Zhu, Tailoring the Photoluminescence of Atomically Precise Nanoclusters, Chem. Soc. Rev., 2019, 48, 2422–2457 RSC.
- C. Zeng, Y. Chen, K. Kirschbaum, K. J. Lambright and R. Jin, Emergence of Hierarchical Structural Complexities in Nanoparticles and Their Assembly, Science, 2016, 354, 1580–1584 CrossRef CAS PubMed.
- Y. Li, M. Zhou, Y. Song, T. Higaki, H. Wang and R. Jin, Double-Helical Assembly of Heterodimeric Nanoclusters into Supercrystals, Nature, 2021, 594, 380–384 CrossRef CAS PubMed.
- G. Yousefalizadeh, S. Ahmadi, N. J. Mosey and K. G. Stamplecoskie, Exciting clusters, what does off-resonance actually mean?, Nanoscale, 2021, 13, 242–252 RSC.
- M. Shabaninezhad, A. Abuhagr, N. A. Sakthivel, C. Kumara, A. Dass, K. Kwak, K. Pyo, D. Lee and G. Ramakrishna, Ultrafast Electron Dynamics in Thiolate-Protected Plasmonic Gold Clusters: Size and Ligand Effect, J. Phys. Chem. C, 2019, 123, 13344–13353 CrossRef CAS.
- P. Maioli, T. Stoll, H. E. Sauceda, I. Valencia, A. Demessence, F. Bertorelle, A. Crut, F. Vallée, I. L. Garzón, G. Cerullo and N. Del Fatti, Mechanical Vibrations of Atomically Defined Metal Clusters: From Nano- to Molecular-Size Oscillators, Nano Lett., 2018, 18, 6842–6849 CrossRef CAS PubMed.
- W. W. Xu, Y. Li, Y. Gao and X. C. Zeng, Unraveling a Generic Growth Pattern in Structure Evolution of Thiolate-Protected Gold Nanoclusters, Nanoscale, 2016, 8, 7396–7401 RSC.
- Y. Pei, P. Wang, Z. Ma and L. Xiong, Growth-Rule-Guided Structural Exploration of Thiolate-Protected Gold Nanoclusters, Acc. Chem. Res., 2019, 52, 23–33 CrossRef CAS PubMed.
- Z. Lei, J.-J. Li, X.-K. Wan, W.-H. Zhang and Q.-M. Wang, Isolation and Total Structure Determination of an All-Alkynyl-Protected Gold Nanocluster Au144, Angew. Chem., Int. Ed., 2018, 57, 8639–8643 CrossRef CAS PubMed.
- M. C. Heaven, V. E. Bondybey, J. M. Merritt and A. L. Kaledin, The Unique Bonding Characteristics of Beryllium and the Group IIA Metals, Chem. Phys. Lett., 2011, 506, 1–14 CrossRef CAS.
- D. A. Tomalia and S. N. Khanna, A Systematic Framework and Nanoperiodic Concept for Unifying Nanoscience: Hard/Soft Nanoelements, Superatoms, Meta-Atoms, New Emerging Properties, Periodic Property Patterns, and Predictive Mendeleev-like Nanoperiodic Tables, Chem. Rev., 2016, 116, 2705–2774 CrossRef CAS PubMed.
- R. Jin, Quantum sized, Thiolate-Protected Gold Nanoclusters, Nanoscale, 2010, 2, 343–362 RSC.
- R. Kubo, Electronic Properties of Small Particles, Annu. Rev. Mater. Sci., 1984, 14, 49–66 CrossRef CAS.
- U. Kreibig and M. Vollmer, Optical Properties of Metal Clusters, Springer-Verlag, New York, 1995 Search PubMed.
- B. von Issendorff and O. Cheshnovsky, Metal to Insulator Transitions in Clusters, Annu. Rev. Phys. Chem., 2005, 56, 549–580 CrossRef CAS PubMed.
- H. Haberland, B. von Issendorff, Y. Ji and T. Kolar, Transition to Plasmonlike Absorption in Small Hg Clusters, Phys. Rev. Lett., 1992, 69, 3212–3215 CrossRef CAS PubMed.
- J. Wang, G. Wang and J. Zhao, Nonmetal–Metal Transition in Znn (n = 2 − 20) Clusters, Phys. Rev. A, 2003, 68, 013201 CrossRef.
- Y. Jiang, K. Wu, J. Ma, B. Wu, E. G. Wang and P. Ebert, Quantum Size Effects in the Nonmetal to Metal Transition of Two-Dimensional Al Islands, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 76, 235434 CrossRef.
- A. Cirri, H. M. Hernández, C. Kmiotek and C. J. Johnson, Systematically Tuning the Electronic Structure of Gold Nanoclusters through Ligand Derivatization, Angew. Chem., Int. Ed., 2019, 58, 13818–13822 CrossRef CAS PubMed.
- M. Zhou and R. Jin, Optical Properties and Excited-State Dynamics ofAtomically Precise Gold Nanoclusters, Annu. Rev. Phys. Chem., 2021, 72, 121–142 CrossRef CAS PubMed.
- F. Hu, Z.-J. Guan, G. Yang, J.-Q. Wang, J.-J. Li, S.-F. Yuan, G.-J. Liang and Q.-M. Wang, Molecular Gold Nanocluster Au156 Showing Metallic Electron Dynamics, J. Am. Chem. Soc., 2021, 143, 17059–17067 CrossRef CAS PubMed.
- T. Zhao, P. J. Herbert, H. Zheng and K. L. Knappenberger Jr, State-Resolved Metal Nanoparticle Dynamics Viewed through the Combined Lenses of Ultrafast and Magneto-optical Spectroscopies, Acc. Chem. Res., 2018, 51, 1433–1442 CrossRef CAS PubMed.
- G. Yousefalizadeh and K. G. Stamplecoskie, A Single Model for the Excited-State Dynamics of Au18(SR)14 and Au25(SR)18 Clusters, J. Phys. Chem. A, 2018, 122, 7014–7022 CrossRef CAS PubMed.
- T. Higaki, C. Liu, D. J. Morris, G. He, T.-Y. Luo, M. Y. Sfeir, P. Zhang, N. L. Rosi and R. Jin, Au130−xAgx Nanoclusters with Non-Metallicity: A Drum of Silver-Rich Sites Enclosed in a Marks-Decahedral Cage of Gold-Rich Sites, Angew. Chem., Int. Ed., 2019, 131, 18974–18978 CrossRef.
- Y. Song, K. Lambright, M. Zhou, K. Kirschbaum, J. Xiang, A. Xia, M. Zhu and R. Jin, Large-Scale Synthesis, Crystal Structure, and Optical Properties of the Ag146Br2(SR)80 Nanocluster, ACS Nano, 2018, 12, 9318–9325 CrossRef CAS PubMed.
- M. Zhou, C. Zeng, Y. Chen, S. Zhao, M. Y. Sfeir, M. Zhu and R. Jin, Evolution from the Plasmon to Exciton State in Ligand-Protected Atomically Precise Gold Nanoparticles, Nat. Commun., 2016, 7, 13240 CrossRef CAS PubMed.
- T. Higaki, M. Zhou, K. J. Lambright, K. Kirschbaum, M. Y. Sfeir and R. Jin, Sharp Transition from Nonmetallic Au246 to Metallic Au279 with Nascent Surface Plasmon Resonance, J. Am. Chem. Soc., 2018, 140, 5691–5695 CrossRef CAS PubMed.
- N. A. Sakthivel, M. Stener, L. Sementa, A. Fortunelli, G. Ramakrishna and A. Dass, Au279(SR)84: The Smallest Gold Thiolate Nanocrystal That Is Metallic and the Birth of Plasmon, J. Phys. Chem. Lett., 2018, 9, 1295–1300 CrossRef CAS PubMed.
- H. Qian, Y. Zhu and R. Jin, Atomically Precise Gold Nanocrystal Molecules with Surface Plasmon Resonance, Proc. Natl. Acad. Sci. U. S. A., 2012, 109, 696–700 CrossRef CAS PubMed.
- H. Qian and R. Jin, Controlling Nanoparticles with Atomic Precision: The Case of Au144(SCH2CH2Ph)60, Nano Lett., 2009, 9, 4083–4087 CrossRef CAS PubMed.
- C. Zeng, Y. Chen, A. Das and R. Jin, Transformation Chemistry of Gold Nanoclusters: From One Stable Size to Another, J. Phys. Chem. Lett., 2015, 6, 2976–2986 CrossRef CAS PubMed.
- N. Yan, N. Xia, L. Liao, M. Zhu, F. Jin, R. Jin and Z. Wu, Unraveling the Long-Pursued Au144 Structure by X-Ray Crystallography, Sci. Adv., 2018, 4, eaat7259 CrossRef CAS PubMed.
- S. Chen, T. Higaki, H. Ma, M. Zhu, R. Jin and G. Wang, Inhomogeneous Quantized Single-Electron Charging and Electrochemical-Optical Insights on Transition-Sized Atomically Precise Gold Nanoclusters, ACS Nano, 2020, 14, 16781–16790 CrossRef CAS PubMed.
- T. Higaki, M. Zhou, G. He, S. D. House, M. Y. Sfeir, J. C. Yang and R. Jin, Anomalous Phonon Relaxation in Au333(SR)79 Nanoparticles with Nascent Plasmons, Proc. Natl. Acad. Sci. U. S. A., 2019, 116, 13215–13220 CrossRef CAS PubMed.
- M. Zhou, C. Zeng, Y. Song, J. W. Padelford, G. Wang, M. Y. Sfeir, T. Higaki and R. Jin, On the Non-Metallicity of 2.2 nm Au246(SR)80 Nanoclusters, Angew. Chem., Int. Ed., 2017, 129, 16475–16479 CrossRef.
- Z. Liu, Y. Li, W. Shin and R. Jin, Observation of Core Phonon in Electron–Phonon Coupling in Au25 Nanoclusters, J. Phys. Chem. Lett., 2021, 12, 1690–1695 CrossRef CAS PubMed.
- M. S. Devadas, S. Bairu, H. Qian, E. Sinn, R. Jin and G. Ramakrishna, Temperature-Dependent Optical Absorption Properties of Monolayer-Protected Au25 and Au38 Clusters, J. Phys. Chem. Lett., 2011, 2, 2752–2758 CrossRef CAS.
- U. Kreibig, Anomalous Frequency and Temperature Dependence of the Optical Absorption of Small Gold Particles, J. Phys., Colloq., 1977, 38, C2-97–C2-103 CrossRef.
- H.-C. Weissker, H. B. Escobar, V. D. Thanthirige, K. Kwak, D. Lee, G. Ramakrishna, R. L. Whetten and X. López-Lozano, Information on Quantum States Pervades the Visible Spectrum of the Ubiquitous Au144(SR)60 Gold Nanocluster, Nat. Commun., 2014, 5, 3785 CrossRef PubMed.
- Y. Negishi, T. Nakazaki, S. Malola, S. Takano, Y. Niihori, W. Kurashige, S. Yamazoe, T. Tsukuda and H. Häkkinen, A Critical Size for Emergence of Nonbulk Electronic and Geometric Structures in Dodecanethiolate-Protected Au Clusters, J. Am. Chem. Soc., 2015, 137, 1206–1212 CrossRef CAS PubMed.
- R. Klingeler, G. Kann, I. Wirth, S. Eisebitt, P. S. Bechthold, M. Neeb and W. Eberhardt, La@C60: A Metallic Endohedral Fullerene, J. Chem. Phys., 2001, 115, 7215–7218 CrossRef CAS.
- V. D. Borman, P. V. Borisyuk, V. V. Lebid'ko, M. A. Pushkin, V. N. Tronin, V. I. Troyan, D. A. Antonov and D. O. Filatov, A Study of Many-Body Phenomena in Metal Nanoclusters (Au, Cu) Close to Their Transition to the Nonmetallic State, J. Exp. Theor. Phys., 2006, 102, 303–313 CrossRef CAS.
- S. Malola, L. Lehtovaara, J. Enkovaara and H. Häkkinen, Birth of the Localized Surface Plasmon Resonance in Monolayer-Protected Gold Nanoclusters, ACS Nano, 2013, 7, 10263–10270 CrossRef CAS PubMed.
- K. Iida, M. Noda and K. Nobusada, Interface Electronic Properties Between a Gold Core and Thiolate Ligands: Effects on an Optical Absorption Spectrum in Au133(SPh-tBu)52, J. Phys. Chem. C, 2016, 120, 2753–2759 CrossRef CAS.
- L. Yang, P. Wang, Z. Yang and Y. Pei, Effect of Thiolate-Ligand Passivation on The Electronic Structure and Optical Absorption Properties of Ultrathin One and Two-Dimensional Gold Nanocrystals, Nanoscale, 2020, 12, 5554–5566 RSC.
- L. Xiong and Y. Pei, Evolution of Electronic Structure of Cuboid Thiolate-Monolayer-Protected Gold Nanocrystals, J. Phys. Chem. C, 2021, 125, 20670–20675 CrossRef CAS.
Footnotes |
| † Electronic supplementary information (ESI) available: Synthesis and characterization, including mass spectrometry (MS) and cryogenic spectroscopy. See DOI: 10.1039/d1sc06819a |
| ‡ These authors contributed equally to this work. |
| This journal is © The Royal Society of Chemistry 2022 |