Rational design of a promising oxychalcogenide infrared nonlinear optical crystal

Abstract

Oxychalcogenides with the performance-advantages of both chalcogenides and oxides are emerging materials class for infrared (IR) nonlinear optical (NLO) crystals that can expand the wavelength of solid-state lasers to IR regions and are of importance in industrial and civil applications. But rationally designing a high-performance oxychalcogenide NLO crystal remains a great challenge. Herein, we chose the melilite-type Sr₂ZnSi₂O₇ as the structure template. Through part isovalent substitution of S²⁻ for O²⁻ anions, the first hetero-anionic thiostannate Sr₂ZnSn₂OS₆ with wide IR transmission has been synthesized. More importantly, compared to the maternal oxide, Sr₂ZnSi₂O₇, the second harmonic generation (SHG) response of Sr₂ZnSn₂OS₆ is enhanced by two orders of magnitude. In addition, Sr₂ZnSn₂OS₆ can exhibit a large band-gap and high laser damage threshold. These advantages make Sr₂ZnSn₂OS₆ a promising IR NLO crystal. Our research will provide insights into the rational design of new IR NLO crystals.

---

Introduction

Mid-infrared (MIR) sources covering 3 to 5 μm and 8 to 12 μm are of great interest in industrial and civil applications such as molecular spectroscopy, remote sensing, free-space communication, and environmental monitoring.^1–5 However, it is difficult for solid-state lasers to directly radiate MIR coherent light. Nevertheless, relying on frequency conversion techniques of nonlinear optical (NLO) crystals, such as optical parametric oscillators (OPOs) and optical parametric amplifiers (OPAs), is an effective way for solid-state lasers to generate MIR radiation. In these processes, the performances of NLO crystals determine the conversion efficiency of IR coherent light. So, they play a strategically important role in the development of IR lasers. In the past three decades, although a series of high-performance NLO crystals have been discovered and widely commercialized in the ultraviolet and visible regions, including β-BaB₂O₄ (BBO),⁶ LiB₃O₅ (LBO),⁷ CsLiB₆O₁₀ (CLBO),⁸ KH₂PO₄ (KDP)⁹ and KTiOPO₄ (KTP),¹⁰ NLO crystals available in the infrared (IR) region are still seldom, and they are mainly some chalcopyrite-type chalcogenides, such as AgGaQ₂ (Q = S, Se)^11,12 and ZnGeP₂.¹³ Although these crystals feature very large second harmonic generation (SHG) coefficients, the low laser damage thresholds (LDTs) of AgGaQ₂ (Q = S, Se) and harmful two-photon absorption (TPA) of ZnGeP₂ limit their wide applications,¹⁴ particularly under high-power conditions. So, exploring new IR NLO crystals with high LDTs and large band gaps is still a current research interest.^15–18

For the design of new IR NLO crystals, metal chalcogenides, oxides and halides are the three most important materials. Regarding chalcogenides, they generally exhibit large SHG responses and wide IR transparency,^19,20 while low LDTs and undesired TPA caused by their small band-gaps as well as the difficulty in crystal growth are the main problems restricting their wide applications, just as observed in typical AgGaQ₂ (Q = S, Se) and ZnGeP₂.²¹ Compared to chalcogenides, metal oxides can exhibit larger band-gaps and thus higher LDTs as well as easier crystal growth.²² But the poor IR transparency and relatively weak SHG responses make their application limited within 5 μm.²³ Therefore, it is clear that chalcogenides can exhibit larger SHG response and better IR transmission, while metal oxides have advantages in band-gaps and LDTs. Therefore, combining two types of anions may be an expectant strategy for designing high-performance IR NLO crystals with balanced properties. Up to now, although some oxychalcogenide IR NLO crystals, e.g. SrZn₂OS₂ (E_g = 3.86 eV, 0.06× AgGaS₂),²⁴ Sr₅Ga₈O₃S₁₄ (E_g = 3.90 eV, 0.8× AgGaS₂),²⁵ SrGeOSe₂ (E_g = 3.16 eV, 1.3× AgGaS₂),²⁶ BaGeOSe₂ (E_g = 3.20 eV, 1.1× AgGaS₂),²⁷ and Sr₆Cd₂Sb₆O₇S₁₀ (E_g = 1.89 eV, 4.0× AgGaS₂),²⁸ have been reported, these compounds are still less investigated than their maternal oxides or chalcogenides. In particular, how to optimize the structure of oxychalcogenides to use the advantages of oxides and chalcogenides to the maximum through a rational structure design is still indistinct.

In this research, we choose the melilite-type Sr₂ZnSi₂O₇ as a structural template to design a new oxychalcogenide through rational chemical substitution. Sr₂ZnSi₂O₇²⁹ crystallizes in the noncentrosymmetric (NCS) space group P4̄2₁m. Its structure features a pentagon-like layer, which endows its structure with better flexibility and designability. But remarkably, the SHG response of Sr₂ZnSi₂O₇ is only 35.0× α-SiO₂,³⁰ almost two orders of magnitude smaller than that of AgGaS₂. And owing to the absorption of the metal–oxygen bonds, it also has poor IR transmission. In our study, we used Sn atoms to substitute Si in melilite-type Sr₂ZnSi₂O₇, first, because Sn atoms are heavier and more polarizable than Si atoms, which is helpful for the material to exhibit a wider IR transmission and larger SHG response.³¹ Furthermore, with the help of the unique pentagonal layer configurations, we expect to use heavier and more polarizable S atoms to selectively substitute the terminal O atoms in the pentagonal layers as well as the bridging O atoms in relatively light Zn–O–Sn bonds, whereas retaining the O atoms in Sn–O–Sn bonds, which have the heaviest equivalent mass for metal–oxygen bonds in the structure and have the smallest effect on IR transmission of the materials.^32,33 Meanwhile, the hetero-anionic [SnOS₃] tetrahedra may exhibit large polarization, which will favor the material to generate a large SHG response.²⁵ By doing these, we have successfully synthesized the first hetero-anionic thiostannate, Sr₂ZnSn₂OS₆. It exhibits not only a wide IR transmission, but also a large SHG response, ∼0.7× AgGaS₂, which is two orders of magnitude larger than that of maternal Sr₂ZnSi₂O₇. These results indicate that Sr₂ZnSn₂OS₆ is a promising IR NLO crystal. Herein, we will report its synthesis, structure, and NLO properties.

Results and discussion

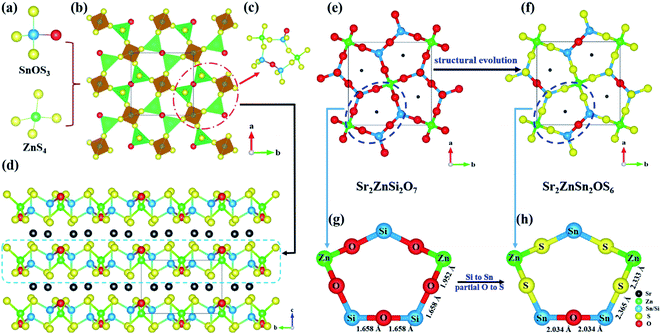
The polycrystalline sample of Sr₂ZnSn₂OS₆ was obtained by a solid-state reaction in a sealed silica tube (for more details see the ESI†). The purity of the sample was confirmed by powder X-ray diffraction (PXRD). In order to investigate the thermal behavior of Sr₂ZnSn₂OS₆, we carried out a series solid state reactions with different calcination temperatures and performed their powder XRD from 500 to 800 °C (Fig. S1†). It is clear that Sr₂ZnSn₂OS₆ is thermally stable until 750 °C. After 750 °C, Sr₂ZnSn₂OS₆ decomposes into SrS, SnS and ZnS (Fig. S1†). The millimeter-size crystal of Sr₂ZnSn₂OS₆ was grown by the solution method with SrS as the flux (Fig. S2†), and the specific crystal photograph is shown in Fig. S3.† The structure was determined by single crystal XRD and the crystallographic data are given in Table S1.†

Sr₂ZnSn₂OS₆ crystallizes in the NCS tetragonal space group P4̄2₁m. In the asymmetric unit of Sr₂ZnSn₂OS₆, there are one unique Sr, one Zn, one Sn, one O and two S atom(s). Among them, the Sn atom is coordinated by one O atom and three S atoms to form the SnOS₃ tetrahedron with the distance of d(Sn–O) = 2.029(4) Å and Sn–S distances in the range of 2.321(2)–2.366(16) Å, respectively. The Zn atom is coordinated with four S atoms forming the typical ZnS₄ tetrahedron with identical Zn–S distance, d(Zn–S) = 2.333(16) Å (Fig. 1a). In the structures, three SnOS₃ tetrahedra and two ZnS₄ tetrahedra are connected to form an interesting pentagon ring (Fig. 1c). In the pentagon ring, the SnOS₃ tetrahedron connects with a ZnS₄ tetrahedron by sharing the S atoms, and the two SnOS₃ tetrahedra are connected by sharing the O atoms. These pentagons further form two-dimensional (2D) [ZnSn₂OS₆]_∞ layers (Fig. 1b), which are separated by Sr²⁺ cations to form the structure of Sr₂ZnSn₂OS₆ (Fig. 1d). Regarding the Sr²⁺ cations, they are located in an eight-coordinated SrOS₇ bicapped trigonal prism with the d(Sr–O) = 2.788(5) Å and d(Sr–S) = 3.113(2)–3.150(17) Å, respectively (Table S2†). These bond distances are comparable to those of other reported compounds.²¹ Bond valence calculations show that the bond valence sums (BVSs) for each atom are 2.12, 2.00, 4.04, and 1.73 for Sr²⁺, Zn²⁺, Sn⁴⁺, and O²⁻, respectively. The BVSs of S²⁻ anions are in the range of 2.08–2.14 (Table S3†). All the BVSs are consistent with the expected valences.

Fig. 1 (a) [SnOS₃] and [ZnS₄] tetrahedra. (b) The 2-dimensional [ZnSn₂OS₆]⁴⁻ layers composed of pentagons. (c) The pentagonal ring composed of [SnOS₃] and [ZnS₄] tetrahedra. (d) The crystal structure of Sr₂ZnSn₂OS₆. (e and f) Structural evolution from Sr₂ZnSi₂O₇ to Sr₂ZnSn₂OS₆. (g) The pentagon consisting of Zn–O–Si and Si–O–Si bonds. (h) The pentagon consisting of Zn–S–Sn and Sn–O–Sn bonds.

Structurally, Sr₂ZnSn₂OS₆ can be seen as a homologue of Sr₂ZnSi₂O₇ (Fig. 1e–h). Compared with Sr₂ZnSi₂O₇, Sr₂ZnSn₂OS₆ exhibits the following structural features: (i) the Si⁴⁺ cations in Sr₂ZnSi₂O₇ were substituted by the heavier Sn⁴⁺ cations, which are the heaviest cations but have similar coordination to Si⁴⁺ in group IV; (ii) all the terminal O atoms in the Zn–O or Sn–O bonds, and all the bridging O atoms in the Zn–O–Sn bonds are substituted by the heavier S atoms, while the O atoms in the Sn–O–Sn bonds are retained (Fig. 1e and f). The above substitution will be favorable for Sr₂ZnSn₂OS₆ to exhibit wide IR transmission. Generally, the IR absorption of materials mainly originates from the vibration of the groups. Thus, in Sr₂ZnSn₂OS₆, the large equivalent mass of SnOS₃ and ZnS₄ groups will be favorable for widening the IR transmission of materials. In Sr₂ZnSi₂O₇, the Si–O bonds have the lightest equivalent mass, so they have the greatest effect on IR transmission. Using the heavier Sn⁴⁺ cations to substitute Si⁴⁺ can reduce the effect. As shown in Fig. S4,† the peaks at 1123, 963, 894, and 832 cm⁻¹ correspond to the asymmetric and symmetric stretching vibrations of Si–O bonds. The peak at 587 cm⁻¹ can be assigned to the bending vibrations of Si–O bonds in Sr₂ZnSi₂O₇. Furthermore, a micron sized crystal was used to measure the IR transmission spectrum of Sr₂ZnSn₂OS₆. Similar measurements have also been found in the previous research.^34–37 It is clear that there is no obvious absorption in the range from 1800 to 800 cm⁻¹, corresponding to 5.5 to 12.5 μm (Fig. 2a). The absorption peak at 800 cm⁻¹ can be assigned to the stretching vibration of the Sn–O–Sn bond.³⁸ In addition, except for the Si⁴⁺ cations, the terminal O atoms and bridging O atoms in those bonds with low equivalent mass (e.g. the O in the Zn–O–Sn bonds) are also more prone to vibrate than the bridging O atoms in the heavy Sn–O–Sn bonds. Therefore, substituting them with the relatively heavy S atoms would also be helpful to further widen the IR transmission region of the material. The rational substitution from Sr₂ZnSi₂O₇ to Sr₂ZnSn₂OS₆ can be attributed to the minimum distortion of pentagonal rings. In Sr₂ZnSi₂O₇, the pentagonal ring is composed of four long Si–O–Zn bonds and one short Si–O–Si bond (Fig. 1g). From Sr₂ZnSi₂O₇ to Sr₂ZnSn₂OS₆, substituting O atoms with S atoms in Sn–O–Zn bonds and retaining O atoms in the Sn–O–Sn bonds can best retain a similar pentagonal-ring-configuration, i.e. also including four long Sn–S–Zn bonds and one short Sn–O–Sn bond (Fig. 1h).

Fig. 2 (a) IR transmission spectrum from single crystals, (b) Raman spectrum, and (c) UV-vis-NIR diffuse reflectance spectrum of Sr₂ZnSn₂OS₆.

Furthermore, Raman spectroscopy of Sr₂ZnSn₂OS₆ is shown in Fig. 2b. In the Raman spectra, the absorption peaks at 400, 368, and 346 cm⁻¹ can be attributed to the characteristic absorption of Sn–S modes, the peaks around 272 cm⁻¹ should be from the Zn–S bonds and the absorption peaks below 200 cm⁻¹ (including 141 and 82 cm⁻¹) are mainly attributed to Sr–S vibrations.^39–41

The UV-vis-NIR diffuse reflectance spectrum in the range of 200–2500 nm was also measured by using a Shimadzu SolidSpec-3700DUV spectrophotometer (Fig. 2c). It was transformed into an F(R) versus (hν) curve based on the Kubelka–Munk function.⁴² Extrapolating the linear part of the rising curve to zero, an experimental band gap of 3.52 eV can be obtained. The band gap of Sr₂ZnSn₂OS₆ is much larger than those in commercial AgGaS₂ (2.56 eV)¹¹ and AgGaSe₂ (1.83 eV).¹² Since the large bandgap will reduce the proportion of two photon or multiphoton absorption, the large band-gap is generally helpful for producing a large LDT.⁴³ So, the LDT of Sr₂ZnSn₂OS₆ was also measured by using a Q-switched pulse laser (1064 nm, 10 ns, 10 Hz) with a powder AgGaS₂ sample as the reference. It indicates that Sr₂ZnSn₂OS₆ has a good laser damage resistance, and the measured LDT (131 MW cm⁻²) is about 10 times that of AgGaS₂ (12 MW cm⁻²).⁴⁴

Sr₂ZnSn₂OS₆ crystallizes in the NCS space group P4̄2₁m. Its SHG response was measured based on the Kurtz–Perry method by using a laser with a wavelength of 2.09 μm and with powder AgGaS₂ as the reference.⁴⁵ The sample of AgGaS₂ was purchased from Sichuan Ninth Research Institute (Fig. S5†) and the powder AgGaS₂ sample was obtained by grinding the AgGaS₂ crystal. As shown in Fig. 3a, the SHG intensities of Sr₂ZnSn₂OS₆ increase with its particle size. So, Sr₂ZnSn₂OS₆ can achieve type-I phase-matching. The SHG response with the particle size in the range of 150–180 μm is approximately 0.7× AgGaS₂ (Fig. 3b), indicating that Sr₂ZnSn₂OS₆ exhibits moderate SHG response. Apart from the large SHG response, a suitable birefringence is also necessary for the application of NLO crystals. Hence, we measured the birefringence of Sr₂ZnSn₂OS₆ by using a cross-polarizing microscope. The orientation of such a utilized crystal was determined as [100] by using the “Index Crystal Faces” program in Bruker SMART APEX III. The observed color of Sr₂ZnSn₂OS₆ in cross-polarized light is second-order yellow. The thickness of the crystal used for the measurement is 8 μm (Fig. S6†). According to the Michel-Levy chart, the retardation (R value) is 920 nm. Thus, the birefringence of Sr₂ZnSn₂OS₆ in the visible region is 0.12, which is large enough for ensuring the phase matching of Sr₂ZnSn₂OS₆.

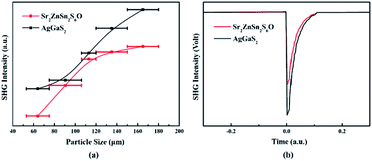Fig. 3 (a) SHG intensity versus particle size for Sr₂ZnSn₂OS₆ and AgGaS₂. (b) Comparison of SHG intensities for Sr₂ZnSn₂OS₆ and AgGaS₂ at the particle sizes of 150–180 μm.

The above measurements indicate that Sr₂ZnSn₂OS₆ not only exhibits well-balanced NLO properties, including a wide transmission region, large band-gap, and high LDT as well as moderate birefringence and SHG response (Fig. 4a), but also presents the first Sn-containing oxychalcogenide IR NLO with [SnOS₃] hetero-anionic tetrahedra (Fig. 4b).^46–52 In order to better understand the origin of these excellent NLO properties, especially the moderate SHG response and birefringence, we further calculated the electronic structure of Sr₂ZnSn₂OS₆ through the first-principles calculation.

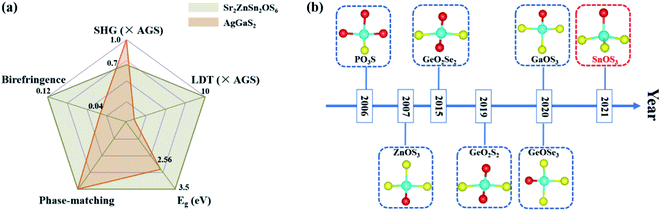Fig. 4 (a) The excellent overall NLO performances of Sr₂ZnSn₂OS₆. (b) Timeline of the hetero-anionic NLO-active unit discovered in oxychalcogenides.

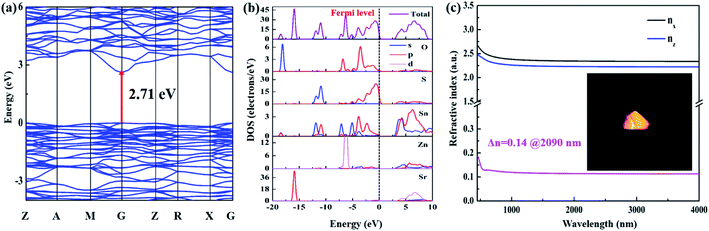
As shown in Fig. 5a, Sr₂ZnSn₂OS₆ is a direct-gap compound and the calculated band gap is 2.71 eV, which is smaller than the experimental value (3.52 eV). The smaller calculated band gap can be attributed to the limitation of the density functional theory (DFT) method.⁵³ The density of states (DOS) and partial densities of states (PDOS) of Sr₂ZnSn₂OS₆ are shown in Fig. 5b. The top of the valence band (VB) is predominately composed of O 2p and S 3p states, mixed with small amounts of Sn 5p, while the bottom of the conductive band (CB) mainly originates from O 2p, S 3p, and Sn 5s,5p states. As we know, the optical properties of a material are mainly affected by the electronic transitions between the energy levels close to the forbidden band. So, the SHG response and birefringence will be mainly determined by [SnOS₃] and [ZnS₄] tetrahedra (Fig. S7†). Particularly for [SnOS₃] tetrahedra, owing to the part substitution of O and S, the dipole moment of the SnOS₃ tetrahedron is obviously larger than that of ZnS₄ (Table S5†). It indicates that SnOS₃ tetrahedra have the main contribution to the SHG response and birefringence of Sr₂ZnSn₂OS₆. Meanwhile, the dipole moments of ZnO₄ and SiO₄ in Sr₂ZnSi₂O₇ are also calculated (Fig. S8†). By comparing the dipole moments of SiO₄ (6.29 D) and SnOS₃ (6.77 D), it can be found that the mixed-anion SnOS₃ units can exhibit larger polarity in the structure. These results indicate that the rational substitutions of S for O and Sn for Si have contributed the enhancement of the NLO response in Sr₂ZnSn₂OS₆.

Fig. 5 (a) Calculated band structures of Sr₂ZnSn₂OS₆. (b) PDOS and TDOS plot of Sr₂ZnSn₂OS₆. (c) The calculated refractive dispersion curve of Sr₂ZnSn₂OS₆ (the inset image shows the crystal for the birefringence determination).

Based on the calculated electronic structure, the NLO coefficient and birefringence of Sr₂ZnSn₂OS₆ can also be calculated. Sr₂ZnSn₂OS₆ belongs to the point group of 4̄2m. It only has one non-zero independent SHG tensor, d₁₄ under the restriction of Kleinman symmetry. The calculation shows d₁₄ = 4.48 pm V⁻¹, which is comparable with the experimental results. Moreover, the refractive indices and birefringence (Δn) were also calculated. The calculated birefringence is shown in Fig. 5c, and the birefringence Δn = 0.14 at 2090 nm. These calculated results are also essentially consistent with the experimental ones.

Conclusions

In summary, based on the rational partial substitution of S²⁻ for O²⁻ anions in Sr₂ZnSi₂O₇, the first Sn-containing oxychalcogenide IR NLO crystal Sr₂ZnSn₂OS₆ has been successfully designed and synthesized. It can exhibit excellent NLO properties, including a wide IR transmission region, large band gap (3.52 eV), and high LDT (10× AGS) as well as a suitable SHG response (0.7× AGS) and birefringence (0.14 @ 2090 nm), which are comparable with other excellent oxychalcogenides (Table 1). These results show that Sr₂ZnSn₂OS₆ would be a promising IR NLO crystal. The excellent NLO properties of Sr₂ZnSn₂OS₆ can be attributed to the rational substitution of S²⁻ for O²⁻, i.e. the terminal O atoms and the bridging O atoms in the light Zn–O–Sn bonds, which would lead to an insufficient IR transparency window, have been substituted by the heavier S, while the bridging O atoms in the heavy Sn–O–Sn bonds, which have a weak effect on the IR transparent region, are retained for constructing the distorted SnOS₃ groups to produce a moderate SHG response. These findings will be helpful to provide some new insights for the design of oxychalcogenide IR NLO crystals.

Table 1 Comparison of properties between Sr₂ZnSn₂S₆O and other NLO oxychalcogenides^a

Crystals	Space group	IR absorption edge (μm)	E g (eV)	SHG (×AGS)	PM/NPM
a PM = phase matchability, NPM = non-phase matchability.
Sr2ZnSn2OS6	P4̄21m	12.5	3.52	0.7	PM
Sr6Cd2Sb6O7S10	Cm	15.5	1.89	4	PM
SrZn2OS2	Pmn21	—	3.86	0.06	PM
Sr3Ge2O4Se3	R3m	—	2.96	0.8	PM
BaGeOSe2	P212121	13.3	3.2	1.1	PM
SrGeOSe2	P212121	12.6	3.16	1.3	PM
BaGeOS2	P212121	—	4.1	0.5	NPM
SrGeOS2	P212121	—	3.9	0.4	NPM
Sr5Ga8O3S14	P21212	13.4	3.9	0.8	NPM

---

Data availability

Supporting data for this article is presented in the ESI.†

Author contributions

Conceptualization and supervision: H. W. Yu and H. P. Wu; synthesis and characterization (single-crystal XRD, powder XRD, IR, UV-vis-NIR and SHG measurements): Y. S. Cheng; all authors proof-read, provided comments, and approved the final version of this manuscript.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work is supported by the National Natural Science Foundation of China (Grant No. 22071179, 52172006, 51972230, 51890864, 61835014, 51890865) and Natural Science Foundation of Tianjin (Grant No. 21JCJQJC00090 and 20JCJQJC00060).

Notes and references

1. P. Becker, Adv. Mater., 1998, 10, 979–992.
2. R. E. Sykora, K. M. Ok, P. S. Halasyamani and T. E. Albrecht-Schmitt, J. Am. Chem. Soc., 2002, 124, 1951–1957.
3. H. Wu, S. Pan, K. R. Poeppelmeier, H. Li, D. Jia, Z. Chen, X. Fan, Y. Yang, J. M. Rondinelli and H. Luo, J. Am. Chem. Soc., 2011, 133, 7786–7790.
4. X. Wang, Y. Wang, B. Zhang, F. Zhang, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2017, 56, 14119–14123.
5. B. Zhang, G. Shi, Z. Yang, F. Zhang and S. Pan, Angew. Chem., Int. Ed., 2017, 56, 3916–3919.
6. C. T. Chen, B. C. Wu, A. D. Jiang and G. M. You, Sci. China, Ser. B: Chem., 1985, 28, 235–243.
7. C. T. Chen, Y. C. Wu, A. D. Jiang, B. C. Wu, G. M. You, R. K. Li and S. J. Lin, J. Opt. Soc. Am. B, 1989, 6, 616–621.
8. G. Ryu, C. S. Yoon, T. P. J. Han and H. G. Gallagher, J. Cryst. Growth, 1998, 191, 492–500.
9. H. Siegfried, Z. Kristallogr.–Cryst. Mater., 1964, 120, 401–414.
10. J. D. Bierlein and H. Vanherzeele, J. Opt. Soc. Am. B, 1989, 6, 622–633.
11. A. O. Okorogu, S. B. Mirov, W. Lee, D. I. Crouthamel, N. Jenkins, A. Y. Dergachev, K. L. Vodopyanov and V. V. Badikov, Opt. Commun., 1998, 155, 307–312.
12. G. Boyd, H. Kasper, J. McFee and F. Storz, IEEE J. Quantum Electron., 1972, 8, 900–908.
13. G. D. Boyd, E. Buehler and F. G. Storz, Appl. Phys. Lett., 1971, 18, 301–304.
14. K. Wu, B. Zhang, Z. Yang and S. Pan, J. Am. Chem. Soc., 2017, 139, 14885–14888.
15. H. Chen, Y. Y. Li, B. X. Li, P. F. Liu, H. Lin, Q. L. Zhu and X. T. Wu, Chem. Mater., 2020, 32, 8012–8019.
16. B. B. Zhang, G. Q. Shi, Z. H. Yang, F. F. Zhang and S. L. Pan, Angew. Chem., Int. Ed., 2017, 56, 3916–3919.
17. J. Lu, J. N. Yue, L. Xiong, W. K. Zhang, L. Chen and L. M. Wu, J. Am. Chem. Soc., 2019, 141, 8093–8097.
18. G. H. Zou, N. Ye, L. Huang and X. S. Lin, J. Am. Chem. Soc., 2011, 133, 20001–20007.
19. Y. Wang, B. Zhang, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2018, 57, 2150–2154.
20. H. Yu, H. Wu, S. Pan, Z. Yang, X. Su and F. Zhang, J. Mater. Chem., 2012, 22, 9665–9670.
21. L. Kang, M. Zhou, J. Yao, Z. Lin, Y. Wu and C. Chen, J. Am. Chem. Soc., 2015, 137, 13049–13059.
22. H. Yu, W. Zhang and P. S. Halasyamani, Cryst. Growth Des., 2016, 16, 1081–1087.
23. G. Han, Y. Wang, X. Su, Z. Yang and S. Pan, Sci. Rep., 2017, 7, 1901.
24. Y. Tsujimoto, C. A. Juillerat, W. Zhang, K. Fujii, M. Yashima, P. S. Halasyamani and H.-C. zur Loye, Chem. Mater., 2018, 30, 6486–6493.
25. R. Wang, Y. Guo, X. Zhang, Y. Xiao, J. Yao and F. Huang, Inorg. Chem., 2020, 59, 9944–9950.
26. M. Y. Ran, Z. Ma, H. Chen, B. Li, X. T. Wu, H. Lin and Q. L. Zhu, Chem. Mater., 2020, 32, 5890–5896.
27. B. W. Liu, X. M. Jiang, G. E. Wang, H. Y. Zeng, M. J. Zhang, S. F. Li, W. H. Guo and G. C. Guo, Chem. Mater., 2015, 27, 8189–8192.
28. R. Wang, F. Liang, F. Wang, Y. Guo, X. Zhang, Y. Xiao, K. Bu, Z. Lin, J. Yao, T. Zhai and F. Huang, Angew. Chem., Int. Ed., 2019, 58, 8078–8081.
29. M. Mutailipu, Z. Li, M. Zhang, D. Hou, Z. Yang, B. Zhang, H. Wu and S. Pan, Phys. Chem. Chem. Phys., 2016, 18, 32931–32936.
30. H. Yu, W. Zhang, J. Young, J. M. Rondinelli and P. S. Halasyamani, J. Am. Chem. Soc., 2015, 138, 88–91.
31. K. Chen, C. Lin, S. Zhang, G. Peng, Y. Chen, D. Zhao, H. Fan, S. Yang, X. Wen, M. Luo, Z. Lin and N. Ye, Chem. Mater., 2021, 33, 6012–6017.
32. X. Chen, Q. Jing and K. M. Ok, Angew. Chem., Int. Ed., 2020, 59, 20323–20327.
33. X. Luo, Z. Li, F. Liang, Y. Guo, Y. Wu, Z. Lin and J. Yao, Inorg. Chem., 2019, 58, 7118–7125.
34. W. Xing, F. Liang, C. Tang, E. Uykur, Z. Lin, J. Yao, W. Yin and B. Kang, ACS Appl. Mater. Interfaces, 2021, 13, 37331–37338.
35. J. Chen, H. Chen, F. Xu, L. Cao, X. Jiang, S. Yang, Y. Sun, X. Zhao, C. Lin and N. Ye, J. Am. Chem. Soc., 2021, 143, 10309–10316.
36. Y. Hu, C. Wu, X. Jiang, Z. Wang, Z. Huang, Z. Lin, X. Long, M. G. Humphrey and C. Zhang, J. Am. Chem. Soc., 2021, 143, 12455–12459.
37. W. Xing, C. Tang, N. Wang, C. Li, E. Uykur, J. Wu, Z. Lin, J. Yao, W. Yin and B. Kang, Adv. Opt. Mater., 2021, 9, 2100563.
38. P. Pascuta, L. Pop, S. Rada, M. Bosca and E. Culea, Vib. Spectrosc., 2008, 48, 281–284.
39. J. He, Y. Guo, W. Huang, X. Zhang, J. Yao, T. Zhai and F. Huang, Inorg. Chem., 2018, 57, 9918–9924.
40. K. Wu, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2016, 55, 6713–6715.
41. X. Luo, Z. Li, F. Liang, Y. Guo, Y. Wu, Z. Lin and J. Yao, Inorg. Chem., 2019, 58, 7118–7125.
42. E. L. Simmons, Appl. Opt., 1975, 14, 1380–1386.
43. T. W. Walker, A. H. Guenther and P. Nielsen, IEEE J. Quantum Electron., 1981, 17, 2053–2065.
44. K. Wu, Z. Yang and S. Pan, Chem. Mater., 2016, 28, 2795–2801.
45. S. K. Kurtz and T. T. Perry, J. Appl. Phys., 1968, 39, 3798–3813.
46. X. Luo, F. Liang, M. Zhou, Y. Guo, Z. Li, Z. Lin, J. Yao and Y. Wu, Inorg. Chem., 2018, 57, 9446–9452.
47. P. A. Maggard, T. S. Nault, C. L. Stern and K. R. Poeppelmeier, Solid State Chem., 2003, 175, 27–33.
48. J. Zhang, Z. Zhang, W. Zhang, Q. Zheng, Y. Sun, C. Zhang and X. Tao, Chem. Mater., 2011, 23, 3752–3761.
49. W. Xing, P. Fang, N. Wang, Z. Li, Z. Lin, J. Yao, W. Yin and B. Kang, Inorg. Chem., 2020, 59, 16716–16724.
50. H. Yan, Y. Matsushita, K. Yamaura and Y. Tsujimoto, Angew. Chem., Int. Ed., 2021, 60, 26561–26565.
51. Y. F. Shi, W. B. Wei, X. T. Wu, H. Lin and Q. L. Zhu, Dalton Trans., 2021, 50, 4112–4118.
52. X. Zhang, Y. Xiao, R. Wang, P. Fu, C. Zheng and F. Huang, Dalton Trans., 2019, 48, 14662–14668.
53. R. W. Godby, M. Schlter and L. J. Sham, Phys. Rev. B: Condens. Matter Mater. Phys., 1987, 36, 6497–6650.

---

Footnote

† Electronic supplementary information (ESI) available. CCDC 2132019. For ESI and crystallographic data in CIF or other electronic format see https://doi.org/10.1039/d2sc00099g

---