Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Giant change of MoS2 optical properties along amorphous–crystalline transition: broadband spectroscopic study including the NIR therapeutic window†
Jan
Mistrik
 *ab,
Milos
Krbal
a,
Vit
Prokop
a and
Jan
Prikryl
a
*ab,
Milos
Krbal
a,
Vit
Prokop
a and
Jan
Prikryl
a
aCenter of Materials and Nanotechnologies, Faculty of Chemical-technology, University of Pardubice, Studentska 95, 53210 Pardubice, Czech Republic. E-mail: jan.mistrik@upce.cz; Tel: +420 466 037 409
bInstitute of Applied Physics and Mathematics, Faculty of Chemical-technology, University of Pardubice, Studentska 95, 53210 Pardubice, Czech Republic
First published on 18th April 2023
Abstract
This work deals with an ellipsometric study of magnetron sputtered thin MoS2 films. The evolution of the UV-VIS-NIR optical properties of as-deposited and subsequently annealed films is thoughtfully investigated, covering amorphous, amorphous relaxed, partially crystallized, and polycrystallized MoS2 films. The transition from the mixed 1T′@2H local order in the amorphous phase toward the long-range 2H order in the polycrystalline phase is systematically correlated with film optical properties. The early stage of a few-layer 2H ordering toward the 2H bulk-like polycrystalline structure during annealing is evidenced through the energy shift of MoS2 prominent excitonic peaks. A considerable change in optical response between metallic (amorphous) and semiconducting (polycrystalline) MoS2 phases is reported and presented in terms of dielectric permittivity and normal reflectance NIR-VIS-UV spectra. Results of light–heat conversion in the NIR therapeutic window show so far uncovered potential of amorphous MoS2 as an agent for photothermal therapy. Spectroscopic ellipsometry provided sensitive characterization disclosing essential results complementary to other characterization tools. The benefit of these results is expected to be employed in fundamental and application-motivated research, for example, in the field of phase change materials, photothermal cancer therapy, and magneto-optical study of magnetic ordering in metal transition dichalcogenides, among others.
1 Introduction
MoS2 is an intensively studied material in its both bulk-like and reduced dimensionality forms, offering a plethora of new promising applications due to its rich and tunable structural properties.1–3 Owing to its polymorphism, crystalline MoS2 offers various phases, which contain Mo and S atoms arranged in different geometries, for example, octahedral (1T) or trigonal prismatic (2H). Nevertheless, all the crystalline phases present a layered structure built by parallel slabs (three atomic layers) of covalently bonded S–Mo–S atoms. The slabs are weakly bonded by van der Waals interactions. The discrepancy in the strength and nature of in-plane and out-of-plain bonding causes strong optical anisotropy (large birefringence phenomena) that can be employed, for example, for a light manipulation in photonic chips.4 Different bonding and symmetry of Mo and S atoms induce different physical properties and the manifestation of 1T and 2H phases. 2H MoS2 is semiconducting, whereas 1T is metallic.5,6 Moreover, the 2H phase is nonmagnetic, but the 1T phase shows ferromagnetic behavior.7 2H MoS2 presents an indirect to direct band gap transition when reducing thickness to the ultrathin limit.8,9 The photoluminescence of the 2H MoS2 monolayer is employed in optoelectronic applications.1 In its 2D form MoS2 shows fundamentally interesting excitonic magneto-optical activity induced by the spin proximity effect.10 Although the metallic 1T phase has limitations for optoelectronic applications, it becomes attractive as a catalyst for hydrogen evolution, photocatalysts, or supercapacitor electrode materials.11,12 Owing to optical absorption in NIR, the metallic 1T phase was proven to be an efficient agent for photothermal therapy in the near-infrared therapeutic window, as well.13–15 Unfortunately, this phase is thermodynamically unstable and easily converts to 2H. Nevertheless, it was recently reported that the stable MoS2 amorphous phase, with a mixture of 1T and 2H local atomic arrangement, reveals a similar bond structure to that in the 1T phase.16 This predicts promising applications of the less studied MoS2 amorphous phase in various fields, for example, high activity of amorphous MoS2 in the hydrogen evolution reaction16 or cancer treatment by photothermal therapy. The knowledge of the optical properties of the amorphous phase and their evolution due to bond rearrangement toward the 2H phase by thermal annealing is therefore crucial for both theoretical and applied research.On the other hand, owing to the attractiveness of MoS2 properties, up-scalable methods for the fabrication of MoS2 in its various phases and dimensions are constantly searched. One relatively simple method of 2H MoS2 preparation is magnetron sputtering of its amorphous phase with subsequent annealing. It is of fundamental and application interest the exact knowledge of phase change in this particular case. Monitoring the evolution of the optical properties of annealed films not only provides a characterization tool for this transformation but also offers information on their large variation during the phase change.
This work focuses on the ellipsometric study of magnetron sputtered thin MoS2 films. The concern is devoted to the evolution of the optical properties of as-deposited and subsequently annealed films. This corresponds with amorphous, amorphous relaxed, partially crystallized, and polycrystallized MoS2 films. In other words, the transition from the amorphous mixed phase of tetragonal distorted (1T′) and the trigonal prismatic (2H) local order (denoted here as 1T′@2H) toward the long-range 2H order in the polycrystalline phase is systematically studied through film optical property modification. Evidence of a few-layer 2H ordering toward a bulk-like polycrystalline structure during annealing is discussed through the energy shift of MoS2 prominent excitonic peaks. A huge change in optical response between metallic (amorphous) and semiconducting (polycrystalline) MoS2 phases is reported and presented in terms of determined dielectric permittivity and normal reflectance NIR-VIS-UV spectra. The optical properties of the amorphous MoS2 phase make an original contribution to the field. Additionally, results of light–heat conversion in the NIR therapeutic window show so far uncovered potential of amorphous MoS2 as an agent for photothermal therapy. Ellipsometry is presented as a rather sensitive characterization tool providing both supporting and complementary results to other material characterization techniques. The value of these results is expected to be found in fundamental and application-motivated research, for example, in phase change materials,17 photothermal therapy, and magneto-optical characterization of MoS2 magnetism, among others.
2 Experimental
Amorphous MoS2 films were sputter deposited onto naturally oxidized c-Si wafers. Their backsides were intentionally grounded to prevent backside reflections in the NIR range. Subsequent annealing in sealed quartz ampules at a residual pressure of 10−3 Pa to prevent oxidation was performed for selected temperatures up to 900 °C. A furnace with a heating rate of 2 °C min−1 was used. Samples were kept at the given temperature for one hour and then naturally cooled down to room temperature. Complementary characterization by XPS, XAS, sheet resistance, and XRD was previously reported in ref. 6 and 18.Ellipsometric spectra were recorded by using a rotating analyzer VASE ellipsometer (Woollam, Co. Ltd) in the spectral range from 0.7 to 6.5 eV at incidence angles 50°, 60°, and 70°. Nearly normal (angle of incidence 18°, p-incident polarization) reflectance measurements were carried out by using the same instrument in a one-beam configuration. Normal incidence transmittance measurements performed on MoS2 films deposited under the same conditions on transparent fused silica substrates were scanned by using a JASCO V-570 spectrophotometer. Besides ellipsometric parameters Ψ and Δ (ratio of rp and rs coefficients and their mutual shift), the degree of depolarization was monitored as well. Ellipsometry is a phase sensitive technique and, when employed in variable angle spectroscopic configuration and further coupled with spectrophotometry (reflectance and transmittance), provides precise and accurate results. Ellipsometry, an indirect characterization tool, requires the construction of a sample model for data interpretation. In our case, a semi-infinitive c-Si substrate, SiO2 overlayer (native oxide), and MoS2 film were employed in reflection configuration, whereas a fused silica substrate and MoS2 film were employed for transmittance measurements. Surface roughness that was considered by Bruggeman effective medium approximation (with 50% of voids) can simultaneously model slight surface oxidization as well. Sputtering yields uniform films that are corroborated by low depolarization values. Modeling does not show any index gradient. Therefore MoS2 is modeled as uniform homogeneous single layers. The optical constants of c-Si and SiO2 native oxide were taken from the software database WVASE32.
Light–heat conversion in the NIR therapeutic window was examined by exposure of selected films (as deposited and 900 °C annealed) to supercontinuum radiation (WhiteLase Micro, NKT Photonics). A longpass optical filter was used to cut wavelengths lower than 1000 nm, and therefore a collimated 4 mm diameter polychromatic (1000–2200 nm) beam was directed to the film surface under the angle of incidence of 45 deg. The total spectral power of the incident beam was 340 mW. The film temperature increase was monitored by using an FLIR i7 infrared camera (FLIR System, Inc.). The emissivity of film surfaces was set to 0.6. NIR transparent fused silica substrates were used to avoid light absorption and subsequent heat generation.
3 Model dielectric function of MoS2
The model dielectric function (MDF) of MoS2 in the NIR-VIS-UV range is usually constructed by a sum of oscillators of various types (for example, Gauss, Lorentz, or Tauc–Lorentz), the number of which depends on the available spectral range and spectral resolution.19–22 The appropriateness of oscillator type selection is discussed in ref. 19. Nevertheless, other mathematical functions specifically designed for electronic transitions in the vicinity of MoS2 band structure critical points and rich excitonic features were employed as well.21,23 Our choice is the sum of Lorentz oscillators due to its high appearance in the literature that facilitates a straightforward comparison of the obtained results. As will be discussed later in the text, the number of oscillators depends on the annealing temperature starting from 3 (as-deposited films) and reaching the number of 9 (900 °C annealed polycrystalline films). Hence, the model dielectric function gets the form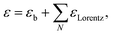 | (1) |
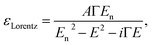 | (2) |
4 Results and discussion
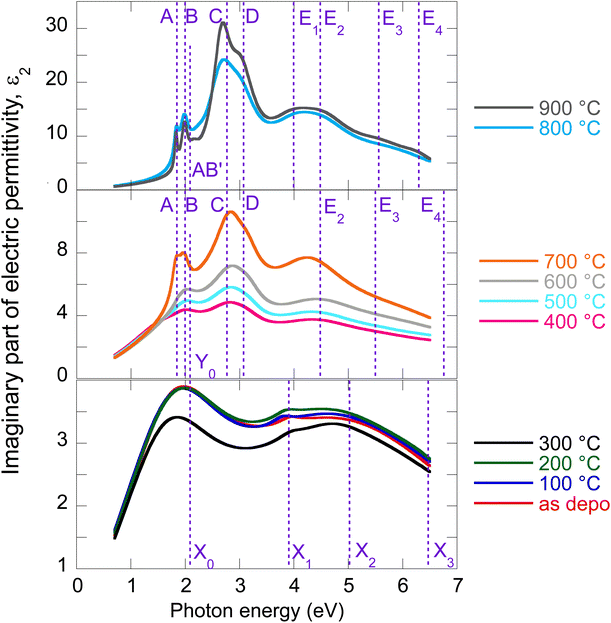
The evolution of optical constants, displayed in the form of real and imaginary parts of MoS2 dielectric permittivity, versus annealing temperature is presented in Fig. 1 (for the wavelength dependence of the refractive index and extinction coefficient, refer to ESI, Fig. S3).† The following sections are devoted to interpreting these changes in terms of MoS2 transformation from its amorphous (as-deposited) phases to the 2H polycrystalline phase (films annealed with the highest temperatures 800 °C and 900 °C).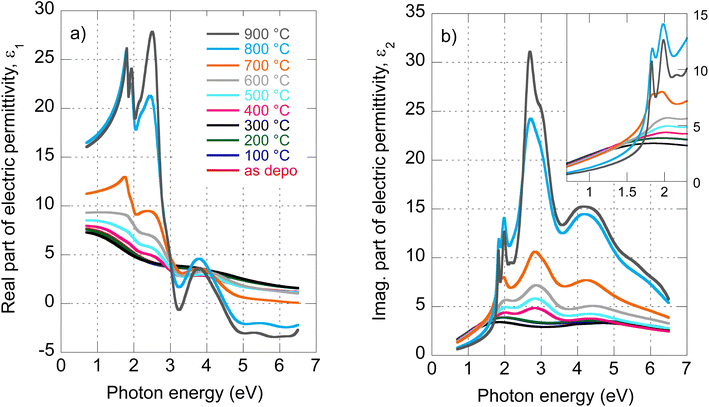 | ||
| Fig. 1 Determined real part (a) and imaginary part (b) of MoS2 electric permittivity spectra as a function of annealing temperature. | ||
4.1 2H polycrystalline phase (900 °C annealed film)
We start our discussion with the polycrystalline film annealed at the highest temperature of 900 °C. Regarding our recent paper,18 this film shows a nearly textured polycrystalline 2H phase with a negligible contribution of other phases (supported by XPS spectra deconvolution). The films are semiconducting with a sheet resistance of about 107 Ω cm−2 that is expected for the 2H MoS2 phase.5The optical properties of 2H MoS2 were extensively studied in its bulk and (ultra)thin film forms (refer, for example, to ref. 4, 19 and 23–25). The strong light–matter coupling of 2H MoS2 in the VIS range includes prominent features of exciton contributions generally labeled A, B, C, and D. It has been reported that the spectral positions of these excitons are, in the ultrathin limit (dimensions less than about 4 nm), more or less thickness dependent due to the quantum confinement. Additionally, the quantum confinement induces 2H MoS2 band structure modifications that transform its band gap from the indirect (bulk) to the direct (monolayer) form. This effect was initially reported in photoluminescence studies.8 In our case, ellipsometry measurements revealed a film thickness of about 20 nm, and therefore, no significant quantum confinement or band gap modification is expected in our polycrystalline 2H MoS2 films.
The electric permittivity of the film was parameterized by 9 Lorentz oscillators accounting for the excitons and critical points of the Brillouin zone. The individual contributions are presented in Fig. 2a in the form of its imaginary part. In the VIS range, the excitonic features A, B, C, and D dominate over other contributions and are clearly identified. The origins and assignments of these transitions are discussed in the literature (see, for example, ref. 20, 21 and references therein). The A and B peaks are assigned to the transition from the spin–orbit split valence band to the lowest conduction band at the K and K′ points, whereas the C and D excitons are predominantly associated with the transition in the part of the Brillouin zone (BZ) between K and Gamma points with parallel valence and conduction bands.8,26 The positions of excitonic peaks that we have determined are mentioned in Table 1. Their respective values are EA = 1.83 eV, EB = 1.98 eV, EC = 2.67 eV, and ED = 3.04 eV, which are representative values for bulk like MoS2.21,23,27,28
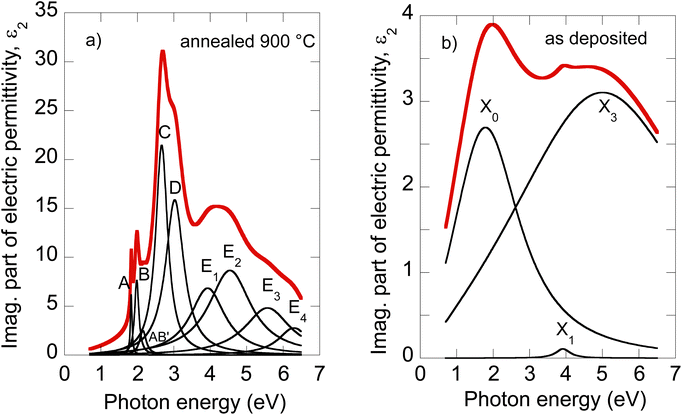 | ||
| Fig. 2 Deconvolution of the MoS2 optical constants of the 900 °C annealed film (a) and as-deposited film (b). | ||
| Temp. | Num. | Y 0 | A | B | AB′ | C | D | E 1 | E 2 | E 3 | E 4 | SR | t | MSE |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 900 | 9 | 1.83 | 1.98 | 2.15 | 2.67 | 3.04 | 3.97 | 4.59 | 5.62 | 6.31 | 1.3 | 22.7 | 6 | |
| 800 | 8 | 1.83 | 1.98 | 2.22 | 2.67 | 3.04 | 4.03 | 4.59 | 5.92 | 0.0 | 21.4 | 3 | ||
| 700 | 8 | 2.68 | 1.83 | 1.97 | 2.83 | 3.15 | 4.34 | 5.39 | 6.47 | 0.0 | 32.5 | 2 | ||
| 600 | 8 | 2.39 | 1.87 | 2.00 | 2.87 | 3.19 | 4.50 | 5.63 | 6.74 | 0.0 | 57.1 | 2 | ||
| 500 | 8 | 2.09 | 1.88 | 2.02 | 2.87 | 3.18 | 4.51 | 5.70 | 7.11 | 0.0 | 62.1 | 1 | ||
| 400 | 8 | 1.61/2.41 | 2.01 | 2.84 | 3.16 | 4.48 | 5.90 | 6.91 | 1.3 | 65.8 | 2 |
| X 0 | X 1 | X 2 | X 3 | SR | t | MSE | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 300 | 4 | 1.99 | 3.95 | 4.78 | 6.09 | 0.3 | 56.2 | 2 | ||||||
| 200 | 4 | 2.16 | 3.86 | 5.30 | 6.70 | 0.0 | 54.6 | 2 | ||||||
| 100 | 4 | 2.17 | 3.79 | 5.06 | 6.90 | 0.0 | 57.2 | 2 | ||||||
| As-deposited | 3 | 2.12 | 3.92 | 5.89 | 0.8 | 57.2 | 2 |
The oscillator strength of A, B, and C excitons reported in the literature spans over a broad range (see, for example, ref. 29 and references therein). It should be noted here that for correct exciton amplitude determination, the transmittance spectra should be added and treated in parallel with ellipsometry and reflectance spectra. This approach minimizes the correlation between film thickness and MoS2 extinction in the film semitransparent spectral region – the issue often encountered for ellipsometry treatment of thin absorbing films. In our case, transmittance measurements carried out on MoS2 films deposited and annealed under the same conditions on transparent fused silica substrates were included in data (ellipsometry and reflectance) treatment, and hence, controlling the final fit and reliability of the values of adjusted parameters. More information about transmittance measurements is reported in the ESI (see Fig. S1).†
It is worth noting that excited states of A and B excitons were also detected and labeled (AB)′ oscillator in Fig. 2a. Hence, well-developed exciton features A, B, C, and D together with the presence of excited states (AB)′ prove the high quality of the 2H MoS2 film.
Other oscillators identified in UV (3.5–6 eV), labeled here E1–E4 (cf.Table 1), are generally interpreted as electronic transitions between the valence band and conduction and exited bands of BZ in its high symmetry points.20,21 A different number of oscillators (for example, two20,22 or four21,30) were used in the literature to parameterize the 2H MoS2 electric permittivity in this spectral range. The assignment of these transition energies depends on spectra deconvolution and is, in general, difficult due to the complex nature of the excited bands of MoS2 BZ.
It is known that monocrystalline MoS2 presents strong optical anisotropy,4 and therefore texturing or preferential crystal orientation can be an issue for ellipsometry data treatment. Preferential (002) orientation of the 900 °C annealed film was reported in our previous XRD study.18 In this case, the uniaxial optical axis is perpendicular to the sample surface. Therefore, only effective optical constants are determined from oblique light incidence ellipsometry measurements (in-plane and out-of-plane contributions cannot be separated). Nevertheless, due to the high MoS2 refractive index value, light refracted to the film propagates nearly perpendicularly to the surface and, therefore, senses mainly in-plane permittivity contribution. Out-of-plane permittivity represented by weak vdW bonds does not show any spectral structures in VIS and can be parameterized by the simple Cauchy dispersion relation as reported in ref. 4.
Defect-free bulk 2H MoS2 monocrystals are transparent in NIR for photon energies lower than the onset of A-exciton absorption.24 Nevertheless, our polycrystalline film shows a moderate absorption tail due to structural imperfections or defects. This was similarly detected and discussed, for example, by Singh et al., who have recently disclosed the importance of the near-infrared optical properties of transition metal disulfides, including MoS2, searching new alternative phase change materials.17
4.2 Amorphous phase (as-deposited film)
Our recent X-ray study proved the amorphous phase in as-deposited films. Moreover, XPS characterization showed bonds with local symmetry of a 2H@1T′ mixed phase,18 quantitatively estimated to be around 50% each with only a slight contribution of MoOx on the film surface. The sheet resistance of as-deposited films (about 103 Ω cm−2) was four orders lower with respect to the polycrystalline 2H phase mainly due to the Mo–Mo bond network, which is not present in the 2H phase but built via partial 1T′ local ordering in the 2H@1T′ mixed phase.18 The change in MoS2 electrical transport properties from the tetragonal 1T (or distorted 1T′) metallic phase containing Mo–Mo bonds to the hexagonal 2H semiconducting phase is well known (see, for example, ref. 5).To our knowledge, the optical properties of amorphous MoS2 films have not been systematically investigated. We can report only absorbance measurements on amorphous MoS2 films31 or nanoparticle suspensions.32 In contrast to the (poly)crystalline 2H phase, no excitonic features are observed in the amorphous phase, as is evident from Fig. 2b, where we present the imaginary part of as-deposited film electric permittivity. The significantly lower absorption (lower light–matter coupling) in the amorphous phase in the VIS is also worth noting. In contrast to the NIR, the amorphous phase shows enhanced extinction over the crystalline phase (cf. also Fig. 1b). This could be an unexpected result considering that sulfur-based amorphous chalcogenides such as As2S3 and related materials with the so-called valence alternation pairs are highly transparent in the NIR.33 Nevertheless, amorphous MoS2 does not show this optical behavior. This is probably due to the absence of valence alternation pairs (VAPs) in the amorphous phase and the existence of homopolar metallic Mo–Mo bonds.18 Accordingly, the effect of band gap shrinking and development of an absorption tail in the NIR was recently reported for an amorphous thin film along the As40S60–MoS3 tie-line.34
Owing to less featured spectral dependence of amorphous MoS2 electric permittivity (compared to the crystalline one), its deconvolution consists of only 3 Lorentz oscillators as indicated in Fig. 2b and Table 1. One contribution labeled X0 is located at 2.1 eV, and the other two labeled X1 and X3 at 3.9 and 5.9 eV, respectively. Considering the metallic nature of the amorphous phase with the contribution of the homopolar Mo–Mo atomic network, we compare the identified electronic transitions with those reported for metallic molybdenum localized at 1.7, 2.3, and 2.4 eV.35 Accordingly, we suggest that the X0 transition at 2.1 eV relates mainly (but not solely) to the homopolar Mo–Mo metallic bonds. As will be shown later, this transition remains present for films annealed up to 600 °C but disappears for highly annealed (700–900 °C) films with a dominantly 2H semiconductor nature.
The other electronic transitions X1 and X3 occur in the spectral range where 2H polycrystalline MoS2 features several, E1–E4, valence to conduction, and higher band transitions (cf.Table 1). However, their precise assignment is difficult due to the mixed contribution of 1T′ and 2H locally coordinated S–S and Mo–S bonds. Nevertheless, it is interesting to point out the close position of X1 and E1, and X3 and E4 transitions disclosed in the mixed 1T′@2H amorphous and 2H polycrystalline phases.
Considering the huge difference between the optical properties of 2H polycrystalline and amorphous MoS2, photonic devices based on order–disorder switching are, in principle, realizable. On the other hand, switching energy (annealing up to 900 °C) is unacceptably high. To overcome this problem designing thermodynamically stable alloys based on transition metal disulfides, including MoS2, is an alternative being explored.3,17 Other routes toward amorphous-to-crystalline phase transformation in MoS2 as ion or electron beam irradiation are investigated as well,36,37 and knowledge of the optical properties of the amorphous phase is beneficial for its characterization or metasurface based photonic applications.
Another benefit from detected enhanced absorption of amorphous MoS2 in the NIR that covers the therapeutic window would be its application in photo-thermal therapy. High photo-thermal conversion has been already reported for metastable 1T MoS2 nanosheets and nanoflakes.13–15,38,39 The high activity of amorphous MoS2 in the Hydrogen Evolution Reaction32 interpreted by similar local bonding to that in 1T MoS2 (ref. 16) and considering high biocompatibility and stability etc. makes amorphous MoS2 a promising candidate for a new generation agent in photothermal therapy. The light–heat conversion of the amorphous film in the NIR therapeutic spectral window will be discussed in more detail in Section 4.5.
4.3 Transition from an amorphous to a polycrystalline phase
From previous sheet resistance measurements and XPS study, three stages were identified while annealing the films: (i) amorphous as-deposited state and its relaxation, (ii) progressive crystallization, and finally, (iii) grain enlargement and texturization.6 Here we supplement our previous findings with an ellipsometry study exploring optical constant evolution along with all these stages. As optical properties are closely related to electrical and structural properties, it is expected that similar trends will be captured in the optical behavior as well.The electric permittivity of the 400 °C annealed film contains already contributions of the B, C, and D excitons that are characteristic of the 2H MoS2 phase and were discussed in the previous text. From 500 °C, all four main excitons A, B, C, and D become present and gain oscillator strength with increasing annealing temperature. This is consistent with the previously presented picture of preferential 2H bond re-ordering and nucleation of 2H phase crystalline grains. The blue shift of the C exciton resonant energy with respect to the polycrystalline 900 °C film (from 2.67 eV to 2.87 eV, cf.Table 1) can be interpreted by the gradual layering of the crystallized 2H phase in nanosized crystalline grains. It is well known that due to the quantum confinement, the excitonic energy is blue-shifted for few layered 2H MoS2 and this is most pronounced for C, D, and A excitons (cf.Table 1). Referring to literature values,23 resonant energy 2.84–2.87 eV, determined in our case for the C exciton (films annealed between 400 and 700 °C), corresponds to 2–5 layered MoS2. Further annealing (800 °C and 900 °C annealed films) shifts this energy towards bulk-like values that correspond in our case to augmented crystalline grains with more than 15 S–Mo–S layers, where the C exciton energy is located at 2.67 eV. A similar shift is detected in the case of A and D excitons as well. The spectral position of the B exciton is nearly independent consistently with literature reports.20,21,28
Y 0 electronic transition located around 2.1–2.7 eV seems to be of a similar origin (i.e., the contribution of the homopolar Mo–Mo bonds) to the X0 transition in the as-deposited and relaxed amorphous phases locally 1T, 1T′, and 2H coordinated. On the other hand, the X1–X3 transitions of the amorphous phase seem to be replaced (for the films annealed between 400 and 700 °C) with the E2–E4 transitions that already capture the spectral position of the pure 2H polycrystalline phase (900 °C annealed film). A slight variation in their values with annealing temperature is due to the presence of other phases with different filling factors.
Although the nature of the bonding and structural changes is rather complex during the film annealing, we have also considered a rough approximation considering the MoS2 film as a mixture of an amorphous phase hosting small 2H crystalline inclusions (nucleated grains, increasing in volume with annealing temperature). The optical constants of the polycrystalline film annealed to 800 °C approximated the pure 2H crystalline phase. This choice was due to the expected slight optical anisotropy of the 900 °C annealed film induced by its partial texturing (discussed in more detail later in the text). In other words, the percentage of the crystalline phase at 800 °C was set to 100%. The as-deposited film approximated the amorphous phase. Applying Maxwell-Garnet and Bruggeman's effective medium theories, we were able to estimate an increase of the filling factor of the 2H crystalline phase: 7% (400 °C), 12% (500 °C), 18% (600 °C) and 48% (700 °C). It is worth noting that relatively good fit quality was obtained with a mean squared error (MSE) value of about 6–12 (cf.Table 2). The above-mentioned effective medium approximations contain an additional parameter, depolarization factor Q, that is related to the shape of inclusions. Adjusting it in a fitting procedure yielded its value to be zero for 400–600 °C annealed films and Q = 0.31 for the 700 °C annealed film. This could be related to the needle-like structure of nucleation centers that increase in volume toward the spherical geometry for 700 °C annealing. The increasing volume fraction of the semiconductive 2H phase makes the films less conductive, which is consistent with increased sheet resistance reported in ref. 6.
| Temp. | MG/BG | Q | Amorph. % | Crystal % | Thickness | SR | MSE |
|---|---|---|---|---|---|---|---|
| 700 | BG | 0.31 | 52 | 48 | 33 | 2 | 6 |
| 600 | MG | 0 | 82 | 18 | 55 | 0 | 11 |
| 500 | MG | 0 | 88 | 12 | 60 | 2 | 12 |
| 400 | MG | 0 | 93 | 7 | 62 | 5 | 12 |
4.4 Reflectivity
Disclosed significant variations of real and imaginary parts of optical constants along amorphous to crystalline transition, both stable phases, opens a new potential path for the application of this material employing the phase change functionality. For this reason, we provide in Fig. 4 reflectance spectra recorded for all samples with given annealing temperatures. Even if experimental data of R are available (angle of incidence 18°), we provide in Fig. 4 re-calculated normal incidence R spectra in the entire spectral range. The large increase in reflectance from the amorphous (20%) to crystalline (50%) phase is noticeable.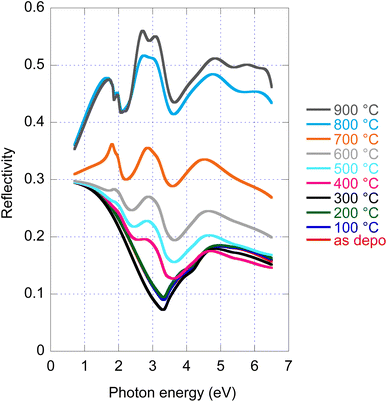 | ||
| Fig. 4 As deposited and annealed MoS2 film reflectance calculated for normal incidence and film with a nominal thickness of 50 nm. | ||
4.5 Light–heat conversion in the NIR therapeutic window
We carried out a supplementary experiment to support our conviction that amorphous MoS2 is a promising candidate for photothermal therapy. Amorphous (as deposited) and crystalline (900 °C annealed) films were exposed to light from a supercontinuum laser source. A long pass filter was inserted into the incident beam to restrict its spectral range in the interval 1000–2200 nm and total transmitted power of 340 mW. The selected spectral range covers the following parts of the biological transparency window:15 NIR-II (1000–1350 nm) and NIR-III (1600–1870 nm) as indicated in Fig. 5a. We are interested in light–heat conversion exclusively generated in the film; therefore we selected samples with transparent fused silica substrates. An infrared camera was used to record film temperature increase while the samples were irradiated (cf. images in Fig. 5b(1) and b(2)). The observed time evolution of sample temperature is plotted in Fig. 5c.The naked substrate was considered as well as a control sample. Irradiation did not cause any temperature change in this case as expected due to fused silica transparency in the NIR. On the other hand, irradiation of the amorphous and 2H polycrystalline MoS2 films resulted in a steep increase of recorded temperature shortly after the beam was switched on (during the first minute), and then the temperature was gradually saturated (cf.Fig. 5c). The saturation temperature is significantly higher for amorphous (≈52 °C) than the crystalline (≈36 °C) film, which is consistent with the higher values of the amorphous MoS2 extinction coefficient with respect to its 2H crystalline phase (see Fig. 5a). Zhou and coworkers reported a temperature increase of polyvinylpyrrolidone-modified MoS2 nanodots to a value of about 32 °C (2H phase) and to about 53 °C (1T phase) when irradiated in a solution (50 ppm) with a NIR laser (1064 nm, 1.0 W cm−2).14 Additionally, Yougbaré et al. reported an increase of temperature in 1T phase MoS2 nanosheet solution (100 μg mL−1) to about 54 °C when irradiated with an 808 nm laser diode with a power density of 1.0 W cm−2.43 Although the mentioned results are not directly comparable with our findings, due to different experimental arrangements (light source spectral range and its power, a form (nanoparticle solution and thin film) of MoS2), our data show that light–heat conversion in the amorphous MoS2 film displays a similar enhanced tendency to that reported for MoS2 in its 1T crystalline phase. Moreover, as the amorphous phase is thermodynamically more stable than 1T, we suggest that amorphous MoS2 is an even better candidate for the photothermal agent for photothermal therapy.
5 Conclusions
A spectroscopic ellipsometry study of as-deposited magnetron sputtered MoS2 thin films disclosed the original UV-VIS-NIR optical properties of the amorphous MoS2 phase with a mixed 1T′@2H local order. The subsequent gradual annealing of the films and their systematic optical investigation revealed a large optical contrast along the transition from the amorphous to the 2H MoS2 crystalline phase that is attractive for phase-change applications. The evolution of the obtained optical constants was correlated with material structural modifications capturing the nucleation of few layer nanostructured 2H centers and their enlargement toward the polycrystalline phase. The results of light–heat conversion in the NIR therapeutic window show so far uncovered potential of amorphous MoS2 as an agent for photothermal therapy. Applied spectroscopic ellipsometry proved to be a sensitive and reliable tool providing consistent results with other characterization tools such as XRD, XPS, and sheet resistance measurements.Author contributions
J. Mistrik: methodology, investigation – ellipsometry and reflectance spectra recording, together with light–heat conversion measurements, formal analyses of the data, their validation and curation, and writing – original draft. M. Krbal: supervision, conceptualization, and writing – review & editing. V. Prokop: sample preparation. J. Prikryl: investigation.Conflicts of interest
There are no conflicts to declare.Acknowledgements
This work was supported by the Czech Science Foundation 19-17997S and the Ministry of Education, Youth, and Sports (LM2018103).Notes and references
- T. Mueller and E. Malic, npj 2D Mater. Appl., 2018, 2, 29 CrossRef.
- W. Li, X. Qian and J. Li, Nat. Rev. Mater., 2021, 6, 829–846 CrossRef CAS.
- A. Singh, Y. Li, B. Fodor, L. Makai, J. Zhou, H. Xu, A. Akey, J. Li and R. Jaramillo, Appl. Phys. Lett., 2019, 115, 161902 CrossRef.
- G. Ermolaev, D. Grudinin, Y. Stebunov, K. Voronin, V. Kravets, J. Duan, A. Mazitov, G. Tselikov, A. Bylinkin, D. Yakubovsky, S. Novikov, D. Baranov, A. Nikitin, I. Kruglov, T. Shegai, P. Alonso-González, A. Grigorenko, A. Arsenin, K. Novoselov and V. Volkov, Nat. Commun., 2021, 12, 854 CrossRef CAS PubMed.
- S. Mukherjee, S. Biswas, A. Ghorai, A. Midya, S. Das and S. K. Ray, J. Phys. Chem. C, 2018, 122, 12502–12511 CrossRef CAS.
- M. Krbal, J. Prikryl, I. Pis, V. Prokop, J. Rodriguez Pereira and A. V. Kolobov, Ceram. Int., 2023, 49(2), 2619–2625 CrossRef CAS.
- Y. Hu, X. Zeng, T. Ren, S. Wu, W. Wang, Y. Zeng, G. Zhou, Z. Guo, W. Jin, S. Wang, Y. Xiao and J. Lu, J. Mater. Chem. C, 2018, 6, 11651–11658 RSC.
- K. F. Mak, C. Lee, J. Hone, J. Shan and T. F. Heinz, Phys. Rev. Lett., 2010, 105, 136805 CrossRef PubMed.
- A. Splendiani, L. Sun, Y. Zhang, T. Li, J. Kim, C.-Y. Chim, G. Galli and F. Wang, Nano Lett., 2010, 10, 1271–1275 CrossRef CAS PubMed.
- G. Catarina, N. M. R. Peres and J. Fernández-Rossier, 2D Mater., 2020, 7, 025011 CrossRef CAS.
- K. Chang, X. Hai, H. Pang, H. Zhang, L. Shi, G. Liu, H. Liu, G. Zhao, M. Li and J. Ye, Adv. Mater., 2016, 28, 10033–10041 CrossRef CAS PubMed.
- L. Jiang, S. Zhang, S. A. Kulinich, X. Song, J. Zhu, X. Wang and H. Zeng, Mater. Res. Lett., 2015, 3, 177–183 CrossRef CAS.
- V. Yadav, S. Roy, P. Singh, Z. Khan and A. Jaiswal, Small, 2019, 15, 1803706 CrossRef PubMed.
- Z. Zhou, B. Li, C. Shen, D. Wu, H. Fan, J. Zhao, H. Li, Z. Zeng, Z. Luo, L. Ma and C. Tan, Small, 2020, 16, 2004173 CrossRef CAS PubMed.
- N. D. Thorat, S. A. M. Tofail, B. von Rechenberg, H. Townley, G. Brennan, C. Silien, H. M. Yadav, T. Steffen and J. Bauer, Appl. Phys. Rev., 2019, 6, 041306 CAS.
- L. Wu, A. Longo, N. Y. Dzade, A. Sharma, M. M. R. M. Hendrix, A. A. Bol, N. H. de Leeuw, E. J. M. Hensen and J. P. Hofmann, ChemSusChem, 2019, 12, 4383–4389 CrossRef CAS PubMed.
- A. Singh, S. S. Jo, Y. Li, C. Wu, M. Li and R. Jaramillo, ACS Photonics, 2020, 7, 3270–3285 CrossRef CAS.
- M. Krbal, V. Prokop, A. Kononov, J. Pereira, J. Mistrik, A. Kolobov, P. Fons, Y. Saito, S. Hatayama, Y. Shuang, Y. Sutou, S. Rozhkov, J. Stellhorn, S. Hayakawa, I. Pis and F. Bondino, ACS Appl. Nano Mater., 2021, 4, 8834–8844 CrossRef CAS.
- G. Ermolaev, Y. Stebunov, A. Vyshnevyy, D. Tatarkin, D. Yakubovsky, S. Novikov, D. Baranov, T. Shegai, A. Nikitin, A. Arsenin and V. Volkov, npj 2D Mater. Appl., 2020, 4, 21 CrossRef CAS.
- D. Li, X. Song, J. Xu, Z. Wang, R. Zhang, P. Zhou, H. Zhang, R. Huang, S. Wang, Y. Zheng, D. W. Zhang and L. Chen, Appl. Surf. Sci., 2017, 421, 884–890 CrossRef CAS.
- X. Zhu, D. Li, R. Zhang, H. Zhang, C. Cong, M. Zhu, Y. Shi, Y. Wu, S. Wang, Y. Zheng and L. Chen, Appl. Surf. Sci., 2020, 519, 146262 CrossRef CAS.
- S. Sato, N. Nitta, M. Sakamoto, L. Liu, P. Rutthongjan, M. Nishi, M. Ueda, T. Yasuoka, R. Hasegawa, Y. Tagashira, T. Ozaki, E. K. C. Pradeep, G. T. Dang and T. Kawaharamura, Jpn. J. Appl. Phys., 2018, 57, 110306 CrossRef.
- Y. Yu, Y. Yu, Y. Cai, W. Li, A. Gurarslan, H. Peelaers, D. Aspnes, C. Van De Walle, N. Nguyen, Y.-W. Zhang and L. Cao, Sci. Rep., 2015, 5, 16996 CrossRef CAS PubMed.
- A. R. Beal and H. P. Hughes, J. Phys. C: Solid State Phys., 1979, 12, 881–890 CrossRef CAS.
- M. Ye, D. Winslow, D. Zhang, R. Pandey and Y. K. Yap, Photonics, 2015, 2, 288–307 CrossRef CAS.
- A. Molina-Sánchez, D. Sangalli, K. Hummer, A. Marini and L. Wirtz, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 88, 045412 CrossRef.
- G. Y. Jia, Y. Liu, J. Y. Gong, D. Y. Lei, D. L. Wang and Z. X. Huang, J. Mater. Chem. C, 2016, 4, 8822–8828 RSC.
- K. P. Dhakal, D. L. Duong, J. Lee, H. Nam, M. Kim, M. Kan, Y. H. Lee and J. Kim, Nanoscale, 2014, 6, 13028–13035 RSC.
- P. R. Stevenson, R. T. Busch, R. Torsi, A. M. Jawaid, R. Rao, D. B. Lioi, J. A. Robinson, N. R. Glavin, R. A. Vaia, W. J. Kennedy and J. P. Vernon, ACS Photonics, 2021, 8, 1705–1717 CrossRef CAS.
- V. Le, T. Kim, H. Park, H. Nguyen, X. Nguyen and Y. Kim, Curr. Appl Phys., 2019, 19, 182–187 CrossRef.
- G. Eda, H. Yamaguchi, D. Voiry, T. Fujita, M. Chen and M. Chhowalla, Nano Lett., 2012, 12, 526 CrossRef CAS.
- N. Saha, A. Sarkar, A. B. Ghosh, A. K. Dutta, G. R. Bhadu, P. Paul and B. Adhikary, RSC Adv., 2015, 5, 88848–88856 RSC.
- M. Kastner, D. Adler and H. Fritzsche, Phys. Rev. Lett., 1976, 37, 1504–1507 CrossRef CAS.
- M. Krbal, V. Prokop, V. Cervinka, S. Slang, B. Frumarova, J. Mistrik, P. Provotorov, M. Vlcek and A. Kolobov, Mater. Res. Bull., 2022, 153, 111871 CrossRef CAS.
- J. H. Weaver, D. W. Lynch and C. G. Olson, Phys. Rev. B: Condens. Matter Mater. Phys., 1974, 10, 501–516 CrossRef CAS.
- B. Kim, H. Gu and Y. Yoon, Sci. Rep., 2017, 7, 3874 CrossRef PubMed.
- P. Valerius, S. Kretschmer, B. V. Senkovskiy, S. Wu, J. Hall, A. Herman, N. Ehlen, M. Ghorbani-Asl, A. Grüneis, A. V. Krasheninnikov and T. Michely, 2D Mater., 2020, 7, 025005 CrossRef CAS.
- M. Xu, K. Zhang, Y. Liu, J. Wang, K. Wang and Y. Zhang, Colloids Surf., B, 2019, 184, 110551 CrossRef CAS PubMed.
- S. Yougbaré, C. Mutalik, P.-F. Chung, D. I. Krisnawati, F. Rinawati, H. Irawan, H. Kristanto and T.-R. Kuo, Nanomaterials, 2021, 11, 3064 CrossRef PubMed.
- A. Castellanos-Gomez, J. Quereda, H. P. van der Meulen, N. Agraït and G. Rubio-Bollinger, Nanotechnology, 2016, 27, 115705 CrossRef PubMed.
- G. Pradhan and A. K. Sharma, Appl. Surf. Sci., 2019, 479, 1236–1245 CrossRef CAS.
- L. Fei, S. Lei, W.-B. Zhang, W. Lu, Z. Lin, C. Lam, Y. Chai and Y. Wang, Nat. Commun., 2016, 7, 12206 CrossRef CAS PubMed.
- S. Yougbaré, C. Mutalik, P.-F. Chung, D. I. Krisnawati, F. Rinawati, H. Irawan, H. Kristanto and T.-R. Kuo, Nanomaterials, 2021, 11, 3064–3076 CrossRef PubMed.
Footnote |
| † Electronic supplementary information (ESI) available: (i) absorbance spectra, (ii) photos of MoS2 films, (iii) spectral deconvolution of MoS2 electric permittivity in a graphical form and (iv) wavelength dependence of the refractive index and extinction coefficient. See DOI: https://doi.org/10.1039/d3na00111c |
| This journal is © The Royal Society of Chemistry 2023 |