Redispersion mechanisms of 2D nanosheets: combined role of intersheet contact and surface chemistry†
Bei
Liu‡
a,
Jingyan
Zhang‡
bc,
Qi
Han
a,
Yufei
Shu
a,
Li
Wang
a,
Hui
Li
 c,
Lei
Li
c,
Lei
Li
 *b and
Zhongying
Wang
*b and
Zhongying
Wang
 *a
*a
aSchool of Environmental Science and Engineering, Southern University of Science and Technology, Shenzhen 518055, China. E-mail: wangzy6@sustech.edu.cn
bDepartment of Material Science and Engineering, Southern University of Science and Technology, Shenzhen 518055, China. E-mail: lil33@sustech.edu.cn
cBeijing Advanced Innovation Center for Soft Matter Science and Engineering, Beijing University of Chemical Technology, Beijing 100029, China
First published on 27th December 2022
Abstract
Redispersion behavior recovers the important features of nanomaterials and thus holds great promise for exciting applications of nanomaterials in different fields. In contrast to the redispersion of nanoparticles, which is mainly determined by surface chemistry, the redispersion of 2D nanosheets could be more complicated and is not well understood. In the present study, the redispersion behavior of 2D NMs was investigated by selecting representative nanosheets, MoS2, graphene oxide and their derivatives with both experimental methods and molecular dynamics (MD) simulations. The good agreement between experiments and MD simulations suggested that the redispersion in response to surface chemistry was regulated by the alignment configurations of the nanosheets. More importantly, we revealed that the difference in the hydrophilicity properties is responsible for the distinctive separation distances of the 1T and 2H MoS2 nanosheets. Appropriately adjusting the alignment configuration of the nanosheets can alter the effect of surface hydrophilicity on the redispersion behavior. Based on these fundamental findings, we identified three distinctive zones for the redispersion tendency of the 2D nanosheets with different surface hydrophilicity, Hamaker constants and intersheet contacts. As one of the implications, the results serve as a prescreening for the stability of the 2D restacking-based membrane. For the first time, the study systematically reported the interplay of intersheet configuration and surface chemistry in the redispersion of nanosheets, which provides a theoretical foundation for the processing and applications of 2D nanomaterials.
Introduction
Two-dimensional nanomaterials (2D NMs) are defined as nanomaterials consisting of a single sheet of covalently bonded atoms, and arranged in one to several atomic layers with extended lateral dimensions.1,2 Neighboring layers are held together by weak van der Waals (vdW) forces, allowing chemical or mechanical exfoliation to generate nanosheets from the bulk counterparts.3,4 The discovery of the unprecedented properties of 2D NMs has invoked a keen interest in research and potential commercialization. To date, many 2D materials have been produced, tested, and demonstrated with peculiar applications in electronic, optoelectronic, electrochemical and biomedical fields.5–9 The industrial uses of 2D NMs inevitably depend on appropriate control of exfoliation to maintain the monolayer or few-layer nanosheets dispersed in aqueous media.10In some scenarios, however, the dispersion of the nanosheets would suffer from agglomeration, where the nanosheets are stuck to flocs during the fabrication processes or applications.11,12 For instance, aggregation or restacking of nanosheets occurs when the surface charges are diminished, thereby leading to insufficient repulsive forces that could hold the colloidal stability under aqueous conditions.13 Unwanted aggregation will largely decrease the accessible surface area and density of edge sites, and thus lose important features of 2D nanosheets and severely hinder their practical applications. Therefore, good control of redispersion is expected to recover the exfoliation-mediated features from the undesired aggregation and thus is vital to guarantee the nanoscale units of the materials. In other words, many 2D NMs are self-assembled into 3D networks or artificially restacked to form paper-like membranes, which employ the sub-nanoscaled interlayer spacing for selective separation.14–16 The redispersion properties will determine the stability of 2D-based lamellar membranes in water and thus provide prescreening for their potential application in contaminant removal and desalination.15,17 Overall, a thorough understanding and thereafter good control for the redispersion of nanosheets are warranted.
Previous studies have managed to elucidate the redispersion process by investigating 3D nanoparticles. It is currently well accepted that the redispersion tendency is determined by the interaction between nanoparticles’ surface and solvent.18,19 Therefore, the coating of particle surfaces with ligand species is regarded as a feasible method for controlling the aggregation–redispersion behavior of nanoparticles.20–23 When it comes to 2D nanomaterials, however, the redispersion could be much more complicated. Similar to the 3D nanoparticles, the redispersion properties are related to the surface properties of the nanosheets, a determinant which could be employed to explain the higher tendency of swelling and redispersion of membrane composting of graphene oxide than that of MoS2.15,17 More importantly, unlike the agglomeration of 3D nanoparticles, where macroscopic aggregates were formed, various structures (random aggregates or aligned stacks) could appear from the assembled nanosheets depending on the intersheet contact, which could also affect the redispersion properties of 2D nanosheets. For example, flat graphene sheets turned to nondispersible chunks while remaining readily dispersible if the flakes were deformed into a wrinkled structure or crumbled graphene nanoparticles upon compression.24 The distinct dispersity of MXenes was obtained from 3D assembly architecture compared to plane-to-plane restacking.25 As a consequence, we hypothesized that the redispersion tendency of 2D NMs is a joint outcome of the surface properties and the structure of the aggregates. Actually, the conformation of the aggregates, e.g. macroaggregates with a random orientation or 2D face-to-face aligned stacks, is supposed to determine the extent of the influences posed by surface properties on the redispersion, given that variation in conformations determines the intersheet forces. Unfortunately, the interplay of the assembling structure and surface chemistry and their subsequent effects on the redispersion behavior were barely reported in previous studies to the best of our knowledge. Such a gap is expected to provide a fundamental understanding of colloidal properties and is the precondition for the application development of 2D NMs.
In this study, two most commonly used and well-researched 2D NMs, graphene oxide (GO) and molybdenum disulfide (MoS2), were selected as our objective nanosheets. Briefly, GO is a typical amphiphilic carbonaceous material with hydrophilic oxygen-containing groups on the surface. MoS2 is one of the inorganic 2D NMs belonging to transition metal dichalcogenides. One reason for the two NMs being selected is that they could easily be transformed into their respective derivatives with distinct surface properties, which enables the redispersion investigations as the evolution of surface chemistry. In other words, MoS2 has stronger intermolecular interactions between nanosheets than GO owing to its larger Hamaker constant.26 Differences in vdW between the two NMs promised their distinct response to the intersheet contact, which coupled with the surface properties would result in their different tendency of redispersion. Given such similarities and differences, looking at GO, MoS2 and their derivative nanosheets is expected to contribute to understanding the interplay of surface chemistry and structure as well as their effects on stability and redispersion behavior. Besides the surface states of the nanosheets and solution conditions considered, the intersheet contact (random aggregation and aligned stacking) that is unique to the 2D nature of nanosheets was also cautiously discussed. Finally, molecular simulation of MoS2 proceeded to further unveil the redispersion mechanisms. The results will for the first time systematically uncover the redispersion mechanisms from the macroscopic and molecular perspectives.
Results and discussion
Characterization of the nanosheets, random aggregates and aligned stacks
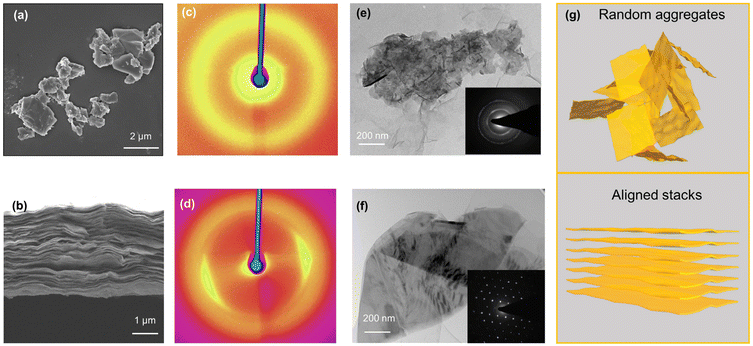
Exfoliated MoS2 and GO nanosheets were subjected to a series of characterization methods as shown in Fig. S1,† which proved the monolayer nature and negatively charged surface of the nanosheets. The monolayer nature of the nanosheets could be seen from the thickness of around 1.1 nm (Fig. S1a and b†), as determined by AFM characterization. The thickness is consistent with the typical thickness in the existing literature and thus has verified the successful acquisition of these two nanosheets in their respective monolayer state. The images of TEM indicated the lateral dimensions of 100–300 nm and 200–300 nm for MoS2 and GO, respectively (Fig. S1c and d†). Both types of nanosheets could be well dispersed in water without any need for surfactants due to the abundant negatively-charged groups on the surface, showing a high zeta potential of −37 to −40 mV at pH around 4. A pH-dependent zeta potential was obtained for these two nanosheets, with a sharp decrease to −20 mV under super acidic conditions due to the protonation of S2− (MoS2) or the carboxyl group (GO) (Fig. S1e and f†).To study the redispersion behavior of the nanosheets as influenced by assembling configurations, an aggregation/alignment-redispersion protocol was proposed and applied (Fig. S2†). Briefly, random aggregates could be formed in the presence of H+/cations, which could destabilize the dispersion through charge screening effects (Fig. S3†), with the ability influenced by the cation's valence, radius, electronegativity, etc.27,28 Aggregates of the nanosheets were created by the addition of 2 mM Ca2+, which was higher than the critical coagulation concentrations required for both dispersions and thus could ensure the full sedimentation of the nanosheets within 24 h (Fig. S4 and S5†). The aggregation under different conditions could be well explained by Derjaguin–Landau–Verwey–Overbeek theory, which describes that the total interaction potential of two colloidal particles is determined by the sum of electric double layer repulsions and vdW attractions.29–31
The random morphology of the MoS2 aggregates without any specific orientation could be well observed from the SEM image (Fig. 1a). For comparison, a different configuration, aligned stacks of the MoS2 nanosheets (face-to-face), was created by vacuum filtration with the aligned lamellar structure confirmed by the cross-section SEM image (Fig. 1b). Fig. 1c and d depict the 2D WAXD diagrams of aggregates and stacks. A Debye ring could be observed in the WAXD patterns of the aggregates, demonstrating a low degree of orientation. For the aligned stacks, the Debye diffractions transformed from rings into symmetric diffraction spots, indicating that the nanosheets were preferentially oriented during restacking.32 The disordered polycrystalline feature of the aggregates and the near perfect planar structure of stacked MoS2 could also be seen from the well-defined “diffraction ring” and diffraction spots, respectively, in the selected area electron diffraction (SAED) patterns (Fig. 1e and f). The characterization results suggested that aggregates and alignments with contrasting morphologies (Fig. 1g) were successfully obtained by our protocol. Characterization of the random aggregates and aligned stacks of GO are provided in the ESI (Fig. S6†).
Redispersion of the random aggregates and aligned stacks
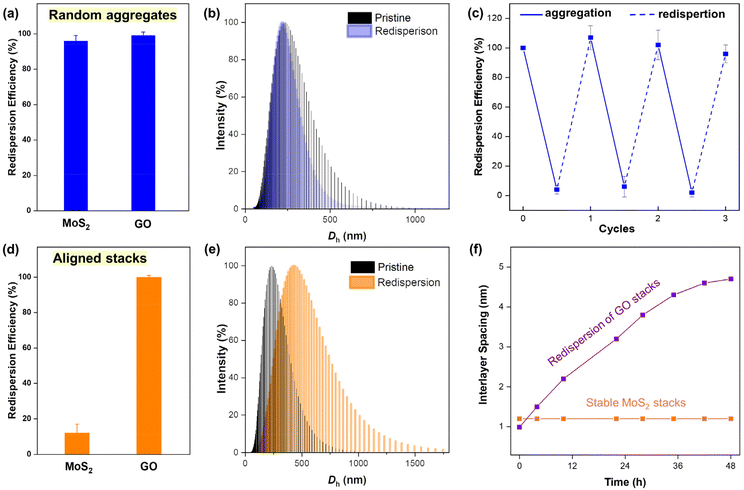
To explore the redispersion behavior of 2D NMs as influenced by attaching morphologies, the redispersion process was initiated by mild ultrasonication in the water bath. The absorbance of the redispersion was compared to that of its original dispersion to quantify the redispersion efficiency, given the good linear relationship between the concentration of nanosheets and their absorbance (Fig. S7†). After 30 min of ultrasonication, the nanosheets of both MoS2 and GO random aggregates could be well recovered with a redispersion efficiency of more than 96% and kept almost unchanged after 24 h of settling (Fig. 2a). Moreover, the size distribution of the MoS2 redispersion was highly identical to pristine nanosheets (Fig. 2b). The results indicated that random aggregates of both MoS2 and GO could be fully redispersed and the redispersion displayed good colloidal stability. The dispersible feature of the random aggregates could be further confirmed under the conditions where the aggregation was induced by other cations or H+. For example, when excessive OH− was added to neutralize H+, the absorbance returns to its initial intensity, indicating that the H+-induced aggregates could be redispersed in an alkaline aqueous solution. The process can be repeated several times (Fig. 2c), corresponding to the reversible aggregation and redispersion of the MoS2 nanosheets in random aggregates.In the case of the aligned stacks, GO could still be fully redispersed in water, whereas the redispersion efficiency for MoS2 was surprisingly low, with a recovery of less than 20% (Fig. 2d). The redispersion from different attaching morphologies of MoS2 and GO was as illustrated in the images of original dispersion, and redispersions from random aggregates and aligned stacks (Fig. S8†). After standing for 24 h, the size of the MoS2 supernatant was obviously higher than the pristine dispersion (Fig. 2e). The results indicated that the MoS2 nanosheets cannot be redispersed from their aligned stacks by mild sonication, and that the recovered absorbance mainly came from the contribution of the fewer-layer nanoflakes in the suspension. Moreover, the redispersion efficiencies of aligned MoS2 were 1%, 29% and 54% from the stacks with thicknesses of 100, 300 and 500 nm, respectively (Fig. S9a†). The increasing redispersion should have resulted from the elevated randomness as the growth of thickness, which could be evidenced by the broadening peak in the XRD patterns (Fig. S9b and c†). The results further confirmed that the redispersion behavior of 2D nanosheets is dependent on the arranged manner or intersheet contact of the nanosheets.
With respect to the contrasting redispersion of the aligned stacks of MoS2 and GO, the distinct redispersion properties from their aligned stacks are probably ascribed to their different surface properties and thus vdW and hydration forces between layers. Comparatively, the vdW force between GO layers is much lower than that of MoS2, making a stronger repulsive force required to redisperse MoS2 nanosheets. Moreover, the hydrophilic functional groups on the GO surface render a high hydration force, which could overcome the attraction for redispersion. The distinct redispersion properties could provide some explanations for the phenomenon that the layer-stacked MoS2 membrane was highly aqueous stable, whereas the GO membrane was going through increased interlayer spacing due to the redispersion upon readily swelling in water (Fig. 2f), which was consistent with the previous report.15 The results suggested that in addition to the interlayer contact, the redispersion of nanosheets is also influenced by the properties of the NMs. Therefore, the study regarding the interplay of surface chemistry and interlayer contact on the redispersion is warranted.
Effects of surface modification on the redispersion efficiency
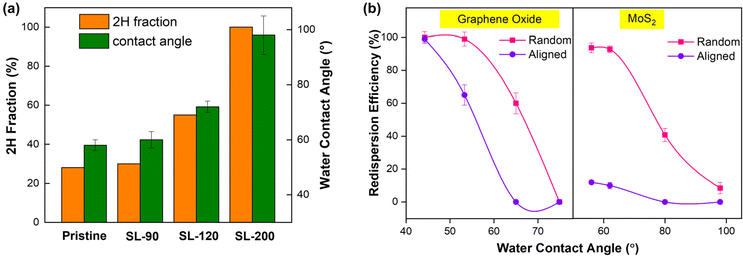
In order to investigate the effects of surface properties on the redispersion of 2D NMs in the two different conformations mentioned above, the nanosheet surface was modified by transforming it into their respective derivatives. Specifically, MoS2 was subjected to phase transformation through a hydrothermal reaction, during which the hydrophilic 1T phase was gradually converted to the hydrophobic 2H phase. Based on the XPS deconvolution results (Fig. S10a†), the 2H components gradually increased from 28% in the as-exfoliated MoS2 nanosheets to 46% at 120 °C and complete phase conversion was achieved at 200 °C. The water contact angles were 58°, 60°, 72° and 98° in SL-pristine, −90, −120 and −200, respectively (Fig. 3a), implying the conversion from a hydrophilic to a hydrophobic nanosheet surface. The losses of the hydrophilicity during transformation resulted from the losses of the 1T phase, which is consistent with a previous study.33 As expected, none of the aligned stacks formed by the pristine or transformed MoS2 could be redispersed in water (Fig. 3b). For the random aggregates, similar redispersion was obtained from SL-90 as pristine MoS2, as little phase transformation has occurred within this condition. However, a more pronounced drop from 99% to 50% in the recovery efficiency was obtained from SL-120, and further to absolutely non-dispersible for SL-200 (Fig. 3b).Similarly, the surface modification of GO was achieved through a chemical reduction reaction, during which hydrophilic groups on the surface were partly removed and the aromatic C–C/C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) C was restored. The reduction extent could be well characterized by XPS spectra, showing that the fraction of C–C/C
C was restored. The reduction extent could be well characterized by XPS spectra, showing that the fraction of C–C/C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) C gradually increased from 46.4% in pristine GO to 48.0%, 53.8% and 57.1% in rGO-0.5, −1.0 and 1.5, respectively (Fig. S10b†). The evolution from GO to rGO could be intuitively observed by a change in the dispersion color from brown to dark, with the absorbance at 330 nm increased from 0.5 to 1.2 at a concentration of 100 mg L−1 (Fig. S11a and b†). The as-obtained rGO could still be well dispersed in water, and the water contact angle was increased from 45° to 88° (Fig. S11c†), indicating that the hydrophilic nature of GO was gradually transforming into hydrophobic during reduction due to the increased fraction of C–C/C
C gradually increased from 46.4% in pristine GO to 48.0%, 53.8% and 57.1% in rGO-0.5, −1.0 and 1.5, respectively (Fig. S10b†). The evolution from GO to rGO could be intuitively observed by a change in the dispersion color from brown to dark, with the absorbance at 330 nm increased from 0.5 to 1.2 at a concentration of 100 mg L−1 (Fig. S11a and b†). The as-obtained rGO could still be well dispersed in water, and the water contact angle was increased from 45° to 88° (Fig. S11c†), indicating that the hydrophilic nature of GO was gradually transforming into hydrophobic during reduction due to the increased fraction of C–C/C![[double bond, length as m-dash]](https://www.rsc.org/images/entities/char_e001.gif) C. After filtration, the peak in the XRD pattern has shifted to the right, corresponding to the decreased interlayer spacing from 0.99 nm (GO) to 0.87 nm (rGO-1.5) of the aligned stacks (Fig. S11d†), which was ascribed to the loss of oxygenated functional groups and interlamellar water layers.32,34 For the redispersion of GO and rGO as a function of the water contact angle after surface modification, we note that there is a large gap between the random and aligned configurations (Fig. 3b). Specifically, the random aggregates of rGO-0.5 could be almost fully redispersed, whereas the redispersion efficiency of aligned stacks decreased dramatically to around 70%. A further reduction treatment leads to the stacks that cannot be redispersed, and the redispersion extents of random aggregates gradually decreased to 50% (rGO-1.0) and finally to non-dispersible (rGO-1.5). Collectively, the results suggested that the redispersion of the nanosheets in water was directly related to the hydrophilicity of the material, i.e. the higher hydrophilicity gives rise to better redispersity, which is consistent with the reported redispersion of 3D nanoparticles.35 Notably, a more dramatic drop of redispersion as the reduced hydrophilicity was obtained from the aligned stacks than the random aggregates, highlighting the interplay role of surface properties and interacting configurations on the redispersion of 2D NMs.
C. After filtration, the peak in the XRD pattern has shifted to the right, corresponding to the decreased interlayer spacing from 0.99 nm (GO) to 0.87 nm (rGO-1.5) of the aligned stacks (Fig. S11d†), which was ascribed to the loss of oxygenated functional groups and interlamellar water layers.32,34 For the redispersion of GO and rGO as a function of the water contact angle after surface modification, we note that there is a large gap between the random and aligned configurations (Fig. 3b). Specifically, the random aggregates of rGO-0.5 could be almost fully redispersed, whereas the redispersion efficiency of aligned stacks decreased dramatically to around 70%. A further reduction treatment leads to the stacks that cannot be redispersed, and the redispersion extents of random aggregates gradually decreased to 50% (rGO-1.0) and finally to non-dispersible (rGO-1.5). Collectively, the results suggested that the redispersion of the nanosheets in water was directly related to the hydrophilicity of the material, i.e. the higher hydrophilicity gives rise to better redispersity, which is consistent with the reported redispersion of 3D nanoparticles.35 Notably, a more dramatic drop of redispersion as the reduced hydrophilicity was obtained from the aligned stacks than the random aggregates, highlighting the interplay role of surface properties and interacting configurations on the redispersion of 2D NMs.
Molecular dynamics simulation for the aggregation and redispersion
To further understand the redispersion behavior of 2D materials, the potential of mean forces (PMFs) calculation was conducted using classical molecular dynamics (MD) simulations, which is a promising tool to investigate the thermodynamic stability of the dispersion.36–41 We computed the PMF of two separate MoS2 single layers in aggregation processes as a function of interlayer spacing (Fig. 4a). In the models, the 2H and 1T phases of the MoS2 monolayer were used to explore the influence of surface properties, which share the same chemical composition but exhibit different surface hydrophilicity. The spring constant and pulling speed were set as 2500 kcal mol−1 Å−2 and 0.000001 Å fs−1, respectively (Fig. S12†). The MD snapshots (Fig. S13†) and movies (Movies S1 and S2†) are generated using the VMD program.42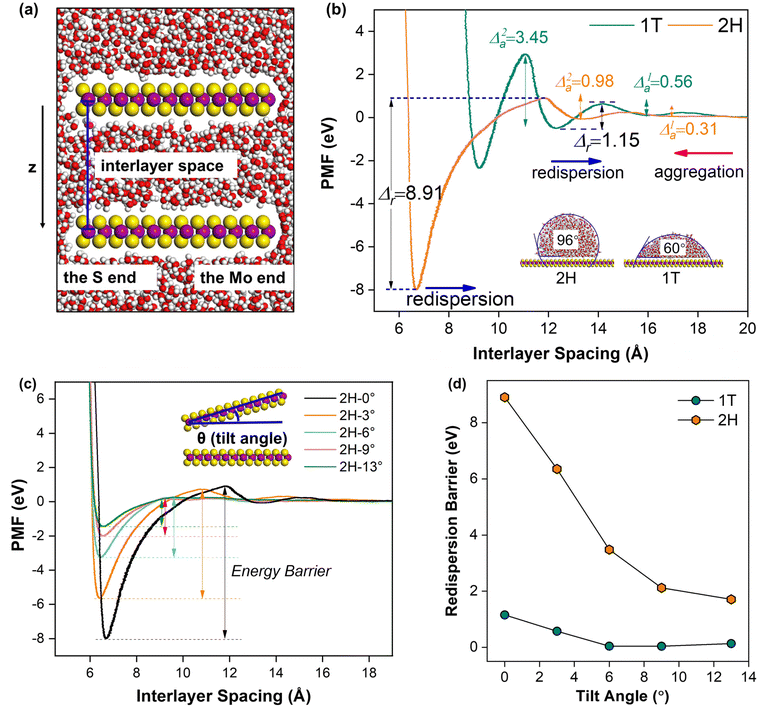 | ||
| Fig. 4 PMF simulations for 1T and 2H phases of MoS2 tilted at the S end: (a) the atomic structure of the initial model used in MD simulations for 2H–MoS2 nanosheets in water solution, in which the interlayer spacing is defined as the distance between the Mo atoms in the two layers; (b) the PMF profiles of two parallel 2H (orange) and 1T (cyan) phases of MoS2 nanosheets as a function of the interlayer spacing. Insets present the contact angles (θ) of water on 1T and 2H MoS2 calculated from MD trajectories using the approach proposed by Caǧin et al.;43 (c) PMF profiles of 2H MoS2 at various tilt angles (inset: the schematic illustration of the tilt angle between the two 2H MoS2 layers) and (d) the redispersion barrier changes with respect to the tilt angles for 1T and 2H phases. Color codes: S, yellow; Mo, rose red; H, white; and O, red. | ||
We first simulated the 2H and 1T phase MoS2 with water contact angles of 96° and 60° (inset images in Fig. 4b), respectively, consistent with experimental results and a previous report.33 That lends credibility to our computational methods and force field parameters used here. As shown in Fig. 4b, during the course of aggregation processes, two local minimum states were found for both the 2H and 1T phases. For example, the 1T phase can be stabilized at distances of 12.3 and 9.2 Å. However, very different barriers are involved for far-away separated 1T MoS2 layers to reach these two states. The aggregation from 20 to 12.3 Å involves a barrier of 0.56 eV (Δ1a) that is easy to surmount, whereas further approaching of these two layers to reach the 9.2 Å state requires overcoming a much higher barrier of 3.45 eV (Δ2a). This indicates that the 1T MoS2 nanosheets most possibly exhibit an interlayer distance of ∼12 Å when restacking in solutions. Compared to the 1T phase, the two barriers involved in the aggregation process of the 2H phase are both smaller than 1.00 eV (0.31 eV and 0.98 eV, respectively), and thus 2H MoS2 nanosheets are predicted with an interlayer spacing of 6.4 Å. The distinct interlayer spacing of restacked 1T and 2H MoS2 is in line with the experimental observation (Fig. S14†), and suggests that the true kinetics of the redispersion process (blue arrows) should be reflected by the first free-energy barrier in the PMF of 2H MoS2 (from 6.4 to 13.0 Å) and the second in the PMF of the 1T phase (from 12.3 to 20.0 Å), respectively, which are noted as the redispersion barrier (Δr). As shown in Fig. 4b, the redispersion barrier for the 2H phase is 8.91 eV, much higher than that for the 1T phase (1.15 eV). The result agrees well with the experimental observation and indicates that the more hydrophobic 2H phase exhibits weaker redispersibility due to a larger energy barrier required for redispersion compared to the more hydrophilic 1T phase.
We should note that the PMFs as influenced by hydrophilicity were computed with a parallel-aligned configuration. To model the situation where MoS2 nanosheets are randomly oriented with various angles in the aggregates, the upper layer of MoS2 was tilted (Fig. 4c) and variation of the redispersion barrier was simulated by computing the PMFs with respect to the tilt angle using the same method stated previously (Movies S3 and S4†). The complete PMF plots for 2H and 1T are displayed in Fig. 4c and Fig. S15,† respectively. It clearly shows that the redispersion barrier decreases with the increasing tilt angle for both 2H and 1T phases due to the weakened interlayer interactions. We note that there is a great drop in the redispersion barrier when the interlayer angle increases from 0° to 6°, which could well explain the much higher redispersion efficiency of the random aggregates compared to the aligned stacks (Fig. 4d). More importantly, despite the same trend, the 2H phase always entails a much larger redispersion barrier than that of the 1T phase, suggesting the weaker redispersibility of the 2H phase irrespective of the contact configuration. Similar trends of redispersion along with the tilt angle were obtained from the model tilted at the Mo end (Fig. S16†). Overall, our simulation results confirmed that the redispersibility of MoS2 nanosheets is positively correlated with the surface hydrophilic property and the interlayer angle. The result is in good agreement with the experimental observation (Fig. 3b) and theoretically demonstrates the unique redispersion behavior of 2D nanosheets, which is synergistically determined by the surface properties and interlayer interactions.
Mechanisms and implications
In principle, redispersion occurs when the net of the surface forces (vdW attractions and hydration repulsions) became even or repulsive at the interlayer spacing set by the water layer.20,44,45 Our experimental and simulation results found that the redispersion behavior of the 2D nanosheets is dependent on the interplay of intersheet contact and surface hydrophilicity: a more hydrophilic surface would impart a higher hydration force and thus better redispersibity in water; meanwhile, vdW between parallel layers was much higher than the randomly-arranged counterpart, rendering a much higher redispersion barrier in aligned stacks, which would enlarge the influences imparted by surface properties. Therefore, the more dramatic decrease of the redispersion tendency in the aligned configuration as the decreasing hydrophilicity is supposed to be the synergistic results of the lowered hydration forces and intense vdW forces between parallel layers.For the nanosheets investigated, the redispersibility as a function of surface hydrophilicity (water contact angle α) and vdW (Hamaker constant H) in two configurations is summarized in Fig. 5. The Hamaker constants of GO and MoS2 materials were calculated as described in Text S1.† Basically, three zones were identified based on the redispersion of 2D nanomaterials and their location on α-H mapping: Zone I where the NMs possess ultrahigh hydrophilicity and weak vdW, thus being able to be readily redispersed from both random and aligned configurations (e.g., GO; hollow symbols); Zone II where the nanosheets could be much more feasibly recovered from the random aggregates rather than the aligned stacks (e.g., rGO-0.5, rGO-1.0, SL-pristine; half symbols); and Zone III where the nanosheets could hardly be recovered from any configurations (e.g., rGO-1.5, SL-120, SL-200; solid symbols), due to the poor hydrophilicities and high vdW forces.
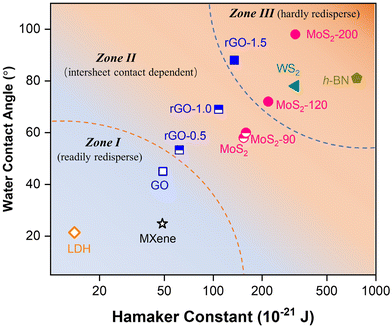 | ||
| Fig. 5 Summary of the nanosheet redispersion as determined by the interplay of the contact configuration and surface properties (i.e., water contact angle and Hamaker constant). | ||
The mapping provides important insights into the applications and implications of 2D nanomaterials. For instance, the materials in Zone I that could be readily redispersed can be stored in dry powder for feasible storage and transportation. In contrast, the ones in Zone III are predicted to exhibit a low tendency of redispersion and thus limited transport once unintentionally released and aggregated in environmental media. Besides, the frame gives a good explanation for the reported stability of GO- and MoS2-based membranes, with increased stability of the GO membrane during GO reduction,46 and superior anti-swelling properties of the MoS2-based membranes.15 Therefore, another implication of the mapping is the prediction for the stability of layer-stacked membranes and thus as a prescreening for suitable 2D nanomaterials for membrane fabrication. By collecting the surface and vdW properties of some commonly researched nanosheets, briefly, their positions on the mapping could be readily addressed for a prediction (Table S1†). For example, based on the location on the map, MXene and layered clay based membranes (e.g., layered double hydroxide, LDH) were predicted to possess a high tendency of redispersion from both its aggregates and restacking, which is consistent with the existing reports that the MXene-based membrane suffers from instability and swelling and thus requires further modification for applications.47,48 In contrast, h-BN and WS2 membranes are expected to possess higher resistance to swelling for their hydrophobic surface and high vdW of the materials.49,50
Conclusions
To the best of our knowledge, this is the first study exploring the redispersion behavior of 2D nanosheets from experimental and theoretical perspectives. Besides the surface chemistry considered, the role of intersheet contact, which is a unique feature of 2D NMs, was particularly discussed. The results demonstrated that the redispersion in response to surface chemistry was regulated by the intersheet contact, i.e. random or aligned configurations: with the decrease of the surface hydrophilicity, the redispersion efficiency decreased and a more dramatic drop was observed in the aligned configuration. The mechanism behind this was further revealed by the potential mean force calculation through molecular dynamics simulation. Finally, three zones of the redispersion tendency were identified based on the intrinsic properties of nanosheets including surface hydrophilicity and Hamaker constant, as well as the dependence on intersheet contacts. The comprehensive study advances our understanding of the redispersion behavior of nanomaterials and provides fundamental information for the applicability of prescreening of 2D nanomaterials.Methods
Preparation and surface modification of the nanosheets
A dispersion of single-layer MoS2 (SL-MoS2) nanosheets was prepared by organolithium intercalation followed by forced hydration as described in our previous studies. The as-prepared nanosheets are mainly in 1T phase.51 A stock dispersion of GO (1000 mg L−1) was purchased from Nanjing XFNANO Materials Tech Co., Ltd, China. To reveal the role of surface functionality of the nanosheets on the redispersion efficiency, the transformation of GO was conducted by reducing GO with L-ascorbic acid (10 mM) at 80 °C for 0.5, 1.0 and 1.5 h to obtain the reduced GO (referred to as rGO-0.5, −1.0 and −1.5, respectively) with different extents of reduction and distribution of surface functionalities. The surface hydrophilicity of MoS2 nanosheets was modified through phase reversion during hydrothermal treatment at 90, 120 and 200 °C (referred to as SL-90, SL-120 and SL-200) for 2 h, where the hydrophilic 1T phase was partly transferred to the hydrophobic 2H phase.Aggregation and redispersion experiments
The random aggregation was induced by the addition of 2 mM Ca2+ into 10 mL of the nanosheet dispersion and agitated for 30 min. Once the dispersion was fully sedimented, the samples were centrifuged at 3000 rpm for 5 min. The aggregates were collected and then redispersed in 10 mL of ultrapure water at pH 10 by ultrasonication in a water bath for 30 min. The aligned stacking of the nanosheets was created using vacuum filtration on a porous polymer substrate (220 nm pore size, Shimadzu, Japan). After the dispersions were fully stacked, the layered film was placed in 10 mL of ultrapure water and ultrasonicated for 30 min. After standing for 24 h, the supernatants of the redispersed solution were subjected to UV-vis absorption measurement (UH5300, Hitachi) at 450 nm and 330 nm for MoS2 (including transformed MoS2) and GO (including rGO), respectively. Given the good linear relationship between the concentration of nanosheets and their absorbance (Fig. S7†), the redispersion efficiency (α) was determined by comparing the absorbance of the dispersion before aggregation/stacking and after redispersion. Once redispersed, the size distribution was recorded by dynamic light scattering (DLS; NanoBrook Omni, Brookhaven) and compared to that of the dispersion before aggregation/stacking.18Characterization
The thickness and lateral size of MoS2 and GO nanosheets were recorded by atomic force microscopy (AFM; MFP-3D-Stand Alone, Asylum Research). The morphology and structure of MoS2 and GO in their respective monolayer aggregated, and aligned states were characterized by transmission electron microscopy (TEM; FEI Talos F200X microscope equipped with a Schottky emitter gun operated at 200 kV) and scanning electron microscopy (SEM; Zeiss Merlin). The 2D wide-angle X-ray diffraction (WAXD) patterns of the MoS2 aggregates and restacked films were recorded using a Rigaku HomeLab diffractometer operated at 45 kV and 200 mA in the reflection mode with Cu Kα radiation (λ = 1.542 Å), a scanning speed of 5 °C min−1 and a step-size of 0.010° over the 2θ range from 3° to 30°. The powder X-ray diffraction (XRD) pattern was collected on a Rigaku SmartLab diffractometer (Cu Kα = 1.54 Å). The hydrodynamic diameter and zeta potential of the dispersion were recorded using a Nanosizer (NanoBrook Omni, Brookhaven). The surface modification of the nanosheets was confirmed and characterized by X-ray photoelectron spectroscopy (XPS; PHI 5000 Versaprobe III equipped with a monochromatic Al anode (Al Kα = 1486.7 eV)). The water contact angles of the nanosheets were measured from the average values for 4 μL water droplets at three different positions of the stacked film (DAS25E, Kruss). The exact concentration of as-prepared MoS2 was determined by inductively coupled plasma optical emission spectroscopy (ICP-OES; iCAP 7400, Thermo Scientific) after complete digestion in 0.2 M HNO3 and 0.5 M H2O2 solution.Molecular dynamics simulation
The steered molecular dynamics (SMD) simulation method implemented in the LAMMPS package52 was used to compute the potential of mean force (PMF) for the separation process of MoS2 bilayers in a solvation environment. All SMD simulations were carried out at a constant temperature of 298 K and a constant pressure of 1 atm (NPT ensemble) with a Nose–Hoover thermostat53–57 and barostat. The timestep was set as 1 fs. During SMD simulations, the center of mass of the upper MoS2 sheet was connected to the bottom fixed one via a virtual spring that drove it to move along the z direction (Fig. 4a). The accumulated potential of PMFs was extracted and averaged over every 1 ps. The MoS2 sheets were held rigid during the simulations. The body forces on each MoS2 sheet along the x and y directions and the body torques were all set to zero to prevent the sheet from sliding and rotating. The spring constant and pulling speed were set as 2500 kcal mol−1 Å−2 and 0.000001 Å fs−1, respectively, to guarantee the accuracy of PMFs (Fig. S12†). The Lennard-Jones potential and Coulomb interactions were introduced to describe the MoS2–H2O interaction. The charge and force field parameters of MoS2 were obtained from the literature58–60 and further adjusted to reproduce the experimental contact angle of the MoS2 sheets. The Lorentz–Berthelot mixing rule was employed in the LJ potential to describe the interaction between dissimilar non-bonded atoms. The SPC/E model61 was applied for H2O–H2O interactions. All structures were stabilized for 400 ps under the NPT ensemble and then 400 ps with the NVT ensemble. Subsequently, the simulation continued for at least 15 ns to compute PMFs. An 11 × 3.8 × 11 nm3 periodic box with 14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 336 water molecules was used to simulate the solution environment. Two separate nanoribbon models with a length of 3 nm along the x-axis were constructed to simulate the approaching MoS2 nanosheets.
336 water molecules was used to simulate the solution environment. Two separate nanoribbon models with a length of 3 nm along the x-axis were constructed to simulate the approaching MoS2 nanosheets.
Associated content
Additional methodology and results can be found in the ESI,† including characterization details, supporting and methodological details for MD simulations, calculation of Hamaker constants, etc.Author contributions
B. L.: experiment conduction, data analysis and writing; J. Z.: MD calculation, formal analysis and writing; Q. H.: characterization and formal analysis; Y. S.: formal analysis and software; L. W.: formal analysis; L. H.: review and editing; L. L.: methodology, writing, review and editing; and Z. W.: conceptualization, writing, review, supervision and funding acquisition. All the authors discussed the results.Conflicts of interest
There are no conflicts of interest to declare.Acknowledgements
This project was financially supported by the Stable Support Plan Program of Shenzhen Natural Science Fund (Grant No. 20200925155303001), the National Natural Science Foundation of China (Grant No. 22179058), the Innovation Team Program of the Department of Education of Guangdong Province (Grant No. 2021KCXTD012), the SUSTech-MIT Joint Center for Mechanical Engineering Education and Research, and the State Environmental Protection Key Laboratory of Integrated Surface Water-Groundwater Pollution Control. The authors acknowledge the assistance of SUSTech Core Research Facilities and computational resources provided by the Center for Computational Science and Engineering at the Southern University of Science and Technology.References
- G. R. Bhimanapati, Z. Lin, V. Meunier, Y. Jung, J. Cha, S. Das, D. Xiao, Y. Son, M. S. Strano, V. R. Cooper, L. Liang, S. G. Louie, E. Ringe, W. Zhou, S. S. Kim, R. R. Naik, B. G. Sumpter, H. Terrones, F. Xia, Y. Wang, J. Zhu, D. Akinwande, N. Alem, J. A. Schuller, R. E. Schaak, M. Terrones and J. A. Robinson, ACS Nano, 2015, 9, 11509–11539 CrossRef CAS PubMed.
- Z. Wang, W. Zhu, Y. Qiu, X. Yi, A. Von Dem Bussche, A. Kane, H. Gao, K. Koski and R. Hurt, Chem. Soc. Rev., 2016, 45, 1750–1780 RSC.
- G. Eda, H. Yamaguchi, D. Voiry, T. Fujita, M. Chen and M. Chhowalla, Nano Lett., 2011, 11, 5111–5116 CrossRef CAS PubMed.
- S. Zhuo, G. Huang, R. Sougrat, J. Guo, N. Wei, L. Shi, R. Li, H. Liang, Y. Shi, Q. Zhang, P. Wang and H. N. Alshareef, ACS Nano, 2022, 16, 3955–3964 CrossRef CAS PubMed.
- A. Midya, A. Ghorai, S. Mukherjee, R. Maiti and S. K. Ray, J. Mater. Chem. A, 2016, 4, 4534–4543 RSC.
- M. A. Lukowski, A. S. Daniel, F. Meng, A. Forticaux, L. Li and S. Jin, J. Am. Chem. Soc., 2013, 135, 10274–10277 CrossRef CAS PubMed.
- T. Liu, C. Wang, X. Gu, H. Gong, L. Cheng, X. Shi, L. Feng, B. Sun and Z. Liu, Adv. Mater., 2014, 26, 3433–3440 CrossRef CAS PubMed.
- D. Lembke, S. Bertolazzi and A. Kis, Acc. Chem. Res., 2015, 48, 100–110 CrossRef CAS PubMed.
- G. Swain, S. Sultana and K. Parida, Nanoscale, 2021, 13, 9908–9944 RSC.
- X. An, T. Simmons, R. Shah, C. Wolfe, K. M. Lewis, M. Washington, S. K. Nayak, S. Talapatra and S. Kar, Nano Lett., 2010, 10, 4295–4301 CrossRef CAS PubMed.
- J. Luo, H. D. Jang and J. Huang, ACS Nano, 2013, 7, 1464–1471 CrossRef CAS PubMed.
- I. Benabdallah, A. Kara and M. Benaissa, Appl. Surf. Sci., 2020, 507, 144826 CrossRef CAS.
- Y. Gao, A. Ahiabu and M. J. Serpe, ACS Appl. Mater. Interfaces, 2014, 6, 13749–13756 CrossRef CAS PubMed.
- S. Zheng, Q. Tu, M. Wang, J. J. Urban and B. Mi, ACS Nano, 2020, 14, 6013–6023 CrossRef CAS PubMed.
- Z. Wang, Q. Tu, S. Zheng, J. J. Urban, S. Li and B. Mi, Nano Lett., 2017, 17, 7289–7298 CrossRef CAS PubMed.
- E. Hoenig, S. E. Strong, M. Wang, J. M. Radhakrishnan, N. J. Zaluzec, J. L. Skinner and C. Liu, Nano Lett., 2020, 20, 7844–7851 CrossRef CAS PubMed.
- C. N. Yeh, K. Raidongia, J. Shao, Q. H. Yang and J. Huang, Nat. Chem., 2015, 7, 166–170 CrossRef CAS PubMed.
- P. Jeevanandam and K. J. Klabunde, Langmuir, 2003, 19, 5491–5495 CrossRef CAS.
- D. Wang and L. Chen, Nano Lett., 2007, 7, 1480–1484 CrossRef CAS PubMed.
- S. Desset, O. Spalla, P. Lixon and B. Cabane, Langmuir, 2001, 17, 6408–6418 CrossRef CAS.
- L. Qi, A. Sehgal, J. C. Castaing, J. P. Chapel, J. Fresnais, J. F. Berret and F. Cousin, ACS Nano, 2008, 2, 879–888 CrossRef CAS PubMed.
- C. Stauch, T. Ballweg, W. Stracke, R. Luxenhofer and K. Mandel, J. Colloid Interface Sci., 2017, 490, 401–409 CrossRef CAS PubMed.
- R. Zirbs, A. Lassenberger, I. Vonderhaid, S. Kurzhals and E. Reimhult, Nanoscale, 2015, 7, 11216–11225 RSC.
- J. Luo, H. D. Jang, T. Sun, L. Xiao, Z. He, A. P. Katsoulidis, M. G. Kanatzidis, J. M. Gibson and J. Huang, ACS Nano, 2011, 5, 8943–8949 CrossRef CAS PubMed.
- L. Xiu, Z. Wang, M. Yu, X. Wu and J. Qiu, ACS Nano, 2018, 12, 8017–8028 CrossRef CAS PubMed.
- X. Lu, U. R. Gabinet, C. L. Ritt, X. Feng, A. Deshmukh, K. Kawabata, M. Kaneda, S. M. Hashmi, C. O. Osuji and M. Elimelech, Environ. Sci. Technol., 2020, 54, 9640–9651 CrossRef CAS PubMed.
- K. Yang, B. Chen, X. Zhu and B. Xing, Environ. Sci. Technol., 2016, 50, 11066–11075 CrossRef CAS PubMed.
- B. Liu, Q. Han, L. Li, S. Zheng, Y. Shu, J. A. Pedersen and Z. Wang, Environ. Sci. Technol., 2021, 55, 16379–16389 CrossRef CAS PubMed.
- E. M. Hotze, T. Phenrat and G. V. Lowry, J. Environ. Qual., 2010, 39, 1909 CrossRef CAS PubMed.
- M. Baalousha, NanoImpact, 2017, 6, 55–68 CrossRef.
- B. Liu, Z. Han, Q. Han, Y. Shu, M. Wang, L. Wang, Z. Wang and J. A. Pedersen, Environ. Sci. Nano, 2022, 9, 1605–1616 RSC.
- I. Jung, M. Vaupel, M. Pelton, R. Pinery, D. A. Dikin, S. Stankovich, J. An and R. S. Ruoff, J. Phys. Chem. C, 2008, 112, 8499–8506 CrossRef CAS.
- L. Ries, E. Petit, T. Michel, C. C. Diogo, C. Gervais, C. Salameh, M. Bechelany, S. Balme, P. Miele, N. Onofrio and D. Voiry, Nat. Mater., 2019, 18, 1112–1117 CrossRef CAS PubMed.
- X. Lin, X. Shen, Q. Zheng, N. Yousefi, L. Ye, Y. W. Mai and J. K. Kim, ACS Nano, 2012, 6, 10708–10719 CrossRef CAS PubMed.
- A. M. Freitas and M. M. Sharma, Langmuir, 1999, 15, 2466–2476 CrossRef CAS.
- C.-J. Shih, S. Lin, M. S. Strano and D. Blankschtein, J. Am. Chem. Soc., 2010, 132, 14638–14648 CrossRef CAS PubMed.
- R. Qiao, A. P. Roberts, A. S. Mount, S. J. Klaine and P. C. Ke, Nano Lett., 2007, 7, 614–619 CrossRef CAS PubMed.
- Z. Xue, Q. Sun, L. Zhang, Z. Kang, L. Liang, Q. Wang and J. W. Shen, Nanoscale, 2019, 11, 4503–4514 RSC.
- C. Hoffmann, A. Centi, R. Menichetti and T. Bereau, Sci. Data, 2020, 7, 1–7 CrossRef PubMed.
- V. Losasso, Y. W. Hsiao, F. Martelli, M. D. Winn and J. Crain, Phys. Rev. Lett., 2019, 122, 208103 CrossRef CAS PubMed.
- J. Lee and W. Im, Phys. Rev. Lett., 2008, 100, 1–4 Search PubMed.
- W. Humphrey, A. Dalke and K. Schulten, J. Mol. Graphics, 1996, 14, 33–38 CrossRef CAS PubMed.
- C. F. Fan and T. Caǧin, J. Chem. Phys., 1995, 103, 9053–9061 CrossRef CAS.
- S. Desset, O. Spalla and B. Cabane, Langmuir, 2000, 16, 10495–10508 CrossRef CAS.
- M. J. Grimson and G. Rickayzen, Chem. Phys. Lett., 1982, 86, 59–65 CrossRef.
- Z. Han, L. Huang, H. Qu, Y. Wang, Z. Zhang, Q. Rong, Z. Sang, Y. Wang, M. J. Kipper and J. Tang, J. Mater. Sci., 2021, 56, 9545–9574 CrossRef CAS.
- X. Xie, Z. Wu and N. Zhang, Chin. Chem. Lett., 2020, 31, 1014–1017 CrossRef CAS.
- Z. Lu, Y. Wei, J. Deng, L. Ding, Z. K. Li and H. Wang, ACS Nano, 2019, 13, 10535–10544 CrossRef CAS PubMed.
- R. Das, P. Solís-Fernández, D. Breite, A. Prager, A. Lotnyk, A. Schulze and H. Ago, Chem. Eng. J., 2021, 420, 127721 CrossRef CAS.
- P. Cheng, Y. Chen, Y.-H. Gu, X. Yan and W.-Z. Lang, J. Membr. Sci., 2019, 591, 117308 CrossRef CAS.
- Z. Wang, A. Von Dem Bussche, Y. Qiu, T. M. Valentin, K. Gion, A. B. Kane and R. H. Hurt, Environ. Sci. Technol., 2016, 50, 7208–7217 CrossRef CAS PubMed.
- S. Plimpton, J. Comput. Phys., 1995, 117, 1–19 CrossRef CAS.
- W. G. Hoover, Phys. Rev. A, 1985, 31, 1695–1697 CrossRef PubMed.
- S. Nosé, Mol. Phys., 2002, 100, 191–198 CrossRef.
- W. Shinoda, M. Shiga and M. Mikami, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 69, 134103 CrossRef.
- G. J. Martyna, D. J. Tobias and M. L. Klein, J. Chem. Phys., 1994, 101, 4177–4189 CrossRef CAS.
- M. Parrinello and A. Rahman, J. Appl. Phys., 1981, 52, 7182–7190 CrossRef CAS.
- Y. Morita, T. Onodera, A. Suzuki, R. Sahnoun, M. Koyama, H. Tsuboi, N. Hatakeyama, A. Endou, H. Takaba, M. Kubo, C. A. Del Carpio, T. Shin-yoshi, N. Nishino, A. Suzuki and A. Miyamoto, Appl. Surf. Sci., 2008, 254, 7618–7621 CrossRef CAS.
- B. Luan and R. Zhou, Appl. Phys. Lett., 2016, 108, 131601 CrossRef.
- U. Becker, K. M. Rosso, R. Weaver, M. Warren and M. F. Hochella, Geochim. Cosmochim. Acta, 2003, 67, 923–934 CrossRef CAS.
- J. Grigera, H. J. C. Berendsen and T. P. Straatsma, J. Phys. Chem., 1987, 91, 6269–6271 CrossRef.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d2nr05471j |
| ‡ These authors contributed equally to this work. |
| This journal is © The Royal Society of Chemistry 2023 |