Excited state energy landscape of phosphorescent group 14 complexes

Abstract

Great progress has been achieved on phosphorescent or photoactive complexes of the Earth-abundant transition metals, while examples for phosphorescent heavy main group element complexes are rare, in particular for group 14 complexes in the oxidation state +II. The known compounds often show only weak phosphorescence with fast non-radiative deactivation. The underlying photophysical processes and the nature of the phosphorescent electronic states have remained essentially unexplored. The present combined photophysical and theoretical study on tin(II) and lead(II) complexes E(bpep) with the dianionic tridentate ligand bpep²⁻ (E = Sn, Pb; H₂bpep = 2-[1,1-bis(1H-pyrrol-2-yl)ethyl]pyridine) provides unprecedented insight in the excited state energy landscape of tetrel(II) complexes. The tin complex shows green intraligand charge transfer (ILCT) phosphorescence both in solution and in the solid state. In spite of its larger heavy-atom effect, the lead complex only shows very weak red phosphorescence from a strongly distorted ligand-to-metal charge transfer (LMCT) state at low temperatures in the solid state. Detailed (TD-)DFT calculations explain these observations and delineate the major path of non-radiative deactivation via distorted LMCT states. These novel insights provide rational design principles for tetrel(II) complexes with long-lived phosphorescence.

---

1 Introduction

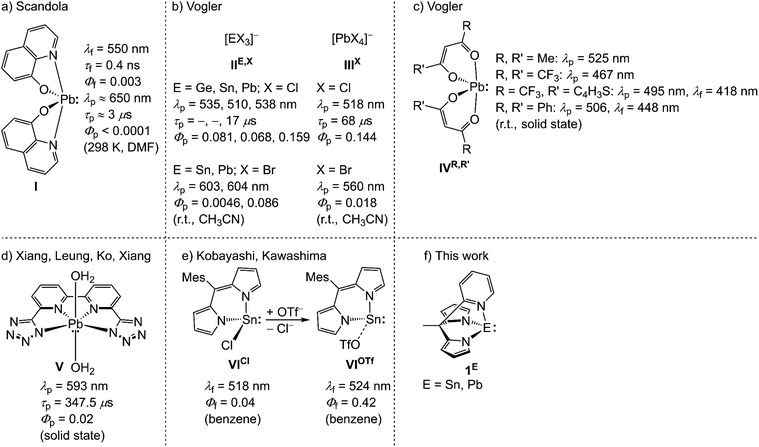
Photoluminescent, in particular phosphorescent and photoactive transition metal complexes (TMCs) of the Earth-abundant elements, mainly 3d elements, evolved to a potential alternative to the precious elements, e.g. ruthenium, iridium or platinum.^1–5 The key prerequisite for applications in bimolecular photochemical reactions is a sufficiently long lifetime of the photoactive excited state (ES). This can be achieved for ESs with different spin multiplicities to the ground state (GS), rendering non-radiative and radiative processes between ES and GS spin-forbidden, thus extending the ES lifetime.⁶ In order to access ESs of different spin multiplicity to the GS and the initially populated Franck–Condon state, intersystem crossing (ISC) must be effective. ISC rates increase for ES's wavefunctions with high metal character invoking spin–orbit coupling (SOC) by a heavy-atom effect or by effective spin–vibronic coupling.⁷ The research on photoactive 3d or 4d TMCs envisaged mainly weakly distorted metal-to-ligand (MLCT) or ligand-to-metal charge transfer (LMCT) and intraconfigurational spin-flip states as potential photoactive states to invoke a high metal character for fast ISC.^1–3 In pseudo-octahedral 3dⁿ TMCs (n = 1–9) the high density of low-lying strongly distorted metal-centered (MC) states typically enables fast non-radiative deactivation of CT states.^1–3 For photoactive pseudo-tetrahedral d¹⁰ copper(I) complexes, the flattening distortion of the photoluminescent ³MLCT states, can facilitate fast non-radiative deactivation.^8–10 The photoluminescence lifetimes of pseudo-tetrahedral copper(I) complexes are also very sensitive to coordinating solvents and anions in the flattened ³MLCT states.^9,11 This illustrates the interplay between ES ordering and ES distortion, which depends on the coordination geometry and rigidification of the complex. For 3d TMCs, the underlying photophysical processes are well understood and ground-breaking progress was achieved in the last few years.^1–5

Examples of heavy main group complexes, mainly group 14 and 15, showing beside conventional fluorescence,^12,13 phosphorescence and/or thermally activated delayed fluorescence (TADF),^10,14–16 are rare.^17–19 Emission is often limited to frozen solutions or even to the solid state, precluding bimolecular reactivity.^13,17–19 Complexes of group 15 elements, in particular bismuth(III) with 6s² electron configuration, can show phosphorescence.^20–28 The oxidation state +IV dominates in tetrel group 14 complexes with potentially photoactive triplet states^29–35 and fluorescent or photoluminescent states of yet unknown character.^36–44 Photoluminescent tetrel(II) complexes are very scarce. Scandola and co-workers reported for Pb(QO)₂I (QOH = 8-hydroxyquinoline) a weak green fluorescence and an even weaker red phosphorescence from intraligand (IL) states in N,N-dimethylformamide (DMF, 298 K; λ_f = 550 nm, τ_f = 0.4 ns, Φ_f ≈ 0.003; λ_p ≈ 650 nm, τ_p ≈ 3 μs, Φ_p < 0.0001) and glassy ethanol (77 K; λ_f = 510 nm, τ_f < 20 ns; λ_p ≈ 610 nm, τ_p = 180 μs), respectively (Scheme 1a).⁴⁵ The triplet state of the Pb complex I is efficiently quenched by energy transfer (EnT) in a bimolecular reaction with [Cr(CN)₆]³⁻ in DMF with a quenching rate constant of k_q = 3.7 × 10⁸ M⁻¹ s⁻¹.⁴⁵ Studies by Vogler and co-workers on photoluminescent halido germanium(II), tin(II) and lead(II) complexes [EX₃]⁻II^E,X (E = Ge–Pb: X = Cl; E = Sn, Pb: X = Br) and [PbX₄]²⁻III^X (X = Cl, Br) revealed phosphorescence from MC states at 510–604 nm after excitation with UV light in fluid acetonitrile solution (Scheme 1b).^46,47 The population of the emissive triplet state with a s¹p¹ electron configuration, is accompanied with large structural reorganization from C_3v to D_3h symmetry for [EX₃]⁻ ions.⁴⁶ This distortion enables efficient non-radiative deactivation of the triplet state. In the series [EX₃]⁻ (E = Ge–Pb, X = Cl, Br), the Sn complexes exhibit short photoluminescence lifetimes below the detection limit and the lowest quantum yields (Φ_p = 0.068 (Cl); Φ_p = 0.0046 (Br)) compared to Ge (Φ_p = 0.081 (Cl)) and Pb (τ_p = 17 μs, Φ_p = 0.159 (Cl); Φ_p = 0.086 (Br)).^46,47 Despite the short photoluminescence lifetime, [SnCl₃]⁻ undergoes bimolecular photo-oxidation reactions to SnOCl₂ with oxygen after excitation with UV-C light (λ_ex = 254 nm).⁴⁸

Scheme 1 Photoluminescent tetrel(II) complexes with selected photophysical data.

Bis(β-diketonato) lead(II) complexes IV^R,R′ prepared by Vogler and co-workers show blue to green intraligand (IL) phosphorescence in the solid state. The phosphorescence is diminished in ethanol solution at r.t. The respective hexafluoroacetylacetonato tin(II) complex is not photoluminescent (Scheme 1c).^27,49 The very weak phosphorescence of the β-diketonato lead(II) complexes is attributed to a large geometric reorganization leading to fast non-radiative relaxation.⁴⁹ Unfortunately, no photoluminescence lifetimes and quantum yields were reported for these compounds. A series of coordination polymers of lead(II) with polydentate tetrazolato ligands are phosphorescent from ³IL, ³MC and ³MLCT states with admixed ³XLCT (halide-to-ligand CT) character. The photoluminescence lifetimes amount up to τ_p = 1.55 ms and a maximum quantum yield of Φ_p = 0.165 in the solid state.⁵⁰ The molecular congener V with 6,6′-bis(1H-tetrazol-5-yl)-2,2′-bipyridine as proligand shows ³MLCT phosphorescence with significantly lower photoluminescence quantum yield in the solid state (λ_p = 593 nm, τ_p = 347.5 μs, Φ_p = 0.02; Scheme 1d).⁵⁰ Substituting a chlorido ligand with a weakly coordinating triflato (OTf⁻) ligand, increases the fluorescence quantum yield of a dipyrromethene tin(II) complex from 0.04 (VI^Cl) to 0.42 (VI^OTf) with a slight bathochromic shift of the fluorescence from λ_f = 518 nm to λ_f = 524 nm (Scheme 1e).⁵¹ The significant increase in quantum yield is ascribed on the basis of an inspection of the GS molecular orbitals from density functional theory (DFT) calculations to a shift of a non-photoluminescent MLCT state to higher energies upon stabilising the occupied non-bonding tin-centered orbital after Cl⁻/OTf⁻ exchange.⁵¹

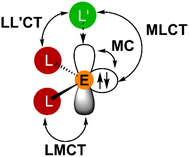
Heavy tetrel(II) complexes are related to tetrylenes, the heavier carbene homologues.^52–56 The singlet ground state of heavier tetrylenes is described by a metal-centered occupied orbital with high s-character and a vacant or donor stabilised orbital with high p-character (Fig. 1).^52–56 Clearly, MC, IL, LMCT, MLCT and ligand-to-ligand charge transfer (LL'CT) states with their respective ES distortions must be considered in exploring the energy landscape of electronic states with the focus on photoluminescent and in particular phosphorescent properties (Fig. 1).

Fig. 1 Schematic representation of selected electronic transitions in donor (L′) stabilised homoleptic tetrylenes EL₂ (E = Si, Ge, Sn, Pb), excluding IL and other possible LMCT (L′ → E) and MLCT (E → L) transitions.

To the best of our knowledge, no combined experimental and quantum chemical studies are reported on the energy ordering and geometric distortions of excited states of photoluminescent tetrel(II) complexes. This study aims to shed more light on the underlying photophysical processes. The tripodal ligand bpep²⁻ (H₂bpep = 2-[1,1-bis(1H-pyrrol-2-yl)ethyl]pyridine)⁵⁷ with electron-rich pyrrolato and electron-poor pyridine donors in combination with the heaviest tetrels Sn and Pb for a large heavy-atom effect and fast ISC was chosen for this initial study (SOC constants: ζ(Sn) = 1855 cm⁻¹, ζ(Pb) = 5089 cm⁻¹).⁵⁸ Sn^II(bpep) 1^Sn shows phosphorescence in the solid state and in solution, while the lead congener Pb^II(bpep) 1^Pb with its larger heavy-atom effect is only very weakly photoluminescent. The elucidation of the different emission properties is supported by UV/vis absorption and variable-temperature steady-state and time-resolved emission spectroscopy as well as with detailed time-dependent (TD) DFT calculations.

2 Results and discussion

2.1. Synthesis, structural and spectroscopic properties

The tetrylenes E^II(bpep) (E = Sn 1^Sn, Pb 1^Pb) are synthesised via a transamination of H₂bpep⁵⁷ with bis[bis(trimethylsilyl)amido]tetrel(II) compounds E^II[N(SiMe₃)₂]₂ (ref. 59) in dichloromethane as colourless, moisture sensitive solids in 74% and 63% yields, respectively (Scheme 2). Complexes 1^E are poorly soluble in most organic solvents. 1^Sn is well soluble in tetrahydrofuran (THF) and sparsely in toluene, while 1^Pb is only soluble in dimethylsulfoxide (DMSO) among the tested solvents. 1^Sn and 1^Pb are fully characterised by elemental analysis, ESI⁺ mass spectrometry (ESI, Fig. S1 and S2†) and multinuclear (¹H, ¹³C, ¹¹⁹Sn, ²⁰⁷Pb) NMR spectroscopy (ESI, Fig. S3–S14†). The ¹¹⁹Sn resonance of 1^Sn appears in the similar chemical shift range of other donor stabilised dipyrrolato stannylenes (ESI, Fig. S5†).⁶⁰ The solids of 1^E are free of coordinating solvents, which could coordinate to the Sn or Pb atoms, as determined by ¹H NMR spectroscopy and elemental analyses. This speciation is important for the discussion of the photoluminescent properties in solution and in the solid state (ESI, Fig. S3 and S9†).

Scheme 2 Syntheses of stannylene and plumbylene E^II(bpep) (E = Sn 1^Sn, Pb 1^Pb) via transamination from E[N(SiMe₃)₂]₂ and H₂bpep, yields given in parentheses.

Crystals of 1^Sn, suitable for X-ray diffraction analyses were obtained from hot toluene. 1^Sn crystallises in the trigonal space group R₃ with two independent molecules 1^Sn,A and 1^Sn,B in the asymmetric unit (Fig. 2a; ESI, Fig. S15 and Table S1†). 1^Sn,B is disordered with a pyrrolato/pyridine site occupation, so that the more precise structural parameters of 1^Sn,A are discussed. Both molecules feature a distorted trigonal pyramidal coordination of the Sn atom. A further solid state structure of 1^Sn could be determined from crystals, obtained from slow diethylether diffusion to a solution of 1^Sn in a 1 : 1 mixture of 1,4-dioxane : tetrahydrofuran. Here, 1^Sn forms a centrosymmetric 1,4-dioxane (diox) bridged dimer (1^Sn)₂(diox) (monoclinic space group I2/a) leading to a four-coordinate tin(II) ion (Fig. 2b; ESI, Table S1†). This solid state structure indicates that 1^Sn will likely form solvates in THF and THF/1,4-dioxane solutions. The 1,4-dioxane coordination with a Sn–O distance of 2.547(2) Å elongates the tin–nitrogen(pyridine) (Sn–N^py) bond from 2.317(5) Å (1^Sn) to 2.375(2) Å ((1^Sn)₂(diox)). This Sn–N^py bond weakening arises from the competing interactions of the pyridine and 1,4-dioxane donors with the tetrel p-type orbital (Fig. 1; ESI Table S1†). This will also influence the photophysical properties of 1^Sn (vide infra). The much lower solubility of 1^Pb in most organic solvents prohibited growing single crystals suitable for X-ray diffraction analysis, but the NMR spectroscopic, mass spectrometric and analytical data confirm the composition and purity.

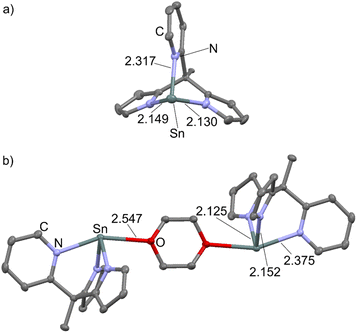Fig. 2 Molecular structures of (a) 1^Sn and (b) of the 1,4-dioxane bridged dimer (1^Sn)₂(diox) with selected structural parameters in Å. H atoms omitted, thermal ellipsoids at 50% probability level.

The structures of 1^Sn, 1^Sn(thf) and 1^Pb were optimised by DFT (CPCM-(THF)-RIJCOSX-B3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn)/SARC-ZORA-TZVPP(Pb)). The structural parameters are well reproduced by the DFT geometry optimised structures of 1^Sn (Sn–N^py: 2.341 Å) and 1^Sn(thf) (Sn–O^thf: 2.606 Å, Sn–N^py: 2.441 Å) as a model for (1^Sn)₂(diox) (Fig. 3; ESI, Table S1†). To probe the presence of different tetrahydrofuran adducts, DFT calculations on 1^Sn(thf)₂ were additionally performed. Two isomers cis-1^Sn(thf)₂ and trans-1^Sn(thf)₂ could be localised as local minima on the potential energy surface as van-der-Waals adducts between two and one THF molecules and 1^Sn (ESI, Fig. S16†). The THF dissociation is exergonic with Gibbs free energies at 298 K for cis-/trans-1^Sn(thf)₂ (cis: ΔG₂₉₈ = −38 kJ mol⁻¹, trans: ΔG₂₉₈ = −47 kJ mol⁻¹) to 1^Sn(thf) and THF and slightly exergonic for dissociation of 1^Sn(thf) (ΔG₂₉₈ = −11 kJ mol⁻¹). This indicates that the solvent-free complex 1^Sn is the dominant species in solution. An exergonic Gibbs free dissociation energy of ΔG₂₉₈ = −31 kJ mol⁻¹ was experimentally determined for the THF dissociation of a cyclic dialkylstannylene THF adduct, supporting the present DFT results.⁶¹

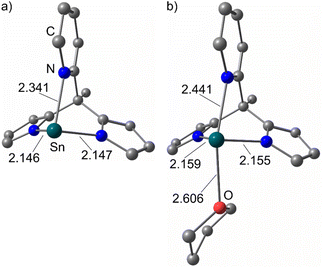Fig. 3 DFT optimised structures of (a) 1^Sn and (b) 1^Sn(thf) in the singlet ground state with selected structural parameters in Å (CPCM-(THF)-RIJCOSX-B3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn)).

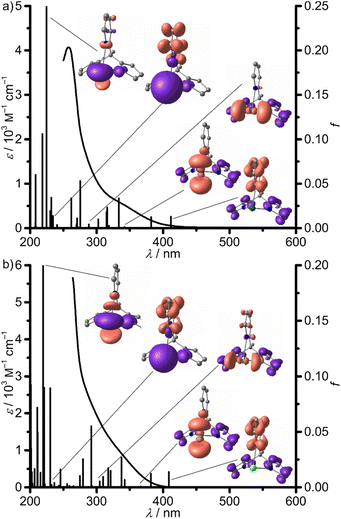
The UV/vis absorption spectra of 1^Sn and 1^Pb in THF and dimethylsulfoxide (DMSO), respectively, show several intense, broad, overlapping absorptions in the UV region tailing into the visible region with extinction coefficients ε > 500 M⁻¹ cm⁻¹ with λ < 350 nm (Fig. 4). TD-DFT calculations reveal several spin-allowed low-energy ¹ILCT transitions (412–303 nm) with significant oscillator strength between the electron-rich pyrrolato and electron-poor pyridine moieties for 1^Sn (Fig. 4a; ESI, Fig. S17†). In addition, pyrrolato → Sn ¹LMCT (342, 288, 275 and 272 nm) transitions at similar energies are observed, which might play a role in the dynamics of 1^Sn (vide infra). Sn → pyridine ¹MLCT (271 and 234 nm) and mixed ¹(ILCT/LMCT) (319 and 316 nm) transitions, as well as ¹MC (225 nm) transitions are found at higher energies at the Franck–Condon geometry. The TD-DFT spectrum of 1^Pb features ¹ILCT transitions (409–305 nm) at very similar energies as found for 1^Sn, indicating that the central ion does not significantly affect these ligand-centered ¹CT transitions. ¹LMCT and mixed ¹(ILCT/LMCT) transitions of 1^Pb are found at lower energies with 367 and 342 nm, respectively, closer to the lowest ¹ILCT transitions (Fig. 4b; ESI, Fig. S18,†¹MLCT (237 nm), ¹MC (220 nm)). The lower ¹LMCT transition energies of 1^Pb are attributed to weaker Pb–N^py and lead–nitrogen(pyrrolato) bonds Pb–N^pyr, associated with a lowering of the vacant σ*-orbitals (LUMO+1, LUMO+3) with high p(Pb)-character with respect to the derivative 1^Sn (LUMO+2, LUMO+3, Fig. 1; ESI, Fig. S19, S20 and Tables S1, S2†).

Fig. 4 UV/vis absorption spectra at 293 K of (a) 1^Sn in tetrahydrofuran and of (b) 1^Pb in dimethylsulfoxide with TD-DFT calculated oscillator strengths on 1^E and difference electron densities of selected characteristic spin-allowed transitions (isosurface value 0.005 a.u., purple = electron loss, orange = electron gain, CPCM-(THF)-RIJCOSX-B3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn)/SARC-ZORA-TZVPP(Pb)).

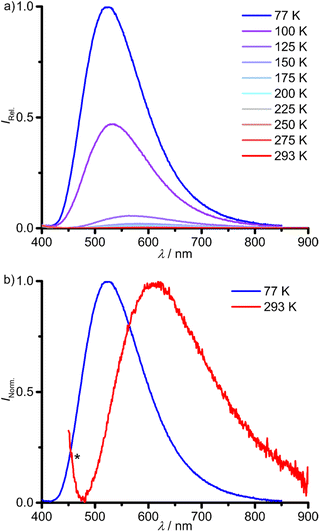
The investigation of the emission properties revealed a very weak photoluminescence of 1^Sn at λ_em,293 = 611 nm after excitation at λ_exc = 325 nm in 2-methyl-tetrahydrofuran (2-Me-THF) solution at 293 K (Fig. 5 and Table 1). Unfortunately, unidentified impurities in different batches of commercial spectroscopic grade 2-Me-THF and THF show photoluminescence in the UV with λ_em < 400 nm tailing into the weak emission of 1^Sn after excitation at the same wavelength (Fig. 5b; ESI, Fig. S21†). Variable-temperature emission spectra of 1^Sn in 2-Me-THF in a temperature range of 293–77 K show a significant increase in emission intensity and a blue-shift of the emission maximum from λ_em,293 = 611 nm in fluid solution at 293 K to λ_em,77 = 523 nm in frozen solution at 77 K (Fig. 5 and Table 1). This is probably a consequence of a rigidochromic effect.⁶² The excitation spectra at 293 and 77 K largely follow the absorption spectrum at the low energy part (Fig. 4; ESI, Fig. S22†). Due to the weak photoluminescence, high concentrations had to be used, which cause spectral distortion in the high energy range. The photoluminescence lifetime measurements of 1^Sn in fluid solution in the 125–293 K range gives ambiguous results due to the contribution of the photoluminescent impurity in 2-Me-THF. The determined lifetimes in the nanosecond range coincide with the emission of the unidentified impurity in 2-Me-THF (τ_em = 8 ns at 125 K). Hence, the photoluminescence lifetime of 1^Sn might be in a similar or smaller order of magnitude as rough estimate (ESI, Fig. S23†). The fluorescent impurity in 2-Me-THF, the very weak emission of 1^Sn at room temperature and the low solubility of 1^Sn in other solvents prevented the photoluminescence quantum yield determination in solution.

Fig. 5 (a) Temperature-dependent photoluminescence spectra of 1^Sn in deaerated 2-Me-THF between 77 K (blue) and 293 K (red) with λ_exc = 325 nm and (b) the normalised emission spectra at 77 K and 293 K. The asterisk denotes the emission of an unidentified impurity in 2-Me-THF.

Table 1 Emission properties of 1^Sn and 1^Pb

	λ p/nm	τ p	Φ p
a 2-Me-THF solution. b Solid state. c Not detectable. d Relative emission amplitudes in parentheses.
1^Sn
293 K^a	611	≤ 8 ns (n.d.^c)	n.d.^c
77 K^a	523	2.0 (37%), 0.5 (43%), 0.1 (20%) ms^d	n.d.^c
293 K^b	535	14 ns	0.005
77 K^b	504	3.6 ms	0.58
1^Pb
77 K^b	700	92 ns	0.0024

---

The photoluminescence lifetime of 1^Sn drastically increases to the millisecond range in frozen solution. The emission decay trace at 77 K is described with three lifetimes in the millisecond range (τ₁ = 2.0 ms, τ₂ = 0.5 ms, τ₃ = 0.1 ms; Table 1). At 100 K, the lifetimes drop by one order of magnitude with τ₃ vanishing in the instrument response function (IRF, τ₁ = 0.1 ms, τ₂ = 0.03 ms; Table 1; ESI, Fig. S24†). The strong temperature dependence of the photoluminescence lifetimes is associated to thermally activated non-radiative deactivation processes, which become dominant at elevated temperatures, even in frozen solution. Competing photophysical processes of different 1^Sn(2-Me-thf)_n adducts with varying number of coordinated 2-Me-THF molecules in (frozen) solution might account for a bi- or triexponential photoluminescence decay. The very long photoluminescence lifetime at 77 K assigns the emission to a phosphorescence process, i.e. emission from a triplet state. According to DFT calculations, phosphorescence occurs from a rather nested ³ILCT state (vide infra).

Solid donor solvent-free 1^Sn emits at shorter wavelength λ_p,293 = 535 nm (293 K) and with a similar band shape as in solution (Fig. 5, 6a and Table 1). The emission blue-shifts to λ_p,77 = 504 nm upon cooling to 77 K (Fig. 6a and Table 1). The phosphorescence lifetimes from monoexponential fits and the photoluminescence quantum yields are determined to τ_p,293 = 14 ns, Φ_p,293 = 0.005 and τ_p,77 = 3.6 ms, Φ_p,77 = 0.58 at 293 and 77 K, respectively (Table 1; ESI, Fig. S25†).

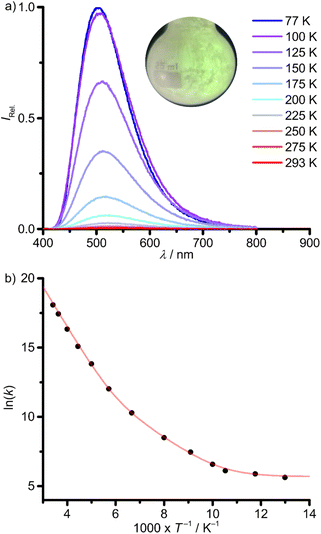Fig. 6 (a) Temperature-dependent photoluminescence spectra of 1^Sn in the solid state between 77 K (blue) and 293 K (red) with λ_exc = 350 nm (inset: photograph of the green photoluminescence at ca. 77 K) and (b) Arrhenius plot of the photoluminescence rate constants (k(T) = 1/τ_p(T), T = 77–293 K) with fit (red curve), according to eqn (1).

The strong temperature dependence of the photoluminescence lifetime and yield is attributed to thermally activated non-radiative processes. According to the high phosphorescence quantum yield at 77 K, ¹ES → ³ES ISC should be very efficient. Hence, non-radiative ES deactivation occurs mainly from the triplet ES. An Arrhenius plot of the photoluminescence rate constants (k(T) = 1/τ_p(T)) is fitted as a sum of three rate constants k₀/k₁/k₂ (eqn (1) and Fig. 6b).⁶³k₀ includes non-thermally activated radiative and non-radiative processes, while k₁(T) and k₂(T) are temperature dependent, expressed as Arrhenius equations with frequency factors A₁/A₂ and activation energies E_a1/E_a2 (eqn 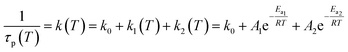(1)).

The obtained fit parameters indicate two thermally activated processes depopulating the photoluminescent state with barriers of E_a1 = 0.25 eV (2000 cm⁻¹), dominating the high temperature regime and E_a2 = 0.10 eV (810 cm⁻¹), dominant in the low temperature regime (Fig. 6b and Table 2).

Table 2 Fit parameters for the emission decays of solid 1^Sn and 1^Pb, obtained from Arrhenius plots, according to eqn (1)

	1^Sn	1^Pb
k 0/s^−1	298	3.77 × 10^6
E a1/eV (cm^−1)	0.25 (2000)	0.11 (890)
E a2/eV (cm^−1)	0.10 (810)	0.02 (200)
A 1/s^−1	1.57 × 10^12	1.88 × 10^11
A 2/s^−1	6.14 × 10^7	2.62 × 10^8

---

Thermally activated depopulation of the photoluminescent state via two non-emissive ESs at similar activation energies has been observed for Rh^III complexes.⁶³

In contrast, 1^Pb shows no photoluminescence in fluid DMSO solution at r.t. The low solubility of 1^Pb in glass forming solvents prevented the investigation of the photoluminescence properties in solution at low temperatures. Yet, 1^Pb shows a weak phosphorescence with λ_p,77 = 700 nm in the solid state at 77 K. Raising the temperature shifts the emission to lower energies with a strong loss of intensity (λ_p,200 = 720 nm, Fig. 7a). A minor impurity, below the NMR detection limit, with λ_em = 500 nm is ascribed to hydrolysis or oxidation products of 1^Pb, indicated by an asterisk in Fig. 7a. This assignment was verified by measuring the sample again after contact to air, showing a decrease of the band at ≈700 nm and an increase of the band at ≈500 nm (ESI, Fig. S26†).

Fig. 7 (a) Temperature-dependent photoluminescence spectra of 1^Pb in the solid state between 77 K (blue) and 200 K (red) with λ_exc = 350 nm. The spectrum at 77 K is composed of two spectra, measured with different detectors. The asterisk denotes the photoluminescence of an unidentified minor impurity (oxidation or solvolysis product of 1^Pb). (b) Arrhenius plot of the photoluminescence rate constants (k(T) = 1/τ_p(T), T = 77–200 K) with fit (red curve), according to eqn (1).

The photoluminescence lifetimes of 1^Pb determined from monoexponential fits drop from τ_p,77 = 92 ns to τ_p,200 = 2.4 ns upon warming from 77 to 200 K, respectively, with a very small photoluminescence quantum yield of Φ_p,77 = 0.0024 at 77 K (ESI, Fig. S27†). Two activation barriers E_a1 = 0.11 eV (890 cm⁻¹) and E_a2 = 0.02 eV (200 cm⁻¹) could be extracted from an Arrhenius plot of the photoluminescence rate constants (k(T) = 1/τ_p(T), eqn (1), Fig. 7b and Table 2). The much larger rate constant k₀ of 1^Pb (3.77 × 10⁶ s⁻¹) vs.1^Sn (298 s⁻¹) includes non-thermally activated radiative and non-radiative processes and will be discussed in the context of DFT calculations (vide infra). The phosphorescence of 1^Pb in the solid state at 77 K (λ_p,77 = 700 nm, 14 300 cm⁻¹) occurs at significantly lower energy as that of 1^Sn (λ_p,77 = 504 nm, 19 800 cm⁻¹), despite the very similar absorption spectra (Fig. 4, 6a and 7a). Hence, the emissive states must be of different nature. DFT calculations serve to assign the different emissive states (vide infra).

2.2. Quantum chemical investigations

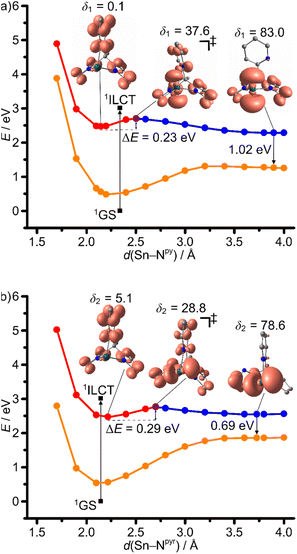
From the TD-DFT analysis, it becomes clear that excitation with 325 and 350 nm as used in the experimental setups populates a number of singlet states with different character in the Franck–Condon region, including ¹ILCT and ¹LMCT states (Fig. 4). It is likely that ISC is very fast and efficient due to the primary heavy atom effect via very large SOC of Sn and Pb (SOC constants: ζ(Sn) = 1855 cm⁻¹, ζ(Pb) = 5089 cm⁻¹).⁵⁸ Consequently, we focus in the following discussion on the triplet states of 1^Sn and 1^Pb only. In order to shed light onto the divergent emission properties of the tin and lead complexes, DFT calculations were performed on the triplet states (CPCM-(THF)-RIJCOSX-UB3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn)/SARC-ZORA-TZVPP(Pb)). In both cases, three triplet states with distinct structural features could be located, namely ³ILCT, ³LMCT_py and ³LMCT_pyr states. The ³ILCT state is essentially nested with the ¹GS. The major ¹GS to ³ILCT state geometric reorganization is an E–N^py bond contraction with a slight E–N^pyr elongation with parallel decrease of the angle between the pyrrolate mean-square planes from 130° to 106° (ESI, Tables S1 and S2†). The ³LMCT_py and ³LMCT_pyr states (py = pyridine and pyr = pyrrolato) are strongly distorted with broken E–N^py and E–N^pyr bonds, respectively, in addition to a tilting of the respective heterocyclic pyridine or pyrrolate rings (Fig. 8–10). The ³LMCT_py state possesses the lowest energy in both complexes. The next higher state is ³ILCT and ³LMCT_pyr for the tin and lead derivatives, respectively (Table 3).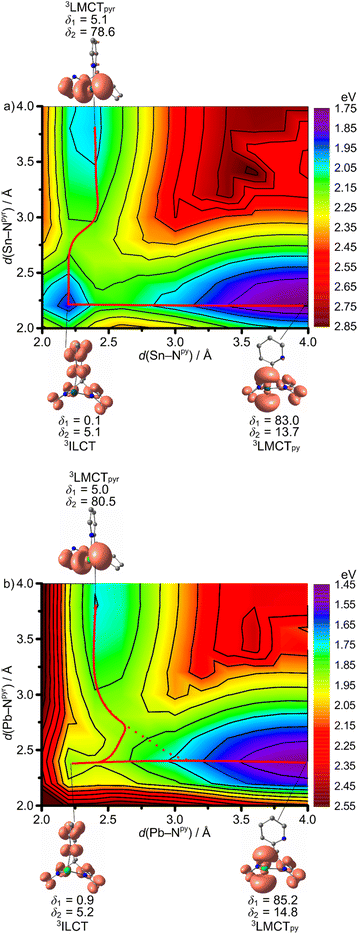

Fig. 8 Relaxed potential energy surface scans as projections along the (a) Sn–N^py and (b) Sn–N^pyr stretching modes on the triplet hypersurface ³1^Sn with selected geometry optimised molecular structures with spin densities (isosurface value: 0.005 a.u.) and single point energies on singlet GS hypersurface ¹1^Sn at ³1^Sn geometries (SCF energies). Selected Sn–N^py/pyr–C^py/pyr–C^backbone dihedral angles δ₁/δ₂ in deg. are indicated. ³ILCT states in red, triplet transition states ³ILCT → ³LMCT_py (a) and ³ILCT → ³LMCT_pyr (b) in purple, ³LMCT_py/³LMCT_pyr states in blue and ¹GS in orange. Vertical transition from relaxed ¹GS to the ¹ILCT state from TD-DFT calculations (3.01 eV) indicated as black arrow. CPCM-(THF)-RIJCOSX-(U)B3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn).

Fig. 9 (a) Relaxed potential energy surface scan as projection along the Pb–N^py stretching mode on the triplet hypersurface ³1^Pb with selected geometry optimised molecular structures with spin densities (isosurface value: 0.005 a.u.) and single point energies on singlet GS hypersurface ¹1^Pb at ³1^Pb geometries (SCF energies). Selected Pb–N^py–C^py–C^backbone dihedral angles δ₁ in deg. are indicated. ³ILCT state in red, triplet transition state ³ILCT → ³LMCT_py in purple, ³LMCT_py state in blue and ¹GS in orange. Vertical transition from relaxed ¹GS to the ¹ILCT state from TD-DFT calculations (3.03 eV) indicated as black arrow. Molecular structures with spin densities (isosurface value: 0.005 a.u.) and Pb–N^py/pyr–C^py/pyr–C^backbone dihedral angles δ₁/δ₂ in deg. of (b) the ³LMCT_pyr state and of (c) the ³LMCT_py/³LMCT_pyr transition state, respectively. CPCM-(THF)-RIJCOSX-(U)B3LYP-D3BJ-ZORA/def2-TZVPP/SARC-ZORA-TZVPP(Pb).

Fig. 10 Contour plots of 2D relaxed potential energy surface scans as projection along the E–N^py and E–N^pyr stretching modes on the triplet hypersurface ³1^E with selected geometry optimised molecular structures with spin densities (isosurface value: 0.005 a.u.) of (a) 1^Sn and (b) 1^Pb. Selected E–N^py/pyr–C^py/pyr–C^backbone dihedral angles δ₁/δ₂ in deg. are indicated and the graphically determined, estimated minimum energy paths for ³ILCT → ³LMCT_py/³LMCT_pyr (red lines) and ³LMCT_py/³LMCT_pyr internal conversion (dotted line). Energies are given vs. the ¹GS energy (ESI, Fig. S28†). CPCM-(THF)-RIJCOSX-UB3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn)/SARC-ZORA-TZVPP(Pb).

Table 3 Relative energies (SCF)^a of triplet states, ¹GS and transition states (TS) in eV, calculated by DFT for 1^Sn and 1^Pb

	1^Sn	1^Pb
a For Gibbs free energies, see: ESI, Table S3. b Relaxed geometry. c ^1GS at relaxed ^3ILCT, ^3LMCTpy and ^3LMCTpyr geometry, respectively. d Relative energy to the relaxed ^3LMCTpy state.
^3ILCT	0	0
^3LMCTpy	−0.19	−0.43
^3LMCTpyr	+0.08	−0.15
^1GSrelaxed^b	−2.47	−2.49
^1GS^3ILCT-geom^c	−1.91	−1.94
^1GS^3LMCTpy-geom^c	−1.21	−1.42
^1GS^3LMCTpyr-geom^c	−0.61	−0.95
TS(^3ILCT → ^3LMCTpy)	+0.23	+0.06
TS(^3ILCT → ^3LMCTpyr)	+0.29	—
TS(^3LMCTpy → ^3LMCTpyr)^d	—	+0.46

---

³ILCT → ³LMCT_py and ³ILCT → ³LMCT_pyr DFT optimized transition states were located for 1^Sn giving activation barriers of 0.23 and 0.29 eV, respectively (Table 3 and Fig. 8). A much lower ³ILCT → ³LMCT_py activation barrier of 0.06 eV was found for 1^Pb, while no ³ILCT → ³LMCT_pyr transition state could be located in this case (Fig. 9a). 2D maps of the triplet surfaces projected along the E–N^py and E–N^pyr modes were calculated on a lower level of theory for 1^Sn and 1^Pb (Fig. 10). The map of 1^Sn clearly shows two trajectories from the ³ILCT state to the ³LMCT states along minimum energy paths (Fig. 10a). In contrast, the map of 1^Pb discloses only a single minimum energy trajectory from the ³ILCT state towards the ³LMCT_py state, but suggests a (higher energy) path from the ³LMCT_py to the ³LMCT_pyr state (Fig. 10b). This excited state landscape explains the failure to locate a ³ILCT → ³LMCT_pyr transition state for 1^Pb.

Assuming that the dynamics after ISC on the triplet surface start at the respective relaxed ³ILCT states, the different barriers to the ³LMCT states of 0.23/0.29 eV and 0.06 eV for 1^Sn and 1^Pb, respectively, will determine the following dynamics (Table 3). Without emphasising the calculated numbers too strongly, we note that for 1^Sn an experimental barrier of E_a1 = 0.25 eV has been determined close the calculated values (Tables 2 and 3). As the ³LMCT_pyr state lies above the ³ILCT state, we assign the observed temperature-dependent emission of 1^Sn at 535 nm to the ³ILCT state and the observed barrier to the ³ILCT → ³LMCT_py population transfer. Consequently, the emission of 1^Sn is a non-Kasha emission due to a significant barrier to the lower energy triplet state (³LMCT_py).

On the other hand, the very small ³ILCT → ³LMCT_py barrier calculated for 1^Pb suggests that rapid evolution to the ³LMCT_py state occurs along with Pb–N^py bond elongation and dissociation. This strongly distorted state at low energy can rapidly decay back to the ¹GS, which fits to the experimental observations of a weak, short-lived, low-energy emission of 1^Pb (Table 1). The ³LMCT_pyr state of 1^Pb lies above the ³LMCT_py state and the ³LMCT_py → ³LMCT_pyr barrier of 0.46 eV impedes its significant population leading to the assignment of the observed low-energy emission as originating from the ³LMCT_py state (Table 3 and Fig. 9b, c). The other small barriers of 0.02–0.11 eV observed in the photoluminescence decays of 1^Sn and 1^Pb might be associated to other modes that promote the ISC from the triplet states to the ¹GS (Table 2). These enabling modes are not covered in our calculations (Table 3).

It is to note, in the frame of these DFT calculations the rigid environment of a solid with constrained geometric reorganisation is not fully described and the barriers and ES energies might be higher due to externally forced rigidification. Yet, distinct geometric flexibility in the solid state has been discussed for photo-switches⁶⁴ and photoluminescent copper(I) complexes.⁶⁵ On the other hand, rigidification by aggregation significantly impacts the GS and ES potential energy surfaces, e.g. raising the energy of conical intersections and surface crossing points, which leads to aggregation-induced emission^66,67 and in particular, aggregation-induced phosphorescence.^18,68 The molecular flexibility in the solid state of 1^Sn and in particular of 1^Pb might be sufficient for thermally activated depopulation of the ³ILCT state via the lower-lying ³LMCT states. However, the lifetime and energy of the ³LMCT_py state are sufficiently high to observe the very weak phosphorescence of 1^Pb at low temperatures (Fig. 7).

The experimental rate constant k₀ = 298 s⁻¹ of 1^Sn is much smaller than k₀ = 3.77 × 10⁶ s⁻¹ of 1^Pb. This finding might be associated with the different vertical energy gaps of 1.91 and 0.99 eV between the emissive states ³ILCT/³LMCT_py and the ¹GS (Fig. 8a, 9a and Table 3) and the different SOC contribution promoting ISC to the ¹GS in the ³ILCT (smaller SOC) and ³LMCT (higher SOC) states, respectively, together with the smaller heavy-atom effect for Sn, compared to Pb.

The excited state dynamics of 1^Sn in frozen 2-Me-THF might additionally be affected by solvent coordination during freezing and hence the presence of different solvates in the frozen sample, e.g.1^Sn(2-Me-thf)_n, which might account for the multiple emission lifetimes. Consequently, TD-DFT calculations were performed on 1^Sn(thf). Similar to 1^Sn, the lowest Franck–Condon states are of ¹ILCT character, slightly shifted to higher energies (ESI, Fig. S29–31†).

The Sn–O^thf distance of 2.606 Å in the ¹GS increases to 2.925 Å in the ³ILCT state, while the Sn–N distances are essentially unchanged, according to DFT calculations (Fig. 3b and 11a; ESI, Table S1†). This suggests that the initially populated ³ILCT state of the adduct 1^Sn(thf) is strongly distorted. The deactivation of the ³ILCT state occurs preferentially via the ³LMCT_py state suggested by a 2D relaxed triplet surface scan as a projection along the Sn–O^thf and Sn–N^py modes (Fig. 12). The trajectory for ³ILCT/³LMCT_py internal conversion follows the Sn–O^thf coordinate with THF dissociation, forming donor-free 1^Sn within the ³ILCT state and then converts to the ³LMCT_py state along the Sn–N^py coordinate (Fig. 12). The ³LMCT_py state is slightly destabilised relative to the ³ILCT in the loose van-der-Waals adduct between THF and 1^Sn in 1^Sn(thf) (Tables 3 and 4; ESI, Tables S3 and S4†). A relaxed surface scan on the ³ILCT surface along the Sn–O^thf stretching mode shows that THF dissociation is essentially barrierless (Fig. 11a). Additionally, a ³MLCT and a ³LMCT_pyr state with dissociated pyrrolato moiety and THF, could be located as local minima for the THF adduct 1^Sn(thf) at higher energies (Fig. 11; ESI, Table S1†). The geometries of the relaxed ³MLCT and ³ILCT states mainly differ by their Sn–O^thf distances and N^py–Sn–O^thf angles δ₃, in accordance to the valence shell electron pair repulsion model as simplest explanation (Fig. 11a; ESI, Table S1†). Due to their comparably high energy, these ³MLCT and ³LMCT_pyr states should not play a significant role in the deactivation of the phosphorescent ³ILCT state (Table 4; ESI, Table S4†).

Fig. 11 (a) Relaxed potential energy surface scans as projections along the Sn–O^thf stretching mode on the triplet hypersurface ³1^Sn(thf) with selected geometry optimised molecular structures with spin densities and single point energies on singlet GS hypersurface ¹1^Sn(thf) at ³1^Sn(thf) geometries (SCF energies). ³ILCT state indicated in red, ³MLCT state in green and ¹GS in orange. Vertical transition from relaxed ¹GS to the ¹ILCT state from TD-DFT calculations (3.15 eV) indicated as black arrow. (b) Molecular structure of the ³LMCT_pyr state with spin density. Spin density isosurface values are at 0.005 a.u. Selected Sn–N^py/pyr–C^py/pyr–C^backbone dihedral angles δ₁/δ₂ and N^py–Sn–O^thf angles δ₃ in deg. are indicated. CPCM-(THF)-RIJCOSX-(U)B3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn).

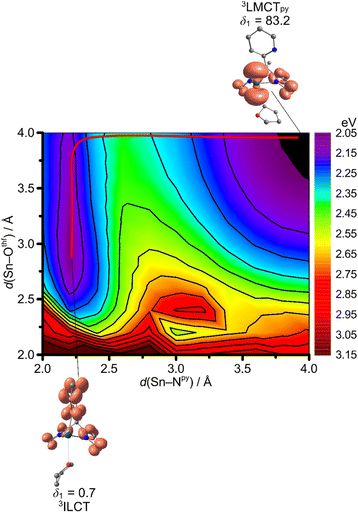Fig. 12 Contour plot of a 2D relaxed potential energy surface scan as projection along the Sn–N^py and Sn–O^thf stretching modes on the triplet hypersurface ³1^Sn(thf) with selected geometry optimised molecular structures with spin densities (isosurface value: 0.005 a.u.). Selected E–N^py–C^py–C^backbone dihedral angles δ₁ in deg. are indicated and the graphically determined, estimated minimum energy path for ³ILCT → ³LMCT_py (red line) internal conversion. Energies are given vs. the ¹GS energy (ESI, Fig. S32†). CPCM-(THF)-RIJCOSX-UB3LYP-D3BJ-ZORA/def2-TZVPP/old-ZORA-TZVPP(Sn).

Table 4 Relative energies (SCF)^a of triplet states and ¹GS in eV, calculated by DFT for 1^Sn(thf)

a For Gibbs free energies, see: ESI, Table S4. b Relaxed geometry. c ^1GS at relaxed ^3ILCT, ^3LMCTpy, ^3MLCT and ^3LMCTpyr geometry, respectively.
^3ILCT	0
^3LMCTpy	−0.11
^3MLCT	+0.79
^3LMCTpyr	+0.10
^1GSrelaxed^b	−2.58
^1GS^3ILCT-geom^c	−2.02
^1GS^3LMCTpy-geom^c	−1.15
^1GS^3MLCT-geom^c	−1.55
^1GS^3LMCTpyr-geom^c	−0.73

---

In summary, the calculations suggest that donor-free 1^Sn shows emission from a weakly distorted ³ILCT state (non-Kasha behavior) due to high enough barriers to the ³LMCT states, while 1^Pb efficiently evolves from the initially populated nested ³ILCT state to the dissociative, low-energy, weakly emissive ³LMCT_py state. Compared to the often weakly distorted CT states of photoluminescent TMCs,^1–3 the low-energy ³LMCT states observed here are highly distorted. Clearly, this arises from the rather non-bonding character of the involved transition metal d-orbitals, while ³LMCT states of 1^Sn and 1^Pb are characterised by the population of σ*(E–X) orbitals leading to large distortions and even donor moiety dissociation (pyridine or pyrrolato). Excited states of ³MLCT nature involving the non-bonding tetrel-centered lone-pair of high s character would be less distorted. Such ³MLCT states indeed exist, yet at higher energies than the dissociative ³LMCT states in 1^Sn and 1^Pb (Fig. 1 and 4; ESI, Fig. S17 and S18†). ³LMCT states involving the empty p-type orbital in non-donor stabilised tetrylenes might provide another weakly distorted and potentially emissive CT state in tetrylenes.

3 Conclusions

Sn^II(bpep) 1^Sn shows phosphorescence from an ³ILCT state (non-Kasha behavior) in solution and in the solid state. The phosphorescence quantum yield and the photoluminescence lifetime drastically increase from τ_p,293 = 14 ns, Φ_p,293 = 0.005 to τ_p,77 = 3.6 ms, Φ_p,77 = 0.58 from 293 to 77 K in the solid state. In contrast, Pb^II(bpep) 1^Pb shows very weak short-lived phosphorescence at 77 K (τ_p,77 = 92 ns, Φ_p,77 = 0.0024) with strong decrease in lifetime to τ_p,200 = 2.4 ns at 200 K. This photoluminescence arises from a ³LMCT state, populated via IC from the initially populated ³ILCT state. The strong temperature dependence is attributed to two thermally activated processes with experimentally determined barriers of E_a1 = 0.25 eV and E_a2 = 0.10 eV for 1^Sn and E_a1 = 0.11 eV and E_a2 = 0.02 eV for 1^Pb. Two strongly distorted, dissociative ³LMCT states, according to (2D) relaxed potential energy surface scans by DFT calculations, open up pathways for fast (non-radiative) deactivation of the nested ³ILCT state. The corresponding barrier is significantly lower for 1^Pb (0.06/0.06 eV) than for 1^Sn (0.23/0.25 eV, DFT SCF/Gibbs free energies). ISC is fast despite the weak contribution of the Sn and Pb atoms to the excited state wavefunctions of the initially populated ¹ILCT state. The expectedly larger heavy-atom effect, leading to faster ISC of the lead compound 1^Pb, is compensated by the much faster deactivation due to the significantly smaller barriers, obtained from variable-temperature photoluminescence lifetime measurements and supported by DFT calculations. In solution, the population of the ³ILCT state of the THF adduct 1^Sn(thf) is accompanied with solvent decoordination and formation of 1^Sn.

In order to obtain phosphorescent tetrel(II) complexes, the focus should not be kept solely on the heavy-atom effect, invoking effective SOC to enable fast singlet-to-triplet ISC, but also on the ES ordering, enabling fast non-radiative deactivation of potential phosphorescent ESs. Geometric excited state reorganisation and solvent (de-)coordination seems to play a more pronounced role for tetrylenes than for pseudo-octahedral TMCs, but seems comparable to pseudo-tetrahedral Cu^I complexes with their ES flattening distortion, prone to solvent or anion coordination.

With the energy landscape of 1^E (E = Sn, Pb), obtained from this initial study, a rational optimisation of the photoluminescent properties emerges. (1) The ³ILCT state should be pushed to energies below the ³LMCT states with electron-withdrawing substituents on the pyridine moieties. (2) The ³LMCT states could be pushed to higher energies with the lighter homologues germanium and silicon, probably with higher ³ILCT/³LMCT barriers by decreased molecular flexibility. (3) Rigidification of the pyridine moiety with bulky substituents could prevent the dissociation and torsional motion of the pyridine. (4) Lowering the energy of the tentatively weakly distorted ³MLCT states below the ³ILCT and ³LMCT states to obtain ³MLCT phosphorescence. (5) Mitigating the dissociative character of the ³LMCT states in non-donor stabilised tetrylenes. Current investigations in these directions are in progress.

Data availability

All experimental and computational data are available in the ESI.† Crystallographic data have been deposited in the Cambridge Crystallographic Data Centre under accession numbers CCDC 2211125 and 2211126.

Author contributions

P. S. performed the synthesis and characterisation as well as (TD-)DFT calculations. R. N. and P. S. measured and interpreted the emission data. C. F. solved the single crystal structures. C. F. conceived and designed the project. C. F. wrote the manuscript with contributions of all authors. K. H. supervised and C. F. co-supervised the project.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work has been financially supported by the Deutsche Forschungsgemeinschaft (DFG) under grant INST 247/1018-1 (K. H.). Parts of this research were conducted using the supercomputer Elwetritsch and advisory services offered by the University of Kaiserslautern-Landau (https://hpc.rz.rptu.de), which is a member of the AHRP and the Gauss Alliance e.V. We thank Dr Dieter Schollmeyer for collecting the XRD data and Dr Mihail Mondeshki for ¹¹⁹Sn and ²⁰⁷Pb NMR measurements.

References

1. C. Wegeberg and O. S. Wenger, JACS Au, 2021, 1, 1860–1876.
2. C. Förster and K. Heinze, Chem. Soc. Rev., 2020, 49, 1057–1070.
3. O. S. Wenger, Chem.–Eur. J., 2019, 25, 6043–6052.
4. B. M. Hockin, C. Li, N. Robertson and E. Zysman-Colman, Catal. Sci. Technol., 2019, 9, 889–915.
5. C. B. Larsen and O. S. Wenger, Chem.–Eur. J., 2018, 24, 2039–2058.
6. V. Balzani, P. Ceroni and A. Juris, Photochemistry and Photophysics. Concepts, Research, Applications, Wiley-VCH, Weinheim, 1st edn, 2014.
7. T. J. Penfold, E. Gindensperger, C. Daniel and C. M. Marian, Chem. Rev., 2018, 118, 6975–7025.
8. M. Iwamura, S. Takeuchi and T. Tahara, Acc. Chem. Res., 2015, 48, 782–791.
9. N. Armaroli, Chem. Soc. Rev., 2001, 30, 113–124.
10. C. Sandoval-Pauker, M. Santander-Nelli and P. Dreyse, RSC Adv., 2022, 12, 10653–10674.
11. Y. Zhang, M. Schulz, M. Wächtler, M. Karnahl and B. Dietzek, Coord. Chem. Rev., 2018, 356, 127–146.
12. S. A. Patra, G. Sahu, P. D. Pattanayak, T. Sasamori and R. Dinda, Inorg. Chem., 2022, 61, 16914–16928.
13. J. C. Berrones-Reyes, C. C. Vidyasagar, B. M. Muñoz Flores and V. M. Jiménez-Pérez, J. Lumin., 2018, 195, 290–313.
14. D. S. M. Ravinson and M. E. Thompson, Mater. Horiz., 2020, 7, 1210–1217.
15. H. Yersin, R. Czerwieniec, M. Z. Shafikov and A. F. Suleymanova, ChemPhysChem, 2017, 18, 3508–3535.
16. H. Yersin, A. F. Rausch, R. Czerwieniec, T. Hofbeck and T. Fischer, Coord. Chem. Rev., 2011, 255, 2622–2652.
17. S. Arunkumar, D. Ghosh and G. R. Kumar, Results Chem., 2022, 4, 100399.
18. S. M. Parke and E. Rivard, Isr. J. Chem., 2018, 58, 915–926.
19. S. M. Parke, M. P. Boone and E. Rivard, Chem. Commun., 2016, 52, 9485–9505.
20. L. A. Maurer, O. M. Pearce, F. D. R. Maharaj, N. L. Brown, C. K. Amador, N. H. Damrauer and M. P. Marshak, Inorg. Chem., 2021, 60, 10137–10146.
21. A. K. Adcock, R. L. Ayscue, L. M. Breuer, C. P. Verwiel, A. C. Marwitz, J. A. Bertke, V. Vallet, F. Réal and K. E. Knope, Dalton Trans., 2020, 49, 11756–11771.
22. S. M. Parke, E. Hupf, G. K. Matharu, I. de Aguiar, L. Xu, H. Yu, M. P. Boone, G. L. C. de Souza, R. McDonald, M. J. Ferguson, G. He, A. Brown and E. Rivard, Angew. Chem., Int. Ed. Engl., 2018, 57, 14841–14846.
23. S. M. Parke, M. A. B. Narreto, E. Hupf, R. McDonald, M. J. Ferguson, F. A. Hegmann and E. Rivard, Inorg. Chem., 2018, 57, 7536–7549.
24. O. Toma, M. Allain, F. Meinardi, A. Forni, C. Botta and N. Mercier, Angew. Chem., Int. Ed., 2016, 55, 7998–8002.
25. O. Toma, N. Mercier, M. Allain, A. Forni, F. Meinardi and C. Botta, Dalton Trans., 2015, 44, 14589–14593.
26. O. Toma, N. Mercier and C. Botta, Eur. J. Inorg. Chem., 2013, 1113–1117.
27. A. Strasser and A. Vogler, Inorg. Chem. Commun., 2004, 7, 528–530.
28. Y. Kang, D. Song, H. Schmider and S. Wang, Organometallics, 2002, 21, 2413–2421.
29. D. Temerova, T.-C. Chou, K. S. Kisel, T. Eskelinen, N. Kinnunen, J. Jänis, A. J. Karttunen, P.-T. Chou and I. O. Koshevoy, Inorg. Chem., 2022, 61, 19220–19231.
30. A. S. Gowda, T. S. Lee, M. C. Rosko, J. L. Petersen, F. N. Castellano and C. Milsmann, Inorg. Chem., 2022, 61, 7338–7348.
31. M. Kocherga, J. Castaneda, M. G. Walter, Y. Zhang, N.-A. Saleh, L. Wang, D. S. Jones, J. Merkert, B. Donovan-Merkert, Y. Li, T. Hofmann and T. A. Schmedake, Chem. Commun., 2018, 54, 14073–14076.
32. D. Maeda, H. Shimakoshi, M. Abe and Y. Hisaeda, Inorg. Chem., 2009, 48, 9853–9860.
33. A. Endo, M. Ogasawara, A. Takahashi, D. Yokoyama, Y. Kato and C. Adachi, Adv. Mater., 2009, 21, 4802–4806.
34. W.-L. Jia, Q.-D. Liu, R. Wang and S. Wang, Organometallics, 2003, 22, 4070–4078.
35. M. Gouterman, F. P. Schwarz, P. D. Smith and D. Dolphin, J. Chem. Phys., 1973, 59, 676–690.
36. M. Kocherga, K. M. Boyle, J. Merkert, T. A. Schmedake and M. G. Walter, Mater. Adv., 2022, 3, 2373–2379.
37. J. Hoffmann, I.-M. Ramirez Y Medina, M. Hissler and A. Staubitz, Dalton Trans., 2021, 50, 6213–6221.
38. M. Gon, K. Tanaka and Y. Chujo, Chem.–Eur. J., 2021, 27, 7561–7571.
39. I.-M. Ramirez Y Medina, M. Rohdenburg, W. Kipke, E. Lork and A. Staubitz, Molecules, 2020, 25, 4993.
40. I.-M. Ramirez Y Medina, M. Rohdenburg, E. Lork and A. Staubitz, Chem. Commun., 2020, 56, 9775–9778.
41. W. Ki, K. Ngo, P. Ghosh, B. Averkiev, G. T. Reeves, I. Ailes, B. C. Pemberton, K. Zhu and J. Li, Chem. Commun., 2020, 56, 9648–9650.
42. M. Yamamura, M. Albrecht, M. Albrecht, Y. Nishimura, T. Arai and T. Nabeshima, Inorg. Chem., 2014, 53, 1355–1360.
43. K. Takano, M. Takahashi, T. Fukushima, M. Takezaki, T. Tominaga, H. Akashi, H. Takagi and T. Shibahara, Bull. Chem. Soc. Jpn., 2012, 85, 1210–1221.
44. S. M. Crawford, A. Al-Sheikh Ali, T. S. Cameron and A. Thompson, Inorg. Chem., 2011, 50, 8207–8213.
45. R. Ballardini, G. Varani, M. T. Indelli and F. Scandola, Inorg. Chem., 1986, 25, 3858–3865.
46. H. Nikol, A. Becht and A. Vogler, Inorg. Chem., 1992, 31, 3277–3279.
47. K. Oldenburg and A. Vogler, Z. Naturforsch., B: J. Chem. Sci., 1993, 48, 1519–1523.
48. A. Becht and A. Vogler, Z. Naturforsch., B: J. Chem. Sci., 1994, 49, 778–780.
49. A. Strasser and A. Vogler, J. Photochem. Photobiol., A, 2004, 165, 115–118.
50. L.-X. Wang, J. Xiang, D. Xiang, S.-C. Cheng, C.-F. Leung and C.-C. Ko, Inorg. Chem., 2022, 61, 16831–16840.
51. J. Kobayashi, T. Kushida and T. Kawashima, J. Am. Chem. Soc., 2009, 131, 10836–10837.
52. J.-D. Guo, D. J. Liptrot, S. Nagase and P. P. Power, Chem. Sci., 2015, 6, 6235–6244.
53. Y. Mizuhata, T. Sasamori and N. Tokitoh, Chem. Rev., 2009, 109, 3479–3511.
54. K. W. Klinkhammer, in The Chemistry of Organic Germanium, Tin and Lead Compounds, ed. Z. Rappoport, John Wiley Sons Ltd, Chichester, 2nd edn, 2002, vol. 2, pp. 283–357.
55. N. Tokitoh and R. Okazaki, Coord. Chem. Rev., 2000, 210, 251–277.
56. P. P. Power, Chem. Rev., 1999, 99, 3463–3504.
57. G. Gao, I. Korobkov and S. Gambarotta, Inorg. Chem., 2004, 43, 1108–1115.
58. M. Montalti and S. L. Murov, Handbook of Photochemistry, CRC/Taylor & Francis, Boca Raton, 3rd edn, 2006.
59. M. J. S. Gynane, D. H. Harris, M. F. Lappert, P. P. Power, P. Rivière and M. Rivière-Baudet, Dalton Trans., 1977, 2004–2009.
60. J. D. Parish, M. W. Snook, A. L. Johnson and G. Kociok-Köhn, Dalton Trans., 2018, 47, 7721–7729.
61. M. Kira, S. Ishida, T. Iwamoto, R. Yauchibara and H. Sakurai, J. Organomet. Chem., 2001, 636, 144–147.
62. A. J. Lees, Comments Inorg. Chem., 1995, 17, 319–346.
63. F. Barigelletti, D. Sandrini, M. Maestri, V. Balzani, A. von Zelewsky, L. Chassot, P. Jolliet and U. Maeder, Inorg. Chem., 1988, 27, 3644–3647.
64. A. Gonzalez, E. S. Kengmana, M. V. Fonseca and G. Han, Mater. Today Adv., 2020, 6, 100058.
65. C. L. Linfoot, M. J. Leitl, P. Richardson, A. F. Rausch, O. Chepelin, F. J. White, H. Yersin and N. Robertson, Inorg. Chem., 2014, 53, 10854–10861.
66. S. Suzuki, S. Sasaki, A. S. Sairi, R. Iwai, B. Z. Tang and G.-I. Konishi, Angew. Chem., Int. Ed., 2020, 59, 9856–9867.
67. R. Crespo-Otero, Q. Li and L. Blancafort, Chem.–Asian J., 2019, 14, 700–714.
68. L. Ravotto and P. Ceroni, Coord. Chem. Rev., 2017, 346, 62–76.

---

Footnote

† Electronic supplementary information (ESI) available: Experimental procedures, spectral details, (TD-)DFT results. CCDC 2211125 and 2211126. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d2sc06984a

---