Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Exploring luminescence quenching mechanisms and temperature sensing capabilities of LiSrYW3O12:Sm3+ phosphors
F.
Ayachi
,
K.
Saidi
 and
M.
Dammak
and
M.
Dammak
 *
*
Laboratoire de Physique Appliquée, Faculté des Sciences de Sfax, département de Physique, Université de Sfax, BP, 1171, Sfax, Tunisia. E-mail: madidammak@yahoo.fr; Mohamed.dammak@fss.usf.tn
First published on 27th June 2024
Abstract
Optical thermometry has emerged as a crucial non-contact method for temperature measurement, serving a broad array of applications. This technique delivers precise readings without the need for physical contact, proving especially valuable in situations where traditional contact-based methods are impractical. However, the pursuit of heightened accuracy in optical thermometry remains an ongoing pursuit. Within this context, the advancement of luminescent thermometers assumes significant importance, offering the promise of enhanced temperature sensing capabilities. Through the exploration of novel materials and innovative strategies, the goal is to elevate the precision of these sensors, paving the way for more dependable temperature measurements in various environments. Therefore, the focus of the present study lies in the synthesis of a series of LiSrYW3O12 (LSYW):x%Sm3+ phosphors using the solid-state reaction technique, where x varies from 0.03 to 0.15. The investigation delves into the structures, morphologies, UV-Visible absorption, and luminescent characteristics of these phosphors. The down-conversion luminescence properties were studied under 405 nm laser excitation, leading to the determination of the optimal doping concentration at x = 0.05. Subsequent in-depth analysis focused on the optical temperature sensing properties, employing the fluorescence intensity ratio (FIR) method. The FIR of the 4F3/2/4G5/2 → 6H7/2 transition consistently increased with temperature across the range of 300–583 K. The maximum relative sensitivities were determined as 1.58% K−1 (at 301 K). These findings underscore the exceptional potential of LSYW doped with Sm3+ as a highly effective material for applications in high-temperature sensing. Through this research, the pursuit of more accurate and reliable temperature measurements takes a significant step forward, offering promising avenues for further exploration and utilization.
1. Introduction
Temperature is a significant factor in the domains of material engineering and equipment manufacture, as it often influences several physical and chemical aspects of goods to a certain degree. Thus, applications in industry are extremely interested in thermometers since they can measure an object's temperature correctly.1–3 When measuring temperature, contact thermometers – including thermocouple thermometers are susceptible to a number of limitations. Rare earth ions, particularly the trivalent Sm3+, play a significant role as activator ions in the development of novel luminescent materials.4–6 Due to its multi-energy structure and orange-red light emissions from its 4G5/2 level, Sm3+ has attracted a lot of interest recently. Meanwhile, its high quantum efficiency makes it a promising candidate for use in high-density optical storage, underwater communication, and color display.7 Extensive theoretical and experimental investigations have been conducted on Sm3+ ions.8,9 Their unique 4f–4f transitions result in red-orange emissions, making materials activated with Sm3+ ions desirable for various applications, including white light-emitting diodes (w-LEDs). Recent research has focused on utilizing different host materials, such as Ba2Ca(BO3)2:Sm3+, Ba2CaZn2Si6O17:Sm3+, and LiBaPO4:Sm3+, to achieve red-orange emissions.10–12 Additionally, the potential applications of Sm3+-doped materials as red phosphors can be enhanced through codoping with other lanthanide ions.13,14 However, Sm3+ doped tungstate phosphors with Li, Na, and K ions show excellent luminous intensity along with chemical and thermal stability.15,16 Due to their combination of high thermal conductivity, excellent thermal and mechanical stability, low phonon energy (around 900 cm−1), high melting point (1240 °C), and chemical stability, complex tungstate structures are considered promising host matrices for rare earth doping.17–20Binary alkaline earth tungstates, denoted as MLn2 (WO4)4 (with M representing an alkaline earth bivalent metal ion and Ln representing trivalent rare-earth ions), are categorized among scheelite-structured compounds. The extensive investigation of scheelite-structured binary molybdates and tungstates has been driven by their remarkable up-conversion (UC) photoluminescence properties, as documented in prior studies.21,22 Specifically, binary NaLn (WO4)2 compounds, when doped with rare-earth ions, display a tetragonal phase with the I41/a space group, falling under the broader classification of scheelite-type structures. This phase is frequently utilized to facilitate the partial substitution of trivalent rare-earth ions (Ln3+) by Ho3+ and Yb3+ ions, introducing disorder in the tetragonal phase at the lanthanide site of Ln3+.23–25
To date, a single report on ternary tungstates with a general composition of NaMLn(WO4)3 has been documented, where M represents Ca2+, Sr2+ and Ba2+, and Ln represents La3+, Gd3+ and Y3+,with Ho3+/Yb3+ codopage.26,27 In this article, we will explore LiSrYW3O12 doped with Sm3+ for thermometry applications. This paper explores the synthesis of LSYW:Sm3+ materials through the solid-state reaction method. The down-conversion luminescence characteristics of LSYW:Sm3+ were assessed at room temperature using a 405 nm laser. Furthermore, the temperature dependence of LSYW doped with 5% Sm3+ was comprehensively investigated over the range of 301 to 583 K.
2. Experimental procedure
2.1. Synthesis
LSYW microcrystalline samples doped with Sm3+ ions were synthesized using a high-temperature solid-state reaction technique. The starting materials, with a high purity of 99.999%, included Li2CO3, SrCO3, Y2O3, WO3 and Sm2O3 (Sigma-Aldrich).Various doping concentrations of Sm3+ (0.03, 0.05, 0.07, 0.1, and 0.15 relative to Y3+ ions) were employed. Stoichiometric amounts of the high-purity chemicals were meticulously mixed and ground using an agate mortar. Subsequently, the mixture was gradually heated to 350 °C for 16 hours to eliminate gases. The resulting powder was then compressed into cylindrical pellets and heated at 900 °C for 10 hours.
2.2. Characterization
The X-ray diffraction (XRD) patterns of the phosphors were measured in the 2θ range of 5–60° using an X-ray diffractometer (Bruker D8, Germany) equipped with Cu Kα radiation (λ = 1.5405 Å), operating at 40 kV and 30 mA for phase identification. UV-vis-NIR absorption was analyzed utilizing a UV-vis-NIR spectrometer (PerkinElmer Lambda 950). Morphological characteristics of the samples were investigated via scanning electron microscopy (SEM) (JEOL, JSM 6510LV). Temperature-dependent emissions were studied using a JOBIN YVON HR 320 spectrometer with a 405 nm laser excitation source. The temperature of the sample was varied from room temperature to 588 K with the interval of 10 K.3. Results and discussions
3.1. Characterization of crystalline phase
The XRD patterns were employed to investigate the crystal structure and phase purity of the materials. Fig. 1. displays the X-ray diffraction patterns of microcrystals LSYW:xSm3+ with x = 0.03, 0.05, 0.07, 0.1, and 0.15, registered in the range of 5–65°. According to the XRD patterns of LSYW (JCPDS No. 96-154-6037), the samples exhibit a tetragonal structure with space group I41/a (88), which is isomorphic to SrWO4, and the peaks are accurately indexed. The produced phosphors are observed to form in a single phase, as no additional peaks are evident in the X-ray diffraction pattern. This confirmation suggests the successful occupation of the Y3+ site by Sm3+ ions. Notably, a shift of the XRD peak at 27.9° to lower angles is observed with increasing Sm3+ concentrations, As show in Fig. 1b. This shift can be explained by the substitution of Y3+ ions by Sm3+ ions and the difference in their ionic radii, leading to an increase in lattice parameters and cell volumes.The SEM pictures of the LSYW:0.05Sm3+ phosphors are displayed in Fig. 2. The micrographs that were gathered show that the particles were aggregated and that their sizes fell within 1 μm, it is significant to keep in mind that solid-state chemical processes frequently exhibit this aggregation phenomena.
4. Optical characterization
4.1. UV-visible spectra
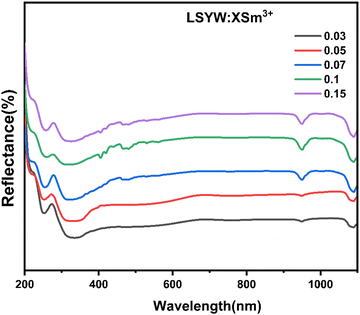
Diffuse reflectance spectra are collected over the wavelength range 200–1100 nm as seen in Fig. 3. In order to find the optical band gaps for the powder samples.According to previously reported literatures,28,29 we can see that the energy band gap (Eg) and absorption coefficient (α) are related in the way shown below (eqn (1)):
| (αhν)n = A(hν − Eg) | (1) |
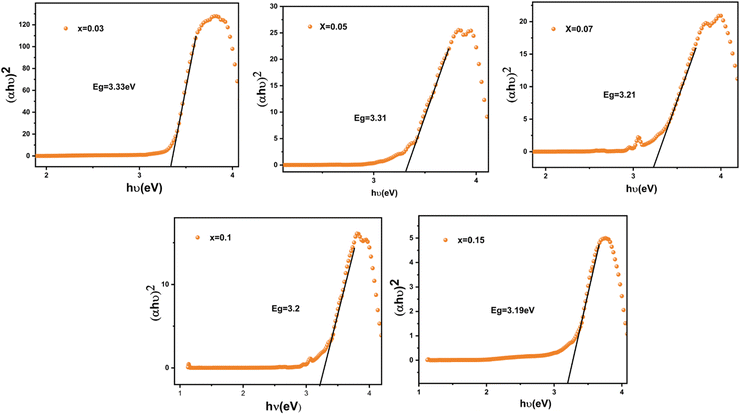
The Eg values are calculated by plotting (αhν)2 against the photon energy hα (eV), as illustrated in Fig. 4. when the absorption coefficient (α) is projected to zero, the band gaps of the LSYW:xSm3+ samples decrease with an increase of the “x” value and vary between 3.31 and 3.19 eV.
4.2. PL and PLE characterizations
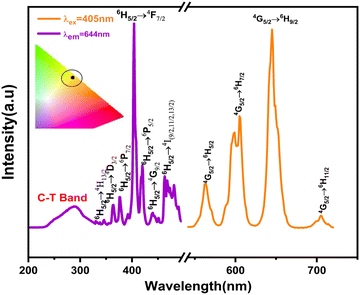
The excitation (PLE) and emission (PL) spectra of Sm3+-activated LSYW phosphors are shown in Fig. 5. In PLE spectra, a broad charge transfer band from 220 to 350 nm and a number of sharp peaks are observed in the 360–550 nm range. The broad charge transfer band (CTB) was assigned to the O2− → W6+ transition. The sharp peaks are assigned to the 4f–4f electronic transition of Sm3+ ions. Which are located at 345, 362, 377, 405, 418, 439, and 462 nm, corresponding to the transitions 6H5/2 → 4H13/2,6H5/2 → 4D3/2, 6H5/2 → 6P7/2, 6H5/2 → 4F5/26H5/2 → 6P5/2, 6H5/2 → 6G9/2 and 6H5/2 → 4I(9/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11/2,
11/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 13/2), respectively.12,30 Since the absorption peak at 405 nm is more intense than the other absorption peaks, the Near UV LED chip can effectively excite the synthesized phosphor, which is essential for the commercial use of WLEDs. PL emission spectra measured at 405 nm excitation, reveals a variety of peaks in the 550–725 nm wavelength region. A total of five emission bands exists: the band emitted by the 4F3/2 → 6H5/2 transition at 523–543, which is weak at room temperature, and the bands emitted by 6H(5/2,
13/2), respectively.12,30 Since the absorption peak at 405 nm is more intense than the other absorption peaks, the Near UV LED chip can effectively excite the synthesized phosphor, which is essential for the commercial use of WLEDs. PL emission spectra measured at 405 nm excitation, reveals a variety of peaks in the 550–725 nm wavelength region. A total of five emission bands exists: the band emitted by the 4F3/2 → 6H5/2 transition at 523–543, which is weak at room temperature, and the bands emitted by 6H(5/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7/2,
7/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9/2,
9/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11/2) transitions whose wavelength ranges are 543–581 nm, 581–634 nm, 634–685 nm, and 685–735 nm, respectively. The Commission International de l'Eclairage (CIE) 1931 chromaticity diagram of the LSYW doped Sm3+ at 405 nm is shown in the inset of Fig. 5. The obtained color coordinates are x = 0.60, y = 0.39, and are located in the reddish-orange area of the spectrum.
11/2) transitions whose wavelength ranges are 543–581 nm, 581–634 nm, 634–685 nm, and 685–735 nm, respectively. The Commission International de l'Eclairage (CIE) 1931 chromaticity diagram of the LSYW doped Sm3+ at 405 nm is shown in the inset of Fig. 5. The obtained color coordinates are x = 0.60, y = 0.39, and are located in the reddish-orange area of the spectrum.
In order to interpret the energy transfer mechanism, the critical distance (Rc) between two adjacent Sm3+ ions in the lattice must be calculated using the Blasse equation (eqn (2)):31
 | (2) |
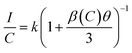 | (3) |
The activator concentration denotes the multipolar interaction constant equal to 6, 8, or 10, which, respectively, correspond to dipole–dipole(d–d), dipole-quadrupole(d–q), and quadrupole-quadrupole(q–q) interactions. Equivalence to Eqn (4) might also be written as:
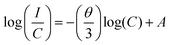 | (4) |
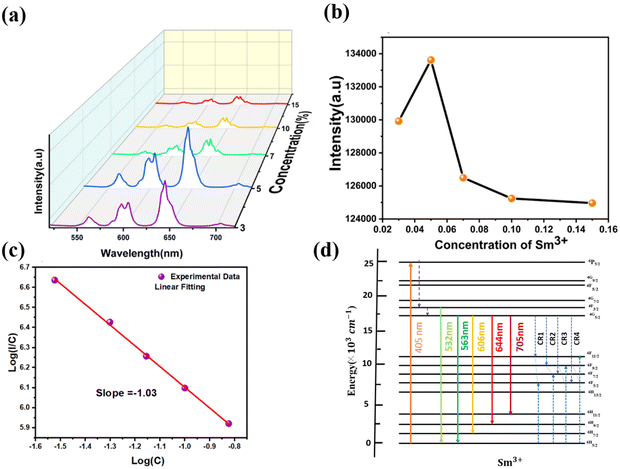
Fig. 6(c) shows the linear relationship between the log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) C and log(I/C) values of the LSYW doped xSm3+ (x = 0.03, 0.05,0.10 and 0.15) phosphors. The slope (−θ/3) was determined using linear fitting to be −1.03, (i.e., θ = 3). It is made clear by the estimated slope value and its value that energy is being transferred between the Sm3+ ions in the LSYW phosphors. LSYW doped with 0.05Sm3+ was used for investigating the optical temperature sensing properties. Fig. 6d illustrates the down-conversion luminescence mechanism of Sm3+ ions.36–39 By absorbing photon energy at 405 nm (24
C and log(I/C) values of the LSYW doped xSm3+ (x = 0.03, 0.05,0.10 and 0.15) phosphors. The slope (−θ/3) was determined using linear fitting to be −1.03, (i.e., θ = 3). It is made clear by the estimated slope value and its value that energy is being transferred between the Sm3+ ions in the LSYW phosphors. LSYW doped with 0.05Sm3+ was used for investigating the optical temperature sensing properties. Fig. 6d illustrates the down-conversion luminescence mechanism of Sm3+ ions.36–39 By absorbing photon energy at 405 nm (24![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 691 cm−1), Sm3+ ions in the 6H5/2 level (ground state) are excited to the 6P5/2 level (excited state). The Sm3+ ions in the excited state continuously proceed via NR processes to the 4F3/2 level. The population of 4G5/2 level increases when the majority of Sm3+ ions at 4F3/2 level non-radiatively relax to 4G5/2 level. Though the radiative transition process, the Sm3+ ions at the 4F3/2 and 4G5/2 levels relax to the 6H5/2 level, producing two emission bands with peak wavelengths at 531 nm (∼18
691 cm−1), Sm3+ ions in the 6H5/2 level (ground state) are excited to the 6P5/2 level (excited state). The Sm3+ ions in the excited state continuously proceed via NR processes to the 4F3/2 level. The population of 4G5/2 level increases when the majority of Sm3+ ions at 4F3/2 level non-radiatively relax to 4G5/2 level. Though the radiative transition process, the Sm3+ ions at the 4F3/2 and 4G5/2 levels relax to the 6H5/2 level, producing two emission bands with peak wavelengths at 531 nm (∼18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 832 cm−1) and 568 nm (∼17
832 cm−1) and 568 nm (∼17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 605 cm−1), respectively. Additionally, the Sm3+ ions in the 4G5/2 level have the ability to relax to the 6H7/2,
605 cm−1), respectively. Additionally, the Sm3+ ions in the 4G5/2 level have the ability to relax to the 6H7/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9/2,
9/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11/2 levels, emitting bands of light that are orange-red in color with peak wavelengths of 616 nm (∼16
11/2 levels, emitting bands of light that are orange-red in color with peak wavelengths of 616 nm (∼16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 234 cm−1), 651 nm (∼15
234 cm−1), 651 nm (∼15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 361 cm−1), and 710 nm (∼14
361 cm−1), and 710 nm (∼14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 084 cm−1), respectively. Regarding nearby Sm3+ ions, there could be four additional cross-relaxation processes as shown in the diagram below.
084 cm−1), respectively. Regarding nearby Sm3+ ions, there could be four additional cross-relaxation processes as shown in the diagram below.
5. Temperature sensing behavior
For the objective of digging out the optical thermometry features of the manufactured products, the typical temperature-dependent emission spectra of the examined samples with the optimal dopant concentration were determined. Fig. 7a displays down-conversion luminescence for LSYW:0.05Sm3+ measured under laser excitation 405 nm at 303–588 K temperature range. The integrated intensities of various emission bands are represented by IF, IG1, IG2, IG2−1, IG2−2, IG3 and IG4 to assist the research of the optical temperature sensing properties because the luminescence spectra of LSYW:0.05Sm3+ contain several emissions peaks.The integrated intensities of the 4F3/2 → 6H5/2 transition are represented by the band IF, whereas those of the 4G5/2 → 6H(5/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7/2,
7/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9/2,
9/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) and
and![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11/2) transitions are represented by IG1, IG2, IG3, and IG4, respectively as shown in Fig. 7b. The integrated intensities of two emission sub-bands from the 4G5/2 → 6H7/2 transition, with peak wavelengths of 598 nm and 607 nm, respectively, are represented by IG2−1 and IG2−2 at 303–588 K temperature range. As illustrated in Fig. 7b, the intensity of the fluorescence of the 4F3/2 → 6H5/2 transition is weak at low temperature; nevertheless, there is a monotonic increase in intensity as the temperature increases. Because of the thermal quenching effect, fluorescence intensities of the transitions 4G5/2 → 6H(5/2,
11/2) transitions are represented by IG1, IG2, IG3, and IG4, respectively as shown in Fig. 7b. The integrated intensities of two emission sub-bands from the 4G5/2 → 6H7/2 transition, with peak wavelengths of 598 nm and 607 nm, respectively, are represented by IG2−1 and IG2−2 at 303–588 K temperature range. As illustrated in Fig. 7b, the intensity of the fluorescence of the 4F3/2 → 6H5/2 transition is weak at low temperature; nevertheless, there is a monotonic increase in intensity as the temperature increases. Because of the thermal quenching effect, fluorescence intensities of the transitions 4G5/2 → 6H(5/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 7/2,
7/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 9/2,
9/2,![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11/2) decrease as temperature increases.
11/2) decrease as temperature increases.
The temperature dependence of FIR of IF/IG2 could be expressed by the Boltzmann's distribution rule as follows (eqn (5)):40
 | (5) |
The rate at which the FIR changes in response to changes in temperature is known as the absolute sensitivity (Sa). Consequently, the Sa can be written as (eqn (6)).41–43
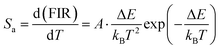 | (6) |
 | (7) |
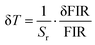 | (8) |
| Materials | Ratio | Temperature range (K) |
S
a![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) max (K−1) max (K−1) |
S
r![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) max (%K−1) max (%K−1) |
Ref. |
|---|---|---|---|---|---|
| YNbO4:Sm3+ | 4F3/2, 4G5/2 → 6H5/2 | 303–773 | — | 0.43 (500 K) | 49 |
| Lu2O3:Sm3+ | 4G5/2 → 6H5/2, 6H9/2 | 293–873 | — | 0.36 (580 K) | 50 |
| Ca2LaNbO6:0.05Sm3+ | 4G5/2 → (6H5/2, 6H7/2) | 313–573 | — | 0.23 (353 K) | 51 |
| 4G5/2 → (6H5/2, 6H9/2) | — | 0.22 (313 K) | |||
| GdVO4:Sm3+ | 4F3/2/4G5/2 → 6H5/2 (IF/IG1) | 293–823 | 4.5 × 10−4 (750 K) | — | 52 |
| TZPN:0.5Sm3+ | 4G5/2 → 6H5/2/4G5/2 → 6H7/2 | 300–725 | — | 0.31 (700 K) | 53 |
| YVO4:Sm3+ | — | 299–466 | — | 0.36 (466 K) | 54 |
| YAG:Sm3+ | I F/IG1 | 303–1178 | 3.046 × 10−4 (1129 K) | 0.5033 (500 K) | 55 |
| YAG:Sm3+ | I G2–1/IG2–2 | 303–1028 | 5.017 × 10−4 (300 K) | 0.1567 (303 K) | 55 |
| LSYW:0.05Sm3+ | I F/IG2 | 301–583 | 1.32 × 10−5 (583 K) | 1.58 (301 K) | This work |
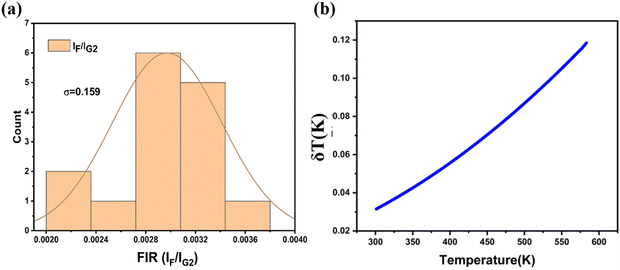 | ||
| Fig. 8 (a) Standard deviations at RT for FIR (IF/IG2) obtained using 15 measurements at 300 K. (b) Temperature resolution values δT obtained for IF/IG2. | ||
The Commission International de l'Eclairage (CIE) coordinates of phosphors (303–583 K) were obtained in order to more clearly see the change in luminescence color in the phosphors. Fig. 9 shows the sample's CIE chromaticity points at different temperature values. From 203 K to 583 K, there is a change in the colour coordinate from x = 0.45, y = 0.36 to x = 0.39, y = 0.35. This phenomenon results from the fact that when temperatures rise, the intensity of the red emission increases. According to these findings, Sm3+ doped LiSrYW3O12 phosphor may be a good option to replace the orange component in an orange light-emitting diode.
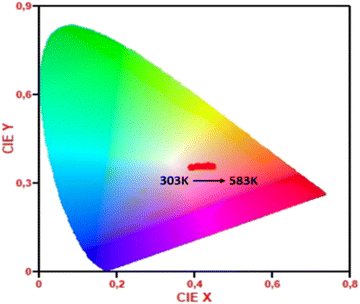 | ||
| Fig. 9 CIE coordinates changes of LSYW:Sm3+ with respect to temperature from 303 to 583 K under 405 nm excitation. | ||
6. Conclusions
LiSrYW3O12 (LSYW):xSm3+ (x = 0.03, 0.05, 0.07, 0.1, and 0.15) phosphors were synthesized through a high-temperature solid-state reaction, yielding samples with a tetragonal crystal structure, as validated by X-ray diffraction (XRD) analysis. The band gaps of the LSYW:xSm3+ samples decrease with increasing Sm3+ doping concentrations, ranging from 3.31 to 3.19 eV. Analysis based on the enhanced Van Uitert model and Dexter's theory revealed that D–D interactions primarily contribute to the concentration-dependent luminescence quenching of Sm3+ ions, particularly for doping concentrations exceeding 5%. Exploration of the optical temperature sensing properties within the range of 301–583 K revealed notable trends: the fluorescence intensity of the 4F3/2 → 6H5/2 transition exhibited a significant increase with rising temperature, while the fluorescence intensities of the 4G5/2 → 6H7/2 transition gradually diminished. Maximum sensitivity ratio values (Sr) were determined to be 1.58% K−1 (301 K) for 4F3/2 → 6H5/2/4G5/2 → 6H7/2 (IF/IG2). The precision of luminescence thermometry is highlighted by the calculated temperature uncertainty (δT) of less than 1 K, with a minimum at 0.03 K for the IF/IG2 ratio corresponding to the 4F3/2 → 6H5/2/4G5/2 → 6H7/2 (IF/IG2) transitions, underscoring its accuracy and potential as a high-precision optical sensing technique. These findings suggest that Sm3+ ions hold promise as optically active centers for luminescence thermometry applications, particularly employing FIR techniques. These results pave the way for the development of luminescent thermometric materials capable of delivering precise temperature information across a broad range of conditions, including demanding and complex scenarios.Conflicts of interest
There are no conflicts to declare.References
- M. Pieprz, W. Piotrowski, P. Woźny, M. Runowski and L. Marciniak, Adv. Opt. Mater., 2024, 12, 2301316 CrossRef CAS.
- C. Hernández-Álvarez, K. Soler-Carracedo, P. Woźny, I. R. Martín and M. Runowski, J. Mater. Chem. C, 2024, 12, 7707–7714 RSC.
- M. Szymczak, P. Woźny, M. Runowski, M. Pieprz, V. Lavín and L. Marciniak, Chem. Eng. J., 2023, 453, 139632 CrossRef CAS.
- K. Saidi, W. Chaabani and M. Dammak, RSC Adv., 2021, 11, 30926–30936 RSC.
- Z. E. A. A. Taleb, K. Saidi and M. Dammak, RSC Adv., 2024, 14, 8366–8377 RSC.
- Q. Zhang, J. Wang, M. Zhang, W. Ding and Q. Su, J. Rare Earths, 2006, 24, 392–395 CrossRef.
- L. Yu, F. Hong, Y. Wang, H. Xu, G. Liu, X. Dong, J. Wang and W. Yu, J. Lumin., 2020, 222, 117155 CrossRef CAS.
- E. Cavalli, F. Angiuli, I. Kebaïli, M. Dammak, P. Boutinaud and M. Bettinelli, J. Lumin., 2013, 143, 562–565 CrossRef CAS.
- I. Kebaïli and M. Dammak, J. Lumin., 2012, 132, 2092–2097 CrossRef.
- J. Sun, X. Zhang, Z. Xia and H. Du, J. Appl. Phys., 2012, 111, 013101 CrossRef.
- I. Charak, M. Manhas, A. K. Bedyal, H. C. Swart and V. Kumar, Inorg. Chem. Commun., 2023, 160, 111885 CrossRef.
- G. Annadurai, S. M. M. Kennedy and V. Sivakumar, J. Rare Earths, 2016, 34, 576–582 CrossRef CAS.
- R. Cao, G. Quan, Z. Shi, T. Chen, Z. Luo, G. Zheng and Z. Hu, J. Phys. Chem. Solids, 2018, 118, 109–113 CrossRef CAS.
- C. Liao, R. Cao, W. Wang, W. Hu, G. Zheng, Z. Luo and P. Liu, Mater. Res. Bull., 2018, 97, 490–496 CrossRef CAS.
- G. Fan, Z. Tian, X. Wang, S. Tang and Y. Chen, J. Mater. Sci.: Mater. Electron., 2018, 29, 17681–17688 CrossRef CAS.
- K. Saidi, I. Kachou, K. Soler-Carracedo, M. Dammak and I. R. Martín, ACS Appl. Nano Mater., 2023, 6(19), 17681–17690 CrossRef CAS.
- J. Fan, H. Zhang, J. Wang, Z. Ling, H. Xia, X. Chen, Y. Yu, Q. Lu and M. Jiang, J. Phys. Appl. Phys., 2006, 39, 1034–1041 CrossRef CAS.
- P. A. Loiko, V. I. Dashkevich, S. N. Bagaev, V. A. Orlovich, A. S. Yasukevich, K. V. Yumashev, N. V. Kuleshov, E. B. Dunina, A. A. Kornienko, S. M. Vatnik and A. A. Pavlyuk, J. Lumin., 2014, 153, 221–226 CrossRef CAS.
- Z. Liu, Q. Meng, H. Liu, C. Yao, Q. Meng, W. Liu and W. Wang, Opt. Mater., 2013, 36, 384–389 CrossRef CAS.
- Y. Yin, Y.-N. Li, S. Liu, Y. Jiang, X.-Y. Liu and P. Zhang, ACS Omega, 2024, 9, 10517–10521 CrossRef CAS PubMed.
- C. S. Lim, Mater. Res. Bull., 2016, 75, 211–216 CrossRef CAS.
- C. S. Lim, A. Aleksandrovsky, M. Molokeev, A. Oreshonkov and V. Atuchin, Phys. Chem. Chem. Phys., 2015, 17, 19278–19287 RSC.
- N. Xue, X. Fan, Z. Wang and M. Wang, J. Phys. Chem. Solids, 2008, 69, 1891–1896 CrossRef CAS.
- C. Ming, F. Song and L. Yan, Opt. Commun., 2013, 286, 217–220 CrossRef CAS.
- L. Li, L. Liu, W. Zi, H. Yu, S. Gan, G. Ji, H. Zou and X. Xu, J. Lumin., 2013, 143, 14–20 CrossRef CAS.
- C. S. Lim, V. V. Atuchin, A. S. Aleksandrovsky and M. S. Molokeev, Mater. Lett., 2016, 181, 38–41 CrossRef CAS.
- F. Ayachi, K. Saidi, M. Dammak, J. Josep Carvajal and M. Cinta Pujol, RSC Adv., 2024, 14, 13494–13504 RSC.
- K. Saidi and M. Dammak, J. Solid State Chem., 2021, 300, 122214 CrossRef CAS.
- Z. E. A. A. Taleb, K. Saidi, M. Dammak, D. Przybylska and T. Grzyb, Dalton Trans., 2023, 52, 4954–4963 RSC.
- K. Raju, C. G. Balaji and P. Venugopal Reddy, J. Magn. Magn. Mater., 2014, 354, 383–387 CrossRef CAS.
- G. Blasse, J. Solid State Chem., 1986, 62, 207–211 CrossRef CAS.
- Y. Tian, Y. Liu, R. Hua, L. Na and B. Chen, Mater. Res. Bull., 2012, 47, 59–62 CrossRef CAS.
- G. S. Ofelt, J. Chem. Phys., 2004, 37, 511–520 CrossRef.
- Z. Yahiaoui, M. A. Hassairi, M. Dammak and E. Cavalli, J. Alloys Compd., 2018, 763, 56–61 CrossRef CAS.
- F. Ayachi, K. Saidi, W. Chaabani and M. Dammak, J. Lumin., 2021, 240, 118451 CrossRef CAS.
- W. Ryba-Romanowski, A. Strzęp, R. Lisiecki, M. Berkowski, H. Rodriguez-Rodriguez and I. R. Martin, Opt. Mater. Express, 2014, 4, 739–752 CrossRef.
- S. Q. Mawlud, M. M. Ameen, Md. R. Sahar, Z. A. S. Mahraz and K. F. Ahmed, Opt. Mater., 2017, 69, 318–327 CrossRef CAS.
- A. Mohan Babu, B. C. Jamalaiah, T. Sasikala, S. A. Saleem and L. Rama Moorthy, J. Alloys Compd., 2011, 509, 4743–4747 CrossRef CAS.
- S. Karki, C. R. Kesavulu, H. J. Kim, J. Kaewkhao, N. Chanthima and Y. Ruangtaweep, J. Lumin., 2018, 197, 76–82 CrossRef CAS.
- K. Saidi, C. Hernández-Álvarez, M. Runowski, M. Dammak and I. R. Martín, Dalton Trans., 2023, 52, 14904–14916 RSC.
- F. Huang and D. Chen, J. Mater. Chem. C, 2017, 5, 5176–5182 RSC.
- H. Zhou, N. An, K. Zhu, J. Qiu, L. Yue, L.-G. Wang and L. Ye, J. Lumin., 2021, 229, 117656 CrossRef CAS.
- Y. Gao, F. Huang, H. Lin, J. Zhou, J. Xu and Y. Wang, Adv. Funct. Mater., 2016, 26, 3139–3145 CrossRef CAS.
- F. Ayachi, K. Saidi, M. Dammak, W. Chaabani, I. Mediavilla-Martínez and J. Jiménez, Mater. Today Chem., 2023, 27, 101352 CrossRef CAS.
- N. An, L. Ye, R. Bao, L. Yue and L.-G. Wang, J. Lumin., 2019, 215, 116657 CrossRef CAS.
- J. Rocha, C. D. S. Brites and L. D. Carlos, Chem. – Eur. J., 2016, 22, 14782–14795 CrossRef CAS PubMed.
- F. Ayachi, K. Saidi, K. Soler-Carracedo, M. Dammak and I. R. Martín, J. Alloys Compd., 2023, 961, 171146 CrossRef CAS.
- J.-C. G. Bunzli and V. K. Pecharsky, Handbook on the Physics and Chemistry of Rare Earths: Including Actinides, Elsevier, 2016 Search PubMed.
- L. R. Đačanin, S. R. Lukić-Petrović, D. M. Petrović, M. G. Nikolić and M. D. Dramićanin, J. Lumin., 2014, 151, 82–87 CrossRef.
- V. Lojpur, Ž. Antić, R. Krsmanović, M. M. Medić, M. G. Nikolić and M. Dramićanin, J. Serbian Chem. Soc., 2012, 77, 1735–1746 CrossRef CAS.
- A. Zhang, Z. Sun, M. Jia, Z. Fu, B. C. Choi, J. H. Jeong and S. H. Park, J. Alloys Compd., 2021, 889, 161671 CrossRef.
- M. G. Nikolić, D. J. Jovanović, V. D. Jordjević, Ž. Antić, R. M. Krsmanović and M. D. Dramićanin, Phys. Scr., 2012, 014063 CrossRef.
- B. Klimesz, R. Lisiecki and W. Ryba-Romanowski, J. Alloys Compd., 2019, 788, 658–665 CrossRef CAS.
- I. E. Kolesnikov, E. V. Golyeva, M. A. Kurochkin, E. Y. Kolesnikov and E. Lähderanta, J. Lumin., 2020, 219, 116946 CrossRef CAS.
- K. Zhu, H. Zhou, J. Qiu, L.-G. Wang and L. Ye, J. Alloys Compd., 2022, 890, 161844 CrossRef CAS.
| This journal is © The Royal Society of Chemistry 2024 |