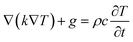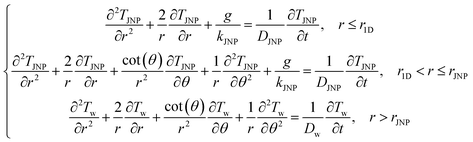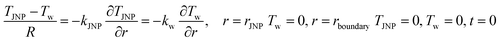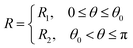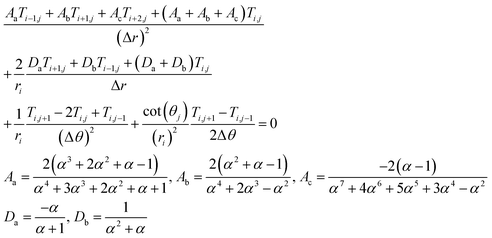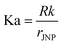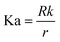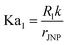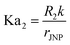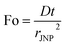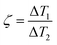DOI:
10.1039/D3NA00781B
(Paper)
Nanoscale Adv., 2024,
6, 3082-3092
Regulating nanoscale directional heat transfer with Janus nanoparticles†
Received
14th September 2023
, Accepted 25th April 2024
First published on 30th April 2024
Abstract
Janus nanoparticles (JNPs) with heterogeneous compositions or interfacial properties can exhibit directional heating upon external excitation with optical or magnetic energy. This directional heating may be harnessed for new nanotechnology and biomedical applications. However, it remains unclear how the JNP properties (size, interface) and laser excitation method (pulsed vs. continuous) regulate the directional heating. Here, we developed a numerical framework to analyze the asymmetric thermal transport in JNP heating under photothermal stimulation. We found that JNP-induced temperature contrast, defined as the ratio of temperature increase on the opposite sides in the surrounding medium, is highest for smaller JNPs and when a low thermal resistance coating covers a minor fraction of JNP surface. Notably, we discovered up to 20-fold enhancement of the temperature contrast based on thermal confinement under pulsed heating compared with continuous heating. This work brings new insights to maximize the asymmetric thermal responses for JNP heating.
Introduction
Nanoparticle (NP) heating has enabled many applications,1–4 including cancer treatment,5–9 neuromodulation,10,11 molecular hyperthermia,12–15 point-of-care diagnosis,16–18 contrast agents,19,20 controlled release,21 energy harvesting,22,23 and catalysis.24,25 Janus nanoparticles (JNPs) refer to nanostructures with asymmetric structures, and have recently brought new opportunities to the field.26–28 Two types of JNPs have been widely studied recently: the first type of JNPs consists of heterogeneous materials, such as combinations of metallic and non-metallic materials;29–31 whereas the second type of JNPs is asymmetrically coated with different ligands, such as hydrophobic versus hydrophilic coatings.32 Upon laser excitation, the asymmetric structures of JNPs can lead to heterogeneous heating source,33 or nonuniform NP–water interfacial thermal resistances (ITRs).34 These asymmetries have been harnessed to regulate the thermal transport around the JNP and lead to directional heating.34–37 Directional heating with the first type of JNPs has enabled novel applications with JNPs, such as thermophoretic nanomotors,33,35,38 nano tweezers,39,40 Janus nano pen injection,41 and energy management.42
For the second type of JPNs, recent theoretical studies have shown the possibility of directional heating with significantly smaller (∼5 nm) than the first type JNPs (50–200 nm),34 thus promising to open the new size range for potential applications. The central issue for JNP heating is a better understanding of thermal transport and temperature regulation.40 Olarte-Plata et al. was among the first to analyze the directional heating of JNPs with heterogeneous coating and ITRs under continuous heating.34 Using molecular dynamics (MD) simulation and continuous modelling, they demonstrated a temperature difference up to 10 K with hydrophilic/hydrophobic (octanethiolate/mercaptohexanol)-coated JNPs and estimated the temperature difference with a wide range of ITR combinations (0.1–100 × 109 m2 K W−1) and sizes (5–100 nm, continuous modeling). Jiang et al. further utilized MD simulation to provide an atomic resolution of ITR variations of Au–water interface and demonstrate a temperature difference on the order of 5 K for with 20–30 nm NPs with 1 μW heating per NP.43 While these studies have greatly advanced our knowledge of directional heating with JNP, several key questions remain unclear, specially how the distribution of heterogeneous ITR on JNP and the laser excitation duration (continuous vs. pulsed) regulate the nanoscale thermal transport.
This work focuses on elucidating how the heterogeneous interface properties (ITRs), the area fraction of heterogeneous ITRs, and the laser excitation method (continuous vs. pulsed) regulate the nanoscale heat transfer (Fig. 1A) for JNPs with gold core and asymmetric surface coatings. Here we developed a numerical framework based on continuous models as it enables us scanning a wide range of parameter space, especially for the pulsed heating (transient process) scenarios. We defined a dimensionless parameter based on the ratio of asymmetric temperature increase (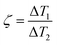 , Fig. 1B) to quantify the magnitude of directional heating. Our modeling results suggest that increasing JNP size reduces the temperature contrast, additionally, an approximate solution is provided to estimate the JNP size-dependent temperature contrast. Furthermore, the distribution of interface thermal resistance (varying the surface coverage of low versus high interface thermal resistance) has a significant impact on the temperature contrast, with a minor coverage by low interface resistance leading to higher temperature contrast. Importantly, we demonstrate a significant enhancement of temperature contrast with pulsed JNP heating compared with continuous heating. Our work advances the understanding of directional heating with JNP by clarifying the contributions of various factors to JNP nanoscale thermal transport.
, Fig. 1B) to quantify the magnitude of directional heating. Our modeling results suggest that increasing JNP size reduces the temperature contrast, additionally, an approximate solution is provided to estimate the JNP size-dependent temperature contrast. Furthermore, the distribution of interface thermal resistance (varying the surface coverage of low versus high interface thermal resistance) has a significant impact on the temperature contrast, with a minor coverage by low interface resistance leading to higher temperature contrast. Importantly, we demonstrate a significant enhancement of temperature contrast with pulsed JNP heating compared with continuous heating. Our work advances the understanding of directional heating with JNP by clarifying the contributions of various factors to JNP nanoscale thermal transport.
 |
| | Fig. 1 Schematic illustration of the JNP heating modeling. (A) A spherical Janus nanoparticle (JNP) with gold core is heterogeneously coated, leading to nonuniform interfacial thermal resistance (ITR). The two ITRs on the JNP are R1, covering the north pole, and R2, covering the south pole. The polar angle (θ0) defines the boundary between two different coatings and determines the fraction of the low thermal resistance coating vs. high thermal resistance coating. Continuous and pulsed heating was imposed on the JNP to model the steady-state and transient heating scenarios. (B) Schematic of the model under spherical coordinates. ΔT1 and ΔT2 indicate the temperature change in the water. | |
Results
Directional heating and temperature contrast under continuous heating
We first developed a numerical framework with finite difference methods (FDM) based on the continuous modeling of thermal transport (Fourier's law) to simulate thermal transport with the second type of JPN, which consists of a gold core and asymmetric coatings (Fig. 1A). It should be noted that continuous modeling are sufficiently accurate for estimating the JNP heating in an aqueous solution as the mean free path of phonon in the water (∼0.3 nm) is orders of magnitude smaller than the characteristic length in this work (5–100 nm).14,44,45 Additionally, a recent study has shown that results from continuous modeling are in well consistence with the MD simulation even for small JNP (5 nm) heating.34 We adopted constant thermal thermophysical properties (c, ρ, k) as we have shown in our previous work that temperature-dependent thermophysical properties have limited effect on the temperature profile compared that with the constant properties.14 The model (Fig. S1†) was vigorously validated including mesh independence and boundary effect analysis (ESI section “FDM model validation” and Fig. S2–S4†). To quantify the temperature contrast for JNP heating, we first define a dimensionless parameter:| | 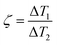 | (1) |
where ΔT1 and ΔT2 indicate the representative temperature rise of the two coating parts (Fig. 1B). When ζ = 1, it indicates no temperature contrast or homogeneous heating, whereas when ζ < 1 or ζ > 1, it indicates directional heating.
Several factors can affect the thermal transport and temperature profile during JNP heating, including the interfacial thermal resistance (ITR), the JNP size (rJNP), the fraction of the low thermal resistance coating vs. high thermal resistance coating (as quantified by the polar angle θ0 of the heterogeneous coatings, Fig. 1A), and the heating method (i.e., continuous heating vs. pulsed heating, can be quantified by heating time, t). First, we analyzed JNP heating under continuous heating and the simplest JNP with a symmetric geometry (polar angle θ0 = π/2). Nonuniform interfacial properties can stem from the heterogeneous coating, including hydrophilicity that leads to different ITR values, and the contrast between ITRs can be tuned by altering the combination of surface coating materials or ligands.46–48 Since the ITR values for NPs in an aqueous solution reported are in the order of 1–100 × 10−9 m2 K W−1 (Table S1†), or interfacial conductance: 0.01–1 × 109 W m−2 K−1,49–53 we will set our parameter range for ITRs as 1–100 × 10−9 m2 K W−1 throughout the paper. Fig. 2A shows that for a given JNP size (rJNP = 15 nm), the combination of R1 and R2 significantly affects the temperature profile and temperature contrasts (ζ). With uniform ITR (case 1, R1 = R2 = 50 × 10−9 m2 K W−1), the temperature increase is uniform around the nanoparticle. With the increase in the ITR contrast (cases 2–4), we observed a higher temperature contrast, referred to as directional heating, in the surrounding water near the north pole and south pole (Fig. 2A). The maximum ITR ratio (R2/R1 = 100) gives a temperature contrast ζ = 2.5 (case 4). It should be noted that a uniform temperature profiled is observed inside JNP across all the combinations of the ITRs as the thermal conductivity and diffusivity of gold is much higher than those of water. To gain a complete picture of the temperature contrast as a function of ITRs, we constructed the 2D map of ζs.s. as a function of R1 and R2 (Fig. 2B), where the subscript s.s. indicates steady-state (i.e. continuous heating). The range for ITRs in Fig. 2B (1–100 × 10−9 m2 K W−1) is consistent with ITRs reported in the literature.14ζs.s. equals to 1 along the diagonal where R1 = R2 while ζs.s. deviates from 1 in the off-diagonal region (when R1 ≠ R2). Fig. 2B is symmetric along the diagonal line since exchanging the R1 and R2 should lead to the inverse of ζs.s..
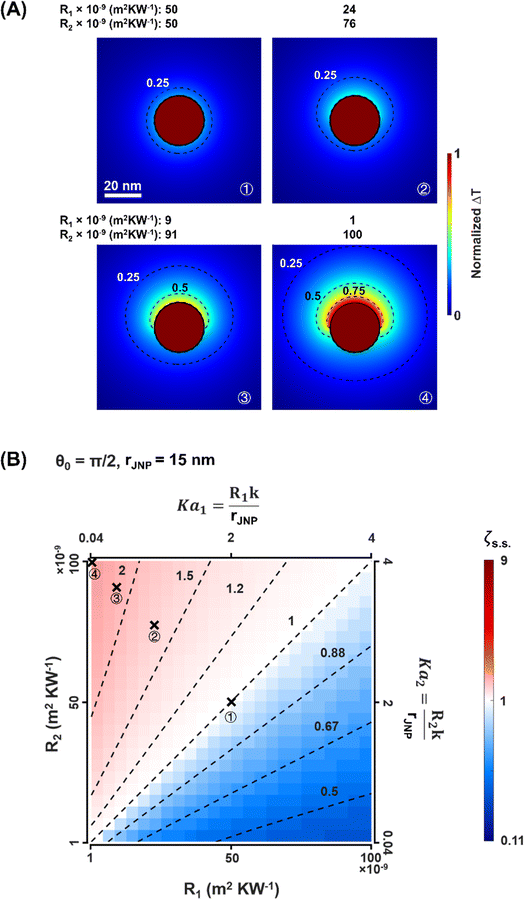 |
| | Fig. 2 Temperature profile and contrast with Janus NP heating under the steady-state. (A) Normalized temperature rise (ΔT) with Janus nanoparticle (JNP) heating. The JNP size (rJNP) is 15 nm, and the polar angle for the heterogeneous coating (θ0) is π/2. (B) 2D map of ζs.s. in terms of the two ITRs, R1, and R2. Dotted lines are contour lines for ζs.s.. The Kapitza number (Ka) is defined as: 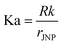 , where R is the interfacial thermal resistance, k is the thermal conductivity of the media, rJNP is the radius of JNP. , where R is the interfacial thermal resistance, k is the thermal conductivity of the media, rJNP is the radius of JNP. | |
To further investigate how the combination of ITRs affects ζs.s., we developed a parallel circuit model (ESI section “Parallel circuit model”†) to analyze the JNP heating analytically. Here we assume no heat transfer across the polar angle (θ0) of heterogeneous ITR in the water domain, and we simplified the model to a parallel circuit analogy (Fig. S5A†) with a set of linear equations to describe the temperature at the critical nodes (eqn (S2) and (S3)†). Based on this parallel circuit model, the temperature contrast under the steady-state can be estimated by:
| | 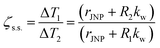 | (2) |
Comparing results from the parallel circuit model with the FDM results (Fig. S5B†), the parallel circuit model overestimates ζs.s. and is more accurate with larger JNPs. This overestimation may arise from the assumption of no heat transfer across θ0. The agreement between the numerical simulation and parallel circuit model improves for larger JNPs because heat flux in the θ direction becomes less important compared with the radial heat flux. Furthermore, eqn (2) demonstrates the size dependence of temperature contrast with JNP heating. For a given combination of R1 and R2, larger JNP size will yield less temperature contrast. This size dependence agrees with Kapitza number analysis,52,54 where for larger NP, the ITR has a smaller effect on the heat dissipation 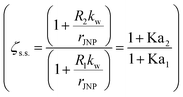 . This size dependence of ζs.s. is also confirmed with the FDM model, and the ζs.s maps with rJNP ranging from 3.75 to 60 nm (Fig. S6†).
. This size dependence of ζs.s. is also confirmed with the FDM model, and the ζs.s maps with rJNP ranging from 3.75 to 60 nm (Fig. S6†).
Effect of JNP size and asymmetric coating on the temperature contrast under steady-state
Next, we analyzed how the asymmetric surface coating impacts the temperature contrast. Under experimental conditions, the surface coating can be highly asymmetric with a major or minor fraction of the low thermal resistance coating vs. high thermal resistance coating, i.e., θ0 deviates from π/2 (Fig. S7†).31,55 Here, we investigated how the asymmetric coating impacts the temperature contrast by examining a range of polar angle θ0 values (Fig. 3A). Fig. 3B shows that the asymmetric coating strongly affects the local heat flux rate across the interface (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) ). The local heat flux at the north pole (
). The local heat flux at the north pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1) is significantly higher (∼50-fold at θ0 = 0.02π) than that of the south pole (
1) is significantly higher (∼50-fold at θ0 = 0.02π) than that of the south pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2) when θ0 < π/2. The difference between
2) when θ0 < π/2. The difference between ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1 and
1 and ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2 with smaller θ0 leads to a remarkably higher ΔT1 (Fig. 3C) than ΔT2. We further examined the asymmetric coating for different JNP sizes (Fig. 3D). Our results suggest that smaller JNP size gives greater temperature contrast (e.g., ζs.s. = 10 for a 3 nm JNP with θ0 ∼ π/12, area fraction for low thermal resistance ∼3%).
2 with smaller θ0 leads to a remarkably higher ΔT1 (Fig. 3C) than ΔT2. We further examined the asymmetric coating for different JNP sizes (Fig. 3D). Our results suggest that smaller JNP size gives greater temperature contrast (e.g., ζs.s. = 10 for a 3 nm JNP with θ0 ∼ π/12, area fraction for low thermal resistance ∼3%).
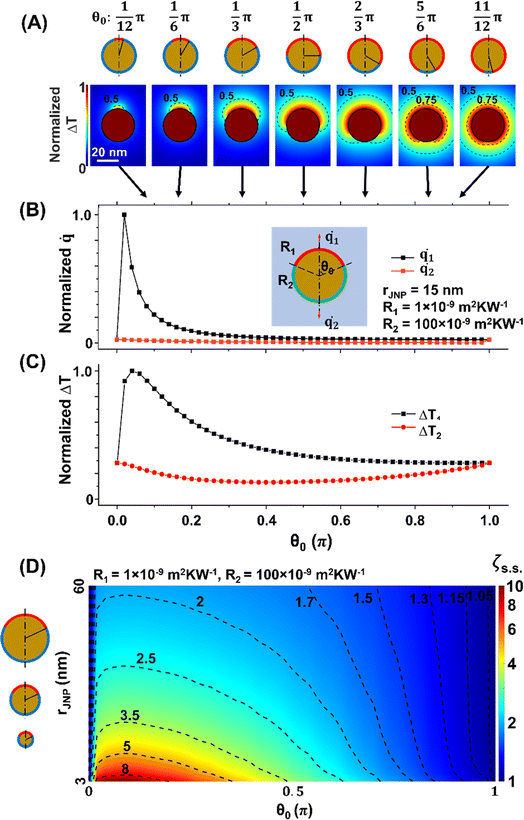 |
| | Fig. 3 Effect of the polar angle (θ0) on the temperature contrast under steady-state. (A) Temperature profile of JNP heating with different θ0. R1 = 1 × 10−9 m2 K W−1 and R2 = 100 × 10−9 m2 K W−1, rJNP = 15 nm. (B) The local heat flux rate across the interface at the northern pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1) and southern pole ( 1) and southern pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2) as a function of θ0. (C) ΔT1 and ΔT2 as a function of θ0. (D) ζs.s. map as a function of rJNP and θ0. R1 = 1 × 10−9 m2 K W−1, R2 = 100 × 10−9 m2 K W−1. The dotted lines are contour lines for ζs.s.. 2) as a function of θ0. (C) ΔT1 and ΔT2 as a function of θ0. (D) ζs.s. map as a function of rJNP and θ0. R1 = 1 × 10−9 m2 K W−1, R2 = 100 × 10−9 m2 K W−1. The dotted lines are contour lines for ζs.s.. | |
Directional heating during pulsed heating
Recently, NP heating with femtosecond to nanosecond pulsed energy excitation has shown unique thermal responses and has played essential roles in various applications.12,15,56 As the characteristic thermal relaxation time is on the order of nanoseconds (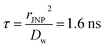 for 30 nm JNP), JNP heating with nanosecond or shorter pulsed heating is far from steady-state and can lead to thermal confinement. Here we imposed 100 ns laser pulse (constant heating) on a 30 nm JNP with R2/R1 = 100 and θ0 = π/2. Fig. 4A illustrates the temperature profiles during the transient heating process. At t = 1 ns, only a thin layer of water adjacent to the JNP–water interface is heated along the northern hemisphere (ΔT ∼ 200 K). On the contrary, minimal heating is observed near the southern hemisphere (ΔT ∼ 25 K) due to higher ITR. By comparing the local heat flux at the north pole and south pole (
for 30 nm JNP), JNP heating with nanosecond or shorter pulsed heating is far from steady-state and can lead to thermal confinement. Here we imposed 100 ns laser pulse (constant heating) on a 30 nm JNP with R2/R1 = 100 and θ0 = π/2. Fig. 4A illustrates the temperature profiles during the transient heating process. At t = 1 ns, only a thin layer of water adjacent to the JNP–water interface is heated along the northern hemisphere (ΔT ∼ 200 K). On the contrary, minimal heating is observed near the southern hemisphere (ΔT ∼ 25 K) due to higher ITR. By comparing the local heat flux at the north pole and south pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1 and
1 and ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2), both the magnitude and slope for
2), both the magnitude and slope for ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1(t) are significantly higher than those for
1(t) are significantly higher than those for ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2(t) for t < 1 ns, leading to enhanced temperature contrast (Fig. 4B–D, ζ ∼ 20 at 0.1 ns). Here we adopted the Fourier's number (
2(t) for t < 1 ns, leading to enhanced temperature contrast (Fig. 4B–D, ζ ∼ 20 at 0.1 ns). Here we adopted the Fourier's number (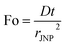 , D: thermal diffusivity, t: time) as dimensionless time. As heating continues, thermal energy continuously diffuses and leads to reduced temperature contrast. At t = 100 ns, the maximum temperature is reached as it is the end time point for the pulsed heating and the heating asymptotically approaches the steady-state value (Fig. 4B, Fo = 63.5), and the temperature contrast ζ asymptotically falls to its steady-state level (Fig. 4C, ζs.s. = 2.5). After the pulsed heating (t > 100 ns), the whole system cools down rapidly, and both ΔT1 and ΔT2 drop to 0 with ζ approaching 1. Therefore, the thermal confinement under short ns pulse heating (Fo ≪ 1) leads to a 8-fold enhancement of temperature contrast under current conditions (Fo = 0.06, R2/R1 = 100, rJNP = 15 nm, θ0 = π/2).
, D: thermal diffusivity, t: time) as dimensionless time. As heating continues, thermal energy continuously diffuses and leads to reduced temperature contrast. At t = 100 ns, the maximum temperature is reached as it is the end time point for the pulsed heating and the heating asymptotically approaches the steady-state value (Fig. 4B, Fo = 63.5), and the temperature contrast ζ asymptotically falls to its steady-state level (Fig. 4C, ζs.s. = 2.5). After the pulsed heating (t > 100 ns), the whole system cools down rapidly, and both ΔT1 and ΔT2 drop to 0 with ζ approaching 1. Therefore, the thermal confinement under short ns pulse heating (Fo ≪ 1) leads to a 8-fold enhancement of temperature contrast under current conditions (Fo = 0.06, R2/R1 = 100, rJNP = 15 nm, θ0 = π/2).
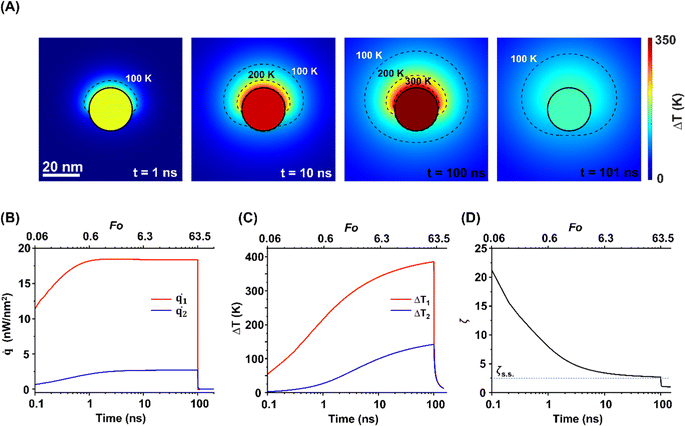 |
| | Fig. 4 The temperature evolution during the transient heating. (A) ΔT profiles during the transient heating process with JNP. rJNP = 15 nm, heating power (g) = 35.6 μW, heating time (theating) = 100 ns. R1 = 1 × 10−9 m2 K W−1, R2 = 100 × 10−9 m2 K W−1, θ0 = π/2. (B) Temporal evolution of local heat flux rate (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) )at the north pole and south pole, (C) ΔT and ΔT2 and (D) ζ during the transient heating process. The blue dotted line in (D) indicates the corresponding ζs.s.. )at the north pole and south pole, (C) ΔT and ΔT2 and (D) ζ during the transient heating process. The blue dotted line in (D) indicates the corresponding ζs.s.. | |
Effect of JNP size and asymmetric coating on the temperature contrast during the transient heating
Lastly, we investigated how the JNP size and asymmetric impacts the temperature contrast under pulsed heating. We started by analyzing JNP size (rJNP) on the temperature contrast ζ and observe a strong size dependence (Fig. 5A). Smaller JNPs are more sensitive to heating time and hence have a stronger enhanced temperature contrast with pulsed heating (Fo = 0.06) compared with steady-state scenarios (14-fold enhancement for rJNP = 3 nm and 4-fold for rJNP = 60 nm). As shown in Fig. 5B, analysis of the asymmetric coating shows a strong enhancement for temperature contrast (13-fold) for a moderate θ0 (π/12 < θ0 < 3π/4) at short heating times (Fo < 1). Lastly, Fig. 5C demonstrates ζ in terms of ITR contrast and heating time and suggests that for high ITR contrast (R2/R1 > 25), the temperature contrast is significantly enhanced with short heating time (ζ ∼ 12–20 when Fo ∼ 0.1). Whereas for low ITR contrast (R2/R1 < 25), ζ is less sensitive to heating time, and for minimal ITR contrast (R2/R1 ∼ 1–2), ζ approximately keeps constant (ζ ∼ 1.5) throughout the transient process. In conclusion, our analysis gives a clear landscape of the transient temperature contrast due to JNP size (rJNP) and ITR distribution (θ0, R2/R1). Specifically, smaller-sized JNP with large ITR contrast and moderate polar angle has the maximal temperature contrast under pulsed heating.
 |
| | Fig. 5 Effect of thermal resistance (R2/R1), asymmetric coating (θ0), and particle size (rJNP) on the temperature contrast during the transient heating. (A) ζ map as a function of rJNP and Fo. (B) ζ map as a function of θ0 and t. (C) ζ map as a ITR ratio and heating time (t). Here we regulate R2 + R1 = 100 m2 K W−1 and alter the R2/R1. The dotted lines are contour lines for ζ. | |
Discussion
Directional heating with the first kind of JNP
In this work, we focused on directional heating with the second type of Janus NP characterized by heterogeneous interfacial thermal resistances (ITRs). One key result from our model is a much-enhanced temperature contrast (ζ) with ultra-short pulsed heating compared with that of continuous heating. However, the mechanism behind this enhanced ζ remains elusive due to the formidable challenge of solving the analytical solution for the temperature function. We propose a potential explanation for the enhanced ζ observed during pulsed heating: the thermal confinement due to short heating time, leading to localized heating only around the north hemisphere, also known as thermal confinement, and consequently amplifying ζ during the pulsed heating.
Recently, FEM modeling has been used to investigate thermal transport with the first kind of JNP which consists of heterogeneous materials. González-Colsa et al. has shown a temperature gap of 10 K with a gold/polymer semi-shell nanostructure under pulsed heating, though the temperature gap under steady-state conditions with the semi-shell remains unclear.36 Drawing parallels with the first type of JNP investigated in our study, we speculate a significantly enhanced directional heating potential for this type of JNP under ultra-fast pulsed heating compared to continuous heating, attributable to similar factors such as thermal confinement. We anticipate that ultra-fast pulsed heating could be an effective method for harnessing directional heating across various types of JNPs.
Effect of Brownian motion on JNP heating
The Brownian motion of particles in a liquid can have a significant impact on thermal transport around JNPs. For instance, the mean velocity associated with Brownian motion for a 10 nm particle in water is approximately 10 μm s−1. Considering the time scale of our analysis (100 ns), this velocity suggests only minimal displacement (on the order of ∼1 pm). As a result, we anticipate that the influence of Brownian motion on thermal transport will be minimal under these conditions.
Conclusion
This work focuses on directional heating with Janus nanoparticle (JNP). Through computational modeling, we analyzed the effect of ITR contrast, JNP size, and asymmetric coating on the temperature contrast under steady-state and transient heating conditions. First, we found a significant size dependence of temperature contrast, where a smaller JNP can lead to greater temperature contrast. We further revealed the role of asymmetric coating and found higher temperature contrast when low thermal resistance coating covers a minor fraction of JNP surface. Importantly, we elucidated the temperature evolution near a JNP during a transient heating process and found up to 20-fold enhancement of the temperature contrast for pulsed JNP heating versus continuous heating. Our work provides the basis to design JNP and maximize the directional heating.
Methods
Theoretical model
Here we considered a JNP with two different surface coatings and interface thermal resistance values (Fig. 1A). The material of our JNP is gold and the JNP is submerged in an aqueous solution. Heat can transfer through the interface into the water when the JNP is excited by laser radiation.40 To facilitate the investigation of thermal transport during the transient, we adopted square pulsed heating in our modeling. Recently, studies reported that heat conduction under the nanoscale could deviate from Fourier's law when the mean free path is comparable to the characteristic length.57 In this work, the temperature profile in the water domain is our primary concern. Considering that the mean free path of phonons in the water (∼0.3 nm) is orders of magnitude smaller than the characteristic length (∼10 nm),45 the Fourier law should be sufficient to model the thermal transport,14,44 we applied Fourier's law with the governing equation:1| | 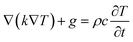 | (3) |
Here, k is the thermal conductivity, g is the heat source, ρ is density, and c is the specific heat. We further expand the governing equation under spherical coordinates with azimuthal symmetry and simplify our model to a 2D problem (Fig. 1A and S1†). To further simplify the model, we assume constant thermal properties. Based on our previous work, the constant property assumption introduces minimal error.14 The theoretical model is shown as follows:| |  | (4) |
where D refers to thermal diffusivity. Expanding the governing equation with spherical coordinates enables us to discretize the system with an orthogonal mesh (Fig. 1B and S1†). However, as shown in eqn (4), the system will have singularities when r → 0 and θ → 0 & π and is discontinuous at the center point where the two singularities meet (r = 0, θ = 0).58 To overcome this, we assume the temperature is uniformly distributed in the θ direction near the center point. Thus, we can further reduce the governing equation in the region near the center point to a 1D problem (Fig. S1†). Since the thermal diffusivity of metal is much higher than that in water, the temperature profile inside the JNP is close to isothermal, and this 1D assumption is acceptable. Consequently, the temperature governing equation is given by:| | 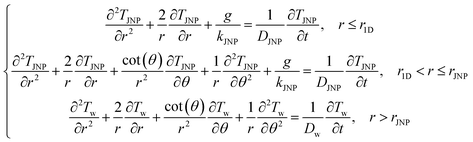 | (5) |
The boundary and initial conditions are:
| | 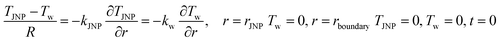 | (6) |
r1D refers to the radius of the 1D region (Fig. S2
†),
rJNP refers to the radius of the JNP, and
rboundary refers to the radius of the boundary for the water domain. It should be noted that
R is the interfacial thermal resistance (ITR), and it is a function of
θ:
| | 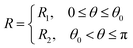 | (7) |
where
R1 and
R2 indicate the ITR for the coating on the northern part and the ITR for the coating on the southern part, respectively, and
θ0 refers to the polar angle boundary between the heterogenous coatings (
Fig. 1A).
Finite difference method model
Next, we used the finite difference method model and discretized eqn (5)–(7).59 Here, we adopted a biased mesh in the radius direction in the water domain to achieve higher accuracy and save computational time (Fig. S1†). For the steady-state problem, the left-hand side of eqn (5) equals zero, and the discretized governing equation in the water domain is as follows:| | 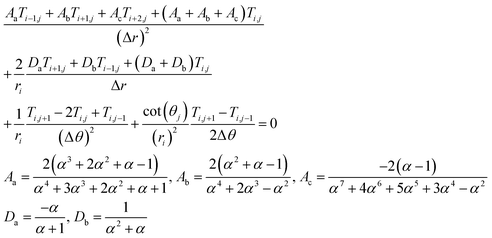 | (8) |
where the subscript i indicates the ith node in the r direction, and j indicates the jth node in θ direction, α indicates the biased mesh factor (Δri+1 = αΔri). The system has a second order of accuracy, and we validated the model by conducting a mesh-independent analysis and boundary effect analysis (Fig. S2 and S3†).
For the transient problem, we discretized eqn (5)–(7) with a Crank–Nicolson scheme,60 and the discretized governing equation in the water domain is:
| | 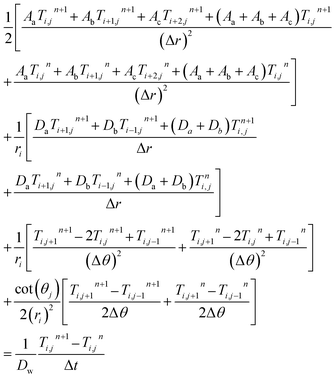 | (9) |
where superscript
n indicates the
nth node in the time direction. We further validated the accuracy and stability of the model (Fig. S4
†). Numerical results are consistent with analytical solutions.
Eqn (8) and
(9) are solved with MATLAB R2020b.
Abbreviations
| JNP | Janus nanoparticle |
| ITR | Interfacial thermal resistance |
| MD | Molecular dynamic |
| FDM | Finite difference method |
| S.S. | Steady-state |
Roman letters
|
R
1
| Interfacial thermal resistance along the north part of Janus nanoparticle, m2 K W−1 |
|
R
2
| Interfacial thermal resistance along the south part of Janus nanoparticle, m2 K W−1 |
|
k
| Thermal conductivity, W m−2 K−1 |
|
k
JNP
| Thermal conductivity in Janus nanoparticle, W m−2 K−1 |
|
k
w
| Thermal conductivity in water, W m−2 K−1 |
|
T
| Temperature, K |
|
T
JNP
| Temperature in the Janus nanoparticle, K |
|
T
w
| Temperature in water, K |
|
g
| Heating power, W m−3 |
|
c
| Specific heat, J kg−1 |
|
t
| Time, s |
|
r
| Radius, m |
|
r
1D
| Radius of the 1D zone, m |
|
r
JNP
| Radius of the Janus nanoparticle, m |
|
r
boundary
| Radius of the water domain, m |
|
D
| Thermal diffusivity, m2 s−1 |
|
D
JNP
| Thermal diffusivity in Janus nanoparticle, m2 s−1 |
|
D
w
| Thermal diffusivity in water, m2 s−1 |
|
R
| Interfacial thermal resistance, m2 K W−1 |
|
R
1
| Interfacial thermal resistance on the north hemisphere, m2 K W−1 |
|
R
2
| Interfacial thermal resistance on the southern hemisphere, m2 K W−1 |
| Ka | Kaptiza number, 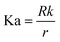 |
| Ka1 | Kaptiza number on the north hemisphere, 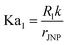 |
| Ka2 | Kaptiza number on the southern hemisphere, 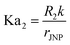 |
|
q
| Heat flux, W |
|
q
1
| Heat flux through the north hemisphere, W |
|
q
2
| Heat flux through the southern hemisphere, W |
![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif)
| Heat flux rate, W m−2 |
![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1
1
| Heat flux rate at the north pole, W m−2 |
![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2
2
| Heat flux rate at the south pole, W m−2 |
| Fo | Fourier number, 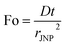 |
Greek symbols
|
θ
| Polar angle |
|
θ
0
| The critical polar angle that pinpoints the boundary between the heterogeneous interfacial thermal resistances |
|
ζ
| Dimensionless parameter to characterize the temperature contrast, 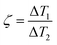 |
|
ζ
s.s.
| Dimensionless parameter to characterize the temperature contrast for steady-state |
|
ρ
| Density, kg m−3 |
|
τ
| Characteristic thermal relaxation time,  , s , s |
Author contributions
C. X. developed the numerical model and did the computational work and analysis. B. W. and Z. Q. participated in conceptualization and analysis. All authors contributed to the writing of the manuscript. Z. Q. directed the research.
Conflicts of interest
All authors declare that they have no conflicts of interest.
Acknowledgements
The High-Impact/High-Risk Research Award from the Cancer Prevention and Research Institute of Texas (award number RP180846). I am grateful for the useful suggestions from Dr Yaning Liu and Dr Hui Ouyang. Research reported in this publication was supported by National Institute of General Medical Sciences and National Institute of Allergy and Infectious Diseases of the National Institutes of Health under award numbers R35GM133653 and R01AI151374, the National Science Foundation under award number 2123971, and the American Heart Association under award number 19CSLOI34770004. The content is solely the responsibility of the authors and does not necessarily represent the official views of funding agencies.
References
- L. Jauffred, A. Samadi, H. Klingberg, P. M. Bendix and L. B. Oddershede, Plasmonic Heating of Nanostructures, Chem. Rev., 2019, 119, 8087–8130 CrossRef CAS PubMed.
- M. Kim, J.-H. Lee and J.-M. Nam, Plasmonic Photothermal Nanoparticles for Biomedical Applications, Adv. Sci., 2019, 6, 1900471 CrossRef PubMed.
- J. Park, J. Huang, W. Wang, C. J. Murphy and D. G. Cahill, Heat Transport between Au Nanorods, Surrounding Liquids, and Solid Supports, J. Phys. Chem. C, 2012, 116, 26335–26341 CrossRef CAS.
- Z. Qin and J. C. Bischof, Thermophysical and biological responses of gold nanoparticle laser heating, Chem. Soc. Rev., 2012, 41, 1191–1217 RSC.
- X. Huang, P. K. Jain, I. H. El-Sayed and M. A. El-Sayed, Plasmonic photothermal therapy (PPTT) using gold nanoparticles, Laser Med. Sci., 2008, 23, 217–228 CrossRef PubMed.
- C.-M. J. Hu, S. Aryal and L. Zhang, Nanoparticle-assisted combination therapies for effective cancer treatment, Ther. Delivery, 2010, 1, 323–334 CrossRef CAS PubMed.
- R. S. Riley and E. S. Day, Gold nanoparticle-mediated photothermal therapy: applications and opportunities for multimodal cancer treatment, Wiley Interdiscip. Rev.: Nanomed. Nanobiotechnol., 2017, 9, e1449 Search PubMed.
- A. M. Goodman, N. J. Hogan, S. Gottheim, C. Li, S. E. Clare and N. J. Halas, Understanding Resonant Light-Triggered DNA Release from Plasmonic Nanoparticles, ACS Nano, 2017, 11, 171–179 CrossRef CAS PubMed.
- X. Li, V. Vemireddy, Q. Cai, H. Xiong, P. Kang and X. Li,
et al., Reversibly Modulating the Blood–Brain Barrier by Laser Stimulation of Molecular-Targeted Nanoparticles, Nano Lett., 2021, 21, 9805–9815 CrossRef CAS PubMed.
- H. Kang, G.-H. Lee, H. Jung, J. W. Lee and Y. Nam, Inkjet-Printed Biofunctional Thermo-Plasmonic Interfaces for Patterned Neuromodulation, ACS Nano, 2018, 12, 1128–1138 CrossRef CAS PubMed.
- J. C. Fraire, M. L. Masseroni, I. Jausoro, E. M. Perassi, A. M. Diaz Añel and E. A. Coronado, Identification, localization, and quantification of neuronal cell membrane receptors with plasmonic probes: role of protein kinase D1 in their distribution, ACS Nano, 2014, 8, 8942–8958 CrossRef CAS PubMed.
- P. Kang, Z. Chen, S. O. Nielsen, K. Hoyt, S. D’Arcy and J. J. Gassensmith,
et al., Molecular Hyperthermia: Spatiotemporal Protein Unfolding and Inactivation by Nanosecond Plasmonic Heating, Small, 2017, 13, 1700841 CrossRef PubMed.
- P. Kang, X. Li, Y. Liu, S. I. Shiers, H. Xiong and M. Giannotta,
et al., Transient Photoinactivation of Cell Membrane Protein Activity without Genetic Modification by Molecular Hyperthermia, ACS Nano, 2019, 13, 12487–12499 CrossRef CAS PubMed.
- P. Kang, C. Xie, O. Fall, J. Randrianalisoa and Z. Qin, Computational Investigation of Protein Photoinactivation by Molecular Hyperthermia, J. Biomech. Eng., 2021, 143, 031004 CrossRef PubMed.
- C. Xie, P. Kang, J. Cazals, O. M. Castelán, J. Randrianalisoa and Z. Qin, Single pulse heating of a nanoparticle array for biological applications, Nanoscale Adv., 2022, 4, 2090–2097 RSC.
- Y. Liu, H. Ye, H. Huynh, C. Xie, P. Kang and J. S. Kahn,
et al., Digital plasmonic nanobubble detection for rapid and ultrasensitive virus diagnostics, Nat. Commun., 2022, 13, 1687 CrossRef CAS PubMed.
- Y. Liu, H. Ye, A. Bayram, T. Zhang, Q. Cai and C. Xie,
et al., Gold Nanourchins Improve Virus Targeting and Plasmonic Coupling for Virus Diagnosis on a Smartphone Platform, ACS Sens., 2022, 7, 3741–3752 CrossRef CAS PubMed.
- P. Parsamian, Y. Liu, C. Xie, Z. Chen, P. Kang and Y. H. Wijesundara,
et al., Enhanced Nanobubble Formation: Gold Nanoparticle Conjugation to Qβ Virus-like Particles, ACS Nano, 2023, 17, 7797–7805 CrossRef CAS PubMed.
- Y. Mantri and J. V. Jokerst, Engineering Plasmonic Nanoparticles for Enhanced Photoacoustic Imaging, ACS Nano, 2020, 14, 9408–9422 CrossRef CAS PubMed.
- J. Russier, L. Oudjedi, M. Piponnier, C. Bussy, M. Prato and K. Kostarelos,
et al., Direct visualization of carbon nanotube degradation in primary cells by photothermal imaging, Nanoscale, 2017, 9, 4642–4645 RSC.
- H. Xiong, E. Lacin, H. Ouyang, A. Naik, X. Xu and C. Xie,
et al., Probing neuropeptide volume transmission in vivo by simultaneous near-infrared light-triggered release and optical sensing, Angew. Chem., 2022, 134, e202206122 CrossRef.
- C. Ma, J. Yan, Y. Huang, C. Wang and G. Yang, The optical duality of tellurium nanoparticles for broadband solar energy harvesting and efficient photothermal conversion, Sci. Adv., 2018, 4, eaas9894 CrossRef CAS PubMed.
- G. Zhu, Z.-H. Lin, Q. Jing, P. Bai, C. Pan and Y. Yang,
et al., Toward large-scale energy harvesting by a nanoparticle-enhanced triboelectric nanogenerator, Nano Lett., 2013, 13, 847–853 CrossRef CAS PubMed.
- D. Mateo, N. Morlanes, P. Maity, G. Shterk, O. F. Mohammed and J. Gascon, Efficient visible-light driven photothermal conversion of CO 2 to methane by nickel nanoparticles supported on barium titanate, Adv. Funct. Mater., 2021, 31, 2008244 CrossRef CAS.
- L. Zhu, M. Gao, C. K. N. Peh and G. W. Ho, Solar-driven photothermal nanostructured materials designs and prerequisites for evaporation and catalysis applications, Mater. Horiz., 2018, 5, 323–343 RSC.
- A. Espinosa, J. Reguera, A. Curcio, Á. Muñoz-Noval, C. Kuttner and A. Van de Walle,
et al., Janus magnetic-plasmonic nanoparticles for magnetically guided and thermally activated cancer therapy, Small, 2020, 16, e1904960 CrossRef PubMed.
- T. C. Le, J. Zhai, W.-H. Chiu, P. A. Tran and N. Tran, Janus particles: recent advances in the biomedical applications, Int. J. Nanomed., 2019, 14, 6749–6777 CrossRef CAS PubMed.
- N. Safaie and R. C. Ferrier Jr, Janus nanoparticle synthesis: Overview, recent developments, and applications, J. Appl. Phys., 2020, 127, 170902 CrossRef.
- M. D. McConnell, M. J. Kraeutler, S. Yang and R. J. Composto, Patchy and multiregion janus particles with tunable optical properties, Nano Lett., 2010, 10, 603–609 CrossRef CAS PubMed.
- J. Hu, S. Zhou, Y. Sun, X. Fang and L. Wu, Fabrication, properties and applications of Janus particles, Chem. Soc. Rev., 2012, 41, 4356–4378 RSC.
- M. Lattuada and T. A. Hatton, Synthesis, properties and applications of Janus nanoparticles, Nano Today, 2011, 6, 286–308 CrossRef CAS.
- X. Li, L. Chen, D. Cui, W. Jiang, L. Han and N. Niu, Preparation and application of Janus nanoparticles: Recent development and prospects, Coord. Chem. Rev., 2022, 454, 214318 CrossRef CAS.
- M. Xuan, Z. Wu, J. Shao, L. Dai, T. Si and Q. He, Near Infrared Light-Powered Janus Mesoporous Silica Nanoparticle Motors, J. Am. Chem. Soc., 2016, 138, 6492–6497 CrossRef CAS PubMed.
- J. D. Olarte-Plata, J. Gabriel, P. Albella and F. Bresme, Spatial Control of Heat Flow at the Nanoscale Using Janus Particles, ACS Nano, 2022, 16, 694–709 CrossRef CAS PubMed.
- S. Auschra, A. Bregulla, K. Kroy and F. Cichos, Thermotaxis
of Janus particles, Eur. Phys. J. E: Soft Matter Biol. Phys., 2021, 44, 90 CrossRef CAS PubMed.
- J. González-Colsa, F. Bresme and P. Albella, Impact of the Interfacial Thermal Conductance on the Thermoplasmonic Response of Metal/Polymer Hybrid Nanoparticles under Nanosecond Pulsed Illumination, J. Phys. Chem. C, 2023, 127, 19152–19158 CrossRef.
- J. González-Colsa, A. Franco, F. Bresme, F. Moreno and P. Albella, Janus-Nanojet as an efficient asymmetric photothermal source, Sci. Rep., 2022, 12, 14222 CrossRef PubMed.
-
A. P. Bregulla and F. Cichos, Polarization of thermophoretic swimmers in external temperature fields, Optical Trapping and Optical Micromanipulation XIII, SPIE, 2016, pp. 192–198 Search PubMed.
- S. Simoncelli, S. Johnson, F. Kriegel, J. Lipfert and J. Feldmann, Stretching and Heating Single DNA Molecules with Optically Trapped Gold–Silica Janus Particles, ACS Photonics, 2017, 4, 2843–2851 CrossRef CAS.
- Z. Chen, J. Li and Y. Zheng, Heat-Mediated Optical Manipulation, Chem. Rev., 2022, 122, 3122–3179 CrossRef CAS PubMed.
- C. M. Maier, M. A. Huergo, S. Milosevic, C. Pernpeintner, M. Li and D. P. Singh,
et al., Optical and Thermophoretic Control of Janus Nanopen Injection into Living Cells, Nano Lett., 2018, 18, 7935–7941 CrossRef CAS PubMed.
- X. Cui, J. Wang and G. Xia, Enhanced thermal conductivity of nanofluids by introducing Janus particles, Nanoscale, 2021, 14, 99–107 RSC.
- M. Jiang, A. Chapman, J. D. Olarte-Plata and F. Bresme, Controlling local thermal gradients at molecular scales with Janus nanoheaters, Nanoscale, 2023, 15, 10264–10276 RSC.
- G. Baffou, P. Berto, E. Bermúdez Ureña, R. Quidant, S. Monneret and J. Polleux,
et al., Photoinduced heating of nanoparticle arrays, ACS Nano, 2013, 7, 6478–6488 CrossRef CAS PubMed.
- A. W. Lawson, R. Lowell and A. L. Jain, Thermal conductivity of water at high pressures, J. Chem. Phys., 1959, 30, 643–647 CrossRef CAS.
- Z. Xu, D. Huang and T. Luo, Molecular-Level Understanding of Efficient Thermal Transport across the Silica–Water Interface, J. Phys. Chem. C, 2021, 125, 24115–24125 CrossRef CAS.
- S.-W. Hung, G. Kikugawa and J. Shiomi, Mechanism of Temperature Dependent Thermal Transport across the Interface between Self-Assembled Monolayer and Water, J. Phys. Chem. C, 2016, 120, 26678–26685 CrossRef CAS.
- T. Q. Vo and B. Kim, Interface thermal resistance between liquid water and various metallic surfaces, Int. J. Precis. Eng. Manuf., 2015, 16, 1341–1346 CrossRef.
- O. M. Wilson, X. Hu, D. G. Cahill and P. V. Braun, Colloidal metal particles as probes of nanoscale thermal transport in fluids, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 66, 224301 CrossRef.
- A. Plech, V. Kotaidis, S. Grésillon, C. Dahmen and G. von Plessen, Laser-induced heating and melting of gold nanoparticles studied by time-resolved x-ray scattering, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 70, 195423 CrossRef.
- Z. Ge, D. G. Cahill and P. V. Braun, Thermal conductance of hydrophilic and hydrophobic interfaces, Phys. Rev. Lett., 2006, 96, 186101 CrossRef PubMed.
- A. J. Schmidt, J. D. Alper, M. Chiesa, G. Chen, S. K. Das and K. Hamad-Schifferli, Probing the Gold Nanorod−Ligand−Solvent Interface by Plasmonic Absorption and Thermal Decay, J. Phys. Chem. C, 2008, 112, 13320–13323 CrossRef CAS.
- X. Wu, Y. Ni, J. Zhu, N. D. Burrows, C. J. Murphy and T. Dumitrica,
et al., Thermal Transport across Surfactant Layers on Gold Nanorods in Aqueous Solution, ACS Appl. Mater. Interfaces, 2016, 8, 10581–10589 CrossRef CAS PubMed.
- G. Baffou and R. Quidant, Thermo-plasmonics:
using metallic nanostructures as nano-sources of heat, Laser Photonics Rev., 2013, 7, 171–187 CrossRef CAS.
- L. Guo, Y. Xu, A. R. Ferhan, G. Chen and D.-H. Kim, Oriented gold nanoparticle aggregation for colorimetric sensors with surprisingly high analytical figures of merit, J. Am. Chem. Soc., 2013, 135, 12338–12345 CrossRef CAS PubMed.
- D. Nelidova, R. K. Morikawa, C. S. Cowan, Z. Raics, D. Goldblum and H. P. N. Scholl,
et al., Restoring light sensitivity using tunable near-infrared sensors, Science, 2020, 368, 1108–1113 CrossRef CAS PubMed.
- K. T. Regner, D. P. Sellan, Z. Su, C. H. Amon, A. J. H. McGaughey and J. A. Malen, Broadband phonon mean free path contributions to thermal conductivity measured using frequency domain thermoreflectance, Nat. Commun., 2013, 4, 1640 CrossRef PubMed.
- W. Dai, L. Shen, R. Nassar and T. Zhu, A stable and convergent three-level finite difference scheme for solving a dual-phase-lagging heat transport equation in spherical coordinates, Int. J. Heat Mass Transfer, 2004, 47, 1817–1825 CrossRef.
- J. Thibault, S. Bergeron and H. W. Bonin, On finite-difference solutions of the heat equation in spherical coordinates, Numer. Heat Transfer, Part A, 1987, 12, 457–474 Search PubMed.
-
D. Anderson, J. C. Tannehill, R. H. Pletcher, R. Munipalli and V. Shankar, Computational Fluid Mechanics and Heat Transfer, CRC Press, 2020 Search PubMed.
|
| This journal is © The Royal Society of Chemistry 2024 |
Click here to see how this site uses Cookies. View our privacy policy here.  Open Access Article
Open Access Article a,
Blake A.
Wilson
a,
Blake A.
Wilson
 a and
Zhenpeng
Qin
a and
Zhenpeng
Qin
 *abc
*abc
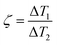 , Fig. 1B) to quantify the magnitude of directional heating. Our modeling results suggest that increasing JNP size reduces the temperature contrast, additionally, an approximate solution is provided to estimate the JNP size-dependent temperature contrast. Furthermore, the distribution of interface thermal resistance (varying the surface coverage of low versus high interface thermal resistance) has a significant impact on the temperature contrast, with a minor coverage by low interface resistance leading to higher temperature contrast. Importantly, we demonstrate a significant enhancement of temperature contrast with pulsed JNP heating compared with continuous heating. Our work advances the understanding of directional heating with JNP by clarifying the contributions of various factors to JNP nanoscale thermal transport.
, Fig. 1B) to quantify the magnitude of directional heating. Our modeling results suggest that increasing JNP size reduces the temperature contrast, additionally, an approximate solution is provided to estimate the JNP size-dependent temperature contrast. Furthermore, the distribution of interface thermal resistance (varying the surface coverage of low versus high interface thermal resistance) has a significant impact on the temperature contrast, with a minor coverage by low interface resistance leading to higher temperature contrast. Importantly, we demonstrate a significant enhancement of temperature contrast with pulsed JNP heating compared with continuous heating. Our work advances the understanding of directional heating with JNP by clarifying the contributions of various factors to JNP nanoscale thermal transport.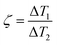
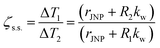
 . This size dependence of ζs.s. is also confirmed with the FDM model, and the ζs.s maps with rJNP ranging from 3.75 to 60 nm (Fig. S6†).
. This size dependence of ζs.s. is also confirmed with the FDM model, and the ζs.s maps with rJNP ranging from 3.75 to 60 nm (Fig. S6†).![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) ). The local heat flux at the north pole (
). The local heat flux at the north pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1) is significantly higher (∼50-fold at θ0 = 0.02π) than that of the south pole (
1) is significantly higher (∼50-fold at θ0 = 0.02π) than that of the south pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2) when θ0 < π/2. The difference between
2) when θ0 < π/2. The difference between ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1 and
1 and ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2 with smaller θ0 leads to a remarkably higher ΔT1 (Fig. 3C) than ΔT2. We further examined the asymmetric coating for different JNP sizes (Fig. 3D). Our results suggest that smaller JNP size gives greater temperature contrast (e.g., ζs.s. = 10 for a 3 nm JNP with θ0 ∼ π/12, area fraction for low thermal resistance ∼3%).
2 with smaller θ0 leads to a remarkably higher ΔT1 (Fig. 3C) than ΔT2. We further examined the asymmetric coating for different JNP sizes (Fig. 3D). Our results suggest that smaller JNP size gives greater temperature contrast (e.g., ζs.s. = 10 for a 3 nm JNP with θ0 ∼ π/12, area fraction for low thermal resistance ∼3%).
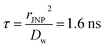 for 30 nm JNP), JNP heating with nanosecond or shorter pulsed heating is far from steady-state and can lead to thermal confinement. Here we imposed 100 ns laser pulse (constant heating) on a 30 nm JNP with R2/R1 = 100 and θ0 = π/2. Fig. 4A illustrates the temperature profiles during the transient heating process. At t = 1 ns, only a thin layer of water adjacent to the JNP–water interface is heated along the northern hemisphere (ΔT ∼ 200 K). On the contrary, minimal heating is observed near the southern hemisphere (ΔT ∼ 25 K) due to higher ITR. By comparing the local heat flux at the north pole and south pole (
for 30 nm JNP), JNP heating with nanosecond or shorter pulsed heating is far from steady-state and can lead to thermal confinement. Here we imposed 100 ns laser pulse (constant heating) on a 30 nm JNP with R2/R1 = 100 and θ0 = π/2. Fig. 4A illustrates the temperature profiles during the transient heating process. At t = 1 ns, only a thin layer of water adjacent to the JNP–water interface is heated along the northern hemisphere (ΔT ∼ 200 K). On the contrary, minimal heating is observed near the southern hemisphere (ΔT ∼ 25 K) due to higher ITR. By comparing the local heat flux at the north pole and south pole (![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1 and
1 and ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2), both the magnitude and slope for
2), both the magnitude and slope for ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 1(t) are significantly higher than those for
1(t) are significantly higher than those for ![[q with combining dot above]](https://www.rsc.org/images/entities/i_char_0071_0307.gif) 2(t) for t < 1 ns, leading to enhanced temperature contrast (Fig. 4B–D, ζ ∼ 20 at 0.1 ns). Here we adopted the Fourier's number (
2(t) for t < 1 ns, leading to enhanced temperature contrast (Fig. 4B–D, ζ ∼ 20 at 0.1 ns). Here we adopted the Fourier's number (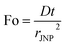 , D: thermal diffusivity, t: time) as dimensionless time. As heating continues, thermal energy continuously diffuses and leads to reduced temperature contrast. At t = 100 ns, the maximum temperature is reached as it is the end time point for the pulsed heating and the heating asymptotically approaches the steady-state value (Fig. 4B, Fo = 63.5), and the temperature contrast ζ asymptotically falls to its steady-state level (Fig. 4C, ζs.s. = 2.5). After the pulsed heating (t > 100 ns), the whole system cools down rapidly, and both ΔT1 and ΔT2 drop to 0 with ζ approaching 1. Therefore, the thermal confinement under short ns pulse heating (Fo ≪ 1) leads to a 8-fold enhancement of temperature contrast under current conditions (Fo = 0.06, R2/R1 = 100, rJNP = 15 nm, θ0 = π/2).
, D: thermal diffusivity, t: time) as dimensionless time. As heating continues, thermal energy continuously diffuses and leads to reduced temperature contrast. At t = 100 ns, the maximum temperature is reached as it is the end time point for the pulsed heating and the heating asymptotically approaches the steady-state value (Fig. 4B, Fo = 63.5), and the temperature contrast ζ asymptotically falls to its steady-state level (Fig. 4C, ζs.s. = 2.5). After the pulsed heating (t > 100 ns), the whole system cools down rapidly, and both ΔT1 and ΔT2 drop to 0 with ζ approaching 1. Therefore, the thermal confinement under short ns pulse heating (Fo ≪ 1) leads to a 8-fold enhancement of temperature contrast under current conditions (Fo = 0.06, R2/R1 = 100, rJNP = 15 nm, θ0 = π/2).