Open Access Article
Open Access ArticleExtrinsic chirality tailors Stokes parameters in simple asymmetric metasurfaces†
Emilija
Petronijevic
 *a,
Tiziana
Cesca
*a,
Tiziana
Cesca
 b,
Carlo
Scian
b,
Giovanni
Mattei
b,
Roberto
Li Voti
a,
Concita
Sibilia
a and
Alessandro
Belardini
b,
Carlo
Scian
b,
Giovanni
Mattei
b,
Roberto
Li Voti
a,
Concita
Sibilia
a and
Alessandro
Belardini
 a
a
aDepartment SBAI, Sapienza University of Roma, Via A. Scarpa 14, I-00161 Rome, Italy. E-mail: emilija.petronijevic@uniroma1.it
bUniversity of Padova, Department of Physics and Astronomy, NanoStructures Group, via Marzolo 8, I-35131 Padova, Italy
First published on 7th August 2024
Abstract
Metasurfaces tailor electromagnetic confinement at the nanoscale and can be appropriately designed for polarization-dependent light–matter interactions. Adding the asymmetry degree to the desing allows for circular polarizations of opposite handedness to be differently absorbed or emitted, which is of interest in fields spanning from chiral sensing to flat optics. Here, we show that simple, low-cost asymmetric metasurfaces can control Stokes parameters in the transmitted far-field. With only 50 nm of asymmetric plasmonic shells on self-assembled polystyrene nanospheres, our metasurfaces allow for great spectral and incident angle tunability. We first investigated broadband extrinsic chirality in metasurfaces with asymmetric plasmonic semishells; we found high extinction circular dichroism (CD) in the near-infrared range. We then excited it with linear polarization and performed hyperspectral Stokes polarimetry on the transmitted field. We showed that the S3 parameter follows the behavior of CD in extinction, and that the output field position on the Poincaré sphere can be widely controlled by using the incidence angle and wavelength. Furthermore, simulations agreed well with the experiments and showed how the near-field chiro-optical response influences the extrinsic chiral behavior in absorption and the polarization state of the transmitted field.
1 Introduction
Plasmonic metasurfaces, made of nanoscale-structured plasmonic materials, offer remarkable properties regarding light–matter coupling, tailoring both near- and far-fields. While the near-field effects pave the way for highly efficient sensing platforms,1–3 the directionality, polarization, intensity, and wavelength of the transmitted or emitted far-fields are potentially applicable in chip-scale components for flat optics and optical and quantum applications.4–8 Breaking the symmetry of the electromagnetic interactions in metasurfaces enriches the fields of plasmonics and nanophotonics with chirality at the nanoscale.9,10 Meta-atoms can be structured and organized to couple with chiral molecules for ultrasensitive chiral detection.11–15 Moreover, as nanotechnology advances, nanophotonic and plasmonic communities propose nanostructured materials with remarkable chiro-optical control and polarization manipulation, which could potentially replace bulky optical components in chip-scale applications. Anisotropic nanoantennas were shown to convert polarization in telecoms16 and the mid-infrared range,8 while gap-plasmon-based metasurfaces have been applied in the determination of Stokes parameters17 and intensity-tuneable metaholography via spin angular momentum selection.18 Chiral meta-atoms have been further applied in chiral holographic displays,19,20 for in situ tuneable circular dichroism by flexible substrates,21 in Stokes near-infrared22 and mid-infrared polarimetry,23 and for giant nonlinear chiro-optical effects.24,25 Finally, sophisticated chiral metasurfaces, coupled with light nanosources, have been used for chiral single-photon emission26 and spontaneous generation of vortex beams.27Self-organization of matter into various chiral shapes is present in nature at all scales; this has inspired the fabrication of self-assembled single nanomaterials with structural 3D chirality and their complex assemblies.28,29 On the other hand, coupling between single nanostructures on substrates opens up unprecedented chiral metasurface potentialities; for example, quasi-3D oligomers of Au and/or Ag led to broad chiral behaviour across the visible and near-infrared ranges,30 while chiral single golden crescents, combined with hexagonal in-plane periodicity, opened up the field of chiral surface lattice resonances.31 Nowadays, the vibrant field of chirality at the nanoscale would surely benefit from the simplification of nanoplasmonic geometries for multifunctional chiro-optical manipulation. Colloidal lithography has emerged as a fast, low-cost, and large-area manufacturing technique where nanosphere-based templates are used to obtain various plasmonic nanostructures based on nanoholes, shells, discs, and cones.32–34 Nanosphere lithography (NSL) can be further combined with tilted metallic deposition to provide remarkable varieties of both asymmetric and chiral plasmonic metamaterials.31,35–39 Self-assembled nanospheres form shadows during the plasmonic deposition, which can be used for simpler, 2D-chiral metasurfaces based on nanohole arrays.40,41 Moreover, the metasurface does not need to be chiral itself in order to exhibit chiro-optical effects: instead, they can be induced in asymmetric (but not chiral) metasurfaces, by appropriately exciting the metasurface at oblique incidence. Such an effect is called “extrinsic” chirality,42–44 and it allows for largely tuneable near- and far-field chiro-optical interactions.45–47 We previously used nanosphere-based lithography (NSL) to obtain samples with asymmetric plasmonic shells that can be manipulated via extrinsic chirality. We showed that such samples, at oblique incidence, differently interact with left and right circular polarizations (LCP and RCP), thus exhibiting circular dichroism (CD) in absorption,48 diffraction,49 and extinction.50 Self-assembled asymmetric plasmonic nanocrescents were also applied for SERS amplification51 and for efficient second harmonic generation exhibiting CD.52
In this study, we show how thin asymmetric plasmonic shells efficiently tailor extrinsic chiro-optical interactions in metasurfaces, leading to hyperspectral control of the transmitted beam polarization state. The low-cost sample has simple geometry, which allows for the broad CD resonance in the near-infrared range. We start with experimental and numerical investigations of the extrinsic chirality. We then excite the sample with linear polarization and perform broadband analysis of the Stokes parameters in the transmitted field. The S3 parameter, which indicates the circular polarization degree, follows the extinction CD, and agrees with the rules of extrinsic chirality.53 Moreover, the polarization state is widely tuneable with respect to wavelength and incidence angle.
2 Results and discussion
2.1 Sample geometry and experimental setup
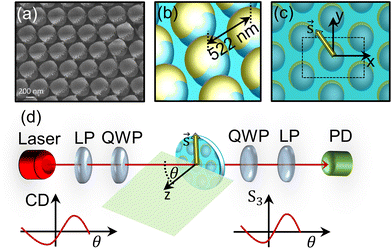
The investigated metasurface consists of polystyrene nanospheres (PSNs) asymmetrically covered by gold, lying on a soda-lime glass substrate. We aimed to investigate asymmetric plasmonic shells with resonances in the near infrared range. Therefore, we combined NSL and tilted metal deposition to obtain asymmetric geometry and 2D arrangement, as explained in our previous works.48–50 However, in this work, we aimed to avoid the formation of nanohole arrays54 on the substrate in order to obtain simpler geometry and overall higher transmission. We started from self-assembled PSNs of 522 nm diameter, and we decreased their diameter by reactive ion etching to around 370 nm, while preserving 2D periodicity. Next, we induced the asymmetry by a tilted evaporation of 50 nm of Au at 60° (i.e., along the second nearest neighbour direction, as sketched in Fig. 1); this angle and diameter choice prevents the nanohole formation, which occurred in the case of evaporation at 45° in our previous works.48–50Fig. 1a and b show a scanning electron microscopy (SEM) image of the sample, and a simplified 3D model, respectively. Fig. 1c shows a top-view schematic of the rectangular unit cell used, with the definition of the average in-plane asymmetry vector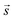 : this is the average direction of Au deposition, projected onto the xy plane. The
: this is the average direction of Au deposition, projected onto the xy plane. The  vector is crucial in performing the extrinsic chirality characterization, as it must make a non-planar triad of vectors with the surface normal, and wave-vector of the incoming light.55 Extrinsic chirality was investigated in reflection and transmission from sophisticated metasurfaces fabricated by electron beam lithography.56,57 Here, instead, we used cost- and time-efficient fabrication to obtain an ordered sample over a large area (mm2). NSL is based on commercially available PSNs, without relying on cleanroom facilities and multiple precise nanofabrication steps. Finally, we were able to obtain large-area metasurfaces, where plasmonic layer of only 50 nm introduces the asymmetry which allows for the control of near- and far-field chiro-optical effects, as further shown.
vector is crucial in performing the extrinsic chirality characterization, as it must make a non-planar triad of vectors with the surface normal, and wave-vector of the incoming light.55 Extrinsic chirality was investigated in reflection and transmission from sophisticated metasurfaces fabricated by electron beam lithography.56,57 Here, instead, we used cost- and time-efficient fabrication to obtain an ordered sample over a large area (mm2). NSL is based on commercially available PSNs, without relying on cleanroom facilities and multiple precise nanofabrication steps. Finally, we were able to obtain large-area metasurfaces, where plasmonic layer of only 50 nm introduces the asymmetry which allows for the control of near- and far-field chiro-optical effects, as further shown.
Fig. 1d presents the experimental setup for characterization of extrinsic chirality in extinction, and for full Stokes polarimetry of the transmitted field. We used a widely tuneable near-infrared laser (Chameleon Ultra II by Coherent Inc.) for excitation in the 720–1060 nm range. We used it in linear mode, with appropriately decreased output power.50,54 A first block, made of a linear polarizer (LP) and a rotating quarter-wave plate (QWP), controls the polarization state of the input beam between left-handed circular polarization (LCP), right-handed circular polarization (RCP) or linear p-polarization (with respect to the green incidence plane). The sample was rotated so that the incidence plane stays perpendicular to the 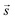 vector. In extinction measurements, the total zeroth order transmission was recorded using a Si photodiode (PD) and normalized to the transmission through air without the sample. For the characterization of full Stokes parameters, another block with a QWP followed by an LP was put on the path of the transmitted field; for each wavelength and incidence angle, four combinations of QWP–LP orientations were used to resolve the S parameters. Definitions of the input polarization states and Stokes parameters are explained in detail in the ESI.†
vector. In extinction measurements, the total zeroth order transmission was recorded using a Si photodiode (PD) and normalized to the transmission through air without the sample. For the characterization of full Stokes parameters, another block with a QWP followed by an LP was put on the path of the transmitted field; for each wavelength and incidence angle, four combinations of QWP–LP orientations were used to resolve the S parameters. Definitions of the input polarization states and Stokes parameters are explained in detail in the ESI.†
2.2 Extrinsic chirality in extinction
We first excited the sample with LCP and RCP polarizations and measured the total extinction as ExtLCP,RCP = 1 − TLCP,RCP, where TLCP,RCP is the transmittance when the sample is excited with LCP or RCP, respectively. Fig. 2 shows the measured extinction CD, defined as: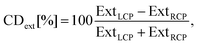 | (1) |
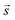 vector, which does not necessarily lie parallel to the lines of hexagonal symmetry.
vector, which does not necessarily lie parallel to the lines of hexagonal symmetry.
 | ||
| Fig. 2 Experimental and simulated extinction CD as a dependence on the incidence wavelength and angle. | ||
To understand the electromagnetic coupling in the near-field and the origin of extrinsic CD, we modeled the metasurface in a commercial 3D finite-difference time domain (FDTD) solver by using Lumerical.61 The model takes into account only the asymmetric Au semishells on periodic PSN (tilted by 60°), without the residual metal deposition on the substrate; details of the model are given in the ESI.† The simulated extinction CD, reported in Fig. 2, closely follows the measured one in the near-infrared range: for the best fit, the 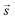 vector is rotated by 30° with respect to the y-direction of the FDTD domain, thus accounting for both intrinsic and extrinsic chirality. As this model correctly reproduces the extinction experiments in our wavelength range, we further use it to investigate the origin of the broad absorption. The metasurface exhibits a broad plasmonic resonance of the asymmetric shell at around 1000 nm, as shown in Fig. 3a; note that this absorption slightly differs for LCP and RCP excitation, due to intrinsic chirality. Oblique incidence by an angle θ with respect to the sample normal splits the resonances and decreases the RCP (LCP) absorption at θ = −30° (θ = +30°). Such large differences can be appreciated by looking at the distribution of the absorption density in one unit cell, as shown in Fig. 3b. LCP light at 1000 nm and θ = −30° strongly couples with the plasmonic asymmetric semishell, leading to a similar peak in the extinction, and further producing large positive CD (Fig. 2).
vector is rotated by 30° with respect to the y-direction of the FDTD domain, thus accounting for both intrinsic and extrinsic chirality. As this model correctly reproduces the extinction experiments in our wavelength range, we further use it to investigate the origin of the broad absorption. The metasurface exhibits a broad plasmonic resonance of the asymmetric shell at around 1000 nm, as shown in Fig. 3a; note that this absorption slightly differs for LCP and RCP excitation, due to intrinsic chirality. Oblique incidence by an angle θ with respect to the sample normal splits the resonances and decreases the RCP (LCP) absorption at θ = −30° (θ = +30°). Such large differences can be appreciated by looking at the distribution of the absorption density in one unit cell, as shown in Fig. 3b. LCP light at 1000 nm and θ = −30° strongly couples with the plasmonic asymmetric semishell, leading to a similar peak in the extinction, and further producing large positive CD (Fig. 2).
2.3 Extrinsic chirality and Stokes parameters
Since the metasurface differently absorbs LCP and RCP under oblique incidence, we further investigated the polarization control of the transmitted field. The excitation is p-polarized, lying perpendicular to the vector. In the output, we resolve the Stokes parameters by appropriately orienting the second QWP–LP pair. Here, the Stokes parameters S1 and S2 show the prevalence of linear polarizations: horizontal with respect to vertical (S1), and diagonal +45° with respect to −45° (S2). More importantly, S3 describes the dominance of RCP with respect to LCP, while all three parameters are normalized to the total intensity (S0). Without the sample, p-polarized excitation corresponds to S1 = 1 (horizontal).
vector. In the output, we resolve the Stokes parameters by appropriately orienting the second QWP–LP pair. Here, the Stokes parameters S1 and S2 show the prevalence of linear polarizations: horizontal with respect to vertical (S1), and diagonal +45° with respect to −45° (S2). More importantly, S3 describes the dominance of RCP with respect to LCP, while all three parameters are normalized to the total intensity (S0). Without the sample, p-polarized excitation corresponds to S1 = 1 (horizontal).
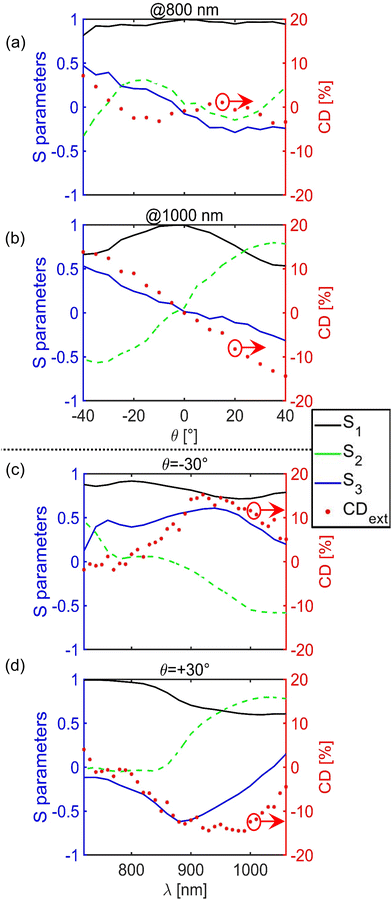
We first measured the Stokes parameters at 800 nm as a function of the incidence angle (Fig. 4a). At this wavelength, there are no particular CD resonances; the red dots represent CDext measured in the same λ–θ configuration. S1 becomes only slightly decreased and converted into S2 and S3. On the other hand, Fig. 4b plots the Stokes parameters and CDext, measured at the broad resonance of 1000 nm. Here, at oblique incidence, linear polarization is strongly converted into right elliptical polarization for negative θ, and into left elliptical polarization for positive θ. S3 remarkably follows the behaviour of CDext, while at normal incidence, the polarization state remains unaffected. We next fixed the incidence angle and controled the wavelength. Fig. 4c shows the wavelength tuning of the polarization state at oblique incidence, θ = −30°. Over the whole wavelength range, S3 agrees with CDext. Interestingly, below 750 nm, while CDext is negligible, S3 decreases with decreasing wavelengths, while S2 increases. Similarly, above 1000 nm, while both CDext and S3 decrease with increasing wavelengths, S2 increases in the absolute value; this could be a signature of optical rotation. Finally, Fig. 4d is close to the mirror image of Fig. 4c for S3 and CDext. Therefore, with the inversion of the incidence angle, extrinsic chirality flips the handedness of the transmitted beam.
Furthermore, we fully characterized the Stokes parameters in the 720–1060 nm range for the incidence angles from θ = −40° to θ = +40°. Fig. 5 clearly shows the conversion of the incident linear polarization into the elliptical and broadband tuning of this effect across different incidence angles. As S1 decreases in broad near-infrared regions for |θ| > 20°, S2 and S3 increase in absolute values, and with opposite signs. Again, neither of the three maps is antisymmetric, due to the influence of the intrinsic chirality. Interestingly, S3 follows the CDext map from Fig. 2. This can be explained by representing the linear polarization as an equal combination of LCP and RCP. At oblique incidence, e.g., for negative angles, LCP becomes strongly absorbed; hence, CDext is positive, and so is the S3 parameter, indicating that the transmitted light is predominantly right in ellipticity.
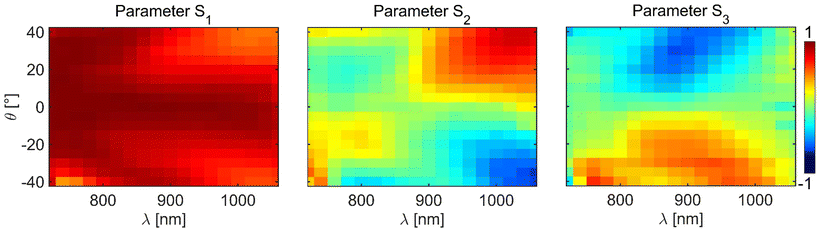 | ||
| Fig. 5 Full characterization of the S parameters of the transmitted light, dependent on the excitation wavelength λ and incidence angle θ. | ||
2.4 Versatile polarization evolution
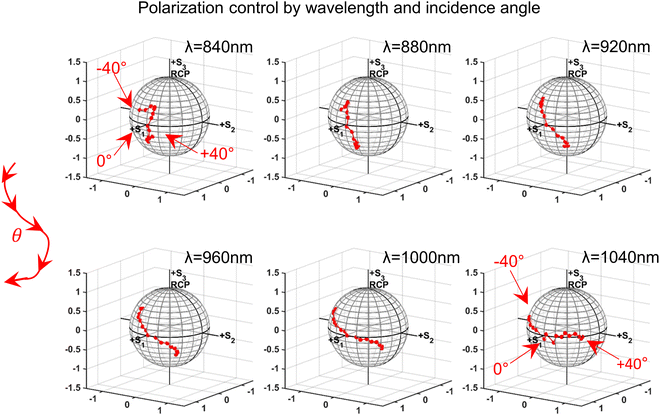
We further represented the evolution of the polarization state at different wavelengths, as the sample is rotated. Fig. 6 shows the path of the polarization state on the Poincaré sphere when the incidence angle θ is changed. In the near-infrared range, for excitation configurations around the CDext resonance, different elliptical polarization states can be finely tuned. At 880 nm, the decrease of the parameter S1 transfers to the enhancement of S3, which reaches values of ±0.5. The polarization state “walks” along the meridian S2 ∼ 0, meaning that it is a right or left elliptical polarization, with respect to the predominant horizontal long axis. The CDext resonance is broad, but as the wavelength is further increased, the ellipse long axis is tilted towards diagonals, and the circular polarization degree decreases as S2 increases. Finally, at 1040 nm, the state stays almost linearly polarized, while its polarization can be gradually changed, following a route along the equator.We next visualize the coupling mechanism from the near-field to the far-field, at 1000 nm. We excite the unit cell with p-polarization, LCP or RCP from the top; the incidence plane is given by the z-direction and a vector perpendicular to the asymmetry,  . Fig. S1† shows that the increase of the incidence angle to |θ| = 30° leads to larger absorption in the plasmonic shell; however, the “total” absorption is largely similar at opposite incidence angles. Extrinsic CD is what is further reflected in the output polarization state because of different interactions between LCP and RCP. In Fig. S2†, we investigated the direction and polarization behaviour of the electric field vector; the white dashed line denotes the substrate surface. As expected, linear polarization at 0° keeps oscillating in line in both transmitted and reflected fields. Increasing the absolute incidence angle to 30° leads to larger coupling with the metasurface, indicated by the strongly enhanced electric field vector above the substrate, with complex near-field behaviour around the asymmetric plasmonic shell. With the switching between +30° and −30°, the transmitted field changes both the handedness and diagonal ellipse tilt. This agrees with the experiment where both S2 and S3 flip signs in a broad range around the resonance. Finally, extrinsic chirality affects the transmitted intensity and polarization, and we can visualize the coupling with RCP and LCP polarizations, observed in the first CDext experiment. At θ = −30°, LCP light is strongly absorbed (also seen in Fig. 3b), while RCP is transmitted better. Indeed, below the metasurface, the intensity for RCP excitation is higher than that for LCP, which also agrees with the positive value of S3 observed in the experiment. This feature is then inverted for θ = +30°. Moreover, apart from coupling with circular polarizations at oblique incidence, we believe that the symmetry of our system could be broken even under normal incidence, with asymmetric helical beams carrying orbital angular momentum.62 Therefore, we envision that such simple asymmetric metasurface geometries have potential in the hot topic of helical dichroism.63
. Fig. S1† shows that the increase of the incidence angle to |θ| = 30° leads to larger absorption in the plasmonic shell; however, the “total” absorption is largely similar at opposite incidence angles. Extrinsic CD is what is further reflected in the output polarization state because of different interactions between LCP and RCP. In Fig. S2†, we investigated the direction and polarization behaviour of the electric field vector; the white dashed line denotes the substrate surface. As expected, linear polarization at 0° keeps oscillating in line in both transmitted and reflected fields. Increasing the absolute incidence angle to 30° leads to larger coupling with the metasurface, indicated by the strongly enhanced electric field vector above the substrate, with complex near-field behaviour around the asymmetric plasmonic shell. With the switching between +30° and −30°, the transmitted field changes both the handedness and diagonal ellipse tilt. This agrees with the experiment where both S2 and S3 flip signs in a broad range around the resonance. Finally, extrinsic chirality affects the transmitted intensity and polarization, and we can visualize the coupling with RCP and LCP polarizations, observed in the first CDext experiment. At θ = −30°, LCP light is strongly absorbed (also seen in Fig. 3b), while RCP is transmitted better. Indeed, below the metasurface, the intensity for RCP excitation is higher than that for LCP, which also agrees with the positive value of S3 observed in the experiment. This feature is then inverted for θ = +30°. Moreover, apart from coupling with circular polarizations at oblique incidence, we believe that the symmetry of our system could be broken even under normal incidence, with asymmetric helical beams carrying orbital angular momentum.62 Therefore, we envision that such simple asymmetric metasurface geometries have potential in the hot topic of helical dichroism.63
3 Conclusion
We have reported on extrinsic chirality and widely tuneable Stokes parameters in simple, low-cost metasurfaces made of asymmetric gold semishells. Starting from measurements of circular dichroism at extinction, we gained insight into the coupling of the metasurface with LCP and RCP; we investigated how the incidence angle and wavelength affect the metamaterial CD resonances in the near-infrared range. We then showed the consequences of the asymmetry on the transmitted field polarization control. We excited the sample with linear polarization and mapped the Stokes parameters over wide ranges of wavelength and incidence angles. The S3 parameter is strongly related to the observed extinction CD, and different paths on the Poincaré sphere can evolve with hyperspectral tuning of the incidence angle. The simulated electromagnetic coupling agrees well with the experiments, and the geometry can be further used for the coupling of emitters.We previously proposed NSL-based geometries for the manipulation of polarization of the emitted field; as seen in ref. 50, we simulated the far-field distribution and polarization for a set of electric dipoles coupled with asymmetric metasurfaces. We observed high polarization degree in the emission, which followed the oblique emission governed by extrinsic chirality. We believe that the present work gives the first experimental proof that the state of output polarizations can be easily manipulated in our low-cost samples and that the coupling mechanisms follow the resonant extrinsic chiral behaviour of such simple geometries. The coupling of a plasmonic layer with molecules emitting in the near-infrared range could be done by doping the substrate below the metasurface, or by burying the metasurface into a dielectric layer doped with emitters. Moreover, given the good numerical model, NSL parameters can now be optimized to give higher CD and lead to circular polarizations of higher purity. Finally, we believe that the simple metasurface arrangement based on a thin asymmetric plasmonic semishell can lead to scalable plasmonic–photonic components for chiro-optical manipulation in the near- and far-fields.
Conflicts of interest
There are no conflicts to declare.Acknowledgements
A. B. acknowledges LASAFEM Sapienza Università di Roma Infrastructure Project No. MA31715C8215A268 and Sapienza Large Research Project 2022 No. RG1221816B6FA2E9, PRIN 2022 PNRR Project INSPIRE n. P2022LETN5 founded by European Union - Next Generation EU. E. P. acknowledges the RTDa–PON “ricerca e innovazione” 2014–2020 for financial and mobility support. T.C. acknowledges the financial support of the Department of Physics and Astronomy through the project “PLANQ” PRD-BIRD-2022-Cesca.References
- F. A. A. Nugroho, D. Albinsson, T. J. Antosiewicz and C. Langhammer, ACS Nano, 2020, 14, 2345–2353 CrossRef CAS PubMed.
- Y. Wang, C. Zhao, J. Wang, X. Luo, L. Xie, S. Zhan, J. Kim, X. Wang, X. Liu and Y. Ying, Sci. Adv., 2021, 7, eabe4553 CrossRef CAS PubMed.
- S. A. Khan, N. Z. Khan, Y. Xie, M. T. Abbas, M. Rauf, I. Mehmood, M. Runowski, S. Agathopoulos and J. Zhu, Adv. Opt. Mater., 2022, 10, 2200500 CrossRef CAS.
- S. I. Bozhevolnyi and N. A. Mortensen, Nanophotonics, 2017, 6(5), 1185–1188 CrossRef.
- J. Zhang, M. ElKabbash, R. Wei, S. C. Singh, B. Lam and C. Guo, Light: Sci. Appl., 2019, 8, 53 CrossRef PubMed.
- A. J. Traverso, J. Huang, T. Peyronel, G. Yang, T. G. Tiecke and M. H. Mikkelsen, Optica, 2021, 8(2), 202–207 CrossRef.
- G. Li, X. Du, L. Xiong and X. Yang, Adv. Opt. Mater., 2023, 11, 2301205 CrossRef CAS.
- J. Bai, C. Wang, X. Chen, A. Basiri, C. Wang and Yu Yao, Photonics Res., 2019, 7, 1051–1060 CrossRef CAS.
- E. Petronijevic, A. Belardini, G. Leahu, R. Li Voti and C. Sibilia, Opt. Mater. Express, 2022, 12(7), 2426–2937 CrossRef.
- H. S. Khaliq, A. Nauman, J.-W. Lee and H.-R. Kim, Adv. Opt. Mater., 2023, 11, 2300644 CrossRef CAS.
- Y. Zhao, A. N. Askarpour, L. Sun, J. Shi, X. Li and A. Alù, Nat. Commun., 2017, 8, 14180 CrossRef CAS PubMed.
- J. García-Guirado, M. Svedendahl, J. Puigdollers and R. Quidant, Nano Lett., 2018, 18(10), 6279–6285 CrossRef PubMed.
- Y. Y. Lee, R. M. Kim, S. W. Im, M. Balamurugan and K. T. Nam, Nanoscale, 2020, 12, 58–66 RSC.
- Y. Liu, Z. Wu, P. S. Kollipara, R. Montellano, K. Sharma and Y. Zheng, ACS Nano, 2021, 15(4), 6448–6456 CrossRef CAS PubMed.
- M. Cen, J. Wang, J. Liu, H. He, K. Li, W. Cai, T. Cao and Y. J. Liu, Adv. Mater., 2022, 34, 2203956 CrossRef CAS PubMed.
- W. Cao, X. Yang and J. Gao, Sci. Rep., 2017, 7, 8841 CrossRef PubMed.
- A. Pors, M. G. Nielsen and S. I. Bozhevolnyi, Optica, 2015, 2(8), 716–723 CrossRef CAS.
- Y. Yang, H. Kim, T. Badloe and J. Rho, Nanophotonics, 2022, 11(17), 4123–4133 CrossRef CAS.
- C. Chen, S. Gao, W. Song, H. Li, S.-N. Zhu and T. Li, Nano Lett., 2021, 21, 1815–1821 CrossRef CAS PubMed.
- Y. Chen, X. Yang and J. Gao, Light: Sci. Appl., 2018, 7, 84 CrossRef PubMed.
- H.-T. Lin, Y.-Y. Hsu, P.-J. Cheng, W.-T. Wang, S.-W. Chang and M.-H. Shih, Nanoscale Adv., 2022, 4, 2428–2434 RSC.
- L. Li, J. Wang, L. Kang, W. Liu, L. Yu, B. Zheng, M. L. Brongersma, D. H. Werner, S. Lan, Y. Shi, Y. Xu and X. Wang, ACS Nano, 2020, 14, 16634–16642 CrossRef CAS PubMed.
- Y. Liang, H. Lin, K. Koshelev, F. Zhang, Y. Yang, J. Wu, Y. Kivshar and B. Jia, Nano Lett., 2021, 21(2), 1090–1095 CrossRef CAS PubMed.
- V. K. Valev, J. J. Baumberg, B. De Clercq, N. Braz, X. Zheng, E. J. Osley, S. Vandendriessche, M. Hojeij, C. Blejean, J. Mertens, C. G. Biris, V. Volskiy, M. Ameloot, Y. Ekinci, G. A. E. Vandenbosch, P. A. Warburton, V. V. Moshchalkov, N. C. Panoiu and T. Verbiest, Adv. Mater., 2014, 26, 4074–4081 CrossRef CAS PubMed.
- J. T. Collins, D. C. Hooper, A. G. Mark, C. Kuppe and V. K. Valev, ACS Nano, 2018, 12(6), 5445–5451 CrossRef CAS PubMed.
- Y. Kan, S. K. H. Andersen, F. Ding, S. Kumar, C. Zhao and S. I. Bozhevolnyi, Adv. Mater., 2020, 32, 1907832 CrossRef CAS PubMed.
- D. Schanne, S. Suffit, P. Filloux, E. Lhuillier and A. Degiron, Phys. Rev. Appl., 2020, 14, 064077 CrossRef CAS.
- Y. Sang and M. Liu, Chem. Sci., 2022, 13, 633 RSC.
- P. Kumar, T. Vo, M. Cha, A. Visheratina, J. Kim, W. Xu, J. Schwartz, A. Simon, D. Katz, V. P. Nicu, E. Marino, W. J. Choi, M. Veksler, S. Chen, C. Murray, R. Hovden, S. Glotzer and N. A. Kotov, Nature, 2023, 615, 418–424 CrossRef CAS PubMed.
- R. Ogier, Y. Fang, M. Svedendahl, P. Johansson and M. Käll, ACS Photonics, 2014, 1(10), 1074–1081 CrossRef CAS.
- E. S. A. Goerlitzer, R. Mohammadi, S. Nechayev, K. Volk, M. Rey, P. Banzer, M. Karg and N. Vogel, Adv. Mater., 2020, 32, 2001330 CrossRef CAS PubMed.
- X. Zhao, J. Wen, L. Li, Y. Wang, D. Wang, L. Chen, Y. Zhang and Y. Du, J. Appl. Phys., 2019, 126, 141101 CrossRef.
- Y. Wang, M. Zhang, Y. Lai and L. Chi, Nano Today, 2018, 22, 36–61 CrossRef CAS.
- A. K. Singh, K. K. Mandal, Y. Gupta, V. S. Abhay Anand, L. Eswaramoorthy, B. Kumar, A. Kala, S. Dixit, V. G. Achanta and A. Kumar, Phys. Rev. Appl., 2023, 19, 044012 CrossRef CAS.
- A. Nemiroski, M. Gonidec, J. M. Fox, P. Jean-Remy, E. Turnage and G. M. Whitesides, ACS Nano, 2014, 8(11), 11061–11070 CrossRef CAS PubMed.
- Y. Guan, Z. Wang, B. Ai, C. Chen, W. Zhang, Y. Wang and G. Zhang, ACS Appl. Mater. Interfaces, 2020, 12(44), 50192–50202 CrossRef CAS PubMed.
- X. Yang, Y. Liu, F. Chen, Q. Lin, R. Chikkaraddy, S. Huang, S. Xian, Y. Hou, J. Du, L. Xia and C. Du, Rare Met., 2024, 43(2), 723–735 CrossRef CAS.
- Y. He, K. Lawrence, W. Ingram and Y. Zhao, Chem. Commun., 2016, 52, 2047 RSC.
- Y. Hou, H. M. Leung, C. T. Chan, J. Du, H. L. Chan and D. Y. Lei, Adv. Funct. Mater., 2016, 26, 7807–7816 CrossRef CAS.
- B. Ai, H. M. Luong and Y. Zhao, Nanoscale, 2020, 12, 2479–2491 RSC.
- J. Qin, L. Deng, T. Kang, L. Nie, H. Feng, H. Wang, R. Yang, X. Liang, T. Tang, J. Shen, C. Li, H. Wang, Y. Luo, G. Armelles and L. Bi, ACS Nano, 2020, 14(3), 2808–2816 CrossRef CAS PubMed.
- A. Belardini, M. Centini, G. Leahu, D. C. Hooper, R. Li Voti, E. Fazio, J. W. Haus, A. Sarangan, V. K. Valev and C. Sibilia, Sci. Rep., 2016, 6, 31796 CrossRef CAS PubMed.
- G. Leahu, E. Petronijevic, A. Belardini, M. Centini, C. Sibilia, T. Hakkarainen, E. Koivusalo, M. Rizzo Piton, S. Suomalainen and M. Guina, Adv. Opt. Mater., 2017, 5(16), 1601063 CrossRef.
- T. Hakkarainen, E. Petronijevic, M. Rizzo Piton and C. Sibilia, Sci. Rep., 2019, 9, 5040 CrossRef PubMed.
- E. Petronijevic, M. Centini, A. Belardini, G. Leahu, T. Hakkarainen and C. Sibilia, Opt. Express, 2017, 25(13), 14148 CrossRef CAS PubMed.
- E. Petronijevic, A. Belardini, G. Leahu, T. Hakkarainen, M. Rizzo Piton, E. Koivusalo and C. Sibilia, Sci. Rep., 2021, 11, 4316 CrossRef CAS PubMed.
- E. Petronijevic, S. A. Dereshgi, M. C. Larciprete, M. Centini, C. Sibilia and K. Aydin, ACS Appl. Nano Mater., 2022, 5(4), 5609–5616 CrossRef CAS.
- E. Petronijevic, G. Leahu, R. Li Voti, A. Belardini, C. Scian, N. Michieli, T. Cesca, G. Mattei and C. Sibilia, Appl. Phys. Lett., 2019, 114, 053101 CrossRef.
- G. Leahu, E. Petronijevic, R. Li Voti, A. Belardini, T. Cesca, G. Mattei and C. Sibilia, Adv. Opt. Mater., 2021, 9, 2100670 CrossRef CAS.
- E. Petronijevic, A. Belardini, T. Cesca, C. Scian, G. Mattei and C. Sibilia, Phys. Rev. Appl., 2021, 16(1), 014003 CrossRef CAS.
- M. C. Giordano, A. Foti, E. Messina, P. G. Gucciardi, D. Comoretto and F. Buatier de Mongeot, ACS Appl. Mater. Interfaces, 2016, 8, 6629–6638 CrossRef CAS PubMed.
- A. Belardini, A. Benedetti, M. Centini, G. Leahu, F. Mura, S. Sennato, C. Sibilia, V. Robbiano, M. C. Giordano, C. Martella, D. Comoretto and F. Buatier de Mongeot, Adv. Opt. Mater., 2014, 2, 208–213 CrossRef.
- L. Zagaglia, S. Zanotti, M. Minkov, M. Liscidini, D. Gerace and L. C. Andreani, Opt. Lett., 2023, 48(19), 5017–5020 CrossRef PubMed.
- Z. El-Ansary, H. P. Ram Kumar, B. Brioual, E. Petronijevic, T. Cesca, C. Scian, G. Mattei, M. El Hasnaoui, C. Sibilia and A. Belardini, Opt. Quantum Electron., 2023, 55, 627 CrossRef.
- E. Plum, X.-X. Liu, V. A. Fedotov, Y. Chen, D. P. Tsai and N. I. Zheludev, Phys. Rev. Lett., 2009, 102, 113902 CrossRef CAS PubMed.
- L. Mao, K. Liu, S. Zhang and T. Cao, ACS Photonics, 2020, 7(2), 375–383 CrossRef CAS.
- T. Cao, L. Mao, H. Fan, M. Lian, J. Jia, Y. Su and H. Ren, Adv. Opt. Mater., 2023, 11, 2202239 CrossRef CAS.
- H. Ali, E. Petronijevic, G. Pellegrini, C. Sibilia and L. C. Andreani, Opt. Express, 2023, 31(9), 14196–14211 CrossRef CAS PubMed.
- L. Cerdán, L. Zundel and A. Manjavacas, ACS Photonics, 2023, 10(6), 1925–1935 CrossRef PubMed.
- B. Lyu, Y. Li, Q. Jia, H. Li, G. Yang, F. Cao, S. Kou, D. Liu, T. Cao, G. Li and J. Shi, Laser Photonics Rev., 2023, 17, 2201004 CrossRef.
- Lumerical Solutions, Inc. https://www.lumerical.com/tcadproducts/fdtd/.
- J. Bégin, A. Jain, A. Parks, F. Hufnagel, P. Corkum, E. Karimi, T. Brabec and R. Bhardwaj, Nat. Photonics, 2023, 17, 82–88 CrossRef.
- J. Ni, S. Liu, G. Hu, Y. Hu, Z. Lao, J. Li, Q. Zhang, D. Wu, S. Dong, J. Chu and C. Qiu, ACS Nano, 2021, 15(2), 2893–2900 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d3nr06085c |
| This journal is © The Royal Society of Chemistry 2024 |