Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
First-principles study of highly sensitive graphene/hexagonal boron nitride heterostructures for application in toxic gas-sensing devices
Viet Bac T. Phung *a,
Ba Lich Pham
*a,
Ba Lich Pham b,
Nguyen Vo Anh Duy
b,
Nguyen Vo Anh Duy c,
Minh Triet Dang
c,
Minh Triet Dang d,
Thi Nhan Tran
d,
Thi Nhan Tran e,
Quang-Huy Tran
e,
Quang-Huy Tran f,
Thi Theu Luong
f,
Thi Theu Luong g and
Van An Dinh
g and
Van An Dinh *h
*h
aCenter for Environmental Intelligence and College of Engineering & Computer Science, Vin University, Hanoi 100000, Vietnam. E-mail: bac.ptv@vinuni.edu.vn
bInstitut de Chimie Physique, Faculté des Sciences d’Orsay, Université Paris-Saclay, Orsay, 91405, France
cFPT University, Can Tho Campus, 600 Nguyen Van Cu Street, Ninh Kieu, Can Tho, Vietnam
dSchool of Education, Can Tho University, 3-2 Road, Can Tho, Vietnam
eFaculty of Fundamental Sciences, Hanoi University of Industry, 298 Cau Dien Street, Bac Tu Liem District, Hanoi 100000, Vietnam
fFaculty of Physics, Hanoi Pedagogical University 2, Phuc Yen, Vinh Phuc, Vietnam
gHoa Binh University, Bui Xuan Phai Str., My Dinh II, Nam Tu Liem, Hanoi 100000, Vietnam
hDepartment of Precision Engineering, Graduate School of Engineering, Osaka University, 2-1 Yamadaoka, Suita, Osaka 565-0871, Japan. E-mail: divan@prec.eng.osaka-u.ac.jp
First published on 6th February 2024
Abstract
Graphene-based sensors exhibit high sensitivity, fast response, and good selectivity towards toxic gases but have low mechanical stability. The combination of graphene and two-dimensional hexagonal boron nitride (h-BN) is expected to increase the mechanical stability and enhance the adsorption performance of these gas sensors. Using first-principles calculations, we demonstrate that two-dimensional graphene/h-BN double layers can be used as good substrates for gas sensors with a small lattice mismatch of only 1.78%. Moreover, the presence of a h-BN layer widens the band gap by about 38 meV and considerably increases the work function, thus positively affecting the gas adsorption performance. Although these graphene/h-BN heterostructures do not change the physical adsorption mechanism of these sensors concerning the graphene-based materials, these bilayers significantly enhance the sensitivity of these sensors for detecting CO2, CO, NO, and NO2 toxic gases. Particularly, compared to the pristine graphene-based materials, the gas adsorption energies of graphene/h-BN increased by up to 13.78% for the adsorption of NO, and the shortest distances between the graphene/h-BN substrates and adsorbed gas molecules decreased. We also show that the graphene/h-BN heterostructure is more selective towards NOx gases while more inert towards COx gases, based on the different amounts of charge transferred from the substrate to the adsorbed gas molecules. Using the non-equilibrium Green functions in the context of density functional theory, we quantitatively associated these charge transfers with the reduction of the current passing through these scattering regions. These results demonstrate that graphene/h-BN heterostructures can be exploited as highly sensitive and selective room-temperature gas sensors for detecting toxic gases.
1. Introduction
Highly sensitive and selective toxic gas sensors have received great attention for their industrial and pharmaceutical applications. Typically, most conventional low-cost metal oxide gas sensors work at high temperatures,1 raising significant concerns in terms of energy consumption for operation and safety issues. Embedding noble metals (Pd, Pt, Au, Ag, and so on) on the surface of metal oxides could reduce the operating temperature of gas sensors because these noble metal atoms act as catalysts promoting dissociation and reducing the activation energy of the adsorbed gas molecules.2,3 Two-dimensional (2D) materials such as graphene, monolayer phosphorene, and transition metal dichalcogenide compounds are considered good candidates for high-performance room temperature gas sensors, which can be substituted for traditional metal oxide materials.4–8Graphene, a single layer of graphite formed by hexagonal structures, has been of great interest for both academic and industrial applications since it was first discovered in 2004 by the group of A. Geim and K. Novoselov.9 Its low-energetic electronic structure, resembling two-dimensional dirac massless fermion dynamics at the speed of light c, replaced by the Fermi velocity vf ≈ c/300, makes ultrarelativistic physics observable for this material.9,10 Graphene has been considered a promising material for flexible and transparent electronic applications owing to its extraordinary electronic and mechanical properties resulting from a perfect hexagonal crystal lattice. However, it is challenging to make graphene into nanoelectronic devices due to the mechanical instability of a freestanding graphene layer. Recent attempts11–16 to assemble graphene layers on the top of silicon dioxide (SiO2), gallium arsenide (GaAs), indium gallium arsenide (InGaAs), and hexagonal boron nitride (h-BN) layers have been widely studied to significantly surmount the mechanical instability of pristine graphene and to enhance the electronic and optical properties of graphene.9
In the field of gas sensing, research has been devoted to expanding these 2D materials into van der Waals (vdW) 2D heterostructures to directly control the electronic structure of graphene-like materials, such as actively opening up the graphene band gaps.12,14,17 However, to successfully assemble a graphene layer on top of a substrate, the chosen substrate should share some geometrical similarity to graphene to maintain its mechanical and electronic stability. Hexagonal boron nitride is an excellent candidate for this purpose because graphene and h-BN monolayers share a similar lattice structure with a lattice mismatch of only ∼1.5%, a smooth surface without any significant charge accumulators, and high-temperature stability.13,14,18–21
Despite many efforts to explore the electronic structure of graphene on an h-BN substrate, the influence of h-BN layers on the adsorption behavior of graphene towards adsorbed toxic gas molecules is still poorly understood. Herein, we have used first-principles methods based on density functional theory (DFT)22,23 to investigate the structural stability, and electronic and electron transport properties of graphene/h-BN heterostructures upon the adsorption of toxic gas molecules. First, we performed DFT optimization calculations of each single layer and then calibrated the energy profile of these heterostructures as a function of the interlayer distance between these two layers to obtain the most relaxed geometric configuration of graphene/h-BN heterostructure. The electronic properties of the most stable structure were compared and discussed with those of the single layers. We then investigated the adsorption mechanism of pristine graphene and graphene/h-BN upon the adsorption of toxic gases, including CO2, CO, NO, and NO2. To increase the reliability of these electronic calculations, we took into account the vdW interactions between these substrates and the adsorbed molecules. To evaluate the selectivity of graphene/h-BN heterostructures as advanced toxic gas sensors, we also employed non-equilibrium Green's function formalism with density functional methods to calculate the electron transport properties across these adsorbed systems.
2. Computational method
In this work, all periodic DFT calculations were performed using the projector-augmented wave (PAW) method24 implemented in the Vienna Ab initio simulation package (VASP).25,26 To resolve the interlayer interactions between graphene and h-BN, we included the van der Waals dispersion corrections and evaluated multiple exchange–correlation functionals in the non-local vdW functional family, such as optPBE-vdW,27 revPBE-vdW,28 and vdW-DF2.29 Such vdW corrections provide reasonable comparisons to experimental observations for heterostructures. The integration in the Brillouin zone was employed using the Gamma-centered 6 × 6 × 1 and 12 × 12 × 1 k-points for the geometry optimization and electronic calculations, respectively, with an energy cutoff of 520 eV. Lattice parameters and atom coordinates were optimized until the Hellmann–Feynman force acting on each atom was less than 0.01 eV Å−1 and the energy difference between two consecutive steps was less than 10−5 eV. To avoid the atomic interactions between the imaged periodic systems, a vacuum of 22 Å thickness was employed between the neighboring layers. All visualizations of atomic configurations and charge density presented in this work have been visually displayed by the VESTA package developed by K. Momma and F. Izumi.30To evaluate the adsorption mechanism of the toxic gas molecules on these heterostructures, a 3 × 3 supercell of graphene/h-BN heterostructures was adopted. Upon adsorption, the precise molecule positions and orientations are crucial for evaluating the optimal geometrical configurations. Thus, we employed the high-resolution computational DFT-based Nanoscope tool31–33 to scan through all possible configurations to obtain the potential energy surface (PES) and the binding energy profile. This tool uses the adsorbate as a scanning tip, moves the gas molecule across the graphene or graphene/h-BN heterostructures, and rotates around its center of mass34 to obtain the high-resolution energy landscape. The potential energy landscape or the adsorption energy of the system can be determined as follows:31,32
| Eads = Ecomplex − Esaturation, | (1) |
The binding energy of graphene/h-BN heterostructures is calculated as follows:
| Ebinding = EG/h-BN − (EG + Eh-BN), | (2) |
To estimate the selectivity of the graphene/h-BN, we investigated the transport behaviors of graphene/h-BN upon the adsorption of toxic gases by employing non-equilibrium Green's function35 (NEGF) with the density functional formalisms implemented in the Amsterdam Modelling Suite36 to compute the transmission functions, which indicated the number of electrons transferred across the scattering region between two electrodes. The electrodes are composed of pristine graphene/h-BN bilayers with the exact size and shapes of the pristine scattering region. The transmission function T(E, Vb) at an energy E under an applied voltage Vb is calculated as follows:37,38
| T(E, Vb) = Tr[G(E)ΓL(E)G+(E)ΓR(E)], | (3) |
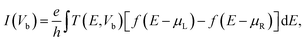 | (4) |
3. Results and discussion
3.1. The graphene/h-BN bilayer heterostructure
The lattice mismatch is a parameter that illustrates the potential to assemble a long-period moiré superstructure without rotation between two different lattices. In other words, the strain effect on the electronic properties of graphene and h-BN can be ignored if this quantity is sufficiently small. The lattice mismatch ε0 is estimated by using the following relation:
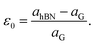 | (5) |
We optimized the geometric structure of graphene and h-BN sheets using the revPBE-vdW functional. The obtained lattice constants for the graphene and h-BN layers are a = b = 2.468 Å and a = b = 2.512 Å, respectively, which are in agreement with previous studies.41,42 Our calculated lattice mismatch between the graphene and h-BN monolayer is relatively small, ε0 ∼ 1.78% (as shown in Table 1), in excellent agreement with previous studies.40,41,43,44 Such a small lattice mismatch demonstrates the possibility of assembling long-range superstructures from these layers. Thus, graphene/h-BN is more beneficial for assembling superstructures as compared to the other heterostructures such as h-BN/phosphorene (5%) and silicene/graphene (<4%), and is as good as phosphorene/borophene (<2%) bilayers.
We evaluated the stacking order dependence of graphene/h-BN heterostructures before adsorbing toxic gas molecules. It is known that there are three possible stacking configurations to assemble a graphene monolayer on top of an h-BN substrate: (1) AA stacking with C atoms in graphene located on top of B and N atoms; (2) AB stacking with C atoms of the upper graphene layer either on top of N atoms or at the center of h-BN hexagon structures as displayed in Fig. 1; (3) AB stacking with C atoms of the upper graphene layer either on top of B atoms or at the center of h-BN hexagons. Instead of manually placing a graphene layer on an h-BN hexagon, as mentioned above, we developed a more robust scanning method by fine-tuning the upper layer horizontally and later vertically to obtain the potential energy surface (PES). The most stable geometrical stacking configuration and the interlayer distance between the graphene and h-BN layers were determined based on the PES profile (Fig. 2(a)). The color gradient on the right of Fig. 2(a) represents the relative energy difference with respect to the energy value of the most stable configuration with X and Y being fractional coordinates based on the lattice vectors of the XY-plane of the graphene unit cell in the simulation model. The vertical axis ΔE in Fig. 2(a) displays the relative energy value. The upper color surface indicates the three-dimensional image of PES, which projected PES on the surface of a unit cell of graphene as shown by the lower one. Dark-colored regions (indicating low energy) correspond to the favorable adsorption sites of the C atom of the graphene layer on the h-BN, while brightly colored regions (exhibiting high energy) represent the less favorable sites. We found that the AB stacking with C atoms located on top of B atoms as illustrated in Fig. 1 is the most stable geometrical stacking. This approach allows the extraction of the most stable geometrical stacking configuration at high accuracy. After determining the most favorable stacking configuration, we rotated the graphene around the z-axis to calculate the binding energy versus the interlayer distance between the graphene and h-BN displayed in Fig. 2(b). Finally, we extracted the most stable configuration of a graphene layer on top of a h-BN with a minimum binding energy.
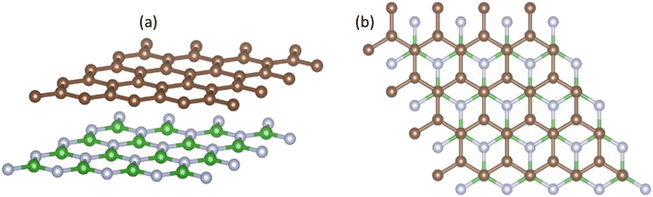 | ||
| Fig. 1 Side view (a) and top view (b) of the graphene/h-BN heterostructures with AB stacking. Boron atoms are marked in green, nitrogen in light blue, and carbon in brown. | ||
To understand the underlying mechanism of this stacking order dependence, we further examined the charge density profile of the graphene/h-BN heterostructures. As indicated in Fig. 2(c) and (d), in the graphene layer, the electron cloud mainly surrounds the C–C bonds in hexagonal honeycombs, while, in the lower h-BN layer, this cloud is mostly distributed around nitrogen atoms since these atoms have a larger electron affinity than those of boron atoms (i.e. electronegativity χN = 3.04 > χB = 2.04). Thus, nitrogen atoms act as anions, whereas boron atoms are cations, inducing attractive interactions towards the electron cloud above, whereas the nitrogen anions located at the center of graphene hexagons repel the graphene's electron cloud. The balance between the attractive and the repulsive forces results in the most stable AB stacking pattern.
Taking the most stable AB stacking configuration with C atoms on top of B atoms, we evaluated the equilibrium interlayer distances between graphene and h-BN and the corresponding binding energies with various exchange–correlation functionals. Table 2 presents the interlayer distances and the corresponding binding energies with multiple exchange–correlation functionals such as revPBE-vdW, optPBE-vdW, and vdW-DF2. Among these functionals, the optimized distance between h-BN and graphene obtained from the revPBE-vdW functional is in quantitative agreement with the experimental value of 3.33 Å reported in ref. 39 and 44. To evaluate the sensitivity of these bilayers for application in gas sensing devices, we further computed the work function (WF) using the revPBE-vdW functional. The calculated WF is 4.28 eV, which is quite close to the value calculated in the previous work (4.44 eV)42 and given by a previous experiment (4.57 eV).45 It was reported that the theoretical WF of h-BN is 5.986 eV,46 which is considerably higher than that of pristine graphene. In the presence of h-BN sheets, the WF of graphene increases considerably, from 4.281 eV to 4.507 eV, implying the modification in the graphene surface potential and interface electronic properties caused by the interaction with h-BN. The WF of graphene/h-BN is comparable to the WF values of the other common semiconductor materials such as the neutral WO3 monolayer (6.97 eV)47 and anode materials such as Cu4S4 (5.42 eV),48 demonstrating that these heterostructures are suitable for application in gas-sensing devices.
| vdW-DFT functionals/characteristics | revPBE-vdW | optPBE-vdW | vdW-DF2 |
|---|---|---|---|
| Distance dz (Å) | 3.252 | 3.250 | 3.552 |
| Response length (Å) | 10.052 | 9.313 | 8.921 |
| Binding energy (eV) | 0.910 | 1.169 | 0.881 |
| Work function (eV) | 4.507 | 4.528 | 4.498 |
3.2. The electronic structure of the graphene/h-BN bilayer
To investigate the influence of the h-BN substrate on the gas response of graphene, we calculated the electronic structure and density of states (DOS) of the relaxed graphene/h-BN. The electronic band structures and DOS analysis with the revPBE-vdW functional are shown in Fig. 3. The Fermi level displayed by dotted lines in Fig. 3, was set up at the highest occupied molecular orbital (HOMO) state. It is widely recognized that pristine graphene possesses a band structure with zero band gap, reducing its sensitivity and selectivity to a wide range of gases. However, the electronic band structure of graphene/h-BN exhibited a gap opening of 38 meV (see the inserted image in Fig. 3), with a binding energy of about 0.056 eV per carbon atom. The interaction with the h-BN sheet helps to open the band gap of graphene by breaking the geometric symmetry of graphene, similar to that happening when applying the strain effect,49 as well as doping boron or nitrogen atoms.50 Additionally, the separation between the HOMO and the lowest unoccupied molecular orbital (LUMO) of the h-BN layer in interaction with graphene is ∼5.2 eV, close to the HOMO–LUMO separation of pristine h-BN. The DOS image around the Fermi level of graphene/h-BN presented in Fig. 3(c) exhibits an inconsiderable change with respect to that of pristine graphene. Graphene/h-BN heterostructures retained the electronic characteristics of both graphene and h-BN.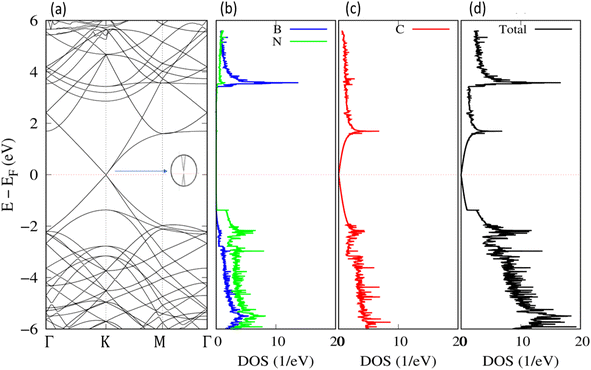 | ||
| Fig. 3 Band structure along the Γ–K–M–Γ path in reciprocal space (a) and DOS for the relaxed structure of graphene/h-BN: (b) h-BN; (c) graphene; and (d) total DOS. | ||
3.3. The influence of the h-BN substrate on the gas adsorption of graphene
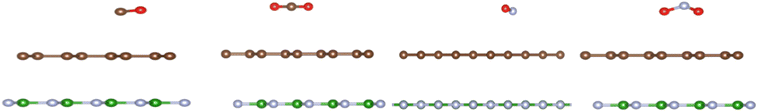 | ||
| Fig. 4 The optimized structures (from left to right) of CO, CO2, NO, and NO2 adsorbed on graphene/h-BN. | ||
| Gases | Characteristics | Gas on G/h-BN | Gas on the pristine graphene | ||||
|---|---|---|---|---|---|---|---|
| revPBE | optPBE | DFT-DF2 | revPBE | optPBE | DFT-DF2 | ||
| CO | d(gas-G) (Å) | 3.383 | 3.276 | 3.425 | 3.432 | 3.334 | 3.418 |
| Eads (eV) | 0.171 | 0.202 | 0.161 | 0.162 | 0.196 | 0.160 | |
| Recovery time τ (ns) | 0.74 | 2.47 | 0.51 | 0.53 | 1.96 | 0.49 | |
| Work function (eV) | 4.531 | 4.443 | 4.532 | 4.452 | 4.562 | 4.545 | |
| Charge transfer (e) | 0.007 | — | — | 0.008 | — | — | |
| CO2 | d(gas-G) (Å) | 3.361 | 3.320 | 3.535 | 3.373 | 3.312 | 3.536 |
| Eads (eV) | 0.226 | 0.259 | 0.218 | 0.214 | 0.262 | 0.209 | |
| Recovery time τ (ns) | 6.24 | 22.37 | 4.58 | 3.93 | 25.12 | 3.24 | |
| Work function (eV) | 4.531 | 4.551 | 4.525 | 4.433 | 4.572 | 4.519 | |
| Charge transfer (e) | 0.011 | — | — | 0.015 | — | — | |
| NO | d(gas-G) (Å) | 3.117 | 3.095 | 3.312 | 3.119 | 3.106 | 3.234 |
| Eads (eV) | 0.223 | 0.268 | 0.213 | 0.196 | 0.213 | 0.180 | |
| Recovery time τ (ns) | 5.56 | 3.17 | 3.78 | 1.96 | 3.78 | 1.05 | |
| Work function (eV) | 4.674 | 4.456 | 4.387 | 4.598 | 4.657 | 4.526 | |
| Charge transfer (e) | 0.049 | — | — | 0.056 | — | — | |
| NO2 | d(gas-G) (Å) | 3.156 | 3.098 | 3.265 | 3.194 | 3.123 | 3.269 |
| Work function (eV) | 5.648 | 4.352 | 4.498 | 5.668 | 4.364 | 4.535 | |
| Eads (eV) | 0.314 | 0.318 | 0.312 | 0.312 | 0.307 | 0.303 | |
| Recovery time τ (ns) | 190 | 220 | 170 | 170 | 140 | 120 | |
| Charge transfer (e) | 0.196 | — | — | 0.194 | — | — | |
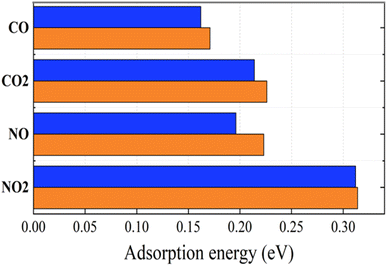 | ||
| Fig. 6 Adsorption energies of toxic gases on graphene (blue) and graphene/h-BN (red) with the revPBE -vdW functional arranged in increasing order from upper to lower. | ||
We calculated the WFs of gas + graphene and gas + graphene/h-BN systems. As listed in Table 3, there is a small difference between the WFs calculated for gas + graphene and gas + graphene/h-BN in almost all cases. However, in the case of NO2 gases, we observed a noticeable difference between these two substrates, indicating the considerable influence of the h-BN substrate on the WFs of graphene-gas systems.
The change in the electrical signal of graphene or graphene/h-BN, caused by the charge transfer inside the adsorption systems, can be exploited to detect the presence of toxic gases. Table 3 presents the charge transfer values of gas + graphene and gas + graphene/h-BN. It shows that four toxic gases function as electron acceptors, implying the reduction of the conductivity of the graphene/h-BN upon gas adsorption. The charge transfer amount in increasing order is as follows: CO < CO2 < NO < NO2, in quantitative agreement with the fact that the shortest distances between the adsorbed gas molecule and the graphene are in the inverse order. Furthermore, the charge transfers from the adsorbents to NO and NO2 molecules are much greater than those to CO and CO2 molecules. This predicts a considerable reduction in the electrical signal of graphene and graphene/h-BN caused by the adsorption of NO and NO2. The calculated results of charge transfer exhibit the high selectivity of graphene for NO and NO2 in the cases without and with a monolayer h-BN. Table 3 indicates that upon introducing h-BN below the graphene, CO, CO2, NO, and NO2 gases received 0.007 e, 0.011 e, 0.049 e, and 0.196 e, respectively. Compared to the adsorption of these gases on pristine graphene, the electron transfer amount from the graphene/h-BN to adsorbed gas molecules decreased, excluding the increase in the electron transfer amount of 1% from the adsorbent to the NO2. The charge transfer decreased by 36.4% for the adsorption of CO2 and by 14.3% for the adsorption of CO. Possibly, the increase in the WFs due to the formation of graphene/h-BN heterostructures, as mentioned above, leads to the impairment of electron transfer. The decrease in the electron transfer amount implies that graphene/h-BN heterostructures are more inert to COx (x = 1, 2) gases as compared to pristine graphene. For NO2 gas, because there is an increase in the electron transfer amount from the adsorbent to the NO2, it can be predicted that the graphene/h-BN heterostructure is more selective toward NO2 than pristine graphene. Although the electron transfer amount from graphene/h-BN to NO decreased by 13.4% as compared to that from pristine graphene to NO molecule, the charge transfer amount is quite significant. Therefore, graphene/h-BN retained a good selectivity for NO as compared to pristine graphene. Graphene/h-BN heterostructures can be exploited as good gas sensing devices to distinguish NOx and COx as indicated in a previous report.53
The recovery time τ is an important parameter for evaluating the performance of gas sensors. It is the time required for gas molecules to dislodge from the material surface, calculated as follows:8
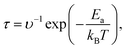 | (6) |
In the context of the adsorption of toxic gases on graphene and the graphene/h-BN bilayer, comparing the DOS can provide insights into the changes in the electronic structure of the system upon adsorption. Fig. 7 displays the spin polarization of graphene and graphene/h-BN upon the adsorption of NO and NO2, while this effect does not occur for the adsorption of CO and CO2. In other words, the introduction of monolayer h-BN does not cause spin polarization for the adsorption of CO and CO2 but it modifies the spin polarization upon the adsorption of NO and NO2 with the appearance of novel polarized states in high densities around the Fermi level. Because any changes in the electronic band structure may affect the adsorption behavior, the highest increase in the adsorption energy of 13.78% for NO, due to the introduction of two-dimensional h-BN, could be affected by the strong change in spin polarization.
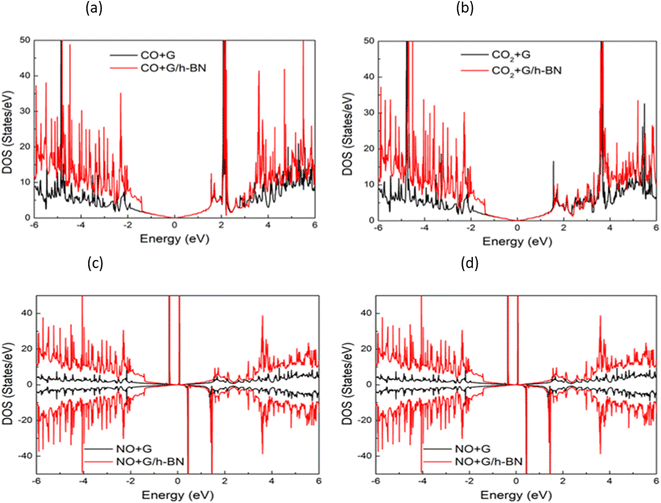 | ||
| Fig. 7 Comparison of total density of states of graphene and graphene/h-BN with different toxic gas adsorption: NO (a), NO2 (b), CO (c), and CO2 (d). | ||
To further explain the physical origin of the interaction of the adsorption systems and evaluate the influence of h-BN on the adsorption behavior of graphene, we performed periodic energy decomposition analyses (PEDA)54,55 implemented in the Amsterdam Modelling Suite36 to calculate the intrinsic bond energies ΔEint and their partial energies.
| ΔEint = ΔEeslast + ΔEPauli + ΔEorb, | (7) |
| Substrate | PEDA types | CO | CO2 | NO | NO2 |
|---|---|---|---|---|---|
| Graphene/h-BN | ΔEint | −39 | −48 | −479 | −156 |
| ΔEPauli | 35 | 53 | 151 | 108 | |
| ΔEelstat | −32 | −56 | −75 | −84 | |
| ΔEorb | −42 | −45 | −556 | −180 | |
| Graphene | ΔEint | −47 | −66 | −545 | −210 |
| ΔEPauli | 34 | 53 | 244 | 90 | |
| ΔEelstat | −43 | −76 | −144 | −93 | |
| ΔEorb | −37 | −43 | −645 | −207 |
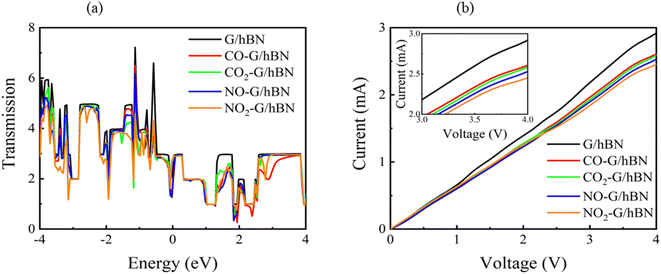 | ||
| Fig. 8 Transmission spectra at zero bias voltage (a) and current–voltage profile (b) of graphene/h-BN heterostructures upon the adsorption of toxic gases. | ||
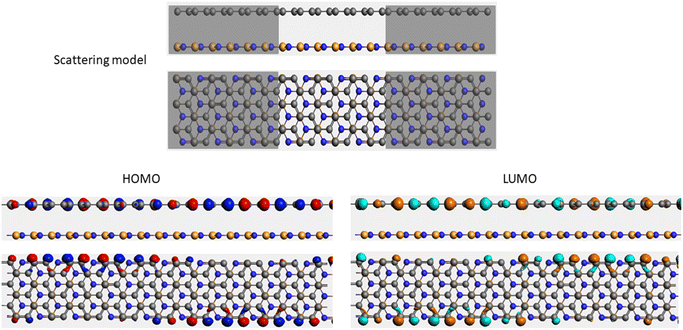
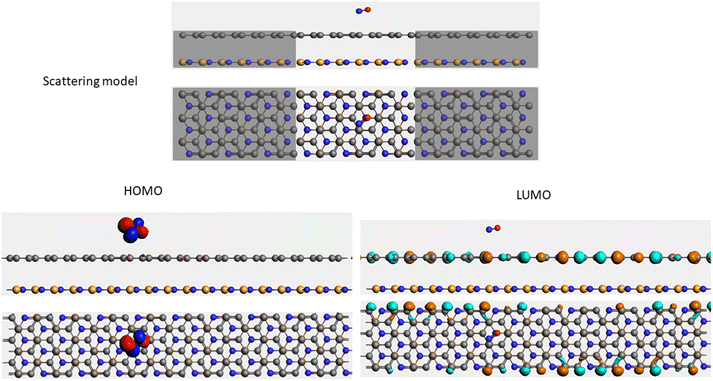
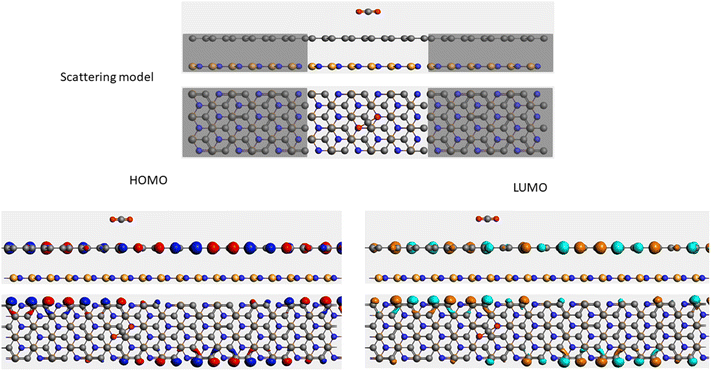
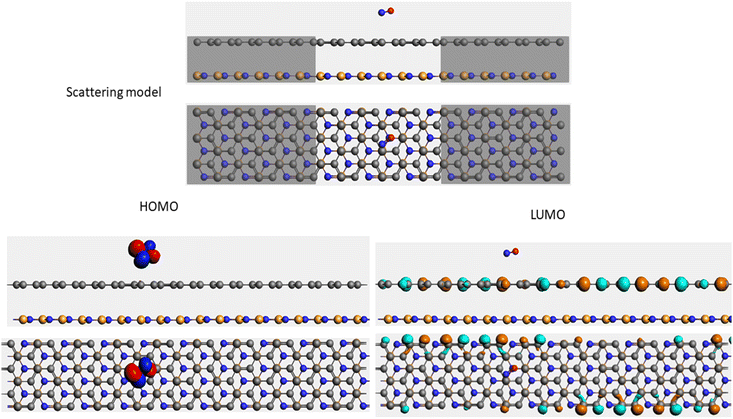
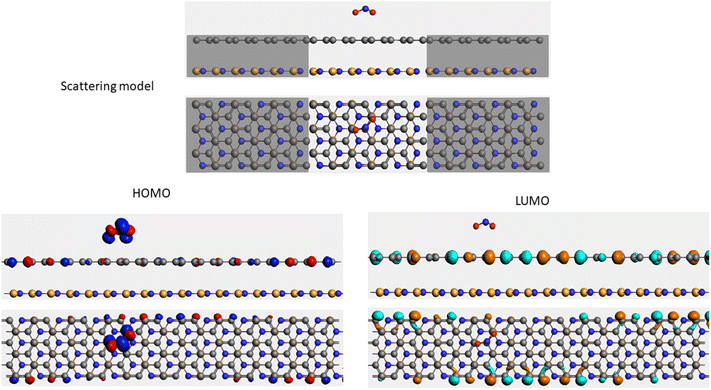
It is well-known that the electronic transport properties of materials are governed by the electrons of the frontier orbitals. To gain further insight into the effects of toxic gas adsorption on the electronic properties of graphene/h-BN heterostructure GQDs, we analyzed the modification of the HOMO and LUMO shapes of the most stable graphene/h-BN due to the interaction with the gas molecules. The LUMO (HUMO) shapes are displayed in Table 5, in which the charge accumulation regions are in red (orange), whereas the charge depletion areas are in blue (green). As shown in Table 5, the LUMO schema of the scattering region changed inconsiderably, whereas the HOMO of graphene/h-BN heterostructures exhibited a considerable modification. The interaction between graphene/h-BN heterostructures and gas molecules leads to the redistribution of electrons of the HOMO on the surfaces of the scattering regions. The electrons of the HOMO of the graphene/h-BN upon adsorption of CO, NO, and NO2 are located around the adsorbed molecules instead of being distributed at the edge of the scattering region for the graphene/h-BN without gas molecules on the surface. For the adsorption of CO2, the electron density of the HOMO was observed to decrease with respect to that of the of the graphene/h-BN. This indicates a transfer of electrons from the HOMO state of graphene to the adsorbed molecules. The redistribution of the electrons of the HOMO state caused by the gas adsorption led to a decrease in the current signals as illustrated in Fig. 8(b).
4. Conclusions
We have presented a systematic study on the effects of h-BN substrate on the gas adsorption performance of graphene via first-principles calculations using vdW functionals. We found that embedding graphene on the top of a two-dimensional monolayer h-BN boosts the mechanical stability with a small mismatch and tunes the electronic properties of graphene, including a gap opening of 38 meV and an increase in the work function. The changes in the electronic properties positively impact the adsorption performances of graphene toward CO, CO2, NO, and NO2. When the orbital relaxation energy is the dominant factor, the introduction of the h-BN substrate changes the orbital relaxation, enhancing the sensitivity of graphene to NO2, such as increasing the adsorption energies and the reduction of the shortest distances between the graphene/h-BN and the adsorbed gas molecules. The graphene/h-BN heterostructure exhibited a greater selectivity for NO2 gas and inertness toward COx gases. Although there were several changes in the gas adsorption properties with respect to pristine graphene, the graphene/h-BN heterostructure still exhibited physisorption to toxic gases similar to pristine graphene. The conductivity of graphene/h-BN heterostructures is predicted to remarkably decrease when adsorbing toxic gases because the adsorbed molecules work as charge acceptors. The decrease in the conductivity strongly depends on the type of adsorbed gas, indicating the selectivity of the graphene/h-BN heterostructures. The findings show the potential for the application of graphene and h-BN heterostructures as room-temperature gas sensors with high performance.Author contributions
All authors have approved the final version of the manuscript.Conflicts of interest
The authors declare no competing financial interest.Acknowledgements
The authors gratefully acknowledge the JICA project at VNU Vietnam Japan University for providing the high-performance computing (HPC) resources.References
- C. Zhang, Y. Luo, J. Xu and M. Debliquy, Sens. Actuators, A, 2019, 289, 118–133 CrossRef CAS.
- Q. Rong, B. Xiao, J. Zeng, R. Yu, B. Zi, G. Zhang, Z. Zhu, J. Zhang, J. Wu and Q. Liu, ACS Sens., 2022, 7, 199–206 CrossRef CAS PubMed.
- R. Chen, S. Luo, D. Xie, Y. Yu and L. Xiang, Chemosensors, 2022, 10, 329 CrossRef CAS.
- D. Chen, X. Zhang, J. Tang, H. Cui, Z. Chen and Y. Li, Sustainable Mater. Technol., 2019, 21, e00100 CrossRef CAS.
- B. Wang, Y. Gu, L. Chen, L. Ji, H. Zhu and Q. Sun, Nanotechnology, 2022, 33, 252001 CrossRef PubMed.
- J. Zhang, L. Liu, Y. Yang, Q. Huang, D. Li and D. Zeng, Phys. Chem. Chem. Phys., 2021, 23, 15420–15439 RSC.
- E. Mansouri, J. Karamdel, M. Berahman and M. T. Ahmadi, Phys. Status Solidi A, 2019, 216, 1800086 CrossRef.
- V. B. T. Phung, T. N. Tran, Q. H. Tran, T. T. Luong and V. A. Dinh, ACS Omega, 2024, 9, 2302–2313 CrossRef CAS PubMed.
- K. S. Novoselov, A. K. Geim, S. V Morozov, D. Jiang, M. I. Katsnelson, I. V Grigorieva, S. V Dubonos and A. A. Firsov, Nature, 2005, 438, 197–200 CrossRef CAS PubMed.
- K. S. Novoselov, A. K. Geim, S. V Morozov, D. Jiang, Y. Zhang, S. V Dubonos, I. V Grigorieva and A. A. Firsov, Science, 2004, 306, 666–669 CrossRef CAS PubMed.
- Y.-J. Kang, J. Kang and K. J. Chang, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 78, 115404 CrossRef.
- M. Ishigami, J. H. Chen, W. G. Cullen, M. S. Fuhrer and E. D. Williams, Nano Lett., 2007, 7, 1643–1648 CrossRef CAS PubMed.
- H. Hirai, H. Tsuchiya, Y. Kamakura, N. Mori and M. Ogawa, J. Appl. Phys., 2014, 116, 083703 CrossRef.
- A. González-García, W. López-Pérez, R. González-Hernández, J. A. Rodríguez, M. V Milośević and F. M. Peeters, J. Phys.: Condens. Matter, 2019, 31, 265502 CrossRef PubMed.
- A. J. Green and A. C. Diebold, Microsc. Microanal., 2014, 20, 1753–1763 CrossRef CAS PubMed.
- M. Friedemann, K. Pierz, R. Stosch and F. J. Ahlers, Appl. Phys. Lett., 2009, 95, 102103 CrossRef.
- V. Van Quang, N. Van Dung, N. Sy Trong, N. Duc Hoa, N. Van Duy and N. Van Hieu, Appl. Phys. Lett., 2014, 105, 013107 CrossRef.
- B. Arnaud, S. Lebègue, P. Rabiller and M. Alouani, Phys. Rev. Lett., 2006, 96, 26402 CrossRef CAS PubMed.
- G. Giovannetti, P. A. Khomyakov, G. Brocks, P. J. Kelly and J. van den Brink, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 76, 73103 CrossRef.
- X. Blase, A. Rubio, S. G. Louie and M. L. Cohen, Phys. Rev. B: Condens. Matter Mater. Phys., 1995, 51, 6868–6875 CrossRef CAS PubMed.
- P. Järvinen, S. K. Hämäläinen, K. Banerjee, P. Häkkinen, M. Ijäs, A. Harju and P. Liljeroth, Nano Lett., 2013, 13, 3199–3204 CrossRef PubMed.
- P. Hohenberg and W. Kohn, Phys. Rev., 1964, 136, B864–B871 CrossRef.
- W. Kohn and L. J. Sham, Phys. Rev., 1965, 140, A1133–A1138 CrossRef.
- G. Kresse and D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758–1775 CrossRef CAS.
- G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 49, 14251–14269 CrossRef CAS PubMed.
- G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15–50 CrossRef CAS.
- J. Klimeš, D. R. Bowler and A. Michaelides, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 83, 195131 CrossRef.
- Y. Zhang and W. Yang, Phys. Rev. Lett., 1998, 80, 890 CrossRef CAS.
- K. Lee, É. D. Murray, L. Kong, B. I. Lundqvist and D. C. Langreth, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 81101 CrossRef.
- K. Momma and F. Izumi, J. Appl. Crystallogr., 2011, 44, 1272–1276 CrossRef CAS.
- T. N. Tran, T. T. Luong, Q. H. Tran, V. B. T. Phung and V. A. Dinh, J. Phys.: Conf. Ser., 2023, 2485, 012005 CrossRef.
- L. T. Ta, I. Hamada, Y. Morikawa and V. A. Dinh, RSC Adv., 2021, 11, 18279–18287 RSC.
- V. B. T. Phung, T. L. Pham and V. A. Dinh, VNU J. Sci. Math – Phys., 2020, 36(1), 71–79 Search PubMed.
- V. O. Vo, T. L. Pham and V. A. Dinh, Mater. Trans., 2020, 61, 1449–1454 CrossRef CAS.
- M. Brandbyge, J.-L. Mozos, P. Ordejón, J. Taylor and K. Stokbro, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65, 165401 CrossRef.
- C. J. O. Verzijl and J. M. Thijssen, J. Phys. Chem. C, 2012, 116, 24393–24412 CrossRef CAS.
- R. Landauer, IBM J. Res. Dev., 1957, 1, 223–231 Search PubMed.
- M. Buttiker, IBM J. Res. Dev., 1988, 32, 63–75 Search PubMed.
- J. D. Correa and E. Cisternas, Solid State Commun., 2016, 241, 1–6 CrossRef CAS.
- J. Wang, F. Ma, W. Liang and M. Sun, Mater. Today Phys., 2017, 2, 6–34 CrossRef.
- Z. Liu, L. Ma, G. Shi, W. Zhou, Y. Gong, S. Lei, X. Yang, J. Zhang, J. Yu, K. P. Hackenberg, A. Babakhani, J.-C. Idrobo, R. Vajtai, J. Lou and P. M. Ajayan, Nat. Nanotechnol., 2013, 8, 119–124 CrossRef CAS PubMed.
- Y.-X. Yu, J. Mater. Chem. A, 2013, 1, 13559–13566 RSC.
- J. Wang, F. Ma and M. Sun, RSC Adv., 2017, 7, 16801–16822 RSC.
- K. Suenaga, C. Colliex, N. Demoncy, A. Loiseau, H. Pascard and F. Willaime, Science, 1997, 278, 653–655 CrossRef CAS.
- Y.-J. Yu, Y. Zhao, S. Ryu, L. E. Brus, K. S. Kim and P. Kim, Nano Lett., 2009, 9, 3430–3434 CrossRef CAS PubMed.
- B. Yu, H. Ren and X. Piao, ChemPhysChem, 2022, 23, e202100828 CrossRef CAS PubMed.
- J.-H. Li, J. Wu and Y.-X. Yu, Appl. Surf. Sci., 2021, 546, 149104 CrossRef CAS.
- Y.-X. Yu, ACS Appl. Energy Mater., 2023, 6, 10048–10060 CrossRef CAS.
- N. Kerszberg and P. Suryanarayana, RSC Adv., 2015, 5, 43810–43814 RSC.
- P. Rani and V. K. Jindal, RSC Adv., 2013, 3, 802–812 RSC.
- F. R. Bagsican, A. Winchester, S. Ghosh, X. Zhang, L. Ma, M. Wang, H. Murakami, S. Talapatra, R. Vajtai, P. M. Ajayan, J. Kono, M. Tonouchi and I. Kawayama, Sci. Rep., 2017, 7, 1774 CrossRef PubMed.
- I. K. Petrushenko and K. B. Petrushenko, Int. J. Hydrogen Energy, 2018, 43, 801–808 CrossRef CAS.
- F. A. L. de Souza, G. Sivaraman, J. Hertkorn, R. G. Amorim, M. Fyta and W. L. Scopel, J. Mater. Chem. A, 2019, 7, 8905–8911 RSC.
- M. Raupach and R. Tonner, J. Chem. Phys., 2015, 142, 194105 CrossRef PubMed.
- J. Anders, H. Wiedenhaupt, F. Kreuter, R. Tonner-Zech and B. Paulus, J. Comput. Chem., 2023, 44, 1986–1997 CrossRef CAS PubMed.
| This journal is © The Royal Society of Chemistry 2024 |