Open Access Article
Open Access ArticleGd2O3-modulated borate glass for the enhancement of near-infrared emission via energy transfer from Gd3+ to Nd3+
I. Ullah a,
C. S. Sarumahacd,
A. Angnanone,
I. Khan
a,
C. S. Sarumahacd,
A. Angnanone,
I. Khan a,
M. Shoaibb,
S. A. Khattak
a,
M. Shoaibb,
S. A. Khattak a,
S. Mukamila,
S. Kothane,
S. K. Shah
a,
S. Mukamila,
S. Kothane,
S. K. Shah a,
S. M. Wabaidurf,
G. Rooha and
J. Kaewkhao*cd
a,
S. M. Wabaidurf,
G. Rooha and
J. Kaewkhao*cd
aDepartment of Physics, Abdul Wali Khan University, Mardan, 23200, Pakistan
bSchool of Energy and Power Engineering, Jiangsu University, Zhenjiang 212013, Jiangsu, P. R. China
cCenter of Excellence in Glass Technology and Materials Science (CEGM), Nakhon Pathom Rajabhat University, Nakhon Pathom, 73000, Thailand. E-mail: jakrapong@webmail.npru.ac.th
dPhysics Program, Faculty of Science and Technology, Nakhon Pathom Rajabhat University, Nakhon Pathom, 73000, Thailand
eCenter of Radiation Research and Medical Imaging, Department of Radiologic Technology, Faculty of Associated Medical Sciences, Chiang Mai University, Chiang Mai, 50200, Thailand
fDepartment of Chemistry, College of Science, King Saud University, Riyadh 11451, Saudi Arabia
First published on 20th May 2024
Abstract
(Li2O)0.20(SrO)0.30(Nd2O3)0.01(B2O3)0.49−x(Gd2O3)x, where x = 0, 3, 5, 7, and 10 mol%, glass was melt-quenched to test it as a laser source in the near-infrared (NIR) region. The structural modification, absorption spectra, Judd–Ofelt (J–O) parameters, luminescence spectra, radiative laser parameters, lifetimes, XRD, and FTIR spectra were studied. Luminescence spectra excited at λexc = 584 nm revealed the highest intensity peak at 1073 nm due to the transition of 4F3/2 → 4I11/2. An important phenomenon of concentration quenching was observed and optimized luminescence was achieved with the sample having the concentration x = 07 mol%. The lifetimes of the donor and acceptor and energy transfer from gadolinium to neodymium were obtained from the luminescence decay kinetics. The findings show that Nd-doped Gd2O3-modified glass materials have potential as NIR laser sources.
1. Introduction
There has been an intense quest for new materials to work as near-infrared (NIR) light sources with superior optical and luminescence properties to fulfill the required demands of several photonic devices such as optical amplification, infrared laser, and optical communication.1,2 Among these optical devices, near-infrared solid-state lasers are attracting attention for their versatility.3–5 Considering the excellent spectral characteristics of RE3+, RE3+-doped inorganic materials are attracting great interest as potential lasing compounds.6,7 With this in view, Nd-added compounds are considered promising because of their significant NIR luminescence.8,9 Many glasses have demonstrated to be better host matrices than single crystals for solid-state lasers.3 Therefore, various Nd-doped inorganic oxide glasses such as silicates,10 phosphates,11 tellurites,12 and borates13 have been developed. It has been reported in different studies that the doping of Nd into various hosts can improve NIR radiative properties of the glasses. However, the improvement in such radiative properties varies across the host matrices. Therefore, the quest for a promising host with the Nd dopant for NIR emission is still ongoing. Although, borate glasses are structurally more complex compared to silicate and phosphate glasses, they possess high transparency, a higher refractive index, and greater REIs solubility.14 Specific glasses require particular properties; for instance, for broadband emission glasses, several emission centers in the host matrix are required that can be obtained through searching a matrix having different local surroundings for activators. However, because of the limited mixing in hosts, the agglomeration of Nd2O3 occurs.15 Thus, since Nd3+ possesses comparatively narrow absorption bands, most of the broadband excitation does not take place.15 A solution to this problem is to co-dope the glass with Nd3+ and another suitable sensitizer/donor that may sensitize Nd3+ via energy transfer (ET). Using this sensitization strategy, the desired optical amplification may be obtained. Previously, several glasses codoped with rare earth and their light-emitting properties have been reported. The ET phenomenon, which takes place non-radiatively, between the dopants in the Nd–Gd-codoped borate matrix was studied by C. R. Kesavulu et al.,16 where these glass systems were suggested to be efficient in NIR lasers operating at λ = 1059 nm. I. Ullah et al.17 studied the intense emission that arose because of ET from gadolinium ions to Nd3+ caused by UV excitation in borate systems. The luminescence and Judd–Ofelt (J–O) analyses, and lifetime studies have demonstrated that these glasses are promising for NIR laser devices having an appropriate energy-gain ratio and suitable lasing threshold. Acting as an efficient sensitizer/donor, gadolinium ions have exhibited intense UV excitation because of 8S7/2 → 6IJ transitions.18 Therefore, the spectroscopic and laser characteristics of neodymium in borate systems may be improved by Gd co-doping via ET from the sensitizer to the activator.Here we aimed to get insights into the enhancement of the neodymium ions photoluminescence in LSBNd_xGd glasses using Gd co-doping by calculating and analyzing the related radiative parameters required for laser applications.
2. Materials and methods
LSBNd_xGd glasses, specifically (Li2O)0.20(SrO)0.30(B2O3)0.49−x(Nd2O3)0.01(Gd2O3)x (where x = 0, 3, 5, 7, 10 mol%), were developed using LiCO3, MgCO3, H3BO3, Nd2O3, and Gd2O3 as precursor materials (procured from Sigma-Aldrich as 99.99% pure), which were weighed and homogeneously mixed in a porcelain crucible and then heated at 1100 °C for 4 h in a furnace at a rate of 10 °C min−1. The melt was quenched on a steel mold. Annealing of the as-prepared glasses was carried out at 300 °C for 3 h to remove the potential thermal stresses. The prepared glass samples were denoted as LSBNd_0Gd, LSBNd_3Gd, LSBNd_5Gd, LSBNd_7Gd, and LSBNd_10Gd, corresponding to 0, 3, 5, 7, and 10 mol% Gd3+ concentrations, as shown in Fig. 1.A densitometer (Diethelm Limited, HR-200) and Abbe refractometer (ATAGO) were used to measure the density (d) and refractive index (n) of the samples, respectively. XRD analysis was conducted using a Shimadzu XRD-6100 diffractometer with Cu Kα-radiation, 40 kV, and 30 mA power. The Agilent Cary 630 FTIR system was used to obtain the FTIR spectra. The absorption spectra were recorded by employing a Shimadzu 3600 UV-VIS-NIR spectrophotometer. The photoluminescence excitation and emission spectra and lifetime decay spectra were obtained using a Cary Eclipse fluorescence spectrophotometer (Agilent Technologies).
3. Results and discussion
3.1. XRD and FTIR features
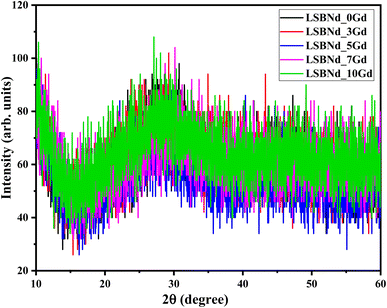
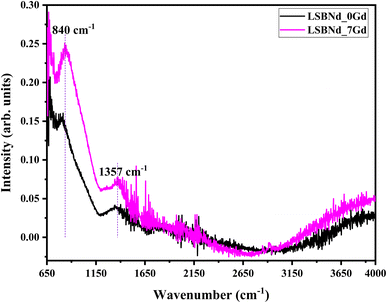
The comprehensive absence of sharp diffraction peaks in the X-ray diffraction (XRD) patterns in Fig. 2 and characteristic broad humps at lower angles, notably around 28°, with an absence of well-defined sharp peaks was indicative of a disordered and random distribution of atoms in these materials, reinforcing the conclusion that the samples exhibited an amorphous phase.19 This structural characteristic is crucial in understanding and assessing the unique properties and applications of amorphous materials in various fields, such as solid-state devices, LEDs, and lasers.Fourier transform infrared spectroscopy (FTIR) can play a crucial role in the analysis of borate glasses, providing valuable insights into their molecular structure and vibrational characteristics. In the FTIR spectra of borate glasses, three distinct frequency regions are typically observed. The bands within the ranges of 1200–1450 cm−1 and 850–1200 cm−1 are associated with B–O stretching vibrations, specifically those of trigonal BO3 units and tetrahedral BO4 borate units, respectively.20 Additionally, a peak around 700 cm−1 can be attributed to the bending vibrations of B–O linkages within the borate network. Further, in-depth analysis reveals that specific bands, such as those between 850–1168 cm−1 and 892–1195 cm−1, represent the B–O stretching vibrations of tetrahedral BO4 units. Notably, the band at approximately 1200 cm−1 is linked to the stretching vibrations of B–O bonds in (BO3)− units, primarily involving the oxygen atoms that connect different groups. These band assignments are often derived from previous reports,21 emphasizing the significance of FTIR in characterizing the structural features and bonding arrangements of borate glasses, thereby contributing to a comprehensive understanding of their properties. Here, the peak at 838 cm−1 was slightly shifted toward higher a wave number (840 cm−1) with the increase in Gd2O3 content. This suggests that the introduction of Gd2O3 induced changes in the spectra of the samples, particularly in terms of peak positioning. This shift in peak position is indicative of structural alterations within the matrix. From the current discussion it is clear that structural modification indicated by the changes in physical parameters (density and molar volume) were also reflected in the FTIR spectra, i.e., the slight redshift of the peak from 838 cm−1 to 840 cm−1 with increasing the Gd dopant from 0 to 7 mol%; whereby the vibrations modes become more stretched because of the increase in density and molar volume (Fig. 3).
3.2. Physical parameters
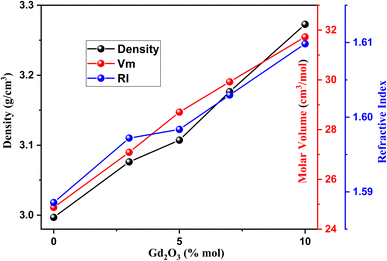
The essential parameters that determine the structural changes when the dopants are added are d, molar volume (Vm), and n.Fig. 4 displays the variation in all these parameters for the LSBNd_xGd glasses with varying the Gd2O3 concentration. Here, d was linearly elevated with the addition of Gd2O3 because of the substitution of BO3 by BO4 as well as due to the replacement of the lightweight B2O3 with a molecular weight of 69.622 g mol−1 by Gd2O3 with a heavier weight of 336.480 g mol−1, making the glass more rigid. Hence, Gd2O3 doping modified the host matrix. Also, Vm was elevated with increasing the Gd2O3 content because the Gd3+ ions have a bigger ionic size than the boron and oxygen residing in the voids caused by bond rupture between boron and oxygen, while the number of Gd3+ per unit volume also rose.22,23 Further, n was elevated with the addition of Gd2O3 as the speed of light in the materials was reduced.
3.3. Absorption spectra and Judd–Ofelt analysis
Fig. 5 displays the absorption spectrum of the LSBNd_7Gd sample showing multiple absorption peaks, characteristic of electronic transitions, positioned at 431, 462, 526, 582, 626, 682, 747, 806, and 876 nm, as per the analysis procedure reported in ref. 24 and 25.To determine the theoretical oscillator strength (ftheo) and intensity parameters, we performed Judd–Ofelt (J–O) analysis on various absorption bands of the Nd ion, in a similar fashion as reported in ref. 28 and 29. The experimental oscillator strengths fexp, ftheo, and the root mean square deviation (δrms) values were found for the LSBNd_7Gd sample listed in Table 1. The slight mismatch between ftheo and fexp validated the J–O theory. In the case of the LSBNd_7Gd sample, ftheo and fexp were the highest for the absorption peak observed at 584 nm.
| Peak positions (λp, nm) | Energy (cm−1) | LSBNd_7Gd | |
|---|---|---|---|
| fexp | ftheo | ||
| 527 | 18![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 975 975 |
1.0190 | 1.2518 |
| 584 | 17![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 123 123 |
17.3332 | 17.3361 |
| 684 | 14![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 619 619 |
1.0637 | 1.2984 |
| 784 | 12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 755 755 |
3.3917 | 3.0340 |
| 806 | 12![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 406 406 |
11.6965 | 11.7759 |
| 879 | 11![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 376 376 |
3.1979 | 3.1229 |
| δrms | 0.204 | ||
The bond covalency with ligands, asymmetry of the localized neodymium centers, rigidity, and viscosity of the host can be predicted from Ωλ,29 where Ω2 represents the covalency, while Ω4 and Ω6 demonstrate the rigidity and viscosity of the host, respectively.29 Here, the parameter Ω6 = 12.43 (×10−20 cm2) was higher than the other two, suggesting a higher viscosity and lower covalency of the bond between Nd and O in the present glass samples. These parameters were calculated from the mixed electric and magnetic dipole absorption transitions. The parameters Ω4 and Ω6, unlike Ω2, are rarely modified with the covalency;30 therefore, the comparatively small covalency of the glass sample showed a tendency of high viscosity. Table 2 shows that the present sample also followed the same trend of J–O parameters as for previously reported borate glasses.
3.4. Luminescence and radiative features of LSBNd_7Gd samples
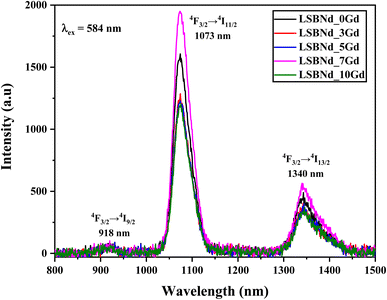
Fig. 7 displays the luminescence spectra of the LSBNd_Gd glass, excited at λ = 584 nm. There were three emission bands present at 918, 1073, and 1340 nm, regardless of the Nd content. The band at 1073 nm was the most intense and exhibited hypersensitive electric dipole characteristics, as validated by the selection rules allowing the transitions ΔS = 0, ΔS ≤ 2, and ΔJ ≤ 2, while the band at 918 nm had the lowest intensity, indicating less sensitive magnetic dipole properties, as validated by the selection rules disallowing the transition ΔJ ≤ 6. The second strongest transition was the broad 4F3/2 → 4I13/2 transition caused by the magnetic dipole effect. This transition is highly important in optical solids, as it is used in 1.057 μm lasers and is suitable for amplification in optical telecommunications within the E-band and O-band35 Due to concentration quenching, the emission of Gd2O3 started to decrease when the concentration exceeded 7 mol%. This decrease was caused by the interaction between host defects and Nd3+ ions, leading to concentration quenching and cross-relaxations. As a result, a non-radiative transition occurred.36 The process of non-radiative energy cross-relaxation and resonance energy transfer significantly impacts the spectral emission profile of Nd3+ ions. There are multiple states with small energy differences between the 4F3/2 and 4IJ levels, facilitating efficient non-radiative transfer and intense quenching. Resonant energy transfer and non-radiative energy transfer can both contribute to radiative transition and relaxation.37 The transition from 2F3/2 to 4I11/2, which occurs at a high intensity at 1073 nm, makes these samples promising for laser applications.The spectral parameters, such as total radiative transition probability (AT), gain bandwidth, optical gain, and branching ratios (βR), were determined using the procedure mentioned in ref. 25, 28 and 29 and are given in Table 3. As found from the ratio of the area of a luminescence band to the total integrated area under the bands, the measured branching ratios (βmeas), an essential parameter, are listed in Table 3. Table 3 shows that the branching ratio (βR) values obtained from 4F3/2 → 4I11/2 transition were comparable to those reported in the literature. Table 3 also indicates that the optical gain (σemi × τR), determining the threshold of laser, had a greater value for 4F3/2 → 4I11/2 transition at 1073 nm for the LSBNd_7Gd sample. In terms of the range of frequencies where the optical amplification occurs, the gain bandwidth (σemi × Δλeff) for the LSBNd_7Gd was comparable with those for glasses reported in previous studies, and is needed for an NIR laser at nearly 1073 nm, a distinct wavelength for laser applications.38 These values for 4F3/2 → 4I11/2 transition in the studied glasses are comparable [Table 3].
| Titled glass | Δλeff, nm | σemi (10−20 cm2) | σemi × Δλeff (×10−27 cm3) | AT (s−1) | σemi × τR (×10−25 cm2 S) | βR | βmeas | References |
|---|---|---|---|---|---|---|---|---|
| ZnAlBiB | 30 | 3.67 | 1.1 | 2136 | — | 72 | 50 | 39 |
| BiZNd | 37 | 3.36 | 1.24 | 5920 | 27.9 | 48 | 62 | 40 |
| BNaNf | 18 | 4.18 | 0.73 | — | 63.11 | 38 | 41 | 41 |
| BSGdCaNd0.5 | 32.87 | 1.39 | 45.73 | 750 | — | 45 | 58 | 16 |
| NaF–Na2O–B2O3 | 57.2 | 5.0 | 28.60 | — | — | 43 | — | 42 |
| LSBNd_7Gd | 28 | 5.56 | 1.56 | 5858 | 19.71 | 56 | 58 | Present sample |
For the 4F3/2 → 4I11/2 transition of the studied glasses, the lasing parameters are better than those of other studied systems, as is evident form Table 3. LSBNd_7Gd is thus considered a beneficial sample for realizing broadband optical amplification at 1073 nm.
3.5. Lifetime profiles evaluation
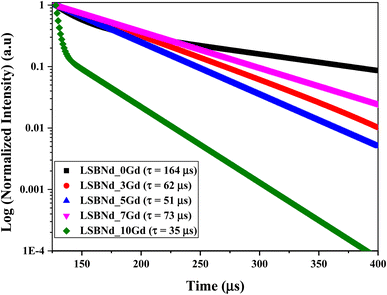
Fig. 8 shows the lifetime profiles at 584 nm excitation and 1073 nm emission for all the samples. The lifetime measured (τexp) and fitted monoexponentially dropped from 164 μs to 35 μs with elevating the Gd2O3 content (Table 4). This shows that the reduction in τexp was caused by non-radiative relaxation and concentration quenching. From the decay profile, it could be noted that the LSBNd_7Gd glass had a longer experimental lifetime than the other samples. This was because the highest luminescent intensity reduces slowly due to the slow energy transfer to the luminescent center. At this particular concentration, i.e., 7 mol% Gd3+, as a glass structure modifier and sensitizer, the addition prevents the activator's (Nd3+) clustering and, hence, its quick decay.43 A similar trend has also been observed in the literature.44The mismatch between the experimental and radiative lifetimes, as shown in Table 4, was due to the energy transfer occurring non-radiatively (NR), which increased with elevating the Gd3+ content.45
The total luminescence quantum efficiency (η) is given by eqn (1):
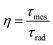 | (1) |
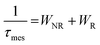 | (2) |
Table 4 depicts that for the LSBNd_7Gd sample, the non-radiative decay probability was the smallest with an efficient luminescence quantum efficiency. This was due to the ET via cross-relaxations, concentration quenching, multi-phonon relaxation, and ET to the luminescence quenching centers.
To study the nature of the interaction mechanism of the Gd fluorescence time, the lifetime curves for 5, 7, and 10 mol% were fitted by the Inokuti–Hirayama (IH) model using eqn (3). A best fit was confirmed for the S = 6 dipole–dipole interaction at high Gd concentrations; however, large deviations were shown at low contents.
The ET parameter (Q) of Nd3+ is given by eqn (3):
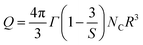 | (3) |
The enhancement in the ET parameter value, i.e., Q, with the elevating dopant concentration, as shown in Table 5, reinforced the dipole–dipole interaction phenomenon among the dopant ions.
Eqn (4) gives the relationship between the interaction parameters CDA and R of Nd3+.
| CDA = Rsτ−1 | (4) |
As shown in Table 5, the CDA increased with increasing the dopant content.
Table 5 shows the parameters Q, R, and CDA as functions of the dopant's concentration, which are more promising than those studied for other borate systems.
3.6. Energy transfer features
Radiative or non-radiative mechanisms are responsible for ET from a donor to an acceptor. Inorganic systems usually have a small absorption strength for the donor, where radiative transfer mostly does not take place,49 while its occurrence is further lessened due to the low-absorption cross-section of neodymium ions. Two resonance mechanisms cause strong non-radiative energy transfer: the Dexter mechanism (electron exchange) and the Förster mechanism (coulombic interaction).50,51 The former is prominent when the donor–acceptor separation ranges from 1–10 nm, while at a distance between the donor and acceptor of less than 1 nm, the latter becomes significant.52 The molar concentration of the dopants in the matrix and density can be utilized by using eqn (5) to estimate the lower limit of their separation:
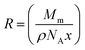 | (5) |
The calculated R (2.46, 2.12, 1.92, and 1.74 nm) decreased with the addition of the dopant (Gd2O3) at 3, 5, 7, and 10 mol%, respectively, confirming that the dominant ET mechanism from Gd to Nd was coulombic interaction in all the samples. The estimated inter Gd and Nd separations could be higher in the presence of Nd agglomerations in the glass; however, the dominancy of the coulombic interaction mechanism would still preserve. The Nd clustering can be minimized by optimizing the dopant concentration and glass-melting conditions.
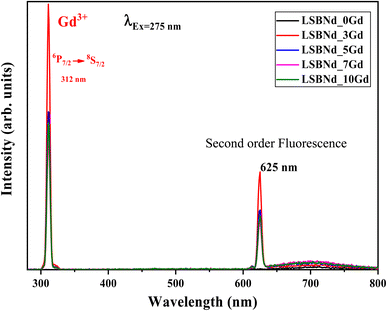
Fig. 9 illustrates PL spectra of the LSBNd_xGd sample at λexc = 275 nm, where a gradual decrease could be noticed in the luminescence intensities (312 nm) of the emission spectra, due to the ET from Gd2O3 to Nd2O3. The peak observed at 625 nm corresponded to the second-order fluorescence in the monochromator, as detailed in ref. 53.
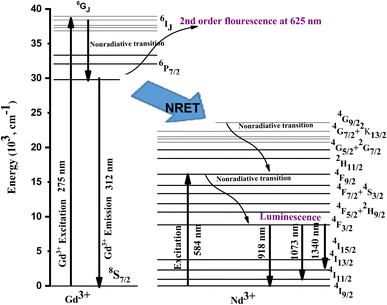
The ET, shown in the energy-level diagram of Fig. 10, takes place by the overlap of the donor emission and acceptor absorption cross-sections.54 The Gd was raised from the 8S7/2 to 6IJ level upon getting the energy. The Gd de-excites radiatively, giving emission at 312 nm while partly non-radiatively transferring energy to the 4F9/2 state of Nd. The ET occurs from Gd3+ to Nd3+ through the 6P7/2 → 8S7/2 transition of Gd. The Nd ions are excited to the 4F9/2 state and thereafter relax by making transitions to the ground state (4I9/2), giving typical emissions at 918, 1073, and 1340 nm.
The probability of releasing phonons for the Gd3+ (6Pj) → Nd3+ (4F9/2) process is much higher than that in capturing phonons for the Nd3+ (4F9/2) → Gd3+(6Pj) process, because the 4F3/2 state (activator) is lower than the 6Pj states (sensitizer), thus, ET population of the 4F9/2 state is enriched. The enhancement of the population due to the addition of Gd3+ leads to the sensitization of Nd3+ luminescence and results in triggering the excitation of the Nd3+ emission.
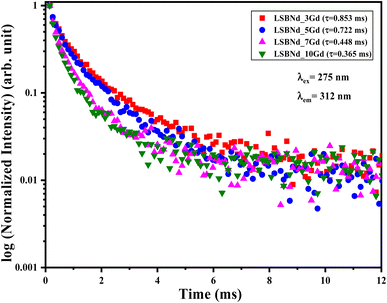
The ET from Gd to Nd was also analyzed from the lifetime of the LSBNd_xGd at λexc = 275 nm and λemi = 312 nm, which was reinforced by a single exponential decay, as illustrated in Fig. 11 and Table 6.
| Glass composition | τGd (ms) | τNd (ms) | ηGd–Nd |
|---|---|---|---|
| LSBNd_0Gd | — | 0.164 | — |
| LSBNd_3Gd | 0.853 | 0.062 | 0.072 |
| LSBNd_5Gd | 0.722 | 0.051 | 0.070 |
| LSBNd_7Gd | 0.448 | 0.073 | 0.162 |
| LSBNd_10Gd | 0.365 | 0.035 | 0.095 |
The ητr listed in Table 6 from the lifetimes of the gadolinium donor and Nd3+ acceptor were determined by eqn (6):17
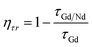 | (6) |
An increase in ET efficiency was expected with the elevating content of Gd2O3 from 3% to 10% as the transfer rate increases with the decrease in Gd3+–Nd3+ separation. The proximity of the Nd3+ acceptor meant the sample contained a significant number of Gd donors, thus increasing the ητr. The ET efficiency increased gradually with the addition of Gd2O3 content. An acceptable explanation for this is the non-radiative luminescence quenching of the Gd via ET to the Nd.
4. Conclusion
By using the typical melt-quenching route, LSBNd_xGd glasses doped with various Gd3+ ions contents were developed. The host matrix modification by doping was confirmed from analysis of the physical parameters as well as the XRD and FT-IR spectra. As determined from the absorption spectra, the J–O parameters (Ω2 = 4.56, Ω4 = 4.64, Ω6 = 5.43) were used for calculation of the radiative and laser parameters of Nd3+. Among the three bands in the emission spectra at λexc = 582 nm, the 2F3/2 → 4I11/2 (λem = 1073 nm) transition was the most intense for all the glass samples and caused the distinctive NIR emission. The determined high stimulated emission cross-sections (5.56 × 10−20 cm2), branching ratios (56%), and quantum efficiencies (42%) for the 2F3/2 → 4I11/2 transition make the LSBNd_xGd glasses favorable for NIR lasers. The lifetimes of Gd (6P7/2 state) and Nd (2F3/2) decreased (from 0.853 to 0.365 ms and 0.164 to 0.035 ms, respectively) with the increase in the dopant ion concentration from 0 to 10 mol%. Gd3+ and Nd3+ containing optical glasses exhibited prominent NIR (λem = 1073 nm) and weak 312 nm emissions, proving the Gd3+ → Nd3+ ET. Moreover, while analyzing the ET to the luminescent center, the phonon-assisted non-radiative dipole–dipole interaction was verified as an effective mechanism. Based on the obtained results, the LSBNd_xGd glass with 07 mol% Gd2O3 content was found to be optimum for the development of NIR lasers.Author contributions
I. Ullah: formal analysis, investigation, methodology, writing – original draft, writing – review & editing. C. Sumarah and A. Angnanon: sample preparation and characterization, data curation, methodology, S. A. Khattak: writing – review & editing. S. K. Shah supervision, G. Rooh: investigation, methodology, project administration, software, supervision. I. Khan, M. Shoaib and S. Mukamil: investigation, methodology. S. M. Wabaidur: resources, J. Kaewkhao and S. Kothan: conceptualization, project administration, funding acquisition, resources, software, supervision, validation.Conflicts of interest
There are no conflicts to declare.Acknowledgements
The authors wish to acknowledge the Centre of Excellence in Glass Technology and Material Science, Nakhon Pathom Rajabhat University, for providing experimental facilities. Acknowledgment is also made to Thailand Science Research and Innovation (TSRI) for partially supporting this research. This research was partially supported by Chiang Mai University. CMU Proactive Researcher. Chiang Mai University [Grant number 805/2566]. This research has received funding support from the NRSF via the Program Management Unit for Human Resources and Institutional Development, Research and Innovation [grant number B41G670028].References
- J.-C. G. Bünzli, S. Comby, A.-S. Chauvin and C. D. Vandevyver, New opportunities for lanthanide luminescence, J. Rare Earths, 2007, 25(3), 257–274 CrossRef.
- J.-C. G. Bünzli and S. V. Eliseeva, Lanthanide NIR luminescence for telecommunications, bioanalyses and solar energy conversion, J. Rare Earths, 2010, 28(6), 824–842 CrossRef.
- G. Neelima, K. V. Krishnaiah, N. Ravi, K. Suresh, K. Tyagarajan and T. J. Prasad, Investigation of optical and spectroscopic properties of neodymium doped oxyfluoro-titania-phosphate glasses for laser applications, Scr. Mater., 2019, 162, 246–250 CrossRef CAS.
- M. Deepa, R. Doddoji, C. D. Viswanath and A. Chandrasekhar, Optical and NIR luminescence spectral studies: Nd3+-doped borosilicate glasses, J. Lumin., 2019, 213, 191–196 CrossRef CAS.
- L. Yao, Q. Shao, X. Xu, Y. Dong, C. Liang and J. He, et al., Broadband emission of single-phase Ca3Sc2Si3O12: Cr3+/Ln3+ (Ln= Nd, Yb, Ce) phosphors for novel solid-state light sources with visible to near-infrared light output, Ceram. Int., 2019, 45(11), 14249–14255 CrossRef CAS.
- H. Lin, D. Yang, G. Liu, T. Ma, B. Zhai and Q. An, et al., Optical absorption and photoluminescence in Sm3+-and Eu3+-doped rare-earth borate glasses, J. Lumin., 2005, 113(1–2), 121–128 CrossRef CAS.
- K. Bodišová, R. Klement, D. Galusek, V. Pouchlý, D. Drdlík and K. Maca, Luminescent rare-earth-doped transparent alumina ceramics, J. Eur. Ceram. Soc., 2016, 36(12), 2975–2980 CrossRef.
- G. Vázquez, I. Camarillo, C. Falcony, U. Caldiño and A. Lira, Spectroscopic analysis of a novel Nd3+-activated barium borate glass for broadband laser amplification, Opt. Mater., 2015, 46, 97–103 CrossRef.
- N. Deopa, A. Rao, M. Gupta and G. V. Prakash, Spectroscopic investigations of Nd3+ doped lithium lead alumino borate glasses for 1.06 μm laser applications, Opt. Mater., 2018, 75, 127–134 CrossRef CAS.
- P. Manasa, D. Ramachari, J. Kaewkhao, P. Meejitpaisan, E. Kaewnuam and A. Joshi, et al., Studies of radiative and mechanical properties of Nd3+-doped lead fluorosilicate glasses for broadband amplification in a chirped pulse amplification based high power laser system, J. Lumin., 2017, 188, 558–566 CrossRef CAS.
- R. Balakrishnaiah, P. Babu, C. Jayasankar, A. Joshi, A. Speghini and M. Bettinelli, Optical and luminescence properties of Nd3+ ions in K–Ba–Al-phosphate and fluorophosphate glasses, J. Phys.: Condens. Matter, 2005, 18(1), 165 CrossRef.
- E. A. Lalla, U. Rodríguez-Mendoza, A. Lozano-Gorrín, A. Sanz-Arranz, F. Rull and V. Lavín, Nd3+-doped TeO2–PbF2–AlF3 glasses for laser applications, Opt. Mater., 2016, 51, 35–41 CrossRef CAS.
- B. Karthikeyan, S. Mohan and M. Baesso, Spectroscopic and glass transition studies on Nd3+-doped sodium zincborate glasses, Phys. B, 2003, 337(1–4), 249–254 CrossRef CAS.
- A. Bajaj, A. Khanna, B. Chen, J. G. Longstaffe, U.-W. Zwanziger and J. Zwanziger, et al., Structural investigation of bismuth borate glasses and crystalline phases, J. Non-Cryst. Solids, 2009, 355(1), 45–53 CrossRef CAS.
- D. R. Rao, G. S. Baskaran, Y. Gandhi and N. Veeraiah, Influence of sesquioxides on luminescence features of Nd3+ ions in PbO–PbF2–B2O3 glass system, Phys. B, 2015, 457, 117–125 CrossRef.
- C. Kesavulu, H. Kim, S. Lee, J. Kaewkhao, N. Wantana and E. Kaewnuam, et al., Spectroscopic investigations of Nd3+ doped gadolinium calcium silica borate glasses for the NIR emission at 1059 nm, J. Alloys Compd., 2017, 695, 590–598 CrossRef CAS.
- I. Ullah, G. Rooh, S. Khattak, I. Khan, M. Shoaib and S. Kothan, et al., Spectral characteristics and energy transfer in Gd3+ and Nd3+ doped borate glasses for NIR laser applications, Infrared Phys. Technol., 2022, 125, 104272 CrossRef CAS.
- D. Ramteke and R. Gedam, Luminescence properties of Gd3+ containing glasses for ultra-violet (UV) light, J. Rare Earths, 2014, 32(5), 389–393 CrossRef CAS.
- K. Nanda, N. Berwal, R. Kundu, R. Punia and N. Kishore, Effect of doping of Nd3+ ions in BaO–TeO2–B2O3 glasses: A vibrational and optical study, J. Mol. Struct., 2015, 1088, 147–154 CrossRef CAS.
- P. Pascuta, R. Lungu and I. Ardelean, FTIR and Raman spectroscopic investigation of some strontium–borate glasses doped with iron ions, J. Mater. Sci.: Mater. Electron., 2010, 21, 548–553 CrossRef CAS.
- N. Bobkova and S. Khot’ko, Structure of zinc-borate low-melting glasses derived from IR spectroscopy data, J. Appl. Spectrosc., 2005, 72, 853–857 CrossRef CAS.
- I. Ullah, C. Sarumaha, A. Angnanon, I. Khan, M. Shoaib and S. Kothan, et al., Effect of Gd2O3 concentration on photo and X-rays excited luminescence characteristics of Dy3+-Activated Li2O3–SrO–B2O3 glass, Opt. Mater., 2022, 133, 113044 CrossRef CAS.
- I. Ullah, C. Sarumaha, A. Angnanon, I. Khan, M. Shoaib and S. Khattak, et al., Spectral features of Gd2O3 modified Sm3+ doped lithium strontium borate glasses for solid-state laser applications, Ceram. Int., 2022, 49(9), 13774–13782 CrossRef.
- C. K. Jørgensen and B. Judd, Hypersensitive pseudoquadrupole transitions in lanthanides, Mol. Phys., 1964, 8(3), 281–290 CrossRef.
- W. T. Carnall, The absorption and fluorescence spectra of rare earth ions in solution, Handbook on the Physics and Chemistry of Rare Earths, 1979, vol. 3, pp. 171–208 Search PubMed.
- J. Tauc, Optical properties of non-crystalline solids, Opt. Prop. Solids, 1972, 277–313 CAS.
- N. F. Mott and E. A. Davis, Electronic Processes in Non-crystalline Materials, Oxford university press, 2012 Search PubMed.
- B. R. Judd, Optical absorption intensities of rare-earth ions, Phys. Rev., 1962, 127(3), 750 CrossRef CAS.
- G. Ofelt, Intensities of crystal spectra of rare-earth ions, J. Chem. Phys., 1962, 37(3), 511–520 CrossRef CAS.
- I. I. Kindrat, B. V. Padlyak, R. Lisiecki and V. T. Adamiv, Spectroscopic and luminescent properties of the lithium tetraborate glass co-doped with Nd and Ag, J. Alloys Compd., 2021, 853, 157321 CrossRef CAS.
- K. V. Kumar and A. S. Kumar, Spectroscopic properties of Nd3+ doped borate glasses, Opt. Mater., 2012, 35(1), 12–17 CrossRef.
- B. Shanmugavelu, V. Venkatramu and V. R. K. Kumar, Optical properties of Nd3+ doped bismuth zinc borate glasses, Spectrochim. Acta, Part A, 2014, 122, 422–427 CrossRef CAS PubMed.
- Y. Chen, Y. Huang, M. Huang, R. Chen and Z. Luo, Effect of Nd3+ on the spectroscopic properties of bismuth borate glasses, J. Am. Ceram. Soc., 2005, 88(1), 19–23 CrossRef CAS.
- S. Mohan and K. S. Thind, Investigation of luminescence and spectroscopic properties of Nd3+ ions in cadmium alkali borate glasses, Opt. Mater., 2016, 57, 134–139 CrossRef CAS.
- E. Kaewnuam, N. Wantana, N. X. Tu, P. H. Minh, K. Yamanoi and J. Kaewkhao, The study on BWGd: Nd glass for new laser amplifier: Properties, theoretical and practical investigations, Opt. Mater., 2022, 129, 112535 CrossRef CAS.
- F. Zaman, N. Srisittipokakun, G. Rooh, S. Khattak, N. Singkiburin and H. Kim, et al., Investigation of Li2O–Gd2O3–MO–B2O3–Nd2O3 (MO= Ba/Bi) glasses for laser applications by Judd–Oflet (J–O) theory, J. Lumin., 2019, 215, 116639 CrossRef CAS.
- al. RSNe, Spectrochim. Acta, Part A, 2017, 193–197, 180 Search PubMed.
- J. Rajagukguk, R. Situmorang, M. Djamal, R. Rajaramakrishna, J. Kaewkhao and P. H. Minh, Structural, spectroscopic and optical gain of Nd3+ doped fluorophosphate glasses for solid state laser application, J. Lumin., 2019, 216, 116738 CrossRef CAS.
- S. Mahamuda, K. Swapna, A. S. Rao, M. Jayasimhadri, T. Sasikala and K. Pavani, et al., Spectroscopic properties and luminescence behavior of Nd3+ doped zinc alumino bismuth borate glasses, J. Phys. Chem. Solids, 2013, 74(9), 1308–1315 CrossRef CAS.
- G. Gupta, A. D. Sontakke, P. Karmakar, K. Biswas, S. Balaji and R. Saha, et al., Influence of bismuth on structural, elastic and spectroscopic properties of Nd3+ doped Zinc–Boro-Bismuthate glasses, J. Lumin., 2014, 149, 163–169 CrossRef CAS.
- R. Karunakaran, K. Marimuthu, S. Arumugam, S. S. Babu, S. Leon-Luis and C. Jayasankar, Structural, optical absorption and luminescence properties of Nd3+ ions in NaO-NaF borate glasses, Opt. Mater., 2010, 32(9), 1035–1041 CrossRef CAS.
- B. Karthikeyan and S. Mohan, Spectroscopic and glass transition investigations on Nd3+-doped NaF–Na2O–B2O3 glasses, Mater. Res. Bull., 2004, 39(10), 1507–1515 CrossRef CAS.
- T. Suzuki, H. Nasu, M. Hughes, S. Mizuno, K. Hasegawa and H. Ito, et al., Quantum efficiency measurements on Nd-doped glasses for solar pumped lasers, J. Non-Cryst. Solids, 2010, 356(44–49), 2344–2349 CrossRef CAS.
- B. He, K. Yang, L. Chen, Y. Hua, F. Huang and J. Zhang, et al., Effect of Gd2O3 on luminescence properties of RE ions in germanium-tellurite glasses, J. Lumin., 2020, 220, 116977 CrossRef CAS.
- I. Kindrat, B. Padlyak and R. Lisiecki, Judd–Ofelt analysis and radiative properties of the Sm3+ centres in Li2B4O7, CaB4O7, and LiCaBO3 glasses, Opt. Mater., 2015, 49, 241–248 CrossRef CAS.
- R. Rajaramakrishna, Y. Ruangtawee and J. Kaewkhao, Sm3+-doped molybdenum gadolinium borate glasses for orange emission laser active medium, Ukr. J. Phys., 2018, 63(8), 720–731 Search PubMed.
- S. S. Babu, C. Jayasankar, P. Babu, T. Tröster, W. Sievers and G. Wortmann, Luminescence and energy transfer properties of Sm3+: borate glasses, Eur. J. Glasses Sci. Technol. B Phys. Chem. Glasses, 2006, 47(4), 548–552 CAS.
- G. Lakshminarayana, A. Meza-Rocha, O. Soriano-Romero, E. Huerta, U. Caldino and A. Lira, et al., Analysis of fluorescence characteristics of Sm3+-doped B2O3-rich glasses for Orange-light-emitting diodes, J. Alloys Compd., 2021, 884, 161076 CrossRef CAS.
- M. Hughes, H. Li, R. Curry, T. Suzuki and Y. Ohishi, Energy transfer in Cr and Nd co-doped Si-B-Na-Al-Ca-Zr-O glasses, J. Non-Cryst. Solids, 2020, 530, 119769 CrossRef CAS.
- D. L. Dexter, A theory of sensitized luminescence in solids, J. Chem. Phys., 1953,(21), 836–850 CrossRef CAS.
- T. Förster, Mechanisms of energy transfer, Comprehensive biochemistry, 1967, pp. 61–80 Search PubMed.
- N. Hildebrandt and I. L. Medintz, FRET-forster Resonance Energy Transfer: from Theory to Applications, Wiley-VCH Verlag GmbH, 2014 Search PubMed.
- R. A. Buchanan and C. Bueno, Terbium Activated Silicate Luminescent Glasses, US Pat., US5122671A, 1992 Search PubMed.
- Y. Zheng, B. Chen, H. Zhong, J. Sun, L. Cheng and X. Li, et al., Optical transition, excitation state absorption, and energy transfer study of Er3+, Nd3+ single-doped, and Er3+/Nd3+ codoped tellurite glasses for mid-infrared laser applications, J. Am. Ceram. Soc., 2011, 94(6), 1766–1772 CrossRef CAS.
| This journal is © The Royal Society of Chemistry 2024 |