Magnetism and electrical and thermal transport in the natural Fe_1−xMn_xWO₄ (x = 0.2) mineral from Potosí, Bolivia

Abstract

The composition of a natural single crystalline specimen from the province of Potosí in Bolivia is found to be Fe0.8Mn0.2WO4. It crystallizes with the primitive monoclinic NiWO4 structure type [space group P2/c, a = 4.74751(6) Å, b = 5.71335(7) Å, c = 4.96847(5) Å, β = 90.15(1)°]. Magnetic susceptibility and specific heat capacity measurements indicated that the mineral undergoes multiple magnetic transitions: TN1 ≈ T\,cpN1 = 67(1) K, TN2 = 28(3) K, and T\,cpN2 = 8(1) K. The reduced magnetic entropy of ≈R ln 3 upon the high-temperature antiferromagnetic ordering suggests the failure of the simplified LS-coupling scheme in the description of the magnetism. Fe0.8Mn0.2WO4 is characterized by enlarged electrical resistivity showing an exponential decrease with temperature for T > 300 K, from which an energy gap of 310 meV is deduced. The well-pronounced maximum occurring in the phononic thermal conductivity just below the TN1 is described by the Debye–Callaway model, indicating the dominance of phonon scattering on defects as well as umklapp processes.

---

1 Introduction

Mixed Fe_1−xMn_xWO₄ tungstates play an important role in the development of supercapacitors and photoluminescent-, photocatalytic- and electrode materials for lithium-ion batteries.^1–8 As a result, investigations into their magnetic, electrochemical and photoluminescence properties have become a prominent area of scientific inquiry. Nevertheless, despite the increased interest in this class of materials, several crystallochemical, magnetic, thermodynamic points remain less clear.

Given that both the ternary FeWO₄ (ferberite) and MnWO₄ (huebnerite) as well as quaternary Fe_1−xMn_xWO₄ (wolframite) tungstates occur as natural minerals, their crystal structures have been the subject of extensive investigation since the nineteenth century.⁹ They were shown to crystallize with a monoclinic (space group P2/c) wolframite (NiWO₄) structure type.^9,10 Such an arrangement foresees d-elements to reside within the condensed distorted [O₆] octahedra, a hypothesis that is both crystallographically and chemically plausible. Nevertheless, the existence of a small monoclinic distortion (angle β ≈ 90°) has prompted a number of reinvestigations.^1,4,9 One of the most recent ones confirming the questionable β found that the FeWO₄ structure is indeed characterized by partial occupancy of the normally empty [O₄]-tetrahedral voids.⁴ Obviously, the nature of the chemical disorder in quaternary Fe_1−xMn_xWO₄ remains unknown due to the neighboring positions of the d-element constituents in the periodic table. Importantly, no phase transition for the natural FeWO₄ is observed up to 20 GPa.¹

Magnetic measurements indicated that FeWO₄ orders antiferromagnetically at T_N = 75 K and reveals anisotropic properties due to the magneto-crystalline anisotropy of the Fe²⁺ ions. Such a behavior is explained by the super-exchange interactions occurring via O²⁻-ions along distinct paths between neighboring Fe²⁺ ions.¹¹ The change of the magnetic entropy ΔS_mag = R ln 5 (gas constant R = 8.314 J mol⁻¹ K⁻¹) for FeWO₄ upon the transition was deduced from the specific heat measurements and it is again in line with the 2+ oxidation state of iron ions (i.e., it corresponds to the total spin angular momentum S = 2).⁹ On the other hand, MnWO₄ is characterized by a much more complex magnetic structure, including three transitions at T_N of 13.5 K, 12.3 K and 8 K, respectively. The low temperature antiferromagnetic phases were shown to possess incommensurately modulated structures.^12,13 Interestingly, both quaternary Fe_1−xMn_xWO₄ ferberite and hubnerite always reveal magnetic behavior reminiscent of those of MnWO₄. Some attempts to construct magnetic phase diagrams for them are made in ref. 11 and 14.

The reported electrical resistivities [ρ(T)] for both FeWO₄ and MnWO₄ are large, varying in the range of Ω m–kΩ m, which are characterized by an exponential decrease with temperature. The increased conductivity in FeWO₄ at RT is believed to be related to the presence of a small admixture of Fe³⁺ ions, which triggers electron hopping along and between the chains in the [001]-direction.¹⁵ On the other hand, higher ρ(T) in MnWO₄ is explained by small and large polaron hopping for LT- and HT-regimes, respectively.¹⁶ Now, in mixed Fe_1−xMn_xWO₄ tungstates, characterized by the presence of Mn²⁺ ions, it is believed that the latter do not block the electron hopping paths and thus, the interchain electron transfer is possible.¹⁵

The Seebeck coefficients [α(T)] of FeWO₄ ^4,7,15,17 and MnWO₄ ¹⁶ are large and positive in the whole studied temperature range, assuming the dominance of hole-like conduction mechanisms. The decrease in the values of α(T) (i.e., they vary between 140 μV K⁻¹ and 350 μV K⁻¹ at RT depending on the synthesis route) in FeWO₄ is explained by the increase of Fe³⁺-impurity ion concentration.⁴

The energy band gaps of ≈2 eV (ref. 8 and 18–20) and ≈2.6 eV (ref. 7, 18 and 20) for FeWO₄ and MnWO₄, respectively, were estimated by spectroscopic measurements and further confirmed by the simulations within density functional theory. The high-pressure studies revealed that the energy gap of FeWO₄ can be suppressed to 1.56 eV at 16 GPa (ref. 1) and to 2.08 eV at 10 GPa for MnWO₄.²¹

Magnetic studies have been conducted on quaternary natural Fe_1−xMn_xWO₄ (x ≈ 0.2) minerals sourced from Portugal and Mexico.²² However, a comprehensive analysis of their electrical and thermal transport properties remains unavailable. In this work, we provide a combined investigation of the structural and physical properties of the Fe_0.8Mn_0.2WO₄ single-crystalline specimen sourced from the Potosí province in Bolivia.

2 Experimental

The studied specimen is a natural single crystal of the Fe_1−xMn_xWO₄ composition belonging to the group of wolframite minerals and originating from the Potosí province in Bolivia, which is known for the excellent quality of such minerals.²³

The specimen was characterized by powder X-ray diffraction performed using a Huber G670 Guinier camera (CuK_α1 radiation, λ = 1.54056 Å). Phase analysis was performed using the WinXpow software package²⁴ and Rietveld refinement was performed using WinCSD.²⁵ Single-crystal diffraction [SCXRD, a STOE STADIVARI diffractometer (MoKα radiation, λ = 0.71073 Å) equipped with a Dectris Pilatus300K detector] was performed on a crystal mechanically extracted from the crushed sample.

The supplementary crystallographic data for Fe_0.8Mn_0.2WO₄ are deposited under CCDC 2425342.†

The surface of the polished wolframite embedded in a conductive resin was investigated using a scanning electron microscope (SEM) (Jeol JSM − 7800F) equipped with an energy dispersive X-ray spectrometer (EDXS) Quantax 400 EDXS system from Bruker. Such an analysis indicated the (Fe,Mn) : W relationship in the main phase to be 1 : 1 with an oxygen content of ≈70 at%. Recalculating further the Fe and Mn contents, we deduced the Fe_0.80(3)Mn_0.20(3)W_1.00(3)O₄ chemical composition for the studied wolframite. Besides the main phase, the investigated specimen contained an admixture of SnO₂ and CuFeS₂ (Fig. 1), which was not visible in the measured PXRD patterns.

Fig. 1 Backscattered electron image of Fe_0.8Mn_0.2WO₄ with the identification of phases.

Temperature (1.8–300 K) and magnetic field (0–7 T) dependencies of magnetic susceptibility, specific heat capacity and thermal conductivity were measured by the corresponding options of DynaCool-12 from Quantum Design. High temperature (450–600 K) electrical resistivity and Seebeck coefficients were obtained using an ULVAC ZEM-3 device. Due to the highly insulating nature of the sample for T < 450 K, electrical resistivity was additionally measured using an AC method at 100 kHz with an Agilent Precision LCR meter, Model E4980A.

3 Results and discussion

3.1 Crystal structure

All reflections collected for Fe_0.8Mn_0.2WO₄ from the single crystal XRD experiment were indexed within the monoclinic lattice with the unit cell parameters a = 4.7466(9) Å, b = 5.711(1) Å, c = 4.969(1) Å and β = 90.21(3)°. The analysis of the extinction conditions indicated l = 2n for h0l and 00l indices and thus, two possible space groups (SG) P2/c or Pc. In the first step we have chosen the centrosymmetric one to find a structural model. By applying direct methods four atomic positions were located as shown in Table 1. The preliminary refinement cycle converged with a low reliability factor R_F = 0.033, indicating the correctness of the obtained model. Performing further extinction correction and refining anisotropic displacement parameters (ADP), R_F was reduced to 0.014. Crystallographic details of this refinement together with the final values of atomic coordinates and equivalent displacement parameters are collected in Table 1. The obtained ADPs are shown in Table 2. Since Fe and Mn are neighboring elements in the periodic table, reasonable refinement of their statistical mixture is rather impossible. Therefore, the composition of the crystal was fixed to the results from EDX analysis.

Table 1 Crystallographic data for Fe_0.8Mn_0.2WO₄ (SG P2/c)

Method	Single crystal	Powder
a Obtained from PXRD and used for single crystal refinement.b M is a statistical mixture of (0.8Fe + 0.2Mn) in accordance with EDX analysis.
Unit cell parameters^a
a (Å)	4.74751(6)
b (Å)	5.71335(7)
c (Å)	4.96847(5)
β (°)	90.15(1)
V (Å^3)	134.76(2)
Calculated density ρ (g cm^−3)	7.48(1)
Radiation, λ (Å)	MoKα, 0.71073	CuKα1, 1.54056
Absorption coeff. (mm^−1)	50.40	122.1
Scan step (°); N (images)	1° (ω-scans); 1414	0.005
Maximal 2Θ (°)	76.47	100.41
Minimal h, k, l	−6, −9, −8	0, 0, −4
Maximal h, k, l	8, 9, 8	4, 5, 5
Crystal size, μm^3	20 × 40 × 96
Absorption correction	Multiscan
Tmax/Tmin	0.376/0.187
N (hkl) measured	6523	156
N (hkl) unique	739
Rint/Rσ	0.066/0.026
N (hkl) observed	6316
Observation criterion	F(hkl) ≥ 4σ(F)
Refined parameters	33	22
Extinction coefficient	0.0389(8)
Goodness of fit, S	1.03	1.01
RF(RI); RW(RP)	0.014/0.015	0.056; 0.103
Residual peaks (e^− Å^−3)	−0.41/0.50	−0.57/0.73
M in 2f (1/2 y 3/4), y, Beq/iso ^b	0.3253(2), 0.70(1)	0.3278(3), 1.05(1)
d(M−2O1), d(M–2O2)	2.069(3), 2.140(3)	2.064(4), 2.171(5)
d(M–2O2), d(M–2M)	2.190(3), 3.1869(8)	2.248(5), 3.169(2)
W in 2e (0 y 1/4), y, Beq/iso	0.18021(4), 0.484(4)	0.1798(1), 1.13(1)
d(W–2O2), d(W–2O1)	1.789(3), 1.913(3)	1.736(4), 1.909(5)
d(W–2O1), d(W–2W)	2.129(3), 3.2267(2)	2.142(4), 3.2237(5)
O1 in 4g (xyz), x, y	0.2129(6), 0.1059(5)	0.2119(9), 0.1101(7)
z, Beq/iso	0.9351(5), 0.70(4)	0.934(1), 0.90(2)
O2 in 4g (xyz), x, y	0.2536(6), 0.3757(5)	0.2363(9), 0.3830(8)
z, Beq/iso	0.3933(6), 0.75(5)	0.379(1), 0.96(2)

---

Table 2 Anisotropic displacement parameters (B_ij, Ų) for Fe_0.8Mn_0.2WO₄

Atom	B11	B22	B33	B12	B13	B23
a M is a statistical mixture of (0.8Fe + 0.2Mn) in accordance with EDX analysis.
M^a	0.69(2)	0.77(2)	0.65(2)	0	0.02(2)	0
W	0.594(7)	0.431(6)	0.427(6)	0	−0.015(4)	0
O1	0.80(8)	0.69(8)	0.62(7)	−0.19(7)	0.04(6)	0.00(6)
O2	0.59(8)	0.86(8)	0.81(8)	−0.15(6)	−0.10(6)	−0.08(7)

---

As one can see from Table 2, the B₁₁ displacement parameter for the heavy W-atom is by a factor of ≈1.4 larger than B₂₂ and B₃₃. This fact together with the partial occupancy by Fe and W of the additional tetrahedral voids reported in ref. 4 prompted us to check the possibility of the latter scenario in the studied wolframite. For these purposes, we performed differential Fourier synthesis before refining ADPs. It indicated an additional electronic density of +2.85 e⁻ Å⁻³ localized at the 4g [0.041(2) 0.176(2) 0.2470(6)] crystallographic site, which is just a split position to the available W in 2e (Table 1). This observation brought us to the conclusion that the model proposed in ref. 4 cannot be applied to the natural wolframite. We assume instead that the enlarged B₁₁ for Fe_0.8Mn_0.2WO₄ is most probably due to mechanical stress in the microcrystal appearing after crushing the initial sample – an effect discussed in more detail elsewhere.^26–29

The obtained structural model from single crystal XRD was used to refine the powder XRD data, which converged with low reliability factors. The atomic coordinates and displacement parameters are collected in Table 1. The experimental, theoretical and differential profiles for it are depicted in Fig. 2. Importantly, the hkl reflections with h ≠ 0 and l ≠ 0 are found to reveal a clear split. It is visible in the inset to Fig. 2, where some of these peaks are presented in comparison with the (230) one. Such an indication of the monoclinic distortion can sometimes remain invisible, as it is the case in the sample observed in ref. 4.

Fig. 2 Powder XRD patterns for Fe_0.8Mn_0.2WO₄. Inset: indexing of the selected reflections. A clear split is visible for those with h ≠ 0 and l ≠ 0, confirming the monoclinic distortion.

The refined unit cell parameters (Table 1) agree well with the predictions made for Fe_1−xMn_xWO₄ tungstates on the basis of the XRD investigations of some natural minerals.⁶ The strongest deviation of ≈0.2% is observed for the a-parameter and thus, deducing from Fig. 5 in ref. 6, the Mn-content for the compound studied here would be x = 0.38. However, one can see from the study⁶ that the data obtained for x < 0.5 (e.g., available in ref. 4, 30 and 31) strongly deviate from the proposed linear x(V)-dependence. For this reason, the higher Mn-content in our mineral is rather unlikely.

The refined interatomic distances in the crystal structure of Fe_0.8Mn_0.2WO₄ (Table 1) agree well with the sums of ionic radii of the corresponding ions [r_(Fe²⁺) = 0.82 Å, r_(Mn²⁺) = 0.91 Å, and r_(O²⁻) = 1.32 Å].³² The maximal shortenings of Fe–O and W–O contacts do not exceed 3.3% and 7.8%, respectively, and agree well with the earlier reports.^4,31

The structural peculiarities of the NiWO₄ type are widely discussed in the literature.^4,10,31,33 It is considered as consisting of [FeO₆]- (distorted due to the Jahn–Teller effect) and [WO₆]-octahedra sharing their edges and forming zigzag chains along the [001] direction. Also, along the [100] direction, filled octahedra alternate with the empty ones, thus possessing common trigonal faces and being organized in columns (Fig. 3a). Such a close packing of octahedra gives rise to interstitial tetrahedral voids. They can become partially filled (Fig. 3b), as has been shown on the basis of combined single crystal XRD, TEM and SEM studies in ref. 4. Alternatively, one could consider the crystal structure of FeWO₄ to be consisting of trigonal puckered nets formed by oxygen atoms extending in the bc-plane and being at x ≈ 0.25 and 0.75, respectively (Fig. 3c). In the free space in-between, the planar trigonal nets (not shown in Fig. 3c) formed by W⁶⁺ (x = 0) and Fe²⁺ (x = 1/2) are incorporated. Such a layered presentation, however, has less chemical meaning due to the absence of bonding Fe–Fe, W–W and O–O contacts in the studied structure.

Fig. 3 (a) Arrangement of filled and empty octahedra in FeWO₄ (NiWO₄ type). (b) Structural model with partially filled tetrahedra (the full occupancy is shown) proposed for FeWO₄ in ref. 4. (c) Arrangement of the puckered trigonal nets W⁶⁺ and Fe²⁺ ions in-between (for more details, see the text).

3.2 Magnetic susceptibility

The temperature dependence of reciprocal magnetic susceptibility χ⁻¹(T) for Fe_0.8Mn_0.2WO₄ is depicted in Fig. 4. In the T-range of 130–300 K, it perfectly fits the Curie–Weiss law χ⁻¹ = (T − Θ_CW)/C. The Weiss temperature Θ_CW = −19(1) K and the effective magnetic moment obtained from such a fit agree well with those reported for pure FeWO₄.^34,35 The somewhat smaller Θ_CW value [−19(1) K instead of −27 K] can be explained by the relatively narrow T-range in which our fit was performed.

Fig. 4 Temperature dependence of reciprocal magnetic susceptibility for Fe_0.8Mn_0.2WO₄ (μ₀H = 3.5 T) together with the Curie–Weiss fit (red line). Inset a: dependence of critical Néel-temperatures (T_N) versus Mn content (x) in Fe_1−xMn_xWO₄. Inset b: temperature dependence of reciprocal magnetic susceptibility for Fe_0.8Mn_0.2WO₄ in different magnetic fields.

With the lowering temperature, Fe_0.8Mn_0.2WO₄ undergoes an antiferromagnetic (AF) transition at T_N1 = 67(1) K, in agreement with ref. 36, and further changes its magnetic structure at T_N2 = 28(3) K. As one can see from inset a to Fig. 4, the value of T_N1 nicely fits that of T_N from Mn-content dependence, confirming the correctness of the chemical composition (established from EDX) of the studied mineral. Additionally, almost no and very weak field dependencies are observed for T_N1 and T_N2, respectively (inset b to Fig. 4). All these findings agree well with the earlier reports about complex magnetic phase formation in Fe_1−xMn_xWO₄, which is caused by the various superexchange couplings between adjacent Mn–Mn, Fe–Fe, and Mn–Fe-ions via one or two intervening oxygen ions.^1,11,34

3.3 Specific heat

The temperature dependence of specific heat capacity cp(T) for Fe0.8Mn0.2WO4 in different magnetic fields in comparison with the synthetic ternary FeWO4 and phonon reference compound ZnWO4 (both these data are taken from ref. 9) is depicted in Fig. 5. In agreement with magnetic susceptibility (inset b to Fig. 4) no suppression of AF with increasing field is observed. Also, expectedly, the anomaly due to the antiferromagnetic ordering at T\,cpN1 = 66(1) K in the studied mineral is shifted towards a lower T (inset to Fig. 4).

Fig. 5 Temperature dependencies of specific heat capacity in different magnetic fields for Fe_0.8Mn_0.2WO₄ together with the literature data for FeWO₄ and ZnWO₄.⁹

Subtracting further the phonon reference, we obtained the electronic specific heat for Fe0.8Mn0.2WO4. Presenting it in cel/T(T) mode and integrating it, we obtained the temperature evolution of the magnetic entropy . As one can see from Fig. 6, a value slightly larger than R ln3 can be reached upon the transition. Obviously, it is much lower than the theoretical expectations for Fe2+ (S = 2) or Fe3+ (S = 5/2) ions given as ΔSmag = R ln(2S + 1) = R ln5 and R ln6, respectively. On the other hand, assuming the influence of the crystal electric field effect on Fe-ions, and thus electronic configurations with S = 1 or 3/2, one could also deduce the entropies R ln3 and R ln4, respectively. It is noteworthy that the reduced total spin angular momenta should also indicate much lower effective magnetic moments disagreeing with our experiment. All these hints towards the failure of the simplified classical LS-coupling scheme in the description of the complex magnetic behavior in Fe0.8Mn0.2WO4. Importantly, as it is also clearly visible from Fig. 4 in ref. 37, ΔSmag upon the HT-magnetic transition in the pristine MnWO4 is well below R ln6. Even more, this value does not reach up to 30 K. Besides that, a well pronounced and broadened (i.e., due to the cation mixture) anomaly at T\,cpN2 ≈ 10(1) K in our sample becomes especially clearly visible in the cel/T(T) presentation (inset to Fig. 6). It perfectly overlaps with the antiferromagnetic anomalies at TN1 = 13.4 K, TN2 = 12.5 K and TN3 = 7.4 K in MnWO4,38,39 stressing additionally the complexity of the magnetic structure of Fe0.8Mn0.2WO4.

Fig. 6 Temperature dependence of the electronic specific heat of Fe_0.8Mn_0.2WO₄ in the c_el/T(T) presentation (left black scale) together with the temperature evolution of the magnetic entropy near an antiferromagnetic transition. Inset: low-temperature dependencies of c_el/T(T) for Fe_0.8Mn_0.2WO₄ together with the literature data for MnWO₄.³⁷ The latter were divided by 20 for better visualization.

3.4 Electrical and thermal transport

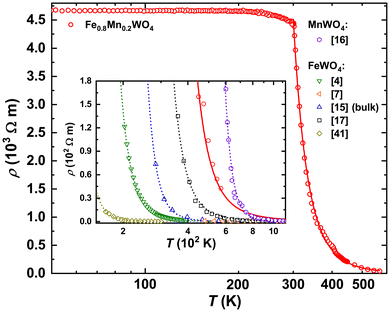
The temperature dependence of electrical resistivity ρ(T) for Fe_0.8Mn_0.2WO₄ is depicted in the inset to Fig. 7. It increases exponentially with decreasing temperature down to ≈300 K and then saturates, reaching the ρ ≈ 4.8 kΩ m value. In the whole studied range, it is by ≈3–5 orders of magnitude higher than ρ(T) reported for CuFeS₂ (i.e., an impurity phase in our sample),⁴⁰ thus confirming the purity of the studied specimen.

Fig. 7 The temperature dependence of electrical resistivity ρ(T) for Fe_0.8Mn_0.2WO₄ together with the Arrhenius plot (red solid line). Inset: ρ(T) for Fe_0.8Mn_0.2WO₄ in comparison with those of undoped FeWO₄ and MnWO₄. Dotted and solid lines correspond to the plots of the Arrhenius approximation.

Its comparison with the ρ(T) of undoped FeWO₄ and MnWO₄ is presented in Fig. 7. As it is seen therein, all these dependencies can be nicely described by the Arrhenius approximation given as ρ = ρ₀e^E_g/K_bT, where E_g is the energy gap. Interestingly, the latter value for FeWO₄ is reported to vary in a broad range of 150–260 meV.^{4,15–17,41,42} Since the authors of ref. 4, 15 and 41 performed their studies on single crystalline materials obtained from chemical vapor transport reactions and in ref. 16 and 17 bulk samples were studied, one has to conclude on the extreme sensitivity of ρ(T) to any even minor impurities.

As it is discussed in ref. 41 and 43, Mn²⁺-ions in Fe_1−xMn_xWO₄ do not block the electron hopping paths, thus allowing charge carrier transfer also between chains, which results in the enhancement of ρ(T). In agreement with this report, Fe_0.8Mn_0.2WO₄ reveals both higher electrical resistivity (Fig. 7) and an energy gap value of E_g = 310 meV (to be able to compare our value with the previously reported one, we performed an Arrhenius fit for the temperature range of 480–560 K). Importantly, the latter value is smaller than E_g = 705 meV of MnWO₄ ¹⁶ and larger than those observed for FeWO₄ (see the discussion above). Fitting ρ(T) in the whole measured range of 300–560 K, one would obtain E_g = 247 meV for Fe_0.8Mn_0.2WO₄, clearly breaking the trend visible in Fig. 7. It is noteworthy that the band gap values deduced from ρ(T) are always underestimated and the true E_g values for MnWO₄ and FeWO₄ deduced from spectroscopic measurements are 2.6–3 eV (ref. 7 and 18) and 2 eV,^18,19,44 respectively.

The Seebeck coefficients of thermopower α(T) of all Fe_1−xMn_xWO₄-specimens studied up to now are positive in the whole temperature range, thus assuming the dominance of the hole-like conduction mechanism therein. Also, the α(T) of single crystalline FeWO₄ decreases nearly linearly with increasing temperature,^4,15,17 whereas for a bulk sample, an increase is reported¹⁴ (Fig. 7). This is again an indication of the sensitivity of thermopower to the samples’ crystallinity, similar to that discussed above for ρ(T) dependencies. Interestingly, the α(T) of polycrystalline FeWO₄ ¹⁴ and MnWO₄ ¹⁶ (not shown in Fig. 8) reveal a change in the slope at a certain temperature, which is explained in both cases by the increase of the charge carrier mobilities. In line with these observations, the α(T) of single crystalline Fe_0.8Mn_0.2WO₄ decreases linearly with T. In the temperature range of ≈450–600 K, it is also the largest one [seemingly, the α(T) of MnWO₄ would become larger for T > 650 K].

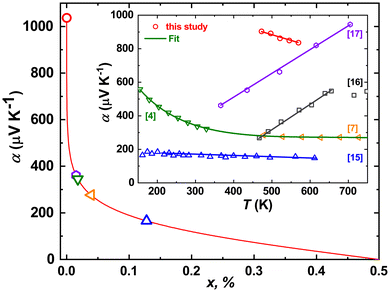Fig. 8 Seebeck coefficient α_{300 K} as a function of holes (Fe³⁺) per Fe-site concentration (x) calculated from the Heikes equation (eqn (1)). Inset: temperature dependence of the Seebeck coefficient of thermopower α(T) for Fe_0.8Mn_0.2WO₄ in comparison with FeWO₄ ^4,7,15,16 and MnWO₄ ¹⁷ samples of different origins.

Assuming now the α(T) of Fe_0.8Mn_0.2WO₄ to remain linear down to 300 K and the applicability of the simplified Heikes equation (i.e., considering the degeneracy factors g_A and g_B to be equal)^45–47 given as:

(where x is holes’ concentration per site), we derived the α(x) dependence depicted in Fig. 8. Comparing α_{300 K} = 1040 μV K⁻¹ with such a plot, one obtains ≈0.002% of Fe³⁺ holes in the studied compound − a value, which is few orders of magnitude smaller than those reported for FeWO₄ of different origins. This finding is also in line with the performed structure refinement, confirming the studied specimen to be more stoichiometric in comparison with the pristine FeWO₄.

The temperature dependence of thermal conductivity κ(T) for Fe0.8Mn0.2WO4 is presented in Fig. 9. It is high for 5 K < T < 50 K (e.g., by a factor of ≈3 higher than those of the high quality pyrite crystal48) and reveals a well pronounced maximum at Tmax ≈ 12 K, which corroborates the good crystallinity and high quality of the sample. For T > 100 K, κ(T) slightly increases almost linearly with temperature, which most probably occurs due to radiation heat losses (inset to Fig. 9).49,50 Interestingly, two anomalies can be observed: (i) the increase of κph(T) that begins at TN1 = 67(1) K and (ii) an irregular behavior visible at T\,cpN2 ≈ 8(1) K, in line with the specific heat measurements.

Fig. 9 Temperature dependence of thermal conductivity κ(T) for Fe_0.8Mn_0.2WO₄ together with the fit to eqn (2). Inset: deviation of experimental data from the theoretical expectation due to radiation heat losses.

Since electrical resistivity for the studied mineral at 300 K is expected to be ≈12 kΩ m, the electronic component of κ(T), in accordance with the Wiedemann–Franz law, would be κ_el(300 K) = L₀T/ρ = 6.1 × 10⁻¹⁰ W m⁻¹ K⁻¹ (L₀ = 2.44 × 10⁻⁸ W Ω K⁻² is the Lorenz number). Comparing this κ_el with the values in Fig. 9, one can conclude that the κ(T) of Fe_0.8Mn_0.2WO₄ is completely dominated by phonons (i.e., κ_ph), and thus, obeys the Debye–Callaway model⁵¹ given as:

where

τ_tot⁻¹ = τ_B⁻¹ + τ_PD⁻¹ + τ_N⁻¹ + τ_U⁻¹ (3)

i.e., τ_tot is the sum of different scattering mechanisms including scattering of phonons on grain boundaries (τ_B) and point defects (τ_PD) as well as normal (τ_N) and umklapp (τ_U) phonon–phonon processes. The former two dominate the LT-range whereas the latter two dominate the HT-range.

The parameter Θ_D in eqn (2) denotes the Debye temperature of Fe_0.8Mn_0.2WO₄. It is rather impossible to deduce it from the above described c_p(T); therefore we fitted the specific heat data for ZnWO₄ taken from ref. 9 in the T-range of 3–20 K to the c_p(T) = γT + βT³ + δT⁵ ansatz, thus obtaining the Sommerfeld coefficient γ = 7.1(9) mJ mol⁻¹ K⁻², β = 0.11(9) mJ mol⁻¹ K⁻⁴ [corresponds to Θ_D (ZnWO₄) = 477(3) K] and δ = 4.1(9) × 10⁻⁴ mJ mol⁻¹ K⁻⁶. To obtain an approximate Debye temperature of Fe_0.8Mn_0.2WO₄, the value above was multiplied by [M_r(ZnWO₄)/M_r(Fe_0.8Mn_0.2WO₄)]^1/2 = 1.016, where M_r is the corresponding molar mass. Having now Θ_D (Fe_0.8Mn_0.2WO₄) = 485 K, we further calculate the velocity of sound for the studied mineral v_S = 3726 m s⁻¹ using eqn (4) (therein n_at = 8.97 × 10²⁸ m⁻³ is the concentration of atoms per unit cell volume).

Introducing the Θ_D and v_S values in eqn (2), we fit κ(T) in the temperature range of 1.8–106 K (Fig. 9) with an overall reliability factor R² = 0.993. The obtained parameters and their standard deviations are summarized in Table 3.

Table 3 Fit parameters (C_i) from the Debye–Callaway model (eqn (2)) together with the expression for the respective phonon scattering mechanisms from ref. 32

τ^−1	Equation	Ci	Units for Ci
τB^−1	vS/C1	2.7(1)	[10^−4 m]
τPD^−1	C2x^4T^4	183(4)	—
τN^−1	C3xT^3	5.96(9)	[10^5 s^−1 K^−3]
τU^−1	C4x^2T^3e^(−ΘD/3T)	3.9(5)	[10^6 s^−1 K^−3]

---

As one can see, the C1 coefficient indicates a rather large grain boundary size in agreement with the excellent microstructural quality of the studied single crystalline specimen. The C2 parameter, which is due to the point defects (i.e., Mn-doping and/or the Fe³⁺-holes), is approximately one order of magnitude smaller than single crystalline PbS⁵² and polycrystalline indium thiospinels.⁵³ In the HT-range, we observe the contribution of both normal-(C3) and umklapp (C4) phonon–phonon processes, as expected for single crystalline materials.

The discrepancies between the fit and experiment observed in the LT-region just below the maximum could be attributed to the features present in c_p(T) (Fig. 5). To shed light on this problem, measurements performed on different samples with the same microstructure (i.e., equal or similar τ_B and τ_PD) are necessary. Interestingly, the further mismatch of fit to eqn (4) to the experiment appears for T > T_N1 = 67(1) K. Obviously, to explain the κ(T) behavior for the temperature range of 70–100 K, perturbation density functional theory (pDFT) calculations combined with additional experiments aiming at the estimation of phonon dispersions in Fe_0.8Mn_0.2WO₄ are required.

4 Conclusions

Combined X-ray powder diffraction (PXRD) and energy dispersive X-ray spectroscopic (EDXS) studies confirmed the natural crystal from the wolframite group originating from the Potosí province in Bolivia to be of high quality containing only local inclusions of chalcopyrite and some silicates. Its chemical formula was found to be Fe_1−xMn_xWO₄ (x = 0.2). Further single crystal and powder XRD experiments confirmed the mineral to crystallize with the monoclinic wolframite structure type. A slight monoclinic distortion is clearly evidenced by the split of hkl reflections with h ≠ 0 and l ≠ 0 in the PXRD patterns.

The measurements of the temperature and field-dependent magnetic susceptibility for Fe1−xMnxWO4 (x = 0.2) indicated an effective magnetic moment close to that expected for Fe2+ ions. A complex magnetic structure with transitions at TN1 = 67(1) K and TN2 = 28(3) K is also in line with the earlier studied quaternary wolframites. Further confirmation of this complexity is deduced from specific heat measurements revealing anomalies at T\,cpN1 = 66(1) K and T\,cpN2 = 8(1) K. It is noteworthy that the reduced magnetic entropy of ≈R ln 3 (instead of R ln5) upon the HT transition indicates the failure of the simplified LS-coupling scheme in the description of the magnetism in the studied mineral.

The electrical resistivity of Fe_1−xMn_xWO₄ (x = 0.2) increases exponentially upon decreasing temperature reaching a saturation value of ρ ≈ 4.8 kΩ m below 300 K. The high-temperature energy gap of 310 meV, deduced from the exponential decrease with temperature, is expectedly closer to FeWO₄ (150–260 meV) than to MnWO₄ (710 meV).

The measured thermal conductivity slightly decreases with decreasing temperature down to TN1 ≈ 66(1) K and then overcomes a huge maximum centered at Tmax ≈ 12 K, revealing some additional anomaly at T\,cpN2 ≈ 8(1) K. Assuming it to be dominated by the phonon processes [the electronic contribution to κ(T) is found to be ≈10−10 W m−1 K−1], the Debye–Callaway model was applied. Such a fit indicated enlarged grain boundary sizes, enhanced defect concentration and the dominance of umklapp processes in the studied single crystal.

Data availability

The data supporting this article have been included as part of the ESI.†

Conflicts of interest

There are no conflicts of interest to declare.

Acknowledgements

The QD DynaCool-12 measurement system was acquired within the DFG project 422219907. We thank S. Kostmann and U. Burkhardt for performing EDX characterization of our sample and H. Borrmann, E. Meier and A. Schwarzer for their assistance during XRD measurements. V. L. is grateful to DFG for financial support (project 467257848).

References

1. D. Diaz-Anichtchenko, J. E. Aviles-Coronado, S. López-Moreno, R. Turnbull, F. J. Manjón, C. Popescu and D. Errandonea, Inorg. Chem., 2024, 63, 6898–6908.
2. O. Fabelo, J. Gonzalez-Platas, S. Savvin, P. Botella and D. Errandonea, J. Appl. Phys., 2024, 136, 175901.
3. S. G. Altendorf, D. Takegami, A. Meléndez-Sans, C. F. Chang, M. Yoshimura, K. D. Tsuei, A. Tanaka, M. Schmidt and L. H. Tjeng, Phys. Rev. B, 2023, 108, 085119.
4. A. Maignan, M. Schmidt, Y. Prots, O. I. Lebedev, R. Daou, C.-F. Chang, C.-Y. Kuo, Z. Hu, C.-T. Chen, S.-C. Weng, S. G. Altendorf, L.-H. Tjeng and Y. Grin, Chem. Mater., 2022, 34, 789–797.
5. M. Assis, A. C. Tello, F. S. Abud, P. Negre, L. K. Ribeiro, R. A. Ribeiro, S. H. Masunaga, A. E. Lima, G. E. Luz Jr, R. F. Jardim, A. B. Silva, J. Andrés and E. Longo, Appl. Surf. Sci., 2022, 600, 154081.
6. D. A. Umbsaar and S. M. Antao, Minerals, 2021, 12, 42.
7. R. Schuler, F. Bianchini, T. Norby and H. Fjellvåg, ACS Appl. Mater. Interfaces, 2021, 13, 7416–7422.
8. B. Huang and J. N. Hart, Phys. Chem. Chem. Phys., 2020, 22, 1727–1737.
9. W. G. Lyon and E. F. Westrum, J. Chem. Thermodyn., 1974, 6, 763–780.
10. R. O. Keeling, Acta Crystallogr., 1957, 10, 209–213.
11. E. García-Matres, N. Stüßer, M. Hofmann and M. Reehuis, Eur. Phys. J. B, 2003, 32, 35–42.
12. G. Lautenschläger, H. Weitzel, T. Vogt, R. Hock, A. Böhm, M. Bonnet and H. Fuess, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 48, 6087–6098.
13. I. Urcelay-Olabarria, J. M. Perez-Mato, J. L. Ribeiro, J. L. García-Muñoz, E. Ressouche, V. Skumryev and A. A. Mukhin, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 87, 014419.
14. H. Obermayer, H. Dachs and H. Schröcke, Solid State Commun., 1973, 12, 779–784.
15. E. Schmidbauer, U. Schanz and F. J. Yu, J. Phys.: Condens. Matter, 1991, 3, 5341–5352.
16. R. Bharati, R. Singh and B. Wanklyn, J. Phys. Chem. Solids, 1982, 43, 641–644.
17. R. Bharati, Y. P. Yadava and R. A. Singh, J. Mater. Sci. Lett., 1983, 2, 808–810.
18. T. Ejima, T. Banse, H. Takatsuka, Y. Kondo, M. Ishino, N. Kimura, M. Watanabe and I. Matsubara, J. Lumin., 2006, 119–120, 59–63.
19. S. Rajagopal, V. Bekenev, D. Nataraj, D. Mangalaraj and O. Khyzhun, J. Alloys Compd., 2010, 496, 61–68.
20. K. Hoang, Phys. Rev. Mater., 2017, 1, 024603.
21. D. Errandonea and J. Ruiz-Fuertes, Crystals, 2018, 8, 71.
22. R. Guillen and J. R. Regnard, Phys. Chem. Miner., 1985, 12, 246–254.
23. T. Birkett, Can. Mineral., 2003, 41, 1296–1297.
24. STOE Powder Software, WinXPow (version 2), STOE and Cie GmbH, Darmstadt, 2001.
25. L. Akselrud and Y. Grin, J. Appl. Crystallogr., 2014, 47, 803–805.
26. P. J. Withers, Rep. Prog. Phys., 2007, 70, 2211–2264.
27. N. Rossini, M. Dassisti, K. Benyounis and A. Olabi, Mater. Des., 2012, 35, 572–588.
28. P. Withers and H. Bhadeshia, Mater. Sci. Technol., 2001, 17, 355–365.
29. A. Nezu, H. Matsuzaka and R. Yokoyama, Rigaku J., 2014, 30, 4–12.
30. C. Escobar, H. Cid-Dresdner, P. Kittl and I. Dümler, Am. Mineral., 1971, 56, 489–498.
31. D. Ülkü, Z. Kristallogr. Krist., 1967, 124, 192–219.
32. J. Emsley, The Elements, Clarendon Press, Oxford, 3rd edn, 1998.
33. M. Almeida, L. Cavalcante, C. Morilla-Santos, P. L. Filho, A. Beltrán, J. Andrés, L. Gracia and E. Longo, Mater. Charact., 2012, 73, 124–129.
34. H. Weitzel, Solid State Commun., 1969, 7, 1249–1252.
35. M. A. P. Almeida, L. S. Cavalcante, C. Morilla-Santos, C. J. Dalmaschio, S. Rajagopal, M. S. Li and E. Longo, CrystEngComm, 2012, 14, 7127–7132.
36. Y.-X. Zhou, H.-B. Yao, Q. Zhang, J.-Y. Gong, S.-J. Liu and S.-H. Yu, Inorg. Chem., 2009, 48, 1082–1090.
37. C. P. Landee and E. F. Westrum, J. Chem. Thermodyn., 1976, 8, 663–674.
38. C. P. Landee, J. Westrum and F. Edgar, AIP Conf. Proc., 1976, 29, 445–446.
39. C. M. N. Kumar, Y. Xiao, P. Lunkenheimer, A. Loidl and M. Ohl, Phys. Rev. B: Condens. Matter Mater. Phys., 2015, 91, 235149.
40. P. Wyżga, M. Bobnar, C. Hennig, A. Leithe-Jasper, T. Mori and R. Gumeniuk, Z. Anorg. Allg. Chem., 2017, 643, 858–863.
41. K. Sieber, K. Kourtakis, R. Kershaw, K. Dwight and A. Wold, Mater. Res. Bull., 1982, 17, 721–725.
42. Y. Noda, M. Shimada, M. Koizumi and F. Kanamaru, J. Solid State Chem., 1979, 28, 379–384.
43. K. Sieber, H. Leiva, K. Kourtakis, R. Kershaw, K. Dwight and A. Wold, J. Solid State Chem., 1983, 47, 361–367.
44. W. S. Choi, K. Taniguchi, S. J. Moon, S. S. A. Seo, T. Arima, H. Hoang, I.-S. Yang, T. W. Noh and Y. S. Lee, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 81, 205111.
45. J. Robert, R. Heikes and R. W. Ure, Thermoelectricity: Science and Engineering, Interscience Publishers, New York - London, 1961.
46. J.-S. Zhou and J. B. Goodenough, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 60, R15002–R15004.
47. P. M. Chaikin and G. Beni, Phys. Rev. B, 1976, 13, 647–651.
48. E. Zuñiga-Puelles, R. Cardoso-Gil, M. Bobnar, I. Veremchuk, C. Himcinschi, C. Hennig, J. Kortus, G. Heide and R. Gumeniuk, Dalton Trans., 2019, 48, 10703–10713.
49. K. A. Borup, J. de Boor, H. Wang, F. Drymiotis, F. Gascoin, X. Shi, L. Chen, M. I. Fedorov, E. Müller, B. B. Iversen and G. J. Snyder, Energy Environ. Sci., 2015, 8, 423–435.
50. Thermal transport option user's manual, Quantum design, Inc. 6325 Lusk Blvd., San Diego, CA, USA technical report, 2002.
51. T. M. Tritt, Thermal Conductivity: Theory, Properties, and Applications, Springer New York, NY, 2004.
52. E. Zuñiga-Puelles, V. Levytskyi, A. Özden, T. Gürel, N. Bulut, C. Himcinschi, C. Sevik, J. Kortus and R. Gumeniuk, Phys. Rev. B, 2023, 107, 195203.
53. E. Zuñiga-Puelles, A. Özden, V. Pacheco, L. Akselrud, R. Cardoso-Gil, A. Straßheim, P. Wyżga, C. Himcinschi and R. Gumeniuk, J. Alloys Compd., 2024, 976, 173055.

---

Footnote

† Electronic supplementary information (ESI) available. CCDC 2425342. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d5dt00332f

---