Magnetic interactions in the S = 1/2 square-lattice antiferromagnets Ba₂CuTeO₆ and Ba₂CuWO₆: parent phases of a possible spin liquid

Abstract

The isostructural double perovskites Ba₂CuTeO₆ and Ba₂CuWO₆ are shown by theory and experiment to be frustrated square-lattice antiferromagnets with opposing dominant magnetic interactions. This is driven by differences in orbital hybridisation of Te⁶⁺ and W⁶⁺. A spin-liquid-like ground state is predicted for Ba₂Cu(Te_1−xW_x)O₆ solid solution similar to recent observations in Sr₂Cu(Te_1−xW_x)O₆.

---

Magnetic frustration can stabilise novel quantum ground states such as quantum spin liquids or valence bond solids.¹ Frustration occurs when not all of the magnetic interactions in a material can be satisfied simultaneously as a result of lattice geometry or competing interactions. We have recently shown that a quantum-spin-liquid-like state forms in the double perovskite solid solution Sr₂Cu(Te_1−xW_x)O₆ with a square lattice of Cu²⁺ (3d⁹, S = 1/2) cations.^2,3 This was the first observation of a spin-liquid-like state in a square-lattice compound after 30 years of theoretical predictions.^4–8

The parent compounds Sr₂CuTeO₆ and Sr₂CuWO₆ are frustrated square-lattice (FSL) antiferromagnets.^9–13 The FSL model (Fig. 1) has two interactions: nearest-neighbour J₁ interaction (side) and next-nearest-neighbour J₂ interaction (diagonal). Dominant antiferromagnetic J₁ leads to Néel type antiferromagnetic order and dominant J₂ leads to columnar magnetic order. Magnetic frustration arises from the competition of J₁ and J₂, and a quantum spin liquid state has been predicted for J₂/J₁ = 0.5 where frustration is maximised.^4–8

Fig. 1 (a) Phase diagram of the frustrated square-lattice model. Antiferromagnetic (negative) J₁ stabilises Néel order and J₂ columnar order respectively. A spin liquid state has been predicted for the Néel–columnar boundary at J₂/J₁ = 0.5 where magnetic frustration is maximised. (b) The double perovskite structure of (Ba,Sr)₂Cu(Te,W)O₆. J₁ and J₂ are the in-plane interactions of the FSL model, whereas J₃ and J₄ are out-of-plane interactions. The blue, dark yellow, red and green spheres represent Cu, Te/W, O and Ba/Sr, respectively. (c) The Cu²⁺ square in the ab plane with J₁ and J₂ interactions.

Sr₂CuTeO₆ and Sr₂CuWO₆ are the first known isostructural FSL systems with different dominant interactions and magnetic structures: dominant J₁ and Néel order for Sr₂CuTeO₆ and dominant J₂ and columnar order for Sr₂CuWO₆ respectively.^9,10 The two compounds have a tetragonal I4/m double perovskite structure with nearly identical bond distances and angles.^11,13 The magnetism becomes highly two-dimensional as a result of a Jahn–Teller distortion as the only unoccupied Cu orbital 3d_x²−y² is in the ab square plane. The major differences in dominant magnetic interactions are due to the diamagnetic Te⁶⁺ d¹⁰ and W⁶⁺ d⁰ cations located in the middle of the Cu²⁺ square (Fig. 1c), which hybridise differently with O 2p allowing different superexchange paths between the Cu²⁺ cations.^14,15 The spin-liquid-like ground state forms when these two perovskites are mixed into a Sr₂Cu(Te_1−xW_x)O₆ solid solution.^2,3,16 Muon spin relaxation experiments revealed the absence of magnetic order or static magnetism in a wide composition range of x = 0.1–0.6.^2,3 The specific heat displays T-linear behaviour suggesting gapless excitations in a similar composition range.^2,3,16 The ground state has been proposed to be a random-singlet state with a disordered arrangement of non-magnetic valence bond singlets.^17,18

Motivated by these exciting findings in the Sr₂Cu(Te_1−xW_x)O₆ system, we have investigated the magnetic interactions of the isostructural barium analogues Ba₂CuTeO₆ and Ba₂CuWO₆. Ba₂CuWO₆ is known to have columnar magnetic order,^19,20 but little is known about Ba₂CuTeO₆ as the perovskite phase requires high pressures to synthesise.²¹ Here we use density functional theory (DFT) calculations and high-temperature series expansion (HTSE) fitting of experimental susceptibility data to show that these compounds are FSL antiferromagnets with opposite dominant interactions similar to Sr₂CuTeO₆ and Sr₂CuWO₆. We predict a quantum-spin-liquid-like state in Ba₂Cu(Te_1−xW_x)O₆ with strong antiferromagnetic interactions.

Magnetic interactions and electronic structure in Ba₂CuTeO₆ and Ba₂CuWO₆ were calculated using the DFT+U framework, where an on-site Coulomb repulsion term U was used to model electron correlation effects of localised Cu 3d orbitals. Interactions up to the fourth-nearest neighbour were evaluated, see Fig. 1b. J₁ and J₂ are the square plane interactions of the FSL model, and J₃ and J₄ are additional out-of-plane interactions. Energies of different spin configurations were mapped onto a Heisenberg Hamiltonian to obtain J₁–J₄. We have previously shown this approach works well for Sr₂CuWO₆.¹⁰ The J₁ and J₂ interactions were also determined from experimental magnetic susceptibility data using high-temperature series expansion fitting. Ba₂CuTeO₆ was prepared by high-pressure synthesis and Ba₂CuWO₆ by conventional solid state synthesis. Details of the DFT calculations, sample synthesis and characterisation are available in the ESI.†

The calculated magnetic interactions of Ba₂CuTeO₆ and Ba₂CuWO₆ are presented in Table 1. The calculated values depend on the Coulomb U term as is typical with DFT+U, but the same trends are observed for reasonable values of U. Despite being isostructural, the magnetic interactions in Ba₂CuTeO₆ and Ba₂CuWO₆ are very different. Ba₂CuTeO₆ has a very dominant antiferromagnetic J₁ interaction with weak J₂, J₃ and J₄ interactions. It is a near-ideal FSL Néel antiferromagnet. Ba₂CuWO₆, in contrast, has a dominant antiferromagnetic J₂ interaction slightly frustrated by an antiferromagnetic J₁ interaction with negligible J₃ and J₄ interactions. The strong J₂ interaction is consistent with the known columnar magnetic structure of this compound.²⁰ Due to the weakness of the out-of-plane J₃ and J₄ interactions, magnetism in both compounds is highly two-dimensional and well described by the FSL model.

Table 1 Exchange constants of Ba₂CuTeO₆ and Ba₂CuWO₆ obtained by density functional theory using different on-site Coulomb U terms and by high-temperature series expansion fitting of magnetic susceptibility data. Negative (positive) values correspond to antiferromagnetic (ferromagnetic) interactions

	U = 7 eV	U = 8 eV	U = 9 eV	HTSE
a Significant uncertainty in this value due to error in J1.
Ba2CuTeO6
J 1 (meV)	−23.65	−20.22	−17.22	−16.54(3)
J 2 (meV)	0.13	0.23	0.06	−0.04(3)
J 3 (meV)	1.28	0.83	0.67	—
J 4 (meV)	−0.30	0.01	0.05	—
J 2/J1	−0.01	−0.01	−0.003	0.002
Ba2CuWO6
J 1 (meV)	−1.25	−1.17	−1.27	0.2(9)
J 2 (meV)	−14.71	−11.94	−9.56	−10.0(1)
J 3 (meV)	0.05	−0.01	0.01	—
J 4 (meV)	0.03	0.37	0.02	—
J 2/J1	11.79	10.18	7.55	−50^a

---

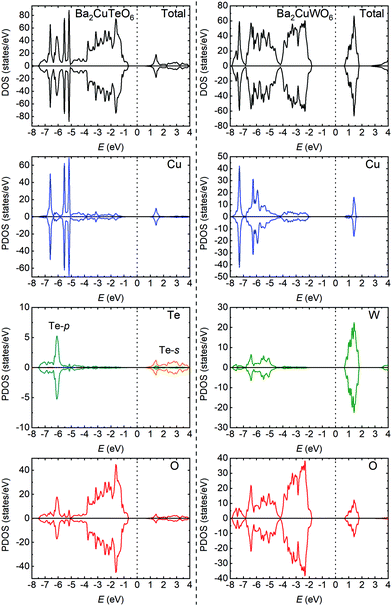
The significant differences in the magnetic interactions of Ba₂CuTeO₆ and Ba₂CuWO₆ can be explained by their electronic structures. We have plotted total and partial densities of states for both compounds in Fig. 2. Ba₂CuTeO₆ and Ba₂CuWO₆ are antiferromagnetic insulators: the band gaps open between the occupied Cu 3d states hybridised with O 2p (valence band) and the unoccupied Cu 3d_x²−y² states hybridised with O 2p (conduction band). In Ba₂CuWO₆ the conduction band is further hybridised with unoccupied W 5d states. The W 5d states also hybridise with the Cu 3d/O 2p states in the valence band, which allows a 180° Cu–O–W–O–Cu superexchange pathway resulting in a strong antiferromagnetic J₂ interaction. This hybridisation does not occur in Ba₂CuTeO₆ and therefore J₂ is negligible. In Ba₂CuTeO₆ the Te 5p states hybridise to a lesser degree with the Cu 3d/O 2p states in the conduction band, which could explain the strong antiferromagnetic J₁ interaction. However, the role of Te in the J₁ superexchange in Sr₂CuTeO₆ is under debate.^9,14 Overall, the electronic structures of Ba₂CuTeO₆ and Ba₂CuWO₆ are similar to their strontium analogues Sr₂CuTeO₆ and Sr₂CuWO₆, and the differences in magnetic interactions are driven by the same orbital hybridisation mechanism.

Fig. 2 Total and partial density of states plots for Ba₂CuTeO₆ (left) and Ba₂CuWO₆ (right). Both compounds are antiferromagnetic insulators. The moderate Te 5p/5s–O 2p hybridisation and stronger W 5d–O 2p hybridisation are seen in the Te/W and O PDOS plots.

The experimental magnetic susceptibilities of synthesised Ba₂CuTeO₆ and Ba₂CuWO₆ samples are shown in Fig. 3. The broad maximum observed in the susceptibility is due to the two-dimensional nature of the magnetism in these materials. Our maximum temperature of 400 K was not enough for reliable Curie–Weiss fits. Previous measurements²¹ up to 800 K yielded the Curie–Weiss constants Θ_CW = −400 K for Ba₂CuTeO₆ and Θ_CW = −249 K for Ba₂CuWO₆ revealing strong antiferromagnetic interactions.

Fig. 3 Magnetic susceptibility and high-temperature series expansion fits for Ba₂CuTeO₆ and Ba₂CuWO₆. Open symbols represent experimental data and the lines are HTSE fits with the parameters J₁ = −16.54(3) meV, J₂ = −0.04(3) meV, g = 2.20(1) and J₁ = 0.2(9) meV, J₂ = −10.0(1) meV, g = 2.26(5) for Ba₂CuTeO₆ and Ba₂CuWO₆, respectively. The ZFC and FC curves overlap and therefore only ZFC data is shown.

The magnetic susceptibilities were fitted to a high-temperature series expansion of the FSL model.²² The molar magnetic susceptibility χ_mol is given by:

where g is the effective g-factor, β = −J₁/k_B, x = J₂/J₁, χ₀ is a temperature independent diamagnetic correction and the coefficients c_m,n are from Table 1 in ref. 22. The model has four parameters: J₁, J₂, g and χ₀, which were fitted to the experimental data using a least squares method. The model always produces two solutions due to internal symmetry: one with dominant J₁ and one with dominant J₂.²³ Our DFT calculations allow us to select the correct dominant J₁ solution for Ba₂CuTeO₆ and the dominant J₂ solution for Ba₂CuWO₆.

The best fits were obtained with the parameters J₁ = −16.54(3) meV, J₂ = −0.04(3) meV, g = 2.20(1) for Ba₂CuTeO₆ and J₁ = 0.2(9) meV, J₂ = −10.0(1) meV, g = 2.26(5) for Ba₂CuWO₆ in the temperature ranges 150–400 K and 90–400 K, respectively. The fitted exchange constants depend slightly on the minimum temperature used. For both compounds the calculated dominant interaction remains stable in a wide fitting range, but the weaker interaction cannot be accurately quantified. In Ba₂CuTeO₆ the sign of J₂ changes depending on the fitting range, whereas in Ba₂CuWO₆ the error of J₁ is much larger than its value. We can conclude, however, that the dominant interaction is much stronger than the weak one in both Ba₂CuTeO₆ (|J₂|/|J₁| < 0.02) and Ba₂CuWO₆ (|J₁|/|J₂| < 0.12) and that the DFT and HTSE results are in good agreement.

The magnetic properties of Ba₂CuTeO₆, Ba₂CuWO₆, Sr₂CuTeO₆ and Sr₂CuWO₆ are summarised in Table 2. Magnetic interactions in Ba₂CuTeO₆ and Ba₂CuWO₆ are notably stronger than their strontium analogues. This is due to the smaller a⁰a⁰c⁻ tilt of the CuO₆ octahedra in the barium phases, which leads to stronger orbital overlap in the ab plane as the Cu–O–Te/W angle is closer to 180°,²¹ see ESI† for further details. As long-range magnetic order is driven by the weak out-of-plane interactions which are of the same order in all compounds, Ba₂CuTeO₆ and Ba₂CuWO₆ are even closer to ideal two-dimensional antiferromagnets than their strontium analogues. The transition temperature of Ba₂CuTeO₆ is not known, but we predict it to have the highest frustration index f = Θ_CW/T_N of these compounds and the Néel magnetic structure due to the very strong J₁ interaction. Magnetic excitations in Sr₂CuTeO₆ and Sr₂CuWO₆ have been observed at temperatures higher than 2T_N driven by the two-dimensional magnetic interactions.^9,10 The stronger in-plane J₁ and J₂ interactions of the barium phases indicate the excitations survive to even higher temperatures.

Table 2 Magnetic properties of Ba₂CuTeO₆, Sr₂CuTeO₆, Ba₂CuWO₆ and Sr₂CuWO₆. Exchange interactions J₁ and J₂ have been obtained by density functional theory (DFT; U = 8 eV), high-temperature series expansion fitting (HTSE) or by inelastic neutron scattering (INS). The data for Ba₂CuTeO₆ and Ba₂CuWO₆ are from this work unless specified otherwise

	Ba2CuTeO6	Sr2CuTeO6	Ba2CuWO6	Sr2CuWO6
a Predicted based on magnetic interactions.
J 1 (meV)	−20.22 (DFT)	−7.18 (INS)^9	−1.17 (DFT)	−2.45 (DFT)^10
	−16.54(3) (HTSE)		−0.2(9) (HTSE)	−1.2 (INS)^10
J 2 (meV)	0.23 (DFT)	−0.21 (INS)^9	−11.94 (DFT)	−8.83 (DFT)^10
	−0.04(3) (HTSE)		−10.0(1) (HTSE)	−9.5 (INS)^10
Θ CW (K)	−400^21	−80^2	−249^21	−165^2
T N (K)	—	29^11	28^20	24^13
f = ΘCW/TN	—	2.8	8.9	6.9
k	[1/2 1/2 kz]^a	[1/2 1/2 0]^11	[0 1/2 1/2]^20	[0 1/2 1/2]^12
Magnetic order	Néel^a	Néel	Columnar	Columnar

---

Since Ba₂CuTeO₆ has a dominant J₁ interaction and Ba₂CuWO₆ has a dominant J₂ interaction, we predict a spin-liquid-like state will form in the Ba₂Cu(Te_1−xW_x)O₆ solid solution similar to Sr₂Cu(Te_1−xW_x)O₆. In the Sr₂Cu(Te_1−xW_x)O₆ system the Néel order is destabilised already at x = 0.1, and spin-liquid-like state exist in the composition region x = 0.1–0.6. Columnar order is observed for x = 0.7–1. Since the J₁ interaction of Ba₂CuTeO₆ is so strong even compared to J₂ in Ba₂CuWO₆, we predict the Néel order remains more stable against W substitution. For the same reason, the columnar order near x = 1 is likely to be less stable in Ba₂Cu(Te_1−xW_x)O₆. The extent of the spin-liquid-like region depends also on disorder, and is difficult to predict just from the properties of the end phases. Finally, the stronger antiferromagnetic interactions in the barium phases indicate that the quantum disordered ground state will remain stable up to higher temperatures.

The previous discussion concerns a double perovskite Ba₂Cu(Te_1−xW_x)O₆ solid solution, which near x = 0 will require high-pressure synthesis to form. The ambient pressure form of Ba₂CuTeO₆ is triclinic with a tolerance factor higher than 1.03.²⁴ Therefore, a Ba₂Cu(Te_1−xW_x)O₆ solid solution prepared in ambient pressure will have a triclinic to tetragonal structural change at some composition. Triclinic Ba₂CuTeO₆ is a spin ladder system close to a quantum critical point,²⁵ and we propose Te-for-W substitution could drive the system from magnetic order to a spin singlet state.

In conclusion, we have investigated the magnetic interactions of the tetragonal double perovskites Ba₂CuTeO₆ and Ba₂CuWO₆ by DFT calculations and by HTSE fitting. Both compounds are well described by the frustrated square-lattice model as out-of-plane interactions are very weak. In Ba₂CuTeO₆ the antiferromagnetic nearest-neighbor J₁ interaction dominates (|J₂|/|J₁| < 0.02), whereas in Ba₂CuWO₆ the antiferromagnetic next-nearest neighbor interaction J₂ dominates (|J₁|/|J₂| < 0.12). The Ba₂Cu(Te,W)O₆ system is the second known FSL system where isostructural compounds have opposite magnetic interactions. This is driven by differences in orbital hybridisation of Te 5p/5s and W 5d with O 2p. A spin-liquid-like ground state is predicted for the Ba₂Cu(Te_1−xW_x)O₆ solid solution similar to the recent findings in the Sr₂Cu(Te_1−xW_x)O₆ system.

The authors wish to acknowledge CSC – IT Center for Science, Finland, for computational resources. The authors are thankful for access to the MPMS3 instrument in the Materials Characterisation Laboratory at the ISIS Muon and Neutron Source. OM, HM and EJC are thankful for funding by the Leverhulme Trust Research Project Grant RPG-2017-109. SV is thankful for the support of the Brazilian funding agencies CNPq (grants no. 150503/2016-4 and 152331/2016-6) and FAPERJ (grant no. 202842/2016). In addition, EBS acknowledges support from FAPERJ through several grants including Emeritus Professor fellow and CNPq for BPA and corresponding grants.

Conflicts of interest

There are no conflicts to declare.

Notes and references

1. L. Balents, Nature, 2010, 464, 199–208.
2. O. Mustonen, S. Vasala, E. Sadrollahi, K. P. Schmidt, C. Baines, H. C. Walker, I. Terasaki, F. J. Litterst, E. Baggio-Saitovitch and M. Karppinen, Nat. Commun., 2018, 9, 1085.
3. O. Mustonen, S. Vasala, K. P. Schmidt, E. Sadrollahi, H. C. Walker, I. Terasaki, F. J. Litterst, E. Baggio-Saitovitch and M. Karppinen, Phys. Rev. B, 2018, 98, 064411.
4. P. W. Anderson, Science, 1987, 235, 1196–1198.
5. F. Mezzacapo, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 045115.
6. H.-C. Jiang, H. Yao and L. Balents, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 024424.
7. W.-J. Hu, F. Becca, A. Parola and S. Sorella, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 88, 060402.
8. W.-Y. Liu, S. Dong, C. Wang, Y. Han, H. An, G.-C. Guo and L. He, Phys. Rev. B, 2018, 98, 241109.
9. P. Babkevich, V. M. Katukuri, B. Fåk, S. Rols, T. Fennell, D. Pajić, H. Tanaka, T. Pardini, R. R. P. Singh, A. Mitrushchenkov, O. V. Yazyev and H. M. Rønnow, Phys. Rev. Lett., 2016, 117, 237203.
10. H. C. Walker, O. Mustonen, S. Vasala, D. J. Voneshen, M. D. Le, D. T. Adroja and M. Karppinen, Phys. Rev. B, 2016, 94, 064411.
11. T. Koga, N. Kurita, M. Avdeev, S. Danilkin, T. J. Sato and H. Tanaka, Phys. Rev. B, 2016, 93, 054426.
12. S. Vasala, M. Avdeev, S. Danilkin, O. Chmaissem and M. Karppinen, J. Phys.: Condens. Matter, 2014, 26, 496001.
13. S. Vasala, H. Saadaoui, E. Morenzoni, O. Chmaissem, T. Chan, J. Chen, Y. Hsu, H. Yamauchi and M. Karppinen, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 89, 134419.
14. Y. Xu, S. Liu, N. Qu, Y. Cui, Q. Gao, R. Chen, J. Wang, F. Gao and X. Hao, J. Phys.: Condens. Matter, 2017, 29, 105801.
15. M. Zhu, D. Do, C. R. Dela Cruz, Z. Dun, H. D. Zhou, S. D. Mahanti and X. Ke, Phys. Rev. Lett., 2014, 113, 076406.
16. M. Watanabe, N. Kurita, H. Tanaka, W. Ueno, K. Matsui and T. Goto, Phys. Rev. B, 2018, 98, 054422.
17. K. Uematsu and H. Kawamura, Phys. Rev. B, 2018, 98, 134427.
18. L. Liu, H. Shao, Y.-C. Lin, W. Guo and A. W. Sandvik, Phys. Rev. X, 2018, 8, 041040.
19. Y. Todate, J. Phys. Soc. Jpn., 2001, 70, 337.
20. Y. Todate, W. Higemoto, K. Nishiyama and K. Hirota, J. Phys. Chem. Solids, 2007, 68, 2107–2110.
21. D. Iwanaga, Y. Inaguma and M. Itoh, J. Solid State Chem., 1999, 147, 291–295.
22. H. Rosner, R. R. P. Singh, W. H. Zheng, J. Oitmaa and W. E. Pickett, Phys. Rev. B: Condens. Matter Mater. Phys., 2003, 67, 014416.
23. A. A. Tsirlin, R. Nath, A. M. Abakumov, Y. Furukawa, D. C. Johnston, M. Hemmida, H. A. Krug Von Nidda, A. Loidl, C. Geibel and H. Rosner, Phys. Rev. B: Condens. Matter Mater. Phys., 2011, 84, 014429.
24. G. N. Rao, R. Sankar, A. Singh, I. P. Muthuselvam, W. T. Chen, V. N. Singh, G.-Y. Guo and F. C. Chou, Phys. Rev. B, 2016, 93, 104401.
25. D. Macdougal, A. S. Gibbs, T. Ying, S. Wessel, H. C. Walker, D. Voneshen, F. Mila, H. Takagi and R. Coldea, Phys. Rev. B, 2018, 98, 174410.

---

Footnote

† Electronic supplementary information (ESI) available: Supporting computational and experimental details, figures and tables. See DOI: 10.1039/c8cc09479a

---