Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Correlated shapeshifting and configurational isomerization†
Burhan A.
Hussein
a,
William
Maturi
ab,
Mary Kate
Rylands
a,
Aisha N.
Bismillah
 ab,
Yuzhen
Wen
ab,
Juan A.
Aguilar
ab,
Yuzhen
Wen
ab,
Juan A.
Aguilar
 a,
Rabia
Ayub
a,
Rabia
Ayub
 b,
Conor D.
Rankine
b,
Conor D.
Rankine
 b and
Paul R.
McGonigal
b and
Paul R.
McGonigal
 *ab
*ab
aDepartment of Chemistry, Durham University, Lower Mountjoy, Stockton Road, Durham, DH1 3LE, UK. E-mail: paul.mcgonigal@york.ac.uk
bDepartment of Chemistry, University of York, Heslington, York, YO10 5DD, UK
First published on 23rd August 2024
Abstract
Herein we demonstrate that the rapid ‘shapeshifting’ constitutional isomerization of a substituted bullvalene is influenced by the E-to-Z configurational isomerization of a remote carbamate group, giving rise to correlated motion. We find that, while the E-configurational isomer of a bulky carbamate favors the β-bullvalene constitutional isomer, a noncovalent bonding interaction within the Z-carbamate tips the equilibrium toward the γ-bullvalene form. Using DFT modelling and NMR spectroscopy, this long-range interaction is identified as being between the bullvalene core and a pendant phenyl group connected to the carbamate. Coupling the constitutional changes of a bullvalene to a reciprocal configurational isomerization through a long-range interaction in this way will allow shapeshifting rearrangements to be exploited as part of collective motion in extended structures.
Introduction
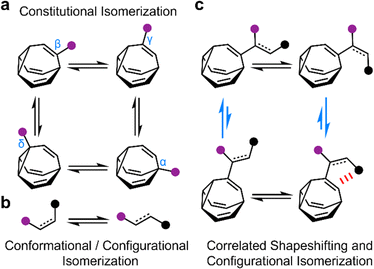
The rapid, successive Cope rearrangements of bullvalenes1 produce a series (Fig. 1a) of constitutional isomers. Recent advances in the synthesis of substituted derivatives,2 alongside the development of related fluxional cages,3 have led to renewed interest in exploiting these ‘shapeshifting’ structures as part of functional molecules and materials. Part of the appeal of using bullvalene derivatives is that they juxtapose the rigidity and well-defined bond angles1e that are typical of cage-like structures with the rapid structural dynamics that are commonly only found in flexible molecules. They exhibit ‘rigid dynamics’ at the single-molecule level.4 Accordingly, investigations have been reported into bullvalene-containing fluorophores,5 antibiotics,6 small-molecule receptors,7 transition metal complexes,8 rigid-rod polymers,9 and single-molecule junctions.10By contrast, most organic structures, particularly those containing rotatable single bonds, rapidly sample stereoisomeric structures through changes in bond angles and dihedral angles.11 The resulting isomerization (Fig. 1b) is not only influenced by local electronic and steric factors, but also by long-range interactions. Bond rotations of groups that are separated by several covalent bonds but are close together in space can, therefore, become correlated with one another,12i.e., the isomerization or motion of one part of the molecule is coupled to a reciprocal conformational or configurational change in another part.
To exploit the shapeshifting rearrangements of bullvalenes in larger collective motions of extended structures,12d–f it is necessary to understand how their fluxional Cope rearrangements are influenced (Fig. 1c) by isomerization of surrounding groups. Here, we report the isomeric distribution of carbamate-functionalized bullvalenes 1 (Scheme 1) and demonstrate that, even in this structurally simple case, conformational changes can become correlated to shapeshifting isomerization. For one of the compounds (benzhydryl derivative 1b), we find that while the E-carbamate is most stable as its β-substituted bullvalene isomer, bond rotation to the Z-carbamate biases the bullvalene unit towards its γ-substituted form instead, i.e., the energetics of bullvalene isomerization and carbamate rotation are coupled to one another. We show that through-space interactions subtly alter the energetics of the dynamic system. As part of this investigation, we have also assessed the extent to which this isomer distribution can be accurately modelled by comparing the calculated energies of the lowest energy conformers, as opposed to analyzing the full conformational landscape.
Results and discussion
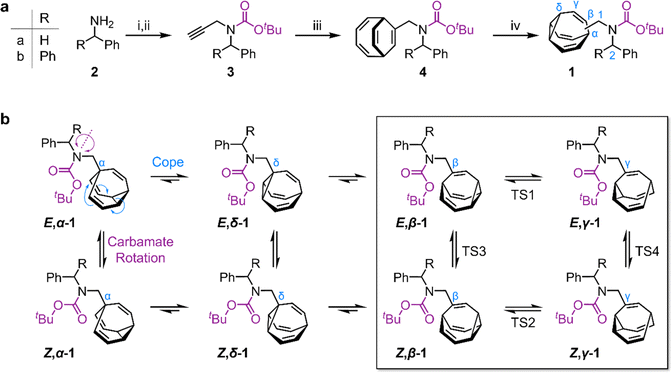
We targeted (Scheme 1) tertiary carbamates 1a and 1b derived from benzylamine 2a and benzhydrylamine 2b, respectively. The partial double bond character of the carbamate C–N bond (c.f., tertiary amide C–N bonds13) gives rise to E-to-Z configurational isomerization that can be slowed down and observed by dynamic NMR (DNMR) spectroscopy at low temperatures, which we reasoned would allow us to experimentally measure distributions of the configurational and constitutional isomers of 1a and 1b.Starting from 2, we performed (Scheme 1a) a sequence of carbamate formation, alkylation with propargyl bromide, and cobalt(I)-catalyzed [6 + 2] cycloaddition with cyclooctatetraene2c,14 to produce bicyclo[4.2.2]deca-2,4,7,9-tetraene intermediates 4. A final photochemical di-π-methane rearrangement step15 using thioxanthone2e as a photosensitizer gave rise to the target bullvalenes 1.
There are four possible constitutional isomers of 1 (Scheme 1b), which we label as α–δ according to the attachment point of the carbamate substituent to the bullvalene cage.1e Consecutive Cope rearrangements from the α-isomer lead to the δ-, β-, then γ-isomers in a reversible linear sequence. Alternatively, rotation around the carbamate C–N bond interconverts the E- and Z-configurational isomers. In combination, these two pathways give rise to a set of eight isomers (Scheme 1b).
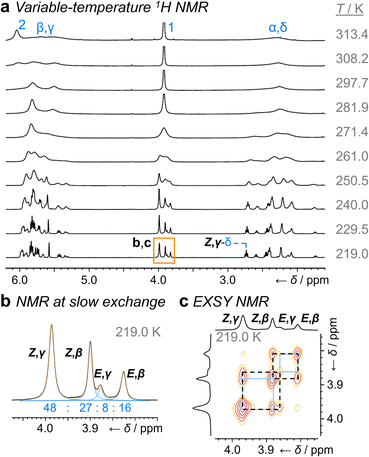
1H NMR Spectroscopic analysis of the benzyl derivative 1a in CDCl3 at 313 K (Fig. 2a) shows two broad resonances corresponding to the two sets of methylene protons, H1 and H2. Rapid chemical exchange at this temperature averages out the contributions from the different isomers to these two methylene resonances and causes the signals arising from the bullvalene methine groups, Hα–Hδ, to broaden and partially merge. At 219 K, the reduced rates of Cope rearrangements and carbamate rotation allow signals from the different species to be resolved.
 | ||
| Fig. 2 1H DNMR (499 MHz, CDCl3) and crystallographic analyses of 1a. (a) Partial 1H NMR spectra at temperatures ranging from 219 K to 313 K. Signals are assigned using the labels in Scheme 1. Regions in orange boxes correspond to those shown in panels b, d, and e. (b) Line fitting was used to deconvolute the H1 signals at 219 K. An overlay of the experimentally measured (black) and simulated (orange) traces is shown, including the integrals of the four simulated peaks (blue). (c) X-ray crystal structure of E,γ-1a. (d) Partial EXSY NMR spectrum (499 MHz, CDCl3, tm = 500 ms). Blue boxes indicate cross peaks arising from Cope rearrangements. (e) Line-shape analysis for the H2 signal at 308 K, comparing the experimentally measured (exp.) and simulated (sim.) line shapes. See Table S1† for simulation parameters. | ||
Although there are eight possible isomers, the α- and δ-forms of mono-substituted bullvalenes are usually several kJ mol−1 higher in energy than the olefin-substituted β- and γ-forms,16 so they are not present in sufficient concentrations to be observed. β-Substitution is usually preferred over γ-substitution by ∼1–2 kJ mol−1.2c In keeping with this expectation, line fitting of the H1 peaks indicates (Fig. 1b) that four isomers are present in a 45![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 23
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 21
21![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
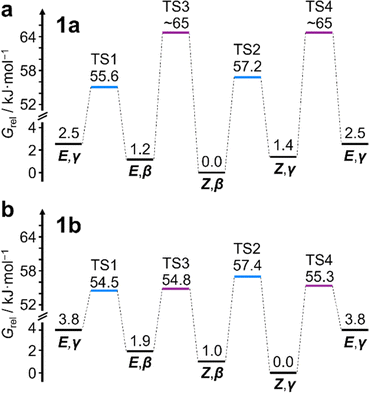
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 11 ratio, which can be assigned to Z,β-1a, E,β-1a, Z,γ-1a, and E,γ-1a, respectively, using 2D NMR (Fig. S15–S22†). Shape-selective crystallization‡2e,17 from this mixture yields E,γ-1a (the least populated of the four isomers in solution) as the sole isomer in the solid state (Fig. 2c). In energetic terms (Table 1), the solution-phase population corresponds to small Gibbs energy biases ΔGexp of ∼1.3 kJ mol−1 toward both β-isomers over their γ-substituted counterparts, and ΔGexp of ∼1 kJ mol−1 for each Z-carbamate over its corresponding E-carbamate. Therefore, for 1a, there is no evidence that the E/Z-state of the molecule substantially changes the energetics of β/γ-isomerization, or vice versa. The two isomerization processes do not appear to be correlated.
11 ratio, which can be assigned to Z,β-1a, E,β-1a, Z,γ-1a, and E,γ-1a, respectively, using 2D NMR (Fig. S15–S22†). Shape-selective crystallization‡2e,17 from this mixture yields E,γ-1a (the least populated of the four isomers in solution) as the sole isomer in the solid state (Fig. 2c). In energetic terms (Table 1), the solution-phase population corresponds to small Gibbs energy biases ΔGexp of ∼1.3 kJ mol−1 toward both β-isomers over their γ-substituted counterparts, and ΔGexp of ∼1 kJ mol−1 for each Z-carbamate over its corresponding E-carbamate. Therefore, for 1a, there is no evidence that the E/Z-state of the molecule substantially changes the energetics of β/γ-isomerization, or vice versa. The two isomerization processes do not appear to be correlated.
| State | 1a, p/% | 1a, ΔG/kJ mol−1 | 1b, p/% | 1b, ΔG/kJ mol−1 | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| p exp | p calc1 | p calcG | ΔGexpa | ΔGcalc1f | ΔGcalcGh | p exp | p calc1 | p calcG | ΔGexpa | ΔGcalc1f | ΔGcalcGh | |
| a Measured at 219 K in CDCl3. b The population of α- and δ-isomers is below the detection limit of the equipment used to acquire 1H NMR spectra. c Population calculated as a Boltzmann distribution based on the DFT calculated energies of the most stable conformer for each constitutional and configurational isomer (ΔGcalc1). d Population calculated as a global Boltzmann distribution by summing the populations of all accessible conformers calculated for each constitutional and configurational isomer (ΔGcalcG). e Apparent Gibbs energy barrier for carbamate rotation (weighted average of TS3 and TS4) at 308 K. f Relative Gibbs energies of only the most stable conformer for each isomer calculated at 219 K using PBE0-D3/def2-SV(P).20i–k,p g Transition states were not modelled. h Effective relative Gibbs energies based on the DFT calculated global Boltzmann distribution (pcalcG) for the purpose of comparison with ΔGexp. | ||||||||||||
| E,α | —b | <0.1 | <0.1 | —b | 25.5 | 25.4 | —b | <0.1 | <0.1 | —b | 34.5 | 34.0 |
| Z,α | —b | <0.1 | <0.1 | —b | 23.1 | 23.2 | —b | <0.1 | <0.1 | —b | 26.5 | 26.8 |
| E,β | 23 | 18 | 22 | 1.2 | 1.7 | 1.4 | 16 | 24 | 23 | 1.9 | 1.4 | 1.2 |
| Z,β | 45 | 47 | 46 | 0.0 | 0.0 | 1.0 | 27 | 18 | 28 | 1.0 | 1.5 | 0.8 |
| E,γ | 11 | 10 | 13 | 2.5 | 2.8 | 2.3 | 8 | 6 | 5 | 3.2 | 3.8 | 3.8 |
| Z,γ | 21 | 14 | 18 | 1.4 | 2.2 | 1.7 | 48 | 51 | 44 | 0.0 | 0.0 | 0.0 |
| E,δ | —b | <1 | <1 | —b | 8.7 | 8.2 | —b | <0.1 | <0.1 | —b | 14.8 | 14.1 |
| Z,δ | —b | <1 | 1 | —b | 7.2 | 6.8 | —b | <1 | <1 | —b | 9.5 | 8.8 |
| TS1 | — | — | — | 55.6 | —g | —g | — | — | — | 54.5 | —g | —g |
| TS2 | — | — | — | 57.2 | —g | —g | — | — | — | 57.4 | —g | —g |
| TS3 | — | — | — | 64.9e | —g | —g | — | — | — | 54.8 | —g | —g |
| TS4 | — | — | — | — | —g | —g | — | — | — | 55.3 | —g | —g |
This system does, however, provide an unusual case of reversible covalent changes occurring in a molecule more rapidly than its bond rotation, i.e., the carbamate isomerization of 1a proceeds at a slower rate than its Cope rearrangement. Using 1H–1H Exchange NMR Spectroscopy18 (EXSY) at 219 K (Fig. 2d), we found that the Cope rearrangements pass through transition states (TS1 and TS2, Scheme 1b) that are ∼55–57 kJ mol−1 above the lowest energy isomer, Z,β-1a, which is typical19 for bullvalene constitutional isomerization. There are no cross peaks visible between E- and Z-isomers at this temperature because of the slow carbamate rotation rate. At the higher temperature of 308 K, 1H NMR line-shape analysis (Fig. 2e) gives an averaged rate of carbamate rotation, kEZ, of 62 s−1, indicating that TS3 and TS4 lie almost 10 kJ mol−1 higher than TS1 at ΔGexp of ∼65 kJ mol−1 relative to Z,β-1a (Table 1).
Unlike 1a, the increased steric crowding present in the benzhydryl derivative 1b leads to correlated isomerization. The additional phenyl ring of 1b experiences long-range interactions with the bullvalene that influence the solution-phase isomerization equilibria. There are some key clues to this behavior in the variable-temperature NMR spectra (Fig. 3a). Crucially, the distribution of H1 methylene resonances (Fig. 3b) has been altered substantially relative to 1a. The Z,β-1b, E,β-1b, Z,γ-1b, and E,γ-1b isomers are present in a 27![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 16
16![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 48
48![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 8 ratio, as assigned by 2D NMR (Fig. S15–S22†). Therefore, the Z,γ-isomer is the most favored form of 1b, despite being only the third most populated isomer of 1a. The changes in relative Gibbs energy (Grel) between the 1a and 1b isomers is summarized graphically as a potential energy diagram in Fig. 4. A structural change from a hydrogen substituent to a phenyl group at a remote site has overridden the inherent preference2c,16 for the bullvalene β-isomer in the reaction network, but only when the carbamate is in its Z-form (Fig. 5a). The benzhydryl carbamate of 1b also rotates at a faster rate than the benzyl carbamate of 1a, exhibiting near-identical energy barriers of ∼54–58 kJ mol−1 for the four isomerization processes (Table 1).
8 ratio, as assigned by 2D NMR (Fig. S15–S22†). Therefore, the Z,γ-isomer is the most favored form of 1b, despite being only the third most populated isomer of 1a. The changes in relative Gibbs energy (Grel) between the 1a and 1b isomers is summarized graphically as a potential energy diagram in Fig. 4. A structural change from a hydrogen substituent to a phenyl group at a remote site has overridden the inherent preference2c,16 for the bullvalene β-isomer in the reaction network, but only when the carbamate is in its Z-form (Fig. 5a). The benzhydryl carbamate of 1b also rotates at a faster rate than the benzyl carbamate of 1a, exhibiting near-identical energy barriers of ∼54–58 kJ mol−1 for the four isomerization processes (Table 1).
 | ||
| Fig. 3 1H DNMR analysis (499 MHz, CDCl3) of 1b. (a) Partial 1H NMR spectra at temperatures ranging from 219 K to 313 K. Signals are assigned using the labels in Scheme 1. The region in an orange box corresponds to that shown in panels b and c. (b) Line fitting was used to deconvolute the H1 signals at 219 K. An overlay of the experimentally measured (black) and simulated (orange) traces is shown, including the integrals of the four simulated peaks (blue). (c) Partial EXSY NMR spectrum (499 MHz, CDCl3, tm = 500 ms). Blue boxes indicate cross peaks arising from Cope rearrangements. Dashed black boxes indicate cross peaks arising from carbamate rotation. | ||
 | ||
| Fig. 5 (a) Network diagrams for the isomerization of 1a and 1b showing the experimentally measured populations (pexp) of the β- and γ-isomers at 219 K as filled grey circles and the calculated populations (pcalG) as hollow orange circles. According to DFT calculations, the α- and δ-isomers are present in <1%. Blue arrows point to the major species of each Cope rearrangement process, highlighting that the preference for the β- and γ-bullvalene isomers is correlated to the E-to-Z isomerization of 1b. (b) NCI plots [LC-PBE/def2-TZVP]22 of Z,γ-1b and Z,β-1b showing the increased Ph–BV interaction in Z,γ-1b. | ||
To understand the unusual constitutional isomer distribution of 1b, we carried out DFT modelling. We generated all the constitutional isomers of 1a and 1b and carried out distance-geometry-based generation of their configurational and conformational isomers before optimizing the geometries at the PBE0-D3/def2-SV(P) level of theory.1e,20 As there are several rotatable single bonds in the structure of 1, each of the isomers observed by low-temperature NMR itself represents a rapidly interconverting population of conformers. The relative energies of all the isomers are tabulated in Tables S3 and S4.† Cartesian coordinates are available in the ESI.† To take one representative example, there are six conformers of Z,β-1a spanning a range of ∼16 kJ mol−1 in energy, of which the two lowest energy structures are within just 2.5 kJ mol−1 of one another.
Despite there being numerous different structures contributing to the overall energetics of E-to-Z and β-to-γ isomerization, the experimentally observed populations, pexp, can be approximated reasonably well by analyzing just the lowest energy conformers (Table 1). For 1a, the calculated energies, ΔGcalc1, of the lowest energy conformer for each of the four observable isomers gives a Boltzmann distribution, pcalc1, that matches closely to pexp. For 1b, however, this simplified approach incorrectly predicts that more of E,β-1b is present than Z,β-1b. Instead, it is necessary to perform a global Boltzmann population analysis (giving pcalcG) that takes into account all the accessible conformers in order to reproduce the experimentally observed hierarchy of isomers. This observation indicates that for bullvalene derivatives with several rotatable single bonds, accurate modelling of the shapeshifting rearrangements requires consideration of the full energetic landscape of accessible conformers.
Noncovalent interaction (NCI) plots21 generated using the DFT-optimized geometries reveal (Fig. 5b and S26–S29†) the interactions that are responsible for the correlated isomerization of 1b. In addition to several close contacts between the crowded tert-butyl, carbonyl, and phenyl groups, which are present in all of the isomers, the lowest energy conformer of Z,γ-1b shows evidence of substantial interaction between an ortho-hydrogen of one phenyl ring with the bullvalene cyclopropyl ring (Ph–BV interaction, Fig. 5b). This long-range interaction is less significant in the NCI analyses of the other 1b isomers (Fig. S26–S29†), such as Z,β-1b (Fig. 5b). Experimental evidence for the interaction in Z,γ-1b is apparent in its low-temperature 1H NMR spectrum (Fig. 3a) as its Hδ resonance is shifted downfield relative to the Hδ signals of the other isomers of 1b, which is indicative of deshielding caused by the aromatic ring current. The NOESY NMR spectrum (Fig. S23†) also confirms Hδ undergoes through-space interaction with a phenyl group. Although this Ph–BV interaction appears to be a relatively weak van der Waals contact, the combination of it together with other subtle differences in the attractive and repulsive noncovalent bonding interactions present in 1b is evidently sufficient to provide enough of an energetic bias to overcome the ∼1–2 kJ mol−1 preference for the β-isomer.
Conclusions
In summary, the shapeshifting constitutional rearrangements of even structurally simple bullvalenes can be influenced by a complementary, remote isomerization process. Given that the energetic differences between bullvalene isomers are often as little as a few kJ mol−1, relatively subtle noncovalent bonding interactions, including weak van der Waals contacts, are sufficient to reshuffle the proportions of each isomer. In the system presented here, the introduction of an additional phenyl ring to the structure of 1a causes the Z,γ-isomer to change from being the third most populous to being the major species of 1b as a result of a Ph–BV interaction. Programming in switchable long-range interactions may allow for control to be exercised over the large number of constitutional isomers that arise in multi-substituted bullvalene derivatives.Data availability
Experimental synthetic procedures, characterization data and theoretical calculation results are available in the ESI.†Author contributions
Conceptualization: BAH, PRM. Investigation: BAH, WM, MKR, ANB, JAA, CR. Writing: BAH, WM, ANB, YW, RA, PRM.Conflicts of interest
There are no conflicts to declare.Acknowledgements
B. A. H. acknowledges an EPSRC SOFI CDT Studentship (EP/L015536/1) and an NSERC Scholarship. W. M. (EP/T518001/1), R. A (EP/X021564/1) and P. R. M (EP/V047817/2, EP/V040049/2) thank the EPSRC for funding. A. N. B. and P. R. M. acknowledge a Leverhulme Trust Research Project Grant (RPG-2020-218). Y. W. thanks the Chinese Scholarship Council. We thank Dr Dmitry Yufit for performing X-ray crystallography. We acknowledge the University of York High Performance Computing service, Viking.Notes and references
-
(a) W. v. E. Doering and W. R. Roth, Tetrahedron, 1963, 19, 715–737 CrossRef CAS
; (b) G. Schröder, Angew. Chem., Int. Ed. Engl., 1963, 2, 481–482 CrossRef
; (c) S. Ferrer and A. Echavarren, Synthesis, 2019, 51, 1037–1048 CrossRef CAS
; (d) A. N. Bismillah, B. M. Chapin, B. A. Hussein and P. R. McGonigal, Chem. Sci., 2020, 11, 324–332 RSC
; (e) R. A. Ives, W. Maturi, M. T. Gill, C. D. Rankine and P. R. McGonigal, Chem. Sci., 2024 10.1039/D4SC03700F
.
-
(a) A. R. Lippert, J. Kaeobamrung and J. W. Bode, J. Am. Chem. Soc., 2006, 128, 14738–14739 CrossRef CAS PubMed
; (b) S. Ferrer and A. M. Echavarren, Angew. Chem., Int. Ed., 2016, 55, 11178–11182 CrossRef CAS PubMed
; (c) O. Yahiaoui, L. F. Pašteka, B. Judeel and T. Fallon, Angew. Chem., Int. Ed., 2018, 57, 2570–2574 CrossRef CAS PubMed
; (d) O. Yahiaoui, L. F. Pašteka, C. J. Blake, C. G. Newton and T. Fallon, Org. Lett., 2019, 21, 9574–9578 CrossRef CAS
; (e) H. D. Patel, T. H. Tran, C. J. Sumby, L. F. Pašteka and T. Fallon, J. Am. Chem. Soc., 2020, 142, 3680–3685 CrossRef CAS PubMed
; (f) A. Sanchez, V. M. Gonzalez, J. Sakamoto, A. Gurajapu and T. J. Maimone, J. Am. Chem. Soc., 2024, 146, 17573–17579 CrossRef CAS PubMed
.
-
(a) P. R. McGonigal, C. de León, Y. Wang, A. Homs, C. R. Solorio-Alvarado and A. M. Echavarren, Angew. Chem., Int. Ed., 2012, 51, 13093–13096 CrossRef CAS
; (b) X. Wang, O. Shyshov, M. Hanževački, C. M. Jäger and M. von Delius, J. Am. Chem. Soc., 2019, 141, 8868–8876 CrossRef CAS
; (c) M. Sanz-Novo, M. Mato, Í. León, A. M. Echavarren and J. L. Alonso, Angew. Chem., Int. Ed., 2022, 61, e202117045 CrossRef CAS
; (d) A. Sanchez and T. J. Maimone, J. Am. Chem. Soc., 2022, 144, 7594–7599 CrossRef CAS PubMed
; (e) A. Sanchez, A. Gurajapu, W. Guo, W.-Y. Kong, C. J. Laconsay, N. S. Settineri, D. J. Tantillo and T. J. Maimone, J. Am. Chem. Soc., 2023, 145, 13452–13461 CrossRef CAS
; (f) A. N. Bismillah, T. G. Johnson, B. A. Hussein, A. T. Turley, P. K. Saha, H. C. Wong, J. A. Aguilar, D. S. Yufit and P. R. McGonigal, Nat. Chem., 2023, 15, 615–624 CrossRef CAS
.
-
(a) H. Deng, M. A. Olson, J. F. Stoddart and O. M. Yaghi, Nat. Chem., 2010, 2, 439–443 CrossRef CAS
; (b) S. Krause and J. V. Milić, Commun. Chem., 2023, 6, 151 CrossRef
; (c) P. K. Saha, T. Tran Ngoc, P. R. McGonigal and J. F. Teichert, Nat. Synth., 2024, 3, 684–697 CrossRef
.
- C. Dohmen, H. Ihmels and T. Paululat, Eur. J. Org Chem., 2022, 2022, e202201172 CrossRef CAS
.
- A. Ottonello, J. A. Wyllie, O. Yahiaoui, S. Sun, R. A. Koelln, J. A. Homer, R. M. Johnson, E. Murray, P. Williams, J. R. Bolla, C. V. Robinson, T. Fallon, T. P. Soares da Costa and J. E. Moses, Proc. Natl. Acad. Sci. U. S. A., 2023, 120, e2208737120 CrossRef CAS
.
-
(a) K. K. Larson, M. He, J. F. Teichert, A. Naganawa and J. W. Bode, Chem. Sci., 2012, 3, 1825 RSC
; (b) J. F. Teichert, D. Mazunin and J. W. Bode, J. Am. Chem. Soc., 2013, 135, 11314–11321 CrossRef CAS
.
-
(a) A. Birve, H. D. Patel, J. R. Price, W. M. Bloch and T. Fallon, Angew. Chem., Int. Ed., 2021, 61, e202115468 CrossRef
; (b) C. Dohmen, T. Paululat and H. Ihmels, Chem.–Eur. J., 2024, 30, e202304311 CrossRef CAS
.
-
(a) M. N. Pomfret, P. B. Sun, Z. Huang, A. C. Freund, T. Miyoshi and M. R. Golder, Angew. Chem., Int. Ed., 2023, 62, e202301695 CrossRef CAS
; (b) P. B. Sun, M. N. Pomfret, M. J. Elardo, A. Suresh, Á. Rentería-Gómez, R. F. Lalisse, S. Keating, C. Chen, Shayna, L. Hilburg, P. Chakma, Y. Wu, R. C. Bell, S. J. Rowan and M. R. Golder, J. Am. Chem. Soc., 2024, 146, 19229–19238 CrossRef CAS
.
- J. R. Reimers, T. Li, A. P. Birvé, L. Yang, A. C. Aragonès, T. Fallon, D. S. Kosov and N. Darwish, Nat. Commun., 2023, 14, 6089 CrossRef CAS
.
- A. Mazzanti and D. Casarini, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2012, 2, 613–641 CAS
.
-
(a) K. Mislow, Acc. Chem. Res., 1976, 9, 26–33 CrossRef CAS
; (b) M. Oki, Angew. Chem., Int. Ed. Engl., 1976, 15, 87–93 CrossRef
; (c) Z. Rappoport and S. E. Biali, Acc. Chem. Res., 1997, 30, 307–314 CrossRef CAS
; (d) S. Erbas-Cakmak, D. A. Leigh, C. T. McTernan and A. L. Nussbaumer, Chem. Rev., 2015, 115, 10081–10206 CrossRef CAS PubMed
; (e) D. Dattler, G. Fuks, J. Heiser, E. Moulin, A. Perrot, X. Yao and N. Giuseppone, Chem. Rev., 2020, 120, 310–433 CrossRef CAS
; (f) S. Corra, C. de Vet, M. Baroncini, A. Credi and S. Silvi, Chem, 2021, 7, 2137–2150 CrossRef CAS PubMed
; (g) C. L. F. Van Beek and B. L. Feringa, J. Am. Chem. Soc., 2024, 146, 5634–5642 CrossRef CAS PubMed
.
-
(a) E. R. Johnston, R. Fortt and J. C. Barborak, Magn. Reson. Chem., 2000, 38, 932–936 CrossRef CAS
; (b) J. Clayden and J. H. Pink, Angew. Chem., Int. Ed., 1998, 37, 1937–1939 CrossRef CAS
; (c) R. A. Bragg and J. Clayden, Org. Lett., 2000, 2, 3351–3354 CrossRef CAS PubMed
; (d) R. A. Bragg, J. Clayden, G. A. Morris and J. H. Pink, Chem.–Eur. J., 2002, 8, 1279–1289 CrossRef CAS PubMed
.
- M. Achard, M. Mosrin, A. Tenaglia and G. Buono, J. Org. Chem., 2006, 71, 2907–2910 CrossRef CAS PubMed
.
-
(a) M. Jones and L. T. Scott, J. Am. Chem. Soc., 1967, 89, 150–151 CrossRef CAS
; (b) M. Jones, S. D. Reich and L. T. Scott, J. Am. Chem. Soc., 1970, 92, 3118–3126 CrossRef CAS
.
- J. F. M. Oth, R. Merényi, J. Nielsen and G. Schröder, Chem. Ber., 1965, 98, 3385–3400 CrossRef CAS
.
-
(a) A. N. Bismillah, J. Sturala, B. M. Chapin, D. S. Yufit, P. Hodgkinson and P. R. McGonigal, Chem. Sci., 2018, 9, 8631–8636 RSC
; (b) J. Nyman and G. M. Day, CrystEngComm, 2015, 17, 5154–5165 RSC
.
- K. Nikitin and R. O'Gara, Chem.–Eur. J., 2019, 25, 4551–4589 CrossRef CAS
.
- R. Merényi, J. F. M. Oth and G. Schröder, Chem. Ber., 1964, 97, 3150–3161 CrossRef
.
-
(a)
bullviso, 2024, https://gitlab.com/conorrankine/bullviso;
(b) S. Riniker and G. A. Landrum, J. Chem. Inf. Model., 2015, 55, 2562–2574 CrossRef CAS PubMed
; (c) S. Wang, J. Witek, G. A. Landrum and S. Riniker, J. Chem. Inf. Model., 2020, 60, 2044–2058 CrossRef CAS PubMed
; (d) RDKit: Open-Source Cheminformatics. https://rdkit.org; (e) RDKit, 2023, https://github.com/rdkit/rdkit; (f) S. Grimme, C. Bannwarth and P. Shushkov, J. Chem. Theory Comput., 2017, 13, 1989–2009 CrossRef CAS PubMed
; (g) C. Bannwarth, S. Ehlert and S. Grimme, J. Chem. Theory Comput., 2019, 15, 1652–1671 CrossRef CAS PubMed
; (h) C. Bannwarth, E. Caldeweyher, S. Ehlert, A. Hansen, P. Pracht, J. Seibert, S. Spicher and S. Grimme, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2020, 11, e01493 Search PubMed
; (i) J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868 CrossRef CAS PubMed
; (j) J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1997, 78, 1396 CrossRef CAS
; (k) C. Adamo and V. Barone, J. Chem. Phys., 1999, 110, 6158–6169 CrossRef CAS
; (l) S. Grimme, J. Antony, S. Ehrlich and H. Krieg, J. Chem. Phys., 2010, 132, 154104 CrossRef PubMed
; (m) F. Neese, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2012, 2, 73–78 CAS
; (n) F. Neese, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2017, 8, e1327 Search PubMed
; (o) F. Neese, F. Wennmohs, U. Becker and C. Riplinger, J. Chem. Phys., 2020, 152, 224108 CrossRef CAS PubMed
; (p) F. Weigend and R. Ahlrichs, Phys. Chem. Chem. Phys., 2005, 7, 3297–3305 RSC
; (q) F. Weigend, Phys. Chem. Chem. Phys., 2006, 8, 1057–1065 RSC
.
-
(a) H. Iikura, T. Tsuneda, T. Yanai and K. Hirao, J. Chem. Phys., 2001, 115, 3540–3544 CrossRef CAS
; (b) A. Najibi, M. Casanova-Paez and L. Goerigk, J. Phys. Chem. A, 2021, 125, 4026–4035 CrossRef CAS PubMed
.
-
(a) E. R. Johnson, S. Keinan, P. Mori-Sánchez, J. Contreras-García, A. J. Cohen and W. Yang, J. Am. Chem. Soc., 2010, 132, 6498–6506 CrossRef CAS
; (b) J. Contreras-García, E. R. Johnson, S. Keinan, R. Chaudret, J.-P. Piquemal, D. N. Beratan and W. Yang, J. Chem. Theory Comput., 2011, 7, 625–632 CrossRef
.
Footnotes |
| † Electronic supplementary information (ESI) available. CCDC 2294194 (E,γ-1a). For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d4sc03699a |
| ‡ The energetics of crystal packing can outweigh the small solution-phase energetic bias towards certain isomers within fluxional mixtures.2e,17 |
| This journal is © The Royal Society of Chemistry 2024 |