Defects in the new oxide-fluoride Ba₂PdO₂F₂: the search for fluoride needles in an oxide haystack

Abstract

The defect chemistry and fluorine insertion properties of the new Ba_2−xSr_xPdO₂F₂ system have been investigated using atomistic simulation techniques. The interatomic potential model produces good agreement between simulated and observed structures for the “parent” oxides Ba₂PdO₃ and Sr₂PdO₃, and the oxide-fluorides Ba₂PdO₂F₂ and BaSrPdO₂F₂. The perfect lattice simulations confirm the most favourable structure type is the T′ (Nd₂CuO₄) structure for the oxide-fluoride phases comprised of square planar Pd, which accords with recent EXAFS studies. The fluorination reaction in the precursor oxide Ba₂PdO₃, involving substitution of two fluorine ions for one oxygen ion, is highly favourable. The formation of fluorine interstitials and holes in Ba₂PdO₂F₂ is an unfavourable process in accord with the observed resistance to excess fluorine, and in contrast to the related cuprate superconductor Sr₂CuO₂F_2+δ.

---

1. Introduction

Fluorination reactions at low temperature have become useful chemical routes in the search for novel materials with interesting properties. The first palladium oxide-fluorides have recently been synthesised by low temperature fluorination of the alkaline-earth palladates, Ba_2−xSr_xPdO₃.¹ Powder X-ray diffraction, EXAFS and magnetic measurements suggest that the fluorinated materials have the general formula Ba_2−xSr_xPdO₂F₂ (0 ≤ x ≤ 1.5) and that they adopt the T′-type (Nd₂CuO₄) structure shown in Fig. 1; in the T′ structure, palladium occupies a perovskite-like layer and is coordinated by four oxygens in a square-planar fashion.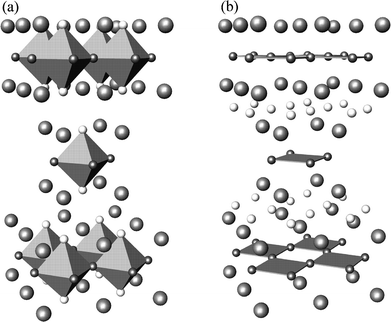

Fig. 1 Crystal structure types of Ba₂PdO₂F₂: (a) T-type, (b) T′-type. The polyhedra indicate the octahedral and square planar coordination of Pd in T and T′ respectively; small grey spheres (O); white spheres (F); large grey spheres (Ba).

This work follows the important discovery of high temperature superconductivity in the copper oxide-fluoride Sr₂CuO₂F_2+δ,² which has stimulated considerable interest in the materials chemistry of ternary oxide-fluorides.^3,4 The Sr₂CuO₂F_2+δ phase displays the related T-type structure (La₂CuO₄) in which the Cu is octahedrally coordinated by four oxygen ions and two fluoride ions at equatorial and apical sites respectively (Fig. 1). Low temperature fluorination is thought to induce an interesting structural rearrangement in the “precursor” oxide Sr₂CuO₃, where one oxygen ion is substituted by two fluoride ions.² The parent Pd-based oxides A₂PdO₃ (A = Ba, Sr) are isostructural with the cuprates A₂CuO₃ (A = Ca, Sr).

It is now well established that defect-related and fluorine insertion processes play a crucial role in determining the properties of these compounds. A key difference between palladium and copper oxide-fluorides is believed to be related to the fluoride stoichiometry in which Sr₂CuO₂F_2+δ contains excess fluorine (δ) at interstitial defect sites, whereas Ba₂PdO₂F₂ does not show such behaviour. However, it is acknowledged that it can be difficult to determine the precise F⁻ positions in these structures since neither X-ray nor neutron diffraction techniques reliably distinguish between fluoride and oxide ions, thus motivating the title of this paper.

Atomic-scale studies in this area are amenable to advanced simulation techniques, which have been used in the present study to investigate the structural, defect and fluorine insertion properties of the precursor oxides and the novel fluorinated products, focusing on Ba₂PdO₃ and Ba₂PdO₂F₂. The computational techniques are now well-tested methods for exploring solid-state properties at the microscopic level, and have been successfully applied to a range of inorganic materials^5–8 including cuprate and oxyhalide superconductors.^5,6 To the best of our knowledge, these are the first atomistic simulations of ternary palladium oxides and oxide-fluorides.

2. Simulation methods

Only a brief account of these widely used techniques (embodied within the GULP code⁹) will be described, as comprehensive reviews are given elsewhere.¹⁰ The basis of the lattice simulations is the specification of a potential model, which describes the potential energy of a system as a function of atomic coordinates and allows the modelling of perfect and defective lattices. The Born model representation is commonly used for polar solids, with the energy partitioned into long-range Coulombic and short-range pair potentials. An analytical function of the Buckingham form is used to describe the effective interatomic potentials:where the parameters A, ρ and C are assigned to each ion-ion short-range interaction within the crystal.

As charged defects will polarise other ions in the lattice, polarisability of the ions must be incorporated into the potential model. The “shell model” describes such effects by treating each ion in terms of a core (representing the nucleus and core electrons) connected via a harmonic spring to a shell (representing the valence electrons). Despite the simple mechanical representation of the ionic dipole, the shell model has been shown to effectively simulate both the dielectric and elastic properties of solid materials, by including the coupling between the short-range repulsive forces and ionic polarisation. It should be stressed, as argued previously,¹⁰ that employing such a potential model does not necessarily mean that the electron distribution corresponds to a fully ionic system, and that the general validity of the model is assessed primarily by its ability to reproduce observed crystal properties. In practice, it is found that potential models based on formal charges work well even for some semi-covalent compounds such as silicates and zeolites.¹⁰

An important feature of these calculations is the treatment of the extensive lattice relaxation about a point defect (e.g. vacancy or interstitial fluoride ion). The Mott–Littleton approach^9,10 is to partition the crystal lattice into two spherical regions. Ions in the central inner region surrounding the defect (typically containing more than 300 ions) are relaxed explicitly. In contrast, the remainder of the crystal, where the defect forces are relatively weak, is treated by more approximate quasi-continuum methods.

It is recognised that there are limitations to such lattice simulation methods where the use of explicit electronic structure techniques may be more appropriate. However, such a large number of ions are not readily treated by quantum mechanical techniques, whereas previous studies on oxide and oxyhalide superconductors^5,6 demonstrate the value (and versatility) of simulation methods in probing the nature of defect-related processes in complex materials.

3. Results and discussion

3.1 Crystal structures

The short-range potential parameters were transferred from previous work on cuprate oxide-fluoride superconductors,⁶ and fluorohalide luminescence compounds,⁸ and then adjusted slightly by simultaneous refinement to the complex crystal structures^1,11 of four different phases Ba₂PdO₂F₂, BaSrPdO₂F₂, Ba₂PdO₃ and Sr₂PdO₃. This provides considerable data within their structures and results in a common set of potentials across the composition range (listed in Table 1). We stress that reproduction of all four observed structures simultaneously is not a trivial exercise. This approach enables some information concerning the curvature of the energy surface to be included, and has the added advantage of improved transferability of the potential parameters. Additional experimental dielectric and elastic data are currently unavailable (in common with most novel ceramics), which would be useful for further validation or refinement of the potential models.

Table 1 Interatomic potentials and shell model parameters

(a) Short range
Interaction	A/eV	ρ/Å	C/eV Å^6
Ba^2+⋯O^2−	2096.8	0.3522	8.0
Ba^2+⋯F^−	4588.5	0.2725	0.0
Pd^2+⋯F^−	838.4	0.4600	0.0
Pd^2+⋯O^2−	1158.0	0.2927	0.1
O^2−⋯O^2−	22764.7	0.1490	43.0
O^2−⋯F^−	198.3	0.1110	73.8
F^−⋯F^−	507.5	0.4035	0.0
Sr^2+⋯O^2−	4630.4	0.2967	0.0
Sr^2+⋯F^−	757.2	0.2887	0.0

---

(b) Shell model^a
Species	Y/eV	k/eV Å^−2
a Y and k refer to the shell charge and harmonic spring constant respectively; Pd is rigid-ion species.
Ba^2+	1.848	29.1
Sr^2+	1.33	21.53
O^2−	−2.239	42
F^−	−2.38	101.2

---

Energy minimisation of the perfect lattices was first performed to generate the equilibrium structures with the calculated and experimental lattice parameters (a, b, c), and selected bond lengths listed in Table 2. Examination of the data shows good agreement between simulated and observed structures; all the bond lengths are reproduced to within 0.06 Å for all four complex materials Ba₂PdO₂F₂, BaSrPdO₂F₂, Ba₂PdO₃ and Sr₂PdO₃, providing additional support for the validity of our potential model.

Table 2 Experimental^1,11 and calculated structural parameters for ternary palladium oxides and oxide-fluorides

a) Unit cell parameters
Phase	Lattice parameter	Experimental/Å	Calculated/Å
Ba2PdO3	a	4.080	4.062
	b	3.836	3.847
	c	13.335	13.311
Sr2PdO3	a	3.977	3.840
	b	3.530	3.528
	c	12.820	12.871
Ba2PdO2F2	a	4.140	4.167
	c	14.046	14.039
BaSrPdO2F2	a	4.091	4.074
	c	13.409	13.413

---

b) Bond lengths
Phase	Bond	Experimental/Å	Calculated/Å
Ba2PdO3	Ba–O	2.708	2.769
		2.802	2.799
		2.724	2.779
	Pd–O	2.025	1.995
		2.040	2.031
Sr2PdO3	Sr–O	2.565	2.597
		2.591	2.608
		2.660	2.626
	Pd–O	1.947	1.894
		1.989	1.920
Ba2PdO2F2	Ba–O	2.806	2.836
	Ba–F	2.627	2.618
	Pd–O	2.070	2.083
	Pd–F	4.076	4.082
BaSrPdO2F2	(Ba,Sr)–O	2.743	2.741
	(Ba,Sr)–F	2.551	2.541
	Pd–O	2.046	2.039
	Pd–F	3.927	3.923

---

XRD studies show that the Ba₂PdO₂F₂ system adopts the T′-type (Nd₂CuO₄) structure in which Pd is coordinated by four oxygens in a square planar fashion, while Ba occupies a fluorite-like layer and coordinated by four oxygens and four fluorines in a distorted cubic arrangement (Fig. 1). The related T-type (La₂CuO₄) structure contains octahedral Cu with four oxygens and two fluorines at equatorial and apical sites respectively.

As noted, there are difficulties in determining the precise O²⁻ and F⁻ lattice positions using purely diffraction techniques based on their scattering power. In this context, our lattice simulation methods can be useful in comparing the energetics of different oxyfluoride structure types of the same stoichiometry. The calculated lattice energies for the specified T (La₂CuO₄) and T′ (Nd₂CuO₄) structure types of both Ba₂PdO₂F₂ and BaSrPdO₂F₂ have been calculated and are listed in Table 3.

Table 3 Calculated lattice energies per formula unit for T (La₂CuO₄) versus T′ (Nd₂CuO₄) structures of the oxide-fluorides

Phase^a	T/eV	T′/eV
a Values for unrelaxed atomic positions.
Ba2PdO2F2	−91.79	−95.18
BaSrPdO2F2	−92.93	−97.62

---

The comparison of the calculated lattice energies for the two alternative structures confirms that the T′structure is the most energetically favourable structure type for both Ba₂PdO₂F₂ and BaSrPdO₂F₂. The expected fluorine positions are therefore confirmed by the modelling results, in which the substituting fluorines do not occupy an apical site of a Pd–O polyhedron but are located in the Ba fluorite-like layers, leaving Pd in a square planar coordination. This result is consistent with the structure type proposed from XRD and Pd K-edge EXAFS measurements.¹ Further work on these materials will encompass studies on possible O/F (dis)ordering effects.

3.2 Intrinsic atomic defects

The basic defect properties of these new palladium-based compounds are not clearly characterised at the atomic level. Calculations were first performed on the energies of isolated point defects (vacancies and interstitials) focusing on the Ba₂PdO₃ and Ba₂PdO₂F₂ (T′) materials. The isolated defect energies were then combined to give the energies of formation of Frenkel and Schottky type defects (Table 4) based on the following equations for Ba₂PdO₂F₂, as an example. Kroger–Vink notation is used where, for instance, V_O˙˙ is an oxygen vacancy and F_i′ is an interstitial fluoride ion.

Table 4 Calculated energies for intrinsic atomic defects in Ba₂PdO₃ and Ba₂PdO₂F₂

Defect type	Ba2PdO3/eV defect^−1	Ba2PdO2F2/eV defect^−1
Full Schottky	10.85	6.99
BaO partial Schottky	3.59	4.90
PdO partial Schottky	6.00	2.30
BaF2 partial Schottky	—	1.47
F Frenkel	—	2.10

---

Full Schottky:

2Ba_Ba^x + Pd_Pd^x + 2O_O^x + 2F_F^x = 2V_Ba″ + V_Pd″ + 2V_O˙˙ + 2V_F˙ + Ba₂PdO₂F₂ (surf) (2)

BaO partial Schottky-type:

Ba_Ba^x + O_O^x = V_Ba″ + V_O˙˙ + BaO (surf) (3)

BaF₂ partial Schottky-type:

Ba_Ba^x + 2F_F^x = V_Ba″ + 2V_F˙ + BaF₂ (surf) (4)

PdO partial Schottky-type:

Pd_Pd^x + O_O^x = V_Pd″ + V_O˙˙ + PdO (surf) (5)

Fluorine Frenkel:

F_F^x = V_F˙ + F_i′ (6)

Examination of Table 4 reveals that the calculated energies for the full Schottky, the BaO and PdO partial Schottky and the F Frenkel, suggest that intrinsic atomic defects of these reaction types are not expected at high levels within the oxide-fluoride. However, the relatively low energy for the BaF₂ partial Schottky disorder suggests that non-stoichiometry related to BaF₂ loss may be possible for the Ba₂PdO₂F₂ system. It is interesting to note that recent experimental evidence indicates that the oxide-fluoride is metastable, decomposing slowly in air, with the increased formation of BaF₂ over time.¹ In addition, it is noted that BaF₂ is often formed during the synthesis of Ba₂PdO₂F₂, sometimes in an amorphous form, which is not detected satisfactorily using X-ray powder diffraction. This topic warrants further experimental investigation.

3.3 Fluorine incorporation

For the Sr₂CuO₂F_2+δ superconductor, the substitution of two fluorine ions for one oxygen ion is believed to be the primary mechanism in the low temperature fluorination of the precursor oxide, Sr₂CuO₃.² However, there is limited atomic-scale information on this process in the Pd-based system. We have therefore considered the energetics of the following fluorination reaction in Ba₂PdO₃ involving the incorporation of two fluoride ions for each oxygen ion lost:

F₂ + O_O^x = V_O˙˙ + 2F_i′ + ½ O₂ (7)

To investigate the preferred fluorine site in Ba₂PdO₃, defect calculations were performed on the isolated fluorine interstitial. Following an extensive search of the potential energy surface, the lowest-energy interstitial position is found at the (¼ ¼ ¼) position in the orthorhombic structure (s.g. Immm). This is in good agreement with the fluorine site postulated by experimental structural studies.¹

It is now well established that the crucial “hole-doping” mechanism for high T_c superconductivity in Sr₂CuO₂F_2+δ is via excess fluorine (δ) accommodated at interstitial sites.^2,6 It is not clear, however, whether this process occurs in the Pd-based material. We have therefore also considered the energetics for fluorine insertion (as interstitial defects) in Ba₂PdO₂F₂ involving the creation of oxygen or palladium holes (h˙) as localised species represented by the following reaction:

½ F₂ = F_i^′ + h˙ (8)

The calculated energies for reactions 7 and 8 are listed in Table 5 and were derived using energies for relevant defect (hole) and intra-atomic terms (for O²⁻ to ½ O₂, and for ½ F₂ to F⁻). Our approach to these reactions follows that first used for transition metal oxides and subsequently the cuprate superconductors.^5,6 There may be uncertainties in the free-ion terms, but our main concern here is to probe the relative energetics of these processes; for this task our simulation procedures have proved to be reliable. The merit of our defect modelling approach is that it includes detailed estimates of lattice polarization and Coulomb energies, which are difficult to make from other sources.

Table 5 Calculated energies for fluorine incorporation reactions

Compound/Process	E/eV per Fi^′^a
a Atomic energy terms in eV: Pd hole (4.15); O^2− → ½ O2 (−9.86); ½ F2 → F^− (−2.58). b From D'Arco and Islam.^6
Ba2PdO3/Reaction (7)	−0.46
Ba2PdO2F2/Reaction (8)	3.33
Sr2CuO2F2/Reaction (8)^b	−0.36

---

Two main points emerge from the results presented in Table 5. First, the exothermic value for the fluorination of Ba₂PdO₃viareaction (7) indicates that the predicted mechanism is a highly favourable process. Our results therefore provide support that this reaction, involving substitution of one oxygen by two fluorine atoms, is the primary mechanism in the low temperature fluorination of the precursor oxide. Studies have already found that the synthesis of ternary oxide-fluorides is difficult by conventional solid-state reactions at high temperature, and that “soft-chemistry” pathways are required.^2,3 Indeed, low temperature fluorination reactions have generally become useful chemical routes to novel materials, and are comparable with the more commonly used method of cation doping.

Second, the higher positive energy for reaction (8) in Ba₂PdO₂F₂ suggests that excess fluorine to form Pd³⁺ holes is relatively unfavourable in this system. Indeed, recent XANES and EXAFS spectroscopy studies indicate that there is no change in local coordination or oxidation state (to Pd³⁺ or Pd⁴⁺) upon fluorination.¹ For direct comparison, it is worth noting that our previous calculated value for the same reaction (8) in Sr₂CuO₂F₂ is highly favourable (−0.36 eV),⁶ and found to be consistent with the available measured oxidation enthalpies of other cuprate materials. This result for the cuprate also agrees with observation as it is known that this reaction is responsible for the hole-doping in Sr₂CuO₂F₂ necessary for superconductivity.² Moreover, these results also assist in rationalising the observed difference between palladium and copper oxide-fluorides in that the fluoride stoichiometry of Sr₂CuO₂F_2+δ has been reported to contain excess fluorine,² whereas Ba₂PdO₂F₂ does not show such behaviour.

4. Conclusion

Our simulation study has allowed us to gain significant insight as to the defect and fluorine insertion properties of the new Ba₂PdO₂F₂ oxide-fluoride system on the atomic scale. The significance of the present study is that it provides some rationalisation, based upon quantitative simulations rather than qualitative proposals, as to the properties of the palladium oxide-fluoride, which show interesting comparisons with the analogous cuprate superconductor.

Our discussion has highlighted three key points. First, the lattice simulations confirm that the most favourable structure type is the T′ (Nd₂CuO₄) structure for both Ba₂PdO₂F₂ and BaSrPdO₂F₂, comprised of square planar Pd²⁺ and fluoride ions in the Ba(Sr) fluorite-like layer. This accords with recent studies using X-ray absorption (EXAFS) techniques. The lowest defect energy in Ba₂PdO₂F₂ is calculated for the Ba/F vacancy disorder, which suggests possible non-stoichiometry related to BaF₂ loss. Second, our calculated exothermic energy for the reaction involving the incorporation of two fluoride ions for each oxygen ion lost in the precursor oxide Ba₂PdO₃ provides support that this is the primary mechanism in the fluorination process. Finally, the formation of fluorine interstitials and holes in Ba₂PdO₂F₂ is an energetically unfavourable process in accord with the observed resistance to excess fluorine, and in contrast to the related cuprate superconductor Sr₂CuO₂F_2+δ.

Acknowledgements

We are grateful to the EPSRC and the Daresbury CCP5 group for financial support, to Dr J. Tolchard, Dr R. A. Davies and Dr P. R. Slater for helpful discussions, and to the Computational Chemistry Working Party for RAL computing facilities.

References

1. T. Baikie, E. L. Dixon, J. F. Rooms, N. A. Young and M. G. Francesconi, Chem. Commun., 2003, 1580.
2. M. Al-Mamouri, P. P. Edwards, C. Greaves and M. Slaski, Nature, 1994, 369, 382; M. G. Francesconi, P. R. Slater, J. P. Hodges, C. Greaves, P. P. Edwards, M. Al-Mamouri and M. Slaski, J. Solid State Chem., 1998, 135, 17.
3. R. L. Needs, M. T. Weller, U. Scheler and R. K. Harris, J. Mater. Chem., 1996, 6, 1219; P. R. Slater and R. K. B. Gover, J. Mater. Chem., 2002, 12, 291; L. D. Aikens, L. J. Gillie, R. K. Li and C. Greaves, J. Mater. Chem., 2002, 12, 264.
4. A. K. Prodjosantoso, B. J. Kennedy, T. Vogt and P. M. Woodward, J. Solid State Chem., 2003, 172, 89; H. Oesterreicher, Solid State Commun., 2003, 127, 563; F. J. Brink, L. Noren and R. L. Withers, J. Solid State Chem., 2004, 177, 2177; T. Hanaguri, C. Lupien, Y. Kohsaka, D. H. Lee, M. Azuma, M. Tanako, H. Takagi and J. C. Davis, Nature, 2004, 430, 1001.
5. M. S. Islam, M. S. D. Read and S. D'Arco, Faraday Discuss., 1997, 106, 367; N. L. Allan, P. S. Baram, A. Gormezano and W. C. Mackrodt, J. Mater. Chem., 1994, 4, 817; J. P. Hill, N. L. Allan and W. C. Mackrodt, Chem. Commun., 1996, 2703.
6. S. D'Arco and M. S. Islam, Phys. Rev. B, 1997, 55, 3141; M. S. Islam and S. D'Arco, Chem. Commun., 1996, 2291.
7. M. S. D. Read, M. S. Islam, G. W. Watson and F. E. Hancock, J. Mater. Chem., 2001, 11, 2597; M. S. Islam, J. R. Tolchard and P. R. Slater, Chem. Commun., 2003, 1486; M. S. Islam and R. A. Davies, J. Mater. Chem., 2004, 14, 86.
8. M. S. Islam and R. C. Baetzold, J. Phys. Chem. Solids, 1992, 53(8), 1105.
9. J. D. Gale, J. Chem. Soc., Faraday Trans., 1997, 93, 629.
10. Computer Modelling in Inorganic Crystallography, ed. C. R. A. Catlow, Academic Press, San Diego, 1997.
11. Y. Laligant, A. LeBail, G. Ferey, M. Hervieu, B. Raveau, A. Wilkinson and A. K. Cheetham, Eur. J. Solid State Inorg. Chem., 1998, 25, 237; H. D. Von Wasel-Nielsen and R. Hoppe, Z. Anorg. Allg. Chem., 1970, 375, 209.

---