Facile fabrication of Bi₂O₃/Bi–NaTaO₃ photocatalysts for hydrogen generation under visible light irradiation

Abstract

A new series of Bi₂O₃/Bi–NaTaO₃ photocatalysts were fabricated by a modified solid state reaction method. After an optimal amount of bismuth doping into NaTaO₃, the incorporated bismuth forms a separate oxide phase instead of remaining in the originally doped form. The band gaps of the Bi₂O₃/Bi–NaTaO₃ samples were tuned to effectively absorb visible light and induced visible light photocatalytic activity, with varying bismuth concentration. Photoluminescence studies confirmed that the optimum amount of bismuth, found to be 0.3 Bi₂O₃/Bi–NaTaO₃, effectively suppresses the recombination rate of photogenerated charge carriers. A further increase in bismuth concentration (>0.3 molar ratio) leads to impurity formation and creates defect states which inhibit the photocatalytic activity of the Bi₂O₃/Bi–NaTaO₃. The energy band positions of Bi₂O₃ and Bi–NaTaO₃ were calculated by PEC measurement. The 0.3 Bi₂O₃/Bi–NaTaO₃ photocatalyst exhibited the highest performance in terms of H₂ evolution, i.e. 102.5 μmol h⁻¹ under visible light irradiation (λ ≥400 nm) in aqueous methanol solution as the sacrificial reagent. The photocatalytic activities of Bi₂O₃/Bi–NaTaO₃ composites were explained on the basis of the photosensitizing effect of Bi₂O₃, electron–hole separation and PL intensity.

---

1. Introduction

Hydrogen energy is expected to become one of the potential candidates to replace fossil fuels in the near future because of its high-energy capacity, recycling potential and environmentally friendly qualities.¹ A number of methods have been attempted to produce hydrogen, such as the electrolysis of water and reforming of hydrocarbons, etc. Actually, these methods can only be considered as short-term H₂ solutions because they have significant environmental and energy input concerns.² Among them, photocatalytic decomposition of water is one route that has great potential for the production of H₂, directly from abundant and renewable water and natural sunlight. Therefore, the development of highly efficient and visible-light driven photocatalysts is urgently required from an energy saving, economically viable and environmentally friendly viewpoint. Since the report of Fujishima and Honda on water splitting using a TiO₂ photocatalyst,³ numerous attempts have been made towards the development of new semiconductor photocatalysts for efficient water splitting. A comprehensive survey of photocatalytic activity, chemical stability and environmental friendliness shows that the most promising photocatalysts might be titanium, niobium or tantalum-based materials.⁴ Among these materials, tantalates are a group of highly active photocatalysts for water splitting. Most of these active tantalates are perovskite-related oxide compounds, such as K₃Ta₃Si₂O₁₃,⁵ A′Ta₂O₆ (A′: Ca, Sr, and Ba),^6,7 KLn₂Ta₅O₁₅,⁸ Sr₂M₂O₇ (M = Nb, Ta),^9,10 KTaO₃,^11,12 RbNdTa₂O₇¹³ and so forth.¹⁴ In particular, NiO/La : NaTaO₃, with a perovskite structure, has been proven to be one of the most promising photocatalysts for water splitting reactions with a highest quantum efficiency of 56% at λ = 270 nm.¹⁵ Sodium tantalate is an important lead-free perovskite-type ferroelectric material.¹⁶ Over the last few years, researchers have paid much attention to the use of ferroelectric materials as photocatalysts.^17–19 Ferroelectric materials have internal dipolar fields which can drive photogenerated electrons and holes in opposite directions and hence reduce the electron–hole recombination. The minimisation of electron–hole recombination in the materials greatly enhances the photocatalytic efficiency.²⁰ However, pure NaTaO₃ becomes active solely under ultraviolet light, which is only a small part (4%) of solar irradiation. But this material might be a good mother structure for design and development of visible light driven photocatalysts. A number of different attempts have been made to modify NaTaO₃ in order to make it active in the visible light region, mainly by doping with cations or anions.^21,24 To date, NaTaO₃ based visible-light-driven photocatalysts are rare. Recently, some reports have been found on the development of visible-light-driven NaTaO₃-based photocatalysts such as Na_1−xLa_xTa_1−xCr_xO₃,^21,22 Na_1−xLa_xTa_1−xCo_xO₃,²³ NaTaO_3−xN_x,^24,25 Ir-La-doped NaTaO₃etc.²⁶ Therefore, it is of great interest to develop novel visible-light responsive NaTaO₃ photocatalysts. Recently, bismuth-doped NaTaO₃ nanoparticles prepared by a hydrothermal method have been studied for photocatalytic reactions. Li et al. reported a visible light active solid solution photocatalyst, i.e. NaTa_1−xBi_xO₃. Hydrogen evolution over these nanoparticles was reported without any co-catalyst loading under visible light radiation.²⁷ The optical absorption properties of Bi³⁺-doped NaTaO₃ nanoparticles were also studied by Wang et al., where a small change in the absorption edge of NaTaO₃ was observed upon Bi doping.²⁸ Kanhere et al. have studied the effects of Bi doping on Na or Ta or both Na and Ta sites and their optical and photocatalytic decomposition of methylene blue.²⁹ However, there have been no reports so far on Bi₂O₃/Bi–NaTaO₃ and their optical properties and photocatalytic activity towards H₂ evolution from aqueous methanol solution. Therefore, detailed investigations on the optical properties and photocatalytic production of hydrogen over Bi₂O₃/Bi–NaTaO₃ powders with different concentrations of Bi are necessary.

In this manuscript, we synthesized a series of Bi₂O₃/Bi–NaTaO₃ photocatalysts with varying bismuth concentrations through a modified solid state reaction method. The impact of bismuth concentration on the crystal structure, morphology, optical properties, photoelectrochemical properties and photocatalytic activity towards hydrogen production over aqueous methanol solution were discussed in detail.

2. Experimental

2.1. Synthesis of Bi₂O₃/Bi–NaTaO₃

The NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts were prepared by a modified solid state reaction method using sodium oxalate Na₂C₂O₄ (BDH AR, purity 99.99%), tantalum oxide Ta₂O₅ (Fluka AG, purity >99.99%) and bismuth nitrate Bi(NO₃)₃·5H₂O (Merck AR, purity 99.9%). The chemicals were mixed with the molar ratio [Na] : [Bi] : [Ta] = 1 − x : x : 1 (where x = 0–0.5) followed by the addition of urea (SDS AR, purity 99.5%) powder. In the present study, the molar ratio of urea to metal ions was kept at [urea] : [Na₂C₂O₄ + Bi (NO₃)₃·5H₂O + Ta₂O₅] = 2 : 1. The mixtures (Ta₂O₅, Na₂C₂O₄, Bi(NO₃)₃·5H₂O and urea) were ground thoroughly in a mortar and transferred into a crucible. Then the mixture was activated in a muffle furnace with the heating rate of 2 °C min⁻¹ ranging from room temperature to 300 °C, 5 °C min⁻¹ ranging from 300 to 600 °C and kept at 600 °C for 4 h. For simplicity, Bi doped NaTaO₃ prepared at a Bi concentration of 0.05 molar ratio is designated as Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts prepared by varying the bismuth concentration from 0.1–0.5 molar ratio are designated as 0.1 Bi₂O₃/Bi–NaTaO₃, 0.2 Bi₂O₃/Bi–NaTaO₃, 0.3 Bi₂O₃/Bi–NaTaO₃, 0.4 Bi₂O₃/Bi–NaTaO₃, 0.5 Bi₂O₃/Bi–NaTaO₃ respectively. The Bi₂O₃ was also prepared by same method.

In this method, urea plays two important roles: complexing agent and fuel. During the reaction process, firstly the urea begins melting into a liquid (melting point: 135 °C) with rising temperature. The molten urea then provides a liquid medium in which the diffusion coefficient is higher than that in the solid medium. In the liquid environment Ta₂O₅, Na₂C₂O₄ and Bi(NO₃)₃ react easily with urea to form the Ta–urea complex, Na–urea complex and Bi–urea complex. Meanwhile, the ignition of urea generates a large amount of heat and results in an instantaneous high local temperature around 1500 °C (inside the reactants), which ignites the metal-precursors at a temperature much lower than the actual phase formation temperature.¹⁶ This temperature will be sufficient to form the crystallized Bi₂O₃/Bi–NaTaO₃ powder. Therefore, the molten urea and combustion of urea play an important role in the whole process.

2.2. Methods of characterization

The samples were characterized by powder X-ray diffraction (PXRD) patterns, BET-surface area, diffuse reflectance UV-vis (DRUV-vis), field emission scanning electron microscopy (FESEM), transmission electron microscopy (TEM), high resolution image transmission electron microscopy (HRTEM), energy dispersive X-ray spectroscopy (EDAX), photoluminescence spectroscopy (PL spectra), X-ray photoelectron spectroscopy (XPS) and photoelectrochemical measurements. The powder X-ray diffraction pattern was recorded on RINT-UltimaIII, Rigaku diffractometer with automatic control. The patterns were run with monochromatic Cu-Kα radiation from 2θ = 10–80° with a scan rate of 2° min⁻¹. Field emission scanning electron microscopy (FESEM) images were taken on a Zeiss Supra 55. Transmission electron microscopy (TEM) and high resolution transmission electron microscopy (HRTEM) were obtained on a Philips TECHNAI G² operated at 200 kV, in which samples were prepared by dispersing the powdered samples in 2-propanol by sonication for 3 min and then drop-drying on a copper grid coated with carbon film. Optical absorbance was measured by diffuse reflectance UV-vis (DRUV-vis) spectra of the catalyst samples with a Varian Cary 100 spectrophotometer equipped with a diffuse reflectance accessory in the region 200–800 nm, with boric acid as the reference. Photoluminescence spectra were recorded with a Jasco FP-6600 spectrofluorimeter. The PL spectra were measured at liquid nitrogen temperature under excitation at 300 nm, provided by emission from a xenon lamp. The Bi content in the sample before and after the preparation of the photocatalysts was analysed by atomic absorption spectroscopy (AAS, Shimadzu AA-6300) and X-ray photoelectron spectroscopy (XPS; ESCA-3200, Shimadzu) by using Al-Kα X-ray source. The binding energy correction was performed using the C1s peak of carbon at 285 eV as a reference. For photoelectrochemical measurements, the electrodes were prepared by electrophoretic deposition in an acetone solution (50 mL) containing photocatalyst powder (40 mg) and iodine (10 mg). Two parallel FTO electrodes were immersed in the solution with a 10–15 mm separation, and a 50 V bias was applied between the two for 3 min under potentiostat control. The coated area was fixed at 1 cm × 3 cm and then dried. The photoelectrochemical measurement was performed using a conventional Pyrex electrochemical cell consisting of a prepared electrode, a platinum wire as a counter electrode (1 mm in diameter, 15 mm in length) and an Ag/AgCl reference electrode. The cell was filled with an aqueous solution of 0.1 M Na₂SO₄ and the pH of the solution was adjusted to 6.5. The electrolyte was saturated with argon prior to electrochemical measurements and the potential of the electrode was controlled by a potentiostat (HZ-5000, Hokuto Denko; SDPS-501C, Syrinx). Irradiation was performed through the conducting glass using a 300 W Xe lamp with a cold mirror and cut-off filters (Hoya) as necessary. The current vs. potential (I–V) measurement was done by using a potentiostat (Versastat 3, Princeton Applied Research) with 300 W Xe lamp. This measurement follows the same process as that of the photoresponse experiment. It should be noted that the FTO (fluorine doped tin oxide) did not show a photoresponse in the solution.

2.3. Photocatalytic hydrogen evolution using aqueous methanol solution

The catalytic activity and deactivation were studied in a batch reactor. About 0.1 g of catalyst was suspended in 100 ml of an aqueous solution containing 10 vol.% of CH₃OH solution for H₂ evolution. The solution was kept under stirring with a magnetic stirrer, prohibiting particles to settle at the bottom of the reactor. Prior to irradiation, the reaction mixture was purged with N₂ in order to remove dissolved gases. A 125 W medium pressure Hg lamp was used as the visible light source and 1 M NaNO₂ solution is used as a UV filter. The evolved gas was collected by the water displacement technique and analyzed by GC-17A using a 5 Å molecular sieve column with a thermal conductivity detector (TCD). A comparison of the retention time of the peak that appeared on the chromatogram with standards confirmed that the evolved gas was hydrogen.

3. Results and discussion

3.1. Structural characterization

The PXRD patterns of neat NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts are shown in Fig. 1(a). In all the samples, the prominent diffraction peaks relate to the monoclinic phase of NaTaO₃ according to JCPDS card FileNo. 74-2478. The lower concentration of Bi (0.05 molar ratio) does not affect the crystal structure of the NaTaO₃. In addition, no significant diffraction peak of Bi species was observed due to the higher dispersion of Bi over the NaTaO₃ cube. The main diffraction peak of NaTaO₃ shifted slightly towards the lower angle region at lower bismuth concentrations (0.05 molar ratio) and no further shift was observed when the Bi concentration increased from 0.1–0.5 molar ratio, as shown in Fig. 1(b). The shift indicated that lower amounts of bismuth were homogeneously doped into the NaTaO₃ lattice, because the ionic radii of Bi³⁺ (1.34 Å) and Na⁺ (1.39 Å) ions are very similar. If Bi³⁺ ions are replaced with Ta⁵⁺ ions in NaTaO₃ structures, a large shift should be observed due to the large difference in ionic radius of Bi³⁺ (1.34 Å) and Ta⁵⁺ (0.64 Å) ions. Moreover, the diffraction pattern of Bi doped NaTaO₃, with the perovskite structure similar to NaTaO₃, was observed at a lower angle than that of NaTaO₃. Therefore, the small shifts to lower angles observed in the diffraction patterns of Bi doped NaTaO₃ suggested the substitution of bismuth ions for sodium ions which occupied A sites in perovskite structures.³⁰ Besides the main diffraction of NaTaO₃, the additional diffraction peaks of Bi₂O₃ with monoclinic phase (JCPDS card File No. 41-1449) were also indexed in Fig. 1(a). The diffraction peaks of Bi₂O₃ started to appear and intensified gradually with increasing bismuth concentration from a 0.1–0.5 molar ratio. The Bi₂O₃ related peaks in the XRD spectra indicate that beyond a certain Bi concentration (0.05 molar ratio), the incorporated bismuth forms a separated oxide phase in addition to doping. Thus, we can confirm that the fabricated Bi₂O₃/Bi–NaTaO₃ samples with varying Bi concentration from 0.1–0.5 molar ratio have a mixture of two-phase composition: i.e. monoclinic phase of both Bi–NaTaO₃ and Bi₂O₃. The intensity of the XRD peaks increased with lower bismuth concentration (0.05 molar ratio) but decreased gradually upon increasing the Bi concentration from a 0.1–0.5 molar ratio. The initial increase of NaTaO₃ peak intensity was due to the incorporation of Bi into the NaTaO₃ lattice. However a higher bismuth concentration causes the samples to be poorly crystalline due to formation of Bi₂O₃ as a separate phase.²⁴ Moreover, impurities such as BiTaO₄ were found when the Bi content was greater than 0.3 molar ratio. Pure NaTaO₃ and Bi–NaTaO₃ have a surface area of 2.02 m² g⁻¹ and 2.16 m² g⁻¹ whereas the surface area of 0.1 Bi₂O₃/Bi–NaTaO₃, 0.2 Bi₂O₃/Bi–NaTaO₃, 0.3 Bi₂O₃/Bi–NaTaO₃, 0.4 Bi₂O₃/Bi–NaTaO₃, 0.5 Bi₂O₃/Bi–NaTaO₃ are found to be 2.26, 2.36, 2.71, 2.82, 2.39 m² g⁻¹, respectively. When Bi was introduced into NaTaO₃, the surface area of the photocatalysts is slightly increased. Since the surface area and particle size are inversely related to each other, the increasing surface area signifies that Bi concentration may inhibit the growth of the particle.^31,32

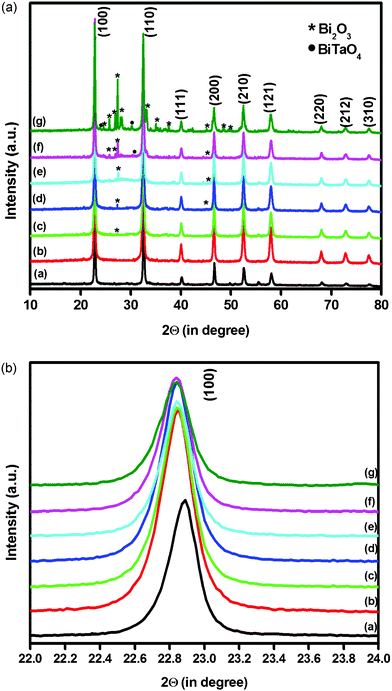Fig. 1 (a) X-ray diffraction patterns of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃; (b) Enlarged profile of the (100) diffraction pattern of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃.

3.2. Optical properties and band gap

Photoabsorbance of the undoped NaTaO₃, Bi–NaTaO₃, Bi₂O₃/Bi–NaTaO₃ and pure Bi₂O₃ photocatalysts were examined by using a diffuse reflectance UV-vis spectrometer. Fig. 2 shows the typical diffuse reflectance spectrum of undoped NaTaO₃, pure Bi₂O₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts at different bismuth concentrations. From the optical absorption spectra, we can see that pure Bi₂O₃ and all the Bi₂O₃/Bi–NaTaO₃ photocatalysts are harvesting the visible light, while pure NaTaO₃ and Bi–NaTaO₃ had no absorption in the visible region i.e. it can only harvest UV light due to its large band gap. The absorption edges of pure NaTaO₃, Bi–NaTaO₃ and Bi₂O₃ samples are roughly estimated from the absorption onset occurring at 311.5 nm (NaTaO₃), 348 nm (Bi–NaTaO₃) and 450 nm (Bi₂O₃) respectively, and accordingly the band gap energies are estimated to be about 3.98, 3.56 and 2.8 eV respectively. However, the Bi₂O₃/Bi–NaTaO₃ photocatalysts showed two different absorption edges at 348 nm and 450 nm respectively. The absorption edges at about 348 nm and 450 nm in the Bi₂O₃/Bi–NaTaO₃ photocatalysts was due to the absorption of Bi–NaTaO₃ and Bi₂O₃ respectively. The absorptions after Bi₂O₃ formation are drastic and stronger in the visible region. Furthermore, the absorption strengthens with increasing bismuth concentration. This is due to the photosensitization effect of Bi₂O₃ nanoparticles which could extend the spectral response of Bi–NaTaO₃ from the UV to the visible region, making the Bi₂O₃/Bi–NaTaO₃ photocatalysts easily activated by visible light. The remarkable red-shifting of absorption edges of the Bi₂O₃/Bi–NaTaO₃ to longer wavelengths suggest that Bi₂O₃/Bi–NaTaO₃ could be responsive to incident photons of lower energy.³³ It is expected that Bi₂O₃ contributed to the red shift due to narrowing of the band gap of the composite samples, which is evidenced by the colour change of the samples from the original white of NaTaO₃ to the slight yellow of Bi–NaTaO₃, and pale yellow to deep yellow of Bi₂O₃/Bi–NaTaO₃ samples. The pre-edge absorption became stronger with increasing bismuth concentration and its intensity increased with further increase of bismuth concentration. The fact that the pre-edge absorption became stronger with increasing bismuth concentration may be due to the presence of defect states and formation of Bi₂O₃ as a separate oxide phase in the materials.^34,35

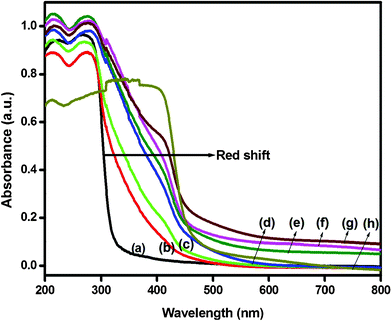Fig. 2 Photoabsorbance spectra of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃ (h) Bi₂O₃.

3.3. Morphology

The surface morphology of the photocatalysts NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ were examined by using field emission scanning electron microscopy (FESEM) as shown in Fig. 3. A cubic morphology was observed in the case of NaTaO₃, whereas irregular shaped morphology was found when Bi was doped into NaTaO₃. The two samples show different surface morphologies; in contrast to the smooth surface of the NaTaO₃ particle, a rough surface was found in the case of Bi–NaTaO₃ as shown in Fig. 3(a,b). These results clearly indicate the effect of bismuth concentration on the morphology of NaTaO₃. With increasing bismuth concentration, the surface morphologies of the Bi₂O₃/Bi–NaTaO₃ samples changed. When bismuth concentration increased from 0.1–0.5 molar ratio, the agglomeration of Bi₂O₃ particles formed on the surface of Bi–NaTaO₃ as shown in Fig. 3(c–g).

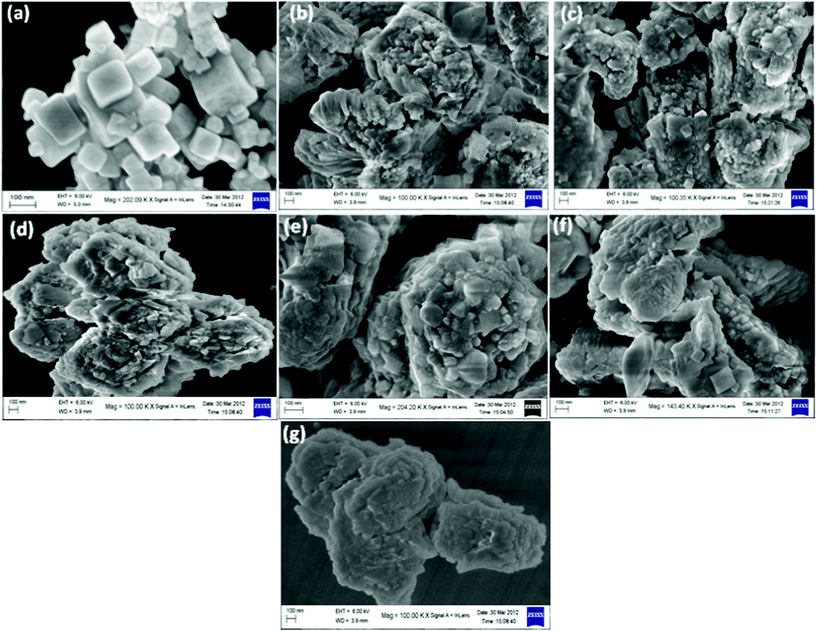Fig. 3 FESEM images of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃.

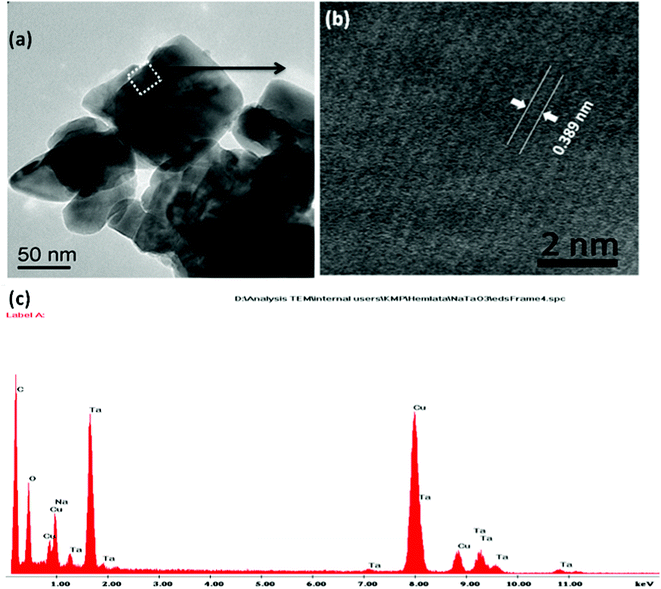
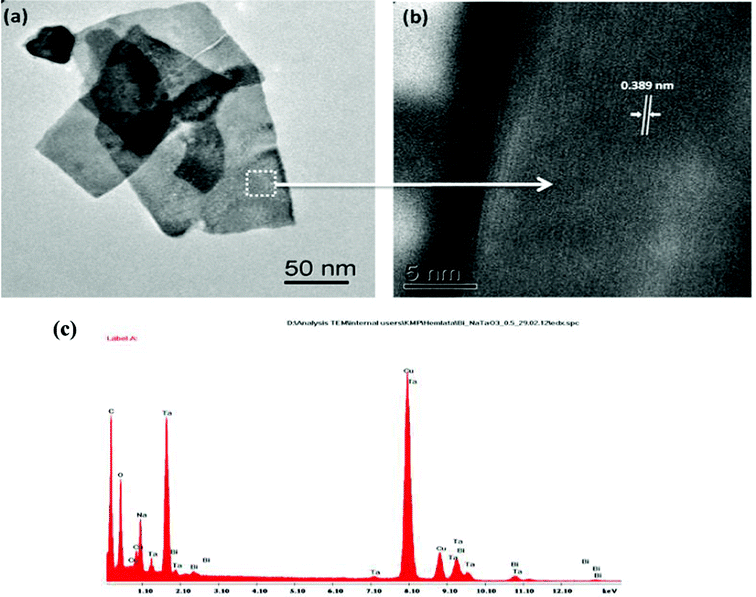
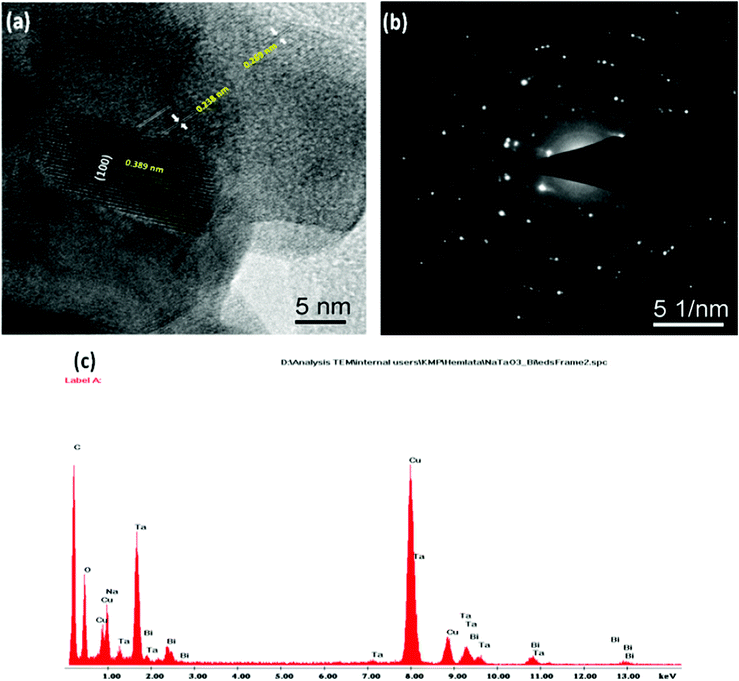
Fig. 4(a–f) shows the TEM micrographs of the undoped NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ samples, from which one could see that all of the samples exhibit an agglomerated cubic morphology. TEM imaging of the NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ powder samples revealed cubes with sizes greater than 100 nm. Most of the particles appeared as perfect cubes, as can be seen in the inset of Fig. 4(a–f). Bi incorporation into NaTaO₃ results in a rough surface morphology which is consistent with the SEM micrographs. Another observation from the TEM micrographs is that when the concentration of Bi increases in Bi₂O₃/Bi–NaTaO₃, the particle size of Bi₂O₃ also increases. Fig. 5 and 6 show the HRTEM images of NaTaO₃ and Bi–NaTaO₃. Fig. 5(b) shows the HRTEM of NaTaO₃, a smooth image of the area labelled with a white dotted line in Fig. 5(a). The d-spacing of 0.389 nm corresponds to the (100) plane of NaTaO₃ (Fig. 5(b)). The HRTEM image of NaTaO₃ with clear lattice fringes confirms the highly crystalline nature of NaTaO₃. The EDAX indicates the presence of Na, Ta and O in the NaTaO₃ sample (Fig. 5(c)). Fig. 6(b) showed the HRTEM image of Bi–NaTaO₃ of the area labelled with a white dotted line in Fig. 6(a). The d-spacing of 0.389 nm corresponds to the (100) plane of Bi–NaTaO₃ (Fig. 6(b)). Bi–NaTaO₃ showed the same d-spacing as that of NaTaO₃, due to the homogenous doping of Bi ions for Na ions in the NaTaO₃ structure. The EDAX indicates the presence of Na, Ta, Bi and O in the Bi–NaTaO₃ sample (Fig. 6(c)). The HRTEM image of Bi–NaTaO₃ with clear lattice fringes confirms the highly crystalline nature of Bi–NaTaO₃. The HRTEM image of 0.3 Bi₂O₃/Bi–NaTaO₃ clearly demonstrates the existence of nanoparticles of bismuth oxide distributed over a single cube of Bi–NaTaO₃ and the micrograph shows many nanoparticles of Bi₂O₃ with a size ranging between 3–5 nm, as shown in Fig. 7(a). Fig. 7(b) shows that 0.3 Bi₂O₃/Bi–NaTaO₃ exhibits clearly resolved lattice fringes with the interplanar d-spacing of 0.389 nm assigned to the (100) plane of the monoclinic Bi–NaTaO₃ structure, indicating the formation of high-quality 0.3 Bi₂O₃/Bi–NaTaO₃. A spot pattern from a 0.3 Bi₂O₃/Bi–NaTaO₃ single-crystal cube is shown in Fig. 7(c). The bright spots in the diffraction pattern of the 0.3 Bi₂O₃/Bi–NaTaO₃ nanocube are well matched with the (100), (111) and (110) planes of monoclinic Bi–NaTaO₃. Fig. 7(d) displays the EDAX spectrum of 0.3 Bi₂O₃/Bi–NaTaO₃ taken in the TEM mode. EDAX indicates the presence of Na, Ta and Bi in the 0.3 Bi₂O₃/Bi–NaTaO₃ sample. The TEM image and electron diffraction pattern of all the 0.3 Bi₂O₃/Bi–NaTaO₃ samples reveals the formation of well-crystallized 0.3 Bi₂O₃/Bi–NaTaO₃. The HRTEM image of 0.5 Bi₂O₃/Bi–NaTaO₃ was shown in Fig. 8(a). Fig. 8(a) shows that the 0.5 Bi₂O₃/Bi–NaTaO₃ composite exhibits clearly resolved lattice fringes with the interplanar d-spacing values of 0.269 and 0.238 nm, indexed to the (122) and (113) planes of monoclinic Bi₂O₃, while the d value of 0.389 nm is consistent with the (100) plane of Bi–NaTaO₃. The HRTEM image and electron diffraction pattern reveals that well-crystallized 0.5 Bi₂O₃/Bi–NaTaO₃ was formed (Fig. 8(a) and (b)). EDAX indicates the presence of Na, Ta and Bi in the 0.5 Bi₂O₃/Bi–NaTaO₃ sample (Fig. 8(c)).

Fig. 4 TEM micrographs of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃.

Fig. 5 (a,b) TEM image with square area and HRTEM image of the square area of NaTaO₃, (c) EDAX of NaTaO₃.

Fig. 6 (a,b) TEM image with square area and HRTEM image of the square area of Bi–NaTaO₃, (c) EDAX of Bi–NaTaO₃.

Fig. 7 (a,b) HRTEM images, (c) SAED pattern, (d) EDAX of 0.3 Bi₂O₃/Bi–NaTaO₃.

Fig. 8 (a) HRTEM image, (b) SAED pattern, (c) EDAX of 0.5 Bi₂O₃/Bi–NaTaO₃.

3.4. Photoluminescence (PL) spectra

The PL spectra of the semiconductors are useful to disclose the migration, transfer and recombination processes of the photogenerated electron–hole pairs, since PL emission results from the recombination of free charge carriers. The PL spectra of the undoped NaTaO₃, Bi–NaTaO₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts under liquid N₂ conditions with excitation at 300 nm are presented in Fig. 9. If the number of emitted electrons resulting from the recombination between excited electrons and holes is increased, the PL intensity increases and consequently, the photoactivity decreases.³⁶ For weaker PL intensity, the migration time of photoinduced charge carriers from the inside to the surface is short. Thus, the photoinduced charge carriers have a greater chance to reach the surface in advance of the recombination.³⁷ The emission intensity changes with varying Bi concentration, and increases with decreasing photocatalytic activity of the catalysts (Fig. 9). The PL spectra of all the Bi₂O₃/Bi–NaTaO₃ samples have similar patterns and are located in a similar wavelength region. Therefore, the emission should be intrinsic in origin for NaTaO₃ and correspond to a self-trapped exciton.³⁴ To understand the luminescence mechanism, the PL spectra deconvoluted into two peaks that were fitted to a symmetric Gaussian function. Fig. 10 shows the peak resolution results for the luminescence, showing that two Gaussian peaks located at λ > 440 nm and λ > 500 nm constitute the PL spectra of the NaTaO₃, Bi–NaTaO₃, 0.3 Bi₂O₃/Bi–NaTaO₃ and 0.5 Bi₂O₃/Bi–NaTaO₃ samples. Many researchers have accepted that the origin of visible luminescence in perovskite-type tantalates (ATaO₃)^38–40 or titanates (ATiO₃)^41–43 is due to the recombination of trapped electrons and holes (excitons), which is a characteristic feature of PL.⁴⁴ The luminescence emission in the region 450 nm is called “blue luminescence” (BL), which is attributed due to the intrinsic radiative emission related to the electron–hole pair recombination of a localized exciton in a TaO₆ octahedron.⁴¹ The lowest BL emission, observed in 0.3 Bi₂O₃/Bi–NaTaO₃, results from improved charge separation; that is why it shows the highest photocatalytic activity towards hydrogen evolution. The emission peaks in the region of 500 nm, called “green luminescence” (GL), can be attributed to an extrinsic defect radiation.^34,45,46 This emission could have originated from a large quantity of localized defect states related to vacancies and the formation of an unwanted phase at the grain boundaries, trapping the migrating excitons.⁴⁵ When Bi concentration exceeds 0.3 molar ratio, the GL intensity of the Bi₂O₃/Bi–NaTaO₃ samples increases, due to the increase in defect states and the formation of other phases such as BiTaO₄, which was confirmed from the XRD pattern. The decreased activity of the catalysts with high-level bismuth concentrations is attributed to the defect/impurity formation which is due to the large amount of bismuth incorporated into the NaTaO₃.

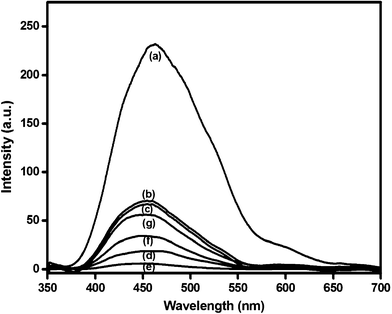Fig. 9 Photoluminescence emission spectra of (a) NaTaO₃ (b) Bi–NaTaO₃ (c) 0.1 Bi₂O₃/Bi–NaTaO₃ (d) 0.2 Bi₂O₃/Bi–NaTaO₃ (e) 0.3 Bi₂O₃/Bi–NaTaO₃ (f) 0.4 Bi₂O₃/Bi–NaTaO₃ (g) 0.5 Bi₂O₃/Bi–NaTaO₃ catalysts measured at −196 °C with 300 nm wavelength excitation.

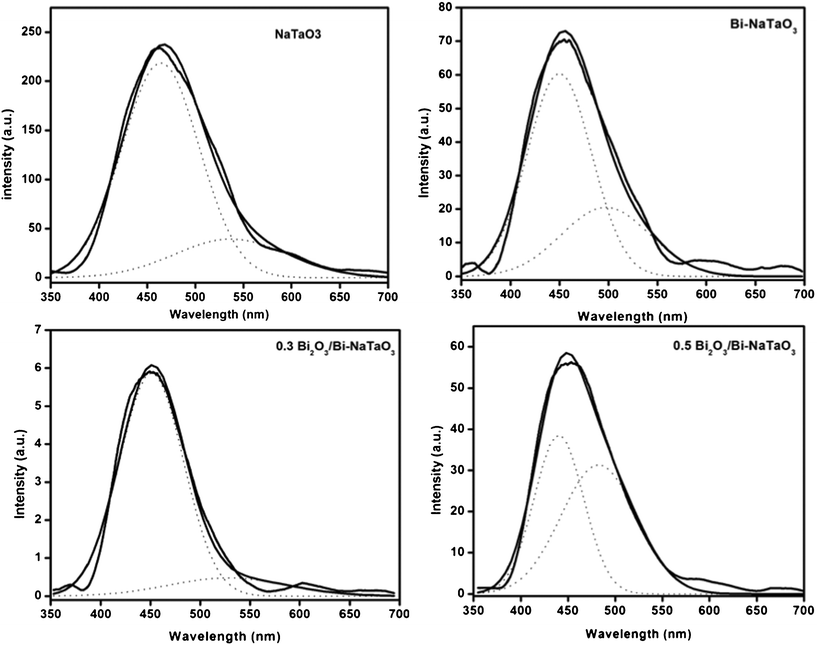Fig. 10 The spectra of NaTaO₃, Bi–NaTaO₃, 0.3 Bi₂O₃/Bi–NaTaO₃, 0.5 Bi₂O₃/Bi–NaTaO₃ were deconvoluted into two Gaussian peaks at ca. λ > 440 and λ > 500 nm.

Overall, the charge transport mechanism explained by the PL study along with the structural data from XRD analysis confirmed the role of the Bi species in the distorted perovskite NaTaO₃ as well as forming localized defect states or impurities such as BiTaO₄ to promote the defect radiation.

3.5. X-ray photoemission spectroscopy (XPS)

To investigate the formal oxidation state and chemical environment of the 0.3 Bi₂O₃/Bi–NaTaO₃ sample, X-ray photoemission (XPS) spectroscopy analysis was carried out. The narrow-scan XPS spectra of the Na 1s, O 1s, Ta 4f and Bi 4f peaks for the surface of the 0.3 Bi₂O₃/Bi–NaTaO₃ are shown in Fig. 11. The Na 1s peak in the 0.3 Bi₂O₃/Bi–NaTaO₃ was observed at 1072.2 eV and is thus assignable to the Na⁺ cation (Fig. 11(a)).⁴⁷ The two strong peaks for the Bi region at 164.8 and 159.5 eV (Fig. 11(b)) are assigned to the Bi 4f_5/2 and Bi 4f_7/2 peaks of Bi³⁺ in the short Bi–O bonds.⁴⁸ The Ta (4f) and O (1s) XPS spectrum was decomposed using 100% Gaussian curve fitting following Shirley background subtraction. The binding energies located at 26.2 and 28.03 eV correspond to Ta (4f_7/2) and Ta (4f_5/2) respectively for the core levels of Ta⁵⁺ cations as shown in Fig. 11(c).⁴⁹ On the other hand, the two Gaussian peaks are located at 530.98 and 532.9 eV respectively for O (1s) spectrum. The lower binding energy peak corresponds to the O (1s) core level of the O²⁻ anions in 0.3 Bi₂O₃/Bi–NaTaO₃. The O (1s) peak is related to the Ta–O, Na–O and Bi–O chemical bonding.^49–52 The higher binding energy peak is attributed to surface contamination, such as carbon oxides or hydroxides.^50–53

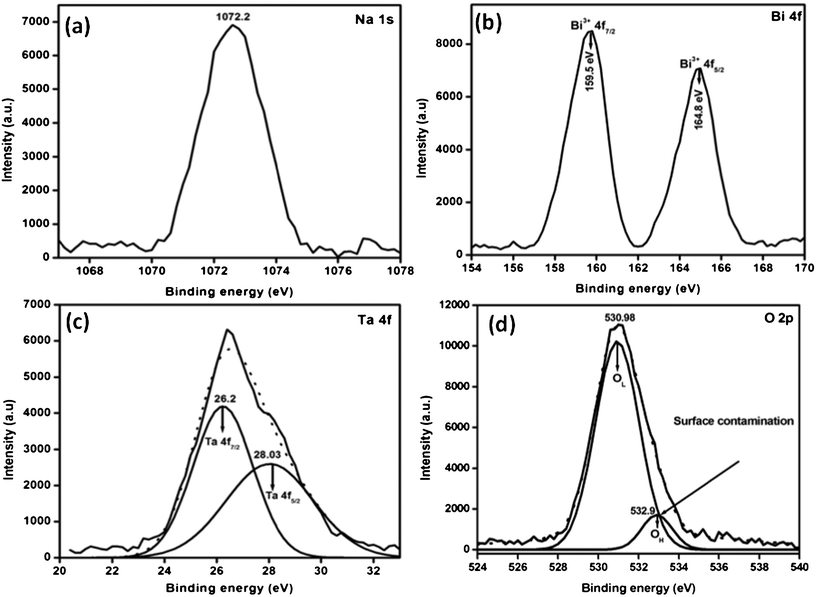Fig. 11 XPS spectra for 0.3 Bi₂O₃/Bi–NaTaO₃ in the regions of (a) Na-1s, (b) Bi-4f, (c) Ta-4f and (d) O-2p.

3.6. Photoelectrochemical (PEC) study

In order to elucidate the relative band edge position of Bi₂O₃ and Bi–NaTaO₃, the photoelectrochemical method has been adopted. Generally, the migration of photoinduced charge carriers depends on their corresponding band edge position. The photocurrent spectra of Bi–NaTaO₃ and Bi₂O₃ are shown in Fig. 12(a) and (b). The photoelectrochemical measurement was carried out at pH 6.5 vs. Ag/AgCl electrode in 0.1 M Na₂SO₄ solution. From the result of the photocurrent spectra, it was observed that Bi–NaTaO₃ is an n-type while Bi₂O₃ is a p-type semiconductor. The conduction band edge was calculated from the flat band potential of the semiconductor, which was supposed to be the photocurrent onset potential since photocurrent is generated when the applied potential exceeds flat band potential for an n-type semiconductor.⁵⁴ The photocurrent onset potential (conduction band edge) of Bi–NaTaO₃ was observed at −1.18 V vs. Ag/AgCl electrode. Since Bi₂O₃ is p-type, the photocurrent onset potential of Bi₂O₃ gives the value of the valence band edge, from which the value of the conduction band edge is estimated. The photocurrent onset potential of Bi₂O₃ was observed at +0.74 V vs. Ag/AgCl at pH 6.5 i.e. the valance band edge of Bi₂O₃ was lying at +0.74 V, hence the position of the conduction band edge was estimated to be at −2.06 V.⁵⁵ The thermodynamic requirement of H₂ evolution from water is that the conduction band edge of the photocatalysts must be more negative than the reduction potential of hydrogen (i.e. −0.58 V vs. Ag/AgCl at pH 6.5). In the present investigation, both Bi₂O₃ and Bi–NaTaO₃ have a more negative conduction band edge potential than the hydrogen evolution potential. The relative conduction band edge potential of Bi₂O₃ and Bi–NaTaO₃ showed both are suitable for water reduction reactions. The calculated band edge position of Bi₂O₃ and Bi–NaTaO₃ were represented in Scheme 1(a). Under thermal equilibrium conditions, the Fermi energy levels of the two materials have to be the same when a junction is formed between p-type Bi₂O₃ and n-type Bi–NaTaO₃ having opposite majority carriers, and an internal electric field is established. Under visible-light illumination, Bi₂O₃ could be easily excited and leads to the generation of photoelectrons and holes. According to Scheme 1(b), the photoexcited electrons on the conduction band of the p-type Bi₂O₃ can be easily transferred to the n-type Bi–NaTaO₃. The proficient transfer of photogenerated charge carriers can be promoted by the internal electric field. Thus, the photogenerated electron–hole pairs will be separated effectively by the p–n junction formed in the Bi₂O₃/Bi–NaTaO₃ interface and reduce the recombination of electron–hole pairs.^56–58 The efficient separation of charge carriers leads to higher photocatalytic activity. The photocurrent spectra of 0.3 Bi₂O₃/Bi–NaTaO₃ was shown in Fig. 13. The photocurrent under dark and light conditions was measured at pH 6.5 in 0.1 M Na₂SO₄ solution. From the photoelectrochemical study, it was observed that the photocatalysts showed both character of Bi₂O₃ and Bi–NaTaO₃ with applied potential. The generation of photocurrent under light conditions was greater than that under dark conditions. The increase in photocurrent under light conditions might be due to the generation of a greater number of excited electron–hole pairs.⁵⁹

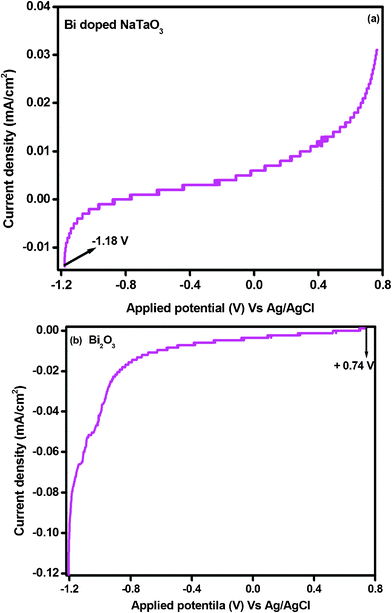Fig. 12 Current–potential curves for (a) Bi–NaTaO₃ and (b) Bi₂O₃ under light irradiation in 0.1 M Na₂SO₄ aqueous solutions at pH 6.5.

Fig. 13 Current–potential curves for 0.3 Bi₂O₃/Bi–NaTaO₃ electrodes under dark and light irradiation in 0.1 M Na₂SO₄ solution at pH 6.5.

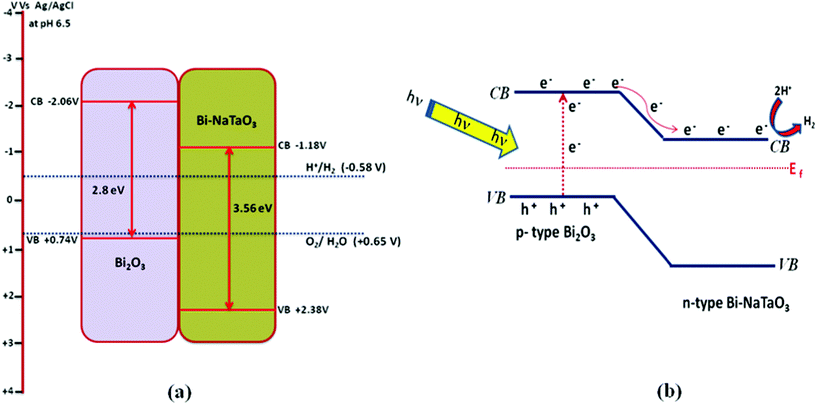Scheme 1 (a) Represents the band edge positions of p-type Bi₂O₃ and n-type Bi–NaTaO₃ calculated at pH 6.5 vs. Ag/AgCl. (b) Represents the charge transfer processes at the Bi₂O₃/Bi–NaTaO₃ composite interface for photocatalytic hydrogen production under visible light irradiation (λ ≥ 400 nm).

3.7. Photoresponse study

Fig. 14 shows the photoresponse spectra of 0.3 Bi₂O₃/Bi–NaTaO₃ with various cut-off wavelengths of incident light. These data were obtained by irradiating samples via appropriate cut-off filters for the wavelength shown, i.e. photon counts decrease upon increasing wavelength. From the spectra, we observe there is generation of appreciable photocurrent for wavelengths below 525 nm. As was observed from the figure, no photocurrent was observed when the sample was irradiated at wavelengths longer than 525 nm, which is consistent with the absorption edge shown in Fig. 2. Maximum photocurrent is generated when light is irradiated with λ ≥ 350 nm. The generation of photocurrent gradually decreases with increasing cut-off wavelengths. This indicates that the photocurrent generated by the electrode is due to the visible light response of the system.

Fig. 14 The dependence of photocurrent 0.3 Bi₂O₃/Bi–NaTaO₃ irradiation wavelength. The DRUV-vis spectrum is also shown by the solid line.

3.8. Photocatalytic reaction over Bi₂O₃/Bi–NaTaO₃

Fig. 15 shows the photocatalytic activities for hydrogen production over NaTaO₃, Bi–NaTaO₃, Bi₂O₃ and Bi₂O₃/Bi–NaTaO₃ in aqueous methanol solution. Blank experiments showed no appreciable H₂ evolution in the absence of either irradiation or photocatalyst. Neat NaTaO₃, Bi–NaTaO₃ are inactive under visible light irradiation due to the large bandgap energy. Photocatalytic H₂ production over Bi₂O₃ and Bi₂O₃/Bi–NaTaO₃ photocatalysts were evaluated under visible light illumination (λ ≥ 400 nm). It was already reported that some of the lower band gap materials such as BiOI and Bi₂O₃ effectively sensitized higher band gap materials like TiO₂ and ZnO and enhanced their activity in the visible region.^57,58,60 In the case of Bi₂O₃/Bi–NaTaO₃, Bi₂O₃ nanoparticles played a vital role in the visible light-induced photocatalytic activity of Bi–NaTaO₃, Bi₂O₃ being an inorganic photosensitizer having a band gap of 2.8 eV.⁶¹ It could be predicted that Bi₂O₃ nanoparticles might promote a photosensitizing effect which leads to enhanced activity in the visible region. Bi₂O₃ nanoparticles could be easily activated by visible light and induced photoelectrons and holes. In the absence of Bi–NaTaO₃, these electrons and holes might recombine rapidly owing to the narrow energy gap, leading to the quenching of spectral response.⁶² The PEC measurement reveals that Bi₂O₃ is a p-type semiconductor and Bi–NaTaO₃ is an n-type semiconductor. When they contact with each other, a junction is formed between them and the photoexcited electrons in the conduction band of the p-type Bi₂O₃ can be easily transferred to the n-type Bi–NaTaO₃. This results in proficient transfer of charge carriers at the composite interface (Scheme 1(b)). Thus, the recombination of the charge carriers could be effectively suppressed and lead to higher photocatalytic activity. It is clear that the photocatalytic activity depended markedly on Bi concentration, and the catalyst prepared with the optimum amount of Bi concentration, i.e. 0.3 Bi₂O₃/Bi–NaTaO₃, showed the highest activity. At this optimum Bi concentration, the photocatalyst was able to evolve 102.5 μmol h⁻¹ of H₂ gas. Higher concentrations of Bi result in agglomeration of Bi₂O₃ nanoparticles on the surface of Bi–NaTaO₃, and could effectively inhibit the light absorption by photocatalytically active Bi–NaTaO₃. To confirm the high charge transfer or low electron–hole recombination rate, photoluminescence (PL) studies have been undertaken. It is known that the PL emission results from the recombination of excited electrons and holes. Thus, the lower PL intensity indicates a lower recombination rate.³⁷Fig. 9 showed the PL spectra of Bi₂O₃/Bi–NaTaO₃. From the PL spectra, it was observed that 0.3 Bi₂O₃/Bi–NaTaO₃ showed lowest blue luminescence intensity, indicating a lower recombination rate of photoinduced charge carriers compared with other photocatalysts. Higher Bi concentrations abruptly decrease the photocatalytic activity of the photocatalysts. At higher concentrations of Bi (>0.3 molar ratio), the photocatalytic activity of Bi₂O₃/Bi–NaTaO₃ is hindered, even though the absorption onset of the photocatalysts is shifted towards the red region. The higher amount of Bi-species results in the formation of defect states and unwanted formation of impurities such as BiTaO₄, due to large amounts of bismuth incorporated into the NaTaO₃ lattice. The creation of defect states and unwanted phase formation acts as a recombination centre, which predominantly hinders the photocatalytic activity of the present investigated system.

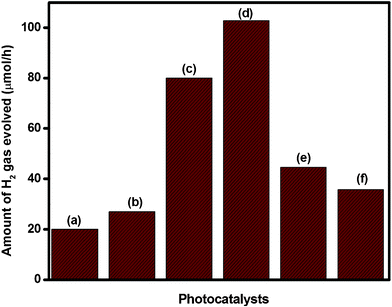Fig. 15 Photocatalytic hydrogen evolution over (a) Bi₂O₃ (b) 0.1 Bi₂O₃/Bi–NaTaO₃ (c) 0.2 Bi₂O₃/Bi–NaTaO₃ (d) 0.3 Bi₂O₃/Bi–NaTaO₃ (e) 0.4 Bi₂O₃/Bi–NaTaO₃ (f) 0.5 Bi₂O₃/Bi–NaTaO₃ in aqueous methanol solution.

4. Conclusions

The present study provides a new approach to synthesize Bi₂O₃/Bi–NaTaO₃ photocatalysts through a low temperature solid state reaction method. At low bismuth concentrations, the incorporated bismuth doped into the NaTaO₃ lattice, but at higher concentrations, the excess bismuth forms its oxide and remains as a separate phase. The FESEM confirmed the deposition of Bi₂O₃ particles on the surface of Bi–NaTaO₃ which is consistent with TEM micrographs. The lowest PL intensity was observed in the case of 0.3 Bi₂O₃/Bi–NaTaO₃, indicating the effective separation of photogenerated charge carriers. Bi concentrations greater than the optimum (>0.3 molar ratio) value result in the formation of defect states and impurities such as BiTaO₄, which decreases the photocatalytic activity. The PEC measurement confirmed the n-type character of Bi–NaTaO₃ and the p-type character of Bi₂O₃. The p–n junction between Bi₂O₃ and Bi–NaTaO₃ effectively suppresses the electron–hole recombination and enhances the photocatalytic activity. The 0.3 Bi₂O₃/Bi–NaTaO₃ photocatalyst exhibits the highest performance of H₂ evolution, 102.5 μmol h⁻¹ in aqueous methanol solution under visible light irradiation (λ ≥ 400 nm).

Acknowledgements

The authors are very grateful to Prof. B.K. Mishra, Director, IMMT, Bhubaneswar for giving permission to publish the work. The financial assistance by CSIR for funding a Networking Project (NWP 22) is greatly acknowledged. The authors are very grateful to Prof. K. Domen and Dr T. Minegishi (University of Tokyo, Japan) for their valuable suggestions regarding this work and for photoelectrochemical measurement studies, and Mr. M. Moriya for performing XPS analyses. The support from DST and JSPS is greatly acknowledged for the INDO-JAPAN collaborative project. The authors K. H. Reddy and S. Martha are thankful to CSIR, New Delhi for SRF.

References

1. J. S. Lee, Catal. Surv. Asia, 2005, 9, 4.
2. R. Marschall, A. Mukherji, A. Tanksale, C. Sun, S. C. Smith, L. Wang and G. Q. Lu, J. Mater. Chem., 2011, 21, 8871.
3. A. Fujishima and K. Honda, Nature, 1972, 238, 37.
4. H. Tong and J. Ye, Eur. J. Inorg. Chem., 2010, 1473.
5. A. Kudo and H. Kato, Chem. Lett., 1997, 867.
6. H. Kato and A. Kudo, Chem. Phys. Lett., 1998, 295, 487.
7. H. Kato and A. Kudo, Chem. Lett., 1999, 1207.
8. A. Kudo, H. Okutomi and H. Kato, Chem. Lett., 2000, 1212.
9. D. W. Hwang, H. G. Kim, J. Kim, K. Y. Cha and J. S. Lee, J. Catal., 2000, 193, 40.
10. A. Kudo, H. Kato and S. J. Nkagawa, J. Phys. Chem. B, 2000, 104, 571.
11. K. Sayama, H. Arakawa and K. Domen, Catal. Today, 1996, 28, 175.
12. T. Ishihara, H. Nishiguchi, K. Fukamachi and Y. Takkkita, J. Phys. Chem. B, 1999, 103, 1.
13. M. Machida, J. Yabunaka and T. Kijima, Chem. Mater., 2000, 12, 812.
14. X. Li and J. Zang, J. Phys. Chem. C, 2009, 113, 19411.
15. H. Kato, K. Asakura and A. Kudo, J. Am. Chem. Soc., 2003, 125, 3082.
16. J. Xu, D. Xue and C. Yan, Mater. Lett., 2005, 59, 2920.
17. D. Tiwari and S. Dunn, J. Mater. Sci., 2009, 44, 5063.
18. J. L. Giocondi and G. S. Rohrer, Chem. Mater., 2001, 13, 241.
19. S. V. Kalinin, D. A. Bonnell, T. Alvarez, X. Lei, Z. Hu, J. H. Ferris, Q. Zhang and S. Dunn, Nano Lett., 2002, 2, 586.
20. Y. Zhang, A. M. Schultz, P. A. Salvador and G. S. Rohrer, J. Mater. Chem., 2011, 21, 4168.
21. Z. G. Yi and J. H. Ye, J. Appl. Phys., 2009, 106, 074910.
22. M. Yang, X. Huang, S. Yan, Z. Li, T. Yu and Z. Zou, Mater. Chem. Phys., 2010, 121, 506.
23. Z. G. Yi and J. H. Ye, Appl. Phys. Lett., 2007, 91(254108), 1.
24. D. R. Liu, C. D. Wei, B. Xue, X. G. Zhang and Y. S. J. Jiang, J. Hazard. Mater., 2010, 182, 50.
25. H. Fu, S. Zhang, L. Zhang and Y. Zhu, Mater. Res. Bull., 2008, 43, 864.
26. A. Iwase, K. Saito and A. Kudo, Bull. Chem. Soc. Jpn., 2009, 82, 514.
27. Z. Li, Y. Wang, J. Liu, G. Chen, Y. Li and C. Zhou, Int. J. Hydrogen Energy, 2009, 34, 147.
28. X. Wang, H. Bai1, Y. Meng, Y. Zhao, C. Tang and Y. Gao, J. Nanosci. Nanotechnol., 2010, 10, 1788.
29. P. D. Kanhere, J. Zheng and Z. Chen, J. Phys. Chem. C, 2011, 115, 11846.
30. A. E. Morales, M. H. Zaldivar and U. Pal, Opt. Mater., 2006, 29, 100.
31. H. Lin, C. P. Huang, W. Li, C. Ni, S. I. Shah and Y. H. Tseng, Appl. Catal., B, 2006, 68, 1.
32. K. M. Parida, K. H. Reddy, S. Martha, D. P. Das and N. Biswal, Int. J. Hydrogen Energy, 2010, 35, 12161.
33. D. Li, J. Zheng, Z. Li, X. Fan, L. Liu and Z. Zou, Int. J. Photoenergy, 2007, 21680, 7,  DOI:10.1155/2007/21860.
34. C. C. Hu, Y. L. Lee and H. Teng, J. Mater. Chem., 2011, 21, 3824.
35. A. Kudo, K. Omori and H. Kato, J. Am. Chem. Soc., 1999, 121, 11459.
36. B. Y. Lee, S. H. Park, S. C. Lee, M. Kang, C. H. Park and S.-J. Choung, Korean J. Chem. Eng., 2003, 20, 812.
37. K. M. Parida, S. Martha, D. P. Das and N. Biswal, J. Mater. Chem., 2010, 20(7144), 29.
38. M. Maruyama, A. Iwase, H. Kato, A. Kudo and H. Onishi, J. Phys. Chem. C, 2009, 113, 13918.
39. Y. C. Lee, H. Teng, C. C. Hu and S. Y. Hu, Electrochem. Solid-State Lett., 2008, 11, P1.
40. S. Cabuk, H. Akkus and A. M. Mamedov, Phys. B, 2007, 394, 81.
41. R. Leonelli and J. L. Brebner, Phys. Rev. B, 1986, 33, 8649.
42. M. Aguilar and F. Agullo-Lopez, J. Appl. Phys., 1982, 53, 9009.
43. T. Hasegawa, M. Shirai and K. Tanaka, J. Lumin., 2000, 87, 1217.
44. V. A. Trepakov, V. S. Vikhnin, S. Kapphan, L. Jastrabik, J. Licher and P. P. Syrnikov, J. Lumin., 2000, 87, 1126.
45. V. V. Laguta, M. D. Glinchuk, I. P. Bykov, A. Cremona, P. Galinetto, E. Giulotto, L. Jastrabik and J. Rosa, J. Appl. Phys., 2003, 93, 6056.
46. S. E. Kapphan, A. I. Gubaev and V. S. Vikhnin, Phys. Status Solidi C, 2005, 2, 128.
47. Y. Lee, T. Watanabe, T. Takata, J. N. Kondo, M. Hara, M. Yoshimura and K. Domen, Chem. Mater., 2005, 17, 2422.
48. J. K. Reddy, B. Srinivas, V. Durga Kumari and M. Subrahmanyam, Chem. Cat. Chem., 2009, 1, 492.
49. R. S. Devan, W. D. Ho, C. H. Chen, H. W. Shiu, C. H. Ho, C. L. Cheng, S. Y. Wu, Y. Liou and Y. R. Ma, Nanotechnology, 2009, 20(445708), 5pp.
50. K. Chen, G. R. Yang, M. Nielsen, T. M. Lu and E. Rymaszewski, J. Appl. Phys. Lett., 1997, 70, 399.
51. E. Atanassova and D. Spassov, Appl. Surf. Sci., 1998, 135, 71.
52. O. Kerrec, D. Devilliers, H. Groult and P. Marcus, Mater. Sci. Eng., B, 1998, 55, 134.
53. E. Atanassova, G. Tyuliev, A. Paskaleva, D. Spassov and K. Kostov, Appl. Surf. Sci., 2004, 225, 86.
54. D. W. Hwang, J. Kim, T. J. Park and J. S. Lee, Catal. Lett., 2002, 80, 53.
55. K. M. Parida, A. Nashim and S. K. Mahanta, Dalton Trans., 2011, 40, 12839.
56. H. G. Kim, P. H. Borse, J.S. Jang, E. D. Jeong, O. S. Jung, Y. J. Suhd and J. S. Lee, Chem. Commun., 2009, 5889.
57. J. Jiang, X. Zhang, P. Sun and L. Zhang, J. Phys. Chem. C, 2011, 115, 20555.
58. G. Dai, J. Yu and G. Liu, J. Phys. Chem. C, 2011, 115, 7339.
59. H. Chen, L. Hu, X. Fang and L. Wu, Adv. Funct. Mater., 2012, 22, 1229.
60. B. Naik, S. Martha and K. M. Parida, Int. J. Hydrogen Energy, 2011, 36, 2794.
61. J. Zhu, S. Wang, J. Wang, D. Zhang and H. Li, Appl. Catal., B, 2011, 102, 120.
62. Z. Bian, J. Zhu, S. Wang, Y. Cao, X. Qian and H. Li, J. Phys. Chem. C, 2008, 112, 6258.

---