Solution superstructures: truncated cubeoctahedron structures of pyrogallol[4]arene nanoassemblies†
Harshita
Kumari
a,
Steven R.
Kline
b,
Drew A.
Fowler
a,
Andrew V.
Mossine
a,
Carol A.
Deakyne
*a and
Jerry L.
Atwood
*a
aDepartment of Chemistry, University of Missouri-Columbia, 601 S. College Avenue, Columbia, MO 65211, USA. E-mail: AtwoodJ@missouri.edu; Fax: +1 573 882 2754; Tel: +1 573 882 8374
bNIST Center for Neutron Research, Gaithersburg, MD 20899-6102, USA
First published on 5th November 2013
Abstract
Giant nanocapsules: the solution-phase structures of PgC1Ho and PgC3Ho have been investigated using in situ neutron scattering measurements. The SANS results show the presence of spherical nanoassemblies of radius 18.2 Å, which are larger than the previously reported metal-seamed PgC3 hexamers (radius = 10 Å). The spherical architectures conform to a truncated cubeoctahedron geometry, indicating formation of the first metal-containing pyrogallol[4]arene-based dodecameric nanoassemblies in solution.
Molecular recognition plays an important role in the self-assembly of biological and supramolecular architectures. Appropriate functionalization of supramolecular building blocks, including the introduction of moieties that selectively bind metal cations, can aid in the construction of useful supramolecular scaffolds. For example, the metalloprotein hemoglobin is an iron–porphyrin complex, whereas vitamin B12 or cyanocobaltamine is a cobalt–porphyrin complex. Supramolecular chemists have utilized the trivalent oxidation state of lanthanides (Ln) to build similar giant functional superstructures. The first complexes of lanthanides with calixarene were [Ln2(hexanion of p-tert–butylcalix[8]arene)2 (DMF)5]-nDMF, reported by Harrowfield and co-workers.1 Later, similar complexes of both homo- and heterodinuclear complexes with metals such as Eu, Gd, Ho and Yb were reported.2 More recently, Raston and co-workers have synthesized bifunctional lanthanide-containing nanocomposites with “koosh ball” structures that have both fluorescent and superparamagnetic properties.3 Ln-containing luminescent probes have proven useful in determining the metal ion sites in macrocyclic complexes and biological systems.4a,b For example, Eu(III) and Tb(III) have been utilized to determine the metal-to-metal ion distances in biological systems.2 Use of lanthanides as luminescent tags for clinical studies5 and sensitive fluoroimmunoassays4b are other important applications that have led chemists to synthesize and manipulate such complexes.
In 1999, we utilized p-sulfonatocalix[4]arene building blocks with varying ratios of pyridine-N-oxide and lanthanide ions to construct spherical and helical tubular structures.6 This study revealed the role of pyridine-N-oxide in governing the self-assembly of lanthanide-containing calix[4]arene nanoassemblies. Later, we shifted our focus to structurally related pyrogallol[4]arene metal-seamed architectures. C-Alkylpyrogallol[4]arenes (PgCn) have been shown to self-assemble as hydrogen-bonded or transition metal-seamed hexamers and dimers.7a,b These metal(II)-seamed hexameric and dimeric entities have a radius of ≈10 Å and ≈7 Å, respectively, in both the solid and solution phases.8a,b The spherical shapes of these nanocapsules in both phases demonstrate the robustness of transition metal-seamed pyrogallol[4]arene architectures.
We have successfully synthesized metal-seamed nanocapsules with several divalent transition metals, but thus far Ga(III) is the only trivalent species that has been successfully inserted into nanocapsular frameworks.7a,b The solid-state PgCnGa rugby ball and the hybrid PgCnGa–Zn spherical hexameric frameworks rearrange into toroidal architectures in solution.9 We note that, to date, amongst the metal-seamed PgCn hexamers, only the Ga-containing hexameric frameworks have been found to have different architectures in the two phases. However, tubular architectures can also change shape in solution.10a,b For example, solid-state H-bonded PgC1 nanotubes with ferrocene/pyrene guests rearrange to dimers in solution.10a,b,11 In contrast, tubes with iron in the framework are much more robust and are stable in solution.12 In short, solution-phase studies conducted thus far suggest that metal(II)-seamed PgCn spherical frameworks are more stable than metal(III)-seamed PgCn spherical and H-bonded PgCn tubular nanoassemblies in solution.8a,b,9 On the contrary, lanthanides have been shown to chelate well with phenolic derivatives.13 Also, the ionic radius of a trivalent Ln metal center is widely different from that of a Ga(III) center. For example, the ionic radius of Ga3+ is ≈76 pm whereas that of Ho3+ or Ce3+ is ≈104 pm and 115 pm respectively.14 The current study focuses on utilizing the pyrogallol[4]arene macrocycle as a ligand for trivalent Ln cations in an effort to form large supramolecular frameworks. Specifically, we began by investigating the solution structures of holmium(III)-containing C-methylpyrogallol[4]arene (PgC1Ho) and C-propylpyrogallol[4]arene (PgC3Ho) assemblies.
Samples were prepared by mixing 1 equiv. of PgCn to 16 equiv. of Ho(NO3)3 and 8 equiv. of pyridine in an acetonitrile![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) water (20
water (20![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1) mixture (ESI†). Note that the higher amount of Ho(NO3)3 is added to ensure the absence of any unreacted macrocycle. The pyridine plays an important role in the formation of capsular and tubular frameworks by deprotonating the pyrogallol hydroxyls.12 The prepared mixture yielded a dark brown precipitate, as opposed to the white precipitate of H-bonded frameworks,15a,b indicating formation of a PgCnHo assembly, which proved resistant to crystallization using vapour diffusion, solvothermal, or solvent evaporation. Thus, structures were investigated in solution using small-angle neutron scattering (SANS). The d6-DMSO-solubilized PgC1Ho (3% mass fraction) and PgC3Ho (3% mass fraction) were measured on the NG7 30m SANS instrument at the NIST Center for Neutron Research (NCNR) in Gaithersburg, MD16 and analyzed with IGOR Pro software.17 A 3% mass fraction was used to ensure minimal precipitation while maintaining sufficient concentration in solution for sufficient scattering.
1) mixture (ESI†). Note that the higher amount of Ho(NO3)3 is added to ensure the absence of any unreacted macrocycle. The pyridine plays an important role in the formation of capsular and tubular frameworks by deprotonating the pyrogallol hydroxyls.12 The prepared mixture yielded a dark brown precipitate, as opposed to the white precipitate of H-bonded frameworks,15a,b indicating formation of a PgCnHo assembly, which proved resistant to crystallization using vapour diffusion, solvothermal, or solvent evaporation. Thus, structures were investigated in solution using small-angle neutron scattering (SANS). The d6-DMSO-solubilized PgC1Ho (3% mass fraction) and PgC3Ho (3% mass fraction) were measured on the NG7 30m SANS instrument at the NIST Center for Neutron Research (NCNR) in Gaithersburg, MD16 and analyzed with IGOR Pro software.17 A 3% mass fraction was used to ensure minimal precipitation while maintaining sufficient concentration in solution for sufficient scattering.
Combining ratios of PgCn to metals of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 and 1
2 and 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4 have been observed for previously reported nanocapsules;7a,b,8a,18 thus, molecular formulas and neutron scattering length densities (SLDs) were calculated for both ratios. The SLDs were calculated for PgC1HoL (Ho4n/2n((L)4n/2n)(C32H30O12)n) and PgC3HoL (Ho4n/2n((L)4n/2n)(C40H48O12)n) as a hexamer, an octamer, a decamer, and a dodecamer. Here, L is nitrate, pyridine or DMSO, as these are the only species that can act as ligands in this system. Because the combining ratio of metal to pyrogallol[4]arene is assumed identical for the four polyhedra, the SLD values were unaffected by the number of combining units (6, 8, 10 or 12). The curved as opposed to flat shape of the scattering plot for both PgC1Ho and PgC3Ho indicates the presence of self-assembled frameworks (Fig. 1 and ESI†). Fits to a variety of structural models, namely core–shell, cylinder, ellipsoid and bimodal Schulz sphere, yielded poor results for both nanoassemblies (ESI†). The poor fit to a bimodal Schulz sphere model, which takes into account multiple spheres in solution, indicates the presence of a single species in solution. Irrespective of the PgCn
4 have been observed for previously reported nanocapsules;7a,b,8a,18 thus, molecular formulas and neutron scattering length densities (SLDs) were calculated for both ratios. The SLDs were calculated for PgC1HoL (Ho4n/2n((L)4n/2n)(C32H30O12)n) and PgC3HoL (Ho4n/2n((L)4n/2n)(C40H48O12)n) as a hexamer, an octamer, a decamer, and a dodecamer. Here, L is nitrate, pyridine or DMSO, as these are the only species that can act as ligands in this system. Because the combining ratio of metal to pyrogallol[4]arene is assumed identical for the four polyhedra, the SLD values were unaffected by the number of combining units (6, 8, 10 or 12). The curved as opposed to flat shape of the scattering plot for both PgC1Ho and PgC3Ho indicates the presence of self-assembled frameworks (Fig. 1 and ESI†). Fits to a variety of structural models, namely core–shell, cylinder, ellipsoid and bimodal Schulz sphere, yielded poor results for both nanoassemblies (ESI†). The poor fit to a bimodal Schulz sphere model, which takes into account multiple spheres in solution, indicates the presence of a single species in solution. Irrespective of the PgCn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ho ratio, alkyl tail length, or type of ligand selected, the SANS data was best fitted to a Schulz sphere model (ESI†). SANS data at q < 0.02 Å−1 likely arises from undissolved precipitate in the sample and is excluded from the analysis of the capsule dimensions.
Ho ratio, alkyl tail length, or type of ligand selected, the SANS data was best fitted to a Schulz sphere model (ESI†). SANS data at q < 0.02 Å−1 likely arises from undissolved precipitate in the sample and is excluded from the analysis of the capsule dimensions.
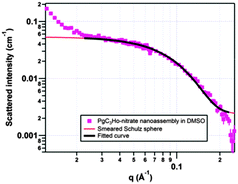 | ||
| Fig. 1 Schulz sphere fit for PgC3Ho (pink) in DMSO. Error bars on the SANS data represent one standard deviation. | ||
For all ligands, the SLD values and volume fractions obtained with a PgCn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ho ratio of 1
Ho ratio of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 differ by about 10% from those obtained with a ratio of 1
2 differ by about 10% from those obtained with a ratio of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4, leading to essentially identical diameters for the two ratios. Additionally, for both PgCn
4, leading to essentially identical diameters for the two ratios. Additionally, for both PgCn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ho ratios, changing the ligand does not change the overall radius of the sphere. For these reasons, only the data for the 1
Ho ratios, changing the ligand does not change the overall radius of the sphere. For these reasons, only the data for the 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 4 ratio and the nitrate ligand are reported below. We obtained an SLD of 2.03 × 10−6 Å−2 and 1.8 × 10−6 Å−2 for (PgC1)nHo4n(NO3)4n and (PgC3)nHo4n(NO3)4n, respectively. The Schulz sphere fit for (PgC1)nHo4n(NO3)4n and (PgC3)nHo4n(NO3)4n yielded a radius of (18.1 ± 0.5) Å and (18.2 ± 0.9) Å, and the chi-squared value of 1.6 and 1.4, respectively, indicating good quality fits. The radius obtained from SANS is orientationally averaged, hence, the difference in the length of the short alkyl side chains (C1 → C3) is too small of a fraction of the overall size of the capsule to be reflected in the fitted radius. Similar results were found when PgC1⊂ferrocene and PgC3Ni dimers were compared.8a,11 In contrast, the much longer alkyl chains of PgCnCu (n = 3, 6, 9, 11, 13, 17)8b,19 do lead to distinct differences in the overall radius of the hexamers.
4 ratio and the nitrate ligand are reported below. We obtained an SLD of 2.03 × 10−6 Å−2 and 1.8 × 10−6 Å−2 for (PgC1)nHo4n(NO3)4n and (PgC3)nHo4n(NO3)4n, respectively. The Schulz sphere fit for (PgC1)nHo4n(NO3)4n and (PgC3)nHo4n(NO3)4n yielded a radius of (18.1 ± 0.5) Å and (18.2 ± 0.9) Å, and the chi-squared value of 1.6 and 1.4, respectively, indicating good quality fits. The radius obtained from SANS is orientationally averaged, hence, the difference in the length of the short alkyl side chains (C1 → C3) is too small of a fraction of the overall size of the capsule to be reflected in the fitted radius. Similar results were found when PgC1⊂ferrocene and PgC3Ni dimers were compared.8a,11 In contrast, the much longer alkyl chains of PgCnCu (n = 3, 6, 9, 11, 13, 17)8b,19 do lead to distinct differences in the overall radius of the hexamers.
The 36 Å diameter spheres of PgCnHoNO3 (n = 1, 3) are larger than the earlier reported 20 Å diameter transition metal (Cu/Ni)-seamed hexameric spheres.8a Our only other reported example of a nanoassembly larger than the hexameric spheres is a H-bonded sphere (d = 32 Å) of the structurally related insulin-templated C-methylresorcin[4]arene nanocapsule.20 In the absence of templation, dimers are the only H-bonded nanocapsules that we have observed in solution.11 These observations provide additional support that Ho seams the 36 Å nanocapsular framework.
Large supramolecular spherical nanoassemblies often conform to Archimedean solids. For example, the geometries of the hexameric H-bonded (PgCn)6 and metal-seamed (PgCn)6M24 nanocapsules are similar to that of a cubeoctahedron, wherein each metal center resides on the vertices of a truncated cube that has six octagonal and eight triangular faces. Similarly, we expect the PgCnHo solution-phase spheres to conform to an Archimedean solid. In the previously reported cubeoctahedron structures of the PgCnM hexamers, each square face is occupied by a macrocycle and the squares are separated by metal triads. Applying this information limited the choices of Archimedean solids to truncated icosadodecahedron, snub cube, truncated cubeoctahedron, truncated octahedron, and cubeoctahedron, i.e. those without adjacent squares. The experimentally obtained volume of the PgCnHo spheres was then compared with the volumes of these Archimedean solids with side dimensions equivalent to the diameter across the upper rim of pyrogallol[4]arene.
In observed pyrogallol[4]arene macrocycles, the distance between the center –OH groups of opposing pyrogallols ranges from 7 Å to 11 Å.8a,21a,b Pinched cone arrangements of the PgCn bowls are common for bilayers and Ga-seamed nanocapsules, whereas perfect cone/symmetric arrangements are common for transition metal-seamed nanocapsules. Interestingly, a perfect cone arrangement is found when all four of the pyrogallols of a given arene are involved in metal coordination, e.g. for PgCnCu/Zn/Ni.8a,18,21b In contrast, the bowl is pinched for the PgCnGa hexamers, wherein two of the pyrogallols of a given arene are involved in metal coordination and two are involved in water coordination. The (7 to 11) Å range in edge length was reduced by considering the edges of the faces other than the squares. These edges connect the various components of PgCnHo and, in the selected Archimedean solids, must be equal in length to that of the edges of the square faces. Possible linkages between the components include Ho⋯O(H)–Pg and Ho–L⋯O(H)–Pg interactions. Nitrate was chosen as the ligand L to help balance the charge on the metal and to provide stronger pyrogallol–L (OH⋯ONO2−) interactions. A pyridine or DMSO ligand positioned along an edge would provide much weaker (H)O⋯HC interactions, but either of them could be an external metal ligand. Reported single-crystal XRD structural data for the [Ho(NO3)3(C12H8N2)2] compound22 and [Ho2(C8H7O3)6(C10H8N2)2(H2O)2]·H2O dinuclear complex23 reveal Ho⋯N, Ho–O and N–O distances of ≈2.9 Å, ≈2.4 Å, and ≈1.3 Å, respectively. The accepted O⋯H distance for O⋯H–O H-bonds ranges from 1.5 Å to 2.6 Å.24a–c These bond lengths allow the possibility of a (H)O–M⋯NO3−⋯O(H) (pyrogallol phenolate-to-metal-to-nitrate-to-pyrogallol hydroxy) coordination motif along the edges of the non-square faces. The total edge length of 8.1 Å to 9.2 Å associated with this coordination motif led to the use of 8 Å, 8.5 Å and 9 Å edge lengths to determine the calculated volumes of the selected Archimedean solids.
Integrating the three distances into the truncated icosadodecahedron, snub cube, truncated octahedron, and cubeoctahedron polyhedra yields volumes that are significantly too large or too small compared to the SANS experimental results. The best agreement with experiment (V = 4/3πr3 = 25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 263 Å3) was obtained for a truncated cubeoctahedron of edge length 8.5 Å (
263 Å3) was obtained for a truncated cubeoctahedron of edge length 8.5 Å (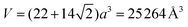 ). Each of the 12 square faces of the truncated cubeoctahedron contains a macrocycle, each of the 8 hexagon faces contains 3 metal centers, and each of the 6 octagon faces contains 4 metal centers (Fig. 2). Alternate 8.5 Å sides consist of either (H)O⋯Ho⋯(NO3)3⋯O(H) coordination networks or pyrogallol[4]arenes. Although no assumption was made with respect to the PgCn
). Each of the 12 square faces of the truncated cubeoctahedron contains a macrocycle, each of the 8 hexagon faces contains 3 metal centers, and each of the 6 octagon faces contains 4 metal centers (Fig. 2). Alternate 8.5 Å sides consist of either (H)O⋯Ho⋯(NO3)3⋯O(H) coordination networks or pyrogallol[4]arenes. Although no assumption was made with respect to the PgCn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) M ratio in selecting the Archimedean solids, with its 12 pyrogallol[4]arene units and 48 metal centers, the best model for the solution-phase structure of PgCnHo has a PgCn
M ratio in selecting the Archimedean solids, with its 12 pyrogallol[4]arene units and 48 metal centers, the best model for the solution-phase structure of PgCnHo has a PgCn![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) M ratio consistent with most previously reported nanocapsules.7a,b,8a,18
M ratio consistent with most previously reported nanocapsules.7a,b,8a,18
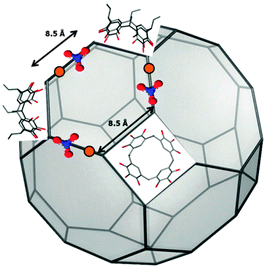 | ||
| Fig. 2 Pictorial representation of the truncated cubeoctahedron geometries of holmium-containing pyrogallol[4]arene nanoassemblies. | ||
In conclusion, we have studied the solution-phase superstructures of the holmium-seamed pyrogallol[4]arene-based PgC1Ho and PgC3Ho nanoassemblies. The SANS data for both nanoassemblies fit to a Schulz sphere model, with a radius of ≈18 Å for both PgC1Ho and PgC3Ho in DMSO. The size of PgCnHo is larger than that of earlier reported H-bonded and metal-seamed pyrogallol[4]arene nanocapsules.8a We suggest that the geometry of PgCnHo is a truncated cubeoctahedron that consists of 12 PgCn units (squares) and 48 metal centers (8 hexagon and 6 octagon faces). This is the first reported example of a dodecameric PgCn-based nanoassembly in solution.
We thank NSF for support of this work (J.L.A.). This work utilized facilities supported in part by the NSF under Agreement No. DMR-0944772 (S.R.K.). Certain trade names and company products are identified to adequately specify the experimental procedure. In no case does such identification imply recommendation or endorsement by the NIST, nor does it imply that the products are necessarily best for the purpose.
Notes and references
- B. M. Furphy, J. M. Harrowfield, D. L. Kepert, B. W. Skelton, A. H. White and F. R. Wilner, Inorg. Chem., 1987, 26, 4231–4236 CrossRef CAS.
- J. C. G. Bunzli, P. Froidevaux and J. M. Harrowfield, Inorg. Chem., 1993, 32, 3306–3311 CrossRef CAS.
- J. Fang, M. Saunders, Y. Guo, G. Lu, C. L. Raston and K. S. Iyer, Chem. Commun., 2010, 46, 3074–3076 RSC.
- (a) W. D. Horrocks, Jr. and M. Albin, Prog. Inorg. Chem., 1984, 31, 1–104 CrossRef; (b) Lanthanide Probes in Life, Chemical and Earth Sciences: Theory and Practice, ed. J. C. G. Buenzli and G. R. Choppin, 1989 Search PubMed.
- C. H. Evans, Biochem. of the Elements, Vol. 8: Biochem. of the Lanthanides, 1990 Search PubMed.
- G. W. Orr, L. J. Barbour and J. L. Atwood, Science, 1999, 285, 1049–1052 CrossRef CAS.
- (a) S. J. Dalgarno, N. P. Power and J. L. Atwood, Coord. Chem. Rev., 2008, 252, 825–841 CrossRef CAS PubMed; (b) P. Jin, S. J. Dalgarno and J. L. Atwood, Coord. Chem. Rev., 2010, 254, 1760–1768 CrossRef CAS PubMed.
- (a) H. Kumari, A. V. Mossine, S. R. Kline, C. L. Dennis, D. A. Fowler, S. J. Teat, C. L. Barnes, C. A. Deakyne and J. L. Atwood, Angew. Chem., Int. Ed., 2012, 51, 1452–1454 CrossRef CAS PubMed; (b) H. Kumari, S. R. Kline, N. J. Schuster and J. L. Atwood, Chem. Commun., 2011, 47, 12298 RSC.
- H. Kumari, S. R. Kline, W. G. Wycoff, R. L. Paul, A. V. Mossine, C. A. Deakyne and J. L. Atwood, Angew. Chem., Int. Ed., 2012, 51, 5086–5091 CrossRef CAS PubMed.
- (a) A. V. Mossine, H. Kumari, D. A. Fowler, A. Shih, S. R. Kline, C. L. Barnes and J. L. Atwood, Chem.–Eur. J., 2012, 18, 10258–10260 CrossRef CAS PubMed; (b) H. Kumari, S. R. Kline, W. Wycoff and J. L. Atwood, Small, 2012, 8, 3321–3325 CrossRef CAS PubMed.
- A. V. Mossine, H. Kumari, D. A. Fowler, A. K. Maerz, S. R. Kline, C. L. Barnes and J. L. Atwood, Isr. J. Chem., 2011, 51, 840–842 CrossRef CAS.
- H. Kumari, C. L. Dennis, A. V. Mossine, C. A. Deakyne and J. L. Atwood, ACS Nano, 2012, 6, 272–275 CrossRef CAS PubMed.
- P. I. Binda, E. E. Delbridge, H. B. Abrahamson and B. W. Skelton, Dalton Trans., 2009, 2777–2787 RSC.
- R. D. Shannon, Acta Crystallogr., Sect. A, 1976, 32, 751–767 CrossRef.
- (a) J. L. Atwood, L. J. Barbour and A. Jerga, Chem. Commun., 2001, 2376–2377 RSC; (b) J. L. Atwood, L. J. Barbour and A. Jerga, J. Supramol. Chem., 2002, 1, 131–134 CrossRef.
- C. J. Glinka, J. G. Barker, B. Hammouda, S. Krueger, J. J. Moyer and W. J. Orts, J. Appl. Crystallogr., 1998, 31, 430–445 CrossRef CAS.
- S. R. Kline, J. Appl. Crystallogr., 2006, 39, 895–900 CrossRef CAS.
- N. P. Power, S. J. Dalgarno and J. L. Atwood, New J. Chem., 2007, 31, 17–20 RSC.
- H. Kumari, S. R. Kline, N. J. Schuster, C. L. Barnes and J. L. Atwood, J. Am. Chem. Soc., 2011, 133, 18102–18105 CrossRef CAS PubMed.
- H. Kumari, S. R. Kline and J. L. Atwood, Chem. Commun., 2012, 48, 3599 RSC.
- (a) H. Kumari, L. Erra, P. Bhatt, A. Webb, J. E. Adams, C. A. Deakyne, L. J. Barbour and J. L. Atwood, J. Am. Chem. Soc., 2013 DOI:10.1021/ja406643b; (b) R. M. McKinlay, G. W. V. Cave and J. L. Atwood, Proc. Natl. Acad. Sci. U. S. A., 2005, 102, 5944–5948 CrossRef CAS PubMed.
- E. Kusrini, M. I. Saleh, R. Kia and H.-K. Fun, Acta Crystallogr., Sect. E, 2008, 64, m1014–m1015 CAS.
- J.-L. Liu, J.-F. Liu and G.-L. Zhao, Acta Crystallogr., Sect. E, 2011, 67, m191–m192 CAS.
- (a) I. K. McDonald and J. M. Thornton, J. Mol. Biol., 1994, 238, 777–793 CrossRef CAS PubMed; (b) I. Y. Torshin, I. T. Weber and R. W. Harrison, Protein Eng., Des. Sel., 2002, 15, 359–363 CrossRef CAS PubMed; (c) G. R. D. T. Steiner, The Weak Hydrogen Bond: Applications to Structural Chemistry and Biology, Oxford Univ. Press, New York, NY, 1999 Search PubMed.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c3cc47029f |
| This journal is © The Royal Society of Chemistry 2014 |
