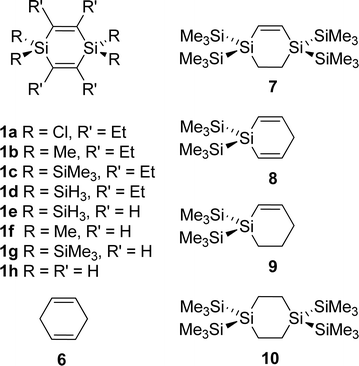
1,4-Disilacyclohexa-2,5-diene: a molecular building block that allows for remarkably strong neutral cyclic cross-hyperconjugation

Abstract

2,3,5,6-Tetraethyl-1,4-disilacyclohexa-2,5-dienes with either four chloro (1a), methyl (1b), or trimethylsilyl (TMS) (1c) substituents at the two silicon atoms were examined in an effort to design rigid compounds with strong neutral cross-hyperconjugation between π- and σ-bonded molecular segments arranged into a cycle. Remarkable variations in the lowest electronic excitation energies, lowest ionization energies, and the first oxidation potentials were observed upon change of substituents, as determined by gas phase ultraviolet (UV) absorption spectroscopy, ultraviolet photoelectron spectroscopy (UPS), and cyclic voltammetry. A particularly strong neutral cyclic cross-hyperconjugation was observed in 1c. Its lowest electron binding energy (7.1 eV) is distinctly different from that of 1b (8.5 eV). Molecular orbital analysis reveals a stronger interaction between filled π(C=C) and π(SiR₂) group orbitals in 1c than in 1a and 1b. The energy shift in the highest occupied molecular orbital is also reflected in the first oxidation potentials as observed in the cyclic voltammograms of the respective compounds (1.47, 0.88, and 0.46 V for 1a, 1b and 1c, respectively). Furthermore, 1,4-disilacyclohexadiene 1c absorbs strongly at 273 nm (4.55 eV), whereas 1a and 1b have no symmetry allowed excitations above 215 nm (below 5.77 eV). Thus, suitably substituted 1,4-disilacyclohexa-2,5-dienes could represent novel building blocks for the design of larger cross-hyperconjugated molecules as alternatives to traditional purely cross-π-conjugated analogues, and could allow for design of molecules with properties that are not accessible to those that are exclusively π-conjugated.

---

Introduction

π-Conjugation and σ-conjugation are well-established concepts in chemistry since many decades and compounds containing these conjugation topologies have been investigated for purely fundamental reasons as well as in the course of development in various applied areas.^1–3 Conjugation in the classical sense is the stabilizing interaction between local orbitals of π-symmetry and occurs in molecules with alternating single and multiple bonds.⁴ π-Conjugation can further be divided into linear- or cross-conjugation depending on the connectivity in the bond alternating path. While a linearly conjugated compound provides strong delocalization across the entire π-bonded path, a cross-conjugated system only provides strong delocalization up until the crossing point.⁵ However, stabilizing conjugative interactions between adjacent orbitals are not restricted to π-bonded compounds, and σ-conjugation is observed in heavy alkanes, such as oligo- and polysilanes.² Indeed, Mulliken and co-workers early emphasized that “the differences in conjugating power” between unsaturated and saturated groups are “quantitative rather than qualitative”.⁶ Yet, the combination of σ- and π-conjugation topologies into a strong σ/π-conjugation, or rather hyperconjugation, in neutral compounds is less explored,⁷ and even less exploited in applications. Herein, we report on a joint theoretical and experimental study of a compound class, the 1,4-disilacyclohexa-2,5-dienes (1, Fig. 1), in which π- and σ-bonded molecular segments are joined into a rigid cyclic structure and where the segments interact in a cross-hyperconjugated manner.⁸ These species could complement 1-silacyclopenta-2,4-dienes (siloles) which are extensively investigated organosilicon compounds of which the oligomers and polymers are used in organic electronics.^9,10

Fig. 1 The 1,4-disilacyclohexa-2,5-dienes (1) examined herein, and an example (2) of the previously investigated neutral hydrocarbons of Sekiguchi, Lee and co-workers which display significant neutral hyperconjugative σ/π-interaction (ref. 11).

Hyperconjugation is exceptionally rare in neutral pure hydrocarbons, even though such a conjugation is observed in the recent perfluoroaryltetrahedranes of Sekiguchi, Lee and co-workers (2, Fig. 1) composed of one or two strained tetrahedranes which interact with an adjacent phenyl group.¹¹ Strong interaction between σ- and π-bonded molecular segments is also observed in poly-1,1-siloles in which the monomers are connected via the Si atoms (3; Fig. 2) as these display significant coupling between the localized π*-orbitals of the diene segments and the delocalized σ*-orbitals (cf., linear combinations of local π(Si(SiR₃)₂ orbitals)) of the Si backbone, respectively.¹² However, oligomeric and polymeric compounds composed of different segments which are either σ- or π-bonded, such as the linearly linked silanylene–thiophene oligomers 4, have drawbacks because the rotational flexibility about the connecting single bonds affects the σ- and π-orbital overlap and, thus, the conjugation strength.^13–19 It should therefore be important to design neutral molecules and repeat units in oligomers/polymers with rigid structures that internally display strong hyperconjugative interactions.

Fig. 2 Earlier investigated oligomers and polymers with interacting σ- and π-bonded segments; the oligo/polysilole (3) and poly(silanylene-thiophene) (4), respectively (ref. 9–19).

Recently, we examined the electronic structure of bis(phenylethynyl)methanes and silanes in dependence of the substituents at the central C or Si atom, and found that when the substituents at the central atom are σ-electron donor groups such as the trimethylsilyl group the electronic structures of these compounds resemble that of a regular cross-π-conjugated hydrocarbon.^20,21 Such molecules have two hyperconjugated paths which are united by the π-symmetric group orbitals of the central ER₂ moiety having the same function as the π-orbitals in a geminally connected C=C double bond. These compounds can therefore be labelled as cross-hyperconjugated. Indeed, hyperconjugative interaction between ethylene fragments bonded to the silicon atom in dimethyldivinylsilane has been shown earlier with photoelectron spectroscopy,²² however, a SiMe₂ segment only provides for very weak interaction.²⁰ We now utilize these findings and investigate 1, a rigid cyclic structure composed of two ER₂ moieties connected via two ethenylene groups and first described in 1963 by West and Bailey,²³ as a molecule with potentially optimal hyperconjugative interaction. The all-carbon analogue, cyclohexa-1,4-diene, has previously been studied theoretically as well as experimentally, both in the ground and the excited states. A weak hyperconjugation has been demonstrated in these earlier studies and attributed to π(CH₂) group orbitals composed of the C–H σ- and σ*-bond orbitals interacting with the π-orbitals of the C=C double bond.²⁴ Moreover, from UPS studies of the tetrakis(TMS) substituted cyclohexa-1,4-diene Bock and Kaim found a significant shift of the HOMO to a higher electron binding energy when compared to the parent cyclo-1,4-hexadiene.²⁵

The silole ring has six electrons in orbitals of π-character; four in the π-orbitals of the 1,3-butadiene segment and two in the π(SiR₂) group orbital. Yet, despite the 6π-electron systems, the geometries of various siloles indicate that cyclic conjugation is modest.^26,27 In this context it is particularly noteworthy that the electronic structure of siloles is valence isolobal to that of pentafulvenes (Fig. 3), cross-π-conjugated hydrocarbons in which the optical properties and degree of aromaticity can be varied extensively through substitution.^28–31

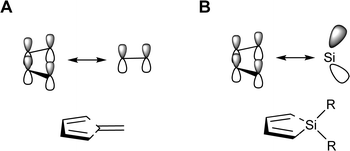Fig. 3 Schematic drawings of the valence isolobal analogy of the interactions between the π-symmetric basis orbitals of (A) pentafulvene and (B) silole, respectively.

Our hypothesis is now that 1 is a good template for the design of neutral cyclic cross-hyperconjugated molecules. In a similar way that the π-orbital interaction in siloles is valence isolobal with that of pentafulvenes, the π-orbital interaction in 1 is isolobal to that between the π-orbitals of the endocyclic and the exocyclic C=C double bonds in para-xylylene (5, Fig. 4). Para-xylylene is a cyclic cross-π-conjugated compound,²⁴ and by analogy, properly substituted derivatives of 1 are potentially cyclic cross-hyperconjugated. The question thus arises whether 1,4-disilacyclohexa-2,5-diene can provide a template for the design of optically and electronically useful compounds.

Fig. 4 Schematic drawings of the valence isolobal analogy of the interactions between the π-symmetric basis orbitals of (A) para-xylylene (5) and (B) 1,4-disilacyclohexa-2,5-diene (1), respectively.

The earlier studies on the parent 1,4-disilacyclohexa-2,5-diene (1) have focused on synthesis rather than spectroscopy.^32–35 In the present study, 1,4-disilacyclohexa-2,5-dienes with either Cl (1a), Me (1b), or SiMe₃ (1c, TMS) substituents at the Si atoms and ethyl substituents at the sp² hybridized C atoms (Fig. 1) were synthesized and examined by X-ray crystallography, UV photoelectron spectroscopy (UPS), cyclic voltammetry and gas phase UV absorption spectroscopy. As a reference for an essentially non-cross-hyperconjugated compound, the all-carbon analogue, i.e., cyclohexa-1,4-diene (6), was also examined by gas phase UV absorption spectroscopy. The computational part of our investigation was also extended to a larger set of 1,4-disilacyclohex-2-enes and related compounds (Fig. 5) so as to reveal the effect of unifying two cross-hyperconjugated moieties into a cycle as compared to ordinary linear hyperconjugation and acyclic cross-hyperconjugation, the latter exemplified by the bis(ethynyl)silanes.^20,21 Properties, such as electronic transitions, ionization energies, and how these are coupled to the substitution pattern of the different compounds, are analysed and discussed. Our aim is to deduce a new monomer unit which in an optimal manner combines σ- and π-bonded segments into a rigid and strongly cross-hyperconjugated cyclic framework. This unit could represent a novel structural motif to be used in oligomers and polymers for various optical and/or electronic applications, similar to the silole unit.

Fig. 5 The substituted 1,4-disilacyclohexa-2,5-dienes (1a–h), the all-carbon reference cyclohexa-1,4-diene (6), and model compounds (7–10) explored computationally to examine the extent of cyclic cross-hyperconjugation.

Results and discussion

The goal of the presently reported investigation is to find ways to maximize the neutral cyclic cross-hyperconjugative interaction between the local π(C=C) and π(ER₂) orbitals in 1,4-disilacyclohexa-2,5-dienes 1a–c. For that reason we first discuss the qualitative molecular orbital (MO) diagram and the calculated MOs before progressing to the experimental data. At the end we compare, through quantum chemical calculations, the strongly cross-hyperconjugated 1c with several model compounds (7–10) which allows for an evaluation of the cyclic cross-hyperconjugation in 1c as compared to hyperconjugation and acyclic cross-hyperconjugation. We also discuss what effect the rigidification in 1, when compared to the previously studied bis(phenylethynyl)methanes and silanes,^20,21 has on the electronic structure and how this could be exploited in future molecular design.

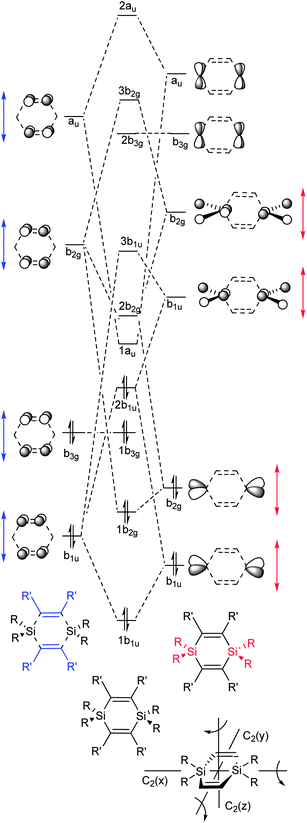
Molecular orbitals

Fig. 6 shows the MOs of π-symmetry and considers a D_2h symmetric 1,4-disilacyclohexa-2,5-diene with substituents R and R′ at the silicon and carbon atoms, respectively. These MOs are derived through combinations of suitable local π-orbitals of the two C=C bonds combined into a 2 × (C=C) fragment (marked blue in Fig. 6) and the two SiR₂ moieties of a 2 × (SiR₂) fragment (marked red). The interaction includes the symmetry-adapted in-phase and out-of-phase combinations of the four π_CC and π*_CC orbitals of 2 × (C=C) with the symmetry-adapted in-phase and out-of-phase combinations of the four π(SiR₂) and π*(SiR₂) orbitals of the 2 × (SiR₂) fragment. For further details on how this diagram was constructed and discussion about the unoccupied orbitals, see the ESI.†

Fig. 6 Qualitative molecular orbital (MO) diagram of D_2h symmetric 1,4-disilacyclohexa-2,5-diene with the lowest few occupied and unoccupied MOs of π-character constructed from suitable fragment orbitals. Red arrows indicate changes in fragment orbital energies in dependence of substituents R and blue arrows indicate changes of substituents R′. The orbitals are labelled in accordance with the irreducible representations of the D_2h point group. The three C₂ rotational axes are arranged as shown in the structure in the lower right corner.

From Fig. 6 it is clear that the energy of MO 2b_1u will vary depending on the energy of the b_1u group orbital of 2 × (SiR₂) relative to that of the b_1u group orbital of 2 × (C=C). Thus, the less electronegative R becomes, the higher the energy of this MO will become. The variation in orbital interaction strength can be recognized from the suitable π-symmetry orbitals of 3-hexene and H₂SiR₂. At the B3LYP/6-31G(d) level the π(C=C) of 3-hexene is located at −6.40 eV whereas the π(SiR₂) of H₂SiR₂ is found at −11.45 (R = Cl), −8.38 (R = Me), and −6.53 eV (R = TMS) (see ESI† for orbital plots). With R = TMS, 2b_1u can thus be expected to be of particularly high energy, and indeed, this orbital becomes HOMO for 1c (orbital 2b₁, Fig. 7). The 1b_3g MO, on the other hand, is not equally affected by the choice of the substituents at Si as it is primarily localized to the C=C bonds. This orbital should instead vary in energy upon a change of substituents R′.

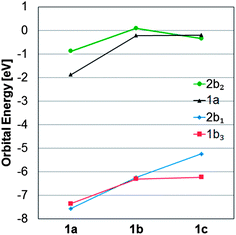Fig. 7 Energy variations of the two occupied frontier molecular orbitals 1b₃ and 2b₁ and the two unoccupied frontier orbitals 1a and 2b₂ of 1a–c calculated at the B3LYP/6-31G(d) level.

For 1a–c, the HOMO − 1, HOMO, LUMO, and LUMO + 1 correspond to either of the 1b_3g, 2b_1u, 1a_u and 2b_2g orbitals of Fig. 6, and as the symmetry is reduced from D_2h to D₂ the four MOs become 1b₃, 2b₁, 1a, and 2b₂. These four MOs of 1c are displayed in Fig. 8, and which orbital is HOMO (1b₃ or 2b₁) and which one is LUMO (1a or 2b₂) depends on R (Fig. 7). It is noteworthy that no occupied MO with σ-symmetry is found between the 2b₁ and 1b₃ orbitals for any of the three compounds. Furthermore, there is a striking similarity of the calculated MOs of 1c with those of para-xylylene (5, Fig. 8) as only LUMO and LUMO + 1 change place between the two compounds. Two further items can be noted in particular. First, HOMO of 1c (−5.24 eV) is nearly isoenergetic to the HOMO of 5 (−5.35 eV) at the B3LYP/6-31G(d) level. Secondly, the a-symmetric orbital which is LUMO + 1 in 5 can interact with the a-symmetric group orbital of 2 × (SiR₂) composed of the two 3d(Si) AOs of 1c. As a consequence, this MO is lowered in energy so that it becomes the LUMO of 1c.

Fig. 8 Frontier orbitals, orbital energies in eV, and orbital symmetries of 1c and para-xylylene (5), as given for the D₂ point group, calculated at the B3LYP/6-31G(d) level. The hydrogen atoms in 1c are omitted for clarity.

When going from 1a to 1b, all four MOs move up in energy, but the energy change is slightly larger for the occupied 2b₁ and the unoccupied 2b₂ than for 1b₃ and 1a, reflecting the fact that the first two MOs directly involve the substituents R. However, the most significant change occurs when going from 1b to 1c because a large split in the orbital energies of 1b₃ and 2b₁ is observed; the latter is raised from −6.24 to −5.24 eV while 1b₃ merely changes from −6.31 to −6.23 eV. It can furthermore be noted that 1b₃ has no 3d(Si) AO contribution from 2 × (SiR₂), in line with the qualitative MO-diagram of Fig. 6.

For LUMO, with a nodal plane coinciding with the SiR₂ plane, the variation in energy of this MO with R is the smallest among the frontier orbitals of Fig. 7.

Thus, the qualitative MO diagram agrees with the quantitative computations because the 2b_1u orbital (HOMO of 1b and 1c at the B3LYP level) changes in energy by a change of substituents R. In contrast, the LUMOs do not vary as extensively with R, and for this reason there are large differences in the HOMO-LUMO energy gap (Δε_H-L) between the three compounds, with values of 5.47 (1a), 6.03 (1b), and 4.89 eV (1c) at B3LYP/6-31G(d) level. Taking into account the earlier reported Δε_H-L of 6.43 eV for the parent species at the same level of computation,³⁶ one may argue that the 1,4-disilacyclohexa-2,5-diene can be a highly valuable template for extensive variations in electronic and optical properties.

Synthesis

The synthesis of 1a is based on Jung's procedure,³⁷ and subsequent addition of MeLi or TMSCl and lithium yielded 1b and 1c, respectively (Scheme 1).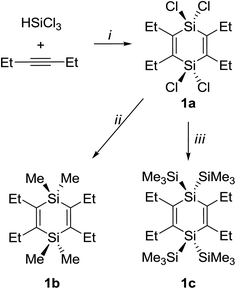

Scheme 1 Reagents and conditions: (i) Bu₄PCl, 180 °C (sealed tube reaction), 10 h, 75%; (ii) MeLi, Et₂O, −78 °C to r.t., 12 h, 68%; (iii) TMSCl, Li, THF, −78 °C to r.t., 13 h, 71%.

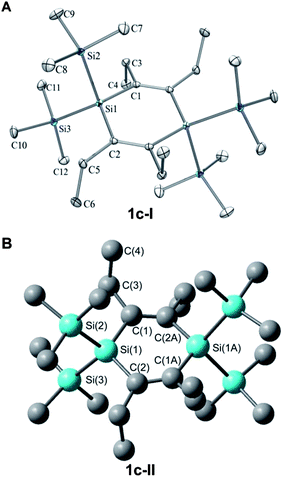
Geometric structure

Here we primarily discuss the geometrical parameters of 1c. The geometries of 1a and 1b are discussed in the ESI.†

The crystal structure of 1c reveals that this 1,4-disilacyclohexa-2,5-diene adopts a slight twist-chair conformer (1c-I, Fig. 9) with C_i symmetry and C–Si–C=C dihedral angles within the ring of approximately 13°. B3LYP/6-31G(d) calculations show that the non-planar structure is a result of the steric bulk as replacement of the Et groups by smaller Me groups leads from a D₂ to a D_2h symmetric molecule with a planar ring. These calculations reveal that a second conformer with the Et groups arranged in an up-down-up-down fashion (conformer 1c-II, Fig. 9) is essentially isoenergetic with 1c-I when based on the calculated free energy at 298 K. However, M06-2X/6-311G(d), a dispersion corrected DFT method suitable to handle sterically congested molecules, indicates that conformer 1c-II is 2.0 kcal mol⁻¹ higher in energy than a C₁ symmetric conformer of 1c-I (the C_i symmetric conformation of 1c-I is a second-order saddle point at 0.6 kcal mol⁻¹ higher energy). Yet, both conformers should be populated at the elevated temperatures of the gas phase UV absorption and UPS spectral measurements (up to 350 °C).

Fig. 9 (A) Crystal structure of 1c (conformer 1c-I) with the measured data (normal print), and the B3LYP/6-31G(d) calculated data (in parentheses normal print), and (B) the calculated geometry of the conformer 1c-II (lower structure, data in parentheses italics). Selected bond lengths (Å) and angles (deg), hydrogens omitted for clarity: Si(1)-C(1) 1.8845(13) (1.907, 1.905), Si(1)-Si(2) 2.3685(6) (2.410, 2.402), Si(1)-Si(3) 2.3690(6) (2.400, 2.402), C(1)-C(2A) 1.3456(18) (1.361, 1.361), C(1)-C(3) 1.5297(16) (1.533, 1.534), C(3)-C(4) 1.5307(19) (1.5421, 1.542), Si(2)-Si(1)-Si(3) 109.33(3) (110.04, 110.36), Si(1A)-Si(1)-Si(2) 140.33 (137.06, 124.82), Si(1A)-Si(1)-Si(3) 110.33 (112.90, 124.82), Si(2)-Si(1)-C(1) 113.66(4) (112.51, 111.28).

Overall, there is good agreement between the crystal structure and the calculated structure of 1c (selected bond distances and angles are given in the caption of Fig. 9). The only notable differences are the B3LYP/6-31G(d) bond lengths, which are slightly longer than the experimental ones, in line with earlier observations.³⁸

The important geometrical parameters for the evaluation of the potential cross-hyperconjugation are the C=C and Si–C bond lengths. A discussion of these bond lengths should be carried out in comparison with those of cross-π-conjugated para-xylylene 5. In the X-ray crystal structure of 1c-I the C=C double bonds (1.346 Å) are slightly longer than ordinary C=C double bonds (1.33 Å),³⁹ and the Si–C bond lengths (1.885 Å) of the ring are moderately elongated as compared to regular Si–C single bonds (1.87 Å).⁴⁰ The elongation of the C=C bonds partially stems from steric congestion between the substituents as revealed through comparisons between the 1,4-disilacyclohexa-2,5-dienes 1d–h (see ESI†). There is also a hyperconjugative component that impacts the geometry, and this is revealed through a comparison with 5. At the B3LYP/6-31G(d) level the C=C bond lengths of 1c are longer than those calculated for the cross-π-conjugated 5 (1.361 vs. 1.349 Å, respectively). Replacement of the ethyl groups (1g, Fig. 5) with hydrogen atoms leads to a shortening of the C=C bonds to 1.351 Å, i.e., essentially identical lengths as found for 5. In contrast, the two isolated C=C bonds in cyclohexa-1,4-diene 6 (1.335 Å) are significantly shorter than in 1g. The calculated C=C bonds in 1g are also longer than those of the parent 1,4-disilacyclohexa-2,5-diene (1h), which are 1.344 Å. Thus, the C=C bond elongations observed in 1c to some extent stem from cross-hyperconjugation. This observation is also true at higher levels of computations because CCSD/6-311G(d) calculations show that 6 has C=C bond lengths of 1.339 Å compared to 1,1,4,4-tetrasilyl substituted 1e at 1.355 Å and cross-π-conjugated 5 at 1.349 Å.

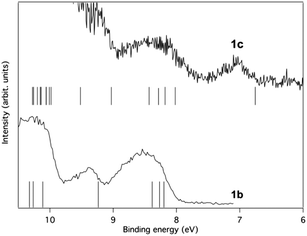
Photoelectron spectroscopy

The experimental verification of the substituent effect on the electronic structures of 1,4-disilacyclohexa-2,5-dienes was obtained through photoelectron and UV absorption spectroscopies, as well as through cyclic voltammetry. The cationic valence electronic states of 1b and 1c were studied by conventional photoelectron spectroscopy using HeIα radiation at hν = 21.22 eV for ionization. Interpretation of the spectra were made on the basis of outer valence Green's function (OVGF) calculations at the OVGF/6-311+G(d)//B3LYP/6-31G(d) level. The D₂ symmetric conformers 1b-II and 1c-II were used to facilitate rationalization in terms of qualitative MO-theory. The ionization energies of the C_i symmetric conformers I differ minutely, and tables which enable comparisons between conformers I and II are given in the ESI.†

With regard to 1b, the valence photoelectron spectrum shows a well-defined onset at 7.9 eV followed by broad rounded bands (Fig. 10). The computed energies group around the spectral features so that a rather good understanding is achievable despite the strong overlap of bands. The experimental and calculated energies for the lower states are summarized in Table 1 along with our interpretations (for a complete table see the ESI†). The first band with a peak maximum at 8.5 eV represents ionization from three different orbitals that are close in energy according to the computations. The two lowest of these are π-type orbitals with b₁ and b₃ symmetries, in line with the qualitative MO-diagram, while the third is a σ-orbital. The second band (structural feature 3) at 9.4 eV represents a single σ-type orbital of b₃ symmetry, whereas the third band with a peak maximum at 10.3 eV corresponds to ionization from four different orbitals, pair-wise of π- and σ-symmetry, respectively. The former two can be characterized as in-phase (b₁) and out-of-phase (b₂) combinations of the two π(SiMe₂) group orbitals, where the first also has contributions from π(C=C) (cf.Fig. 6).

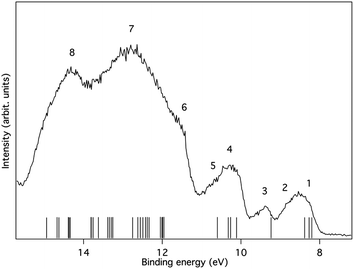Fig. 10 The photoelectron spectrum of 1b excited using HeIα radiation at 21.22 eV. The bands are numbered as in Table 1. Ionization energies calculated at the OVGF/6-311+G(d)//B3LYP/6-31G(d) level are included as bars on the energy axis.

Table 1 Experimental (peak maximum from the photoelectron spectrum) and calculated binding energies (eV),^a with assignments (term) of 1b with D₂ symmetry (1b-II conformer)

Structure number	Binding energy (exp)	Binding energy (calc)	Assignment (orbital type)	Comment
a Calculated at ROVGF/6-311+G(d)//B3LYP/6-31G(d) level.
1	7.9			Onset
1	8.5	8.20	b1 (π)	Peak max
		8.27	b3 (π)	Peak max
2	8.9	8.38	b1 (σ)	Shoulder
3	9.4	9.24	b3 (σ)	Peak max
4	10.3	10.11	a (σ)	Peak max
		10.26	b2 (π)
		10.32	b2 (σ)
5	10.7	10.60	b1 (π)	Shoulder

---

The pressure that could be obtained in the ionization chamber for 1c was much lower than for 1b. Due to these experimental constraints we recorded the spectral region between 5.5 eV and 9.5 eV several times and summed the individual spectra (Fig. 11). However, the region above 9.5 eV was included in only one of the recordings. The statistics are therefore not as good as for 1b, in particular in the higher binding energy region where signals from remaining H₂O and N₂ in the spectrometer are present. Yet, in the lower energy range the spectrum seems to be free of such influences. Some broad structures can be identified in this part, and their energies are given along with orbital characters in Table 2.

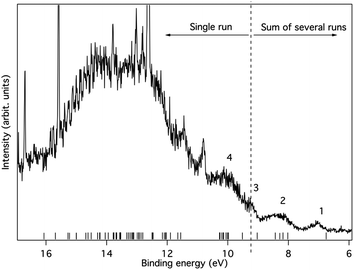Fig. 11 The photoelectron spectrum of 1c excited using HeIα radiation at 21.22 eV. The bands are numbered in accordance with Table 2. Ionisation energies calculated at the OVGF/6-311+G(d)//B3LYP/6-31G(d) level are included as bars on the energy axis.

Table 2 Experimental (peak maximum from the photoelectron spectrum) and calculated binding energies (eV) with assignments (term) of the 1c molecule with D₂ symmetry (1c-II)^a

Structure number	Binding energy (exp)	Binding energy (OVGF)	Assignment (orbital type)	Comment
a Calculated at the ROVGF/6-311+G(d)//B3LYP/6-31G(d) level.
1	6.7			Onset
	7.1	6.76	b1 (π)	Peak max
2	7.6			Onset
	8.3	8.02	b3 (π)
		8.18	b2 (π)
		8.28	b1 (σ)
		8.43	b3 (σ)
3	9.3	9.03	a (σ)
		9.52	b1 (π)
		9.98	b2 (σ)

---

The first band is located at 7.1 eV (peak maximum), and according to the calculations it corresponds to the ionization from a single π-orbital of b₁ symmetry. It should particularly be noted that the energy is substantially lower than for any orbital of 1b, suggesting that the influence of the SiMe₃ substituent character is significant. Thus, in line with the MO-theoretical description of Fig. 6, the Si(SiMe₃)₂ segment provides local π(ER₂) orbitals which are matched energetically with the π-orbitals of the 2 × (C=C) fragment, pushing up the MO which is the out-of-phase combination of the b₁ symmetric group orbitals of the 2 × (C=C) and 2 × (SiR₂) segments when compared to 1b. This confirms experimentally the large energy difference between HOMO and HOMO − 1 of 1c, as compared to 1a and 1b, observed in the B3LYP calculations (cf.Fig. 7).

The second band is broader and stronger, indicating ionization from more than one orbital. The peak maximum is observed at approximately 8.3 eV, and the calculations put four orbitals in this energy range. These orbitals are mostly of π-character and primarily located on the ring. The photoelectron band is therefore expected to resemble the outermost band of 1b, both in energy and general shape, due to the mutual similarity between the orbitals. This similarity is indeed observed as seen in Fig. 12 where the lower binding energy regions of the photoelectron spectra of 1b and 1c are shown in comparison to the numerical results. The only notable difference between the two compounds is found in the energies needed for ionization from the HOMOs.

Fig. 12 A comparison of the lower binding energy regions of the photoelectron spectra of 1b and 1c excited using HeIα radiation at 21.22 eV. Ionisation energies calculated at the OVGF/6-311+G(d)//B3LYP/6-31G(d) level are included as bars on the energy axis.

Cyclic voltammetry

To provide further evidence for the role of the substituents on the frontier orbital energies, compounds 1a–1c were investigated by cyclic voltammetry. Fig. 13 displays the anodic scan of compounds 1a (black), 1b (blue) and 1c (red). All three compounds show electrochemically irreversible oxidation processes at 1.47, 0.88 and 0.46 V vs. Fc/Fc⁺, respectively. Thus, the oxidative peak potential of 1c is cathodically shifted by 400 mV compared to that of 1b and by 1 V relative to 1a. These findings strongly support the results obtained in the valence photoelectron spectroscopy as well as in the computational studies. The findings also hint to extensive variations in redox properties that could be useful for future applications.

Fig. 13 Cyclic voltammograms (anodic scans) of 1a (black), 1b (blue), 1c (red) (1 mM solutions in CH₂Cl₂) containing 0.1 M NBu₄PF₆vs. Fc^+/0, v = 200 mV s⁻¹ * Fc⁺/Fc⁰ couple as internal standard.

UV absorption spectroscopy

The excitation energies of the three 1,4-disilacyclohexa-2,5-dienes 1a–c and cyclohexa-1,4-diene 6 were recorded by gas phase UV absorption spectroscopy (Fig. 14). The wavelengths and extinction coefficients at the absorption peaks are summarized in the ESI.† The UV absorption spectra of 1a and 1b were only recorded in the gas phase as solvent interferences when recorded in cyclohexane were so strong that no reliable spectral information was obtained. For 1c, both gas phase and solution spectra were recorded, and the gas phase measurement clearly reveals a thermal redistribution of the curve as compared to the measurement at room temperature in cyclohexane, a result of vibrational broadening.

Fig. 14 UV absorption spectra of 1a (black), 1b (blue), 1c (red), and 6 (green) recorded in gas phase, and for 1c also the spectrum recorded at room temperature in cyclohexane solution (black dashed curve).

Compounds 1a and 1b have similar characteristics as their spectra are composed of two overlapping bands; one centered at 210–220 nm and the second in the range 190–200 nm. The strongest absorptions are found in the lower wavelength range (198 and 196 nm for 1a and 1b, respectively). 1,4-Disilacyclohexa-2,5-diene 1c, on the other hand, shows notably different spectral features, with the most intriguing feature being the strong absorption at 273 nm (4.55 eV). A comparison with the UV absorption spectrum of cyclohexa-1,4-diene (6) further reveals the difference between the SiR₂ and CH₂ units as mediators between the two C=C bonds because 6 does not absorb above 220 nm. Moreover, the molecular extinction coefficients of the excitations of 6 are much smaller than for the excitations of any of the three 1,4-disilacyclohexa-2,5-dienes.

In 1c, two weakly chromophoric trisilane segments have been incorporated, and it is apparent from the UV spectrum that these couple strongly with the two C=C bonds because the first absorption of permethylated trisilane, Si₃Me₈, has been reported at 216 nm (5.75 eV).⁴¹ Hence, the chromophore of 1c constitutes the complete cross-hyperconjugated cycle involving the two C=C bonds and the two trisilane segments.

In order to analyze the experimental UV absorption spectra, excitation energies and oscillator strengths were calculated using time-dependent DFT (TD-DFT) at the TD-PBE0/6-31+G(2d)//B3LYP/6-31G(d) level. To allow for a facile comparison with the D_2h symmetric 5 we discuss the type-II conformers for all three compounds, although both conformers I and II will be nearly equally populated at the elevated temperatures used. The calculated excitation energies and oscillator strengths of conformer I closely resemble those of conformer II (for a full comparison between I and II, see the ESI†). In general, the calculated excitation wavelengths (energies) of the three compounds 1a-II to 1c-II agree with the recorded values of Fig. 14.

The most interesting spectrum among the 1,4-disilacyclohexa-2,5-dienes studied is displayed by 1c. Its first calculated excitation is a dark transition at 311 nm (3.98 eV) of B₁ symmetry and it has significant character of an excitation from HOMO to LUMO (cf.Fig. 8). However, the second excitation calculated at 276 nm (4.50 eV) is strongly allowed and of B₃ symmetry. This transition should correspond to the strong peak observed experimentally at 273 nm and it has a significant contribution of the HOMO to LUMO + 1 excitation, but also of the HOMO − 1 to LUMO excitation. As the transition has HOMO to LUMO + 1 excitation character, i.e., a transition between the two MOs which have clear cross-hyperconjugative character involving both trisilane and olefin fragments, this transition can only be observed in 1c and closely related 1,4-disilacyclohexadiene derivatives.

We also examined the emissive properties of 1c but no emission could be detected upon excitation at 273 nm. However, 1c also does not photodecompose when irradiated with λ > 220 nm light, indicating that non-radiative pathways bring it back to the electronic ground state. In contrast, a rapid photodegradation, possibly due to silylene extrusion from the trisilane segments, is observed upon irradiation at shorter wavelengths than 220 nm.

On the impact of cyclic cross-hyperconjugation in 1c

To determine the importance of the cyclic nature of the cross-hyperconjugation in 1cvs. linear hyperconjugation and acyclic cross-hyperconjugation we calculated the UV absorption and electron binding energies for a series of additional compounds (7–10, Fig. 5) at TD-PBE0/6-31+G(2d)//B3LYP/6-31G(d) and OVGF/6-311+G(d)//B3LYP/6-31G(d) levels (results from additional TD-M06-2X/6-31+G(2d)//B3LYP/6-31G(d) calculations are found in the ESI†). The tetraethyl analogue of one of these compounds, 7, could at first glance be generated experimentally through hydrogenation of 1c. However, despite numerous attempts using different conditions we were unable to reduce any of the double bonds in 1c, presumably due to the extensive steric bulk of the four ethyl and four TMS substituents.

Computations of 1g, the derivative of strongly cross-hyperconjugated 1c with hydrogens instead of ethyl substituents, reveal that the ethyl groups have a small effect on the electronic structure of 1c (first excitations at 299 and 311 nm (4.16 and 3.98 eV) for 1g and 1c, respectively, and a moderate effect on the first binding energy (7.14 and 6.76 eV, respectively)). The replacement of one of the C=C double bonds by a saturated C–C single bond to yield 7, rendering this compound linearly hyperconjugated, results in a significant change in the calculated spectral properties with the first electronic excitation at 263 nm (4.71 eV) and a first binding energy of 7.76 eV. Breakage of the cyclic nature of the cross-hyperconjugation by replacement of one of the Si(SiMe₃)₂ groups with a CH₂ group, yielding the acyclic cross-hyperconjugated 8, has a similar large effect leading to a first transition at 261 nm (4.75 eV) and a first binding energy of 7.53 eV. Replacing one of the C=C double bonds of 8 with a saturated C–C bond leading to 9 with a short linearly hyperconjugated path, gives a compound which is less hyperconjugated according to the TD-DFT and OVGF results (first transition at 236 nm (5.25 eV) and binding energy at 7.77 eV). Finally, replacing both the double bonds of 1g with saturated C–C bonds results in compound 10 with two isolated Si(SiMe₃)₂ segments. This compound has experimentally been found to not absorb above 220 nm in accordance with computations.⁴² Taken together, this shows on the effect of having two cross-hyperconjugated moieties unified in a cycle.

On the orientational effect of cross-hyperconjugation

In comparison to our previously examined bis(phenylethynyl)methanes and silanes^20,21 the two SiR₂ segments of the disilacyclohexa-1,4-dienes 1 are not connected to ethynylene (–C≡C–) fragments but to ethenylene (–C=C–) fragments. In the earlier studies we used the ethynylenes as spacers so as to reduce the steric congestion between two phenyl groups. However, ethenylene fragments are more attractive targets for two reasons; (i) they can be substituted so as to further influence the electronic and optical properties of the system, and (ii) the local π(C=C) orbitals are of higher energy than local π(C=C) orbitals, providing a better orbital energy match with the π(SiR₂) orbitals.

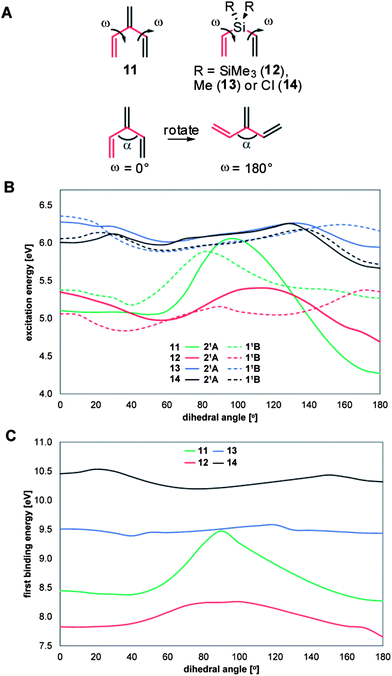
In 1, the geometry is constrained, however to probe the orientational effect of the ethenylene moieties several model compounds, 11–14 (Fig. 15A) were investigated computationally using the same levels as above. For these compounds we gradually drove the two C=C–C–C dihedral angles ω from 0° to 180°, keeping C₂ symmetry. The planar structures should provide for optimal cross-hyperconjugation while the remaining structures have the ethenyl groups out-of-plane. From the calculations the ω-dependences of the first excitation energies and first binding energies are obvious for 11 and 12; when 11 and 12 are planar (0 and 180°) their first binding energies are at the lowest values whereas for the first excitation energies the lowest energies are found at 180° while slightly higher values are observed at 0°. This raise in excitation energy should stem from steric congestion between the terminal hydrogen atoms of the ethenyl groups (in 11, α = 126° at ω = 0° and α = 114° at ω = 180°) which influences the excitation energy (Fig. 15). On the contrary, if the two double bonds are connected as in 8, avoiding the steric congestion and reducing the angle α, the excitation energy is again lowered (see above). Hence, the rotation of the double bonds out-of-plane displays the importance of the rigid structure for optimal cross-hyperconjugation. Neither the methyl substituted 13 nor the chloro substituted 14 show the same dependence on ω, but instead display more modest variations as is expected for two nearly isolated double bonds. For discussions and comparisons between the different orbitals, see the ESI.†

Fig. 15 (A) The model compounds 11–14, the C=C–C–C dihedral angles ω (one marked in red), and the C–C–C valence angle α. (B) The calculated first excitation energies of the 2¹A and 1¹B states of 11–14 calculated at TD-PBE0/6-31+G(2d)//B3LYP/6-31G(d) level. (C) The calculated first binding energies of 11–14 calculated at OVGF/6-311+G(d)//B3LYP/6-31G(d) level.

Comparison with previous work and future synthetic targets

When compared with our previously reported bis(phenylethynyl)methanes and silanes^20,21 the ethenylene fragments can interact more strongly with the central ER₂ segment than can ethynylene fragments because bis(ethynyl)silanes with SiMe₃ and Me substituents, when compared to 12 and 13, have higher first excitation and electron binding energies (for R = SiMe₃: 237 nm (5.23 eV) and 8.1 eV, and for R = Me: 209 nm (5.93 eV) and 10.0 eV, respectively). Indeed, these values are similar or slightly above the values found for 12 and 13 at ω = 90° (Fig. 15), where the conjugation is the weakest. Attachment of additional ethyl substituents at the ends of the triple bonds, so as to allow for comparison with the ethyl substituted 1, marginally lowers the first excitation and binding energies. However, for the double bonds it is essential to lock the conformation into a planar structure for optimal conjugation. Taken together, it is apparent that the ethenylene fragments are attractive as they provide for both further substitution opportunities and for stronger interaction with the ER₂ group than the ethynylene fragments. One structure with (near) optimal and fixated neutral hyperconjugation is our disilacyclohexa-1,4-dienes, yet, the computed results on 12 indicate that even stronger cyclic cross-hyperconjugation can be achieved through a “cis”-conformation with ω = 180°, provided a suitable rigid structure can be tailored. Overall, it is likely that rigid planar structures with properly substituted ER₂ fragments provide for substantial σ/π-interactions and represent new synthetic targets for research on optically and electronically active compounds. Oligomers of such compounds with potential connecting points from both the π-system and the ER₂ segment should be attractive targets and investigations into these and related compounds are on-going in our laboratories.

Conclusions

Herein we have shown that 1,4-disilacyclohexa-2,5-dienes are a compound class that allows for remarkable variations in the electronic and optical characteristics through the choice of the substituents. The large tunability arises from the fact that two SiR₂ segments as parts of the cyclic 1,4-disilacyclohexa-1,4-diene allow for neutral cyclic cross-hyperconjugation to various degrees. A strong cyclic cross-hyperconjugation is found with R = SiMe₃ and much weaker when R = Cl and Me as manifested in the valence photoionization energies, the first oxidation potentials, and the lowest UV absorption energies. One can predict that a change of the substituents at the C=C double bonds provides for further tuning of the electronic and optical properties. The neutral cross-hyperconjugation strengths in the 1,4-disilacyclohexadienes were clarified with quantum chemical calculations combined with a MO-theoretical analysis. Interestingly, the orbital pattern and the electronic structure of 1c has similarities with that of the cross-π-conjugated para-xylylene. The 1,4-disilacyclohexa-2,5-diene is therefore a new monomer unit which in an optimal way combines σ- and π-bonded segments into a rigid neutral and cyclic cross-hyperconjugated framework. It should represent an interesting structural motif for new molecules with applications in the molecular and organic electronics areas, and it could allow for design of strongly hyperconjugated compounds with properties that are not available to purely π-conjugated compounds.

Acknowledgements

We thank Dr. Carla Puglia for valuable discussions. We are grateful for financial support from the Swedish Research Council, from Uppsala University for the U³MEC KoF07 Initiative, from the Wenner-Gren Foundations in the form of a postdoctoral fellowship to A.W., and from the Göran Gustafsson Foundation (S.O and D.N.). K. Y. is grateful for the fellowship and Grant-in-Aid for JSPS Fellows Number 251672 from Japan Society for the Promotion of Science (JSPS). The National Supercomputer Center (NSC) in Linköping, Sweden is acknowledged for a generous allotment of computer time.

Notes and references

1. See complete volume, Chem. Rev., 2005, 105, 3433–3947.
2. (a) R. D. Miller and J. Michl, Chem. Rev., 1989, 89, 1359–1410; (b) J. Michl and R. West, Acc. Chem. Res., 2000, 33, 821–823.
3. T. A. Skotheim and J. R. Reynolds, Handbook of Conducting Polymers, CRC Press LLC, Bocata Raton, 2007.
4. R. Hoffmann, C. Janiak and C. Kollmar, Macromolecules, 1991, 24, 3725–3746.
5. (a) H. Wang, R. Helgeson, B. Ma and F. Wudl, J. Org. Chem., 2000, 65, 5862–5867; (b) M. Bruschi, M. G. Giuffreda and H. P. Lüthi, Chem.–Eur. J., 2002, 8, 4216–4227; (c) N. F. Phelan and M. Orchin, J. Chem. Educ., 1968, 45, 633–637.
6. R. S. Mulliken, C. A. Rieke and W. G. Brown, J. Am. Chem. Soc., 1941, 63, 41–56.
7. (a) R. S. Mulliken, J. Chem. Phys., 1939, 7, 339–352; (b) I. V. Alabugin and T. A. Zeidan, J. Am. Chem. Soc., 2002, 124, 3175–3185; (c) J. B. Lambert and R. A. Singer, J. Am. Chem. Soc., 1992, 114, 10246; (d) I. V. Alabugin, K. M. Gimore and P. W. Peterson, WIREs Comput. Mol. Sci., 2011, 1, 109–141.
8. We use the term cross-hyperconjugation instead of hypercross-conjugation to stress that the geminal connectivity of the conjugated paths should have higher priority than the specific conjugation type.
9. For recent reviews in silole-containing oligomers and polymers see, e.g.: (a) K. Tamao and S. Yamaguchi, J. Organomet. Chem., 2000, 611, 5–11; (b) S. Yamaguchi and K. Tamao, J. Organomet. Chem., 2002, 653, 223–228; (c) S. Yamaguchi and K. Tamao, Chem. Lett., 2005, 2; (d) J. Chen and Y. Cao, Macromol. Rapid Commun., 2007, 28, 1714–1742; (e) J. Ohshita, Macromol. Chem. Phys., 2009, 210, 1360–1370.
10. M. Ishikawa and J. Ohshita, in Organic Conductive Molecules and Polymers, ed. H. S. Nalwa, Wiley, New York, 1997, ch. 30.
11. (a) M. Nakamoto, Y. Inagaki, M. Nishina and A. Sekiguchi, J. Am. Chem. Soc., 2009, 131, 3172–3173; (b) T. Ochiai, M. Nakamoto, Y. Inagaki and A. Sekiguchi, J. Am. Chem. Soc., 2011, 133, 11504–11507; (c) Y. Inagaki, M. Nakamoto and A. Sekiguchi, J. Am. Chem. Soc., 2011, 133, 16436–16439; (d) A. Chrostowska, A. Dargelos, P. Baylère, A. Graciaa, Y. Inagaki, M. Nakamoto, V. Ya. Lee and A. Sekiguchi, ChemPlusChem, 2013, 78, 398–401.
12. Y. Yamaguchi, Synth. Met., 1996, 82, 149–153.
13. J. Ohshita and A. Kunai, Acta Polym., 1998, 49, 379–403.
14. J. Ohshita, H. Takata, H. Kai, A. Kunai, K. Komaguchi, M. Shiotani, A. Adachi, K. Sakamaki, K. Okita, Y. Harima, Y. Kunugi, K. Yamashita and M. Ischikawa, Organometallics, 2000, 19, 4492–4498.
15. J. Ohshita, D.-H. Kim, Y. Kunugi and A. Kunai, Organometallics, 2005, 24, 4494–4496.
16. D.-H. Kim, J. Ohshita, T. Kosuge, Y. Kunugi and A. Kunai, Chem. Lett., 2006, 266–267.
17. J. Ohshita, Y. Izumi, D.-H. Kim, A. Kunai, T. Kosuge, Y. Kunugi, A. Naka and T. Ishikawa, Organometallics, 2007, 26, 6150–6154.
18. J. Ohshita, Y. Hatanaka, S. Matsui, T. Mizumo, Y. Kunugi, Y. Honsho, A. Saeki, S. Seki, J. Tibbelin, H. Ottosson and T. Takeuchi, Dalton Trans., 2010, 39, 9314–9320.
19. J. Ohshita, Y. Hatanaka, S. Matsui, Y. Ooyama, Y. Harima and Y. Kunugi, Appl. Organomet. Chem., 2010, 8, 540–544.
20. R. Emanuelsson, A. Wallner, E. A. M. Ng, J. R. Smith, D. Nauroozi, S. Ott and H. Ottosson, Angew. Chem., Int. Ed., 2013, 52, 983–987.
21. E. Göransson, R. Emanuelsson, K. Jorner, L. Hammarström and H. Ottosson, Chem. Sci., 2013, 4, 3522–3532.
22. U. Weidner and A. Schweig, Angew. Chem., Int. Ed. Engl., 1972, 11, 536–537.
23. R. West and R. E. Bailey, J. Am. Chem. Soc., 1963, 85, 2871–2872.
24. (a) R. Hoffmann, Acc. Chem. Res., 1971, 4, 1–9; (b) R. Srinivasan, L. S. White, A. R. Rossi and G. A. Epling, J. Am. Chem. Soc., 1981, 103, 7299–7304.
25. H. Bock and W. Kaim, J. Am. Chem. Soc., 1980, 102, 4429–4438.
26. R. West, H. Sohn, U. Bankwitz, J. Calabrese, Y. Apeloig and T. Müller, J. Am. Chem. Soc., 1995, 117, 11608–11609.
27. R. West, H. Sohn, D. R. Powell, T. Müller and Y. Apeloig, Angew. Chem., Int. Ed. Engl., 1996, 35, 1002–1004.
28. B. T. Stępień, T. M. Krygowski and M. K. Cyrański, J. Org. Chem., 2002, 67, 5987–5992.
29. H. Ottosson, K. Kilså, K. Chajara, M. C. Piqueras, R. Crespo, H. Kato and D. Muthas, Chem.–Eur. J., 2007, 13, 6998–7005.
30. A. P. Scott, I. Agranat, P. U. Biedermann, N. V. Riggs and L. Radom, J. Org. Chem., 1997, 62, 2026–2038.
31. A. Stanger, J. Org. Chem., 2006, 71, 883–893.
32. O. M. Nefedov and M. N. Manakov, Angew. Chem., Int. Ed. Engl., 1966, 5, 1021–1038.
33. W. H. Atwell and D. R. Weyenberg, Angew. Chem., Int. Ed. Engl., 1969, 8, 469–477.
34. P. P. Gaspar and R. West, in The Chemistry of Organosilicon Compounds, Wiley, New York, 1998, vol. 2, pp. 2463–2568.
35. F. Wudl, R. D. Allendoerfer, J. Demirgian and J. M. Robbins, J. Am. Chem. Soc., 1971, 93, 3160–3162.
36. A. Kunai, J. Ohshita, T. Iida, K. Kanehara, A. Adachi and K. Okita, Synth. Met., 2003, 37, 1007–1008.
37. S.-H. Kang, J. S. Han, M. E. Lee, B. R. Yoo and N. Jung, Organometallics, 2003, 22, 2551–2553.
38. B. Ma, J.-H. Lii, H. F. Schaefer III and N. L. Allinger, J. Phys. Chem., 1996, 100, 8763–8769.
39. (a) L. S. Bartell and R. A. Bonham, J. Chem. Phys., 1959, 31, 400–404; (b) F. H. Allen, O. Kennard, D. G. Watson, L. Brammer, A. G. Orphen and R. Taylor, J. Chem. Soc., Perkin Trans. 2, 1987, S1–S19.
40. M. Kaftory, M. Kapon and M. Botoshansky, in The Chemistry of Organic Silicon Compound, ed. Z. Rapport and Y. Apeloig, Wiley, Chichester, UK, vol. 2, ch. 5, p. 181.
41. C. G. Pitt, J. Am. Chem. Soc., 1969, 91, 6613–6622.
42. A. Wallner, R. Emanuelsson, J. Baumgartner, C. Marschner and H. Ottosson, Organometallics, 2013, 32, 396–405.

---

Footnote

† Electronic supplementary information (ESI) available: Full computational and experimental procedures as well as details on the gas phase UV absorption spectroscopy, analysis of the impact of steric and electronic factors on the geometries of 1,4-disilacyclohexa-2,5-dienes 1a–h, orbital plots, absolute electronic energies, Cartesian coordinates, tables with TD-DFT data, tables with complete photoelectron spectroscopic data and X-ray crystallographic information for compound 1c. CCDC 775978. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c3sc52389f

---