Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Can acyclic conformational control be achieved via a sulfur–fluorine gauche effect?†
C.
Thiehoff
a,
M. C.
Holland
ab,
C.
Daniliuc‡
a,
K. N.
Houk
*b and
R.
Gilmour
*ac
aOrganisch Chemisches Institut, and Excellence Cluster EXC 1003, Cells in Motion, Westfälische Wilhelms-Universität Münster, Corrensstrasse 40, Münster, Germany. E-mail: ryan.gilmour@uni-muenster.de
bDepartment of Chemistry and Biochemistry, University of California Los Angeles, 607 Charles E. Young Drive East, Los Angeles 90095-1569, USA. E-mail: houk@chem.ucla.edu
cExcellence Cluster EXC 1003, Cells in Motion, Westfälische Wilhelms-Universität Münster, Münster, Germany
First published on 17th April 2015
Abstract
The gauche conformation of the 1,2-difluoroethane motif is known to involve stabilising hyperconjugative interactions between donor (bonding, σC–H) and acceptor (antibonding, σ*C–F) orbitals. This model rationalises the generic conformational preference of F–Cβ–Cα–X systems (ϕFCCX ≈ 60°), where X is an electron deficient substituent containing a Period 2 atom. Little is known about the corresponding Period 3 systems, such as sulfur and phosphorus, where multiple oxidation states are possible. Conformational analyses of β-fluorosulfides, -sulfoxides and -sulfones are disclosed here, thus extending the scope of the fluorine gauche effect to the 3rd Period (F–C–C–S(O)n; ϕFCCS ≈ 60°). Synergy between experiment and computation has revealed that the gauche effect is only pronounced in structures bearing an electropositive vicinal sulfur atom (S+–O−, SO2).
Controlling rotation about C(sp3)–C(sp3) bonds is strategically important in molecular design, not least to determine the spatial positioning of substituents on the component atoms.1 Of the various acyclic conformational control strategies in common practice, the fluorine gauche effect2 has gained momentum in recent years on account of the minimal steric footprint imposed by this substituent; this often leads to conformer populations that are inaccessible by traditional steric locking approaches. The counterintuitive preference of the parent 1,2-difluoroethane scaffold to populate the gauche conformer preferentially can be rationalised by invoking hyperconjugative σC–H → σ*C–F interactions. This conformational preference is conserved in a number of F–C–C–X systems where X is electron deficient (Fig. 1).3 A simplified donor–acceptor model is didactically valuable in rationalising and predicting conformation, while more detailed analysis reveals that both orbital and electrostatic effects are involved.4 This is particularly true when X carries a (partial) positive charge, and electrostatic interactions contribute significantly. The strategic installation of the F–C–C–X motif can lead to predictable molecular topologies on account of the gauche effect (ϕFCCX ≈ 60°): the caveat that stereoelectronic effects can be overridden by prevailing steric factors must always be considered.
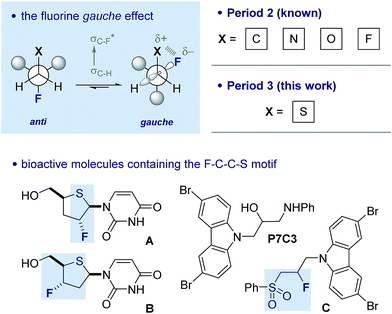 | ||
| Fig. 1 The fluorine gauche effect. Selected literature precedence for a potential sulfur–fluorine gauche effect. Lower left: fluorinated deoxy-4′-thio pyrimidine nucleosides A and B.11 Lower right: proneurogenic compound P7C3 and its β-fluorinated sulfone derivative (C).12 | ||
This approach to molecular design has found widespread application in catalysis,5 bioactive molecule design,6 material science7 and agrochemistry.8 In the majority of cases, the substituent (X) is a Period 2 atom, typically oxygen or nitrogen. In contrast, the manifestation of this phenomenon in combination with 3rd row elements has been largely ignored despite the importance of sulfur and phosphorus containing compounds in industry and academia. Recent interest in the preparation9 and properties of compounds containing the F–C–C–S(O)n (n = 0, 1 and 2) unit prompted this study.
There is limited structural evidence consistent with the postulated sulfur–fluorine gauche effect. In the mid-1980s, Carretero and co-workers reported a NMR study of β-fluorinated thioethane derivatives, including various sulfides, sulfoxides, sulfones and sulfonium salts.10 Vicinal coupling constant analysis is consistent with a gauche orientation of the sulfur and fluorine atoms. Further evidence of this phenomenon derives from the X-ray structure analyses of fluorinated deoxy-4′-thiopyrimidine nucleosides such as A and B (Fig. 1), where torsional angles of ϕFCCS ≈ 80° approach the expected stereoelectronic requirements despite the constraints imposed by the ring.11 Finally, a recent study by Ready and co-workers identified carbazole P7C3 as displaying potent neuroprotective activity.12 A lead structure in this investigation is derivative C, containing the β-fluorosulfone unit. Herein we report a combined experimental and computational study of the fluorine–sulfur gauche effect with specific emphasis on sulfides, sulfoxides and sulfones.
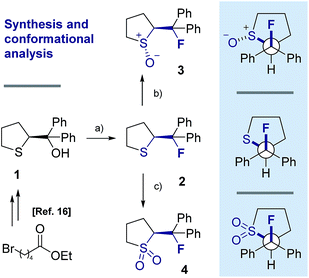
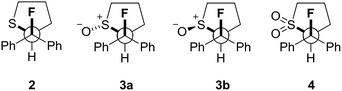
Our recent interest in the fluorine gauche effect in pyrrolidine organocatalysts (X = N)5a,b,f led us to explore tetrahydrothiophene derivatives 2, 3 and 4 (Scheme 1) as scaffolds for this study. It was envisaged that the diffuse nature of sulfur orbitals, and the polarised nature of the oxidised forms (e.g., S+–O−, SO2) would generate hyperconjugative and/or charge–dipole interactions that might manifest themselves in diagnostic conformations. The heterocycles can exist as synclinal-endo and synclinal-exo conformers that can easily be distinguished by vicinal (3J) coupling constant analysis. Structures 2, 3 and 4 were prepared from the intermediate 1 (Scheme 1).13 Direct deoxyfluorination of 1 was facile and furnished 2 in 54% yield. This is noteworthy given the dearth of information of fluorination of this substrate class. Subsequent oxidation to the corresponding sulfoxide 3 proceeded smoothly in 68% yield and with excellent levels of diastereoselectivity (97![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3), giving a first insight into the possible role of fluorine in influencing the conformation of such systems. Finally, upon exposure to excess mCPBA, the sulfone 4 was generated in 85% yield.
3), giving a first insight into the possible role of fluorine in influencing the conformation of such systems. Finally, upon exposure to excess mCPBA, the sulfone 4 was generated in 85% yield.
X-ray crystal structure analysis
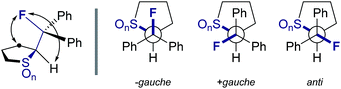
Single crystals suitable for X-ray analysis were obtained in all cases (Fig. 2).14 Each structure exhibited a gauche preference, favouring the synclincal-endo conformation (2, 3, 4, ϕFCCS = −62.07°, −60.89°, −62.37°, respectively; Table 1). Common to all structures is an unusually long S1–C4 bond length as compared to the S1–C1 bond length (Table 1, Δd ≈ 0.027 Å, 0.039 Å, 0.053 Å, for 2, 3 and 4, respectively). To place this observation in context with comparable sulfur containing structures, a selection of C–S bond lengths are provided in Table 1 (right column).15 This may be noteworthy in view of the importance of fractional bonds in translating small changes in ground state structures to reactivity.16 Importantly, the vicinal C–H and C–F bonds are antiperiplanar (179°, −177°, 178.15°) thus allowing for stabilising hyperconjugative interactions (σC–H → σ*C–F), with C–F bond lengths of 1.41 Å, 1.42 Å and 1.42 Å, for 2, 3 and 4 respectively. The solid state structure of sulfoxide 3, prepared by diastereoselective oxidation, reveals a conformation in which the C–F and S–O dipoles are minimised. The sulfone derivative 4 preferentially adopts the conformation placing the fluorine atom synclinal-endo to the sulfur centre: this minimises repulsion with the non-bonding electron pairs of the oxygen atoms.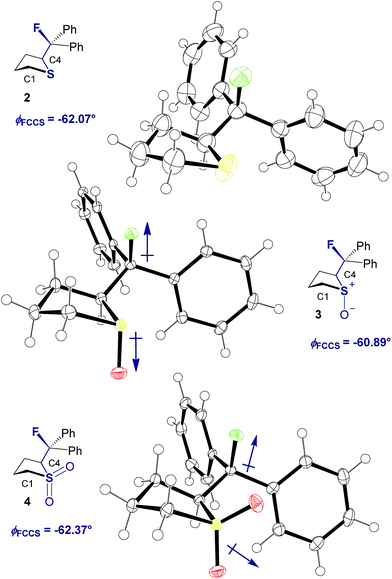 | ||
| Fig. 2 X-ray structural analyses of cyclic compounds 2, 3 and 4. Thermal ellipsoids shown at 50% probability level.14 | ||
| Compound | ϕ FCCS [°] | d S1–C4 [Å] | d S1–C1 [Å] | Δd [Å] | Literature d S–C [Å]15 |
|---|---|---|---|---|---|
| a In tetrahydrothiophene. b In a C–S(O)–C motif. c In a C–S(O2)–C motif. d S–C(sp3) bond. e S–C(Ar) bond. f In CAr–S(O)–C motif. g In CAr–S(O2)–C motif. | |||||
| 2 | −62.07 | 1.8379(16) | 1.8107(19) | 0.027 | 1.827a |
| 3 | −60.89 | 1.853(2) | 1.814(2) | 0.039 | 1.818b |
| 4 | −62.37 | 1.832(2) | 1.799(2) | 0.054 | 1.786c |
| 6 | −55.87 | 1.764(9)d | 1.799(6)e | 0.035 | 1.790f/1.818b |
| 7 | −68.12 | 1.7789(15)d | 1.7791(15)e | 0.0002 | 1.763g/1.786c |
To ensure that the gauche orientation observed in the tetrahydrothiophene derivatives 2, 3 and 4 is not a consequence of unfavourable non-bonding interactions with the ring, a sterically less demanding, linear system was synthesised for comparison. Reaction of 4-nitrothiophenol with tosylated 2-fluoroethanol17 afforded the linear sulfide 5; this was subsequently converted to sulfoxide 6 and sulfone 7 (Scheme 2). It was possible to grow crystals of compounds 6 and 7 that were suitable for X-ray analysis.14 In both cases, the C–S and C–F bonds were oriented in the expected gauche arrangement (6 and 7, ϕFCCS = −55.87°, −68.12°, respectively; Table 1). These conformations allow for σC–H → σ*C–F interactions, again indicating that this effect likely is due in part to hyperconjugative stabilisation. Consistent with structure 3 (Fig. 2, centre), the X-ray analysis of sulfoxide 6 reveals a conformation where the C–F and S–O dipoles oppose each other. In sulfone 7, the C–F bond adopts a gauche arrangement that circumvents interaction with the SO2 unit.
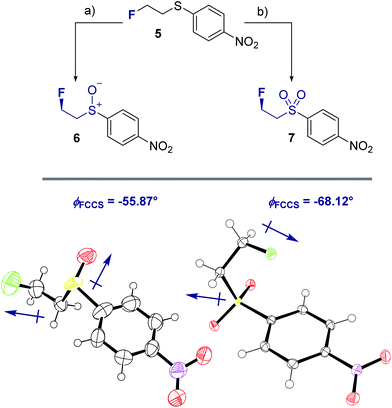 | ||
| Scheme 2 Syntheses and X-ray crystal structure analyses14 of linear sulfoxide 6 and sulfone 7. (a) mCPBA (1.0 eq.), CH2Cl2, 0 °C to rt, 26 h; (b) mCPBA (3.0 eq.), CH2Cl2, 0 °C to rt, 17 h. Thermal ellipsoids shown at 50% probability level. | ||
NMR solution phase conformational analysis
To complement the solid state investigation, a solution phase NMR conformer population analysis of the cyclic compounds was performed.18 Assuming that only staggered conformers with torsion angles of −60° (−gauche), 60° (+gauche) and 180° (anti) contribute significantly to the population in solution phase, the measured coupling constant 〈J〉 can be described by the equation 〈J〉 = x−gJ−g + x+gJ+g + xaJa. Furthermore, the approximation that the dependency of J is symmetrical about 0° (ref. 19) renders the following simplification valid: J−g = J+g = Jg. Hence, the molar fraction of the anti conformer can be determined according to the following expression: xa = (〈J〉 − Jg)/(Ja − Jg).20 Whilst literature values for 3JCF are available (Jg = 1.2 Hz, Ja = 11.2 Hz)21 the related 3JHF can be calculated based on a modified Karplus equation.22 Inserting the measured 3J coupling constants (Table 2) allows for the determination of combined populations of the −gauche and +gauche conformers of >80% in all cases. Comparison of the 3JHF coupling constants of both series reveals the following trend: upon oxidation of the sulfide to the corresponding sulfoxide, a significant increase in the magnitude of the coupling constant is observed (3JHF = 29.2, 37.8 and 19.2, 30.0 Hz for 2, 3 and 5, 6, respectively). Further oxidation to the sulfone results in coupling constant values that are only slightly augmented relative to those of the parent sulfides (3JHF = 31.2 and 23.8 Hz for 4 and 7, respectively). These analyses reveal that the major solution phase conformers closely resemble the solid state structures determined by crystallography, and are fully consistent with the notion of a sulfur–fluorine gauche effect.| Compound | 3 J HF [Hz] | 3 J CF [Hz] | −gauche [%] | +gauche [%] | anti [%] |
|---|---|---|---|---|---|
| a Group electronegativity values for calculation22 of 3JHF were taken from the literature23 (2: 0.76 [SH group] and 3/4: 0.69 [SO2Cl group]). | |||||
| 2 (n = 0) | 29.2 | 3.2 | 61.3 | 20.0 | 18.7 |
| 3 (n = 1) | 37.8 | 3.2 | 74.7 | 20.0 | 5.3 |
| 4 (n = 2) | 31.2 | 4.2 | 62.3 | 30.0 | 7.7 |
DFT conformational analysis
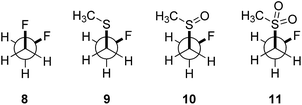
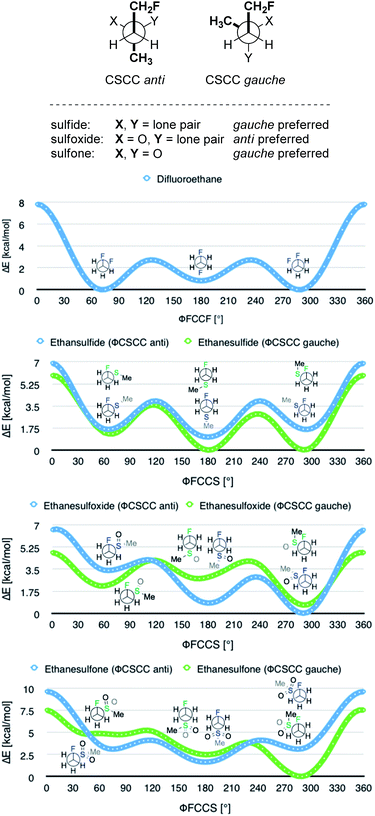
In order to quantify the observed conformational preferences using DFT, a series of structures containing the key F–C–C–S(O)n unit (n = 0, 1 and 2) were optimised at the B3LYP24/6-311+G(d,p)25 level of theory. Solvation by dichloromethane was taken into account using the integral equation formalism polarizable continuum model (IEFPCM).26 Dichloromethane was chosen to ensure consistency with the NMR solution phase conformational analysis. The choice of basis set was based on a previous computational study of the gauche effect in α-X-β-fluoro-ethane derivatives (X = F, NR, OR, CR) by O'Hagan and co-workers.4c All computations were performed using Gaussian09.27 Free energy corrections were calculated using Truhlar's quasi-harmonic approximation.28 The lowest energy conformers of 1,2-difluoroethane (8) and the corresponding (2-fluoroethyl)-(methyl)-derivatives (sulfide = 9, sulfoxide = 10, sulfone = 11) were investigated (Table 3). Additionally, the Cα–Cβ bond rotational profiles (step size = 5°, 72 steps, B3LYP/6-311+G(d,p) in vacuum) of 9–11, with both the CSCC anti and gauche conformations, were calculated and compared to the rotational profile of 1,2-difluoroethane (8) (Fig. 3, top).| ϕ CSCC | ϕ FCC(F/S) | ΔG [kcal mol−1] | ϕ FCC(F/S) [°] | Q S | μ [D] | |
|---|---|---|---|---|---|---|
| a Mulliken atomic charges with hydrogens summed into heavy atoms. b Molecular dipole moment in Debye. | ||||||
| 8 | — | −gauche | 0.0 | 69 | — | 3.71 |
| — | anti | 1.9 | 180 | — | 0.00 | |
| 9 | anti | −gauche | 1.0 | −66 | 0.01 | 4.11 |
| anti | anti | 1.2 | 180 | 0.01 | 2.30 | |
| −gauche | −gauche | 0.7 | 66 | 0.23 | 4.08 | |
| +gauche | anti | 0.0 | −179 | 0.23 | 2.20 | |
| +gauche | −gauche | 0.5 | −68 | 0.21 | 1.89 | |
| 10 | anti | +gauche | 1.8 | 67 | 0.67 | 7.97 |
| anti | anti | 1.0 | 180 | 0.68 | 5.03 | |
| anti | −gauche | 0.0 | −67 | 0.70 | 5.98 | |
| +gauche | +gauche | 1.4 | 62 | 0.64 | 6.04 | |
| +gauche | anti | 2.1 | 170 | 0.66 | 5.20 | |
| +gauche | −gauche | 0.9 | −70 | 0.66 | 4.44 | |
| −gauche | anti | 1.9 | −166 | 0.65 | 5.38 | |
| 11 | anti | −gauche | 1.2 | −70 | 0.57 | 8.01 |
| anti | anti | 1.6 | 180.0 | 0.51 | 5.15 | |
| +gauche | −gauche | 0.0 | −70 | 0.45 | 4.91 | |
| −gauche | anti | 1.8 | −178 | 0.42 | 5.26 | |
| −gauche | −gauche | 2.1 | −76 | 0.39 | 7.79 | |
This analysis confirmed that the well known gauche preference of 1,2-difluoroethane (8) is also inherent to the linear sulfoxide and sulfone derivatives (ΔGanti/gauche = 1.9, 1.0, and 1.6 kcal mol−1, for 8, 10 and 11 respectively). However, the sulfide derivative 9 displays a slight preference for the anti conformation (ΔGgauche/anti = 0.5 kcal mol−1); this is at variance with the X-ray structures of 2 (Fig. 2). As expected, the gauche conformation appears to be more pronounced in structures bearing a more electropositive vicinal sulfur atom (S+–O−, SO2).
As a quantitative measurement for this effect, the Mulliken atomic charges (QS) of the sulfur atom in each of the conformers studied were calculated. These are listed in Table 3 (right). Interestingly, comparison of the energy minima in compounds 9–11 displayed some variation with respect to the CSCC chain. Whereas the sulfide 9 and the sulfone 11 position the two alkyl-groups gauche to each other in the lowest lying minima, the sulfoxide 10 preferentially orients the groups anti (Fig. 3).
A computational analysis of the linear 4-nitrothiophenol derived systems 5–7 (Table 4) revealed similar trends to those observed with the ethane derivatives (Table 3, also see Scheme 2). The sulfide derivative 5 displayed no significant preference for the gauche or anti conformation (5, ΔGgauche/anti = 0.1 kcal mol−1), whilst the sulfoxide and sulfone derivatives both exhibited a gauche preference (6 and 7, ΔGanti/anti = 1.3 and 1.3 kcal mol−1, respectively).
| ϕ CSCC | ϕ FCCS | ΔG [kcal mol−1] | ϕ FCCS [°] | Q S | μ [D] | |
|---|---|---|---|---|---|---|
| a Mulliken atomic charges with hydrogens summed into heavy atoms. b Molecular dipole moment in Debye. | ||||||
| 5 | anti | +gauche | 0.0 | 66 | −0.67 | 7.07 |
| anti | anti | 0.4 | 180 | −0.65 | 6.20 | |
| +gauche | +gauche | 0.2 | 64 | −0.38 | 6.68 | |
| +gauche | anti | 0.1 | 179 | −0.34 | 6.12 | |
| +gauche/eclipsed | −gauche | 0.1 | −69 | −0.30 | 9.55 | |
| 6 | anti | +gauche | 1.7 | 68 | 0.85 | 6.62 |
| anti | anti | 1.3 | 180 | 0.95 | 4.32 | |
| anti | −gauche | 0.0 | −66 | 0.89 | 4.65 | |
| +gauche | +gauche | 1.7 | 62 | 1.01 | 4.62 | |
| +gauche | anti | 2.9 | 173 | 1.01 | 4.15 | |
| eclipsed | −gauche | 1.8 | −67 | 0.82 | 6.55 | |
| 7 | anti | −gauche | 0.9 | −70 | 0.63 | 5.74 |
| anti | anti | 1.3 | −179 | 0.58 | 2.50 | |
| +gauche | −gauche | 0.0 | −72 | 0.66 | 5.85 | |
| +gauche | anti | 1.4 | 179 | 0.63 | 2.55 | |
| +gauche | +gauche | 1.4 | 72 | 0.61 | 5.65 | |
The three possible rotamers of cyclic compounds 2, 3 and 4 were also investigated (Table 5). For the sulfoxide derivative 3 both diastereoisomers (oxygen anti3a, and syn3b) were considered, although only the anti diastereoisomer was isolated following oxidation. In this case, the syn conformer is significantly higher in energy than the anti (ΔGsyn/anti = 3.7 kcal mol−1).
| ΔdS1–C4/S1–C1 [Å] | ϕ FCCS | ΔG [kcal mol−1] | ϕ FCCS [°] | Q S | μ [D] | |
|---|---|---|---|---|---|---|
| a Mulliken atomic charges with hydrogens summed into heavy atoms. b Molecular dipole moment in Debye. c Oxygen anti. d Oxygen syn. | ||||||
| 2 | 0.021 | +gauche | 3.3 | 64 | 0.07 | 4.92 |
| 0.018 | anti | 1.7 | −176 | −0.08 | 1.76 | |
| 0.022 | −gauche | 0.0 | −63 | −0.12 | 3.25 | |
| 3a | 0.057 | +gauche | 2.4 | 46 | 0.84 | 8.38 |
| 0.045 | anti | 2.7 | 172 | 0.84 | 5.51 | |
| 0.050 | −gauche | 0.0 | −63 | 0.81 | 5.09 | |
| 3b | 0.012 | +gauche/eclipsed | 5.7 | 26 | 0.78 | 7.15 |
| 0.012 | anti | 4.2 | 158 | 0.62 | 3.89 | |
| 0.042 | −gauche | 3.7 | −70 | 0.68 | 7.25 | |
| 4 | 0.039 | +gauche | 3.0 | 34 | 0.71 | 9.12 |
| 0.034 | anti | 2.9 | 161 | 0.52 | 5.42 | |
| 0.050 | −gauche | 0.0 | −66 | 0.70 | 6.88 | |
The sulfide (2) again shows the smallest energetic difference between the lowest lying anti and syn conformations, consistent with the results for the acyclic derivatives. Contrary to the other structures investigated, this cyclic derivative exhibits a significant preference for the gauche conformation (ΔGanti/gauche = 1.7 kcal mol−1). For the derivatives bearing a more electron deficient sulfur atom, the gauche preference is more pronounced (3 and 4, ΔGanti/gauche = 2.4 and 2.9 kcal mol−1, respectively). The synclinal-endo conformation is significantly lower in energy than the synclinal-exo conformation, and the latter is higher in energy than the anti. The optimised structures displayed the same lengthening of the S1–C4 bond as was observed by crystallography. The global energy minima for compounds 2, 3 and 4 are shown in Fig. 4 and closely match the corresponding X-ray structures (Fig. 2).
Conclusions
Computation and experiment confirm that the F–C–C–S motif has an intrinsic gauche conformational preference. Conformational analysis in both solid and solution phase confirmed that the C–F bond aligns anti to a vicinal C–H bond, reasonably to allow for stabilising hyperconjugative interactions of the type σC–H → σ*C–F. The ΔGgauche/anti value is larger when the sulfur centre is more electron deficient; this is especially pronounced in sulfoxides. Computational analyses indicate that the conformational preference is not solely due to overall molecular dipole minimisation (Table 3, 4 and 5, right column). This study extends the well-known gauche effect to 3rd Period substituents, and validates the notion that the F–C–C–S(O)n unit may be strategically embedded into functional scaffolds to achieve acyclic conformational control.Acknowledgements
We acknowledge generous financial support from the WWU Münster, the Deutsche Forschungsgemeinschaft (SFB 858, and Excellence Cluster EXC 1003 “Cells in Motion – Cluster of Excellence”), the German Academic Exchange Service DAAD (P.R.I.M.E. fellowship M. C. Holland) and the U.S. National Science Foundation (CHE-1361104).Notes and references
- R. W. Hoffmann, Angew. Chem., Int. Ed., 2000, 39, 2054 CrossRef.
- (a) S. Wolfe, Acc. Chem. Res., 1972, 5, 102 CrossRef CAS; (b) D. O'Hagan, Chem. Soc. Rev., 2008, 37, 308 RSC; (c) L. Hunter, Beilstein J. Org. Chem., 2010,(6), No. 38, DOI:10.3762/bjoc.6.38; (d) L. E. Zimmer, C. Sparr and R. Gilmour, Angew. Chem., Int. Ed., 2011, 50, 11860 CrossRef CAS PubMed.
- For selected examples see: (a) K. S. Buckton and R. G. Azrak, J. Chem. Phys., 1970, 52, 5652 CrossRef CAS; (b) K. Hagen and K. Hedberg, J. Am. Chem. Soc., 1973, 95, 8263 CrossRef CAS; (c) J. Huang and K. Hedberg, J. Am. Chem. Soc., 1989, 111, 6909 CrossRef CAS; (d) D. A. Dixon and B. E. Smart, J. Phys. Chem., 1991, 95, 1609 CrossRef CAS; (e) D. O'Hagan, C. Bilton, J. A. K. Howard, L. Knight and D. J. Tozer, J. Chem. Soc., Perkin Trans. 2, 2000, 605 RSC; (f) C. R. S. Briggs, M. J. Allen, D. O'Hagan, D. J. Tozer, A. M. Z. Slawin, A. E. Goeta and J. A. K. Howard, Org. Biomol. Chem., 2004, 2, 732 RSC; (g) C. R. S. Briggs, D. O'Hagan, H. S. Rzepa and A. M. Z. Slawin, J. Fluorine Chem., 2004, 125, 19 CrossRef CAS; (h) M. Morgenthaler, E. Schweizer, A. Hoffman-Röder, F. Benini, R. E. Martin, G. Gaeschke, B. Wagner, H. Fischer, S. Bendels, D. Himmerli, J. Schneider, F. Diederich, M. Kansy and K. Müller, ChemMedChem, 2007, 2, 1100 CrossRef CAS PubMed; (i) N. E. J. Gooseman, D. O'Hagan, M. J. G. Peach, A. M. Z. Slawin, D. J. Tozer and R. J. Young, Angew. Chem., Int. Ed., 2007, 46, 5904 CrossRef CAS PubMed; (j) G. Deniau, A. M. Z. Slawin, T. Lebl, F. Chorki, J. P. Issberner, T. van Mourik, J. M. Heygate, J. J. Lambert, K. T. Sillar and D. O'Hagan, ChemBioChem, 2007, 8, 2265 CrossRef CAS PubMed; (k) C. Bucher, C. Sparr, W. B. Schweizer and R. Gilmour, Chem.–Eur. J., 2009, 15, 7637 CrossRef CAS PubMed; (l) C. Sparr and R. Gilmour, Angew. Chem., Int. Ed., 2010, 49, 6520 CrossRef CAS PubMed; (m) C. Sparr, E.-M. Tanzer, J. Bachmann and R. Gilmour, Synthesis, 2010, 1394 CAS; (n) S. Paul, W. B. Schweizer, M.-O. Ebert and R. Gilmour, Organometallics, 2010, 29, 4424 CrossRef CAS; (o) C. Sparr, E. Salamanova, W. B. Schweizer, H. M. Senn and R. Gilmour, Chem.–Eur. J., 2011, 17, 8850 CrossRef CAS PubMed; (p) S. Paul, W. B. Schweizer, G. Rugg, H. M. Senn and R. Gilmour, Tetrahedron, 2013, 69, 4647 CrossRef.
- (a) L. Goodman, H. Gu and V. Pophristic, J. Phys. Chem. A, 2005, 109, 1223 CrossRef CAS PubMed; (b) P. R. Rablen, R. W. Hoffmann, D. A. Hrovat and W. T. Borden, J. Chem. Soc., Perkin Trans. 2, 1999, 1719 RSC; (c) D. Y. Buissonnneaud, T. van Mourik and D. O'Hagan, Tetrahedron, 2010, 66, 2196 CrossRef; (d) M. Baranac-Stojanović, RSC Adv., 2014, 4, 43834 RSC; (e) For a recent study of the gauche effect in 2,3-difluorobutane see S. J. Fox, S. Gourdain, A. Coulthurst, C. Fox, I. Kuprov, J. W. Essex, C. K. Skylaris and B. Linclau, Chem.–Eur. J., 2015, 21, 1682 CrossRef CAS PubMed.
- (a) C. Sparr, W. B. Schweizer, H. M. Senn and R. Gilmour, Angew. Chem., Int. Ed., 2009, 121, 3111 CrossRef; (b) E.-M. Tanzer, L. E. Zimmer, W. B. Schweizer and R. Gilmour, Chem.–Eur. J., 2012, 18, 11334 CrossRef CAS PubMed; (c) E.-M. Tanzer, W. B. Schweizer, M.-O. Ebert and R. Gilmour, Chem.–Eur. J., 2012, 18, 2006 CrossRef CAS PubMed; (d) Y. P. Rey and R. Gilmour, Beilstein J. Org. Chem., 2013, 9, 2812 CrossRef PubMed; (e) Y. P. Rey, L. E. Zimmer, C. Sparr, E.-M. Tanzer, W. B. Schweizer, H. M. Senn, S. Lakhdar and R. Gilmour, Eur. J. Org. Chem., 2014, 1202 CrossRef CAS; (f) I. G. Molnár, E.-M. Tanzer, C. Daniliuc and R. Gilmour, Chem.–Eur. J., 2014, 20, 794 CrossRef PubMed.
- (a) D. O'Hagan and H. S. Rzepa, Chem. Commun., 1997, 645 RSC; (b) M. L. DeRider, S. J. Wilkens, M. J. Waddell, L. E. Bretscher, F. Weinhold, R. T. Raines and J. L. Markley, J. Am. Chem. Soc., 2002, 124, 2497 CrossRef CAS PubMed; (c) For an excellent review see: S. Purser, P. R. Moore, S. Swallow and V. Gouverneur, Chem. Soc. Rev., 2008, 37, 320 RSC.
- (a) L. Hunter and D. O'Hagan, Org. Biomol. Chem., 2008, 6, 2843 RSC; (b) D. Farran, A. M. Z. Slawin, P. Kirsch and D. O'Hagan, J. Org. Chem., 2009, 74, 7168 CrossRef CAS PubMed; (c) L. Hunter, P. Kirsch, A. M. Z. Slawin and D. O'Hagan, Angew. Chem., Int. Ed., 2009, 48, 5457 CrossRef CAS PubMed; (d) P. Kirsch, A. Hahn, R. Fröhlich and G. Haufe, Eur. J. Org. Chem., 2006, 4819 CrossRef CAS; (e) M. Nicoletti, M. Bremer, P. Kirsch and D. O'Hagan, Chem. Commun., 2007, 5075 RSC.
- D. Stierli, J. J. Taylor, H. Walter, P. A. Worthington and R. Rajan, Preparation of N-(1-alkyl-2-phenylethyl)azole carboxamides as agrochemical fungicides, PCT Int. Appl. 2007, WO 2007141009 A1 20071213, 2007.
- For a recent example see: Z. Yuan, H.-Y. Wang, X. Mu, P. Chen, Y.-L. Guo and G. Liu, J. Am. Chem. Soc., 2015, 137, 2468 CrossRef CAS PubMed.
- (a) J. C. Carretero, J. L. Garcia Ruano, M. C. Martinez and J. H. Rodriguez, Tetrahedron, 1985, 41, 2419 CrossRef CAS; (b) J. C. Carretero, J. L. Garcia Ruano, M. C. Martinez and J. H. Rodriguez, An. Quim., Ser. C, 1987, 83, 300 CAS.
- (a) L. S. Jeong, M. C. Nicklaus, C. George and V. E. Marquez, Tetrahedron Lett., 1994, 35, 7569 CrossRef CAS; (b) L. S. Jeong, M. C. Nicklaus, C. George and V. E. Marquez, Tetrahedron Lett., 1994, 35, 7573 CrossRef.
- (a) K. S. MacMillan, J. Naidoo, J. Liang, L. Melito, N. S. Williams, L. Morlock, P. J. Huntington, S. J. Estill, J. Longgood, G. L. Becker, S. L. McKnight, A. A. Pieper, J. K. De Brabander and J. M. Ready, J. Am. Chem. Soc., 2011, 133, 1428 CrossRef CAS PubMed; (b) J. Naidoo, H. De Jesus-Cortes, P. Huntington, S. Estill, L. K. Morlock, R. Starwalt, T. J. Mangano, N. S. Williams, A. A. Pieper and J. M. Ready, J. Med. Chem., 2014, 57, 3746 CrossRef CAS PubMed; (c) For a review see: A. A. Pieper, S. L. McKnight and J. M. Ready, Chem. Soc. Rev., 2014, 43, 6716 RSC and references cited therein.
- H.-Y. Wu, C.-W. Chang and R.-J. Chein, J. Org. Chem., 2013, 78, 5788 CrossRef CAS PubMed.
- ESI.†.
- For bond lengths of organic compounds determined by X-ray and neutron diffraction see: F. H. Allen, O. Kennard, D. G. Watson, L. Brammer, A. G. Orpen and R. Taylor, J. Chem. Soc., Perkin Trans. 2, 1987, S1–S19 RSC.
- H.-B. Bürgi and J. D. Dunitz, J. Am. Chem. Soc., 1987, 109, 2924 CrossRef.
- D. Asanuma, Y. Takaoka, S. Namiki, K. Takikawa, M. Kamiya, T. Nagano, Y. Urano and K. Hirose, Angew. Chem., Int. Ed., 2014, 53, 6085 CrossRef CAS PubMed.
- W. B. Dolbier Jr, Guide to Fluorine NMR for Organic Chemists, Wiley, 2009 Search PubMed.
- R. Contreras and J. Peralta, Prog. Nucl. Magn. Reson. Spectrosc., 2000, 37, 321 CrossRef CAS.
- K. Pachler, Spectrochim. Acta, 1964, 20, 581 CrossRef CAS.
- A. Dahbi, S. Hamman and C. G. Béguin, Magn. Reson. Chem., 1986, 24, 337 CrossRef CAS.
- C. Thibaudeau, J. Plavec and J. Chattopadhyaya, J. Org. Chem., 1998, 63, 4967 CrossRef CAS.
- C. Altona, J. H. Ippel, A. J. A. Westra Hoekzema, C. Erkelens, M. Groesbeek and L. A. Donders, Magn. Reson. Chem., 1989, 27, 564 CrossRef CAS.
- (a) S. H. Vosko, L. Wilk and M. Nusair, Can. J. Phys., 1980, 58, 1200 CrossRef CAS; (b) C. Lee, W. Yang and R. G. Parr, Phys. Rev. B: Condens. Matter Mater. Phys., 1988, 37, 785 CrossRef CAS; (c) A. D. Becke, J. Chem. Phys., 1993, 98, 5648 CrossRef CAS; (d) P. J. Stephens, F. J. Devlin, C. F. Chabalowski and M. J. Frisch, J. Phys. Chem., 1994, 98, 11623 CrossRef CAS.
- E. R. Davidson and D. Feller, Chem. Rev., 1986, 86, 681 CrossRef CAS.
- E. Cancès, B. Mennucci and J. Tomasi, J. Chem. Phys., 1997, 107, 3032 CrossRef.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr, J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, T. Keith, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, J. M. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, Ö. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 09, revision D.01, Gaussian, Inc., Wallingford, CT, 2013 Search PubMed.
- (a) R. F. Ribeiro, A. V. Marenich, C. J. Cramer and D. G. Truhlar, J. Phys. Chem. B, 2011, 115, 14556 CrossRef CAS PubMed; (b) Y. Zhao and D. G. Truhlar, Phys. Chem. Chem. Phys., 2008, 10, 2813 RSC.
Footnotes |
| † Electronic supplementary information (ESI) available. CCDC 1048074–1048078. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c5sc00871a |
| ‡ X-ray crystallographer. |
| This journal is © The Royal Society of Chemistry 2015 |