New assembly design suitable for tower-shaped large size single-crystal diamond growth under high pressure and high temperature
Yadong
Li
a,
Xiaopeng
Jia
a,
Ning
Chen
a,
Liangchao
Chen
a,
Longsuo
Guo
a,
Chunxiao
Wang
a,
Gang
Li
a,
Shishuai
Sun
b and
Hong-an
Ma
*a
aState Key Lab of Superhard Materials, Jilin University, Changchun 130012, China. E-mail: jlu_li1989@163.com; Fax: +86 431 85168858; Tel: +86 431 85168858
bCollege of Science, Tianjin University of Technology, Tianjin 300384, China
First published on 21st November 2016
Abstract
During the synthesis of tower-shaped large size single crystal diamonds, a concave growth defect always occurs on the top surface of the diamonds when using the temperature gradient growth (TGG) method under high pressure and high temperature (HPHT) conditions. To explain the formation of these defects, we have analyzed experimental results, and performed theoretical calculations. Then, we designed a new assembly that is suitable for tower-shaped diamond growth. Compared to the traditional assembly, it can effectively eliminate the growth defects and provide a more suitable convection field for tower diamond growth. Simulation and experimental results confirm the effectiveness of the proposed design. The new assembly can be widely used in the industrial and commercial production of synthetic diamonds.
1. Introduction
Since the advent of synthetic diamonds in the 1950s,1 there has been great progress in their application in scientific research and in production/manufacturing environments. This is due to the exciting properties of diamonds, such as high thermal conductivity, chemical inertness, extreme hardness and electrical insulativity.2–9 For decades, the temperature gradient growth (TGG) method under high-pressure and high-temperature (HPHT) conditions has been an effective way to synthesize large single-crystal diamonds of gem quality for scientific research and commercial production.10–14 High-quality, large, single-crystal diamonds with a tower shape, which are composed of (111) and (100) crystal faces, can be widely used as precision and ultraprecision machining tools and the anvil of a diamond anvil cell (DAC). The tower-shaped diamond can also be processed into fine jewellery due to its similar morphology to natural diamond. Therefore, it is an important research topic to explore methods of how to synthesise gem-quality, tower-shaped, large, single-crystal diamonds by the TGG method under HPHT conditions.By using the traditional assembly with a tabular catalyst, different crystal morphologies of diamond have been synthesized. It has been shown that the traditional assembly is more suitable for high-quality sheet cubic diamond crystal growth.15,16 However, tower-shaped diamond synthesis based on the traditional assembly cannot easily obtain high-quality products, and defects such as metal inclusions regularly occur.17 Controlling the growth rate is an effective method to grow high-quality crystals.18,19 However, some defects still occur during the process of diamond growth that have not been solved. In our recent works, we found that diamonds always develop concave growth defects during the traditional synthesis process of tower-shaped diamonds. Generally, the traditional synthesis process, in which the catalyst is of a tabular shape, is not considered to be very suitable for tower-shaped diamond growth at this time.
Here, we design a new type of assembly that has been proven to be able to prevent growth defects. The finite element method (FEM) was used to simulate the temperature and convection fields of the catalysts in the assembly. The results of the simulation study support the validity of this design. Using this design, we conducted a large number of synthetic experiments and obtained results that confirm the effectiveness of the proposed design. Thus, a new assembly suitable for tower-shaped diamond growth has been manufactured, overcoming the main challenge impeding the further development of tower-shaped diamond synthesis to date.
2. Experimental details
Experiments on diamond crystallization were carried out in a China-type large volume cubic high-pressure apparatus (CHPA), as shown in Fig. 1(a), with a sample assembly of 38 × 38 mm2 under a pressure of 5.7 GPa and a temperature of 1300 °C. A schematic diagram of the high-pressure and high-temperature apparatus is shown in Fig. 1(b). High-purity graphite powder (99.9% purity) and NiMnCo alloy (70![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 25
25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 5 by wt%) were used as the carbon source and the catalyst/solvent, respectively. A diamond with a well-faceted {100} crystal face of 0.5 × 0.5 mm2 was used as the diamond seed crystal. The temperature was measured in each experiment using a Pt-30% RH/Pt-6% Rh thermocouple whose junction was placed near the crystallization sample. The pressure was estimated by the oil press load, which was calibrated by a curve that was established on the pressure-induced phase transitions of Bi, Tl, and Ba. After the HPHT experiments, the samples containing the synthesized diamond crystals were collected and placed in a bottle of a boiling mixture of H2SO4 and HNO3 to remove the remaining graphite and metal catalysts. The recovered diamonds were examined by optical microscopy (OM) to observe the crystal morphology and defects.
5 by wt%) were used as the carbon source and the catalyst/solvent, respectively. A diamond with a well-faceted {100} crystal face of 0.5 × 0.5 mm2 was used as the diamond seed crystal. The temperature was measured in each experiment using a Pt-30% RH/Pt-6% Rh thermocouple whose junction was placed near the crystallization sample. The pressure was estimated by the oil press load, which was calibrated by a curve that was established on the pressure-induced phase transitions of Bi, Tl, and Ba. After the HPHT experiments, the samples containing the synthesized diamond crystals were collected and placed in a bottle of a boiling mixture of H2SO4 and HNO3 to remove the remaining graphite and metal catalysts. The recovered diamonds were examined by optical microscopy (OM) to observe the crystal morphology and defects.
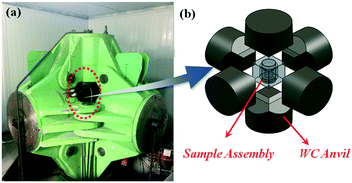 | ||
| Fig. 1 (a) A China-type large volume cubic high-pressure apparatus, (b) a schematic drawing: a high pressure cell used for diamond growth. | ||
The finite element method (FEM) was used to simulate the temperature and convection fields in the melted catalyst/solvent of the growth cell. A three-dimensional (3D) model of the prototype was constructed using SOLIDWORKS and imported into ANSYS software for analysis. Only the WC anvil and the sample assembly were considered for the analysis. Solid 69 element and Fluid 142 element were chosen for thermal-electrical-fluid analysis for meshing models. The growth cell was plane symmetric with symmetric loadings, so a 1/4 model can be used, which reduces the computational cost. The boundary conditions used in the finite element simulation and material parameters were originated from other previous reports.20–23
3. Results and discussion
3.1. Growth defect of tower-shaped diamond
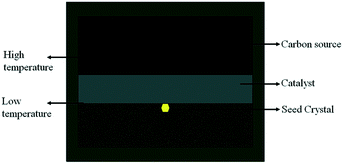
Diamond crystallization is established in the NiMnCo-C system at a pressure of 5.7 GPa and temperatures ranging from 1290 °C to 1300 °C. A long run time is applied to produce large diamonds by the TGG method. The diamond nucleation and growth are established with a catalyst height of 2.0 mm, and the tabular carbon source has a height of 4 mm with a 10 mm diameter in the traditional assembly.The traditional diamond growth assembly that uses a tabular-shaped catalyst is shown in Fig. 2. In our recent works, many experiments were carried out for the synthesis of type Ib diamond using the traditional diamond growth assembly, and we found that a long run time could produce large size tower-shaped diamonds, but these diamonds possess growth defects on their top surface. Fig. 3(a)–(d) show OM images of the obtained diamonds. It can be seen that the obtained diamond exhibits a concave growth defect depression in the middle of the upper crystal face, which increases in size and depth as the crystal grows. In addition, we can observe that the defect gradually expands “inside out” at the centre of the top surface. With an increase in the growth time, the defect becomes more serious. This defect seriously influences the crystal quality and precludes the utilization of the diamond.
 | ||
| Fig. 3 Optical images of the growth defect of diamond crystals synthesized from NiMnCo-C systems by the traditional assembly. (a) for 30 h, (b) for 32 h, (c) for 34 h, (d) for 36 h. | ||
In general, tower-shaped diamond crystals have a higher height-diameter ratio than sheet cubic-shaped diamonds, which means that the top surface of the tower-shaped diamond is closer to the surplus carbon source than that of the sheet-shaped diamond during the process of growth. In ref. 21, upon using a tabular carbon source, Ma found that the shape of the surplus carbon source transforms from tabular into a spherical cap. In addition, as the tower-shaped diamond continues to grow, the crystal top face comes so close to the surplus carbon source that it nearly comes into contact with it. According to the melt–solvent theory, the temperature gradient between the carbon source and the diamond seed is the driving force for diamond growth, and the carbon element can be transported from the carbon source to the crystal surface by the “power”. The distance between the diamond and the surplus carbon source becomes shorter and shorter as the diamond grows upward, and the temperature gradient between the carbon source and the crystal is also reduced. Thus, the carbon element cannot be effectively transported to the crystal surface, causing the defect on the crystal surface.
3.2. Formation mechanism of the defect
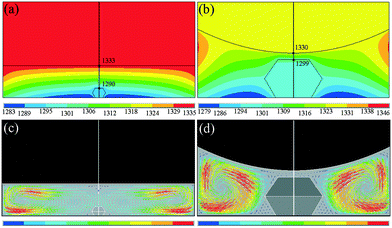
To explore the reason for the formation of the defect, we have simulated the temperature and convection fields of the diamond growth cell by the FEM. Fig. 4(a) and (b) show the distributions of the temperature fields of the growth cell for the synthesis times of 0.5 h and 35 h, respectively. From the simulation results in Fig. 4(a), we can see that the temperature gradient between the carbon source and the crystal surface is 43 °C, and the temperature gradient in Fig. 4(b) is 31 °C. The temperature gradient is reduced, which is consistent with our speculation above. The driving force of diamond growth becomes weaker in the later portion of the 35 h than in the initial 0.5 h. Additionally, we can see that the growth space in the later portion of the 35 h is not sufficient for diamond growth and that on the top surface is much closer to the carbon source than that for the 0.5 h synthesis time.In general, the greater the convection intensity of the carbon is, the faster is the transport of the carbon atoms from the source to the seed. Fig. 4(c) and (d) show the distributions of the carbon convection fields for the synthesis times of 0.5 h and 35 h, respectively. The results of the theoretical simulation indicate that the distributions of the carbon convection fields show visible differences in the diamond growth cell, and the convection intensity at the edge of the cavity is stronger than that in the centre area around the diamond, leading the {111} surface to grow faster than the {100} surface. More importantly, the crystal top surface convection intensity in Fig. 4(d) is much weaker than that in Fig. 4(c). This type of convection trend must effectively affect the diamond growth. Convection is the main transport method of carbon atoms. As time elapses, the lateral surface of the diamond grows faster and faster, while the growth rate on the top surface gradually slows down. On the top surface of the diamond, the convection is also significantly different. The convection on the middle area of the top surface is the weakest, while that far away from the centre of the top surface gradually becomes stronger. This indicates that the ability of obtaining the carbon source is not homogeneous on the top surface. Thus, the unmatched relative growth rates explain the appearance of the concave growth defects.
3.3. New assembly design for tower-shaped diamond growth
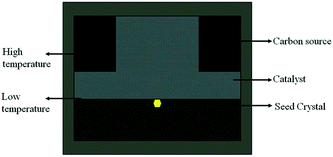
From the formation mechanism of the growth defect we have discussed above, we know that the carbon convection field is significant for diamond growth, especially the convection intensity on the top {100} surface of the diamond. Meanwhile, enough growth space in the vertical direction is necessary for the tower-shaped diamond growth. The carbon convection field in the HPHT metal solvent is mainly determined by the sample assembly, such as the shape of the catalyst. To solve the difficulty of tower-shaped growth experienced when using the traditional sample assembly with a tabular catalyst, we first developed a new assembly, as shown in Fig. 5, which is expected to result in a better convection field and larger growth space for tower-shaped diamond growth. In this assembly, we use a cylindrical catalyst with a diameter of 6 mm and a height of 4 mm instead of the centre zone of the carbon source, which provides a larger growth space in the vertical direction.To confirm the effectiveness of the newly designed assembly, the FEM was used to simulate the temperature and convection fields of the catalyst again. Fig. 6(a) shows the temperature field distribution of the growth cell. It may be observed that the temperature distribution is different from that of the traditional assembly, although both are symmetrical, with the high-temperature region near the carbon source and the lower-temperature region near the surface of the crystal. The difference is in the distribution of the temperature on the top surface of the diamond. The newly designed assembly possesses a more uneven temperature distribution because of the larger catalytic space. This difference in the temperature distribution changes the convection situation in the molten metallic solvent, providing a large convection intensity on the top surface of the growing diamond.
The simulation result of the convection field distribution is shown in Fig. 6(b). We may observe that the convection distribution in the convex shape catalyst is different from that in the tabular catalyst. The strong convection region is near the crystal growth area, and the weak convection region is near the outer edge of the catalyst, which is contrary to the case for the tabular catalyst convection field. We are excited about the result that there is a strong convection intensity on the top surface of the diamond. This convection trend is of great help to eliminate the surface growth defects, making the tower-shaped diamond growth more suitable.
To verify our simulated results, experiments were performed using the newly designed assembly on the CHPA in NiMnCo-C systems at a pressure of 5.7 GPa and a temperature of 1300 °C. Fig. 7 shows the experimental results. It can be seen that there are no defects on the top surface of the synthesized diamonds in all experiments. With the increasing growth time, the sizes of the diamonds are gradually enlarged, and they grow faster in the vertical direction, achieving a higher height-diameter ratio, which is determined by the convection distribution and growth space in the growth cell. The results indicate that the newly designed assembly can not only eliminate defects effectively but can also be more conducive to gem-quality large size tower-shaped diamond growth. The use of the convex shape catalyst has successfully demonstrated that high-quality, large, tower-shaped diamonds can be synthesized successfully.
3.4. Applications of gem-quality tower-shaped diamonds
Fig. 8 shows applications of the synthetic gem-quality, single-crystal tower-shaped diamonds. Fig. 8(a) shows a synthetic type Ib diamond anvil of a DAC. Fig. 8(b) shows a circular-shaped jewellery and Fig. 8(c) and (d) show multi-rhombus-shaped jewellery which are both processed from type Ib tower-shaped diamonds. The synthetic type Ib diamonds contain fewer impurities and can be sold for a lower price than natural diamonds. For these reasons, synthetic diamond crystals are expected to be of great use as diamond anvils and other parts of an apparatus, for high-pressure studies in the fields of material science and commercial jewellery production. | ||
| Fig. 8 Optical images of the synthetic type Ib, gem-quality, single-crystal, tower-shaped diamonds as the anvil of a DAC (a) and jewellery (b)–(d). | ||
4. Conclusions
The formation mechanism of the growth defect in tower-shaped diamonds has been explained by the FEM simulation results. We first developed a new assembly to solve the difficulty. As expected, the convection simulation results show that the new design assembly has a strong convection intensity on the top {100} crystal face of the grown diamond, which not only can effectively eliminate the defect but is also more suitable for tower-shaped diamond growth. Results of the long run time synthetic experiment confirm the effectiveness of the new design. This design is more suitable for large, gem-quality, tower-shaped diamond growth than the traditional assembly. Manmade diamonds have a broad prospect of application in high-pressure studies, commercial jewellery production, and other fields.Acknowledgements
This project was supported by the National Natural Science Foundation of China (grant no. 51172089 11504267) and the Graduate Innovation Fund of Jilin University (grant no. 2016065).Notes and references
- F. P. Bundy, H. T. Hall, H. M. Strong and R. H. Wentorf, Nature, 1955, 176, 51 Search PubMed.
- S. Koizumi, K. Watanabe, M. Hasegawa and H. Kanda, Science, 2001, 292, 1899 Search PubMed.
- D. C. Shin, B. V. Sarada, D. A. Tryk and A. Fujishima, Anal. Chem., 2003, 75, 530 Search PubMed.
- Y. D. Kim, W. Choi, H. Wakimoto, S. Usami, H. Tomokage and T. Ando, Appl. Phys. Lett., 1999, 75, 3219 Search PubMed.
- M. Barletta, G. Rubino, R. Valle and R. Polini, ACS Appl. Mater. Interfaces, 2012, 4, 694 Search PubMed.
- X. B. Liu, X. P. Jia, Z. F. Zhang, Y. Li, M. H. Hu, Z. X. Zhou and H. A. Ma, Cryst. Growth Des., 2011, 11, 3844 Search PubMed.
- H. Kanda, M. Akaishi and S. Yamaoka, Diamond Relat. Mater., 1999, 8, 1441 Search PubMed.
- G. D. Fuchs, G. Burkard, P. V. Klimov and D. D. Awschalom, Nat. Phys., 2011, 7, 789 Search PubMed.
- P. Achatz, O. A. Williams, P. Bruno, D. M. Gruen, J. A. Garrido and M. Stutzmann, Phys. Rev. B: Condens. Matter Mater. Phys., 2006, 74, 155429 Search PubMed.
- Y. Li, X. P. Jia, W. Shi, S. L. Leng, H. A. Ma, S. S. Sun, F. B. Wang, N. Chen and Y. Long, Int. J. Refract. Hard Met., 2014, 43, 147 Search PubMed.
- R. C. Burns, J. O. Hansen, R. A. Spits, M. Sibanda, C. M. Welbourn and D. L. Welch, Diamond Relat. Mater., 1999, 8, 1433 Search PubMed.
- X. B. Liu, X. P. Jia, X. K. Guo, Z. F. Zhang and H. A. Ma, Cryst. Growth Des., 2010, 10, 2895 Search PubMed.
- S. S. Sun, X. P. Jia, B. M. Yan, F. B. Wang, N. Chen, Y. Li and H. A. Ma, CrystEngComm, 2014, 16, 2290 Search PubMed.
- S. S. Sun, X. P. Jia, B. M. Yan, F. B. Wang, Y. Li, N. Chen and H. A. Ma, Diamond Relat. Mater., 2014, 42, 21 Search PubMed.
- M. H. Hu, N. Bi, S. S. Li, T. C. Su, Q. Hu, X. P. Jia and H. A. Ma, Int. J. Refract. Hard Met., 2015, 48, 61 Search PubMed.
- M. H. Hu, H. A. Ma, B. M. Yan, Y. Li, Z. C. Li, Z. X. Zhou and X. P. Jia, Cryst. Growth Des., 2012, 12, 518 Search PubMed.
- M. H. Hu, S. S. Li, H. A. Ma, T. C. Su, X. L. Li, Q. Hu and X. P. Jia, Chin. Phys. B, 2012, 21, 098101 Search PubMed.
- H. Sumiya, N. Toda and S. Satoh, J. Cryst. Growth, 2002, 237, 1281 Search PubMed.
- H. Kanda, Braz. J. Phys., 2000, 30, 482 CrossRef CAS.
- Y. D. Li, X. P. Jia, B. M. Yan, N. Chen, C. Fang, Y. Li, S. S. Sun and H. A. Ma, RSC Adv., 2016, 6, 40330 Search PubMed.
- Z. C. Li, X. P. Jia, G. F. Huang, M. H. Hu, Y. Li, B. M. Yan and H. A. Ma, Chin. Phys. B, 2013, 22, 014701 Search PubMed.
- R. Li, H. A. Ma, Q. G. Han, Z. Z. Liang, B. M. Yan, W. Q. Liu and X. P. Jia, High Pressure Res., 2007, 27, 249 Search PubMed.
- R. F. Brooks, I. Egry, S. Seetharaman and D. Grant, High Temp. - High Pressures, 2001, 33, 631 Search PubMed.
| This journal is © The Royal Society of Chemistry 2017 |