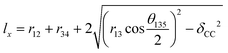Sign-tunable Poisson's ratio in semi-fluorinated graphene†
Rui
Qin‡
*a,
Jiaxin
Zheng‡
b and
Wenjun
Zhu
a
aNational Key Laboratory of Shock Wave and Detonation Physics, Institute of Fluid Physics, Mianyang, 621900, P. R. China. E-mail: qinrui.phy@outlook.com
bSchool of Advanced Materials, Peking University, Shenzhen Graduate School, Shenzhen, 518055, P. R. China
First published on 23rd September 2016
Abstract
Poisson's ratio is a fundamental property of a material which reflects the transverse strain response to the applied axial strain. Negative Poisson's ratio is allowed theoretically, but is rare in nature. Besides the discovery and tailoring of bulk auxetic materials, recent studies have also found a negative Poisson's ratio in nanomaterials, while their negative Poisson's ratio is mainly based on conventional rigid mechanical models as bulk auxetic materials. In this work, we report the existence of in-plane negative Poisson's ratio in a two-dimensional convex structure of newly synthesized semi-fluorinated graphene by using first-principles calculations. In addition, the sign of the Poisson's ratio can be tuned by the applied strain. Interestingly, we find that this unconventional negative Poisson's ratio cannot be explained by conventional rigid mechanical models but originates from the enhanced bond angle strain over the bond strain due to chemical functionalization. This new mechanism of auxetics extends the scope of auxetic nanomaterials and can serve as design principles for future discovery and design of new auxetic materials.
Introduction
Materials with a negative Poisson's ratio, the so-called auxetic materials, become wider rather than thinner when stretched. Although this counter intuitive property is allowed by the theory of elasticity in principle, it is rare in nature. Moreover, negative Poisson's ratio typically results in enhanced toughness, improved shear stiffness, and enhanced vibrational damping and shock absorption.1 It can be applied in many fields, such as fasteners,2 sandwich panels for aircraft or automobiles,3 piezocomposites,4,5 filters,6 and superior dampers.7 Thus great interest has been aroused in searching for and designing materials with a negative Poisson's ratio. Many cubic metals8 and α-cristobalite9 are reported to have a negative Poisson's ratio, and some materials are found to exhibit a negative Poisson's ratio near phase transition.10–13 In addition, special structures are also engineered to obtain a negative Poisson's ratio, such as molecular networks,14 reentrant foam15,16 or honeycomb,17–19 hierarchical structures,20 special origami structures21 and hinged structures.22,23 However, such current studies of tailored auxetic materials have focused on bulk materials and are mainly directed by rigid mechanical models. In this model, the building unit is supposed to be rigid, and the negative Poisson's ratio is achieved by carefully designed microstructures composed of rigid units.1,24 For example, the reentrant honeycomb is one such special structure, which has an inverted or reentrant cell structure and can be viewed as coupled hinges. When stretched, the reentrant cells unfold and result in a negative Poisson's ratio.On the other hand, recently, nanoscale auxetic materials have also been extensively studied. Theoretically, Özçelik et al.25 predicted a single-layer honeycomb-like allotrope of silica (hα silica) with a negative Poisson's ratio, and Zhang et al.26 proposed a two-dimensional metastable allotrope of carbon(penta-graphene) with a negative Poisson's ratio. Jiang et al.27 reported a negative Poisson's ratio in single-layer black phosphorus. Grima et al.28 showed that graphene could exhibit a negative Poisson's ratio by introducing vacancy defects. Ho et al.29 found negative Poisson's ratios in several nanoscale metal plates. Experimentally, Hall et al.30 found that the sign of the Poisson's ratio of carbon nanotube sheets can be tuned by adjusting the proportion of multi-walled carbon nanotubes in the carbon nanotube sheets. Nevertheless, the mechanisms of auxeticity in these nanomaterials are similar to those discovered in bulk auxetic materials previously. For example, the negative Poisson's ratios in hα silica, penta-graphene, black phosphorus, and graphene with vacancy defects can be explained by rigid mechanical models. The negative Poisson's ratio in metal nanoplates originates from free surface effects and phase transformations, while phase transformation also leads to auxeticity in many bulk materials. Hereto, the question has been raised: is there any new mechanism to realize a negative Poisson's ratio? If it exists, it will extend fundamental understanding and widen ways to generate and tailor auxetic materials.
Graphene is one of the two-dimensional wonder materials due to its exceptional mechanical and electronic properties.31–35 Chemical functionalization can make it even more competitive by further manipulating its optical, electronic, or magnetic properties. Recently, a single-sided fluorinated graphene of boat structure (C2F boat), which is viewed as a promising two-dimensional semiconductor, has been synthesized on a SiC(0001) substrate by exposing graphene to XeF2 at the temperature of 200 °C for 3–4 hours,36 and a quasi-freestanding C2F boat structure is further obtained experimentally by hydrogen intercalation.37 We also notice that the C2F chair structure has been produced recently by exposing both sides of graphene to XeF2 in a Teflon container at 70 °C.38 While previous calculations39,40 show that the C2F boat structure is energetically more stable than the C2F chair structure due to the formation of a double bond in the C2F boat structure, the C2F chair structure may also be obtained experimentally by adjusting the reacting path which depends on the reaction conditions, and Stine et al.41 have already showed that graphene fluorination depends on several reaction conditions, including XeF2 exposure and the substrate. Extensive theoretical studies have been conducted on electronic, optical or magnetic properties of semi-fluorinated graphene.40,42–48 In this work, we study the mechanical properties of the C2F boat structure by using first-principles calculations and show that the C2F boat structure exhibits a directional auxetic response. Moreover, the sign of Poisson's ratio can be tuned by the strain in an appropriate direction. Interestingly, unlike former engineered auxetic materials with a reentrant structure which can produce a negative Poisson's ratio based on rigid mechanical models, here the C2F boat structure has a convex hexagonal lattice, and this unusual auxeticity originates from the competition between angle strain and bond strain of the hexagonal ring due to chemical functionalization.
Models and methods
The C2F boat structure is studied by using a periodic supercell model. A vacuum of at least 10 Å is chosen to eliminate the interaction between adjacent sheets. The mechanical and electronic properties of the C2F boat structure are investigated by first-principles density functional theory (DFT) calculations, using the Quantum ESPRESSO package.49 Phonon frequencies are calculated using DFT perturbation theory.50 The generalized gradient approximation (GGA) of the Perdew–Burke–Ernzerhof (PBE) form51 is employed for the exchange–correlation functional. Ultrasoft pseudopotentials52 from the GBRV high-throughput pseudopotentials set,53 a kinetic energy cutoff of 50 Ry, and a charge density cutoff of 300 Ry are used through the calculations. A 20 × 32 × 1 Monkhorst–Pack k-point mesh54 for the Brillouin zone sampling is used for the unit cell without strain, and this sampling is scaled according to the size of the supercells in our calculations. The structures are optimized until the maximum force allowed on each atom is less than 3 × 10−4 eV Å−1.Results and discussion
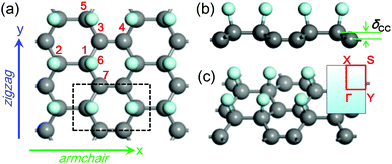
Fig. 1 shows the optimized C2F boat structure without strain, which possesses the Pmm2 symmetry with an orthorhombic lattice. The unit cell contains four carbon atoms and two fluorine atoms, and the optimized lattice constants, lx and ly, are 4.42 and 2.57 Å, respectively. Unlike graphene where all carbon atoms are sp2 hybridized, two carbon atoms in the C2F boat structure become sp3 hybridized after fluorination, while the two left carbon atoms remain sp2 hybridized and form double bonds. For convenience of discussion, we label the atoms as in Fig. 1a. There are three inequivalent C–C bonds in the structure: the C1–C2, C1–C3, and C3–C4 bonds, and one C–F bond: the C1–F6 bond. Their bond lengths r12, r13, r34, and r16 are 1.63, 1.53, 1.37, and 1.42 Å, respectively, which show pronounced characters of the corresponding bond types. Due to sp3 hybridization, carbon atoms are no longer in the same plane, and a small buckling, δCC, of 0.42 Å appears between carbon atoms of different hybridizations (Fig. 1b). The bond angles between carbon atoms, θ213 and θ135, are 117.82° and 114.38°, respectively, indicating the distorted sp3 and sp2 characters of carbon atoms. These structure parameters agree well with previous calculations39 and the experimental values.36We then calculate the electronic structure of the C2F boat structure without strain. The band structure shows that it is a semiconductor with an indirect band gap (Fig. S1a†). We also investigate the phonon dispersion to study the lattice dynamics. If imaginary frequency (conventionally drawn as negative) appears in the phonon spectra, the vibrational displacement of atoms will diverge with time, and the structure is dynamically unstable. The phonon dispersion in the absence of strain is shown in Fig. 2a. No imaginary mode is found in the Brillouin zone, which agrees with previous calculations40 and confirms that the C2F boat structure is stable without strain. Like graphene, three distinct acoustic modes appear in the phonon spectra of the C2F boat structure: the in-plane transverse (TA), in-plane longitudinal (LA), and out-of plane (ZA) modes. It is well known that the ZA mode of graphene shows a quadratic dispersion due to the high D6h point-group symmetry of graphene,55 and graphene has a finite phonon density of states (DOS) at zero frequency. However, the C2F boat structure has a lower symmetry, and all three acoustic modes display the conventional linear dispersion around the Γ point, which induces a vanishing phonon DOS at zero frequency (Fig. 2b). To better characterize the C2F boat structure, we also plot the projected phonon DOS, which shows that the strong double bonds between sp2 hybridized carbon atoms (C3 and C4) lead to the dispersionless modes at high frequencies around 1500 cm−1 (Fig. 2b).
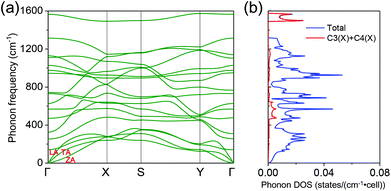 | ||
| Fig. 2 (a) Phonon band structure of C2F boat structure without strain. (b) Total and projected phonon density of states (DOS) of C2F boat structure without strain. | ||
Due to the lower symmetry than graphene, the C2F boat structure is expected to exhibit a significant anisotropic mechanical response to strain. We study the effect of uniaxial stress along two special directions: the zigzag, ZZ, (y axis in Fig. 1(a)) and armchair, AC, (x axis in Fig. 1(a)) directions. In our calculations, strain ε is defined as ε = (a − a0)/a0, where a and a0 are lattice constants with and without strain, respectively. ZZ and AC strains are applied by gradually increasing the corresponding axial lattice constants, while the transverse lattice constant is optimized to relax the other stress components. Here the axial direction is the strain direction, and the transverse direction is the in-plane direction perpendicular to the strain direction.
The ideal strength is the highest possible strength of a material, which is an important mechanical property characterizing the material stability. We first investigate the ideal strength for the C2F boat structure under uniaxial stress from the change of stress. The stress at various uniaxial strains is shown in Fig. 3a. Under small strains, the stress–strain curves coincide for both types of uniaxial strains. The stresses increase linearly with respect to strains, indicating that the system is in the harmonic region. As the system goes into the anharmonic region, stress shows large anisotropy for ZZ and AC strains. Stress increases more quickly under the ZZ strain than under the AC strain, and reaches the maximum at strains of 26% and 11% for ZZ and AC strains, respectively. Although the ideal strengths are excitingly large, previous studies of graphene56–58 suggest that phonon instability might occur before mechanical failure. In order to check the existence of phonon instability in the C2F boat structure, we calculate the phonon spectrum with the increasing uniaxial strains. Imaginary phonon frequencies are found to appear near the zone center along the Γ–Y path when the ZZ strain approaches 24% (Fig. 4a), which is smaller than the critical strain of 26% from the stress–strain curve. Analysis of the eigenvectors of these long-wavelength soft modes shows that they are acoustic modes corresponding to vibration in the y direction. We also notice that a phonon gap opens around 900 cm−1, which may be used as a signature to spectroscopically determine a highly stretched C2F boat structure along the ZZ direction, while with the increasing AC strain, the phonon mode softens at the Y point, which is at the boundary of the Brillouin zone. Imaginary phonon frequency appears at the Y point at the AC strain of 10% (Fig. 4b), which is also smaller than the critical strain of 11% from the stress–strain curve. Analysis of the eigenvectors of the soft modes shows that they are out-of plane modes with vibration in the xz plane. The different critical strains and natures of soft modes show large anisotropy of the C2F boat structure under ZZ and AC strains. Hereafter we only focus on the mechanical properties below the critical strains, which are 24% and 10% for ZZ and AC strains, respectively.
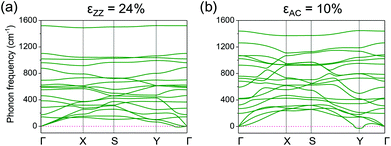 | ||
| Fig. 4 Phonon bands of C2F boat structure under (a) ZZ strain of 24% and (b) AC strain of 10%, respectively. Imaginary phonon frequencies (conventionally drawn as negative) appear in the two cases. | ||
We next investigate the lattice response to the uniaxial stress. The transverse strains are shown in Fig. 3b as the function of the axial strains. At small axial strains, the responses of transverse strain nearly coincide for both types of uniaxial stress, and the lattices both shrink in the transverse direction. But for large axial strains, the transverse strains respond quite differently for the AC and ZZ strains. In the AC strain case, the lattice keeps shrinking in the transverse direction in the considered strain range. By contrast, in the ZZ strain case, the transverse lattice shrinks more and more slowly with the increasing ZZ strain and reaches a minimum at the strain of 9.0%. More surprisingly, when the ZZ strain is larger than 9.0%, the transverse lattice even begins to expand. When the ZZ strain is slightly larger than 18%, the transverse strain becomes zero, which means that the transverse lattice constant returns to its original value without strain. This abnormal observation indicates the special anisotropic arrangement of chemical bonds and their different responses to uniaxial stress in the C2F boat structure. Poisson's ratio is calculated to describe the transverse lattice response to the uniaxial stress. To depict the nonlinear lattice response, Poisson's ratio is defined as 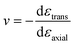 , where εtrans and εaxial denote transverse and axial strains, respectively. The Poisson's ratios for the AC and ZZ strains are shown in Fig. 3b. They are 0.035 and 0.034 for infinitesimal AC and ZZ strains, respectively. When εaxial is smaller than 0.5%, v changes only slightly for both strains. For larger AC strain, v changes non-monotonously and remains positive in the considered strain range, while v decreases monotonously for the ZZ strain up to the critical strain. Poisson's ratio decreases to zero at the ZZ strain of 9.0%, and then becomes negative and reaches −0.053 at the critical strain. Thus we obtain a sign-tunable in-plane Poisson's ratio in the C2F boat structure. This negative Poisson's ratio of −0.053 in semi-fluorinated graphene is two times larger than that of −0.027 in recently found black phosphorus.27 Moreover, the negative Poisson's ratio is in-plane in 2D semi-fluorinated graphene, while in 2D black phosphorus, the negative Poisson's ratio is along the out-of-plane direction.
, where εtrans and εaxial denote transverse and axial strains, respectively. The Poisson's ratios for the AC and ZZ strains are shown in Fig. 3b. They are 0.035 and 0.034 for infinitesimal AC and ZZ strains, respectively. When εaxial is smaller than 0.5%, v changes only slightly for both strains. For larger AC strain, v changes non-monotonously and remains positive in the considered strain range, while v decreases monotonously for the ZZ strain up to the critical strain. Poisson's ratio decreases to zero at the ZZ strain of 9.0%, and then becomes negative and reaches −0.053 at the critical strain. Thus we obtain a sign-tunable in-plane Poisson's ratio in the C2F boat structure. This negative Poisson's ratio of −0.053 in semi-fluorinated graphene is two times larger than that of −0.027 in recently found black phosphorus.27 Moreover, the negative Poisson's ratio is in-plane in 2D semi-fluorinated graphene, while in 2D black phosphorus, the negative Poisson's ratio is along the out-of-plane direction.
A negative Poisson's ratio usually occurs in a reentrant cell structure. Nevertheless, the C2F boat structure remains convex in the considered strain range (Fig. S2†). No phase transformation occurs, and the system retains the semiconducting nature (ESI Fig. 1†). In order to investigate the unconventional negative Poisson's ratio in the C2F boat structure, we first analyse the geometric structure. For the C2F boat structure, the lattice constants lx and ly can be expressed as
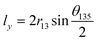 , respectively. The changes of related bond lengths and bond angles under uniaxial stress are shown in Fig. 5. Under tensile ZZ strain, the stretch of axial lattice ly requires the increase of r13 and θ135. In addition, the C1–C2 and C3–C4 bonds are perpendicular to the strain direction, and their bond lengths change very slightly. The alteration of buckling distance δCC is also quite small, which is within 0.014 Å under the considered ZZ strains. Therefore, the variation of the transverse lattice constant lx mainly depends on the competition of increasing r13 and θ135. Generally, the bond tends to rotate rather than to stretch in the presence of strain. In rigid mechanical models, the bond primarily rotates to release the strain. Consequently, the increase of bond angles dominates the change of lx, which leads to a positive Poisson's ratio. However, for the C2F boat structure, when ZZ strain increases, θ135 increases more and more slowly. Instead, the increase of bond length r13 dominates the stretch of axial lattice ly at large ZZ strain, and eventually causes the enlargement of lx and the negative Poisson's ratio. In the AC strain case, the C1–C2 and C3–C4 bonds are along the strain direction. Since the C1–C2 single bond is much weaker than the C3–C4 double bond, r12 increases much more rapidly than r34 under tensile AC strain and makes the primary contribution to the increase of lx. The C1–C3 bond stretches only slightly and rotates toward the strain direction. Thus θ135 decreases significantly and leads to a positive Poisson's ratio in the AC strain.
, respectively. The changes of related bond lengths and bond angles under uniaxial stress are shown in Fig. 5. Under tensile ZZ strain, the stretch of axial lattice ly requires the increase of r13 and θ135. In addition, the C1–C2 and C3–C4 bonds are perpendicular to the strain direction, and their bond lengths change very slightly. The alteration of buckling distance δCC is also quite small, which is within 0.014 Å under the considered ZZ strains. Therefore, the variation of the transverse lattice constant lx mainly depends on the competition of increasing r13 and θ135. Generally, the bond tends to rotate rather than to stretch in the presence of strain. In rigid mechanical models, the bond primarily rotates to release the strain. Consequently, the increase of bond angles dominates the change of lx, which leads to a positive Poisson's ratio. However, for the C2F boat structure, when ZZ strain increases, θ135 increases more and more slowly. Instead, the increase of bond length r13 dominates the stretch of axial lattice ly at large ZZ strain, and eventually causes the enlargement of lx and the negative Poisson's ratio. In the AC strain case, the C1–C2 and C3–C4 bonds are along the strain direction. Since the C1–C2 single bond is much weaker than the C3–C4 double bond, r12 increases much more rapidly than r34 under tensile AC strain and makes the primary contribution to the increase of lx. The C1–C3 bond stretches only slightly and rotates toward the strain direction. Thus θ135 decreases significantly and leads to a positive Poisson's ratio in the AC strain.
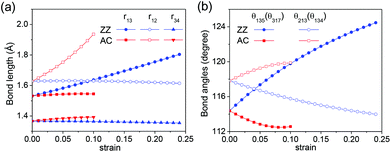 | ||
| Fig. 5 Strain dependencies of (a) bond lengths r13, r12, and r34 and (b) bond angles θ135(θ317) and θ213(θ134). | ||
Based on the analysis of the geometric structure, it is found that the negative Poisson's ratio can be attributed to the slowed increase of bond angle θ135 with the increasing ZZ strain. Namely, the C1–C3 bond prefers stretching rather than bending at large ZZ strains. This is different from the conventional cases, in which rigid bond length is assumed according to the rigid mechanical model. While elastic properties of one-dimensional single-walled carbon nanotubes can be investigated by the bond stretching and angle variation,59 this abnormal behavior of the two-dimensional C2F boat structure could be explained from the standpoint of the chemical strains of bond strain and bond angle strain. In the C2F boat structure, fluorination breaks the π bond between the C1 and C3 carbon atoms, and lowers the strength of the C1–C3 bond. C1 and C3 carbon atoms become sp3 and sp2 hybridized, respectively. It is well known that the ideal bond angles at the sp3 and sp2 hybridized carbon atoms are 109°28′ and 120°, respectively. However, θ135 has to be equal to θ317 due to the confinement of the atomic structure, and they are 114.38° for the C2F boat structure without strain. Thus both C1 and C3 atoms suffer from bond angle strain even without the presence of strain. When ZZ strain is applied, θ135 and θ317 increase with the increasing strain (Fig. 5b). Since θ317 is initially larger than the corresponding ideal angle (109°28′), the related bond angle strain always increases with the increasing strain. In contrast, as ZZ strain increases, θ135 first reaches its ideal bond angle (120°) and then continues to increase. Correspondingly, the related bond angle strain first decreases to zero and then increases with the increasing ZZ strain. Thus the total bond angle strain corresponding to θ135 and θ317 will increase rapidly at large ZZ strain. It is also noticed that the increase of θ135 (θ317) is accompanied by the decrease of θ134 (θ312). θ134 and θ312 change less than θ135 and θ317 (Fig. 5b), and the change trends of their bond angle strains are opposite to each other. Hence the total bond angle strain of θ134 and θ312 has a smaller contribution than that of θ135 and θ317. Overall, the rapidly increasing bond angle strain corresponding to θ135 and θ317 and weakened C1–C3 bond strength make it easier to stretch than to bend the C1–C3 bond to reduce the strain energy at large ZZ strains, which induces the negative Poisson's ratio and makes the transverse lattice constant recover to its original value and even become larger.
Based on our new mechanism of auxetics, we further study the lattice response of the C2H boat structure to uniaxial stress. The phonon dispersion indicates that the C2H boat structure is stable without strain (Fig. S3a†). The stress of the C2H boat structure reaches the maximum at ZZ strain of 30%. Nevertheless, phonon instability appears at the ZZ strain of 18% (Fig. S3b†). Before the critical strain, the negative Poisson's ratio occurs at the ZZ strain of 14% and reaches −0.013 at the ZZ strain of 18%. The maximum Poisson's ratio (−0.013) of the C2H boat structure is smaller than that (−0.053) of the C2F boat structure, which can be attributed to the fact that fluorination breaks the π bond between carbon atoms more strongly than the hydrogen does. For comparison, we also investigate the lattice response of pristine graphene to uniaxial stress. We find that the Poisson's ratio becomes negative at a large AC strain which is larger than 17% and very close to the critical AC strain of 19.4%![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 56 (Fig. S5†). We notice that a very recent molecular dynamics study shows that Poisson's ratio becomes negative after the AC strain of 6%.60 The large discrepancy in the turning strain at which the sign of Poisson's ratio changes may be due to the empirical potential used in the molecular dynamics calculations. Our study shows that chemical functionalization could enhance the auxeticity of graphene, and we believe that more unconventional auxetic nanomaterials with larger in-plane negative Poisson's ratio could be found in other chemical functionalized 2D materials.
56 (Fig. S5†). We notice that a very recent molecular dynamics study shows that Poisson's ratio becomes negative after the AC strain of 6%.60 The large discrepancy in the turning strain at which the sign of Poisson's ratio changes may be due to the empirical potential used in the molecular dynamics calculations. Our study shows that chemical functionalization could enhance the auxeticity of graphene, and we believe that more unconventional auxetic nanomaterials with larger in-plane negative Poisson's ratio could be found in other chemical functionalized 2D materials.
Conclusions
In summary, we find a sign-tunable in-plane Poisson's ratio in semi-fluorinated graphene. To our knowledge, the negative Poisson's ratio has been discovered for the first time in an experimentally synthesized convex two-dimensional nanomaterial beyond the conventional rigid mechanical model frame. Although negative Poisson's ratios are also recently found in other nanomaterials, the mechanism is mainly due to their intrinsic reentrant structures and based on conventional rigid mechanical models. Here we find that the negative Poisson's ratio in semi-fluorinated graphene is not based on the conventional rigid mechanical model but comes from the enhanced bond angle strain over the bond strain due to chemical functionalization with fluorine atoms. This study suggests that the new mechanism of chemical functionalization could also be an effective means of manufacturing new auxetic nanomaterials. Our finding greatly extends the scope of materials with potential applications in nanomechanical devices, and the new mechanism also provides new principles to design auxetic materials.Acknowledgements
We thank Professor Lin-Wang Wang, Dr Yi Xia, Ziyu Chen, and Shi Chen for helpful discussions. This work is supported by the National Natural Science Foundation of China (Grant No. 11204281) and the Shenzhen Science and Technology Research Grant (Grant No. JCYJ20140903101617271).Notes and references
- G. N. Greaves, A. L. Greer, R. S. Lakes and T. Rouxel, Nat. Mater., 2011, 10, 823–837 CrossRef CAS PubMed.
- J. B. Choi and R. S. Lakes, Cell. Polym., 1991, 10, 205–212 CAS.
- R. S. Lakes and R. Witt, Int. J. Mech. Eng. Educ., 2002, 30, 50–58 CrossRef.
- L. V. Gibiansky and S. Torquato, J. Mech. Phys. Solids, 1997, 45, 689–708 CrossRef.
- O. Sigmund, S. Torquato and I. A. Aksay, J. Mater. Res., 1998, 13, 1038–1048 CrossRef CAS.
- A. Alderson, J. Rasburn, S. Ameer-Beg, P. G. Mullarkey, W. Perrie and K. E. Evans, Ind. Eng. Chem. Res., 2000, 39, 654–665 CrossRef CAS.
- F. Scarpa, L. G. Ciffo and J. R. Yates, Smart Mater. Struct., 2004, 13, 49–56 CrossRef CAS.
- R. H. Baughman, J. M. Shacklette, A. A. Zakhidov and S. Stafström, Nature, 1998, 392, 362–365 CrossRef CAS.
- A. Yeganeh-Haeri, D. J. Weidner and J. B. Parise, Science, 1992, 257, 650–652 CAS.
- L. Dong, D. S. Stone and R. S. Lakes, Philos. Mag. Lett., 2010, 90, 23–33 CrossRef CAS.
- S. Hirotsu, Macromolecules, 1990, 23, 903–905 CrossRef CAS.
- S. Hirotsu, J. Chem. Phys., 1991, 94, 3949–3957 CrossRef CAS.
- C. Li, Z. Hu and Y. Li, Phys. Rev. E: Stat. Phys., Plasmas, Fluids, Relat. Interdiscip. Top., 1993, 48, 603–606 CrossRef CAS.
- K. E. Evans, M. A. Nkansah, I. J. Hutchinson and S. C. Rogers, Nature, 1991, 353, 124–124 CrossRef CAS.
- B. Brandel and R. S. Lakes, J. Mater. Sci., 2001, 36, 5885–5893 CrossRef CAS.
- R. Lakes, Science, 1987, 235, 1038–1040 CAS.
- A. C. Glavan, R. V. Martinez, A. B. Subramaniam, H. J. Yoon, R. M. D. Nunes, H. Lange, M. M. Thuo and G. M. Whitesides, Adv. Funct. Mater., 2014, 24, 60–70 CrossRef CAS.
- R. Lakes, Adv. Mater., 1993, 5, 293–296 CrossRef CAS.
- T. L. Warren, J. Appl. Phys., 1990, 67, 7591–7594 CrossRef.
- R. Lakes, Nature, 1993, 361, 511–515 CrossRef.
- Z. Y. Wei, Z. V. Guo, L. Dudte, H. Y. Liang and L. Mahadevan, Phys. Rev. Lett., 2013, 110, 215501 CrossRef CAS PubMed.
- J. N. Grima, R. Jackson, A. Alderson and K. E. Evans, Adv. Mater., 2000, 12, 1912–1918 CrossRef CAS.
- Y. Ishibashi and M. Iwata, J. Phys. Soc. Jpn., 2000, 69, 2702–2703 CrossRef CAS.
- J. Grima, A. Alderson and K. Evans, Physica Status Solidi B, 2005, 242, 561–575 CrossRef CAS.
- V. O. Özçelik, S. Cahangirov and S. Ciraci, Phys. Rev. Lett., 2014, 112, 246803 CrossRef PubMed.
- S. Zhang, J. Zhou, Q. Wang, X. Chen, Y. Kawazoe and P. Jena, Proc. Natl. Acad. Sci. U. S. A., 2015, 112, 2372–2377 CrossRef CAS PubMed.
- J.-W. Jiang and H. S. Park, Nat. Commun., 2014, 5, 4727 CAS.
- J. N. Grima, S. Winczewski, L. Mizzi, M. C. Grech, R. Cauchi, R. Gatt, D. Attard, K. W. Wojciechowski and J. Rybicki, Adv. Mater., 2015, 27, 1455–1459 CrossRef CAS PubMed.
- D. T. Ho, S.-D. Park, S.-Y. Kwon, K. Park and S. Y. Kim, Nat. Commun., 2014, 5, 3255 Search PubMed.
- L. J. Hall, V. R. Coluci, D. S. Galvão, M. E. Kozlov, M. Zhang, S. O. Dantas and R. H. Baughman, Science, 2008, 320, 504–507 CrossRef CAS PubMed.
- C. Berger, Z. Song, X. Li, X. Wu, N. Brown, C. Naud, D. Mayou, T. Li, J. Hass, A. N. Marchenkov, E. H. Conrad, P. N. First and W. A. d. Heer, Science, 2006, 312, 1191–1196 CrossRef CAS PubMed.
- A. K. Geim and K. S. Novoselov, Nat. Mater., 2007, 6, 183–191 CrossRef CAS PubMed.
- C. Lee, X. Wei, J. W. Kysar and J. Hone, Science, 2008, 321, 385–388 CrossRef CAS PubMed.
- K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, M. I. Katsnelson, I. V. Grigorieva, S. V. Dubonos and A. A. Firsov, Nature, 2005, 438, 197–200 CrossRef CAS PubMed.
- K. S. Novoselov, A. K. Geim, S. V. Morozov, D. Jiang, Y. Zhang, S. V. Dubonos, I. V. Grigorieva and A. A. Firsov, Science, 2004, 306, 666–669 CrossRef CAS PubMed.
- A. L. Walter, H. Sahin, K.-J. Jeon, A. Bostwick, S. Horzum, R. Koch, F. Speck, M. Ostler, P. Nagel, M. Merz, S. Schupler, L. Moreschini, Y. J. Chang, T. Seyller, F. M. Peeters, K. Horn and E. Rotenberg, ACS Nano, 2014, 8, 7801–7808 CrossRef CAS PubMed.
- A. L. Walter, H. Sahin, J. Kang, K.-J. Jeon, A. Bostwick, S. Horzum, L. Moreschini, Y. J. Chang, F. M. Peeters, K. Horn and E. Rotenberg, Phys. Rev. B: Condens. Matter, 2016, 93, 075439 CrossRef.
- R. J. Kashtiban, M. A. Dyson, R. R. Nair, R. Zan, S. L. Wong, Q. Ramasse, A. K. Geim, U. Bangert and J. Sloan, Nat. Commun., 2014, 5, 4902 CrossRef CAS PubMed.
- L. Li, R. Qin, H. Li, L. Yu, Q. Liu, G. Luo, Z. Gao and J. Lu, ACS Nano, 2011, 5, 2601–2610 CrossRef CAS PubMed.
- M. T. H. Şahin and S. Ciraci, Phys. Rev. B: Condens. Matter, 2011, 83, 115432 CrossRef.
- R. Stine, W.-K. Lee, K. E. Whitener, J. T. Robinson and P. E. Sheehan, Nano Lett., 2013, 13, 4311–4316 CrossRef CAS PubMed.
- W. Feng, P. Long, Y. Feng and Y. Li, Adv. Sci., 2016, 3, 1500413 CrossRef.
- W. Yu and S.-P. Gao, Surf. Sci., 2015, 635, 78–84 CrossRef CAS.
- F. Karlický, K. Kumara Ramanatha Datta, M. Otyepka and R. Zbořil, ACS Nano, 2013, 7, 6434–6464 CrossRef PubMed.
- H. Y. Liu, Z. F. Hou, C. H. Hu, Y. Yang and Z. Z. Zhu, J. Phys. Chem. C, 2012, 116, 18193–18201 CAS.
- J. E. Johns and M. C. Hersam, Acc. Chem. Res., 2012, 46, 77–86 CrossRef PubMed.
- Y. Ma, Y. Dai, M. Guo, C. Niu, L. Yu and B. Huang, Nanoscale, 2011, 3, 2301–2306 RSC.
- O. Leenaerts, H. Peelaers, A. D. Hernández-Nieves, B. Partoens and F. M. Peeters, Phys. Rev. B: Condens. Matter, 2010, 82, 195436 CrossRef.
- P. Giannozzi, S. Baroni, N. Bonini, M. Calandra, R. Car, C. Cavazzoni, D. Ceresoli, G. L. Chiarotti, M. Cococcioni, I. Dabo, A. D. Corso, S. d. Gironcoli, S. Fabris, G. Fratesi, R. Gebauer, U. Gerstmann, C. Gougoussis, A. Kokalj, M. Lazzeri, L. Martin-Samos, N. Marzari, F. Mauri, R. Mazzarello, S. Paolini, A. Pasquarello, L. Paulatto, C. Sbraccia, S. Scandolo, G. Sclauzero, A. P. Seitsonen, A. Smogunov, P. Umari and R. M. Wentzcovitch, J. Phys.: Condens. Matter, 2009, 21, 395502 CrossRef PubMed.
- S. Baroni, S. d. Gironcoli, A. D. Corso and P. Giannozzi, Rev. Mod. Phys., 2001, 73, 515–562 CrossRef CAS.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865 CrossRef CAS PubMed.
- D. Vanderbilt, Phys. Rev. B: Condens. Matter, 1990, 41, 7892–7895 CrossRef.
- K. F. Garrity, J. W. Bennett, K. M. Rabe and D. Vanderbilt, Comput. Mater. Sci., 2014, 81, 446–452 CrossRef CAS.
- H. J. Monkhorst and J. D. Pack, Phys. Rev. B: Solid State, 1976, 13, 5188–5192 CrossRef.
- R. Saito, G. Dresselhaus and M. S. Dresselhaus, Physical Properties of Carbon Nanotubes, Imperial College Press, 1998 Search PubMed.
- F. Liu, P. Ming and J. Li, Phys. Rev. B: Condens. Matter, 2007, 76, 064120 CrossRef.
- F. Ma, H. B. Zheng, Y. J. Sun, D. Yang, K. W. Xu and P. K. Chu, Appl. Phys. Lett., 2012, 101, 111904 CrossRef.
- C. A. Marianetti and H. G. Yevick, Phys. Rev. Lett., 2010, 105, 245502 CrossRef CAS PubMed.
- T. Chang and H. Gao, J. Mech. Phys. Solids, 2003, 51, 1059–1074 CrossRef CAS.
- J.-W. Jiang, T. Chang, X. Guo and H. S. Park, Nano Lett., 2016, 16, 5286–5290 CrossRef CAS PubMed.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c6nr04519g |
| ‡ These authors contributed equally to this work. |
| This journal is © The Royal Society of Chemistry 2017 |