Electronic, optical and mechanical properties of SrSi₆N₈ and SrSi₆N₈O via first-principles

Abstract

The different properties of two structurally similar nitridosilicates, SrSi₆N₈ and SrSi₆N₈O, are attributed to the oxygen atom. To explore the effects of the O atom on the properties, structural, electronic, optical and mechanical properties of SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ have been simulated via first-principles in this work. The results indicate the Si–O–Si bond is stronger than the Si–Si bond, resulting in a more stable crystal structure of SrSi₆N₈O compared with SrSi₆N₈. The calculated bandgaps (E_g) of SrSi₆N₈ and SrSi₆N₈O are 3.10 and 3.97 eV, respectively. The lower E_g of SrSi₆N₈ originates from the Si–Si σ* bond, however, the E_g can be enlarged through introducing the O atom into the Si–Si bond. The optical properties illustrate that the refractive peaks are blue-shifted with O doping into the lattice of SrSi₆N₈. Furthermore, the elastic properties show that SrSi₆N₈ and SrSi₆N₈O are comparable to traditional mechanical ceramics such as sialons. This work not only expounds the difference between SrSi₆N₈ and SrSi₆N₈O, but also gives theoretical foundation for their potential applications as optical and mechanical materials.

---

1. Introduction

Nitridosilicates doped with active ions (such as Eu²⁺ and Ce³⁺) have been widely used as phosphors in phosphor-converted light emitting diodes (pc-LEDs) in consideration of their high chemical and thermal stability in combination with low thermal quenching and high conversion efficiencies.^1–3 Compared to traditional phosphors such as orthosilicates (e.g. Sr₂SiO₄:Eu²⁺)⁴ or alkaline earth sulfides (e.g. SrCa₂S₄:Eu²⁺),⁵ nitridosilicates have more diversiform crystal structures owing to their more varied crosslinking patterns.⁶ In nitridosilicates, the N can connect up to four neighboring Si tetrahedral centers, causing a higher degree of lattice condensation to improve the chemical stability of nitride phosphors, preventing the hydrolysis reaction which often happens in phosphors with terminal or ionic O or S atoms over time.^7,8

In the last few years, research into rare earth metals activating alkaline-earth-metal nitridosilicates-based phosphors has become increasingly important, such as MSi₆N₈ (M = Sr and Ba),^9,10 MSi₇N₁₀ (M = Sr, Ba),^11,12 M₂Si₅N₈ (M = Ca, Sr and Ba),^13–15 MSiN₂ (M = Sr, Ba),¹⁶ and Ba₅Si₂N₆.¹⁷ Among the available alkaline earth nitridosilicates-based phosphors, SrSi₆N₈ is very different from the others. For example, the oxidation states of Si are +III and +IV in SrSi₆N₈, and the framework contains single Si–Si bonds in N₃Si–SiN₃,⁹ as seen in Fig. 1(a). Also, SrSi₆N₈:Eu²⁺ was reported to have blue luminescent property of 450–455 nm,^18,19 while most of nitridosilicates-based phosphors emitted yellow to red wavelengths. Besides, because of the structural similarity compared with MSi₆N₈, MSi₆N₈O (M = Ca and Ba) based material has also been reported to have excellent luminescent properties. Fig. 1(b) shows the crystal structure of SrSi₆N₈O, which can be gained by inserting one O atom into the single Si–Si bond in SrSi₆N₈.⁹ Although there is no experimental report of the preparation of pure SrSi₆N₈O, the compound of Sr₂Si_12−xAl_xN_16−xO_2+x:Eu²⁺ (x ≈ 2) has been synthesized by Z Shen.²⁰ Sr₂Si_12−xAl_xN_16−xO_2+x exhibits an isotypic structure compared with SrSi₆N₈O, therefore the analogous silicon atoms are also bridged by oxygen atoms. Also, the photoluminescence properties of Eu activating CaSi₆N₈O and BaSi₆N₈O have been studied experimentally. The reported main photoluminescent peaks are at about 500 nm for BaSi₆N₈O:Eu²⁺,^21,22 614 and 587 nm for BaSi₆N₈O:Eu³⁺,²³ and 405 nm for CaSi₆N₈O:Eu²⁺.²⁴ Furthermore, some alkaline-earth-metal nitridosilicates have been reported to have excellent mechanical properties. For instance, SrSi₇N₁₀ has a yield hardness of 16.1(5) GPa,¹¹ and CaAlSiN₃ has been considered as a relatively hard materials from first-principles simulation.²⁵ However, even SrSi₆N₈ and SrSi₆N₈O have been investigated a lot in previous works, there is almost no paper having reported their basic characteristics such as electronic, optical and mechanical properties. In order to take full advantage of the properties of SrSi₆N₈ and SrSi₆N₈O for eventual technological applications, a better understanding of their electronic, optical and mechanical properties is required. What's more, the photoluminescence peaks of intrinsic and O-replacement defective SrSi₆N₈ have been reported by Wen Yeh et al. to locate at 452 and 652 nm, respectively.²⁶ The results show that the oxygen atom has significant effect on electronic and optical properties of SrSi₆N₈. Actually, nitrogen-rich sialon materials, with partial substitutions of Si by Al and N by O, have attracted great interest owing to their excellent optical and mechanical performance.³ The ratio of O : N has the significant effect on the properties of sialon materials. So introducing the O atom into the lattice of SrSi₆N₈ may generate positive effects on electronic, optical and mechanical properties. Considering that the big difference between SrSi₆N₈ and SrSi₆N₈O is the O atom, investigating the effect of O atom on SrSi₆N₈ may also give some ideas to understand the difference in their properties.

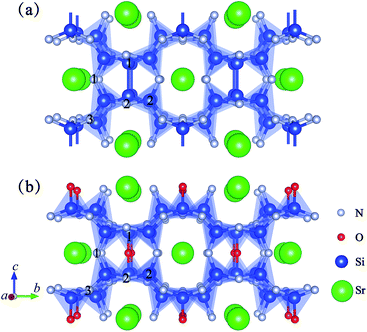Fig. 1 Projective representation of 2 × 2 × 1 supercells of (a) SrSi₆N₈ and (b) SrSi₆N₈O.

Herein, in this work, first-principles calculations^27–29 have been used to investigate the electronic, optical and mechanical properties of SrSi₆N₈, SrSi₆N₈O, and O-doped SrSi₆N₈. On the one hand, this work has given the comparison and explanation of the difference between SrSi₆N₈ and SrSi₆N₈O in electronic, optical and mechanical properties. On the other hand, the effects of O atom on SrSi₆N₈ have also been investigated through introducing O atoms to replace different N atoms and to insert into the single Si–Si bond. This work not only explains the difference between SrSi₆N₈ and SrSi₆N₈O, but also gives the theoretical foundation for their potential applications.

2. Theoretical and computational methods

Fig. 1 shows the crystal structures of 2 × 2 × 1 SrSi₆N₈ and 2 × 2 × 1 SrSi₆N₈O supercells. As seen in Fig. 1(a), there are two types of connectivity models of N atoms, which are bonded with two Si atoms (marked as N^[2]) and three Si atoms (marked as N^[3]). And the spatial locations of Si–N^[3]–Si bonds are vertical and with a certain angle to the b axis.⁹ So, we marked the N^[2] as N₁, the two N atoms in the different Si–N^[3]–Si bonds as N₂ and N₃, the Si atoms in the Si–Si bonds as Si₁, and the other Si atoms as Si₂, as seen in Fig. 1(a). Herein, four types of O-doped 2 × 2 × 1 SrSi₆N₈ supercells were considered in our work, which were O_i substituting to N_i (i = 1, 2 and 3) and O₄ inserting into the Si–Si bond, and marked as O_i–SrSi₆N₈ (i = 1, 2, 3 and 4), respectively.

All first principle calculations were performed using Cambridge Serial Total Energy Package (CASTEP) code.²⁹ The electronic exchange–correlation function was Perdew Burke Ernzerhof (PBE) of generalized gradient approximation (GGA).³⁰ Ultrasoft pseudopotentials were used with 5s² 5p⁰, 3s² 3p², 2s² 2p³ and 2s² 2p⁴ as the valence-electron configurations for Sr, Si, N and O atoms, respectively, to describe the interactions between ions and electrons.³¹ With convergence testing, the plane-wave cutoff energy of 400 eV and k-point meshes of 3 × 2 × 3 were used to ensure the accuracy and efficiency of calculations. The threshold for self-consistent field iterations was 5.0 × 10⁻⁷ eV per atom. The convergence tolerance parameters of optimized calculation were the energy of 1.0 × 10⁻⁵ eV per atom, the maximum force of 0.03 eV Å⁻¹, the maximum inner stress of 0.05 GPa and the maximum displacement of 1.0 × 10⁻⁴ nm. After finishing geometry optimizations, electronic, optical and mechanical properties of all models were calculated. The lattice stabilities of all the optimized structures were evaluated by the phonon dispersions, where the imaginary frequency was the sign of lattice instability.^32,33

Considering no experimental report of the preparation of pure SrSi₆N₈O, we used the acid–base idea as mentioned by M. Mikami³⁴ to assume the solid-state reaction for production of SrSi₆N₈O. The binary oxide is generally considered as acid while binary nitride as base. So the reaction for production of SrSi₆N₈O should be defined as following:

SrO + 2Si₃N₄ → SrSi₆N₈O (1)

The formation energy (E_form) of reaction [1] was calculated as following equation:

E_form = E_SrSi6N8O − E_SrO − 2E_Si3N4 (1)

where the E_SrSi6N8O, E_SrO and E_Si3N4 were the total energies of SrSi₆N₈O, SrO and α-Si₃N₄, respectively. A negative value of formation energy indicates the reaction is exothermic and the production is stable.

The binding energy (E_b) can be used to investigate the relative stability of the model. The value of binding energies of all models were calculated by the following formula:³⁵

where the E_tot was the total energy of the considered model, and the chemical potentials of Si, N and O were defined as μ_Si = μ_bulk−Si, μ_N = μ_N2/2 and μ_O = μ_O2/2, respectively.^36,37 The coefficients n_Si, n_N and n_O were the numbers of the Si, N and O atoms in each model. For O-doped SrSi₆N₈ models, the values of bonding energies were used to predict the preferential position for O doping in the lattice of SrSi₆N₈.

Understanding elastic properties of nitridosilicates is very important for their application and improvement. For orthorhombic crystals, it is known that the mechanical stability requires elastic constants satisfying the following conditions:^38,39

c_ij > 0 (i =1∼6),

c₁₁ + c₂₂ + c₃₃ + 2c₁₂ + 2c₁₃ + 2c₂₃ > 0,

c₁₁ + c₂₂ − 2c₁₂ > 0,

c₁₁ + c₃₃ − 2c₁₃ > 0,

c₂₂ + c₃₃ − 2c₂₃ > 0 (3)

The mechanical constants: bulk modulus (B), shear modulus (G), Young's modulus (E), and Poisson's ratio (ν) have been calculated using the following equations:^38,39

B = (1/9)(c₁₁ + c₂₂ + c₃₃ + 2c₁₂ + 2c₁₃ + 2c₂₃) (4)

G = (1/15)[(c₁₁ + c₂₂ + c₃₃ + 3(c₄₄ + c₅₅ + c₆₆) − c₁₂ − c₁₃ − c₂₃)] (5)

E = 9BG/(3B + G) (6)

ν = (1/2)(3B − 2G)/(3B + G) (7)

3. Results and discussion

3.1 Geometry optimization results

Table 1 lists the calculated lattice parameters, average bond lengths and binding energies of all models. The lattice parameters of 2 × 2 × 1 SrSi₆N₈ and 2 × 2 × 1 SrSi₆N₈O supercells are well agreement with previous results.^9,24 There is no big change in the lattice parameters after O doping into SrSi₆N₈. The slight volume expansions come from the changes of local bond lengths. The average bond lengths of Si–O^[2] and Si–O^[3] are larger than the Si–N^[2] and Si–N^[3] in O_i–SrSi₆N₈ (i = 1, 2 and 3), while the average bond length of Si–O^[2] is smaller than the Si–N^[2] in O₄–SrSi₆N₈. The results indicate that different coordination environments of O atom has different effects on the Si–O bond lengths. The E_form of pure SrSi₆N₈O calculated by eqn (1) is −0.37 eV, which indicates that the reaction [1] is somewhat exothermic and SrSi₆N₈O is stable. The lower value of E_b indicates the more stable degree of the model. The E_b (−1.71 eV) of SrSi₆N₈O means that it has the more stable crystal structure and can be easier to synthesis compared with SrSi₆N₈ (−1.40 eV). These phenomenon is corresponding to the fact that SrSi₆N₈ is a kind of reduced nitridosilicate and must be synthesized under the condition of extreme lack of oxygen.⁹ The E_b of O₄–SrSi₆N₈ (−1.45 eV) is the lowest one among all O-doped SrSi₆N₈ models, meaning that O atom prefers to insert into the single Si–Si bond to stabilize the crystal structure of SrSi₆N₈.

Table 1 Optimized lattice parameters, bond lengths and binding energies of all models

Models	Lattice parameters (Å)			Volume (Å^3)	Average bond length (Å)					Eb (eV)
	a	b	c		Si–N^[2]	Si–N^[3]	Si–Si	Si–O^[2]	Si–O^[3]
a Ref. 9.b Ref. 19.
SrSi6N8	9.664	15.854	9.331	1429.56	1.679	1.755	2.379			−1.40
SrSi6N8^a	4.801	7.855	9.260	349.21			2.352
SrSi6N8^b	4.803	7.836	9.261	350.93
O1–SrSi6N8	9.682	15.897	9.302	1431.80	1.680	1.757	2.367	1.688		−1.43
O2–SrSi6N8	9.679	15.869	9.331	1433.13	1.678	1.757	2.370		1.850	−1.42
O3–SrSi6N8	9.670	15.890	9.320	1432.10	1.679	1.757	2.370		1.811	−1.42
O4–SrSi6N8	9.673	15.939	9.400	1449.29	1.681	1.756	2.406	1.589		−1.45
SrSi6N8O	9.722	16.400	9.706	1547.62	1.683	1.752		1.620		−1.71
SrSi6N8O^a	4.865	8.206	9.721

---

Furthermore, phonon dispersions of all optimized models have been calculated to evaluate the structural stability, as seen in Fig. 2. The results shows that there is no imaginary frequency of pure SrSi₆N₈ and SrSi₆N₈O, which means that SrSi₆N₈ and SrSi₆N₈O are structurally stable. The lowest frequencies of O_i–SrSi₆N₈ (i = 1, 2, 3 and 4) are −0.20, −0.81, −0.53 and −0.05 THz, respectively. According to the previous works, the small negative frequencies of O₁–SrSi₆N₈ and O₄–SrSi₆N₈ could be ignored because the small negative frequencies may be caused by the relatively smaller supercells and lower computational accuracies.^32,33 However, the calculation of phonon dispersion is time consuming with a bigger supercell and higher computational accuracy. So we think it is reasonable to consider O₁–SrSi₆N₈ and O₄–SrSi₆N₈ as structurally stable models based on the calculated phonon dispersions. While, the structures of O₂–SrSi₆N₈ and O₃–SrSi₆N₈ are metastable or unstable according to their obvious imaginary frequencies as seen in Fig. 2(d) and (e). The results of phonon dispersions are well agreement with the results of bonding energies (E_b), which means that the structures of O₁–SrSi₆N₈ and O₄–SrSi₆N₈ are more stable than O₂–SrSi₆N₈ and O₃–SrSi₆N₈.

Fig. 2 Phonon dispersion curves (a) SrSi₆N₈, (b) SrSi₆N₈O, (c) O₁–SrSi₆N₈, (d) O₂–SrSi₆N₈, (e) O₃–SrSi₆N₈ and (f) O₄–SrSi₆N₈.

3.2 Electronic properties

Fig. 3 shows the band structures and partial density of states (PDOS) of SrSi₆N₈ and SrSi₆N₈O. The calculated bandgaps (E_g) of SrSi₆N₈ and SrSi₆N₈O are 3.10 and 3.97 eV, respectively. Although, there is no experimentally reported the E_g of SrSi₆N₈ or SrSi₆N₈O, our GGA calculated E_g are expected to be smaller than experimental values since the underestimation of GGA calculation.³⁹ While the effects of oxygen on electronic properties of SrSi₆N₈ can be expounded through the relative changes. The top of valence bands of SrSi₆N₈ and SrSi₆N₈O are occupied by N 2p states. Differing in band structures from the other alkaline-earth-metal nitridosilicates,⁶ there are some energy levels below the conduction bands of SrSi₆N₈, which are mainly constituted of Si 3p states as seen from the PDOS. Furthermore, the 3p states of Si₁ contribute the more parts of the bottom of conduction bands compared to Si₂, which indicates that the energy levels below the conduction bands are attributed to the single Si–Si bond. The previous works reported that Si–Si bond forms an empty σ* state under the bottom of conduction bands in some nitrides.^40,41 Therefore, the Si–Si σ* state is the main reason for the lower bandgap of SrSi₆N₈ compared to SrSi₆N₈O.

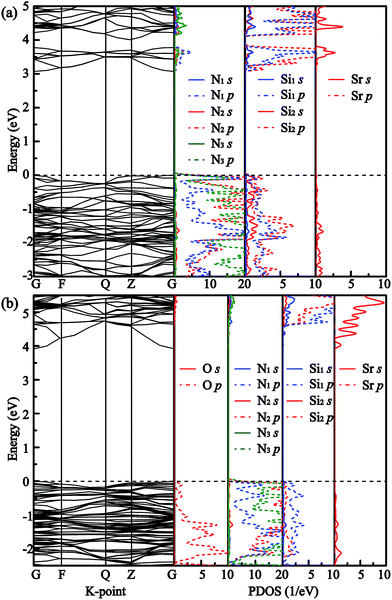Fig. 3 Band structures and partial density of states (PDOS) of (a) SrSi₆N₈ and (b) SrSi₆N₈O.

Furthermore, the electron density distribution (EDD) plots are calculated to investigate the difference between Si–Si and Si–O–Si bonds. Fig. 4(a) and (b) illustrate the partial atom distributions and corresponding EDD plots on the (010) plane of SrSi₆N₈ and SrSi₆N₈O, respectively. We can find that there is a small amount of electron density distributing in the center of Si–Si bond in SrSi₆N₈, while a high density of electron gathers on the O atoms in SrSi₆N₈O. The stronger electronic interaction of Si–O–Si bond gives the more stable crystal structure for SrSi₆N₈O, which is consistent with results of the calculated E_b. Besides, the electron densities of O atoms are obviously higher than that of N atoms in SrSi₆N₈O, which is attributed to the stronger electronegativity of O atom.

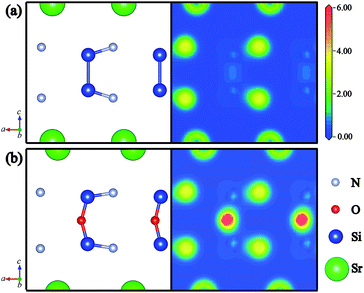Fig. 4 Partial atom distributions and corresponding electron density distribution plots of (a) SrSi₆N₈ and (b) SrSi₆N₈O on the (010) plane.

The calculated band structures and PDOS of the O atoms and its adjacent Si atoms for all O-doped SrSi₆N₈ models are plotted in Fig. 5. The calculated bandgaps (E_g) of O_i–SrSi₆N₈ (i = 1, 2, 3 and 4) are 3.17, 2.84, 3.09 and 3.33 eV, respectively. For O₁–SrSi₆N₈, as seen in Fig. 5(a), the band structure of O₁–SrSi₆N₈ is very close to that of undoped SrSi₆N₈, which indicates that the O^[2] has the similar properties of N^[2]. As seen in Fig. 5(b) and (c), the reason of the E_g decreasing for O₂–SrSi₆N₈ and O₃–SrSi₆N₈ is that the hybrid Si–O bonds generate energy levels under the conduction bands. For O₄–SrSi₆N₈, the distribution of O 2p states are very similar to that of SrSi₆N₈O, showing that the O₄ atom has the same character to the O atoms in SrSi₆N₈O. The E_g of O₄–SrSi₆N₈ is larger than that of SrSi₆N₈, which indicates that inserting O atom into the single Si–Si bond to decrease the concentration of Si–Si bonds can increase the E_g.

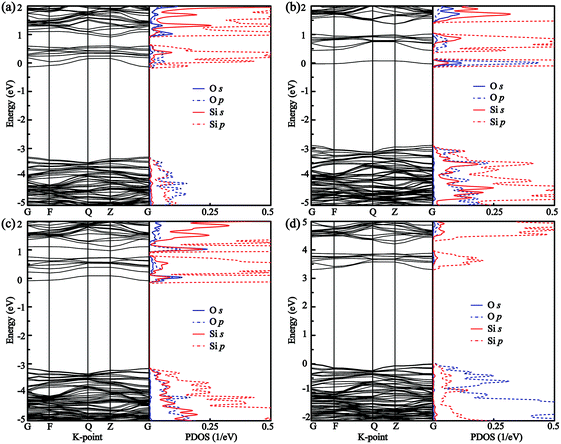Fig. 5 Band structures and PDOS of (a) O₁–SrSi₆N₈, (b) O₂–SrSi₆N₈, (c) O₃–SrSi₆N₈, and (d) O₄–SrSi₆N₈.

3.3 Optical properties

The absorption coefficient is one of key parameters to evaluate the performance for phosphors. The absorption spectra can be described in terms of time-dependent perturbations of the ground-state electronic states, which has always been investigated combined the electron transition between valance bonds and conduction bonds.⁴² Fig. 6 shows the calculated absorption spectra of all models. The absorption peak for undoped SrSi₆N₈ is located at about 3.0–15.0 eV, which are corresponding to the electron transitions from N 2p states in the valence bands to Si 3p and Sr 5s states in the conduction bands as shown from PDOS in Fig. 3(a). The absorption edge of SrSi₆N₈O starts at 3.9 eV, with a large blue shift compared to that of SrSi₆N₈ at 2.9 eV. The positions of the main absorption peaks for O_i–SrSi₆N₈ (i = 1, 2, 3 and 4) are almost the same as SrSi₆N₈, while the absorption intensities become lower. Besides, as show in the enlarged view in Fig. 5, the weak peaks located around 0–3.0 eV of the O₂–SrSi₆N₈ and O₃–SrSi₆N₈ derive from the electron transitions between Si–O hybrid states under the bottom of conduction bands and conduction bands, as shown in Fig. 5(b) and (c).

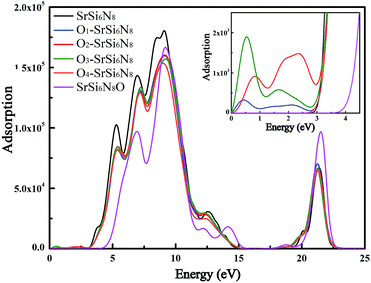Fig. 6 Calculated absorption spectra of SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ models.

Fig. 7 shows the refractive spectra of SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ models. Although, there is no experimentally reported refractive index (n) of SrSi₆N₈ or SrSi₆N₈O, the static refractive indexes (n₀) of SrSi₆N₈ (1.67) and SrSi₆N₈O (1.39) are expected to be lower than the experimental value due to the underestimated calculation of GGA-PEB.³⁹ Nevertheless, the relative changes can reflect the effects of O atoms on SrSi₆N₈. The calculated static refractive indexes of O_i–SrSi₆N₈ (i = 1, 2 and 3 and 4) are 1.65, 2.08, 1.69 and 1.56, respectively, which are well corresponding to Moss rule that the static refractive index is inversely proportional to the E_g.⁴³ Except for the static refractive indexes, the main peaks of refractive indexes of all O-doped SrSi₆N₈ models are lower than that of SrSi₆N₈. Furthermore, compared with SrSi₆N₈, the locations of main peaks for SrSi₆N₈O are significantly blue-shift. The results of optical properties show that different doping sites of O atoms in SrSi₆N₈ have different effects on its basic absorption and refractive spectra.

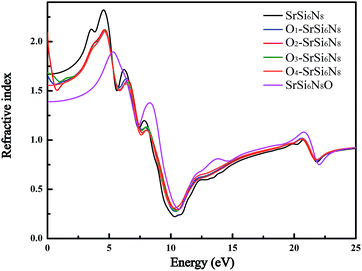Fig. 7 Calculated refractive spectra of SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ models.

3.4 Mechanical properties

The optimized structures of all models have been applied to calculate the elastic tensors which consist of nine independent components, as tabulated in Table 2. It can be seen that the relationships of elastic constants satisfy the conditions as shown in eqn (3), which indicates that SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ are mechanically stable.

Table 2 Calculated elastic tensor C_ij (GPa) for all models

Models	C11	C22	C33	C44	C55	C66	C12	C13	C23
SrSi6N8	380.08	308.27	341.91	94.79	81.51	131.64	91.21	84.21	101.08
O1–SrSi6N8	272.6	288.96	349.77	76.14	120.84	85.98	88.23	68.13	65.22
O2–SrSi6N8	326.66	320.01	376.75	89.42	148.04	96.83	102.69	95.72	77.35
O3–SrSi6N8	308.17	332.72	378.19	83.16	133.37	96.20	100.24	94.78	79.93
O4–SrSi6N8	299.77	320.38	371.85	81.32	135.48	96.61	99.14	96.71	77.35
SrSi6N8O	324.25	377.85	374.25	92.30	67.54	142.86	85.77	71.16	119.8

---

Furthermore, the mechanical constants: bulk modulus (B), shear modulus (G), Young's modulus (E), and Poisson's ratio (ν) for all models are listed in Table 3. The mechanical constants: B, G and E of SrSi₆N₈O are larger than that of SrSi₆N₈, which mainly attributes to the more stable Si–O–Si bond than Si–Si bond. Though introducing O atom produces a certain degree of lattice distortion in SrSi₆N₈, there is no obvious deterioration on mechanical properties of O-doped SrSi₆N₈ compared to undoped SrSi₆N₈, even some mechanical constants are improved. Besides, with the comparison of traditionally mechanical ceramics such as sialons (B = 170–240 GPa, G = 118.84 GPa and E = 197–283 GPa),^44,45 the mechanical properties of all models considered in this work indicate that they have the application prospect for mechanical ceramics.

Table 3 Calculated bulk modulus B (GPa), shear modulus G (GPa), Young's modulus E (GPa) and Poisson's ratio ν for all models

Models	B	G	E	ν
SrSi6N8	175.92	111.84	276.85	0.24
O1–SrSi6N8	150.5	102.58	250.77	0.22
O2–SrSi6N8	174.99	116.7	286.43	0.23
O3–SrSi6N8	174.33	112.15	277.04	0.24
O4–SrSi6N8	170.93	100.6	252.30	0.25
SrSi6N8O	181.09	113.85	282.37	0.24

---

4. Conclusions

In this work, first-principles simulation has been performed to investigate the structural, electronic, optical and mechanical properties of SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈. Firstly, this work has given the comparison and explanation of the difference between the two structurally similar nitridosilicates SrSi₆N₈ and SrSi₆N₈O. The results show that SrSi₆N₈O is more stable than SrSi₆N₈, and the E_g of SrSi₆N₈O is larger than that of SrSi₆N₈. The key factor of the difference between SrSi₆N₈ and SrSi₆N₈O is the O atom. Namely, the Si–O–Si bond is stronger than the single Si–Si bond, which contributes to the more stable crystal structure of SrSi₆N₈O. And the Si–Si σ* states under the conduction bonds are the main reason for the smaller E_g of SrSi₆N₈ compared to SrSi₆N₈O.

Secondly, the effects of O on electronic, optical and mechanical properties of SrSi₆N₈ have been investigated systematically. The crystal structure of SrSi₆N₈ can be stabilized with doping O into the lattice and the most preferential doping position of O is insertion between the single Si–Si bonds. And introducing O into the Si–Si bond can significantly increase the E_g of SrSi₆N₈. The static refractive indexes become larger for O substituting to N models while become smaller for the O insert into the Si–Si bond. Besides, the calculated mechanical constants are comparable to traditionally mechanical ceramics such as sialons, which indicates SrSi₆N₈, SrSi₆N₈O and O-doped SrSi₆N₈ have potential applications as mechanical materials. This work is helpful to understand the difference between SrSi₆N₈ and SrSi₆N₈O, and opens up their possibility of new application.

Acknowledgements

The project is supported by the National Natural Science Foundation of China (51202171, 51472188 and 51521001), the Specialized Research Fund for the Doctoral Program of Higher Education of China (20120143120004), the “111” project (B13035), the Fundamental Research Funds for the Central Universities (WUT: 2016-YB-003) and the China Scholarship Council (CSC).

Notes and references

1. R. J. Xie and N. Hirosaki, Sci. Technol. Adv. Mater., 2007, 8, 588–600.
2. S. Ye, F. Xiao, Y. X. Pan, Y. Y. Ma and Q. Y. Zhang, Mater. Sci. Eng., R, 2010, 71, 1–34.
3. M. Zeuner, S. Pagano and W. Schnick, Angew. Chem., Int. Ed., 2011, 50, 7754–7775.
4. N. Lakshminarasimhan and U. V. Varadaraju, J. Electrochem. Soc., 2005, 152, H152–H156.
5. S. M. Jacobsen, J. Soc. Inf. Disp., 1996, 4, 331–335.
6. R. J. Xie, Y. Q. Li, N. Hirosaki and H. Yamamoto, Nitride Phosphors and Solid-State Lighting, Taylor & Francis, 2011.
7. P. Schmidt, A. Tuecks, J. Meyer, H. Bechtel, D. Wiechert, R. Mueller-Mach, G. Mueller and W. Schnick, 7th Inter. Conf. Solid State Lighting, 2007.
8. H. Huppertz and W. Schnick, Z. Anorg. Allg. Chem., 1997, 623, 212.
9. F. Stadler, O. Oeckler, J. Senker, H. A. Höppe, P. Kroll and W. Schnick, Angew. Chem., Int. Ed., 2005, 117, 573–576.
10. F. Stadler and W. Schnick, Z. Anorg. Allg. Chem., 2007, 633, 589–592.
11. G. Pilet, H. A. Höppe, W. Schnick and S. Esmaeilzadeh, Solid State Sci., 2005, 7, 391.
12. Y. Q. Li, A. C. A. Delsing, R. Metslaara, G. de Witha and H. T. Hintzen, J. Alloys Compd., 2009, 487, 28.
13. M. Zeuner, F. Hintze and W. Schnick, Chem. Mater., 2009, 21, 336.
14. K. S. Sohn, B. Lee, R. J. Xie and N. Hirosaki, Opt. Lett., 2009, 34, 3427.
15. H. Nersisyan, H. I. Won and C. W. Won, Chem. Commun., 2011, 47, 11897.
16. C. J. Duan, X. J. Wang, W. M. Otten, A. C. A. Delsing, J. T. Zhao and H. T. Hintzen, Chem. Mater., 2008, 20, 1597.
17. H. Yamane and F. J. DiSalvo, J. Alloys Compd., 1996, 240, 33–36.
18. K. Shioi, N. Hirosaki, R. J. Xie, T. Takeda and Y. Li, J. Mater. Sci., 2008, 43, 5659–5661.
19. S. M. Wu, H. E. Hung, C. H. Hsieh, Y. C. Lin, L. C. Wang, Y. T. Tsai, C. C. Lin and R. S. Liu, J. Am. Ceram. Soc., 2015, 98, 2662–2669.
20. Z. Shen, J. Grins, S. Esmaeilzadeh and H. Ehrenberg, J. Mater. Chem., 1999, 9, 1019–1022.
21. N. Karkada, D. Porob, N. K. Prasanth and A. Setlur, ECS Trans., 2009, 16, 41–50.
22. R. J. Xie, N. Hirosaki, Y. Li and T. Takeda, J. Lumin., 2010, 130, 266–269.
23. S. A. Fartode, B. S. Reddy, V. Nayar and S. J. Dhoble, Ferroelectr., Lett. Sect., 2016, 43, 34–51.
24. Y. H. Song, T. Y. Choi, Y. Y. Luo, K. Senthil and D. H. Yoon, Opt. Mater., 2011, 33, 989–991.
25. Z. Wang, B. Shen, F. Dong, S. Wang and W. S. Su, Phys. Chem. Chem. Phys., 2015, 17, 15065–15070.
26. C. W. Yeh, Y. P. Liu, Z. R. Xiao, Y. K. Wang, S. F. Hu and R. S. Liu, J. Mater. Chem., 2012, 22, 5828–5834.
27. G. Kresse and D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758.
28. Q. Peng, X. J. Chen, S. Liu and S. De, RSC Adv., 2013, 3, 7083–7092.
29. M. D. Segall, P. J. Lindan, M. A. Probert, C. J. Pickard, P. J. Hasnip, S. J. Clark and M. C. Payne, J. Phys.: Condens. Matter, 2002, 14, 2717.
30. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865.
31. M. Marlo and V. Milman, Phys. Rev. B: Condens. Matter Mater. Phys., 2000, 62, 2899.
32. L. Zhu, L. Li, T. Cheng and D. Xu, J. Mater. Chem. A, 2015, 3, 5449–5456.
33. S. S. Bhat, U. V. Waghmare and U. Ramamurty, Cryst. Growth Des., 2014, 14, 3131–3141.
34. M. Mikami, ECS J. Solid State Sci. Technol., 2013, 2, R3048–R3058.
35. S. Yang, D. Li, T. Zhang, Z. Tao and J. Chen, J. Phys. Chem. C, 2011, 116, 1307–1312.
36. F. Oba, K. Tatsumi, I. Tanaka and H. Adachi, J. Am. Ceram. Soc., 2002, 85, 97–100.
37. K. Yang, Y. Dai and B. Huang, J. Phys. Chem. C, 2007, 111, 12086–12090.
38. Z. Wu, X. Hao, X. Liu and J. Meng, Phys. Rev. B: Condens. Matter Mater. Phys., 2007, 75, 054115.
39. B. Tu, H. Wang, X. Liu, W. Wang and Z. Fu, J. Am. Ceram. Soc., 2014, 97, 2996–3003.
40. L. E. Hintzsche, C. M. Fang, M. Marsman, G. Jordan, M. W. P. E. Lamers, A. W. Weeber and G. Kresse, Phys. Rev. B: Condens. Matter Mater. Phys., 2013, 88, 155204.
41. Z. Huang, F. Chen, Q. Shen and L. Zhang, RSC Adv., 2016, 6, 7568–7574.
42. R. Asahi, Y. Taga, W. Mannstadt and A. J. Freeman, Phys. Rev. B: Condens. Matter Mater. Phys., 2000, 61, 7459.
43. T. S. Moss, Phys. Status Solidi B, 1985, 131, 415–427.
44. J. Hafner, Z. Lenčéš and P. Šajgalík, J. Am. Ceram. Soc., 2003, 86, 1162–1167.
45. L. Benco, J. Hafner, Z. Lences and P. Sajgalik, J. Eur. Ceram. Soc., 2008, 28, 995–1002.

---